2. Analytical Approaches
For this type of transient viscous fluid with sufficiently small Reynolds numbers, the convection along with the turbulent effects can be ignored. Moreover, utilizing the superposition principle applicable to any linear system, we can easily separate the transient viscous effects from the steady viscous effects. In a separate study on water hammering, we will also demonstrate that a weak shock, the so-called pressure wave or acoustic signals can also be carried through the fluid assumed to be a compressible or nearly incompressible medium [
17]. Moreover, the steady viscous flow due to the pressure differential yields the so-called Poiseuille flow [
8]. This seemingly different physical phenomenon from the viscous effects can be in superposition on top of the transient viscous fluid model within the overall assumption of linear systems attributed to a sufficiently small Reynolds number. Of course, to the other extreme, different from incompressible, compressible, or almost compressible viscous fluid models, rich and elaborated studies have been conducted for the so-called inviscid and irrotational, or simply ideal or potential fluid [
18,
19].
For validation purposes, in this paper, typical water, with the kinematic viscosity
at 20 °C, around
or
is employed as the internal Newtonian fluid within the pipe. For an averaged velocity
in the range of
within the pipe with a diameter of 5 mm, the so-called Reynolds number computed as
is around 5, which is much smaller than 2000, a threshold for pipe turbulence with various levels of surface roughness. Although the Reynolds number is a clear indication of the quasi-static nature of the Poiseuille flow within the flow region, in order to have some guidance with respect to the selection of the sampling time in the experimental measurements of the pressure and the displacement within the fluid delivery system, in engineering practices, we must further investigate the inertia effects and other time-dependent issues. Again, for this type of transient viscous fluid with sufficiently small Reynolds numbers, we can ignore the convection along with the turbulent effects. Consider the overall governing equation for the viscous flow within the axisymmetric pipe region
with an axial pressure gradient in
x direction, namely,
as expressed as
where the dynamic viscosity is
, the density is
, the pipe length is
L, and the axial direction or
x direction pressure gradient
is expressed as
.
Note that the pipe flow direction is in the positive
x direction, i.e., from the left to the right, the pressure difference
is positive when the left-side pressure is higher than the right-side pressure, which is consistent with common sense physical understanding, namely, the fluid flows from the high pressure area to the low pressure area, a concept similar to that of the Second Law of Thermodynamics, namely, the thermal energy or heat propagates from the high temperature region to the low temperature region. Utilizing the Poiseuille flow velocity profile
and separating the transient viscous effects denoted as
in the internal laminar flow with the superposition principle, the final unsteady velocity profile
can be written as
Hence, as a simplified version of the Navier-Stokes equation in the cylindrical coordinate system, the steady Poiseuille flow solution due to a pressure difference is governed by the following equation:
with the radial coordinate
r between 0 and
R.
From Equation (
3), we derive
where
and
can be decided based on the boundary conditions.
Moreover, with the finite velocity at the center of the pipe, namely, at
,
is finite; thus we must have
Finally, utilizing the kinematic conditions on the surface of the pipe interior, i.e., at
, we have
; thus for the steady Poiseuille flow, the velocity profile within the pipe region can be expressed as
where at
and
, both velocity boundary conditions are satisfied.
Employing Equation (
5), we can easily establish the volume flow rate
within the circular cross-sectional region as
Hence, the average axial velocity
can be expressed as
Here, according to the Moody’s diagram [
8], which matches with the steady volume flow rate solution as derived in Equation (
6), for laminar flow, using the so-called head loss
and the major friction loss
, in which the Reynolds number
is expressed as
with the averaged axial velocity
defined as the ratio between the total volume flow rate
and the cross-sectional area
A, namely,
, or
, the kinematic viscosity
defined as the ratio between the dynamic viscosity
and the density
, the pressure drop can be expressed as
As a consequence, the viscous shear force acting on the pipe surface in the flow direction, namely, from the left to the right, can be calculated as
which matches with the force equilibrium for a typical control volume over the pipe with a length
L and a radius
Notice that if the fluid flow is from the left to the right in the positive
x direction, the viscous force exerted on the inner surface of the pipe can be expressed as in Equation (
9), which is equivalent to the entire inlet and outlet pressure difference
multiplied by the cross section area
For the precision control of the glue delivery in EV manufacturing plants, due to the highly viscous nature of these non-Newtonian fluids, the initial pressure impulse can be as high as a few hundred bars, which can easily surpass 2000 to 4000 psi. For a typical tube diameter of 5 mm or around 0.2 inch, the cross section area is around 19.635
or 0.030434
, the force exerted on the structure due to the fluid viscosity could easily reach
to
Furthermore, the governing equation for the transient part
is expressed as
with the boundary condition
with
R as the pipe radius.
Using the separation of variable method and common special functions [
19], we introduce
. As a consequence, we have
and the following governing equations
where
is the kinematic viscosity and the time scale
is also called the relaxation time.
For an exponentially decreasing function expressed as
, the tangent line at the origin always provides a horizontal intercept
, and in general, within 5 and 6 times the relaxation time
, the function is considered sufficiently close to the steady solution. From Equations (
11) and (
12), we have
along with the expression for the characteristic function with constants
and
A,
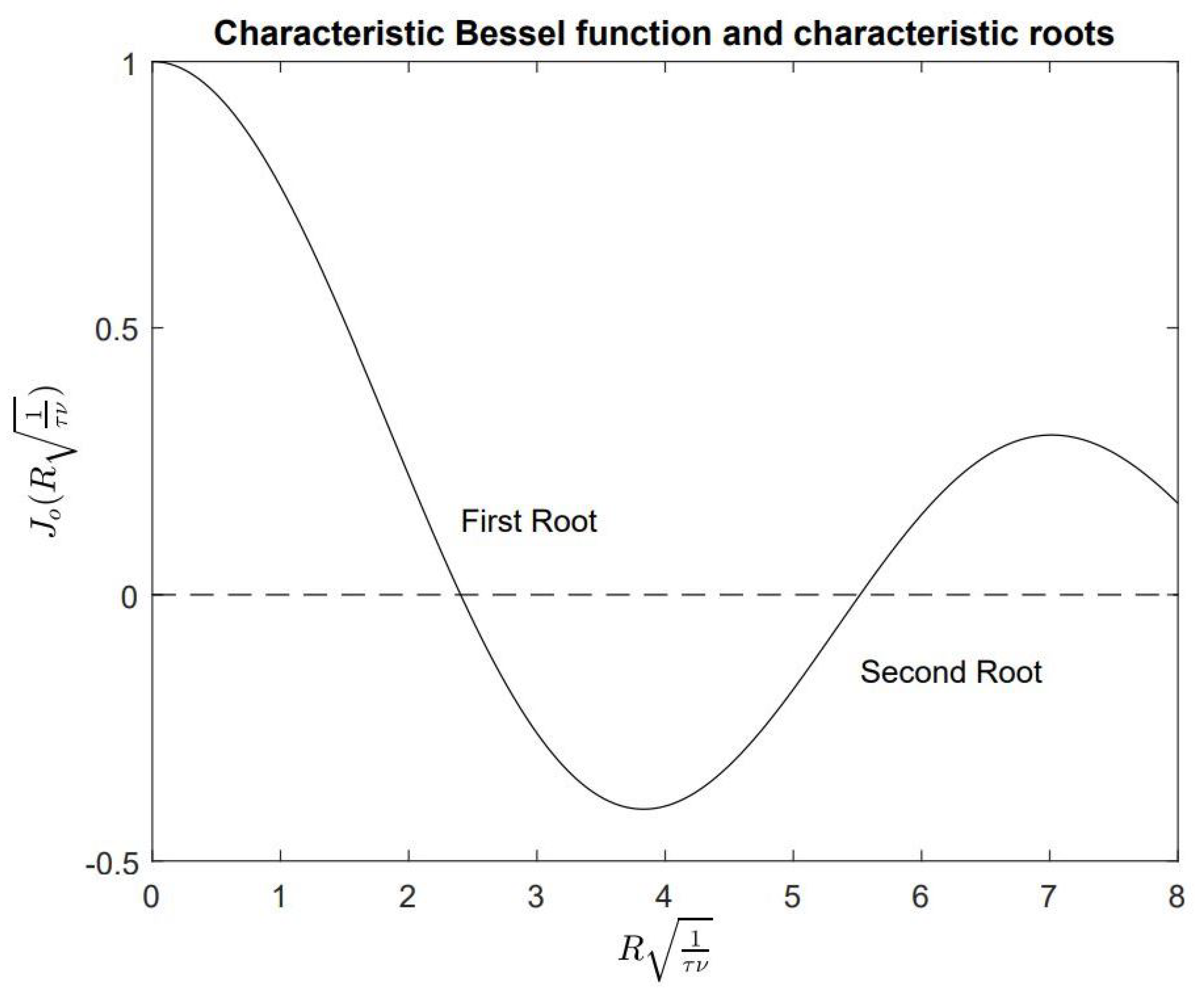
which is based on the Bessel function of the first kind satisfying the physical assumption of a finite velocity profile within the center of the pipe, and the characteristic time
defined as the relaxation time in Equation (
11) is determined by the boundary condition of
at
namely,
.
Therefore, in order for Equations (
11) and (
12) to have nontrivial solutions, we must have nontrivial or nonzero solutions of
A for the corresponding equation
Finally, according to Ref. [
4], combining the steady and the transient solutions, the complete velocity profile can be expressed as
and the coefficient
is calculated as
Again, in this paper, for initial validation, water is employed as a reference Newtonian fluid with a dynamic viscosity
of
, which is equivalent to
and the corresponding kinematic viscosity
is
, which is equivalent to
or
. Thus, the first root, based on Equation (
14) and depicted in
Figure 1, is around
and the second root is
With the realistic dimensions for the actual fluid dispensing system, namely, the pipe diameter
and the pipe length
, the first two relaxation times
and
can be evaluated as 1.0806 s and 0.2051 s, respectively. In general, the higher the dynamic viscosity
, the lower the flow rate
for the same pressure drop. In fact, for viscous incompressible internal fluid, with a time-dependent pressure applied to the inlet surface and a constant pressure, or ambient pressure, applied to the outlet surface, we can easily establish the transient to steady flow characteristics with the concept of the relaxation time [
20,
21]. Thus, the smaller the kinematic viscosity, the longer the relaxation time. For transient viscous internal fluid, another set of boundary conditions, namely, a constant volume flow rate or average axial flow velocity with a constant pressure at the outlet, might even trigger more studies and discussions of compressible or almost incompressible fluid with pressure pulse and pressure wave propagation within the pipe, the subject of which will be elaborated in a separate paper. Again, our attention in this paper is focused on the relaxation time for Newtonian fluids and non-Newtonian fluids, with respect to a combination of analytical and computational approaches.
Notice that in comparison with the Newtonian fluid example, in this case, water, the non-Newtonian fluids of interest to us have a similar density, yet the dynamic viscosity is often four million times larger than that of water. Hence, for the same diameter of the pipe, the largest characteristic time or relaxation time could approach a microsecond depending on the flow rate, or rather, the inlet and outlet pressure drop. Nevertheless, in comparison with water, the transient response of these non-Newtonian fluids is much more instantaneous. The relaxation time is visible only when the simulation time step is sufficiently small.
For the pipe’s steady delivery of Newtonian fluid, theoretical solutions as documented in Moody’s chart will be sufficient for the calculation of pressure drop or head loss at different volume flow rate or average axial velocity. Furthermore, we must be aware of the derivation of Equation (
1) from the full-fledged Navier-Stokes equations and also identify whether or not the fluid flow is in the turbulent region as well as the transient effects [
22,
23]. In this study, the precision controls of the intermittent delivery of non-Newtonian fluids depend on a better understanding of the relaxation time, transient behaviors, and the inlet and outlet pressure drops, as well as the volume flow rates with the consideration of nonlinear rheological fluid properties.
3. Computational Approaches
Unlike the purely analytical study of the mixing jet trajectory and the purely computational study of the turbulent mixing jets published earlier by the author [
24,
25], in this paper, in order to confirm our theoretical predictions with Bessel functions in cylindrical coordinate systems, we have employed the computational fluid dynamics (CFD) feature of the commercial computational mechanics code (ADINA AUI 23 from Bentley Systems) to model the flow within a circular region with various prescribed pressure differentials and ramp sizes [
26]. We consider here the transient laminar flow of a homogeneous, viscous, incompressible fluid with constant properties, and obtain the following governing equations from the mass and linear momentum conservation equations:
where
,
, and
stand for fluid mass density, fluid flow velocity in direction
, and stress tensor, respectively.
For Newtonian fluids, the stress tensor
can be expressed as
, where
stands for the dynamic viscosity, in fluid mechanics, the respective kinematic viscosity is also defined as
, and the shear stress tensor
can be denoted as
For non-Newtonian fluids, in this paper, for the shear-thinning fluids of interest to us, we employ a power law model in which the equivalent dynamic viscosity
can be expressed as
where
A and
a, in some literatures, also expressed as
, are constants and
is the effective deformation rate or shear rate defined as
In this study, the polymers of interest have a clear display of shear thinning effects, namely,
or
, as documented in Refs. [
27,
28]. The governing equations in Equation (
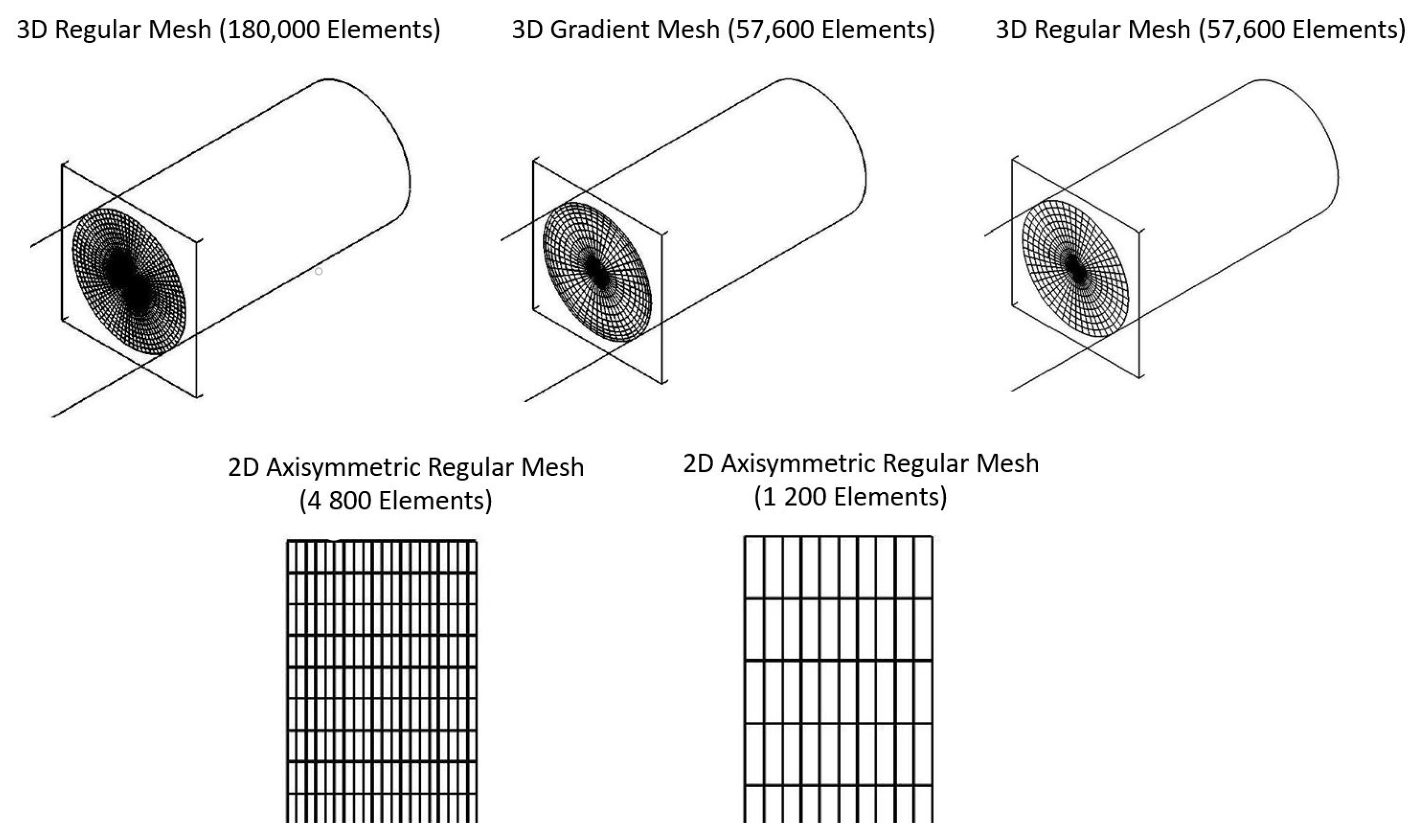
17) are implemented in the ADINA-F, Star-CCM, Solidworks Flow Simulations, and ANSYS Fluent programs. In this paper, ADINA AUI 23 from Bentley Systems is employed for both three-dimensional and two-dimensional axisymmetric models which are computed with dimensions close to the actual fluid dispenser utilized in EV manufacturing plants; for instance, the length 0.1 m and the diameter 5 mm. As illustrated in
Figure 2, the full-fledged 3D CFD model has 181,351 nodes and 180,000 elements, while the 3D CFD coarse meshes with and without a mesh gradient have 58,201 nodes and 57,600 elements, respectively. The gradient mesh has a length ratio of 0.2. Moreover, the coarse 2D axisymmetric CFD model has 1331 nodes and 1200 elements, whereas the dense 2D axisymmetric CFD model has 5061 nodes and 4800 elements. In this study, all CFD simulations have been carried out on a desktop with an Intel(R) Xeon(R) E-2124G 64-bit CPU at 3.4 GHz with 32 GB RAM (Intel, Santa Clara, CA, USA). For the reference Newtonian fluid, namely, water, the fluid kinematic viscosity
is
, and the fluid density is
. The total cross-sectional area estimate
utilized in the three-dimensional CFD model is
in comparison with the analytical solution of the pipe cross-sectional area
A, namely,
, or
. It is clear that the computational domain, discretized with finite elements, is fairly close to the mathematical domain. Furthermore, the average axial velocity
is calculated as
with the pressure differential
as
.
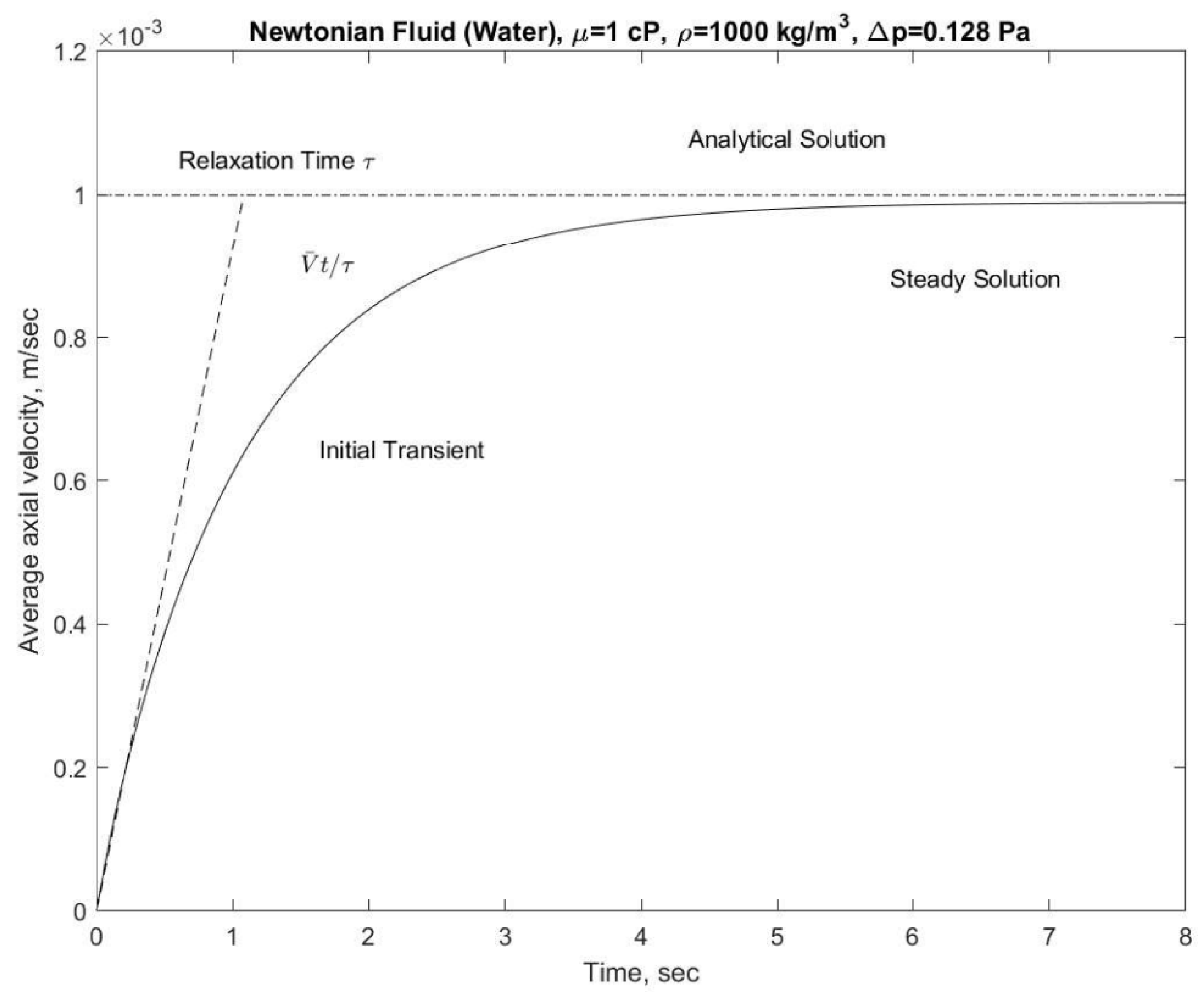
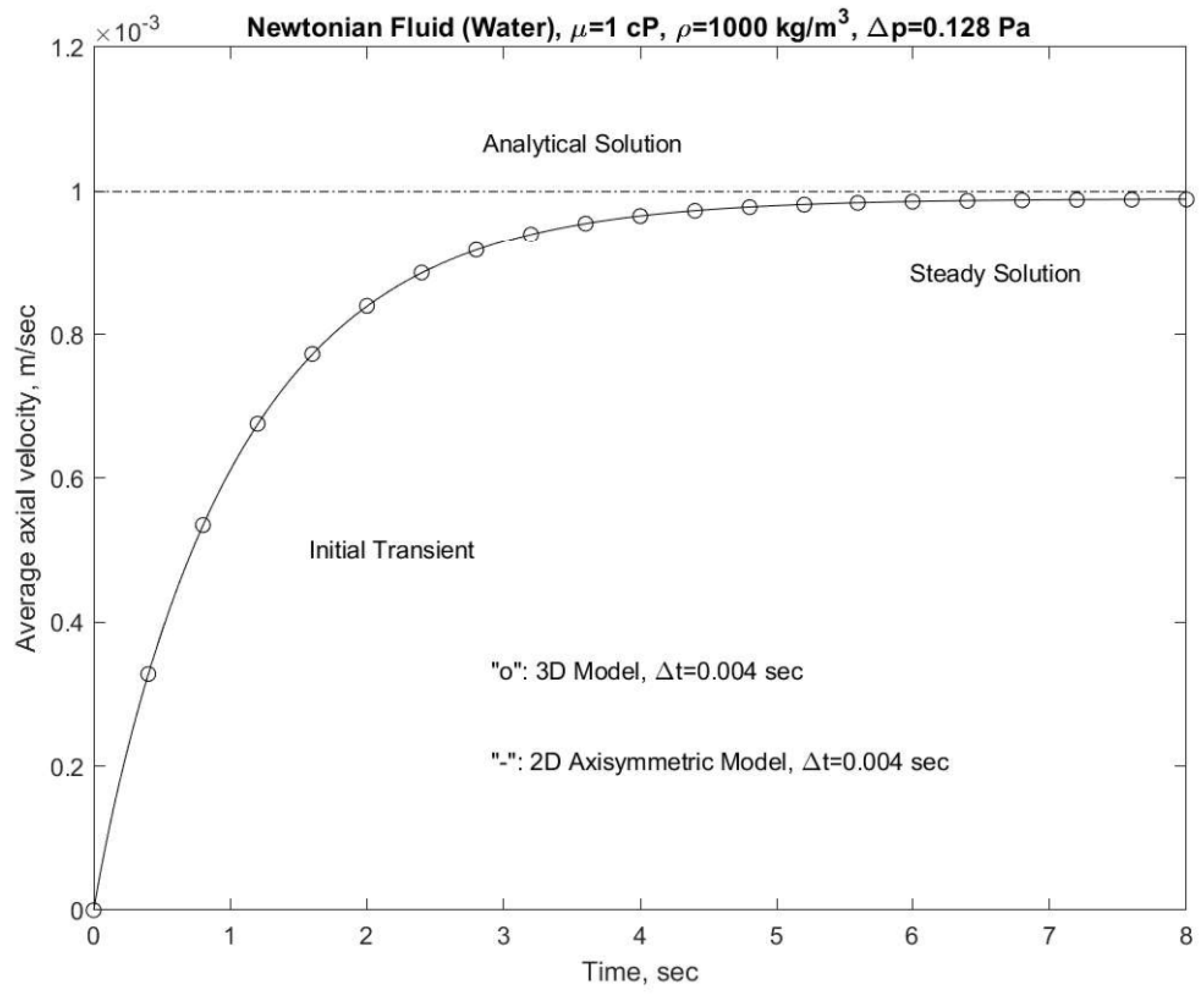
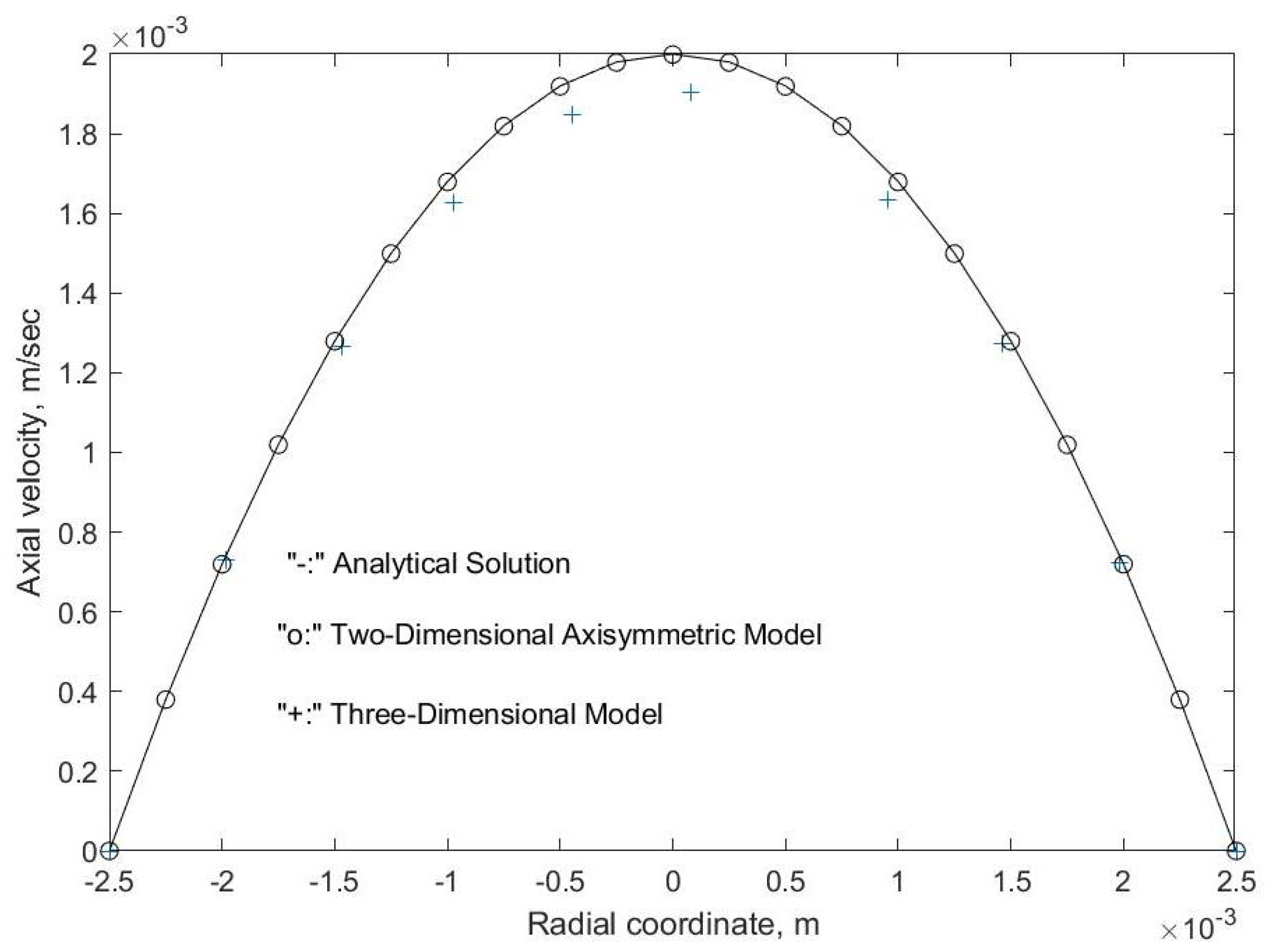
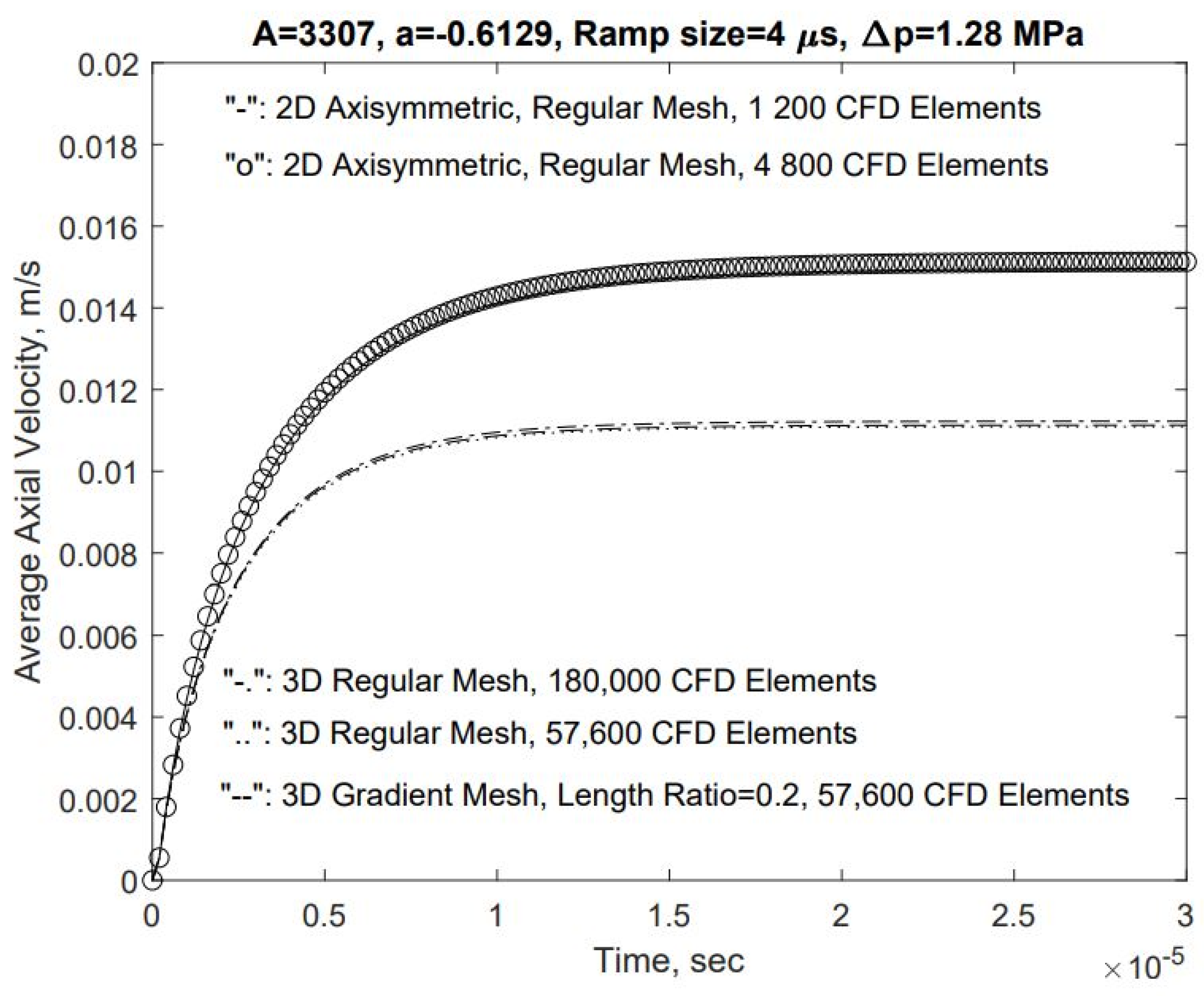
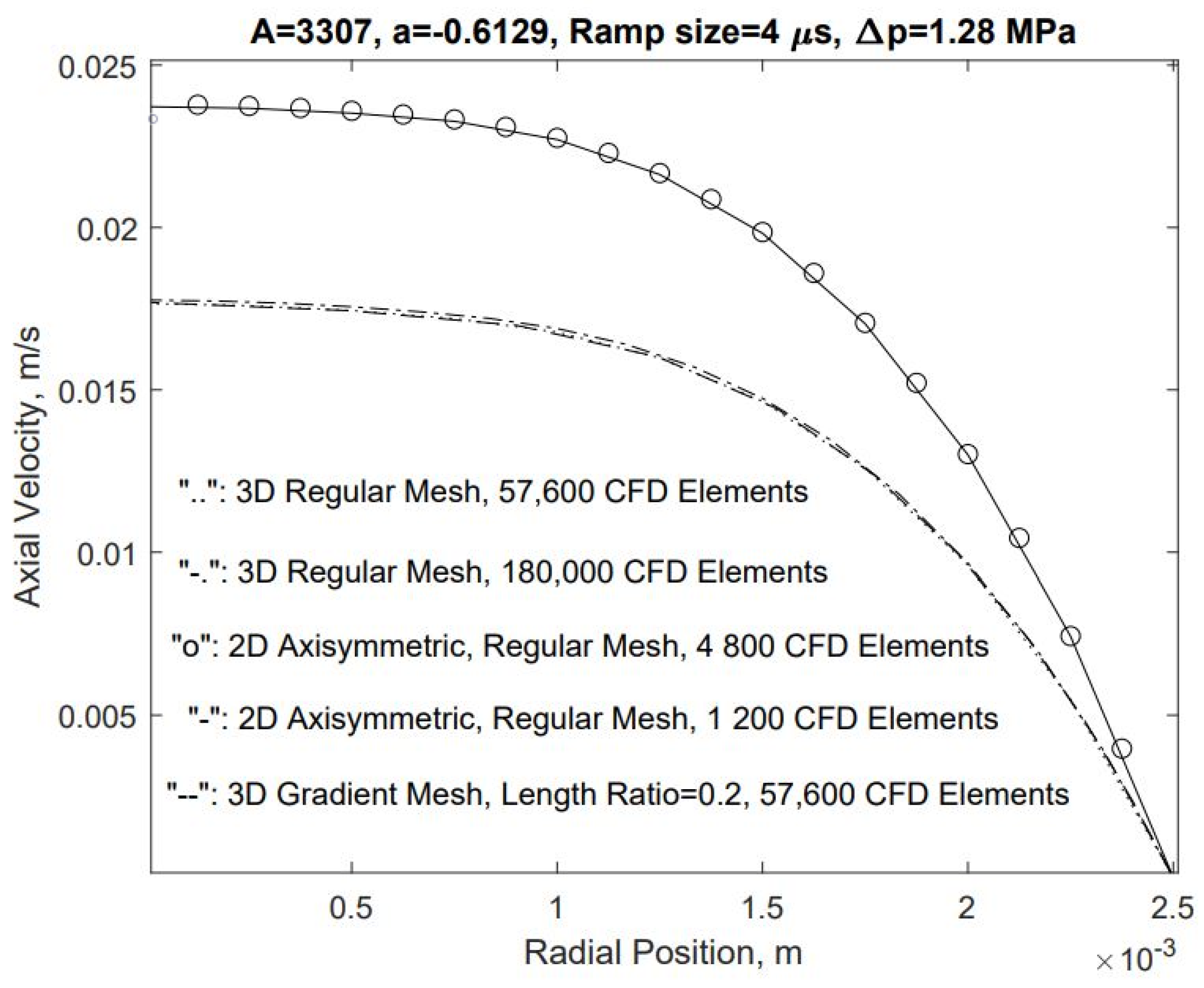
As shown in
Figure 3 and
Figure 4, the relaxation time predicted in Equation (
14) and
Figure 1 matches with both 3D and 2D computation models. Furthermore, the fully developed cross-sectional velocity profiles evaluated at a cut surface with a distance of 0.01 m from the outlet for both 3D and 2D models match very well with the analytical solution as stipulated in Equation (
5) and shown in
Figure 5. In
Figure 3 and
Figure 4, the transient solutions do approach exponentially to the theoretical or analytical solutions after five or six times the relaxation time. Of course, it is also clear that on a much larger time scale, significantly larger than that of the relaxation time, the computational results will coincide with the theoretical or analytical solutions. It is interesting to note that, as shown in
Figure 5, the 2D axisymmetric model does provide better results than the 3D model relative to the analytical approach due to the spatial resolution issues. As shown in
Figure 5, it is possible to use a denser mesh in the 2D axisymmetric model, whereas in the 3D model, with a comparable computation cost, a coarser mesh is adopted. It is then clear that in the 2D axisymmetric models, we can afford to use denser meshes for improved spatial resolutions. This, in fact, demonstrates that there is an advantage in implementing 2D axisymmetric models in comparison with comparable 3D models. Similarly, for non-Newtonian fluids, modeled in this study with the power law with
,
, and
, as depicted in
Figure 6 and
Figure 7, all three 3D models and two 2D models yield comparable results with the identical time function (the ramp size
, the time step
, and the number of steps 4000). Notice here for non-Newtonian fluids, much smaller time step is adopted in comparison with Newtonian fluids.
In fact, as illustrated in
Figure 7, unlike the parabolic distribution, the fully developed cross-sectional velocity profile for non-Newtonian fluids does resemble the velocity distribution for turbulent flows [
29,
30]. Furthermore, due to the definition of the effective shear flow rate in non-Newtonian fluid [
31,
32], although all three 3D CFD models yield almost identical results as well as all two 2D axisymmetric CFD models, there exists a seemingly systematic deference among 3D and 2D models, which might be attributed to the definition of the effective shear rate for 3D and 2D models. Nevertheless, for the non-Newtonian fluid with 4000 steps and a time step
, with the full-fledged 3D CFD mesh, the computation time is 320,964.95 s, with the coarse 3D CFD mesh with a gradient, in this case, a length ratio
, the computation time is 47,491.26 s, and with the coarse 3D CFD mesh without a gradient, the computation time is 47,504.77 s. These computational costs are virtually prohibitive for parametric studies and engineering designs. In contrast to these 3D CFD models, for non-Newtonian fluid with 4000 steps and a time step of
, with the coarse 2D axisymmetric model without a gradient, the computation time is 239.72 s, and with the dense 2D axisymmetric model without a gradient, the computation time is 1068.76 s. It is therefore clear that we must use 2D axisymmetric CFD models for physical insights into various design and rheological model variations. The discrepancies between the 2D axisymmetric models and the 3D models shown in
Figure 6 and
Figure 7 are in fact similar to those of
Figure 5. They also suggest that in the 2D axisymmetric models, we can afford to use denser meshes for improved spatial resolutions, which again demonstrates the advantage of employing 2D axisymmetric models in comparison with comparable 3D models.
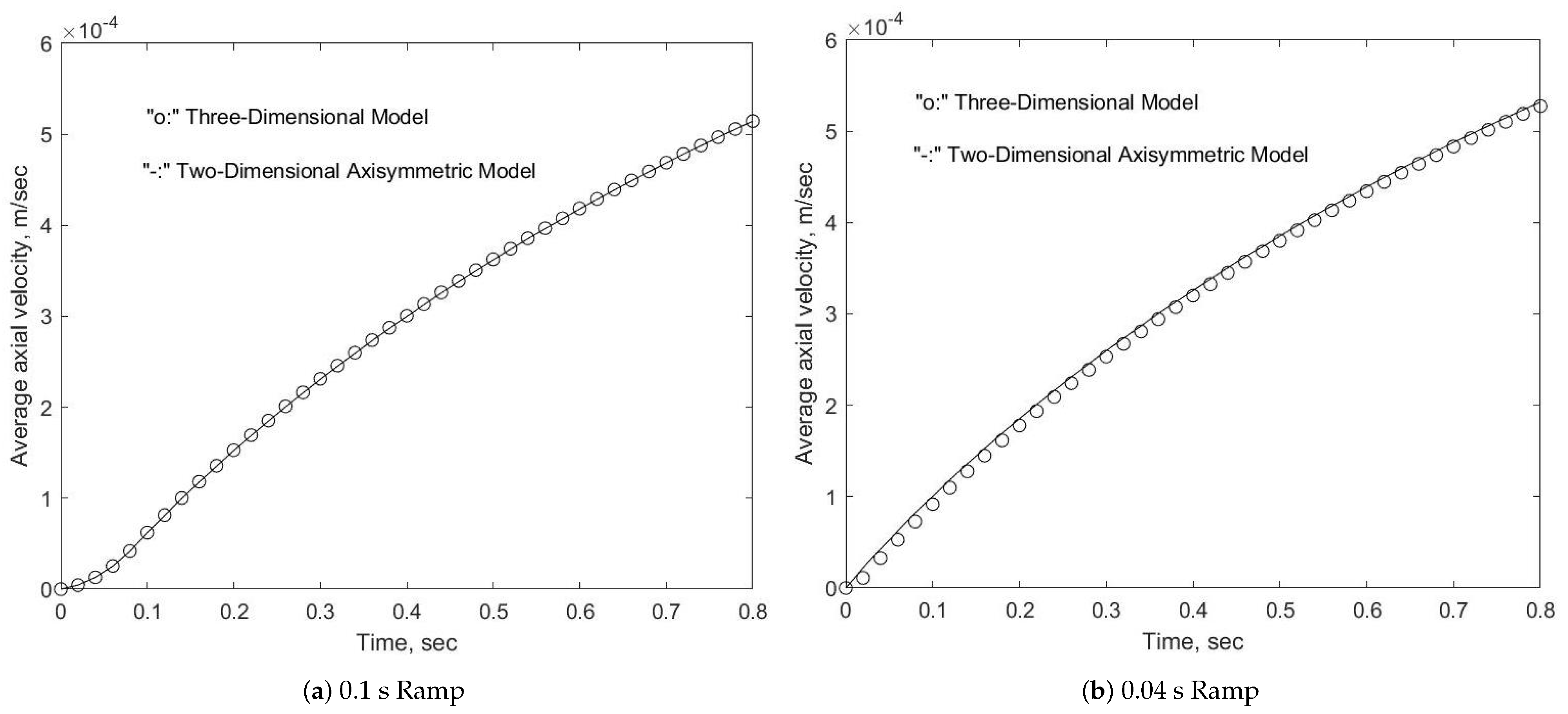
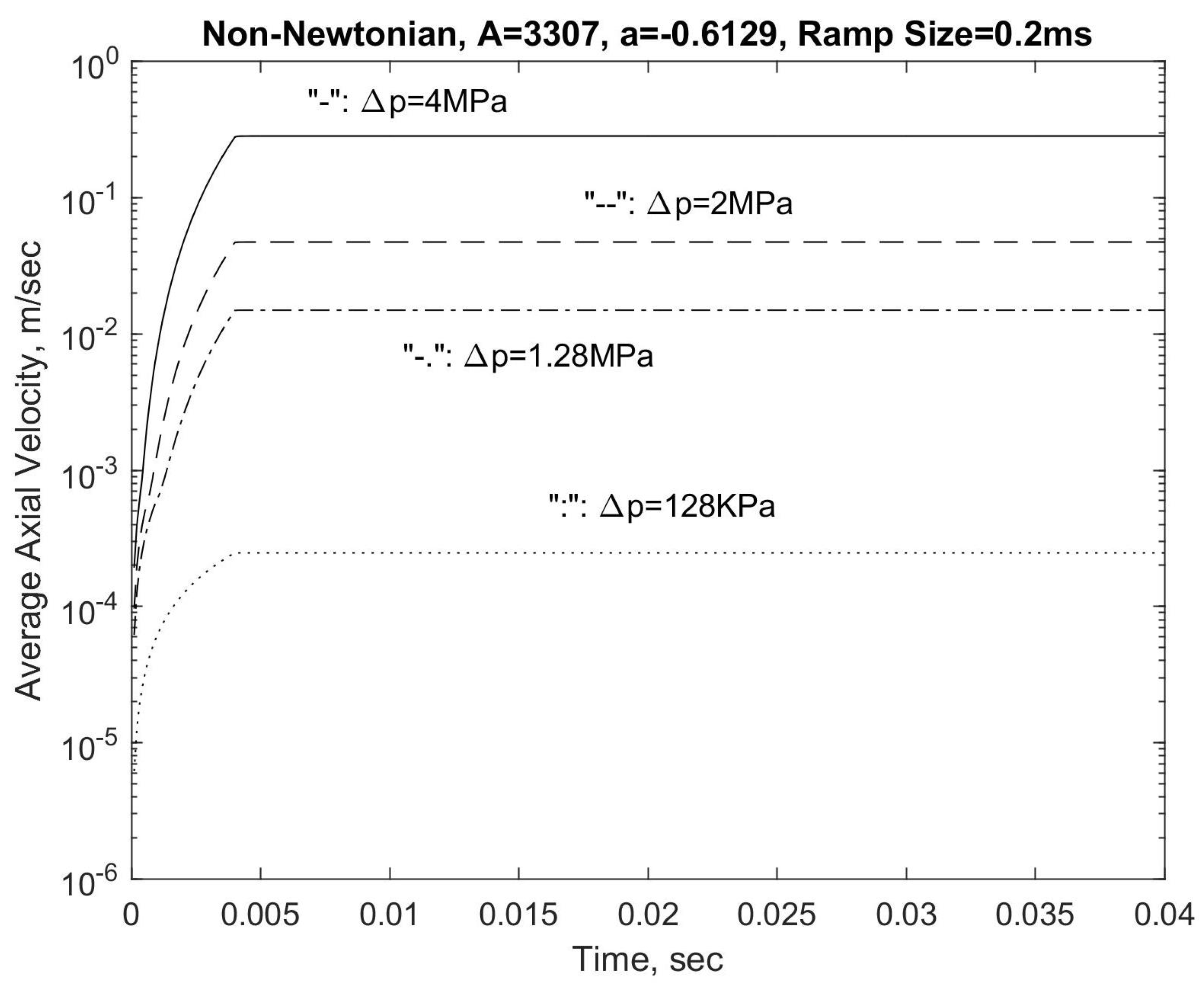
In addition, according to
Figure 8, the time-dependent average axial velocity in the transient phase does not depend too much on the size of the time function ramp, which is a piece of important information for the set of boundary conditions with the inlet pressure pulse and the constant outlet pressure. Likewise, the relaxation time is also not altered with the selection of different time step ramps. More importantly, with the same time function with a ramp size of 0.04 s, a time step of 0.02 s, and a number of time steps 40, the computational time for the two-dimensional axisymmetric CFD model is mere 8.25 s with a model with 400 fluid elements and 4411 nodes. Finally, we must note that at the end of 40 time steps, namely,
, the total transient solution is still not fully developed since the first or largest relaxation time is
as depicted in
Figure 4 and
Figure 5, therefore the transient responses in
Figure 8 suggest that the average axial velocity or the volume flow rate has not reached the fully developed stage. Moreover, as shown in
Figure 5, the cross-sectional velocity profiles for the fully developed stage are very close between the analytical solutions in Equation (
5) and results from both 3D and 2D axisymmetric models. Note that, just as predicted in Equation (
5), the peak velocity in the center of the pipe is two times the average value, which can be easily validated with Equation (
7).
Furthermore, as suggested by
Figure 3,
Figure 4 and
Figure 5, the transient behavior is very much dependent on the relaxation time, as discussed in the analytical part of this study. In fact, based on Equation (
13), for Newtonian fluids, the relaxation time depends solely on the pipe radius
R and the constant kinematic viscosity
. For Newtonian fluids, the higher the radius, the higher the relaxation time; whereas the lower the kinematic viscosity, the higher the relaxation time. In the convergence study with both 3D and 2D CFD models, the computational solution is very close to the analytical prediction based on Moody’s chart or diagram stipulated in Equation (
6). More importantly, as predicted by the Bessel function of the first kind, the relaxation time
does provide us with an accurate estimate with respect to how long the flow takes to reach the steady solution. With the current configurations, as predicted in Equation (
14) and depicted in
Figure 1, the dominant, or rather the first relaxation time is 1.0806 s, which matches very well with the computational results in
Figure 3 and
Figure 4. Moreover, around the origin, the exponential curve for the dominant relaxation
expressed as
in Equation (
15) can be simplified as
as illustrated by the tangent in
Figure 3. This information is important in the design of a dispensing system. Moreover, for the axisymmetric two-dimensional model, FCBI-C elements, as elaborated in Ref. [
33], are introduced, which carry less numerical dissipation. Moreover, we also discover that the regular finite element does provide better accuracy than unstructured mesh.
We did model all non-Newtonian fluid models with three different 3D CFD models as well as comparable 2D axisymmetric computational models.
Figure 6 clearly suggests that the relaxation times for non-Newtonian fluids are extremely small in comparison with those for Newtonian fluids. In fact, the initial transient of the volume flow rate or average axial velocity follows closely with the transient inlet pressure pulse defined by the ramp size. Notice, however, for non-Newtonian fluids, that the effective kinematic viscosity will be different for different pressure differentials or volume flow rates. Therefore, an in-depth study of the non-Newtonian fluid behaviors, especially with intermittent precision controls for different types of glues introduced in EV manufacturing plants, is essential and very much needed.
In engineering design, sometimes it is more direct to relate the shear stress
with the shear rate
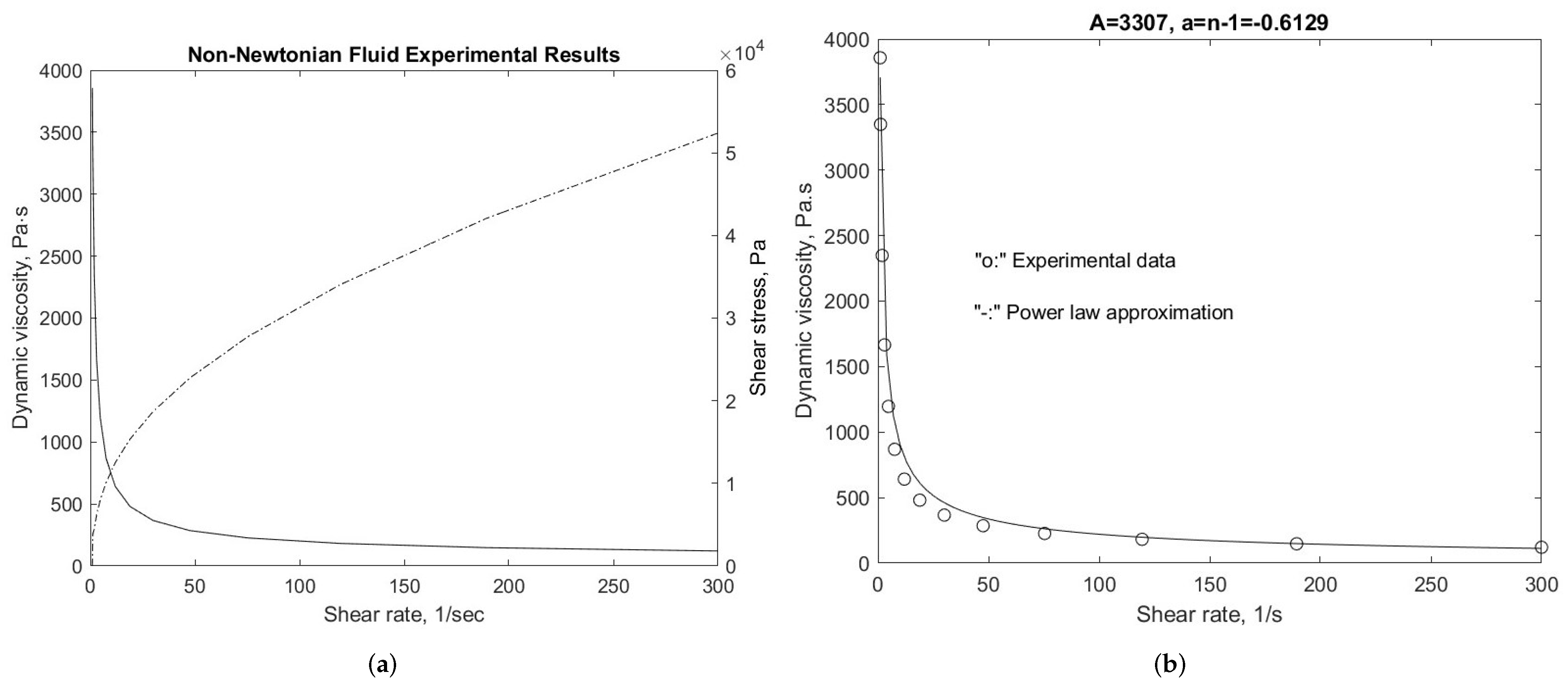
. There are in general two types of non-Newtonian fluids, namely, shear thinning and shear thickening. In this paper, we adopt the rheological properties of the non-Newtonian fluid as depicted in
Figure 9, which is clearly a shear-thinning fluid [
27,
34]. We did condense the dynamic viscosity and shear stress as functions of shear rate in
Figure 9. These characteristic curves for non-Newtonian fluids represented by a power law are directly derived based on the results of experiments using those glues for EV manufacturing. Such a rheological property is based on the direct experimental measurements of various polymers utilized in car industries. Moreover, from the force equilibrium between the shear force
F and the pressure drop
, it is obvious that the high pressure drop produces high shear stress.
In the ADINA CFD simulation, with
, the build-in power law distribution is expressed as
where
is the shear rate and
stands for the shear stress.
Assume that we have a set of
I experimental observations
with
to
I. We would like to find out the best choices for these two parameters,
A and
a. Let’s say we would like to employ
as a curve fitting the experimental data. If such a curve fits perfectly, we then have an equation
for each measurement with
to
I. In reality, there will always be an error
in each observation with
. Overall, to have the so-called best fit, we need to minimize the total error
Notice here that we use the square of the difference between
and
just to make sure that the errors will not cancel each other out. Based on the optimization concept discussed in Ref. [
4], we discover immediately that the gradient or derivative with respect to
and
a must be zero, which yields the following equation, the so-called normal equation,
where the unknown vector is
with
and
Equation (
22) is indeed the projection of the original curve-fitting equation
which requires the observation data represented by the vector
within the subspace spanned by the columns of the
coefficient matrix
.
Based on the tabulated relationship between the dynamic viscosity
and the shear rate
obtained through a series of experiments, utilizing the definition of the dynamic viscosity for a Newtonian fluid,
we have the expression for the shear stress
for the non-Newtonian fluid,
With the initial condition
with
, we can easily derive the relationship between the shear stress
and the shear rate
based on the relationship between the dynamic viscosity
and the shear rate
, with
,
For this shear-thinning polymer melt, we can easily draw the conclusion that the effective viscosity for high pressure drop or high shear stress is smaller; thus, the average axial velocity will be larger and the relaxation time will be larger as well. With this prescribed linear regression and normal equation, it is not difficult to come up with the constant
A and the power
a, in this case,
and
respectively. Note that the non-Newtonian fluid with
or
is also called pseudo-plastic. Of course, regression analysis can be used to come up with other non-Newtonian rheological properties, such as the Carreau model in Ref. [
35]. As shown in
Figure 9, these parameters yield a very close relationship between the dynamic viscosity
and the shear rate
.
In order to identify the corresponding relaxation time for the non-Newtonian polymer melt, which cannot be easily derived with analytical studies, a series of CFD models have been employed, as depicted in
Figure 10 and
Figure 11, with a sufficiently small time step of
and a ramp size of
, respectively. In
Figure 10 and
Figure 11, semi-logarithmic and logarithmic scales are introduced to depict the average axial velocity results for different pressure drops ranging from
to 4 MPa. Furthermore, as long as the shear rate is sufficiently small, namely, the pressure drop is sufficiently small, a constant minimum dynamic viscosity
will be introduced instead of the power law distribution, which explains the sudden transition in
Figure 10 and
Figure 11. In fact, with such a time step, with compressible or nearly compressible fluid models, pressure wave propagations will also be captured. In this paper, we focus on the initial transient effects and the steady solutions. The pressure waves and respective fluid-structure interaction (FSI) physical phenomena will be addressed in a separate paper.
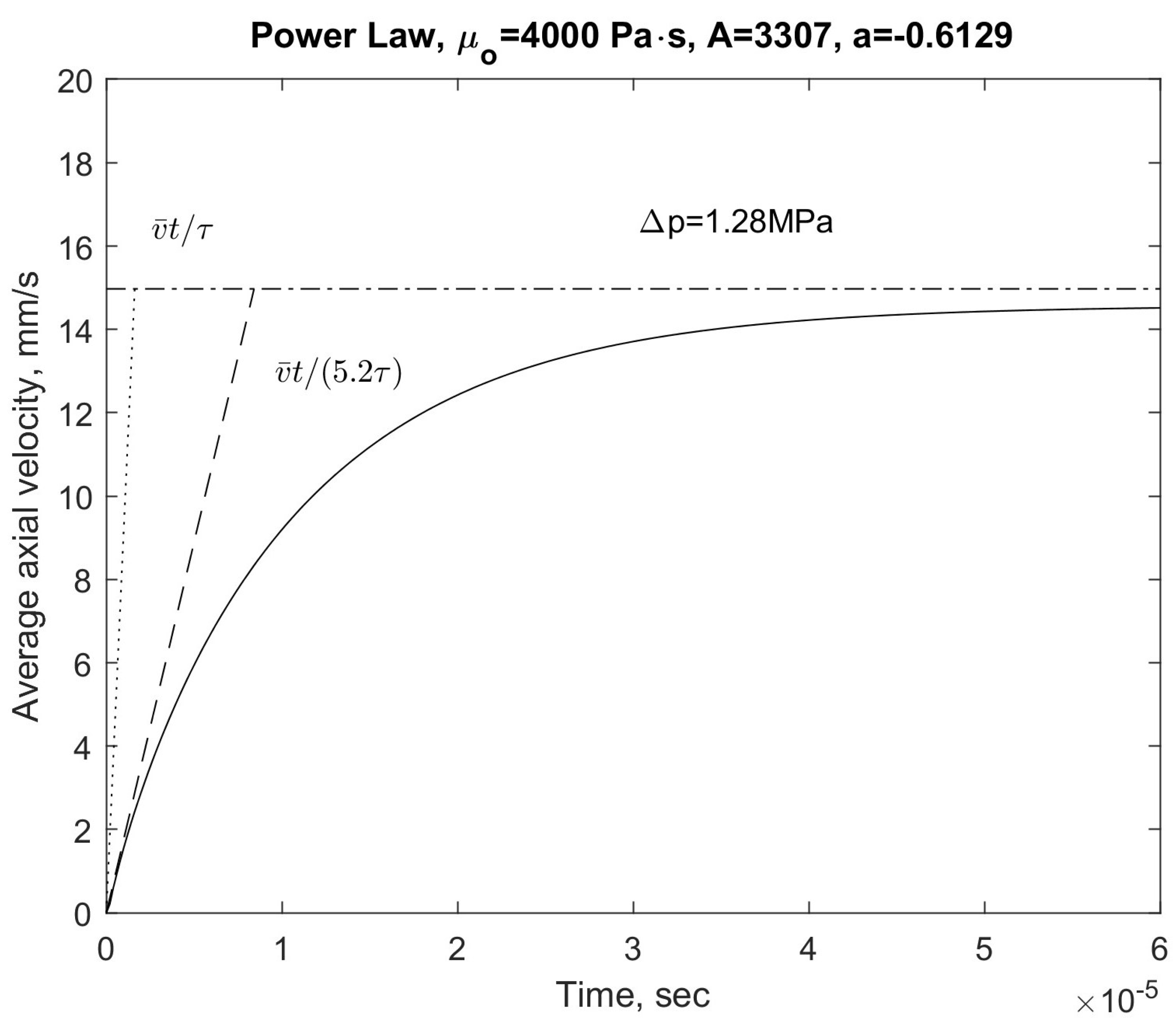
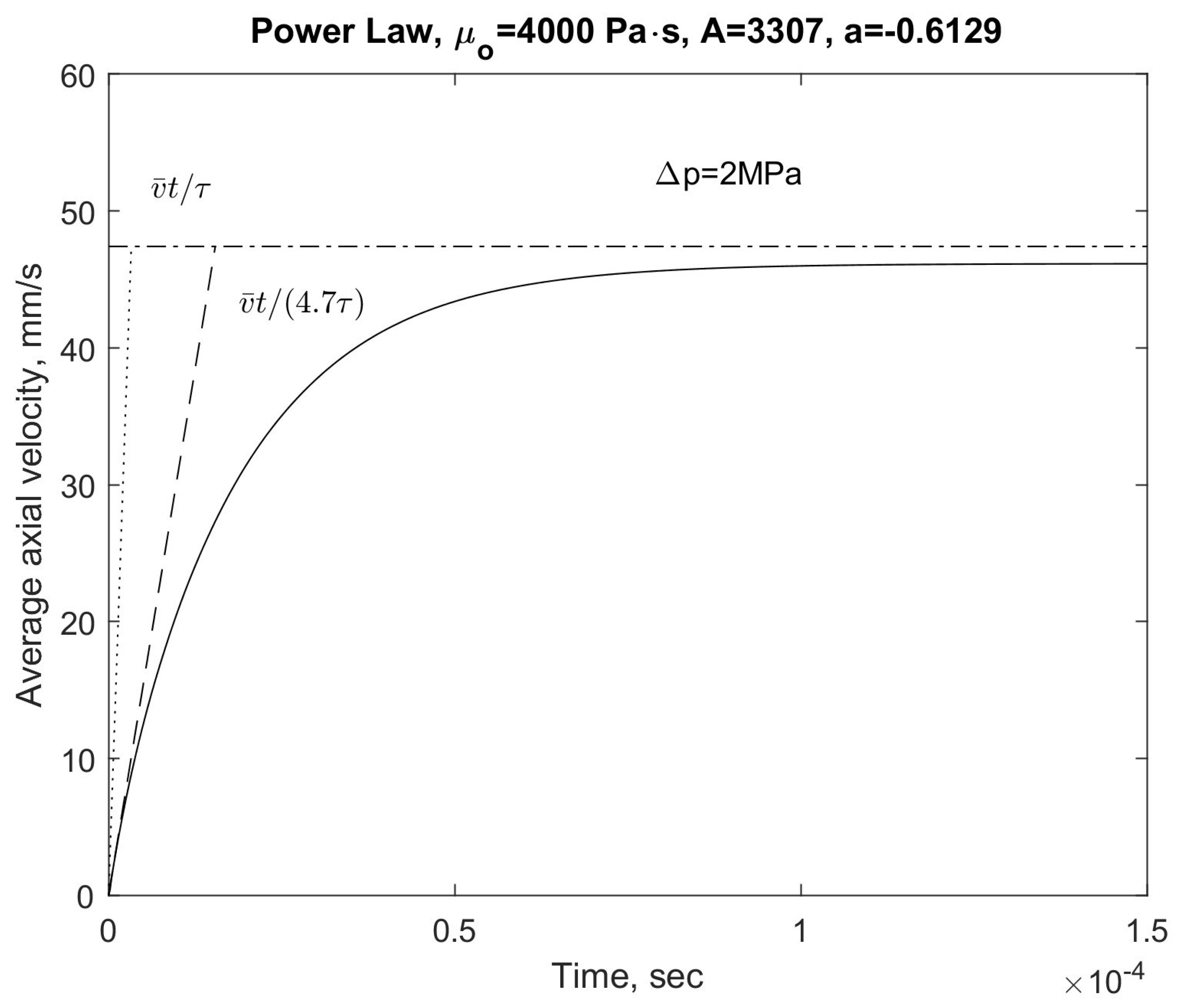
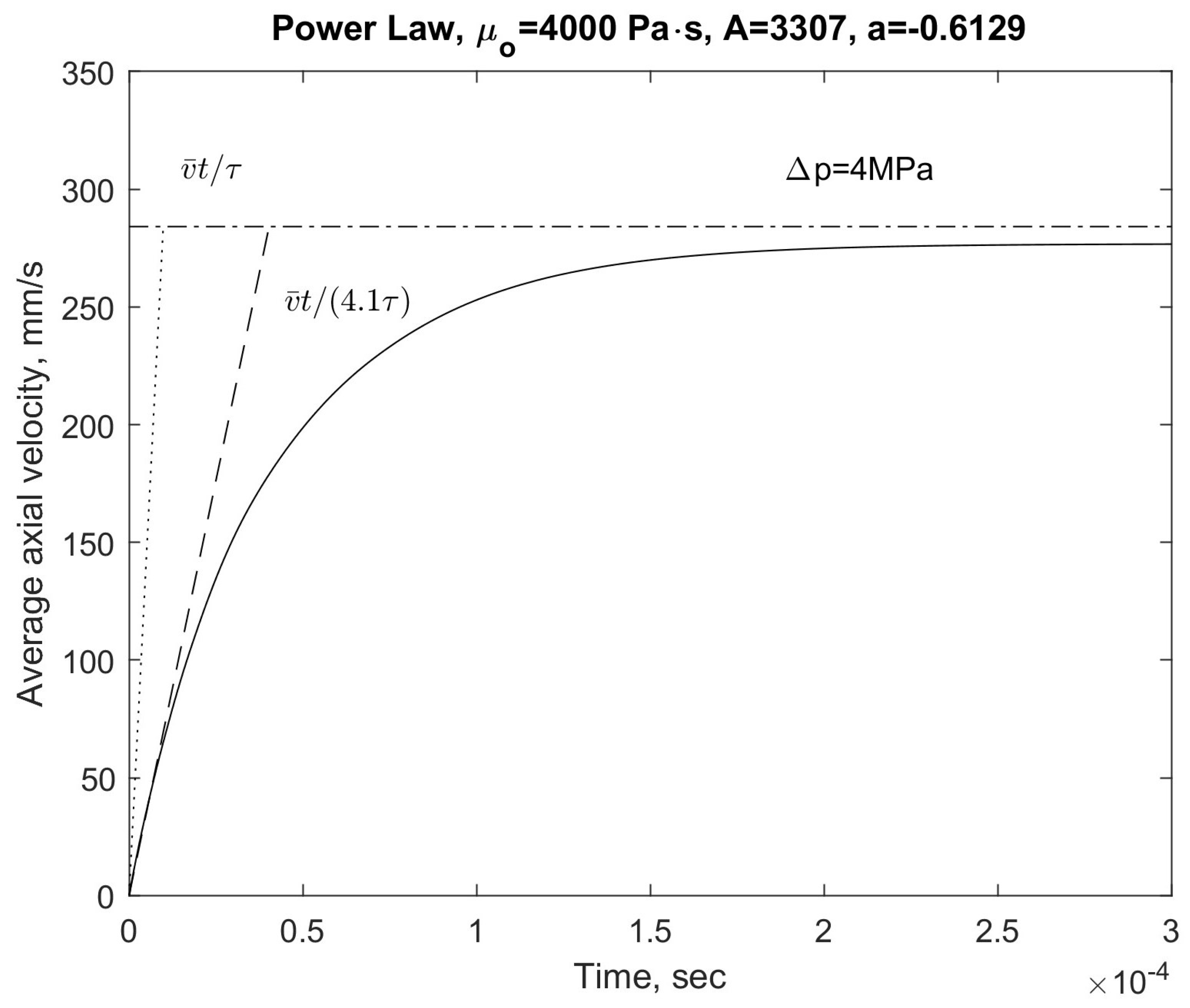
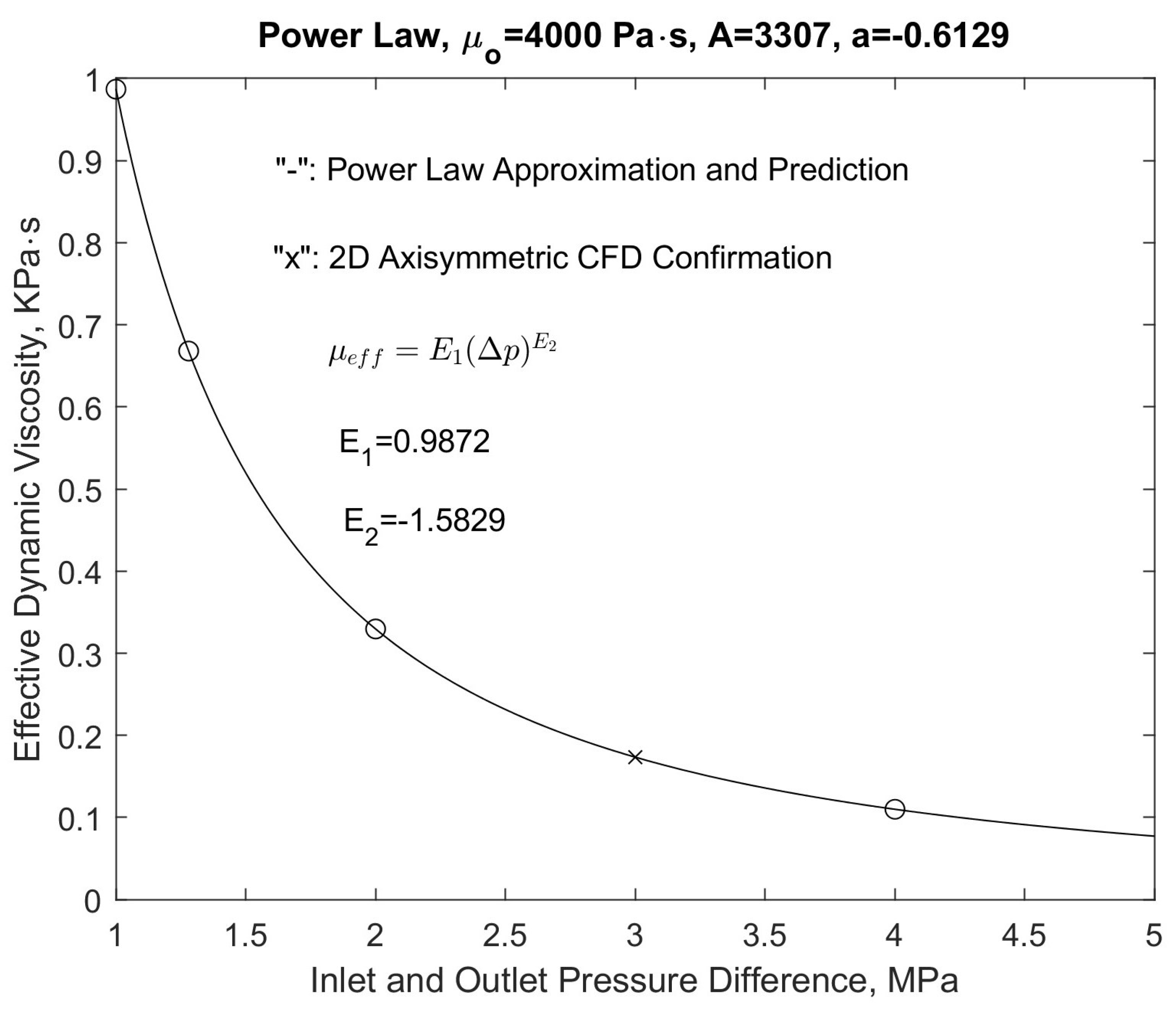
It is clear based on the CFD results depicted in
Figure 12,
Figure 13,
Figure 14 and
Figure 15, with the small ramp size and the corresponding time step, relaxation times do exist in initial transients along with the steady solution. Moreover, since the 2D axisymmetric cases are comparable with the respective three-dimensional cases and the computation times are much smaller, a sufficiently large number of time steps and cases with various inlet and outlet pressure drops have been computed for further validations. For the inlet and outlet pressure drop 1 MPa, based on Equations (
6) and (
8), we can establish the effective dynamic viscosity for this non-Newtonian polymer melt as
Notice that for a Newtonian fluid, as long as the fluid is laminar, the average velocity will be proportional to the pressure drop, which might be extended to non-Newtonian fluid at that particular inlet and outlet pressure drop. Consequently, assuming the approximate density of the polymer melt is similar to that of water, namely,
, we have the corresponding effective kinematic viscosity
Furthermore, according to the discussion of the relaxation time for a Newtonian fluid based on the zeroth order Bessel function of the first kind in Equation (
14), the effective relaxation time is evaluated as
where the radius of the pipe
R is 2.5 mm and the first root of the zeroth order Bessel function of the first kind
equals
.
As illustrated in
Figure 12,
Figure 13,
Figure 14 and
Figure 15, similar relaxation times exist for non-Newtonian fluids just as those for Newtonian fluids as depicted in
Figure 3,
Figure 4 and
Figure 8. However, for non-Newtonian fluids, the nonlinear dynamic viscosity depends on the shear rate or the axial fluid flow velocity, which is a function of the inlet and outlet pressure drop or difference. Thus, a so-called multiplier can be introduced to adjust the relaxation time derived from the relationship as presented in Equation (
14) and depicted in
Figure 1. In fact, as illustrated in
Figure 12, the equivalent relaxation time
seems to be closer to the overall viscous effect of the non-Newtonian fluid. Therefore, we introduce in this paper a so-called 2D axisymmetric multiplier
. This suggests that, with respect to the calculation of the relaxation time, the dynamic viscosity is actually
times smaller than the effective dynamic viscosity. With this comprehensive understanding of the effects of the ramp size with respect to the relaxation time and the final steady solution, which is independent of the ramp size, we can now proceed to change the peak pressure drop and establish its nonlinear relationship with the steady solution represented by the average pipe velocity [
18,
36]. The steady state volume flow rate, average axial velocity, effective dynamic viscosity, relaxation time, and 2D axisymmetric multiplier corresponding to the peak inlet and outlet pressure drop at 1,
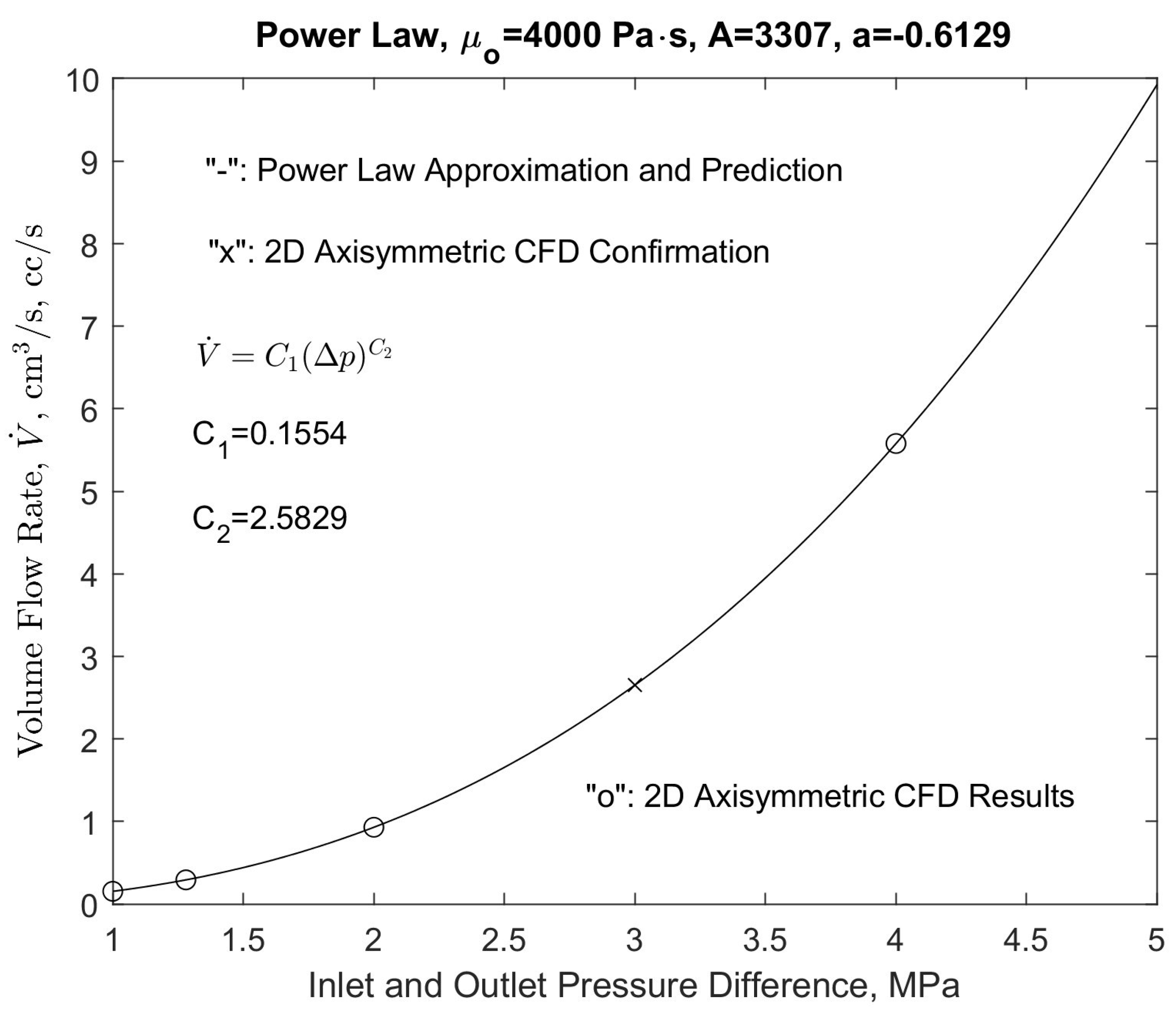
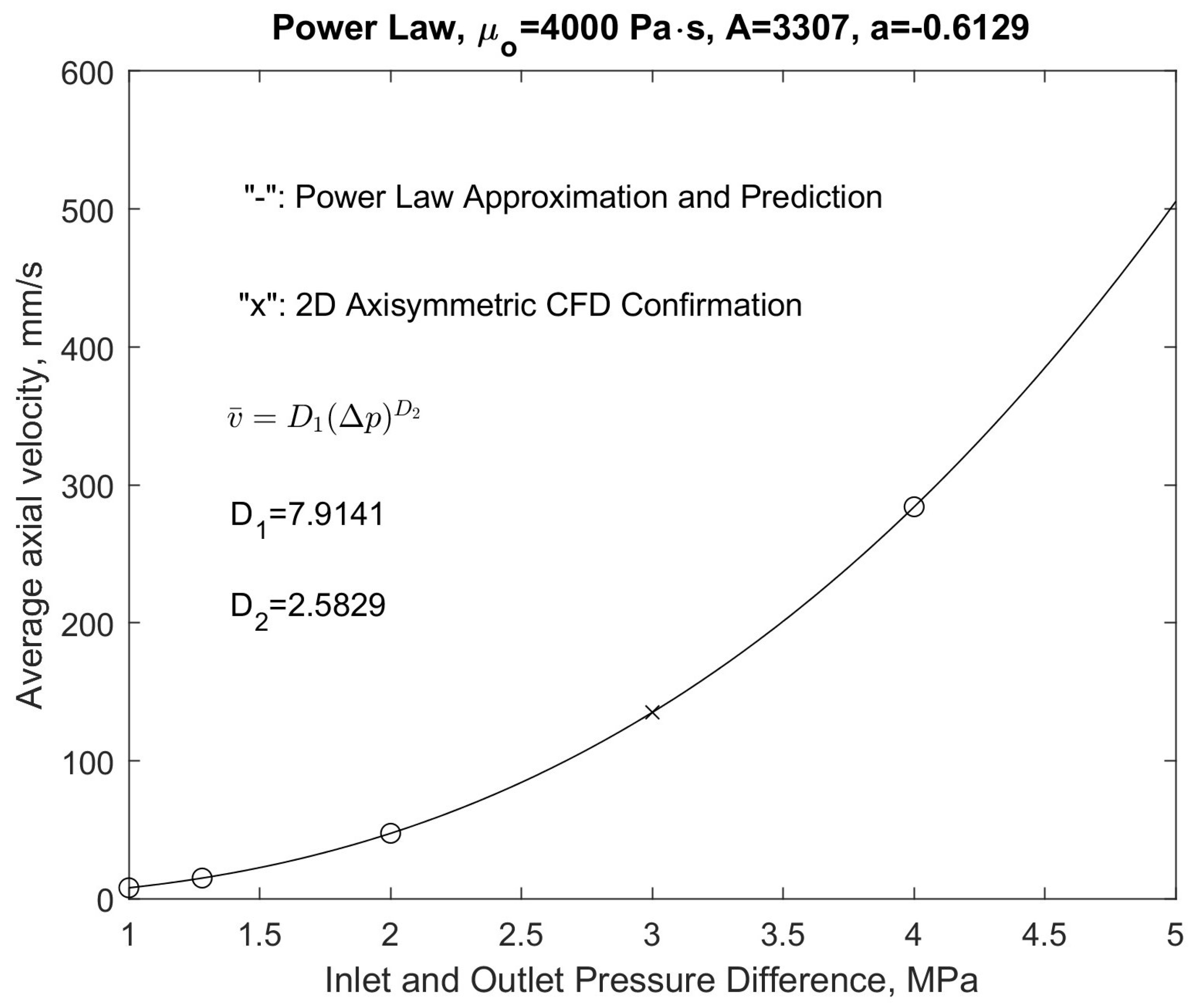
, 2, and 4 MPa are tabulated in
Table 1. In comparison with the case of the Newtonian fluid, the transient behaviors of the non-Newtonian fluid are definitely much more complex [
27,
34]. The computational results do demonstrate that due to the shear rate-induced thinning, namely, the effective dynamic viscosity decreases with the increase of the shear rate and the effective relaxation time will increase, which corresponds to the decrease of the slope of the initial tangent. It is interesting to point out that the 2D axisymmetric multiplier does experience a slight and insignificant decrease. Moreover, a power law similar to that for the non-Newtonian rheology is introduced to link these key results with the peak pressure drop. These power laws will provide designers and operators with much-needed guidelines in the precision controls of intermittent delivery of these non-Newtonian fluids.
With merely two times the peak pressure drop, namely,
increases from 2 MPa to 4 MPa, the average velocity for the steady solution is more than quadrupled, namely,
increases from 47.4075 mm/s to 284.1155 mm/s; the same for the volume flow rate, namely,
increases from
to
or cc/s. Consequently, the effective dynamic viscosity, namely,
, decreases from
to
, whereas the effective relaxation time, namely,
, increases from
to
These tabulated results match with the displays according to
Figure 12,
Figure 13,
Figure 14 and
Figure 15.
In this study, for the specific implementation in the power law of the non-Newtonian fluid, a cut-off dynamic viscosity of is introduced along with the coefficient and the power . As a result, when the inlet and outlet pressure drop is sufficiently low, namely, the shear rate is sufficiently low, instead of having an extremely high value of the effective dynamic viscosity, a cut-off dynamic viscosity is implemented in the CFD model. Thus, the transient behaviors of the non-Newtonian fluid remain the same during the very low shear laminar flow region, similar to the case for a Newtonian fluid.
Furthermore, the shear thinning effect represented by a typical power law yields, as shown in
Figure 12,
Figure 13,
Figure 14 and
Figure 15, a relationship similar to non-Newtonian rheologies modeled in
Figure 9. To reiterate, as we increase the peak pressure
from 1 to 4 MPa, the effective dynamic viscosity is significantly reduced, thus the effective kinematic viscosity is significantly decreased, and the relaxation time is increased accordingly. In addition, the 2D axisymmetric multiplier
decreases from
to
Figure 16,
Figure 17 and
Figure 18 again demonstrate clearly the thinning effect, which is consistent with what the rheological properties suggest for this type of polymer melt. To reiterate, under sufficient pressure drop, the effective kinematic viscosity will decrease significantly, and as a result, the relaxation time will increase accordingly.
As shown in
Figure 12,
Figure 13,
Figure 14 and
Figure 15, the steady solution yields a significantly larger average velocity, which confirms that the effective viscosity is much smaller. In addition, the multiplier for the relaxation time changes from
to
, which indicates that the equivalent relaxation time decreases according to the effective kinematic viscosity and, more importantly, gets closer to the relaxation time predicted with the effective kinematic viscosity. Moreover, to depict the thinning effects, we also plot the pressure drop in
vs. the steady flow rate defined as
in
as shown in
Figure 16 as well as the pressure drop in
vs. the effective relaxation time in
as shown in
Figure 19.
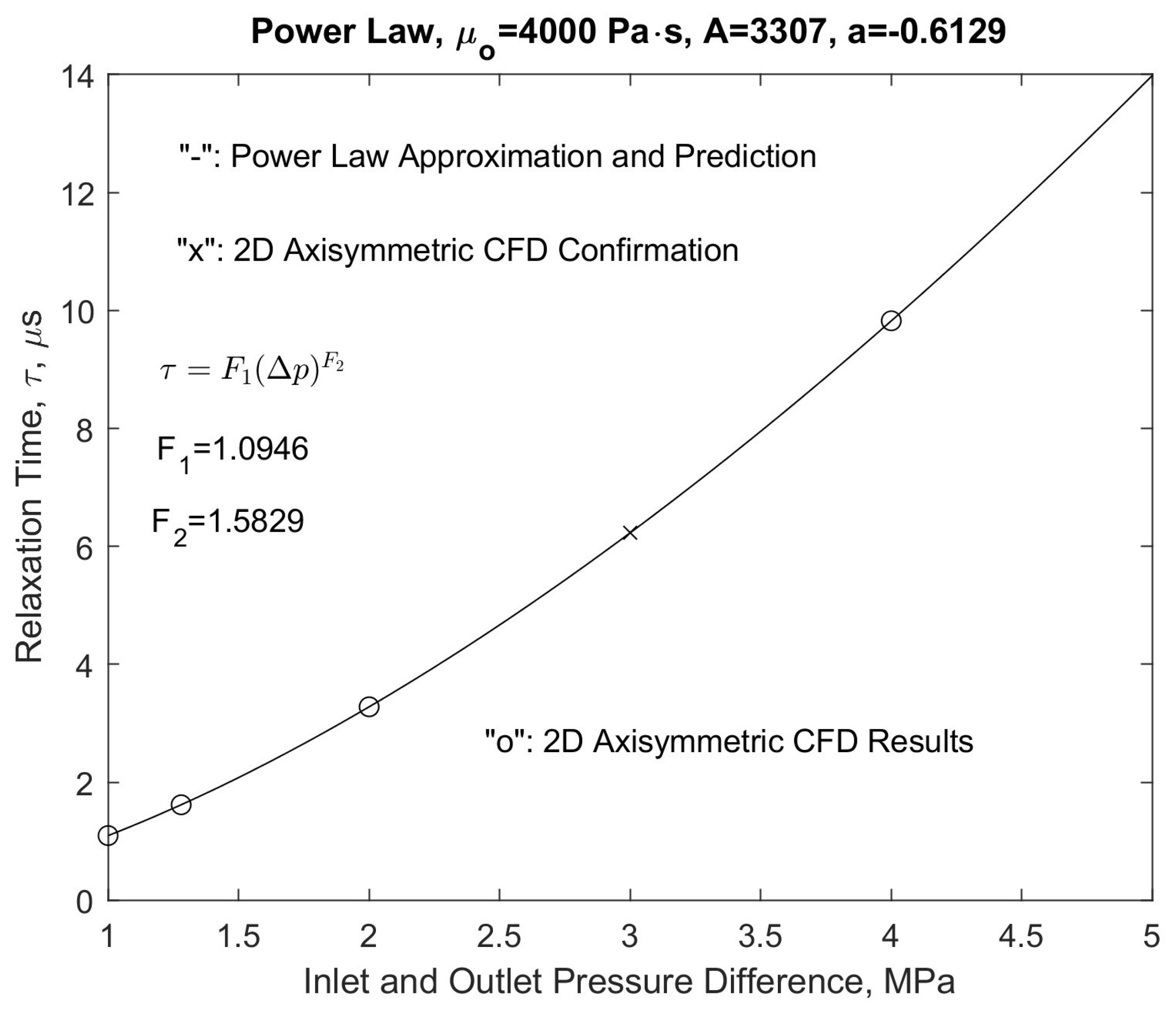
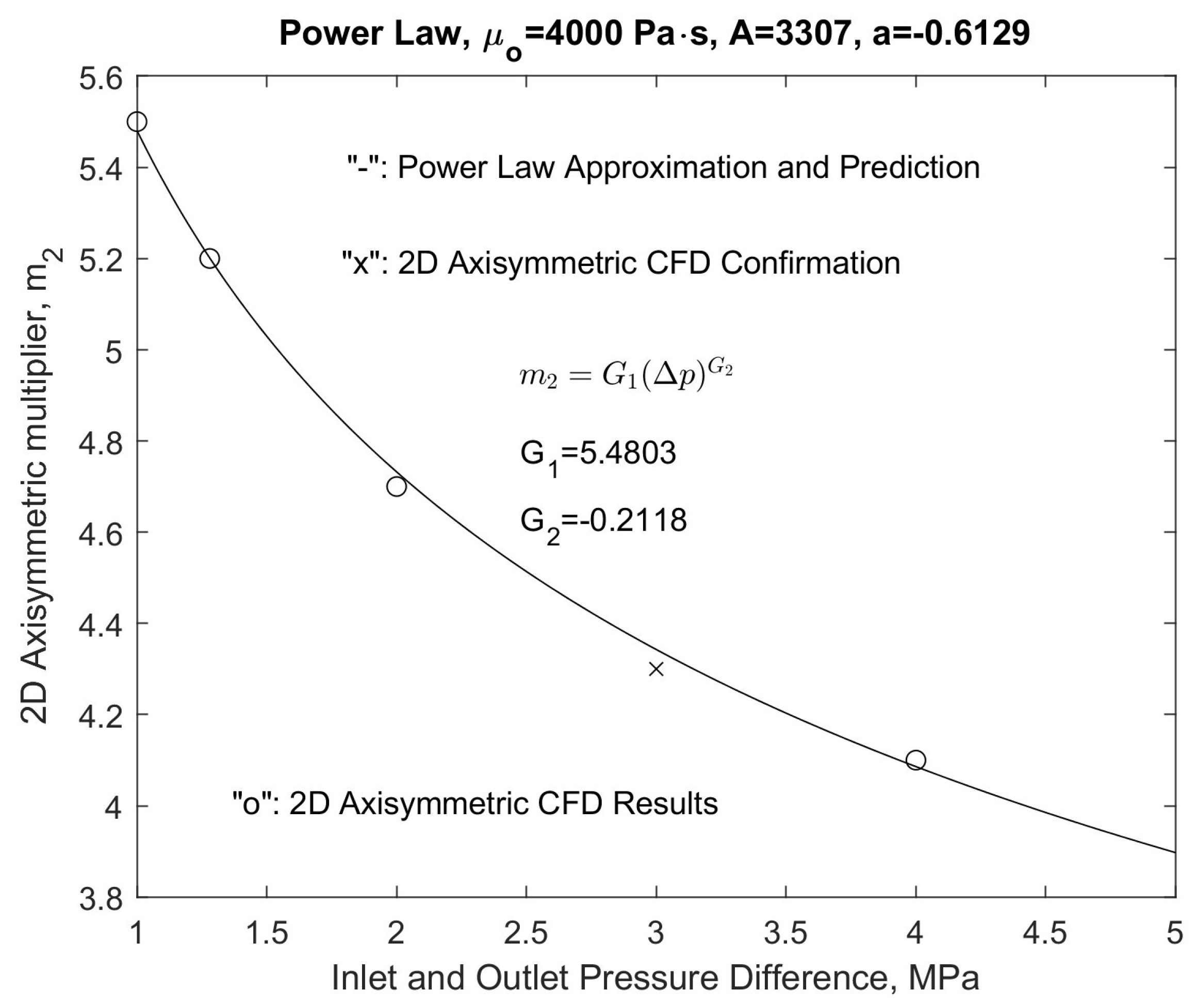
Moreover, in order to derive empirical power laws for the relationship between the pressure drop
and the volume flow rate
, the average axial velocity
, the effective dynamic viscosity
, the corresponding relaxation time
, and the multiplier
, the following power laws or the equivalent logarithmic forms are introduced
Using the same projection method for the normal equations as we have employed for the power law rheological relationship between the shear rate and the dynamic viscosity, the coefficient solutions for these power laws can be easily derived, namely, and are and , respectively; and , are and , respectively; and are and , respectively; and are and , respectively; and and , are and , respectively. As a consequence, using the power laws, we can predict a priori the transient flow response for the inlet and the outlet pressure differential 3 MPa, marked by a cross, namely, volume flow rate 2.6532 cc/s; average axial velocity 13.5127 mm/s; effective dynamic viscosity 0.1734 ; effective relaxation time ; and 2D axisymmetric multiplier , respectively, which are virtually identical to the 2D axisymmetric CFD simulation results.
Notice the relationship, especially the power, among the average axial velocity
or the volume flow rate
, the effective dynamics viscosity
, the corresponding relaxation time
, and the 2D axisymmetric multiplier
. As illustrated in
Figure 16,
Figure 17,
Figure 18,
Figure 19 and
Figure 20, with the open circle representing the individual cases and the solid line the effective power law relationship, the curve fit is extremely accurate, which suggests that the power law distribution with constant coefficients does match with the physical reality. To test the validity of these approximations, a new peak pressure drop of 3 MPa is selected
a priori. With the 2D axisymmetric model, it only takes 177 s per set of test conditions, instead of a few days for the full-fledged 3D model. We have the predicted results using the power law distributions for the volume flow rate, average axial velocity, effective dynamic viscosity, relaxation time, and 2D axisymmetric multiplier match very closely with the computational modeling, namely, 2.6532 cc/s, 135.126 mm/s,
,
, and
, respectively, as denoted with the symbol
x in
Figure 16,
Figure 17,
Figure 18,
Figure 19 and
Figure 20. The fact that the actual simulation results, with a different boundary condition, as reported in
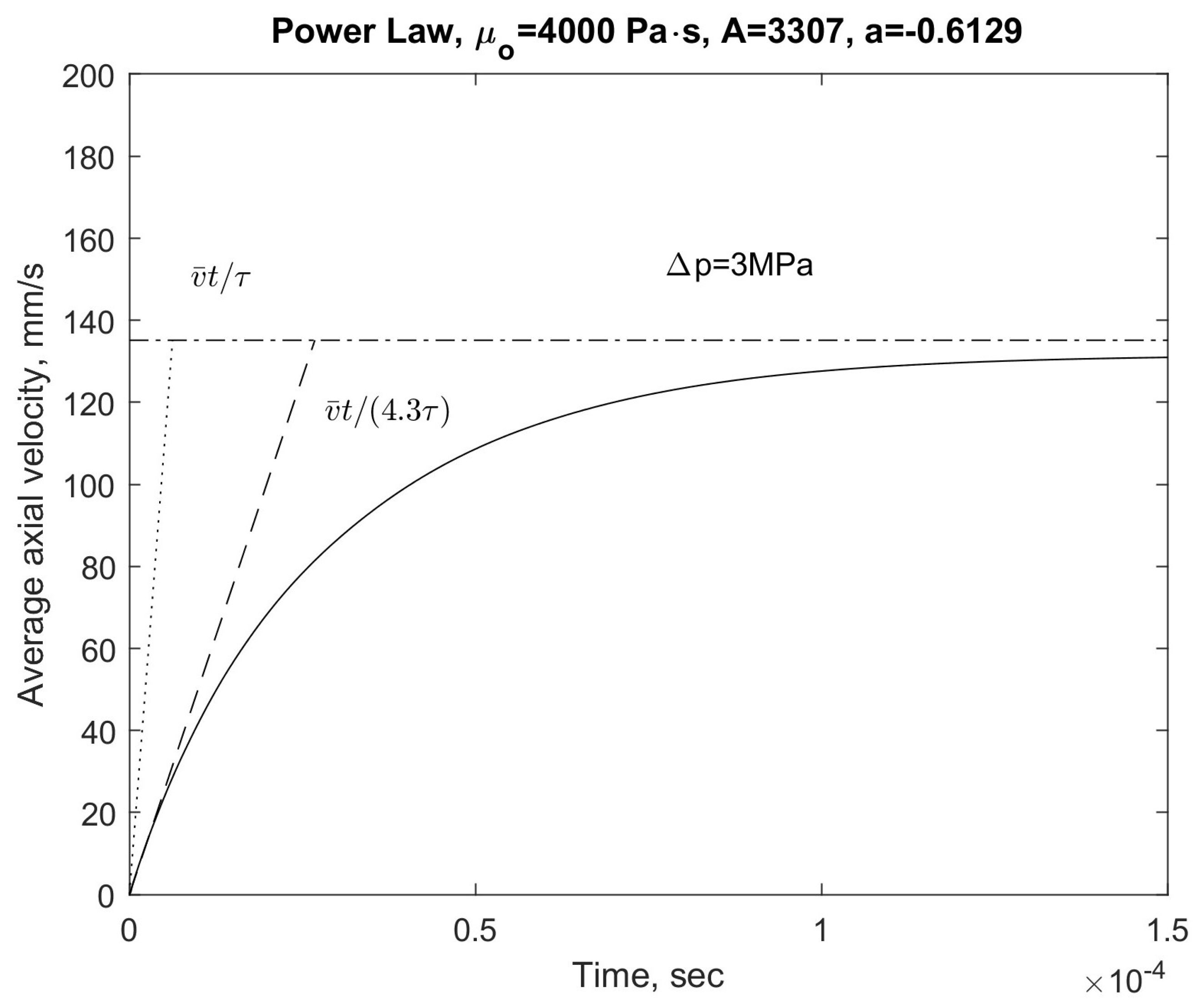
Figure 21, match very well with the predictions from the power law distributions
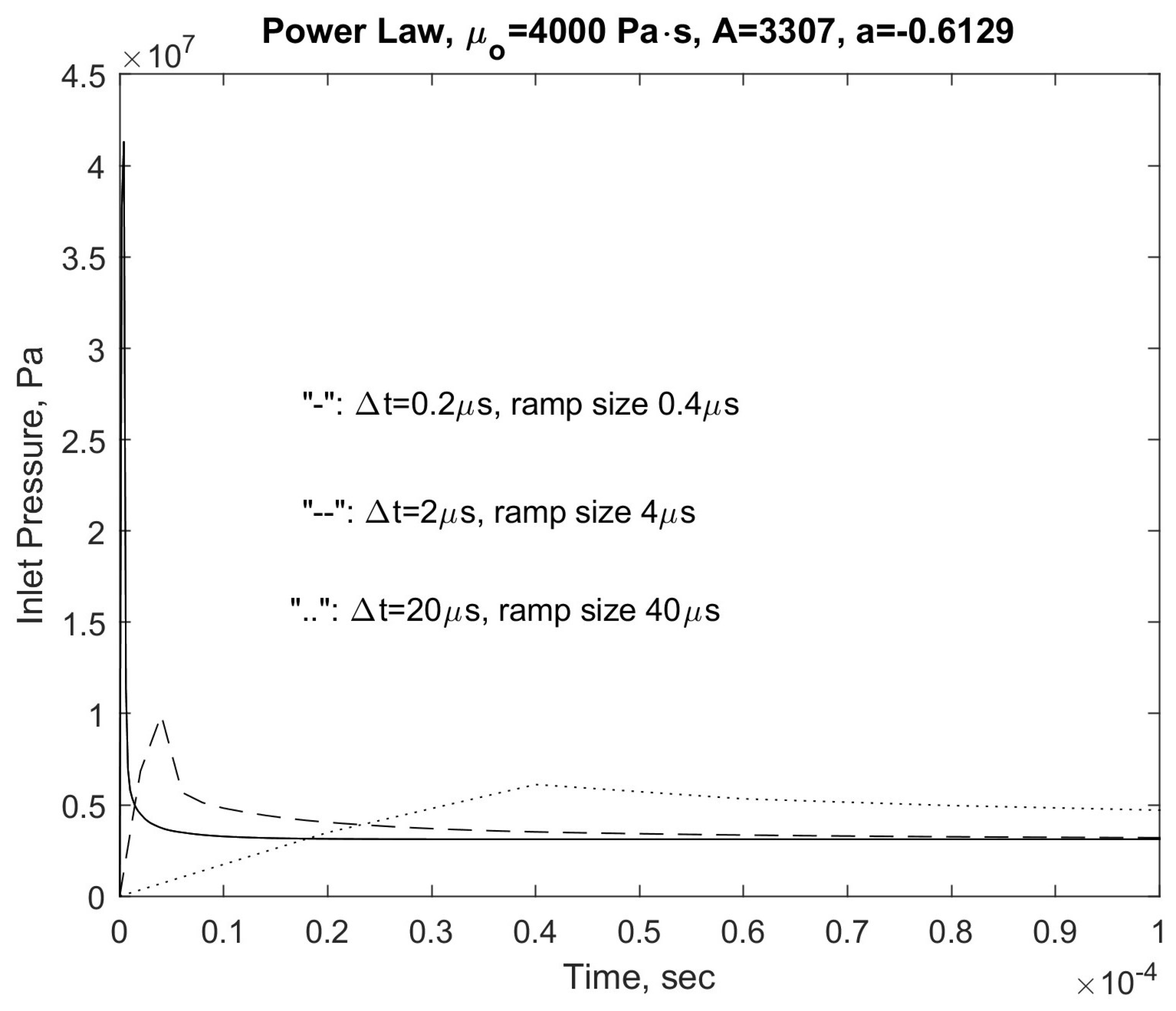
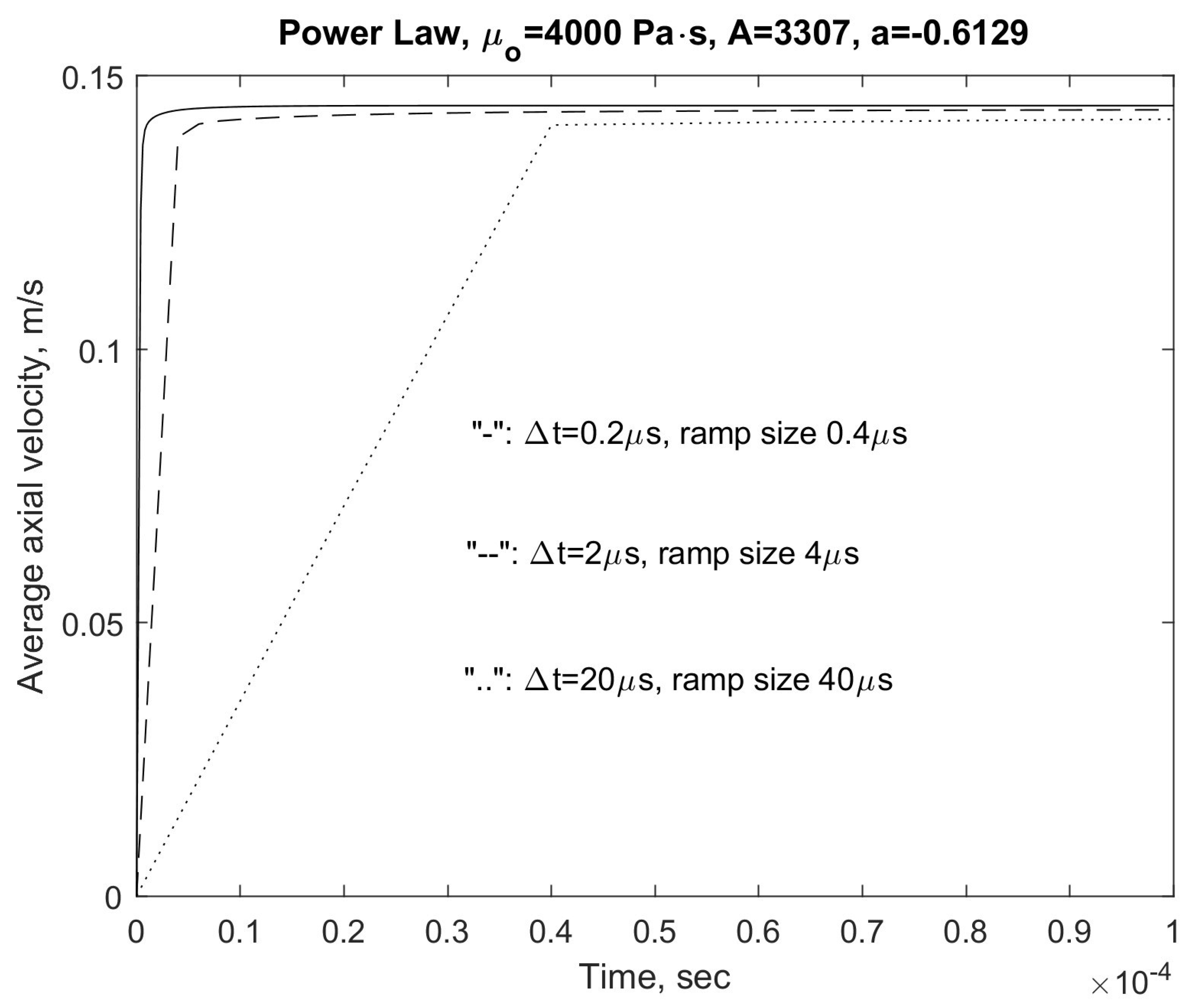
a priori confirms the validity of the behaviors of these non-Newtonian fluids modeled with a power law distribution. Finally, to further validate the investigations and conclusions in this paper, we replace the inlet boundary condition with an average axial velocity of 150 mm/s. The steady average inlet pressure as shown in
Figure 22 approaches 3 MPa with an average axial velocity around 135 mm/s. As depicted in
Figure 22, the inlet pressure does eventually approach the same steady solution regardless of the ramp sizes, in this case, 0.4
s, 4
s, and 40
s, and the time step sizes, in this case, 0.2
s, 2
s, and 20
s. Furthermore, as illustrated in
Figure 22 and
Figure 23, the choice of the ramp size, which essentially determines how fast the servo motor is actuated, does have tremendous effects on the initial inlet pressure, even for an incompressible non-Newtonian fluid model due to inertia effects. Naturally, to fully understand such phenomena, more complex FSI with the flexible tube and the wave propagations within both fluid and solid continua must be considered. However, it is clear and could be suggested for precision control of the dispensing of non-Newtonian glues in EV manufacturing, the ramp size of the servo motor must be tuned properly to ensure the timely fluid delivery and to suppress unnecessary structure oscillation. As depicted in
Figure 22 and
Figure 23, the smaller the ramp size, the quicker the servo motor generates the desired volume flow rate and the higher the inlet and outlet pressure drop. We must also point out that the numerical treatment of the average axial velocity has a slight discrepancy in comparison with the volume flow rate due to the actual cross-sectional area representation and the boundary wall conditions. For the 2D axisymmetric CFD mesh, in the cross-sectional direction, 10 CFD elements are employed. In the particular setting of the average axial velocity, the velocity boundary for the wall is zero; thus, the average velocity is in effect applied to nine out of the ten elements, which accounts for the discrepancy of 150 mm/s vs. 135 mm/s average axisymmetric velocity. In most engineering practices, however, such accuracy with a relatively coarse CFD mesh does provide engineers with important guidelines in the selection of operation parameters in the controlled intermittent distributions of such non-Newtonian fluids.