Experiments on Steam Injection into Preformed Steam Chambers of Various Shapes for Maximum Condensate Recovery
Abstract
1. Introduction
2. Preformed Steam Chambers and Parameters Governing the Steam Flow
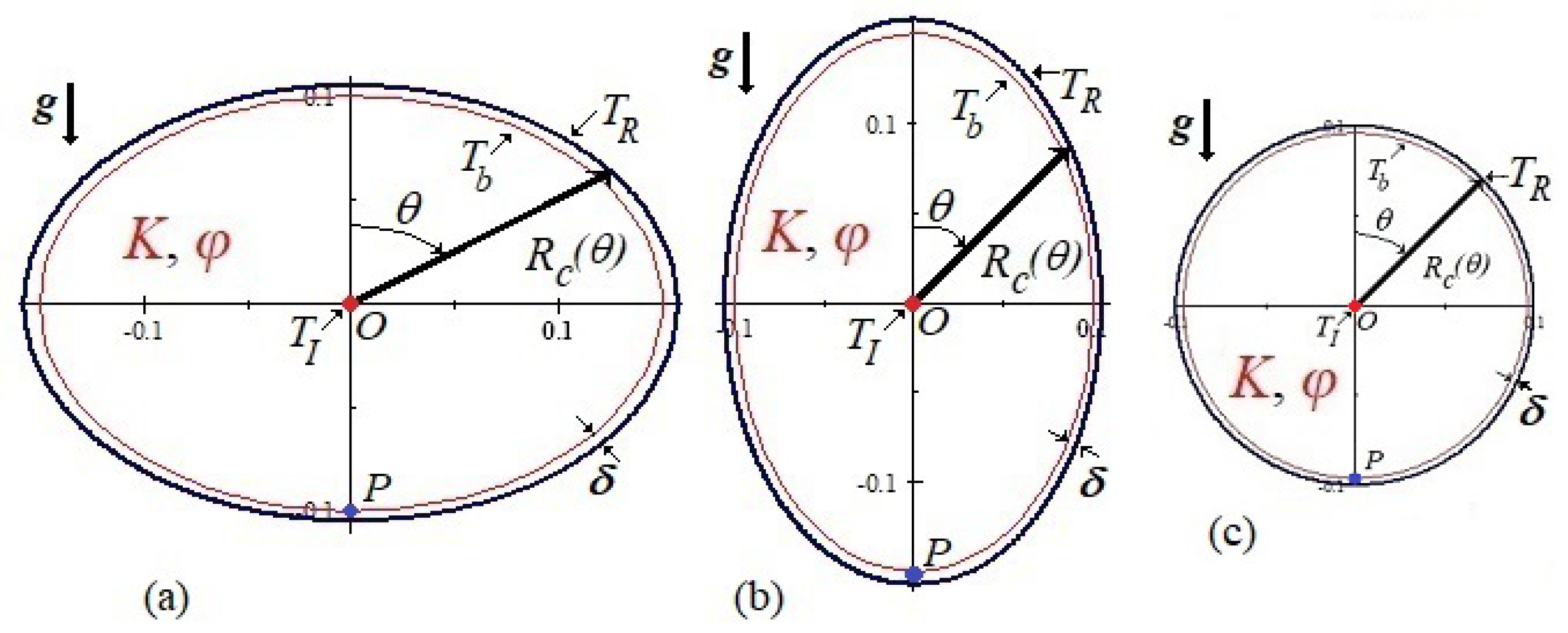
2.1. Preformed Steam Chambers
2.2. Parameters of the Steam Flow in SAGD
3. Experiments of Steam Injection into Elliptical and Circular Chambers
3.1. Steam Chambers
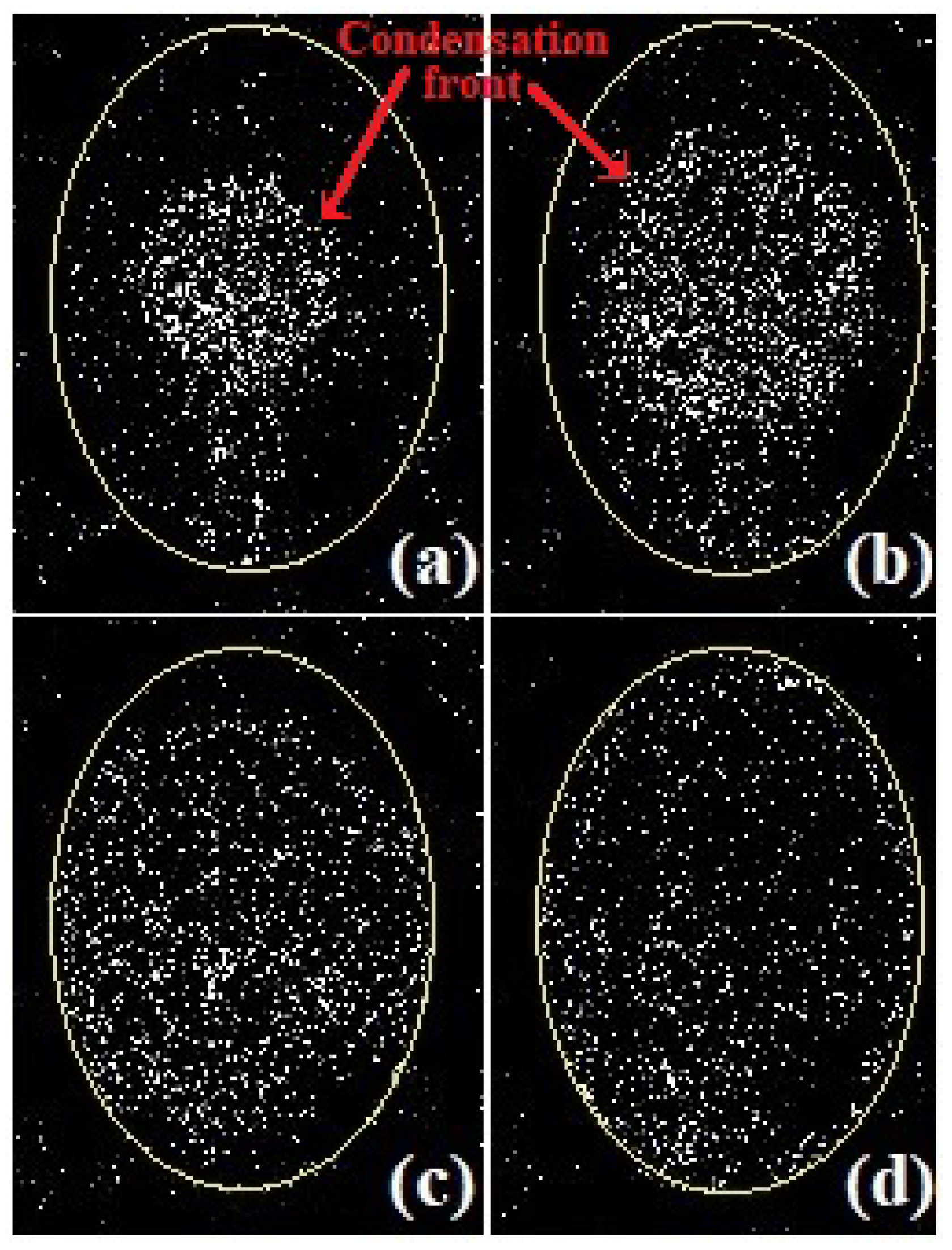
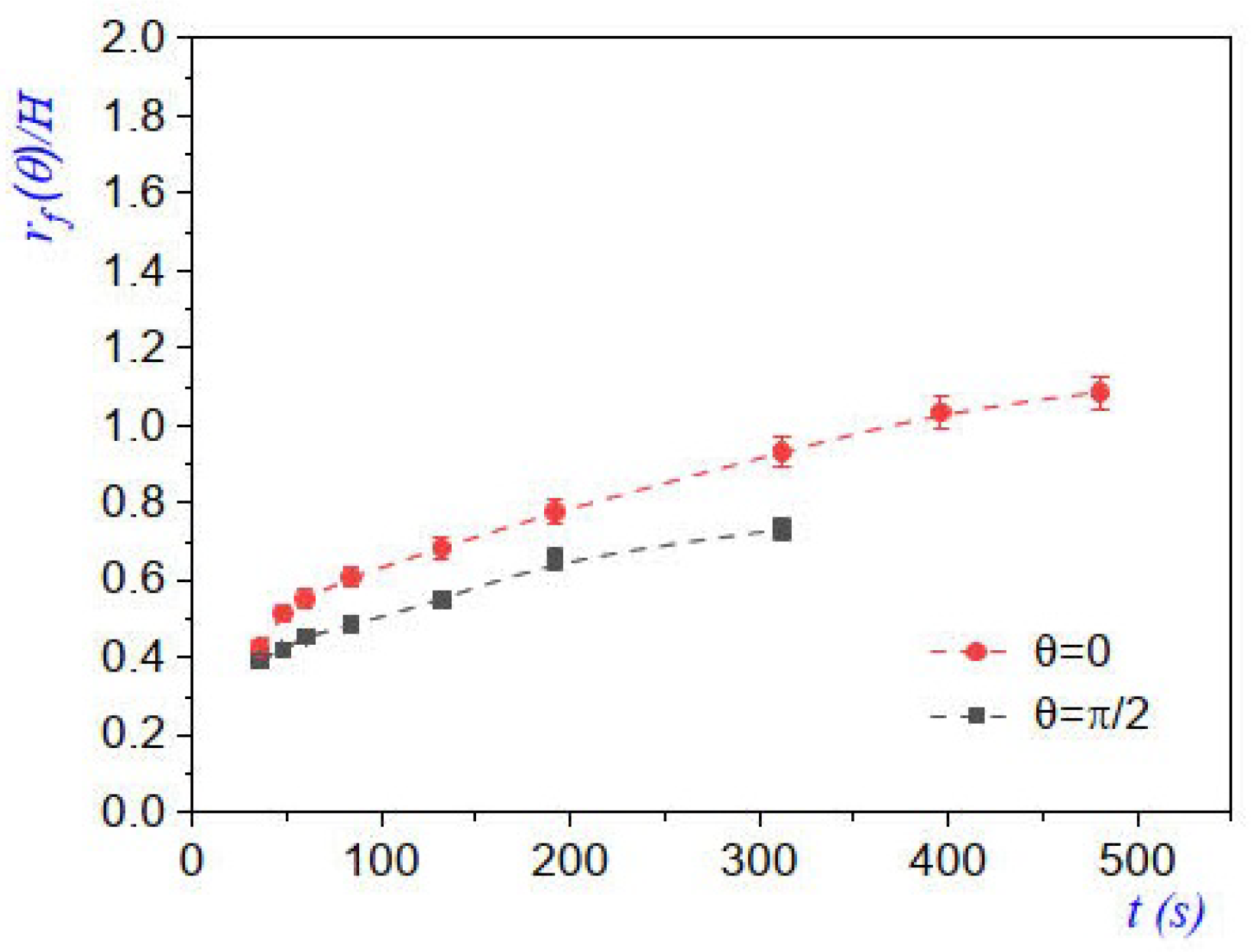
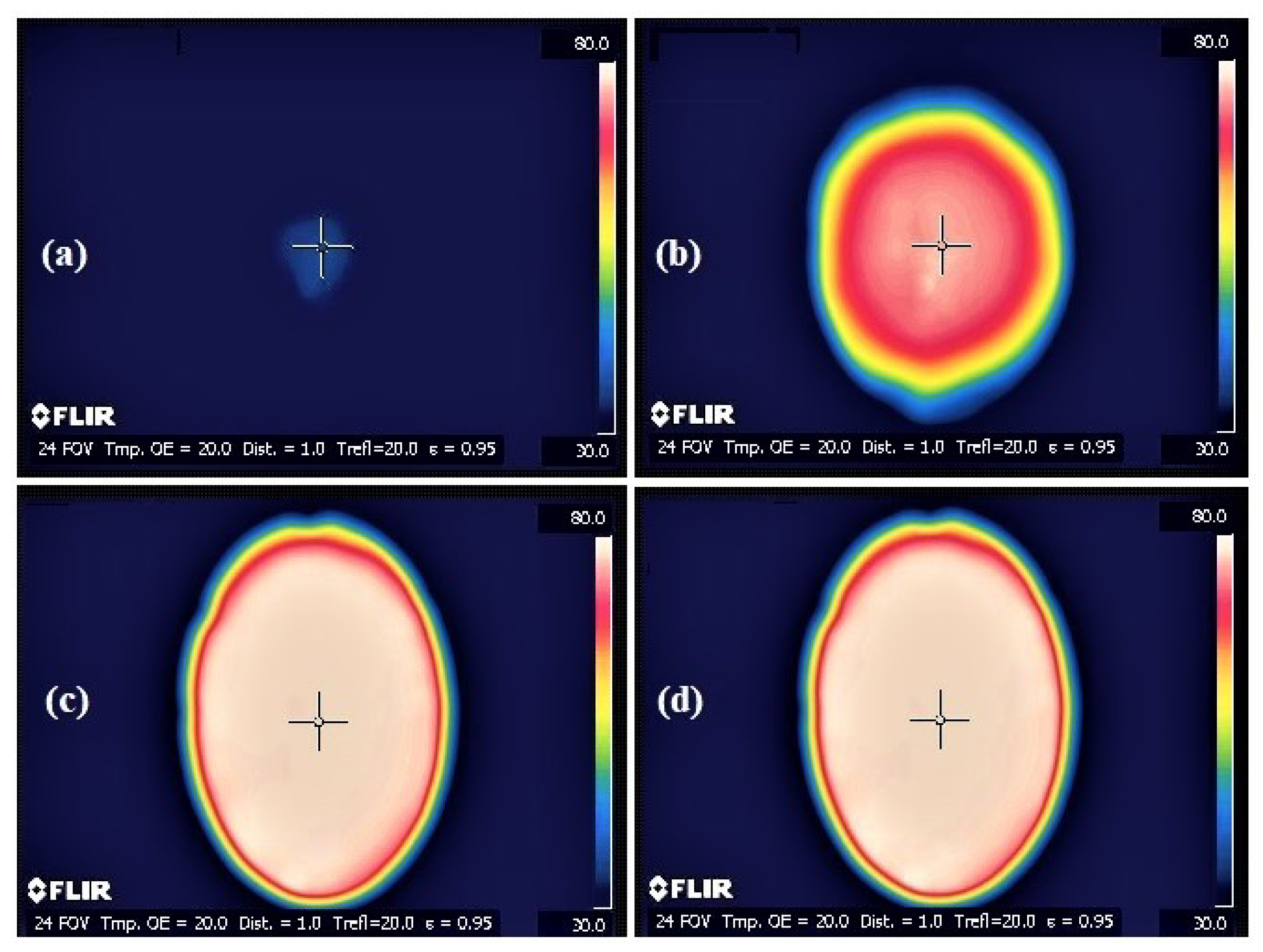
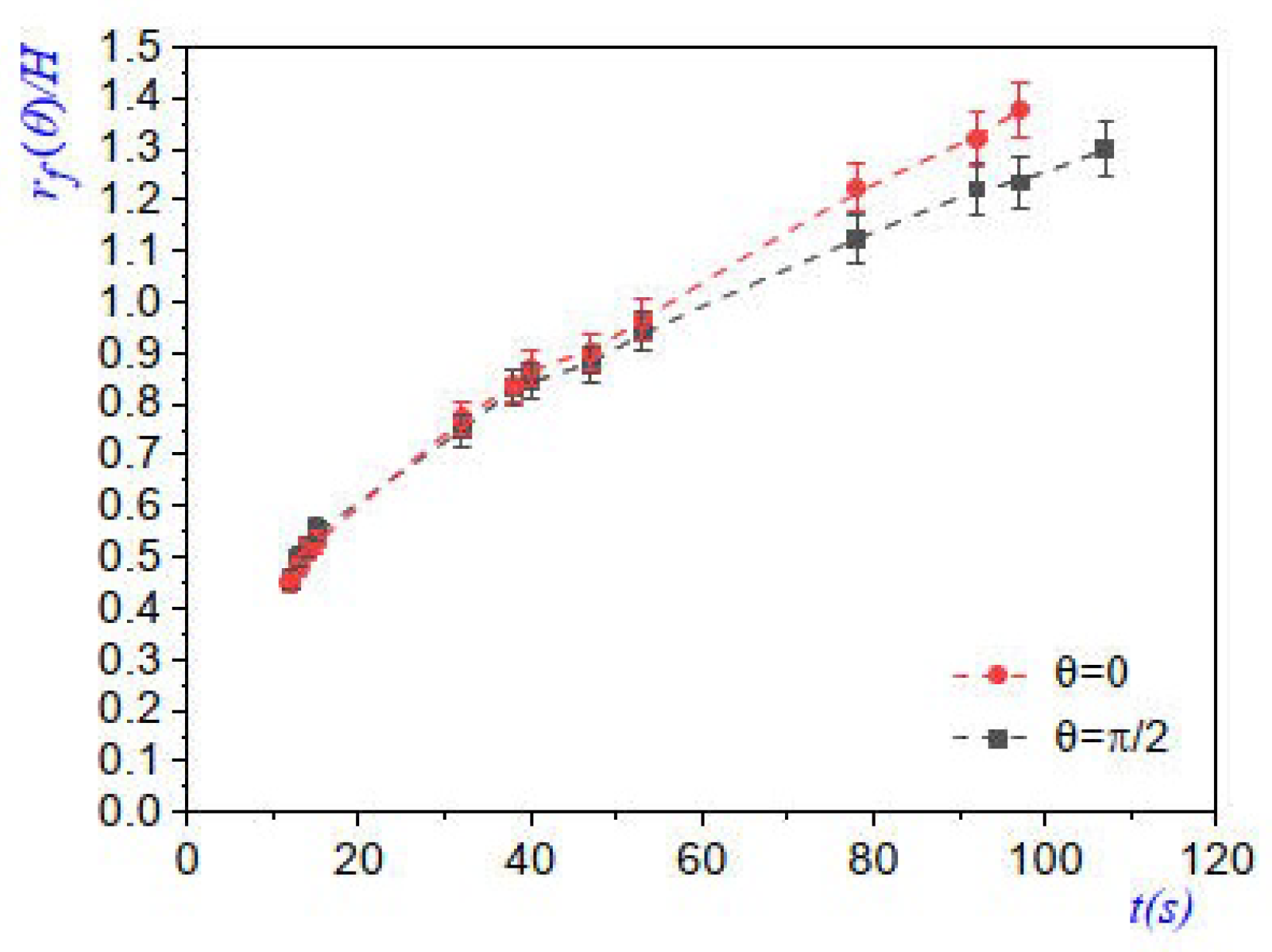
3.2. Transient Steam Flow in an Elliptical Steam Chamber
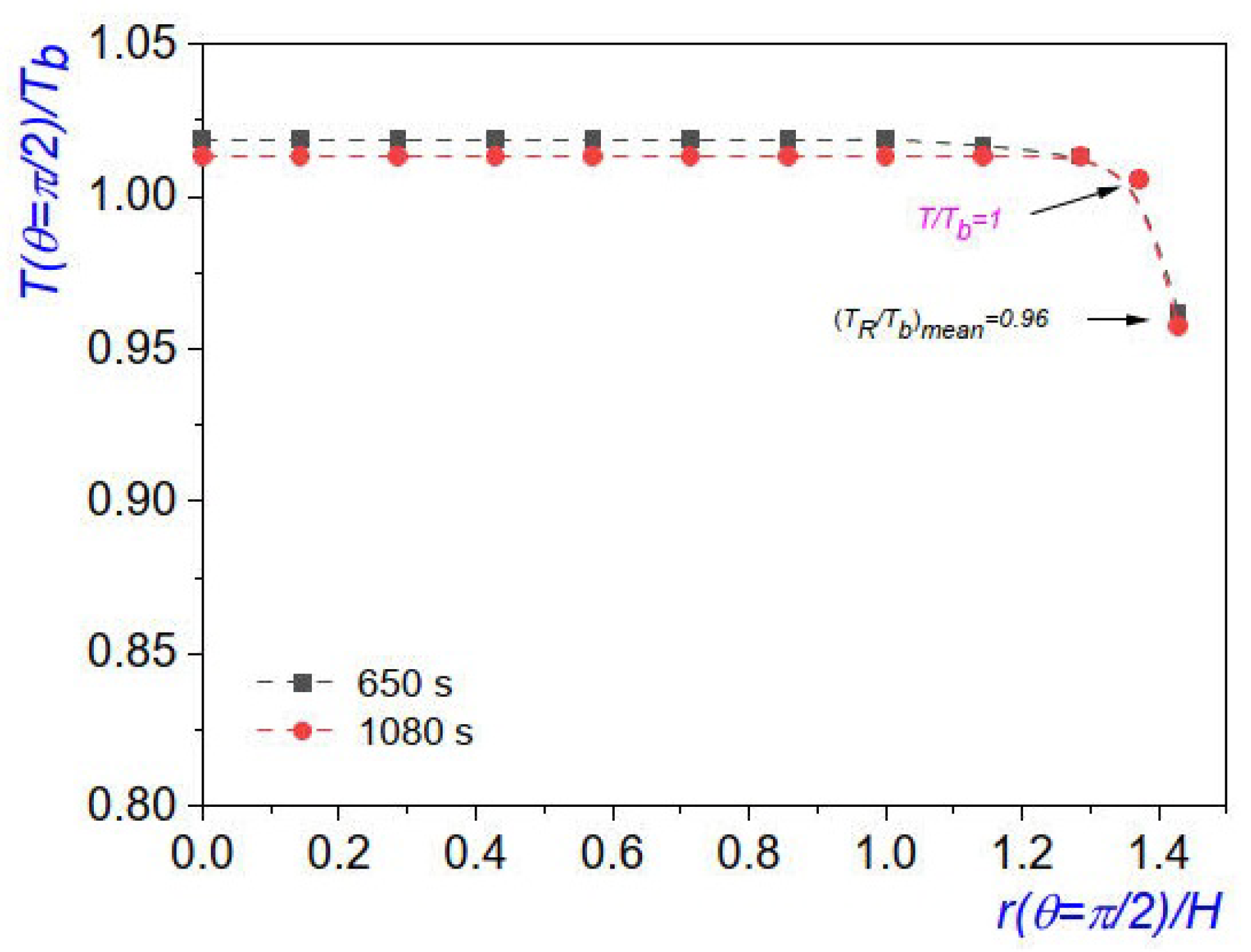
3.3. Transient Steam Flow in a Circular Steam Chamber
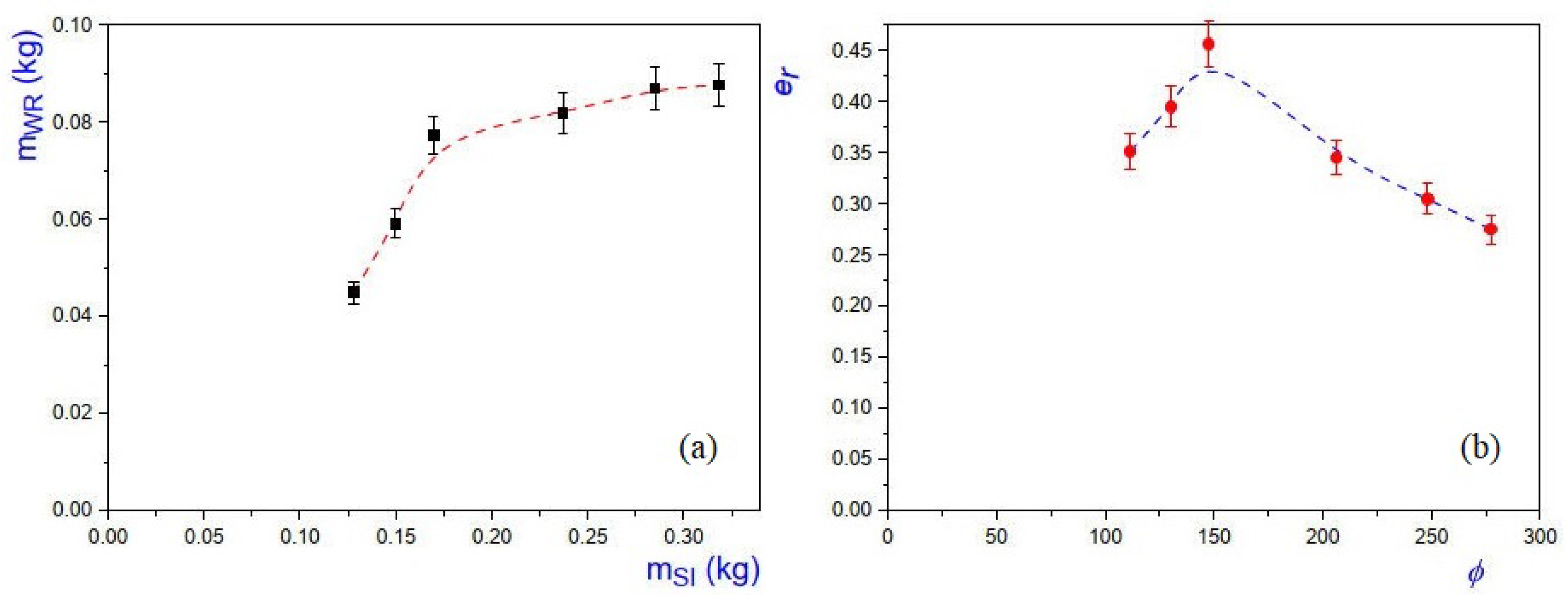
4. Optimal Injected Mass Flow Rates in the Steam Chambers
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Butler, R.M.; Stephens, D.J. The gravity drainage of steam-heated heavy oil to parallel horizontal wells. J. Can. Pet. Technol. 1981, 22, 90–96. [Google Scholar] [CrossRef]
- Butler, R.M. A new approach to the modelling of steam-assisted gravity drainage. J. Can. Pet. Technol. 1985, 24, 42–51. [Google Scholar] [CrossRef]
- Chung, K.H.; Butler, R.M. Geometrical effect of steam injection on the formation of emulsions in the steam-assisted gravity drainage process. J. Can. Pet. Technol. 1988, 27, 36–42. [Google Scholar] [CrossRef]
- Butler, R.M. Thermal Recovery of Oil and Bitumen; Prentice Hall: Hoboken, NJ, USA, 1991. [Google Scholar]
- Hein, F.J. Geology of bitumen and heavy oil: An overview. J. Pet. Sci. Eng. 2017, 154, 551–563. [Google Scholar] [CrossRef]
- Canadian Association of Petroleum Producers (CAPP). Upstream Dialogue. The Facts on: Oil Sands; Canadian Association of Petroleum Producers: Calgary, AB, Canadia, 2009; pp. 1–21. [Google Scholar]
- Voskov, D.; Zaydullin, R.; Lucia, A. Heavy oil recovery efficiency using SAGD, SAGD with propane co-injection and STRIP-SAG. Comp. Chem. Engn. 2016, 88, 115–125. [Google Scholar] [CrossRef]
- Heins, B.; Xiao, X.; Deng-chao, Y. New technology for heavy oil exploitation wastewater reused as boiler feedwater. Petrol. Explor. Dev. 2008, 35, 113–117. [Google Scholar]
- Guirgis, A.; Gay-de-Montella, R.; Faiz, R. Treatment of produced water streams in SAGD processes using tubular ceramic membranes. Desalination 2015, 358, 27–32. [Google Scholar] [CrossRef]
- Higuera, F.J.; Medina, A. A simple model of the flow in the steam chamber in SAGD oil recovery. In Supercomputing. ISUM 2019. Communications in Computer and Information Science; Torres, M., Klapp, J., Eds.; Springer: Cham, Switzerland, 2019; Volume 1151. [Google Scholar]
- Bublik, S.; Semin, M. Numerical simulation of phase transitions in porous media with three-phase flows considering steam injection into the oil reservoir. Computation 2022, 10, 205. [Google Scholar] [CrossRef]
- Fernandez, B.; Ehlig-Economides, C.A.; Economides, M.J. Multilevel Injector/Producer Wells in Thick Heavy Crude Reservoirs. In Proceedings of the 1999 SPE Latin American and Caribbean Petroleum Engineering Conference, Caracas, Venezuela, 21–23 April 1999. Paper SPE 53950. [Google Scholar]
- Siavashi, M.; Garusi, H.; Derakhshan, S. Numerical simulation and optimization of steam-assisted gravity drainage with temperature, rate, and well distance control using an efficient hybrid optimization technique. Numer. Heat Transf. Part A Appl. 2017, 72, 721–744. [Google Scholar] [CrossRef]
- Tao, L.; Xu, L.; Yuan, X.; Shi, W.; Zhang, N.; Li, S.; Si, S.; Ding, Y.; Bai, J.; Zhu, Q.; et al. Visualization experimental study on well spacing optimization of SAGD with a combination of vertical and horizontal wells. ACS Omega 2021, 6, 30050–30060. [Google Scholar] [CrossRef] [PubMed]
- Tao, L.; Yuan, X.; Cheng, H.; Li, B.; Huang, S.; Zhang, N. Experimental study on well placement optimization for steam-assisted gravity drainage to enhance recovery of thin layer oil sand reservoirs. Geofluids 2021, 2021, 9954127. [Google Scholar] [CrossRef]
- Martínez-Gómez, J.E.; Medina, A.; Higuera, F.J.; Vargas, C.A. Experiments on water gravity drainage due to steam injection into elliptical steam chambers. Fluids 2022, 7, 206. [Google Scholar] [CrossRef]
- Butler, R.M. Steam-Assisted Gravity Drainage: Concept, development, performance and future. J. Can. Pet. Technol. 1994, 33, 44–50. [Google Scholar] [CrossRef]
- Gao, Y.; Fan, T.; Gao, J.; Li, H.; Dong, H.; Ma, S.; Yue, Q. Monitoring of steam chamber in steam-assisted gravity drainage based on the temperature sensitivity of oil sand. Petrol. Explor. Dev. 2021, 48, 1411–1419. [Google Scholar] [CrossRef]
- Xu, J.; Chen, Z.; Cao, J.; Li, R. Numerical Study of the Effects of Lean Zones on SAGD Performance in Periodically Heterogeneous Media. In Proceedings of the SPE Heavy Oil Conference-Canada, Calgary, AB, Canada, 10–12 June 2014. Paper Number: SPE-170138-MS. [Google Scholar]
- Xu, J.; Chen, Z.; Dong, X.; Zhou, W. Effects of Lean Zones on Steam-Assisted Gravity Drainage Performance. Energies 2017, 10, 471. [Google Scholar] [CrossRef]
- Sheikha, A.; Sheikha, H. Top lean zone and cardinal parameters affecting SAGD. J. Petr. Sci. Eng. 2021, 201, 108437. [Google Scholar] [CrossRef]
- Purkayastha, S.N.; Gates, I.D.; Trifkovic, M. Real-time multivariable model predictive control for steam-assisted gravity drainage. AIChE J. 2018, 64, 3034–3041. [Google Scholar] [CrossRef]
- Zhang, Q.; Kang, X.; Liu, H.; Dong, X.; Wang, J. Breccia interlayer effects on steam-assisted gravity drainage performance: Experimental and numerical study. J. Petrol. Explor. Prod. Technol. 2022, 12, 613–629. [Google Scholar] [CrossRef]
- Fattahpour, V.; Mahmoudi, M.; Roostaei, M.; Wang, C.; Kotb, O.; Nouri, A.; Sutton, C.; Fermaniuk, B. Sand control testing for steam injection wells. In Proceedings of the SPE Canada Heavy Oil Technical Conference, Calgary, AB, Canada, 13 March 2018. Paper SPE-189766-MS. [Google Scholar]
- Chun, M.-H.; Kim, Y.-S.; Park, J.-W. An investigation of direct condensation of steam jet in subcooled water. Int. Comm. Heat Mass Transf. 1996, 23, 947–958. [Google Scholar] [CrossRef]
- Kim, H.Y.; Bae, Y.Y.; Song, C.H.; Park, J.K.; Choi, S.M. Experimental study on stable steam condensation in a quenching tank. Int. J. Energy Res. 2001, 25, 239–252. [Google Scholar] [CrossRef]
- Miller, S. Methods for computing the boiling temperature of water at varying pressures. Bull. Am. Meteorol. Soc. 2017, 98, 1485–1491. [Google Scholar] [CrossRef]
- Medina, A.; Higuera, F.J.; Pliego, M.; Gomez, G. Temperature profiles due to continuous hot water injection into homogeneous fluid-saturated porous media through a line source. Rev. Mex. Fís. 2021, 67, 365–368. [Google Scholar] [CrossRef]
- Incropera, F.P.; DeWitt, D.P.; Bergman, T.L.; Lavine, A.S. Fundamentals of Heat and Mass Transfer; John Wiley & Sons: Hoboken, NJ, USA, 2006. [Google Scholar]
- Banerjee, M.; Hascakir, B. Design of flow control devices in steam-assisted gravity drainage (SAGD) completion. J. Petrol. Explor. Prod. Technol. 2018, 8, 785–797. [Google Scholar] [CrossRef]
- Properties of Saturated Steam-SI Units. Available online: https://www.engineeringtoolbox.com/saturated-steam-properties-d_101.html (accessed on 23 June 2023).
- Somlyai-Sipos, L.; Baumli, P. Wettability of Metals by Water. Metals 2022, 12, 1274. [Google Scholar] [CrossRef]
- Zhao, C.; Kern, V.R.; Carlson, A.; Lee, T. Engulfment of a drop on solids coated by thin and thick fluid films. J. Fluid Mech. 2023, 958, A41. [Google Scholar] [CrossRef]
- Velayati, A.; Nouri, A. Emulsification and emulsion flow in thermal recovery operations with a focus on SAGD operations: A critical review. Fuel 2020, 267, 117141. [Google Scholar] [CrossRef]
- Kokal, S.L. Crude oil emulsions: A state-of-the-art review. SPE Prod. Facil. 2005, 20, 5–13. [Google Scholar] [CrossRef]
- Gotawala, D.R.; Gates, I.D. A basis for automated control of steam trap subcool in SAGD. SPE J. 2012, 17, 680–686. [Google Scholar] [CrossRef]
- Liang, G.; Liu, S.; Shen, P.; Liu, Y.; Luo, Y. A new optimization method for steam-liquid level intelligent control model in oil sands steam-assisted gravity drainage (SAGD) process. Petr. Explor. Dev. 2016, 43, 301–307. [Google Scholar] [CrossRef]



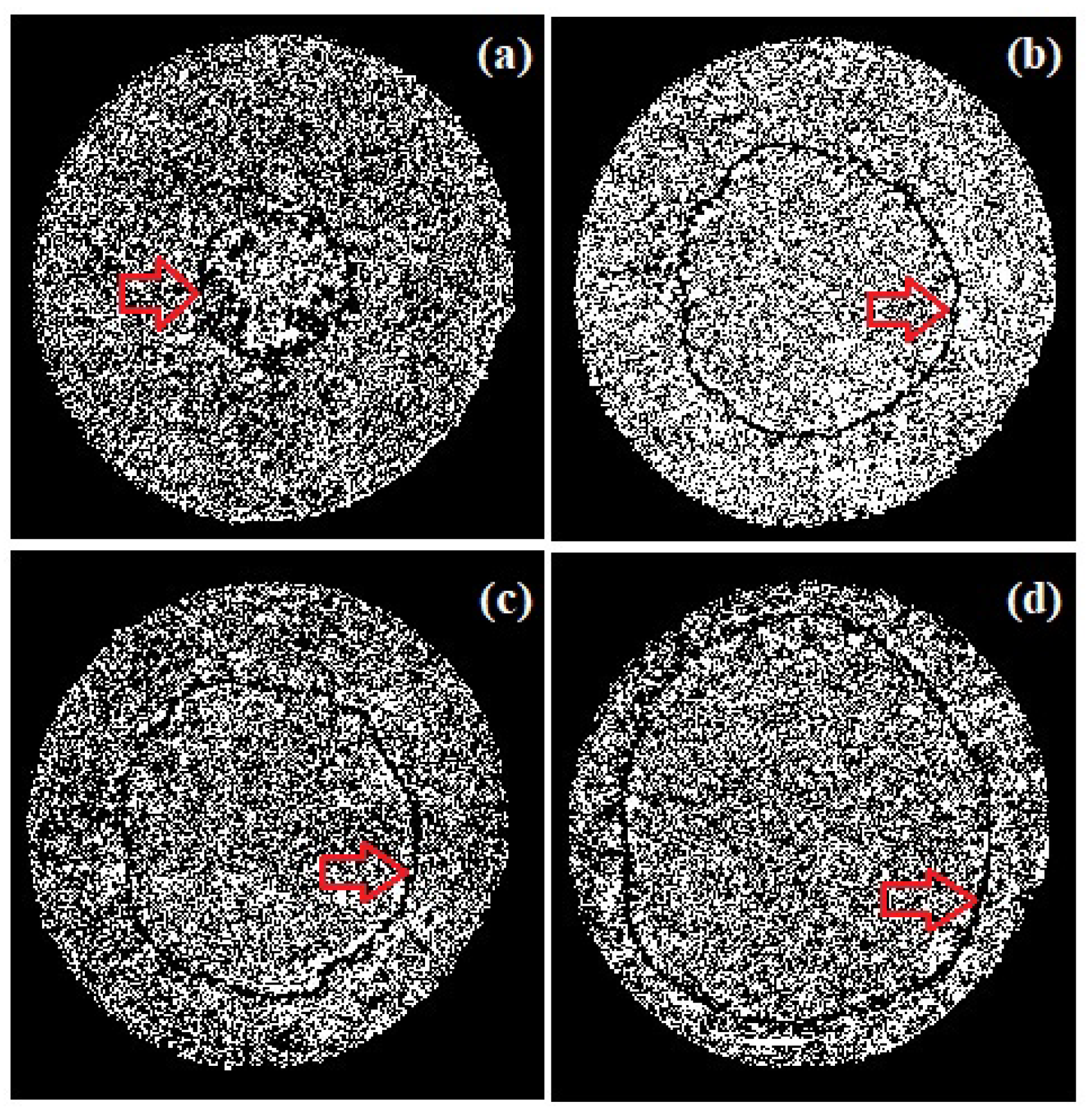


| Shape of Chamber Edge | a (m) | b (m) |
|---|---|---|
| Ellipse (horizontal semi-major axis) | 0.157 | 0.105 |
| Ellipse (vertical semi-major axis) | 0.105 | 0.157 |
| Circumference | 0.10 | 0.10 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Medina, A.; García, D.B.; López Villa, A.; Castillo-Morales, B.; Polupan, G. Experiments on Steam Injection into Preformed Steam Chambers of Various Shapes for Maximum Condensate Recovery. Fluids 2023, 8, 202. https://doi.org/10.3390/fluids8070202
Medina A, García DB, López Villa A, Castillo-Morales B, Polupan G. Experiments on Steam Injection into Preformed Steam Chambers of Various Shapes for Maximum Condensate Recovery. Fluids. 2023; 8(7):202. https://doi.org/10.3390/fluids8070202
Chicago/Turabian StyleMedina, Abraham, Diego Benjamin García, Abel López Villa, Benjamin Castillo-Morales, and Georgiy Polupan. 2023. "Experiments on Steam Injection into Preformed Steam Chambers of Various Shapes for Maximum Condensate Recovery" Fluids 8, no. 7: 202. https://doi.org/10.3390/fluids8070202
APA StyleMedina, A., García, D. B., López Villa, A., Castillo-Morales, B., & Polupan, G. (2023). Experiments on Steam Injection into Preformed Steam Chambers of Various Shapes for Maximum Condensate Recovery. Fluids, 8(7), 202. https://doi.org/10.3390/fluids8070202






