Prediction of Flow Properties of Porous Triply Periodic Minimal Surface (TPMS) Structures
Abstract
:1. Introduction
2. Design of Triply Periodic Minimal Surface (TPMS) Structures

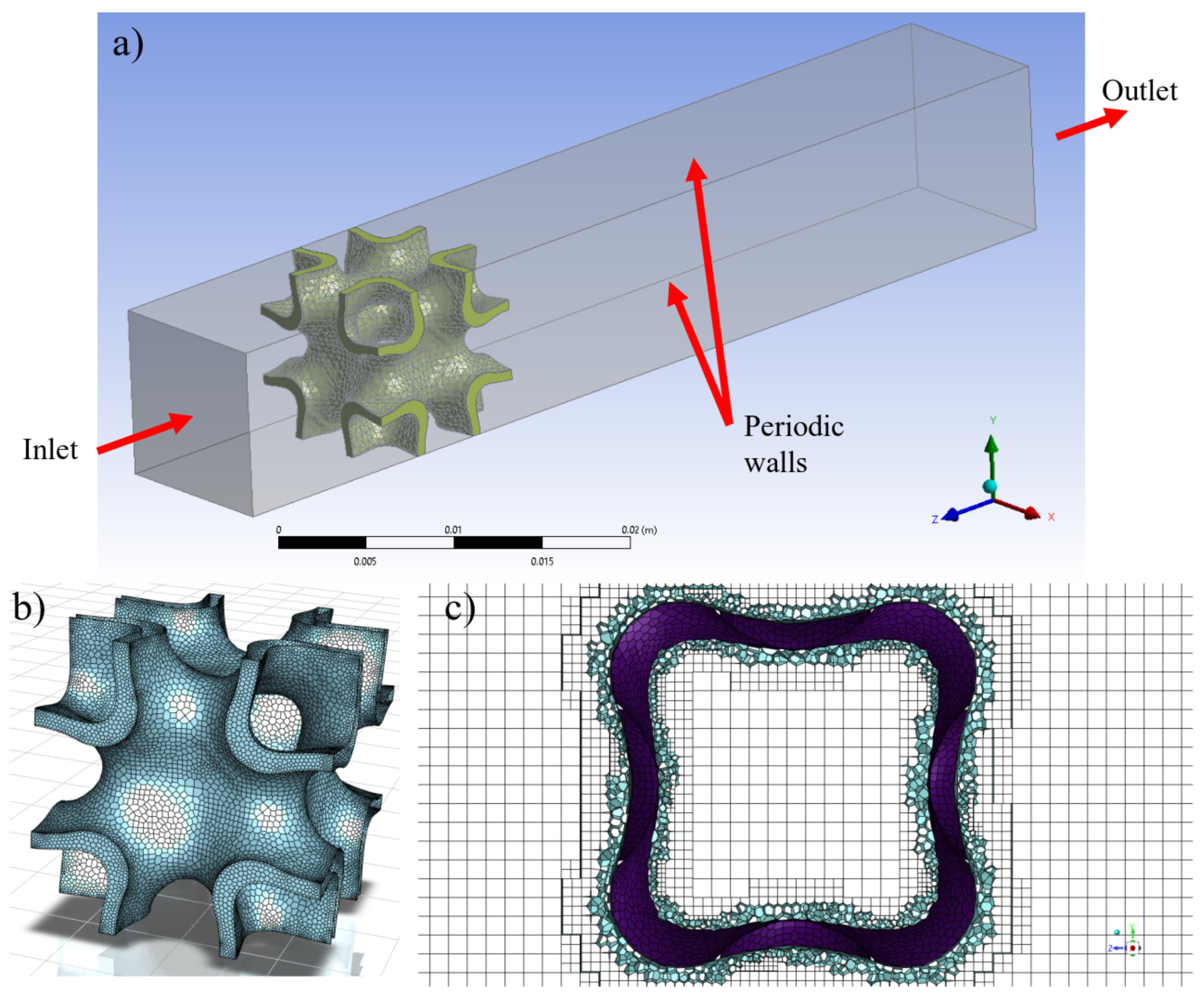
3. Mathematical Model and Numerical Implementation
4. Results and Discussion
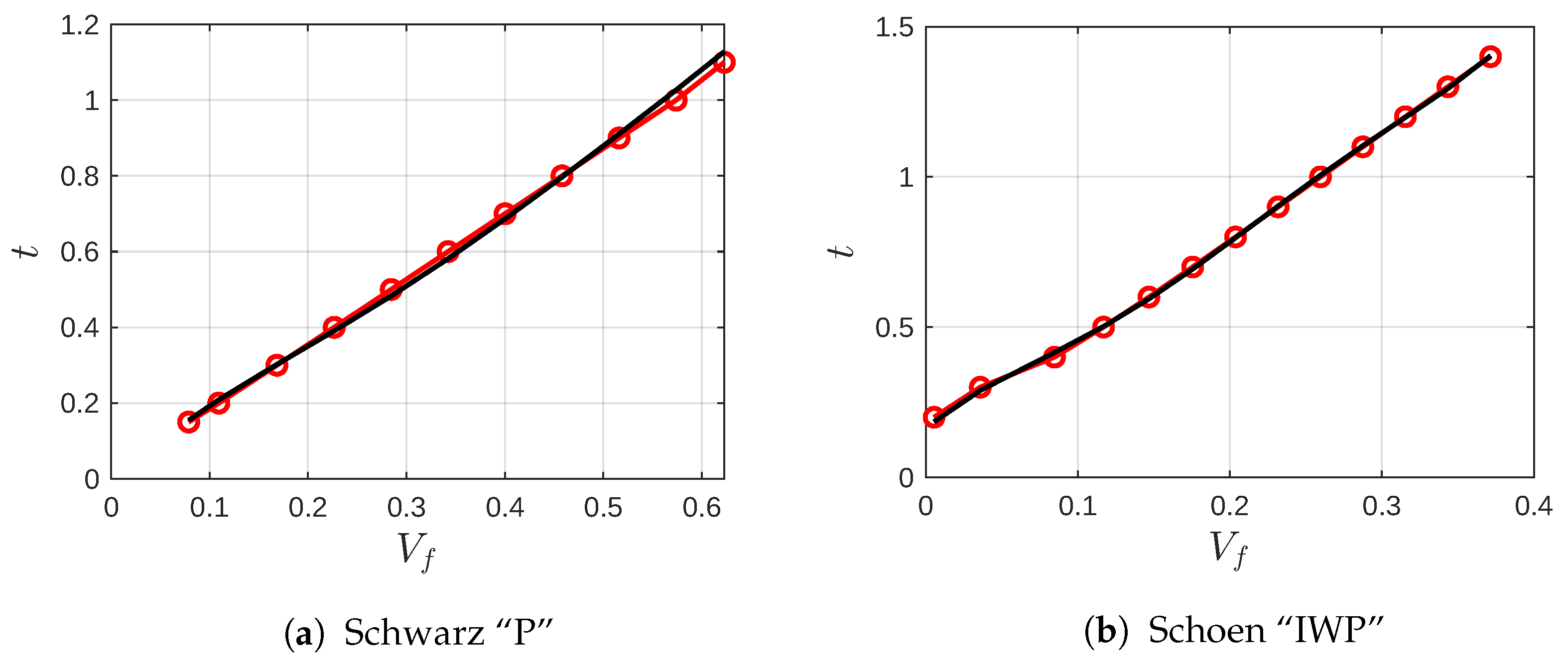
4.1. TPMS Structures Design and Porosity Characterization
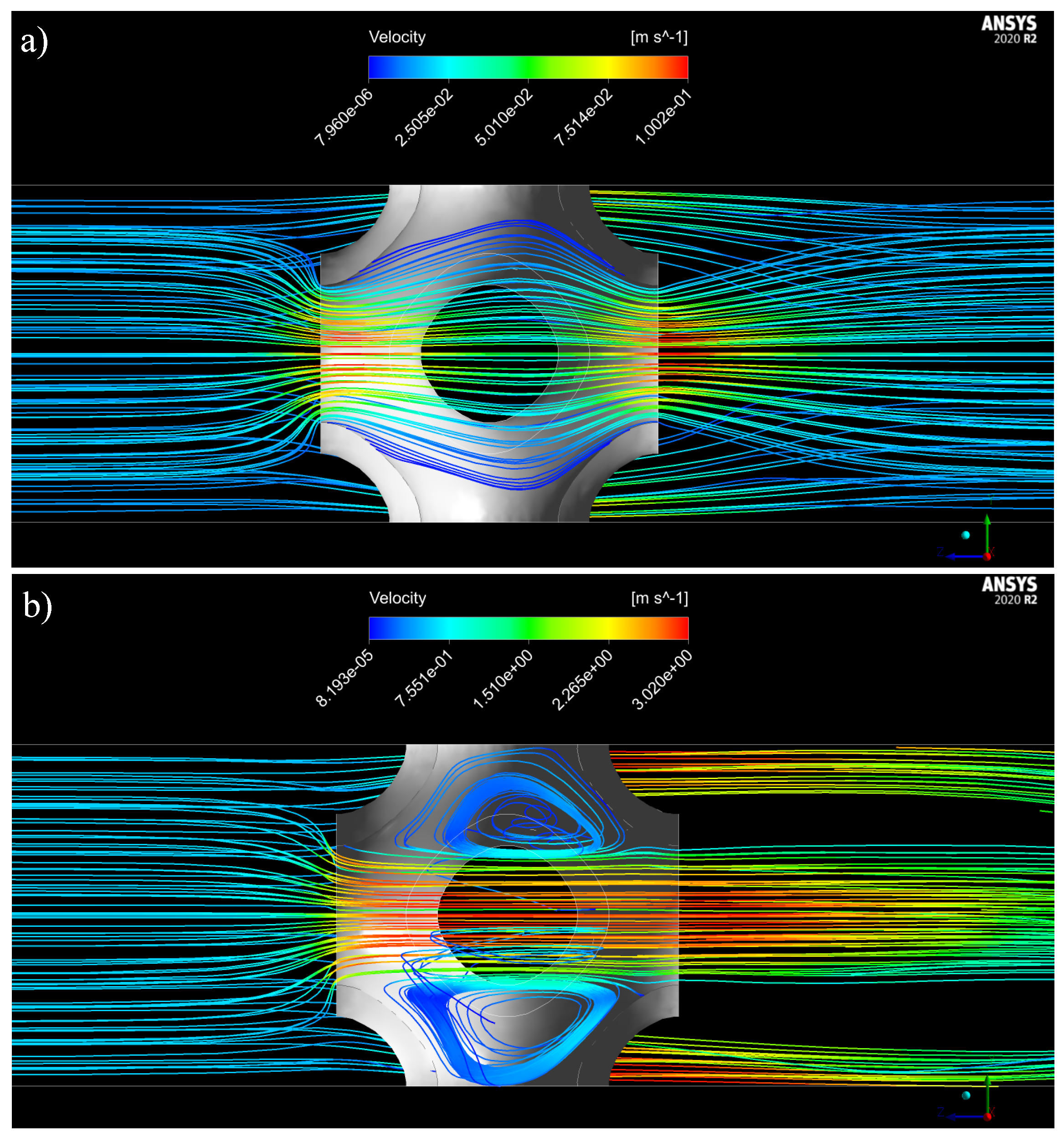
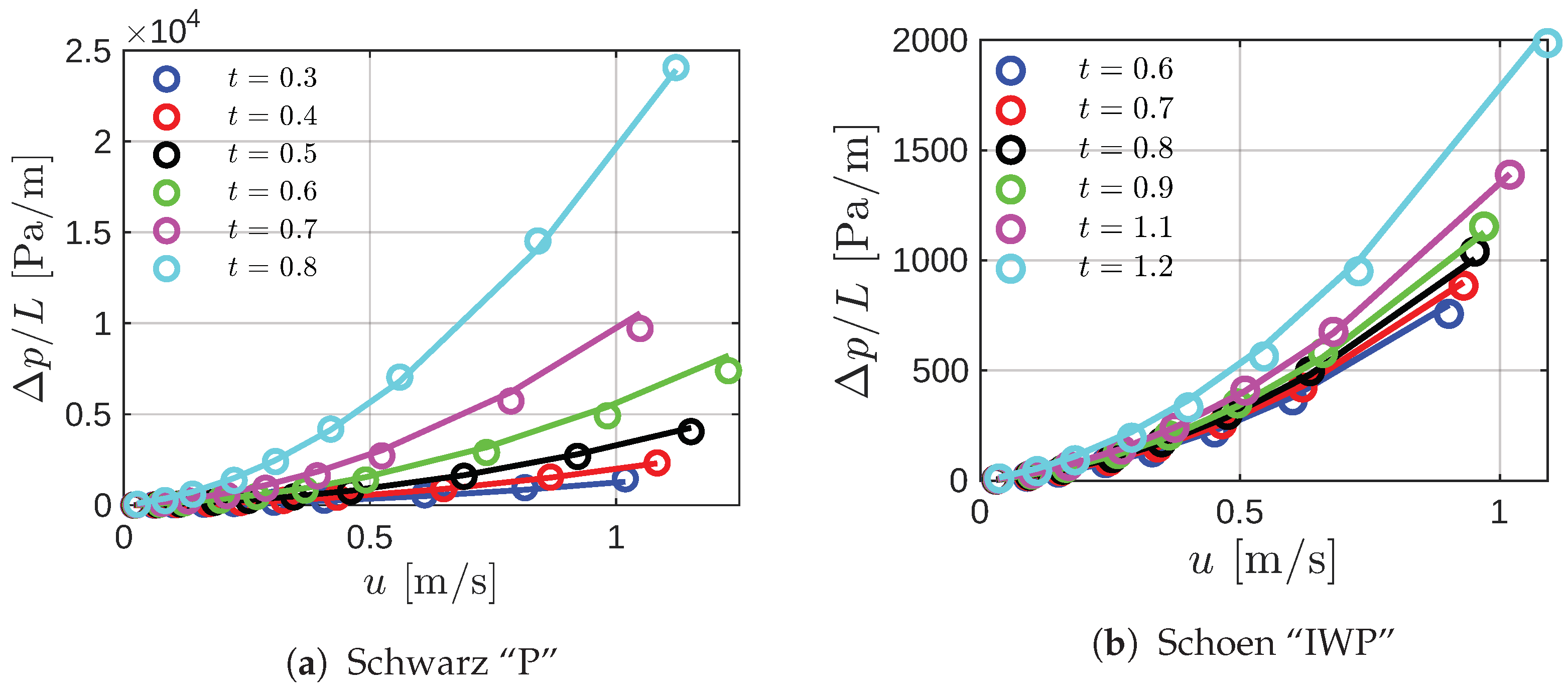
4.2. Mean Flow Characteristics
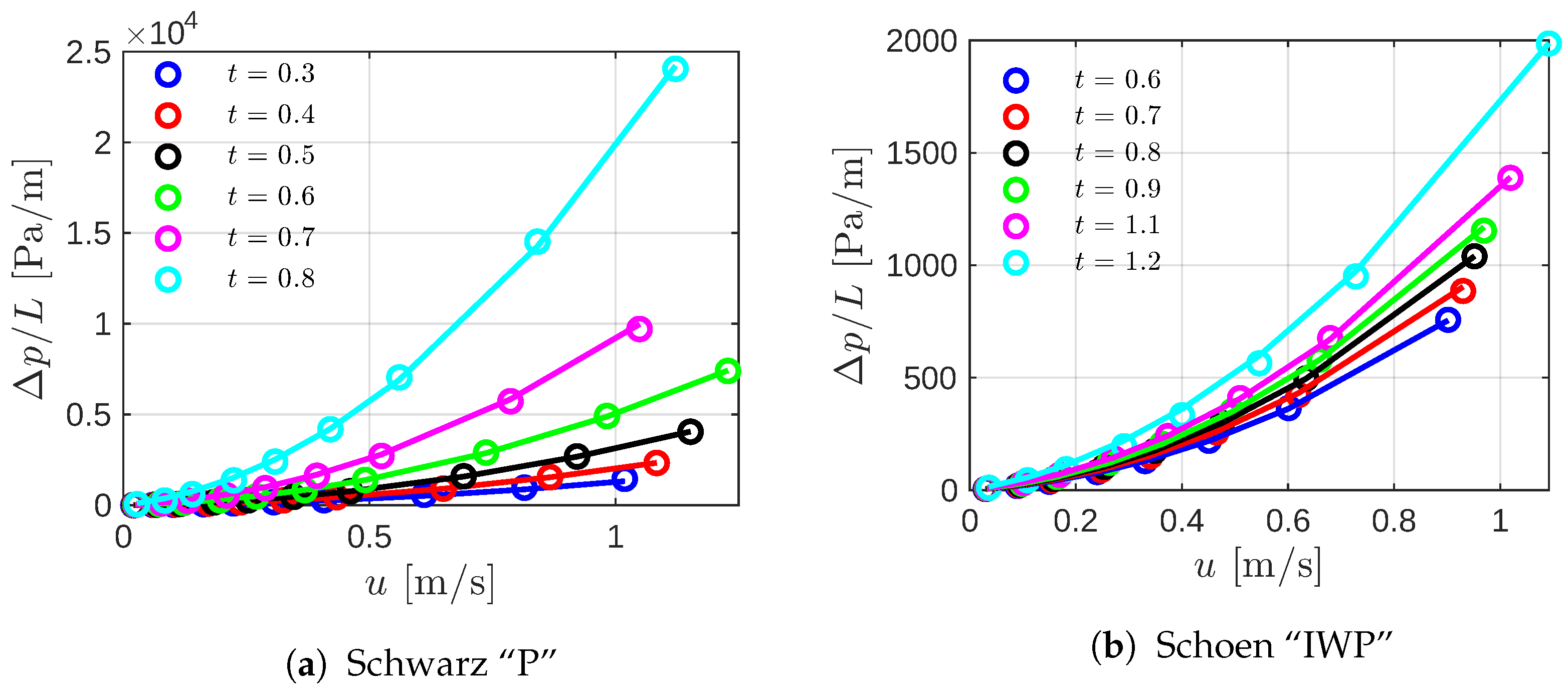
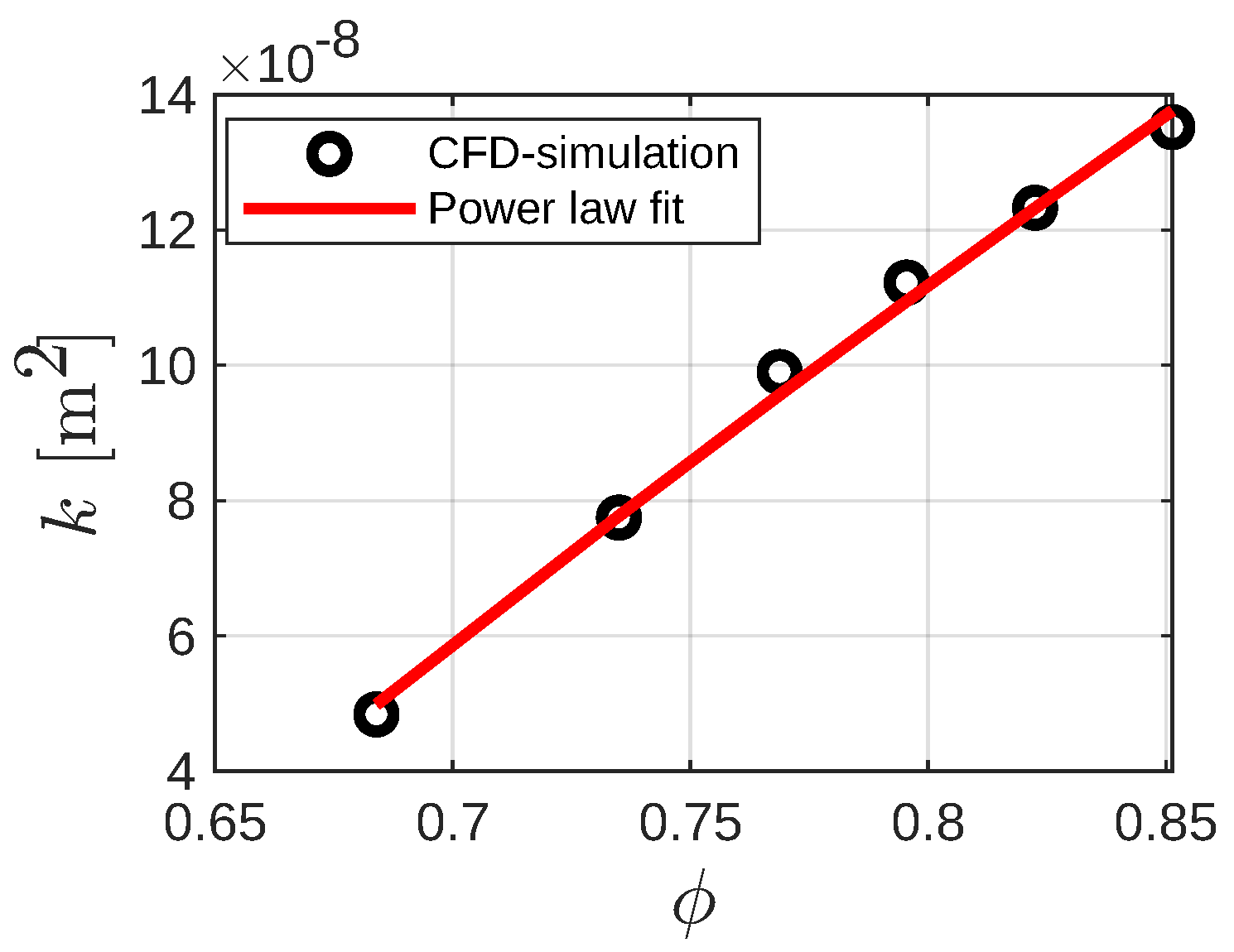
4.3. Modeling the TPMS Structures as Porous Media
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| AM | Additive Manufacturing |
| CFD | Computational Fluid Dynamics |
| DF | Darcy–Forchheimer |
| FVM | Finite Volume Method |
| SIMPLE | Semi-Implicit Method for Pressure-Linked Equations |
| TPMS | Triply Periodic Minimal Surface |
| Nomenclature | |
| a | Unit cell size (m) |
| Forchheimer coefficient | |
| k | Permeability (m2) |
| Dynamic viscosity (kg m−1 s−1) | |
| Kinematic viscosity, (m2 s−1) | |
| p | Pressure field (N m−2) |
| TPMS porosity, | |
| Reynolds number, | |
| Mass density (kg m−3) | |
| t | Isovalue of the TPMS |
| Vector velocity field (m s−1) | |
| U | Inlet flow velocity (m s−1) |
| TPMS Solid volume fraction | |
| w | Width of the channel (m) |
References
- Forchheimer, P. Wasserbewegung durch boden. Z. Vereins Dtsch. Ingenieure 1901, 45, 1782–1788. [Google Scholar]
- Tosco, T.; Marchisio, D.L.; Lince, F.; Sethi, R. Extension of the Darcy-Forchheimer Law for Shear-Thinning Fluids and Validation via Pore-Scale Flow Simulations. Transp. Porous Media 2013, 96, 1–20. [Google Scholar] [CrossRef]
- Hayat, T.; Haider, F.; Muhammad, T.; Alsaedi, A. Darcy-Forcheimer flow with Cattaneo-Christov heat flux and homogeneous-hetegoreneous reactions. PLoS ONE 2017, 12, e0174938. [Google Scholar] [CrossRef] [PubMed]
- Hayat, T.; Haider, F.; Alsaedi, A. Darcy-Forchheimer flow with nonlinear mixed convection. Appl. Math. Mech. 2020, 41, 1685–1696. [Google Scholar] [CrossRef]
- Majdeed, A.; Zeeshan, A.; Noori, F.M. Numerical study of Darcy-Forchheimer model with activation energy subject to chemically reactive species and momentum slip of order two. AIP Adv. 2019, 6, 045035. [Google Scholar] [CrossRef]
- Abbas, A.; Jeelani, M.B.; Alharthi, N.H. Magnetohydrodynamic Effects on Third-Grade Fluid Flow and Heat Transfer with Darcy-Forchheimer Law over and Inclined Exponentially Stretching Sheet Embedded in a Porous Medium. Magnetochemistry 2022, 8, 61. [Google Scholar] [CrossRef]
- Khan, M.I.; Hayat, T.; Alsaedi, A. Numerical analysis for Darcy-Forchheimer flow in presence of homogeneous-heterogeneous reactions. Results Phys. 2017, 7, 2644–2650. [Google Scholar] [CrossRef]
- Hayat, T.; Haider, F.; Muhammad, T.; Alsaedi, A. An optimal study for Darcy-Forchheimer flow with generalized Fourier’s and Ficl’s laws. Results Phys. 2017, 7, 2878–2885. [Google Scholar] [CrossRef]
- Hayat, T.; Haider, F.; Muhammad, T.; Alsaedi, A. On Darcy-Forchheimer flow of viscoelastic nanofluids: A comparative study. J. Mol. Liq. 2017, 233, 278–287. [Google Scholar] [CrossRef]
- Rasool, G.; Khan, W.A.; Bilal, S.M.; Khan, I. MHD Squeezed Darcy-Forchheimer nanofluid flow between two h-distance apart horizontal plates. Open Phys. 2020, 18, 1100–1107. [Google Scholar] [CrossRef]
- Tlili, I.; Shahmir, N.; Ramzan, M.; Kadry, S.; Kim, J.Y.; Nam, Y.; Lu, D. A novel model to analyze Darcy Forchheimer nanofluid flow in a permeable medium with Entropy generation analysis. J. Taibah Univ. Sci. 2020, 14, 916–930. [Google Scholar] [CrossRef]
- Ullah, M.Z.; Serra-Capizzano, S.; Baleanu, D. A Numerical Simulation for Darcy-Forchheimer Flow of Nanofluid by a Rotating Disk With Partial Slip Effects. Front. Phys. 2020, 7, 219. [Google Scholar] [CrossRef]
- Rao, D.P.C.; Thiagarajan, S.; Kumar, V.S. Heat Transfer in Darcy-Forchheimer Flow of Tangent Hyperbolic Fluid Over and Inclined Plate with Joule Heating. J. Appl. Math. Comput. Mech. 2021, 20, 31–40. [Google Scholar] [CrossRef]
- Grillo, A.; Carfagna, M.; Federico, S. The Darcy-Forchheimer Law for Modelling Fluid Flow in Biological Tissues. Theor. Appl. Mech. 2014, 41, 283–322. [Google Scholar] [CrossRef]
- Grillo, A.; Carfagna, M.; Federico, S. Non-Darcian flow in a fibre-reinforced biological tissues. Meccanica 2017, 52, 3299–3320. [Google Scholar] [CrossRef]
- Takhanov, D. Forchheimer Model for Non-Darcy Flow in Porous Media and Fractures. Master’s Thesis, Imperial College London, London, UK, 2011. [Google Scholar]
- Plocher, J.; Panesar, A. Review on design and structural optimisation in additive manufacturing: Towards next-generation lightweight structures. Mater. Des. 2019, 183, 108164. [Google Scholar] [CrossRef]
- Arredondo-Soto, M.; Cuan-Urquizo, E.; Gómez-Espinosa, A. A Review on Tailoring Stiffness in Compliant Systems, via Removing Material: Cellular Materials and Topology Optimization. Appl. Sci. 2021, 11, 3538. [Google Scholar] [CrossRef]
- Schmelzle, J.; Kline, E.V.; Dickman, C.J.; Reutzel, E.W.; Jones, G.; Simpson, T.W. (Re)Designing for Part Consolidation: Understanding the Challenges of Metal Additive Manufacturing. J. Mech. Des. 2015, 137, 111404. [Google Scholar] [CrossRef]
- Yang, S.; Tang, Y.; Zhao, Y.F. A new part consolidation method to embrace the design freedom of additive manufacturing. J. Manuf. Process. 2015, 20, 444–449. [Google Scholar] [CrossRef]
- Attarzadeh, R.; Rovira, M.; Duwig, C. Design analysis of the “Schwartz D” based heat exchanger: A numerical study. Int. J. Heat Mass Transf. 2021, 177, 121415. [Google Scholar] [CrossRef]
- Attarzadeh, R.; Attarzadeh-Niaki, S.H.; Duwig, C. Multi-objective optimization of TPMS-based heat exchangers for low-temperature waste heat recovery. Appl. Therm. Eng. 2022, 212, 118448. [Google Scholar] [CrossRef]
- Yeranee, K.; Rao, Y. A Review of Recent Investigations on Flow and Heat Transfer Enhancement in Cooling Channels Embedded with Triply Periodic Minimal Surfaces (TPMS). Energies 2022, 15, 8994. [Google Scholar] [CrossRef]
- Coimbra, J.C.; Martins, M.A.; Oliveira, P.S.; Minim, L.A. The potential use of a gyroid structure to represent monolithic matrices for bioseparation purposes: Fluid dynamics and mass transfer analysis via CFD. Sep. Purif. Technol. 2021, 254, 117594. [Google Scholar] [CrossRef]
- Shi, J.; Wei, F.; Chouraki, B.; Sun, X.; Wei, J.; Zhu, L. Study on Performance Simulation of Vascular-like Flow Channel Model Based on TPMS Structure. Biomimetics 2023, 8, 69. [Google Scholar] [CrossRef] [PubMed]
- Rathore, S.S.; Mehta, B.; Kumar, P.; Asfer, M. Flow Characterization in Triply Periodic Minimal Surface (TPMS)-Based Porous Geometries: Part I–Hydrodynamics. Transp. Porous Media 2023, 146, 669–701. [Google Scholar] [CrossRef]
- Ali, D.; Ozalp, M.; Blanquer, S.B.; Onel, S. Permeability and fluid flow-induced wall shear stress in bone scaffolds with TPMS and lattice architectures: A CFD analysis. Eur. J. Mech. B/Fluids 2020, 79, 376–385. [Google Scholar] [CrossRef]
- Zeng, C.; Wang, W. Modeling method for variable and isotropic permeability design of porous material based on TPMS lattices. Tribol. Int. 2022, 176, 107913. [Google Scholar] [CrossRef]
- Zou, S.; Mu, Y.; Pan, B.; Li, G.; Shao, L.; Du, J.; Jin, Y. Mechanical and biological properties of enhanced porous scaffolds based on triply periodic minimal surfaces. Mater. Des. 2022, 219, 110803. [Google Scholar] [CrossRef]
- Al-Ketan, O.; Ali, M.; Khalil, M.; Rowshan, R.; Khan, K.A.; Abu Al-Rub, R.K. Forced Convection Computational Fluid Dynamics Analysis of Architected and Three-Dimensional Printable Heat Sinks Based on Triply Periodic Minimal Surfaces. J. Therm. Sci. Eng. Appl. 2020, 13, 021010. [Google Scholar] [CrossRef]
- Passos, A.G.P. Laminar Flow and Heat Transfer in Triply Periodic MINIMAL Surfaces. Master’s Thesis, Instituto Superior Técnico, Universidade de Lisboa, Lisboa, Portugal, 2019. [Google Scholar]
- Li, W.; Yu, G.; Yu, Z. Bioinspired heat exchangers based on triply periodic minimal surfaces for supercritical CO2 cycles. Appl. Therm. Eng. 2020, 179, 115686. [Google Scholar] [CrossRef]
- Yan, C.; Hao, L.; Yang, L.; Hussein, A.Y.; Young, P.G.; Li, Z.; Li, Y. Triply Periodic Minimal Surface Lattices Additively Manufactured by Selective Laser Melting, 1st ed.; 3D Printing Technology Series; Academic Press: Cambridge, MA, USA, 2021. [Google Scholar] [CrossRef]
- Jin, M.; Feng, Q.; Fan, X.; Luo, Z.; Tang, Q.; Song, J.; Ma, S.; Nie, Y.; Jin, P.; Zhao, M. Investigation on the mechanical properties of TPMS porous structures fabricated by laser powder bed fusion. J. Manuf. Process. 2022, 76, 559–574. [Google Scholar] [CrossRef]
- Wang, H.; Tan, D.; Liu, Z.; Yin, H.; Wen, G. On crashworthiness of novel porous structure based on composite TPMS structures. Eng. Struct. 2022, 252, 113640. [Google Scholar] [CrossRef]
- Sun, Q.; Sun, J.; Guo, K.; Wang, L. Compressive mechanical properties and energy absorption characteristics of SLM fabricated Ti6Al4V triply periodic minimal surface cellular structures. Mech. Mater. 2022, 166, 104241. [Google Scholar] [CrossRef]
- Qureshi, Z.A.; Elnajjar, E.; Al-Ketan, O.; Al-Rub, R.A.; Al-Omari, S.B. Heat transfer performance of a finned metal foam-phase change material (FMF-PCM) system incorporating triply periodic minimal surfaces (TPMS). Int. J. Heat Mass Transf. 2021, 170, 121001. [Google Scholar] [CrossRef]
- Oh, S.H.; Ha, J.W.; Park, K. Adaptive Conformal Cooling of Injection Molds Using Additively Manufactured TPMS Structures. Polymers 2022, 14, 181. [Google Scholar] [CrossRef] [PubMed]
- Savio, G.; Rosso, S.; Meneghello, R.; Concheri, G. Geomteric Modeling of Cellular Materials for Additive Manufacturing in Biomedical Field: A Review. Appl. Bionics Biomech. 2018, 2018, 14. [Google Scholar] [CrossRef] [PubMed]
- Dong, Z.; Zhao, X. Application of TPMS structure in bone regeneration. Eng. Regen. 2021, 2, 154–162. [Google Scholar] [CrossRef]
- nTopology. 2021. Next-Generation Engineering Design Software. Windows. nTop. Available online: https://www.ntop.com/ (accessed on 4 October 2023).
- Karakoç, A. RegionTPMS—Region based triply periodic minimal surfaces (TPMS) for 3-D printed multiphase bone scaffolds with exact porosity values. SoftwareX 2021, 16, 100835. [Google Scholar] [CrossRef]
- Al-Ketan, O.; Al-Rub, R.K.A. MSLattice: A free software for generating uniform and graded lattices based on triply periodic minimal surfaces. Mater. Des. Process. Commun. 2020, 3, e205. [Google Scholar] [CrossRef]
- Maskery, I.; Parry, L.A.; Padrão, D.; Hague, R.J.M.; Ashcroft, I.A. FLatt Pack: A research-focussed lattice design program. Addit. Manuf. 2022, 49, 102510. [Google Scholar] [CrossRef]
- Perez-Boerema, F.; Barzegari, M.; Geris, L. A flexible and easy-to-use open-source tool for designing functionally graded 3D porous structures. Virtual Phys. Prototyp. 2022, 3, 682–699. [Google Scholar] [CrossRef]
- Tenorio-Suárez, M.I.; Gómez-Ortega, A.; Canales, H.; Piedra, S.; Pérez-Barrera, J. MaSMaker: An open-source, portable software to create and integrate maze-like surfaces into arbitrary geometries. SoftwareX 2022, 19, 101203. [Google Scholar] [CrossRef]
- Autodesk. Meshmixer: Free Software for Making Awesome Stuff (Version 3.5). Windows. Autodesk, Inc. 2020. Available online: https://meshmixer.com/ (accessed on 4 October 2023).
- Riegel, J.; van Havre, W.M.Y. FreeCAD: Your Own 3D Parametric Modeler (Version 0.21.1).Windows . The FreeCAD Team, 2021. Available online: https://meshmixer.com/ (accessed on 4 October 2023).
- ANSYS, Inc. ANSYS Fluent User’s Guide, (Version 2020 R2).Windows. ANSYS, Inc., 2020. Available online: https://www.ansys.com/ (accessed on 4 October 2023).
- Community, B.O. Blender—A 3D Modelling and Rendering Package; Blender Foundation, Stichting Blender Foundation: Amsterdam, The Netherlands, 2023. [Google Scholar]
- Schulz, R.; Ray, N.; Zech, S.; Rupp, A.; Knabner, P. Beyond Kozeny–Carman: Predicting the Permeability in Porous Media. Transp. Porous Med. 2019, 130, 487–512. [Google Scholar] [CrossRef]
- Hommel, J.; Coltman, E.; Class, H. Porosity-Permeability Relations for Evolving Pore Space: A Review with a Focus on (Bio-)geochemically Altered Porous Media. Transp. Porous Med. 2018, 124, 589–629. [Google Scholar] [CrossRef]
- Mishra, A.; Korba, D.; Kaur, I.; Singh, P.; Li, L. Prediction and Validation of Flow Properties in Porous Lattice Structures. J. Fluids Eng. 2023, 145, 041402. [Google Scholar] [CrossRef]


| Grid | Cells | (Pa/m) |
|---|---|---|
| 1 | 102253 | 389.14 |
| 2 | 258222 | 405.34 |
| 3 | 345876 | 410.10 |
| 4 | 431847 | 409.45 |
| 5 | 760027 | 411.95 |
| TPMS | a | b | c | d | e | f | |
|---|---|---|---|---|---|---|---|
| Primitive | 17.03 | −34.93 | 26.9 | −8.89 | 2.83 | −0.025 | 0.99 |
| IWP | 746.2 | −744.3 | 260.2 | −35.49 | 4.56 | 0.16 | 0.98 |
| k | ||||
|---|---|---|---|---|
| TPMS | a | b | d | e |
| Primitive | 4.439 | 7.015 | 0.1865 | −2.806 |
| d | e | f | ||
| IWP | 1.005 | 0.4505 | −7.971 | ≈0.25 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Piedra, S.; Gómez-Ortega, A.; Pérez-Barrera, J. Prediction of Flow Properties of Porous Triply Periodic Minimal Surface (TPMS) Structures. Fluids 2023, 8, 312. https://doi.org/10.3390/fluids8120312
Piedra S, Gómez-Ortega A, Pérez-Barrera J. Prediction of Flow Properties of Porous Triply Periodic Minimal Surface (TPMS) Structures. Fluids. 2023; 8(12):312. https://doi.org/10.3390/fluids8120312
Chicago/Turabian StylePiedra, Saúl, Arturo Gómez-Ortega, and James Pérez-Barrera. 2023. "Prediction of Flow Properties of Porous Triply Periodic Minimal Surface (TPMS) Structures" Fluids 8, no. 12: 312. https://doi.org/10.3390/fluids8120312
APA StylePiedra, S., Gómez-Ortega, A., & Pérez-Barrera, J. (2023). Prediction of Flow Properties of Porous Triply Periodic Minimal Surface (TPMS) Structures. Fluids, 8(12), 312. https://doi.org/10.3390/fluids8120312








