Probability Distribution of Extreme Events in a Baroclinic Wave Laboratory Experiment
Abstract
1. Introduction
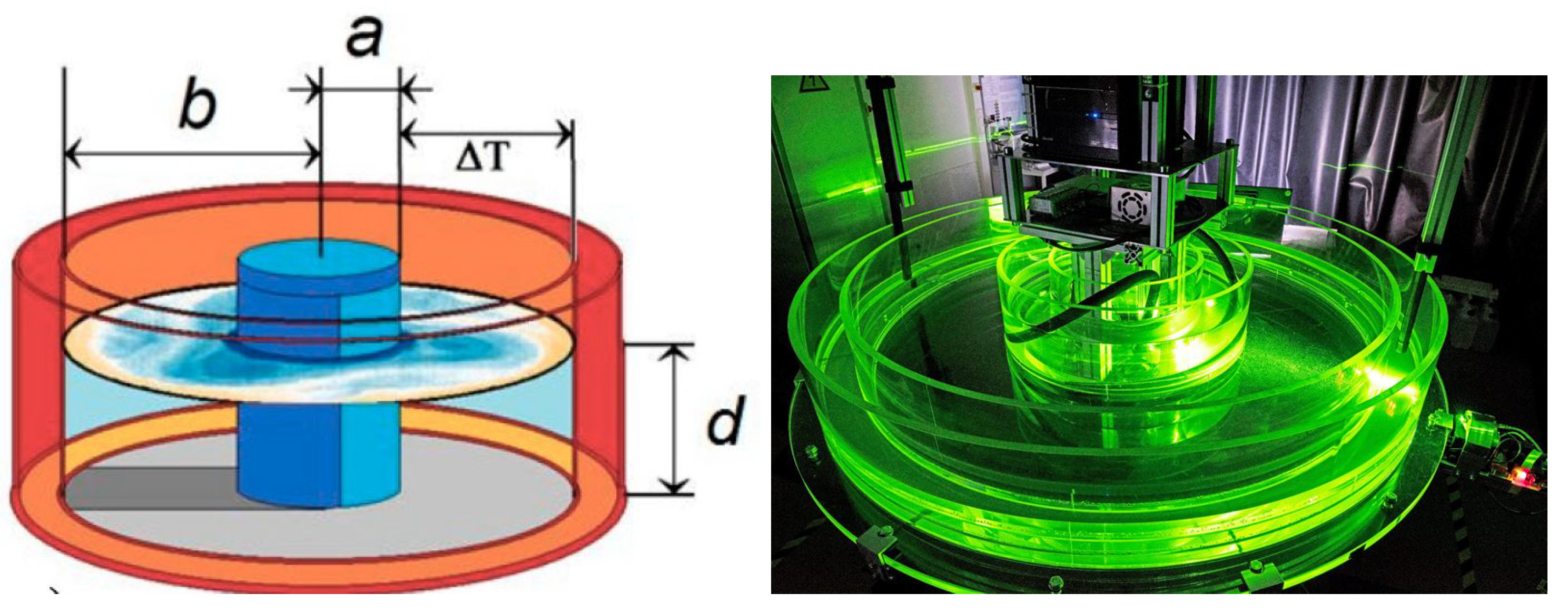
2. Experimental Set-Up
3. Data
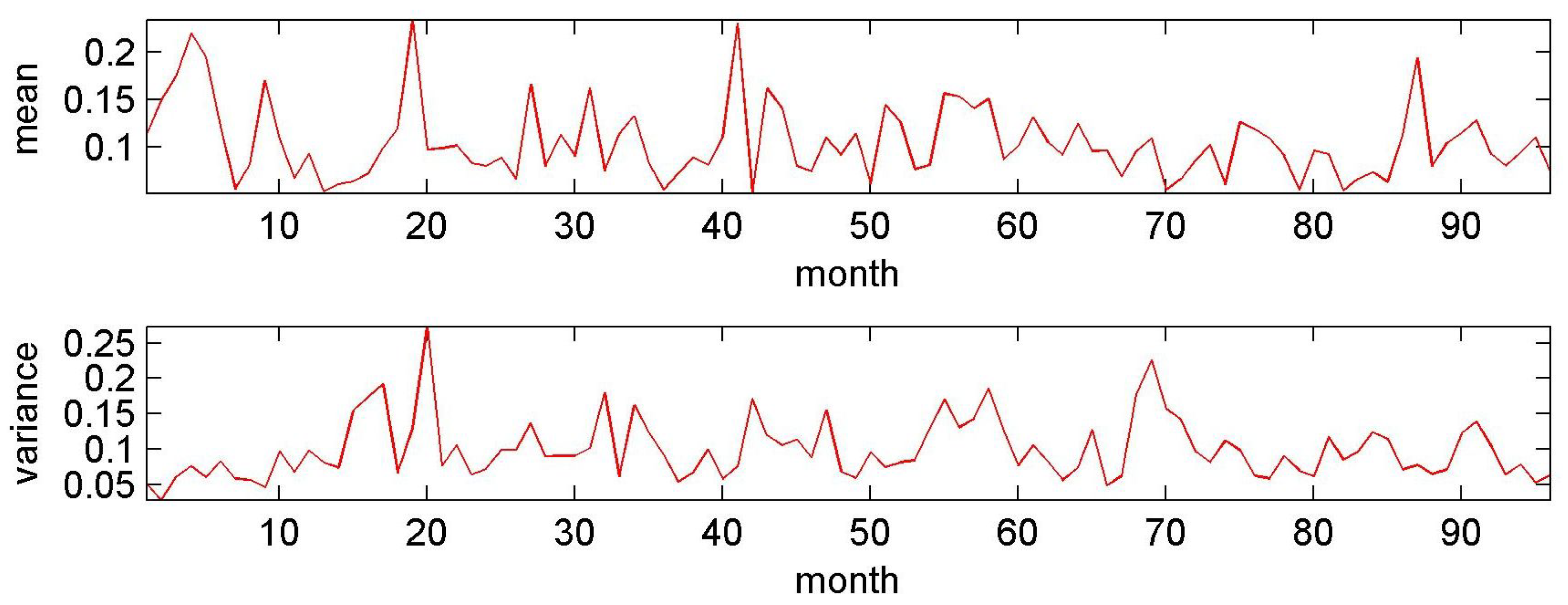
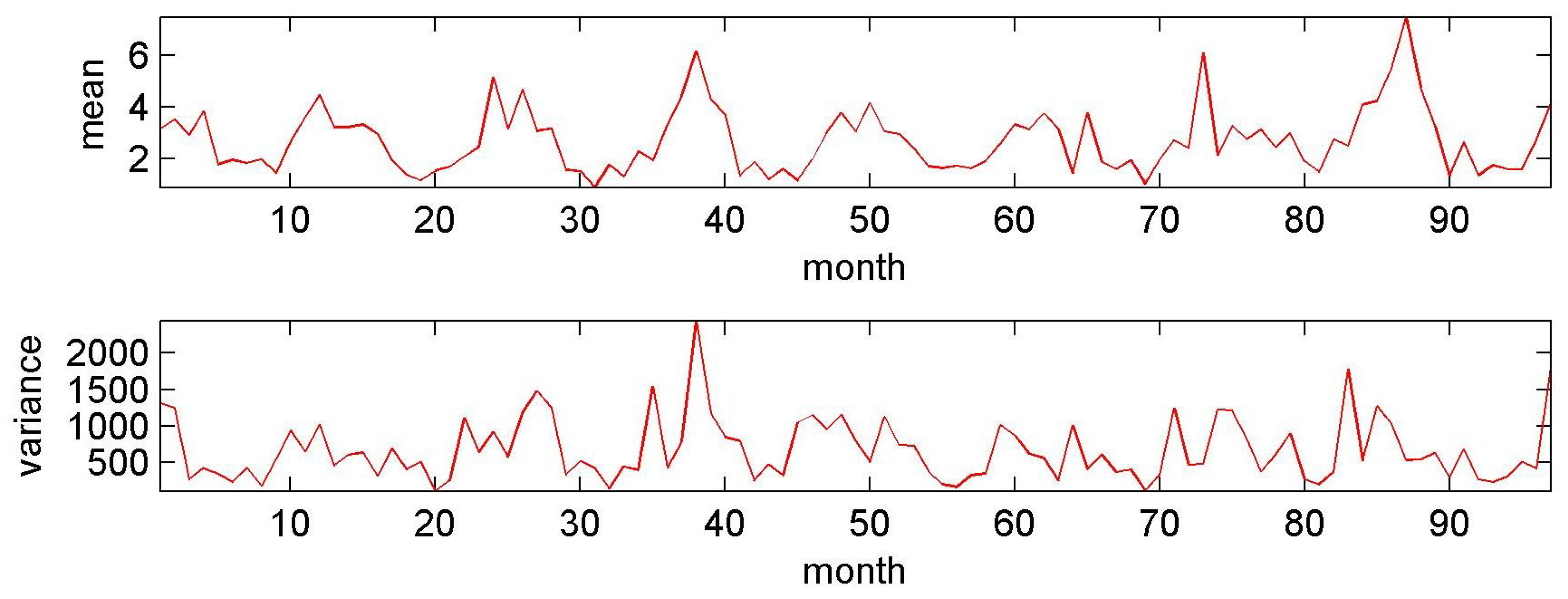
4. Results
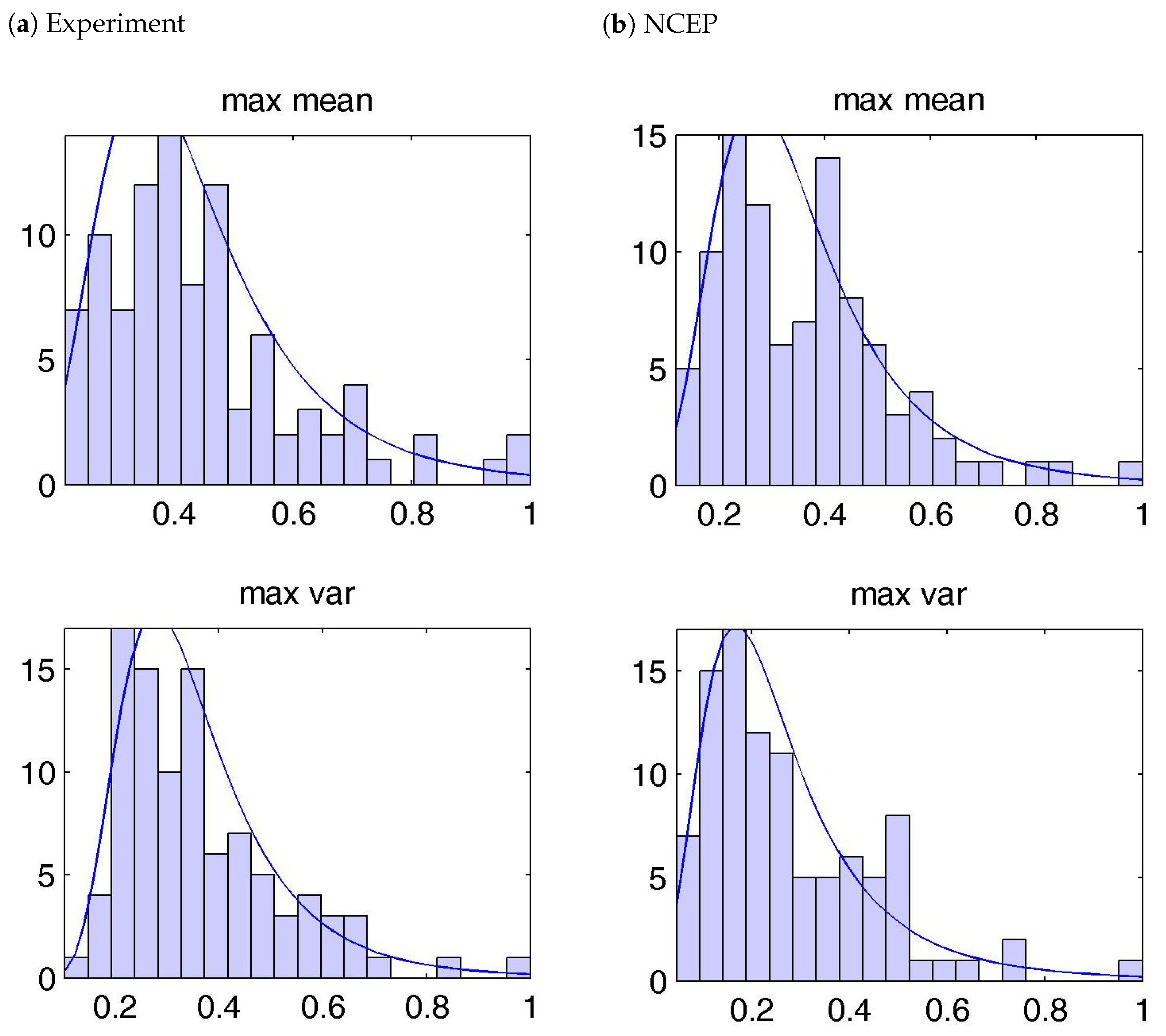
4.1. Extreme Value Distributions
4.2. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Fultz, D.; Long, R.R.; Owens, G.V.; Weil, J. Two-dimensional flow around a circular barrier in a rotating shell. AMS Meteorol. Monogr. 1959, 4, 61–68. [Google Scholar]
- Hide, R. Some experiments on thermal convection in a rotating liquid. Q. J. R. Meteoro. Soc. 1953, 79, 294–297. [Google Scholar] [CrossRef]
- Harlander, U.; von Larcher, T.; Wright, G.B.; Hoff, M.; Alexandrov, K.; Egbers, C. Chapter 17—Orthogonal decomposition methods to analyze PIV, LDA, and Thermography data of thermally driven rotating annulus laboratory experiments. In Modeling Atmospheric and Oceanic Flows: Insight from Laboratory Experiments and Numerical Simulations; von Larcher, T., Williams, P.D., Eds.; Wiley: Hoboken, NJ, USA, 2014; pp. 315–337. [Google Scholar]
- Read, P.L.; Perez, E.P.; Moraz, I.M.; Young, R.M.B. Chapter 1—Circulation of planetary atmospheres: Insights from rotating annulus and related experiments. In Modeling Atmospheric and Oceanic Flows: Insight from Laboratory Experiments and Numerical Simulations; von Larcher, T., Williams, P.D., Eds.; Wiley: Hoboken, NJ, USA, 2014; pp. 9–45. [Google Scholar]
- Folis, W.W.; Hide, R. Thermal convection in a rotating annulus of liquid: Effect of viscosity on the transition between axisymmetric and non-axisymmetric flow regimes. J. Atmos. Sci. 1965, 22, 541–558. [Google Scholar]
- Vincze, M.; Borcia, I.D.; Harlander, U. Temperature fluctuations in a changing climate: An ensemble based experimental approach. Sci. Rep. 2017, 7, 254. [Google Scholar] [CrossRef] [PubMed]
- Rodda, C. Gravity Wave Emission from Jet Systems in the Differentially Heated Rotating Annulus Experiment; Cuvillier Verlag: Göttingen, Germany, 2019; 200p. [Google Scholar]
- Morita, O. Transition between flow regimes of baroclinic flows in a rotating annulus of fluid, phase transitions. J. Atmos. Sci. 1990, 46, 213–244. [Google Scholar]
- Hart, J.E. Wavenumber Selection in Nonlinear Baroclinic Instability. J. Atmos. Sci. 1981, 38, 400–408. [Google Scholar] [CrossRef]
- Früh, W.G.; Read, P.L. Wave interactions and the transition to chaos of baroclinic waves in a thermally driven rotating annulus. Phil. Trans. R. Soc. Lond. 1997, A355, 101–153. [Google Scholar] [CrossRef]
- Lindzen, R.S.; Farrell, B.; Jacqmin, D. Vascillations due to wave interference: Application to the atmosphere and to annulus experiments. J. Atmos. Sci. 1981, 39, 14–23. [Google Scholar] [CrossRef]
- Hoff, M.; Harlander, U.; Egbers, C. Empirical singular vectors of baroclinic flows deduced from experimental data of a differentially heated rotating annulus. Meteorol. Z. 2015, 23, 581–597. [Google Scholar] [CrossRef]
- Rodda, C.; Harlander, U. Transition from geostrophic flows to inertia-gravity waves in the spectrum of a differentially heated rotating annulus experiment. J. Atmos. Sci. 2020, 77, 2793–2806. [Google Scholar] [CrossRef]
- Charney, J.G.; DeVore, J.G. Multiple-flow equilibria in the atmosphere and blocking. J. Atmos. Sci. 1979, 36, 1205–1216. [Google Scholar] [CrossRef]
- Weeks, E.R.; Tian, Y.; Urbach, J.S.; Ide, K.; Swinney, H.L.; Ghil, M. Transitions Between Blocked and Zonal Flows in a Rotating Annulus with Topography. Science 1997, 278, 1598–1601. [Google Scholar] [CrossRef] [PubMed]
- Marshall, S.D.; Read, P.L. An experimental investigation into topographic resonance in a baroclinic rotating annulus. Geophys. Astrophys. Fluid Dyn. 2005, 109, 391–421. [Google Scholar] [CrossRef]
- Vincze, M.; Bozóki, T.; Herein, M.; Borcia, I.D. The Drake Passage opening from an experimental fluid dynamics point of view. Sci. Rep. 2021, 11, 19951. [Google Scholar] [CrossRef] [PubMed]
- Rodda, C.; Harlander, U.; Vincze, M. Jet stream variability in a polar warming scenario—A laboratory perspective. Weather Clim. Dyn. 2022; accepted. [Google Scholar]
- Borchert, S.; Achatz, U.; Fruman, M.D. Gravity wave emission in an atmosphere-like configuration of the differentially heated rotating annulus experiment. J. Fluid Mech. 1979, 36, 287–311. [Google Scholar] [CrossRef][Green Version]
- Rodda, C.; Hien, S.; Achatz, U.; Harlander, U. A new atmospheric-like differentially heated rotating annulus configuration to study gravity wave emission from jets and fronts. Exp. Fluids 2019, 62, 2. [Google Scholar] [CrossRef]
- Read, P.L. Dynamics and circulation regimes of terrestrial planets. Planet. Space Sci. 2011, 59, 900–914. [Google Scholar] [CrossRef]
- Embrechts, P.; Klüppelberg, C.; Mikosch, T. Modelling Extremal Events for Insurance and Finance; Springer: New York, NY, USA, 1997. [Google Scholar]
- Mudelsee, M. Climate Time Series Analysis: Classical Statistical and Bootstrap Methods; Springer: Cham, Switzerland, 2014; 454p. [Google Scholar]
- Fein, J.S.; Pfeffer, R.L. An experimental study of the effects of Prandtl number on thermal convection in a rotating, differentially heated cylindrical annulus of fluid. J. Fluid Mech. 1976, 75, 81–112. [Google Scholar] [CrossRef]
- Eady, E.T. Long waves and cyclone waves. Tellus 1949, 1, 33–52. [Google Scholar] [CrossRef]
- Stendel, M.; Francis, J.; White, R.; Williams, P.D.; Woollings, T. Chapter 15—The jet stream and climate change. In Climate Change, 3rd ed.; Letcher, T.M., Ed.; Elsevier: Amsterdam, The Netherlands, 2021; pp. 327–357. [Google Scholar] [CrossRef]


| Geometry | |||
|---|---|---|---|
| inner radius | a (mm) | 350 | |
| outer radius | b (mm) | 700 | |
| gap width | b–a (mm) | 350 | |
| fluid depth | d (mm) | 60 | |
| Exp. Parameters | |||
| temperature difference | (K) | 4.0 | |
| revolution speed | (rpm) | 2.0 | |
| Fluid Properties | |||
| density | () | 997 | |
| kin. viscosity | () | 1.004 | |
| therm. conductivity | () | 0.1434 | |
| exp. coefficient | (1/K) | 0.207 | |
| Similarity Parameters | |||
| Prandtl number | Pr = | 7.00 | |
| Rossby number | Ro | 0.91 | |
| Taylor number | Ta | 1.52 |
| Image format | 1024 × 680 pixel |
|---|---|
| Spectral range | 7.5–14 m |
| Range for measuring and visualization | – K |
| Thermal sensitivity | <80 mK |
| Measurement accuracy | ±1.5 K |
| Dynamic range | 16 bit |
| Image rate | 60 Hz |
| GEV | 0.1230 | 0.1157 | 0.3621 | −0.0585 | 0.0967 | 0.3353 |
| 0.3044 | 0.1384 | 0.3889 | ||||
| GEV | 0.1136 | 0.1055 | 0.2903 | −0.0387 | 0.0890 | 0.2666 |
| 0.2660 | 0.1252 | 0.3140 | ||||
| GEV | 0.1204 | 0.1161 | 0.2809 | −0.0800 | 0.0965 | 0.2536 |
| 0.3207 | 0.1396 | 0.3082 | ||||
| GEV | 0.1996 | 0.1115 | 0.1870 | −0.0020 | 0.0922 | 0.1608 |
| 0.4012 | 0.1347 | 0.2131 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Harlander, U.; Borcia, I.D.; Vincze, M.; Rodda, C. Probability Distribution of Extreme Events in a Baroclinic Wave Laboratory Experiment. Fluids 2022, 7, 274. https://doi.org/10.3390/fluids7080274
Harlander U, Borcia ID, Vincze M, Rodda C. Probability Distribution of Extreme Events in a Baroclinic Wave Laboratory Experiment. Fluids. 2022; 7(8):274. https://doi.org/10.3390/fluids7080274
Chicago/Turabian StyleHarlander, Uwe, Ion Dan Borcia, Miklos Vincze, and Costanza Rodda. 2022. "Probability Distribution of Extreme Events in a Baroclinic Wave Laboratory Experiment" Fluids 7, no. 8: 274. https://doi.org/10.3390/fluids7080274
APA StyleHarlander, U., Borcia, I. D., Vincze, M., & Rodda, C. (2022). Probability Distribution of Extreme Events in a Baroclinic Wave Laboratory Experiment. Fluids, 7(8), 274. https://doi.org/10.3390/fluids7080274