Analysis of Temperature Field in the Dielectrophoresis-Based Microfluidic Cell Separation Device
Abstract
:1. Introduction
2. Materials and Methods
2.1. Sample Preparation
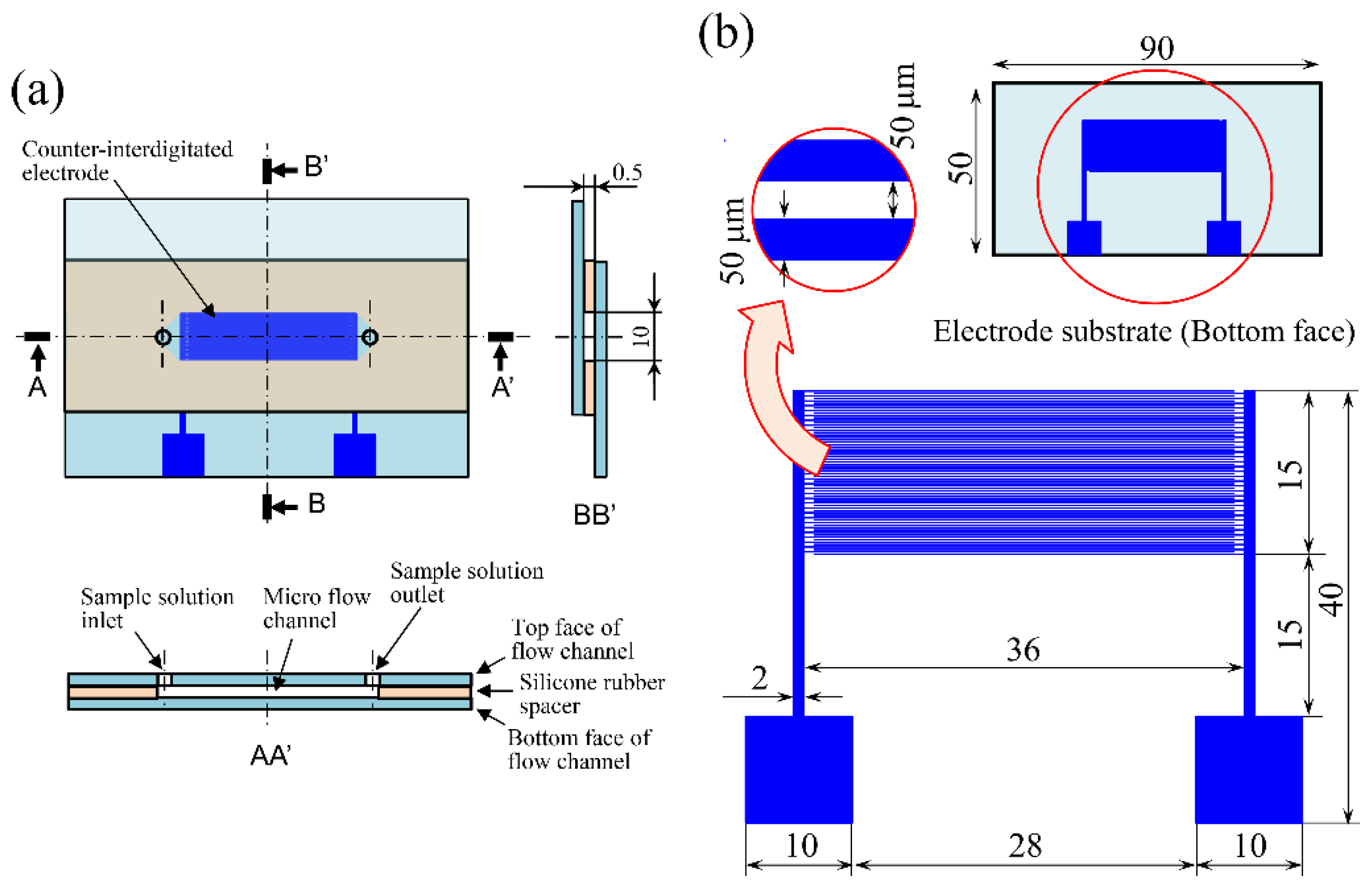
2.2. Device Fabrication
2.3. Experimental Setup
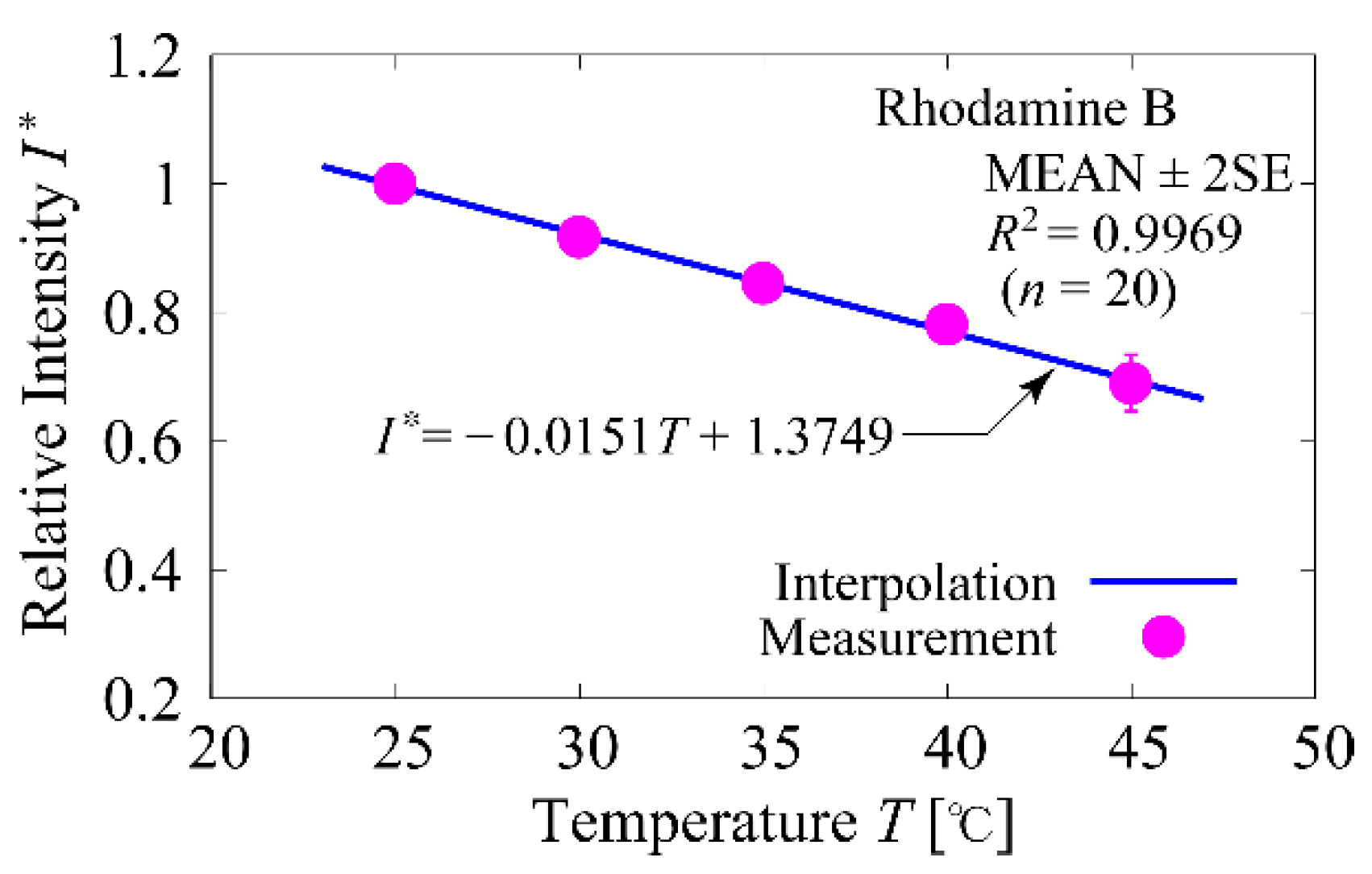
2.4. Temperature Calibration
2.5. Image Processing
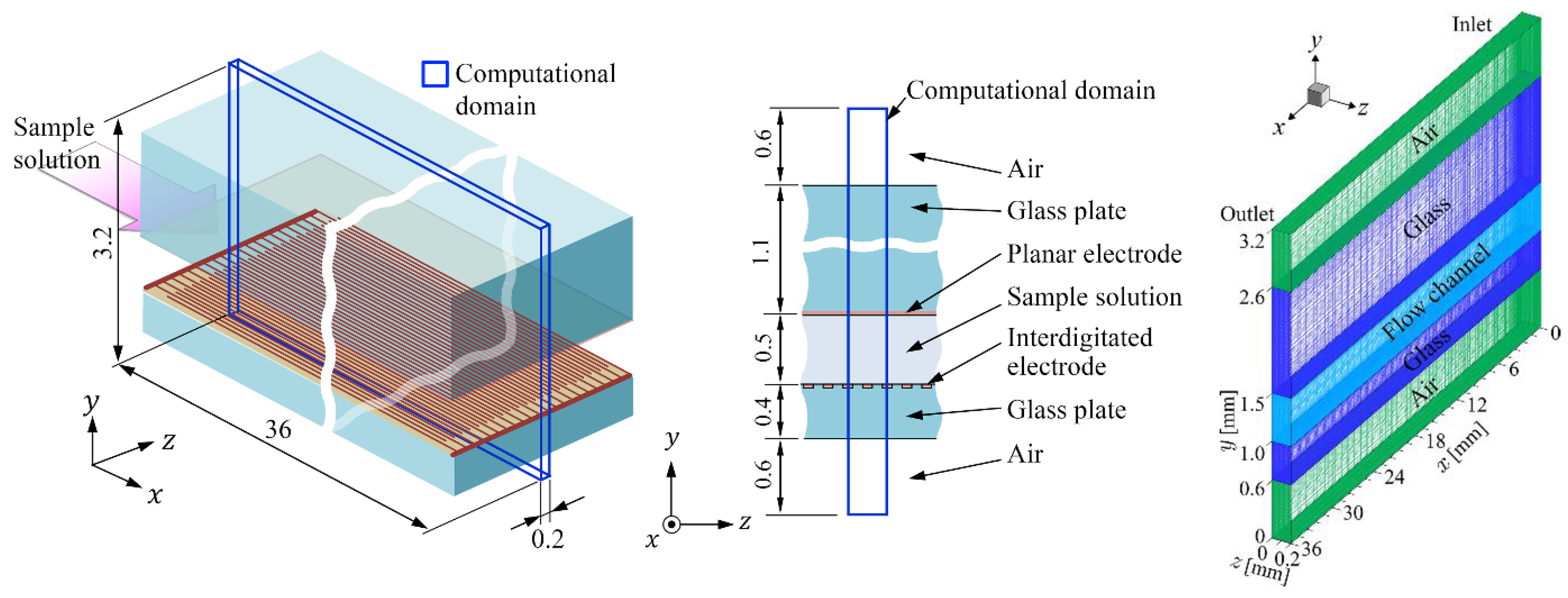
3. Numerical Simulation
4. Results
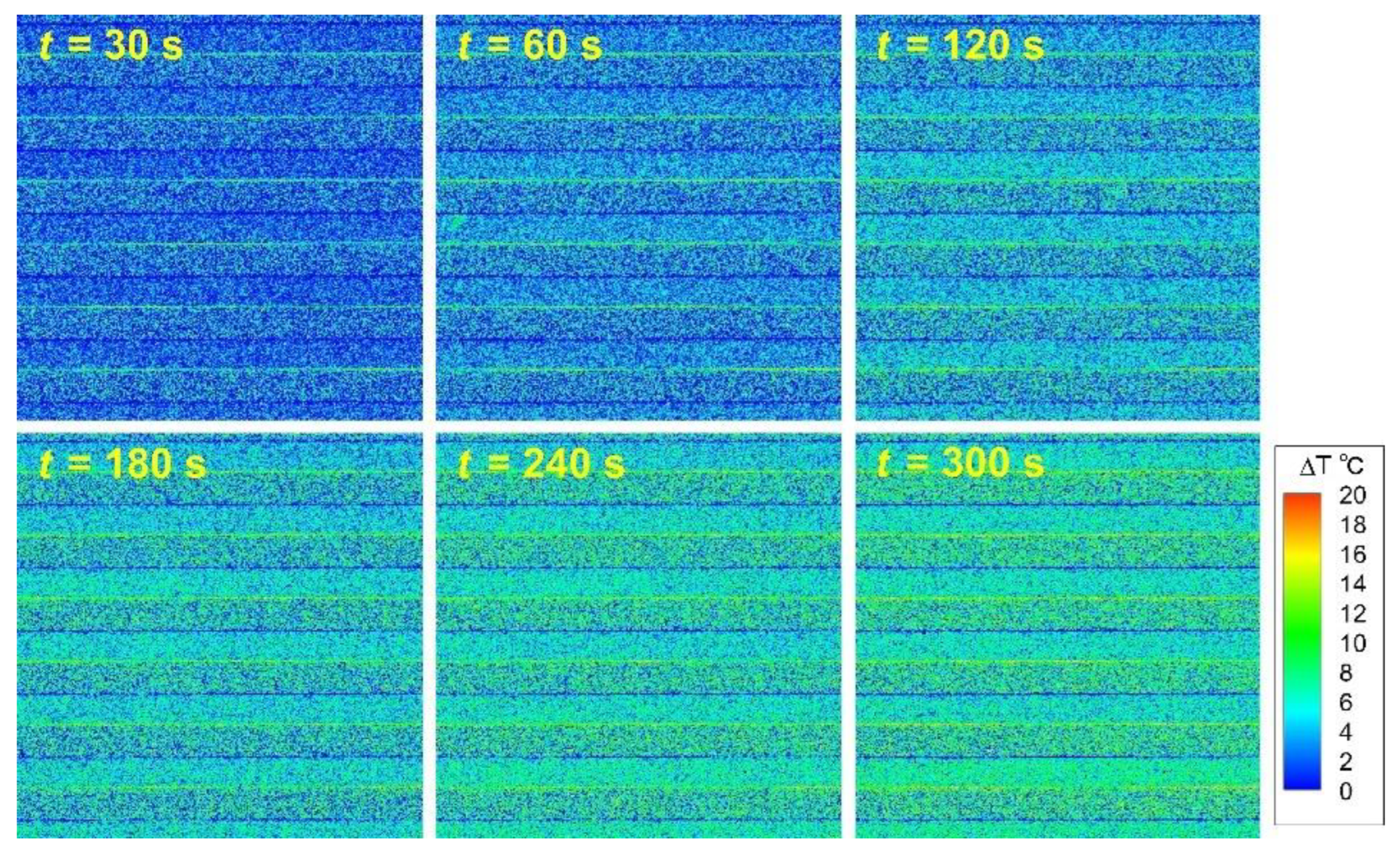
4.1. Distribution of Temperature Rise at the Bottom of the Flow Channel
4.2. Mean Temperature Rise at the Bottom of the Channel
4.3. Dependence of Average Temperature Rise on Frequency
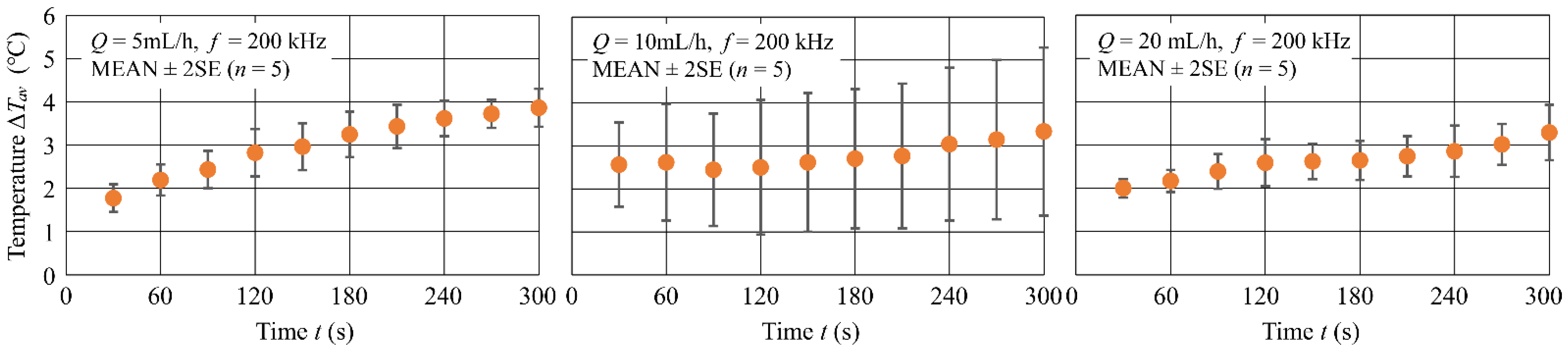
4.4. Dependence of Average Temperature Rise on Flow Rate
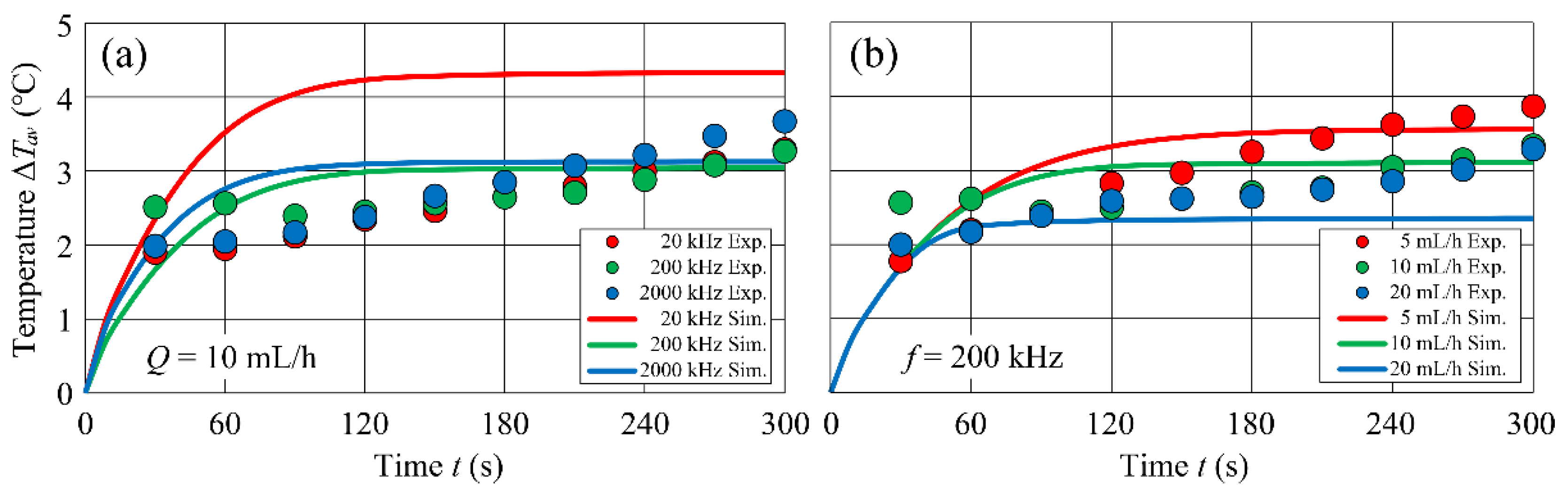
4.5. Comparison with Numerical Simulation
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kung, Y.; Niazi, K.R.; Chiou, P. Tunnel dielectrophoresis for ultra-high precision size-based cell separation. Lab Chip 2021, 21, 1049–1060. [Google Scholar] [CrossRef]
- Shirmohammadli, V.; Manavizadeh, N. Application of differential electrodes in a dielectrophoresis-based device for cell separation. IEEE Trans. Electron Devices 2019, 66, 4075–4080. [Google Scholar] [CrossRef]
- Shen, S.; Yi, Z.; Li, X.; Xie, S.; Jin, M.; Zhou, G.; Yan, Z.; Shui, L. Flow-field-assisted dielectrophoretic microchips for high-efficiency sheathless particle/cell separation with dual mode. Anal. Chem. 2021, 93, 7606–7615. [Google Scholar] [CrossRef]
- Jones, T.B. Electromechanics of Particles; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Rahmati, M.; Chen, X. Separation of circulating tumor cells from blood using dielectrophoretic DLD manipulation. Biomed. Microdevices 2021, 23, 49. [Google Scholar] [CrossRef] [PubMed]
- Saydé, T.; Manczak, R.; Saada, S.; Bégaud, G.; Bessette, B.; Lespes, G.; Coustumer, P.L.; Gaudin, K.; Dalmay, C.; Pothier, A.; et al. Characterization of glioblastoma cancer stem cells sorted by sedimentation field-flow fractionation using an ultrahigh-frequency range dielectrophoresis biosensor. Anal. Chem. 2021, 93, 12664–12671. [Google Scholar] [CrossRef] [PubMed]
- Oh, M.; Jayasooriya, V.; Woo, S.O.; Nawarathna, D.; Choi, Y. Selective manipulation of biomolecules with insulator-based dielectrophoretic tweezers. ACS Appl. Nano Mater. 2020, 3, 797–805. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhang, J.; Song, Z.; Liu, Q.; Song, Y. Recent advances in dielectrophoresis-based cell viability assessment. Electrophoresis 2020, 41, 917–932. [Google Scholar] [CrossRef]
- Metaxas, A.C.; Meredith, R.J. Industrial Microwave Heating; Institution of Engineering and Technology: London, UK, 1988. [Google Scholar]
- Samalia, A.; Holmberg, C.I.; Sistonen, L.; Orrenius, S. Thermotolerance and cell death are distinct cellular responses to stress: Dependence on heat shock proteins. FEBS Lett. 1999, 461, 306–310. [Google Scholar] [CrossRef] [Green Version]
- Reissis, Y.; García-Gareta, E.; Korda, M.; Blunn, G.W.; Hua, J. The effect of temperature on the viability of human mesenchymal stem cells. Stem Cell Res. Ther. 2013, 4, 139. [Google Scholar] [CrossRef] [Green Version]
- Tay, F.E.H.; Yu, L.; Panga, A.J.; Iliescu, C. Electrical and thermal characterization of a dielectrophoretic chip with 3D electrodes for cells manipulation. Electrochim. Acta 2007, 52, 2862–2868. [Google Scholar] [CrossRef]
- Sridharan, S.; Zhu, J.; Hu, G.; Xuan, X. Joule heating effects on electroosmotic flow in insulator-based dielectrophoresis. Electrophoresis 2011, 32, 2274–2281. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kale, A.; Patel, S.; Qian, S.; Hu, G.; Xuan, X. Joule heating effects on reservoir-based dielectrophoresis. Electrophoresis 2014, 35, 721–727. [Google Scholar] [CrossRef] [Green Version]
- Nakano, A.; Luo, J.; Ros, A. Temporal and spatial temperature measurement in insulator-based dielectrophoretic devices. Anal. Chem. 2014, 86, 6516–6524. [Google Scholar] [CrossRef] [Green Version]
- Kwak, T.J.; Hossen, I.; Bashir, R.; Chang, W.; Lee, C.H. Localized dielectric loss heating in dielectrophoresis devices. Sci. Rep. 2019, 9, 18977. [Google Scholar] [CrossRef] [Green Version]
- Seki, Y.; Nagasaka, A.; Gondo, T.; Tada, S.; Eguchi, M. Continuous separation of biological cells using a new type of dielectrophoresis-based microfluidic device. In Proceedings of the SB3C2022 Summer Biomechanics, Bioengineering and Biotransport Conference, Eastern Shore, MD, USA, 20–23 June 2022; p. 173. [Google Scholar]
- Shah, J.J.; Gaitan, M.; Geist, J. Generalized temperature measurement equations for rhodamine B dye solution and its application to microfluidics. Anal. Chem. 2009, 81, 8260–8263. [Google Scholar] [CrossRef]
- Glawdel, T.; Almutairi, Z.; Wang, S.; Ren, C. Photobleaching absorbed rhodamine B to improve temperature measurements in PDMS microchannels. Lab Chip 2009, 9, 171–174. [Google Scholar] [CrossRef]
- Puri, P.; Kumar, V.; Belgamwar, S.U.; Ananthasubramanian, M.; Sharma, N.N. Microfluidic platform for dielectrophoretic separation of bio-particles using serpentine microelectrodes. Microsyst. Technol. 2019, 25, 2813–2820. [Google Scholar] [CrossRef]
- Nie, X.; Luo, Y.; Shen, P.; Han, C.; Yu, D.; Xing, X. High-throughput dielectrophoretic cell sorting assisted by cell sliding on scalable electrode tracks made of conducting-PDMS. Sens. Actuators B Chem. 2021, 327, 128873. [Google Scholar] [CrossRef]
- Mishra, Y.N.; Yoganantham, A.; Koegl, M.; Zigan, L. Investigation of five organic dyes in ethanol and butanol for two-color laser-induced fluorescence ratio thermometry. Optics 2020, 1, 1–17. [Google Scholar] [CrossRef] [Green Version]
- Piskunov, M.V.; Strizhak, P.A. Using planar laser induced fluorescence to explain the mechanism of heterogeneous water droplet boiling and explosive breakup. Exp. Therm. Fluid Sci. 2018, 91, 103–116. [Google Scholar] [CrossRef]
- Tada, S. Numerical simulation of dielectrophoretic separation of live/dead cells using a three-dimensional nonuniform AC electric field in micro-fabricated devices. Biorheology 2015, 52, 211–224. [Google Scholar] [CrossRef]
- Puri, P.; Kumar, V.; Belgamwar, S.U.; Sharma, N.N. Microfluidic device for cell trapping with carbon electrodes using dielectrophoresis. Biomed. Microdevices 2018, 20, 102. [Google Scholar] [CrossRef] [PubMed]
- Midi, N.S.; Sasaki, K.; Ohyama, R.; Shinyashiki, N. Broadband complex dielectric constants of water and sodium chloride aqueous solutions with different DC conductivities, IEEJ Trans. Electr. Electron. Eng. 2014, 9, S8–S12. [Google Scholar]
- Gómez-Galván, F.; Lara-Ceniceros, T.; Mercado-Uribe, H. Device for simultaneous measurements of the optical and dielectric properties of hydrogels. Meas. Sci. Technol. 2012, 23, 025602. [Google Scholar] [CrossRef]
- Shayegani Akmal, A.A.; Borsi, H.; Gockenbach, E.; Wasserberg, V.; Mohseni, H. Dielectric behavior of insulating liquids at very low frequency. IEEE Trans. Dielectr. Electr. Insul. 2006, 13, 532–538. [Google Scholar] [CrossRef]
- Rander, D.N.; Joshi, Y.S.; Kanse, K.S.; Kumbharkhane, A.C. Dielectric dispersion and hydrogen bonding interactions study of aqueous D-mannitol using time domain reflectometry. Phys. Chem. Liquids 2015, 53, 187–192. [Google Scholar] [CrossRef]
- Zhang, C.; Hou, Z.; Zhang, B.; Fang, H.; Bi, S. High sensitivity self-recovery ethanol sensor based on polyporous graphene oxide/melamine composites. Carbon 2018, 137, 467–474. [Google Scholar] [CrossRef]




| Water | ||||
| Glass | ||||
| Air |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tada, S.; Seki, Y. Analysis of Temperature Field in the Dielectrophoresis-Based Microfluidic Cell Separation Device. Fluids 2022, 7, 263. https://doi.org/10.3390/fluids7080263
Tada S, Seki Y. Analysis of Temperature Field in the Dielectrophoresis-Based Microfluidic Cell Separation Device. Fluids. 2022; 7(8):263. https://doi.org/10.3390/fluids7080263
Chicago/Turabian StyleTada, Shigeru, and Yoshinori Seki. 2022. "Analysis of Temperature Field in the Dielectrophoresis-Based Microfluidic Cell Separation Device" Fluids 7, no. 8: 263. https://doi.org/10.3390/fluids7080263
APA StyleTada, S., & Seki, Y. (2022). Analysis of Temperature Field in the Dielectrophoresis-Based Microfluidic Cell Separation Device. Fluids, 7(8), 263. https://doi.org/10.3390/fluids7080263






