A Hydrodynamic Analog of the Casimir Effect in Wave-Driven Turbulent Flows
Abstract
1. Introduction
2. Materials and Methods
2.1. Generation of Faraday Waves and Wave-Driven Flows
2.2. Characterisation of the Wave Field Topography and of the Wave-Driven Flows
2.3. Manufacturing of the Floating Beams
2.4. Force Measurements
- a high-resolution measurement of forces typically in the range of (2–200) μN.
- to ensure the fluctuations of the position of the moving beam are smaller than the mean beam spacing W.
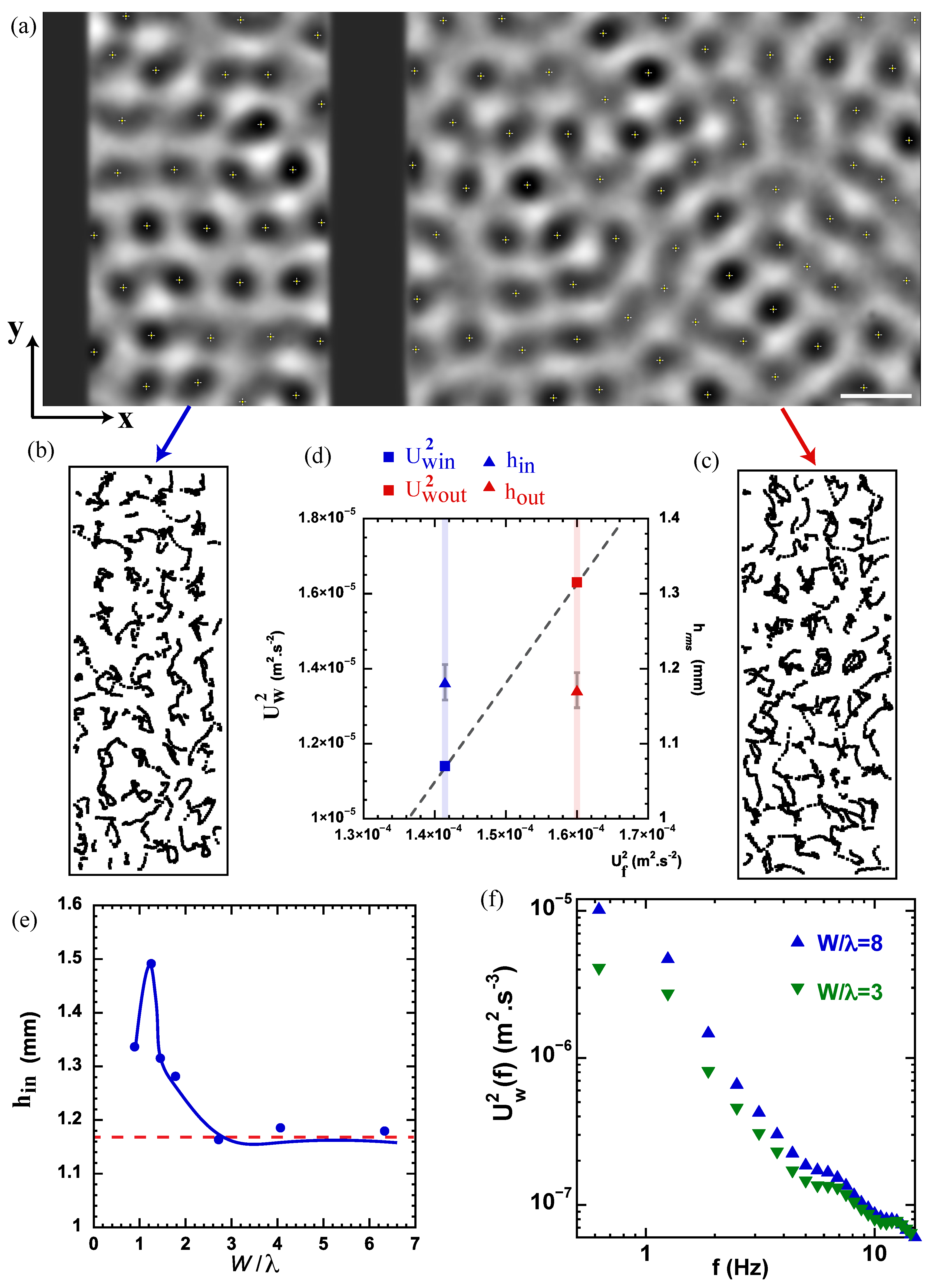
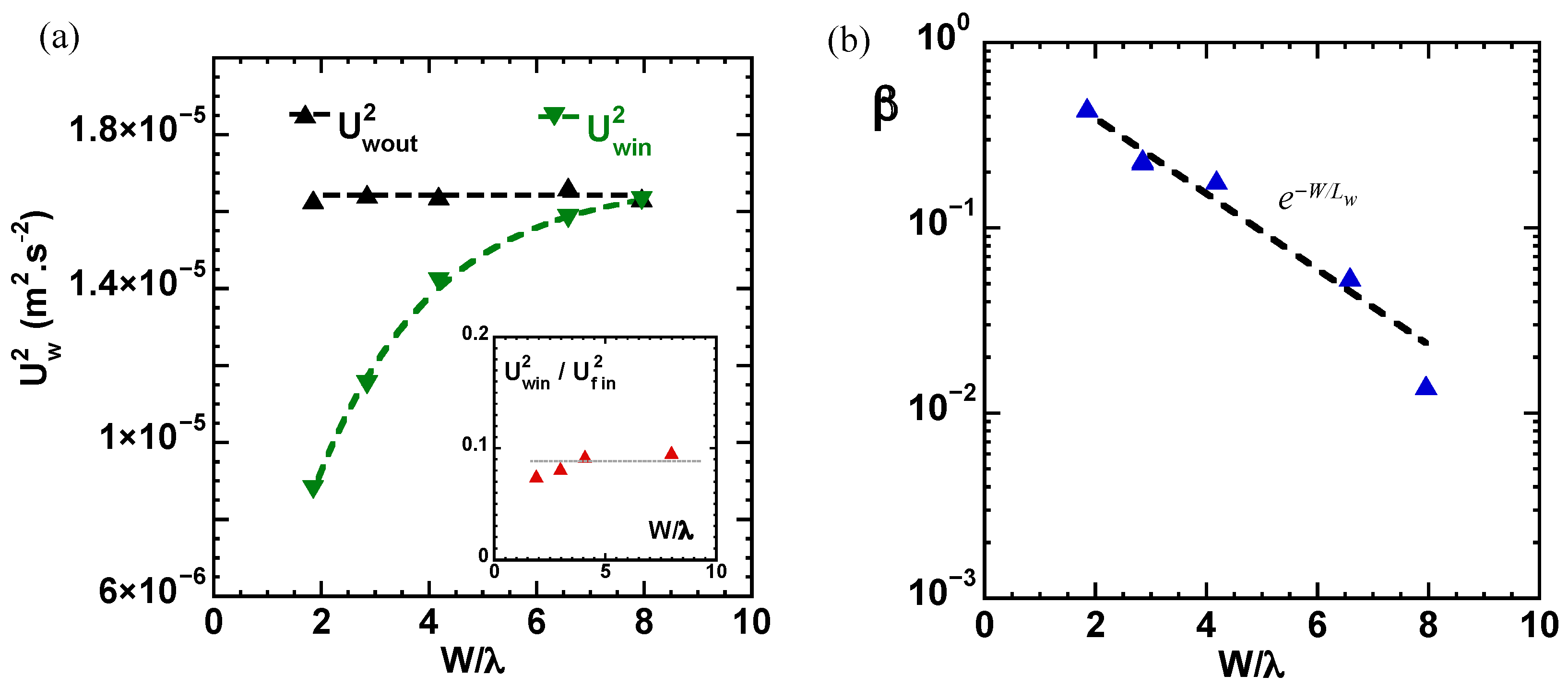
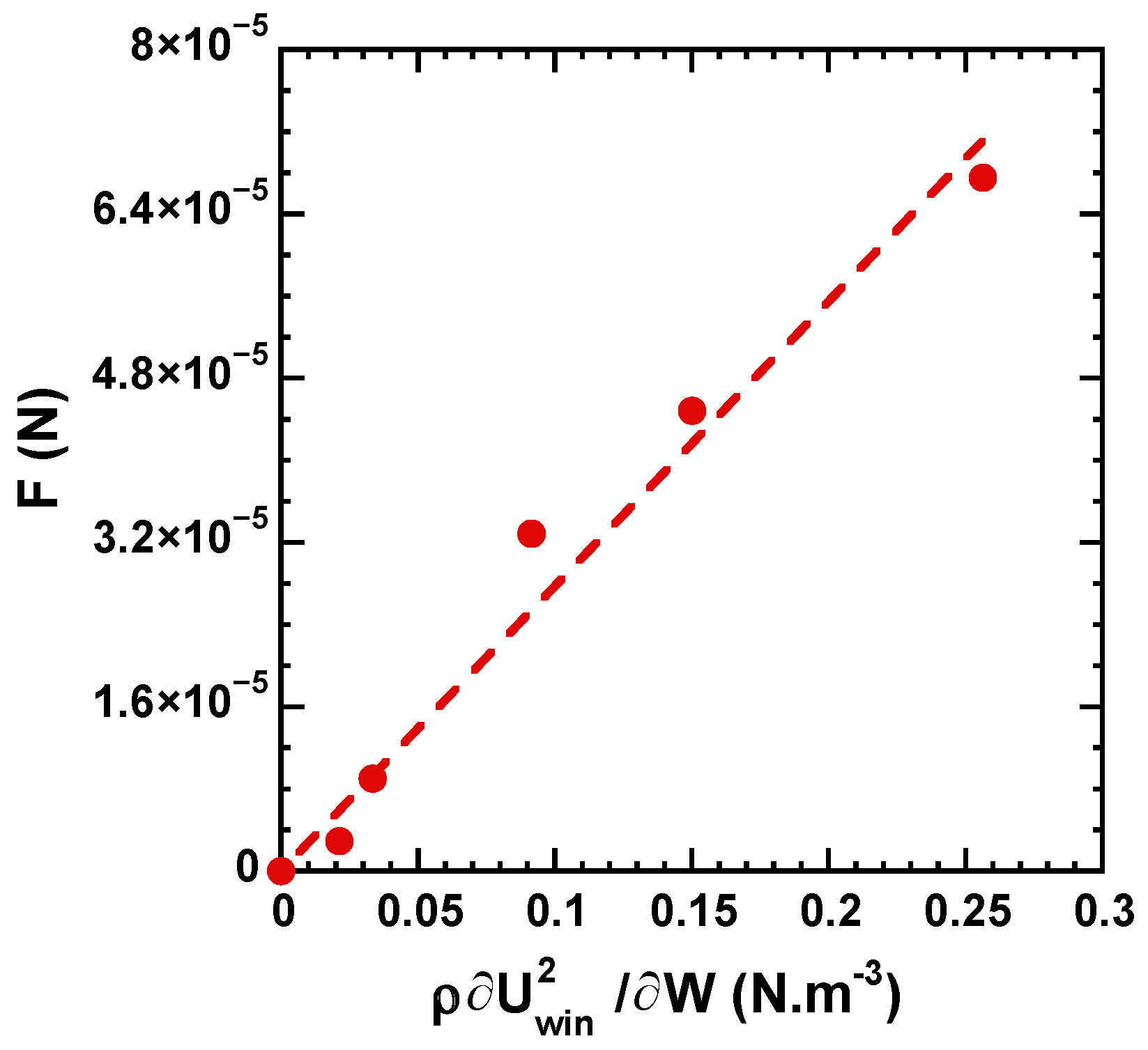
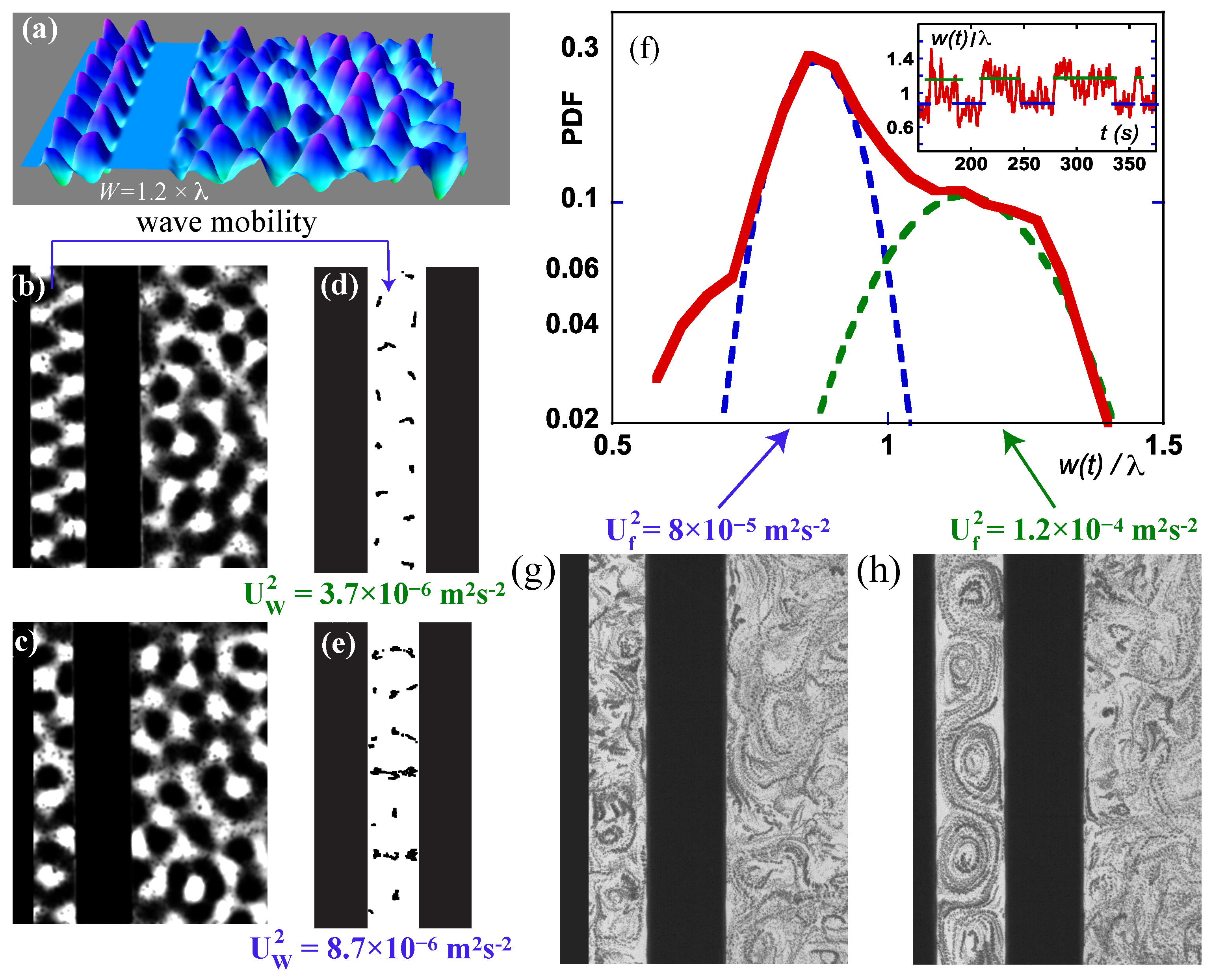
3. Results
3.1. Long-Range Attraction Force
3.2. Short-Range Interaction at the Faraday Wavelength
4. Discussion
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Kardar, M.; Golestanian, R. The “friction” of vacuum, and other fluctuation-induced forces. Rev. Mod. Phys. 1999, 71, 1233–1245. [Google Scholar] [CrossRef]
- Casimir, H.B. On the attraction between two perfectly conducting plates. Proc. K. Ned. Akad. Wet. 1948, 51, 793–795. [Google Scholar]
- Bonn, D.; Otwinowski, J.; Sacanna, S.; Guo, H.; Weddam, G.; Schall, P. Direct observation of colloidal aggregation by critical Casimir forces. Phys. Rev. Lett. 2009, 103, 156101. [Google Scholar] [CrossRef] [PubMed]
- Ray, D.; Reichhardt, C.; Olson Reichhardt, C.J. Casimir effect in active matter systems. Phys. Rev. E 2014, 90, 013019. [Google Scholar] [CrossRef] [PubMed]
- Hertlein, C.; Helden, L.; Gambassi, A.; Dietrich, S.; Bechinger, C. Direct measurement of critical Casimir forces. Nature 2008, 451, 172–175. [Google Scholar] [CrossRef] [PubMed]
- Lee, A.A.; Vella, D.; Wettlaufer, J.S. Fluctuation spectra and force generation in nonequilibrium systems. Proc. Natl. Acad. Sci. USA 2017, 114, 9255–9260. [Google Scholar] [CrossRef]
- Boersma, S.L. A maritime analogy of the Casimir effect. Am. J. Phys. 1996, 64, 539–541. [Google Scholar] [CrossRef]
- Denardo, B.C.; Puda, J.J.; Larraza, A. A water wave analog of the Casimir effect. Am. J. Phys. 2009, 77, 1095. [Google Scholar] [CrossRef]
- Zuriguel, I.; Boudet, J.F.; Amarouchene, Y.; Kellay, H. Role of fluctuation-induced interactions in the axial segregation of granular materials. Phys. Rev. Lett. 2005, 95, 258002. [Google Scholar] [CrossRef]
- Tennekes, H.; Lumley, J.L. A First Course in Turbulence; Cambridge Massachusetts Press: Cambridge, MA, USA, 1972. [Google Scholar]
- Kolmogorov, A.N. The local structure of turbulence in incompressible viscous fluid for very large Reynolds’ numbers. Dokl. Akad. Nauk SSSR 1941, 30, 301–305. [Google Scholar]
- Kuhnen, J.; Song, B.; Scarselli, D.; Budanur, N.B.; Riedl, M.; Willis, A.P.; Avila, M.; Hof, B. Destabilizing turbulence in pipe flow. Nat. Phys. 2018, 14, 386–390. [Google Scholar] [CrossRef]
- Tran, T.; Chakraborty, P.; Guttenberg, N.; Prescott, A.; Kellay, H.; Goldburg, W.; Goldenfeld, N.; Gioia, G. Macroscopic effects of the spectral structure in turbulent flows. Nat. Phys. 2010, 6, 438–441. [Google Scholar] [CrossRef]
- Vilquin, A.; Jagielka, J.; Djambov, S.; Herouard, H.; Fischer, P.; Bruneau, C.-H.; Chakraborty, P.; Gioia, G.; Kellay, H. Asymptotic turbulent friction in 2D rough-walled flows. Sci. Adv. 2021, 7, 5. [Google Scholar] [CrossRef] [PubMed]
- Francois, N.; Xia, H.; Punzmann, H.; Shats, M. Rectification of chaotic fluid motion in two-dimensional turbulence. Phys. Rev. Fluids 2018, 3, 124602. [Google Scholar] [CrossRef]
- Yang, J.; Davoodianidalik, M.; Xia, H.; Punzmann, H.; Francois, N. Passive propulsion in turbulent flows. Phys. Rev. Fluids 2019, 4, 104608. [Google Scholar] [CrossRef]
- Francois, N.; Xia, H.; Punzmann, H.; Shats, M. Nonequilibrium thermodynamics of turbulence-driven rotors. Phys. Rev. Lett. 2020, 124, 254501. [Google Scholar] [CrossRef]
- Spandan, V.; Putt, D.; Ostilla-Mónico, R.; Lee, A.A. Fluctuation-induced force in homogeneous isotropic turbulence. Sci. Adv. 2020, 6, 14. [Google Scholar] [CrossRef]
- Davoodianidalik, M.; Punzmann, H.; Kellay, H.; Xia, H.; Shats, M.; Francois, N. Fluctuation-induced interaction in turbulent flows. Phys. Rev. Lett. 2022, 128, 024503. [Google Scholar] [CrossRef]
- Faraday, M. On the Forms and States Assumed by Fluids in Contact with Vibrating Elastic Surfaces. Phil. Trans. R. Soc. Lond. 1831, 121, 299. [Google Scholar]
- Douady, S. Experimental Study of the Faraday Instability. J. Fluid Mech. 1990, 221, 383. [Google Scholar] [CrossRef]
- Kudrolli, A.; Gollub, J.P. Patterns and Spatiotemporal Chaos in Parametrically Forced Surface Waves: Systematic Survey at Large Aspect Ratio. Physica D 1996, 97, 133. [Google Scholar] [CrossRef]
- Edwards, W.S.; Fauve, S. Patterns and Quasipatterns in the Faraday Experiment. J. Fluid Mech. 1994, 278, 123. [Google Scholar] [CrossRef]
- Goldman, D.I.; Shattuck, M.D.; Moon, S.J.; Swift, J.B.; Swinney, H.L. Lattice Dynamics and Melting of a Nonequilibrium Pattern. Phys. Rev. Lett. 2003, 90, 104302. [Google Scholar] [CrossRef] [PubMed]
- Epstein, T.; Fineberg, J. Necessary Conditions for Mode Interactions in Parametrically Excited Waves. Phys. Rev. Lett. 2008, 100, 134101. [Google Scholar] [CrossRef]
- Huepe, C.; Ding, Y.; Umbanhowar, P.; Silber, M. Forcing function control of Faraday wave instabilities in viscous shallow fluids. Phys. Rev. E 2006, 73, 016310. [Google Scholar] [CrossRef]
- Shani, I.; Cohen, G.; Fineberg, J. Localized Instability on the Route to Disorder in Faraday Waves. Phys. Rev. Lett. 2010, 104, 184507. [Google Scholar] [CrossRef]
- Batchelor, G.K. An Introduction to Fluid Dynamics; Cambridge Massachusetts Press: Cambridge, MA, USA, 1967. [Google Scholar]
- Longuet-Higgins, M.S. Mass Transport in Water Waves. Phil. Trans. R. Soc. 1953, A245, 535. [Google Scholar]
- Punzmann, H.; Francois, N.; Xia, H.; Falkovich, G.; Shats, M. Generation and reversal of surface flows by propagating waves. Nat. Phys. 2014, 10, 658–663. [Google Scholar] [CrossRef]
- Ramshankar, R.; Berlin, D.; Gollub, J.P. Transport by Capillary Waves. Part 1. Particle Trajectories. Phys. Fluids A 1990, 2, 1955. [Google Scholar] [CrossRef][Green Version]
- Mesquita, O.N.; Kane, S.; Gollub, J.P. Transport by Capillary Waves: Fluctuating Stokes Drift. Phys. Rev. A 1992, 45, 3700. [Google Scholar] [CrossRef]
- Hansen, A.E.; Schroder, E.; Alstrom, P.; Andersen, J.S.; Levinsen, M.T. Fractal Particle Trajectories in Capillary Waves: Imprint of Wavelength. Phys. Rev. Lett. 1997, 79, 1845. [Google Scholar] [CrossRef]
- Francois, N.; Xia, H.; Punzmann, H.; Shats, M. Inverse energy cascade and emergence of large coherent vortices in turbulence driven by Faraday waves. Phys. Rev. Lett. 2013, 110, 194501. [Google Scholar] [CrossRef] [PubMed]
- von Kameke, A.; Huhn, F.; Fernandez-Garcia, G.; Munuzuri, A.P.; Perez-Munuzuri, V. Double cascade turbulence and Richardson dispersion in a horizontal fluid flow induced by Faraday waves. Phys. Rev. Lett. 2011, 107, 074502. [Google Scholar] [CrossRef] [PubMed]
- Xia, H.; Francois, N.; Punzmann, H.; Shats, M. Lagrangian scale of particle dispersion in turbulence. Nat. Commun. 2013, 4, 2013. [Google Scholar] [CrossRef]
- Francois, N.; Xia, H.; Punzmann, H.; Ramsden, S.; Shats, M. Three-dimensional fluid motion in Faraday waves: Creation of vorticity and generation of two-dimensional turbulence. Phys. Rev. X 2014, 4, 021021. [Google Scholar] [CrossRef]
- Francois, N.; Xia, H.; Punzmann, H.; Fontana, P.W.; Shats, M. Wave-based liquid-interface metamaterials. Nat. Commun. 2017, 8, 14325. [Google Scholar] [CrossRef] [PubMed]
- Xia, H.; Francois, N. Two-dimensional turbulence in three-dimensional flows. Phys. Fluids 2017, 29, 111107. [Google Scholar] [CrossRef]
- Francois, N.; Xia, H.; Punzmann, H.; Shats, M. Wave-particle interaction in the Faraday waves. Eur. Phys. J. E 2015, 38, 106. [Google Scholar] [CrossRef]
- Kraichnan, R. Inertial ranges in two-dimensional turbulence. Phys. Fluids 1967, 10, 1417. [Google Scholar] [CrossRef]
- Boffetta, G.; Ecke, R.E. Two-dimensional turbulence. Annu. Rev. Fluid Mech. 2012, 44, 427. [Google Scholar] [CrossRef]
- Francois, N.; Xia, H.; Punzmann, H.; Faber, B.; Shats, M. Braid entropy of two-dimensional turbulence. Sci. Rep. 2015, 5, 18564. [Google Scholar] [CrossRef] [PubMed]
- Colombi, R.; Schluter, M.; von Kameke, A. Three-dimensional flows beneath a thin layer of 2D turbulence induced by Faraday waves. Exp. Fluids 2021, 62, 8. [Google Scholar] [CrossRef]
- Colombi, R.; Rohde, N.; Schluter, M.; von Kameke, A. Coexistence of Inverse and Direct Energy Cascades in Faraday Waves. Fluids 2022, 7, 148. [Google Scholar] [CrossRef]
- Xia, H.; Francois, N.; Punzmann, H.; Shats, M. Tunable diffusion in wave-driven two-dimensional turbulence. J. Fluid Mech. 2019, 865, 811–830. [Google Scholar] [CrossRef]
- Kellay, H.; Wu, X.-L.; Goldburg, W.I. Experiments with turbulent soap films. Phys. Rev. Lett. 1995, 74, 3975. [Google Scholar] [CrossRef]
- Francois, N.; Lasne, D.; Amarouchene, Y.; Lounis, B.; Kellay, H. Drag enhancement with polymers. Phys. Rev. Lett. 2008, 100, 018302. [Google Scholar] [CrossRef]
- Francois, N.; Amarouchene, Y.; Lounis, B.; Kellay, H. Polymer conformations and hysteretic stresses in nonstationary flows of polymer solutions. EPL (Europhys. Lett.) 2009, 86, 34002. [Google Scholar] [CrossRef]
- Francois, N.; Xia, H.; Punzmann, H.; Combriat, T.; Shats, M. Inhibition of wave-driven two-dimensional turbulence by viscoelastic films of proteins. Phys. Rev. E 2015, 92, 023027. [Google Scholar] [CrossRef]
- Couder, Y.; Protiere, S.; Fort, E.; Boudaoud, A. Dynamical phenomena: Walking and orbiting droplets. Nature 2005, 437, 208. [Google Scholar] [CrossRef]
- Bush, J.W. Pilot-wave hydrodynamics. Ann. Rev. Fluid Mech. 2015, 47, 269–292. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Davoodianidalik, M.; Kellay, H.; Francois, N. A Hydrodynamic Analog of the Casimir Effect in Wave-Driven Turbulent Flows. Fluids 2022, 7, 155. https://doi.org/10.3390/fluids7050155
Davoodianidalik M, Kellay H, Francois N. A Hydrodynamic Analog of the Casimir Effect in Wave-Driven Turbulent Flows. Fluids. 2022; 7(5):155. https://doi.org/10.3390/fluids7050155
Chicago/Turabian StyleDavoodianidalik, Mahdi, Hamid Kellay, and Nicolas Francois. 2022. "A Hydrodynamic Analog of the Casimir Effect in Wave-Driven Turbulent Flows" Fluids 7, no. 5: 155. https://doi.org/10.3390/fluids7050155
APA StyleDavoodianidalik, M., Kellay, H., & Francois, N. (2022). A Hydrodynamic Analog of the Casimir Effect in Wave-Driven Turbulent Flows. Fluids, 7(5), 155. https://doi.org/10.3390/fluids7050155





