On the Thermodynamics of Self-Organization in Dissipative Systems: Reflections on the Unification of Physics and Biology
Abstract
:1. Introduction
1.1. Dissipative Structures and Organisms
- The structure and function of a dissipative structure arises from processes within the system, while the structure of a machine is a result of external design;
- A dissipative structure is created and maintained by entropy-generating and Gibbs- or Helmholtz-energy-dissipating irreversible processes. In contrast, a machine’s structure does not require any entropy generation, and in fact, a machine becomes more efficient with decreasing entropy generation: an ideal machine has no losses and produces no entropy. It is a fundamental difference between the two classes of systems;
- Generally, the design of a machine is based on reversible laws of mechanics, while dissipative structures are described using irreversible thermodynamic processes. It could be argued that mechanics gave us machines, while the thermodynamic theory of dissipative structures is the foundation for a science of biological organisms;
- Dissipative structures are stable and self-healing in the sense that if the structure is perturbed, the processes that created it can also restore it. The processes that create the structure also “heal” the structure from damage, an important property of all living organisms. With rare exceptions that we discuss below, machines are generally not self-healing;
- Machines are designed to perform a specific function, which they do independent of the context. End-directed behavior in dissipative structures shows context-dependent behavior [15].
1.2. Optimality and Final Cause
1.3. Side-Effects and Control
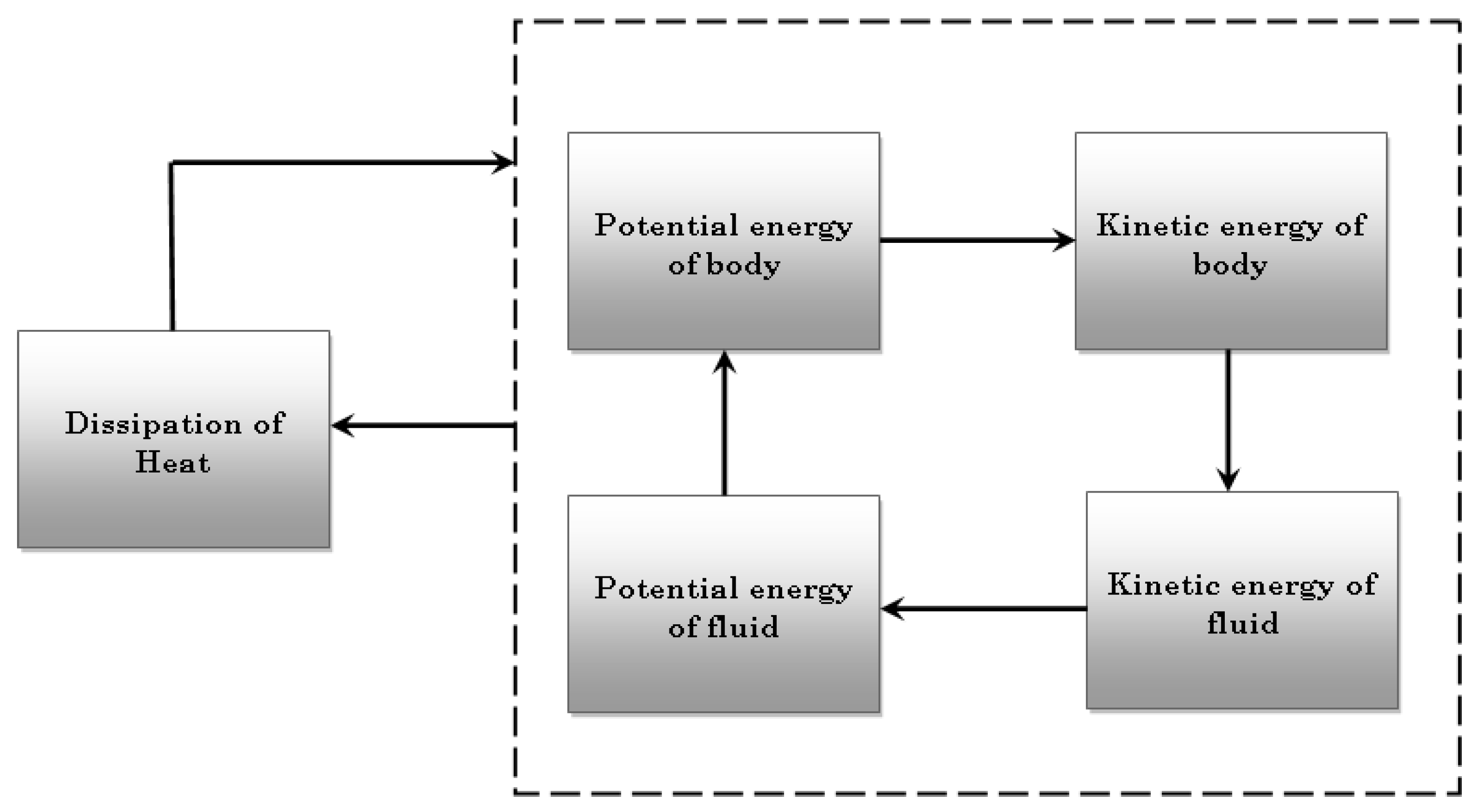
2. Theory of Dissipative Systems
3. Self-Organization in Fluids
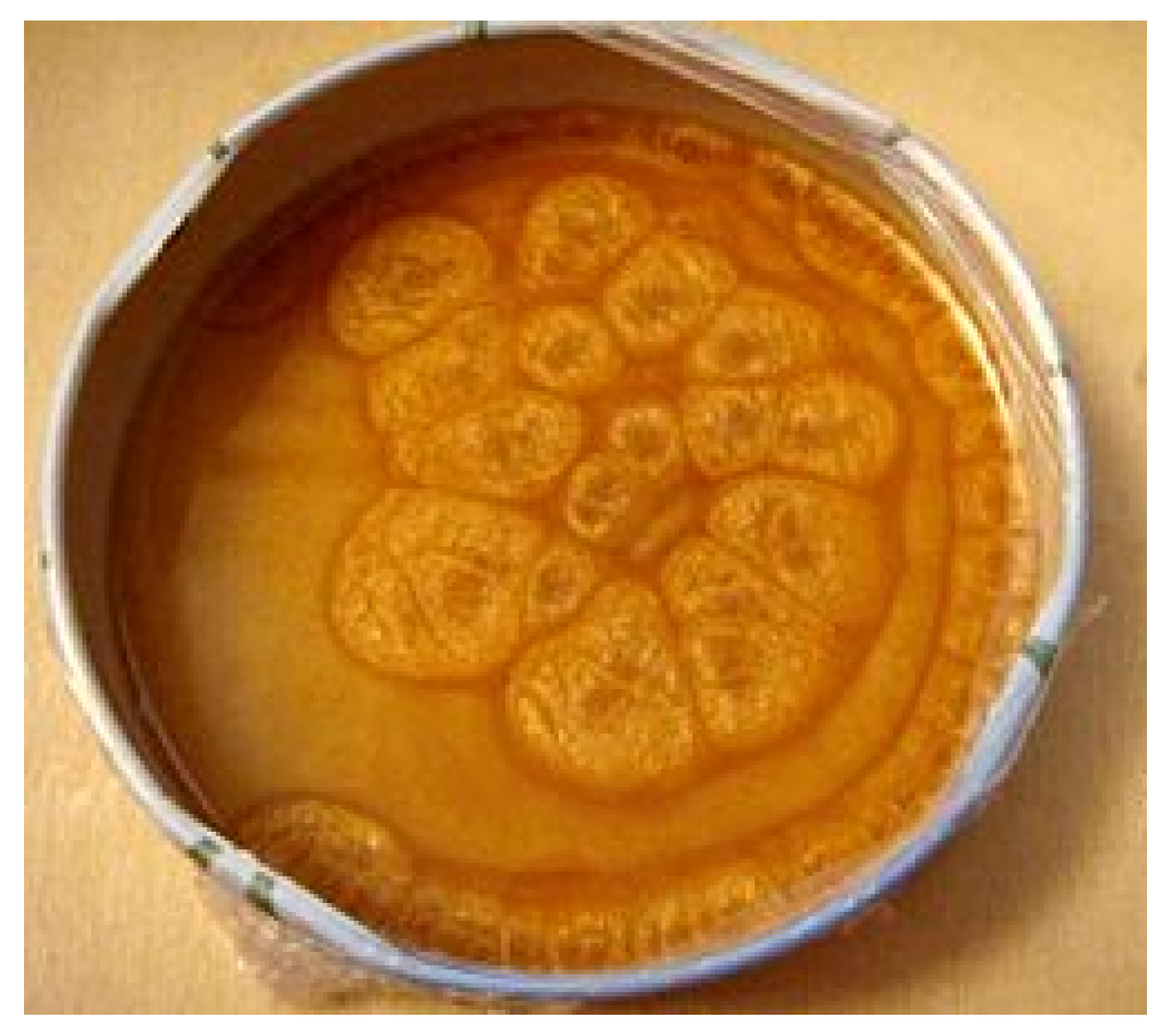
3.1. Benard Convection
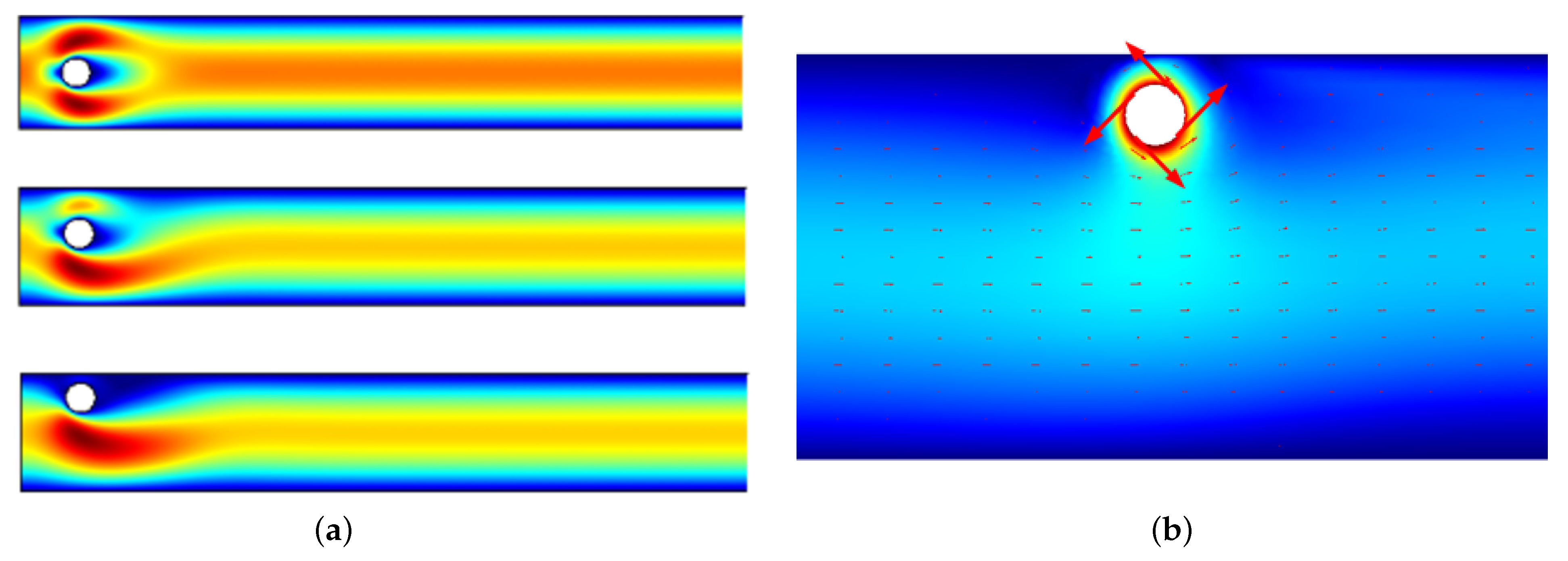
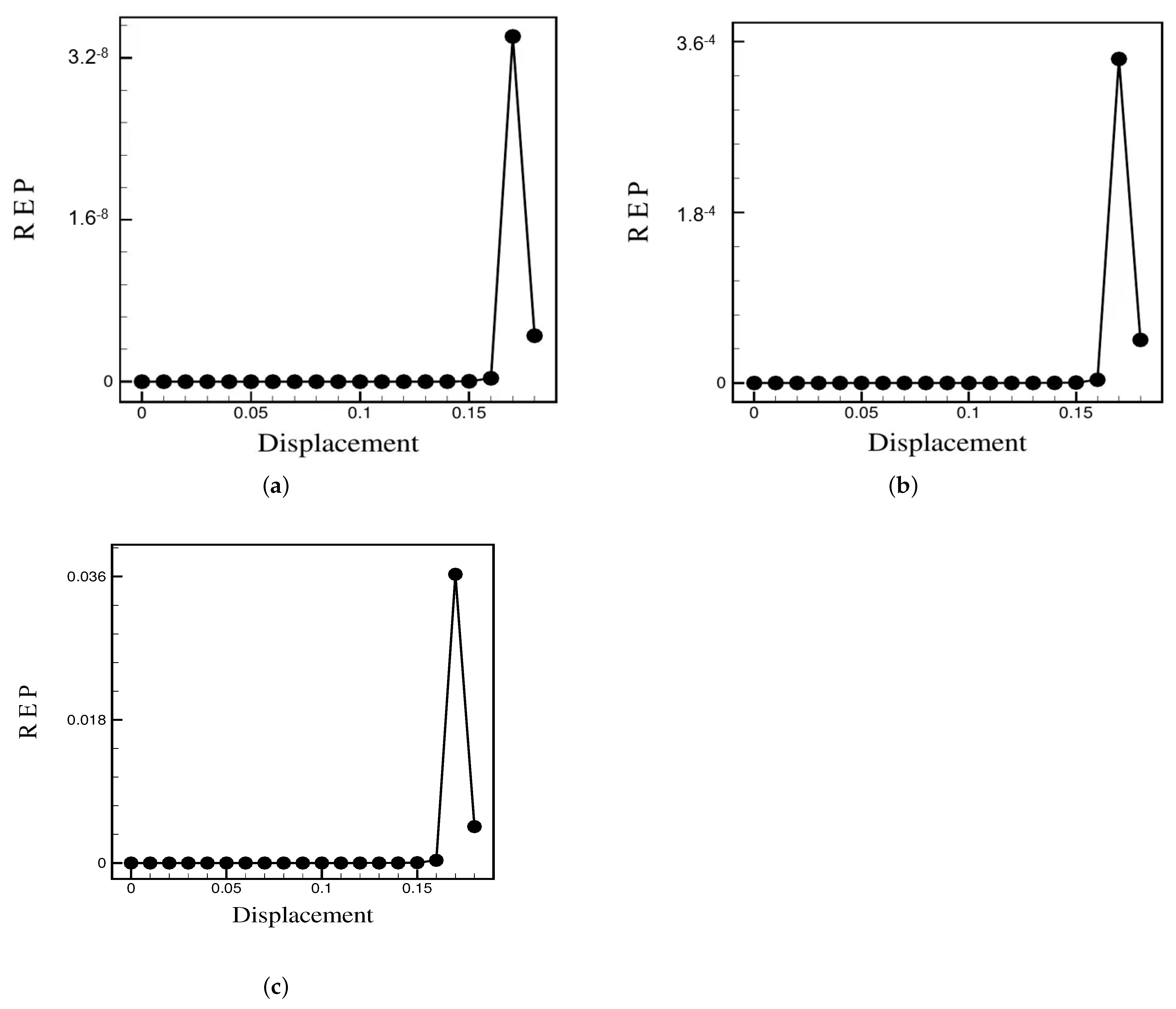
3.2. Pattern Selection of a Rigid Body in a Fluid
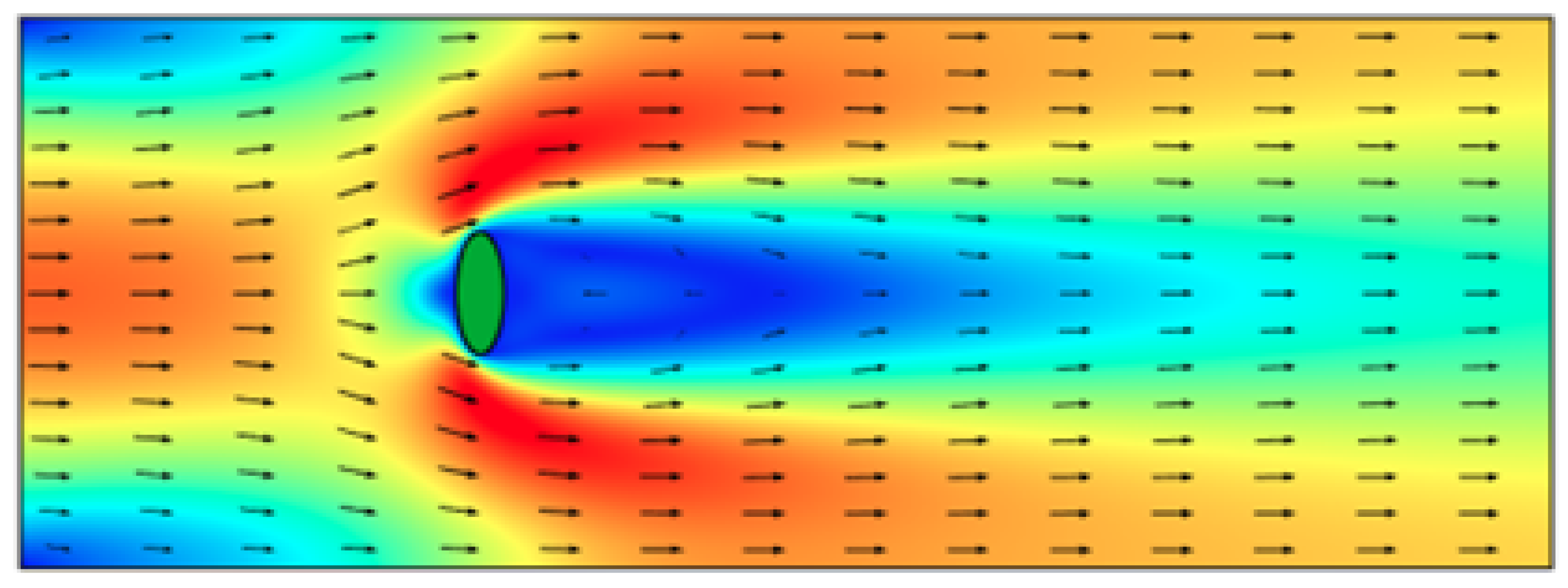
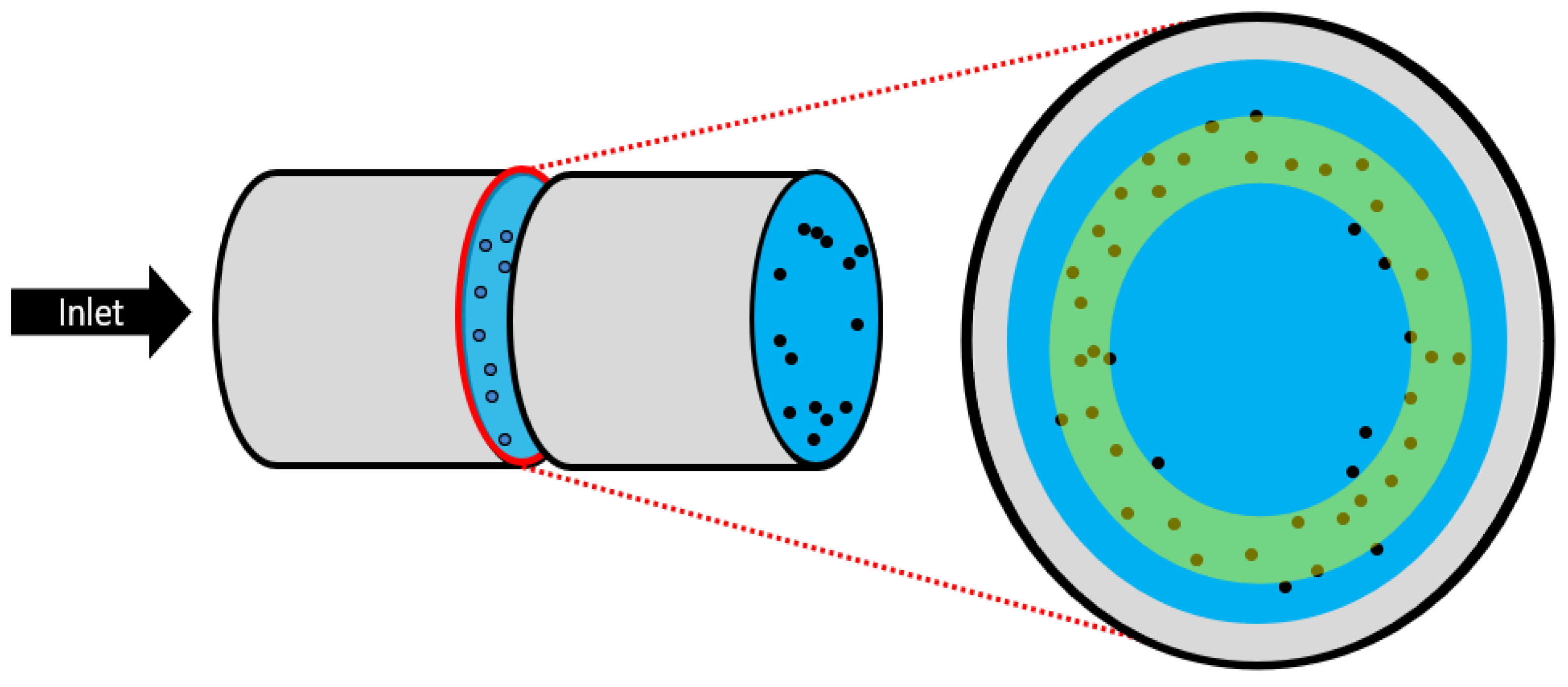
3.3. The Segré–Silberberg Effect
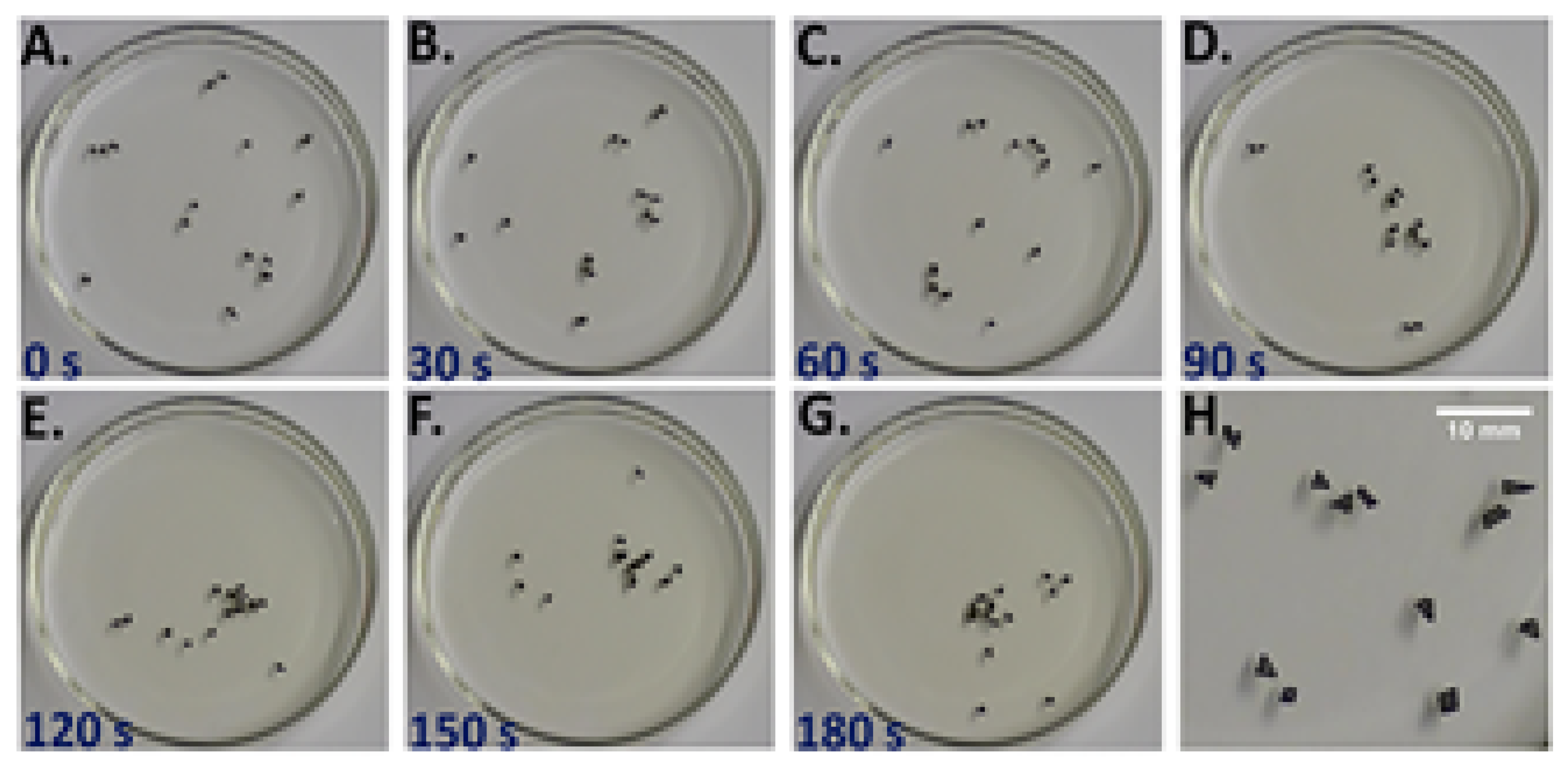
3.4. Benzoquinone Particles at the Air–Water Interface
4. Self-Organization in Nature
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Rosen, R. Life Itself: A Comprehensive Inquiry into the Nature, Origin, and Fabrication of Life; Columbia University Press: Columbia, UK, 1991. [Google Scholar]
- Rosen, R. Essays on Life Itself; Columbia University Press: Columbia, UK, 2000. [Google Scholar]
- Friston, K. Life as we know it. J. R. Soc. Interface 2013, 10, 20130475. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dawkins, R. The Selfish Gene; Oxford University Press: Oxford, UK, 2016. [Google Scholar]
- Bertalanffy, L.V. General system theory: Foundations, development, applications. JAMA 1969, 208, 870. [Google Scholar] [CrossRef]
- Gibson, J.J. The Ecological Approach to Visual Perception: Classic Edition; Psychology Press: New York, NY, USA, 2014. [Google Scholar]
- Goldenfeld, N.; Woese, C. Life is physics: Evolution as a collective phenomenon far from equilibrium. Annu. Rev. Condens. Matter Phys. 2011, 2, 375–399. [Google Scholar] [CrossRef] [Green Version]
- Vitas, M.; Dobovišek, A. Towards a general definition of life. Orig. Life Evol. Biosph. 2019, 49, 77–88. [Google Scholar] [CrossRef]
- Davis, T.J.; Kay, B.A.; Kondepudi, D.; Dixon, J.A. Spontaneous interentity coordination in a dissipative structure. Ecol. Psychol. 2016, 28, 23–36. [Google Scholar] [CrossRef]
- Prigogine, I.; Nicolis, G.; Babloyantz, A. Thermodynamics of Evolution. Phys. Today 1972, 25, 23. [Google Scholar] [CrossRef]
- Nicolis, G.; Prigogine, I. Self-Organization in Non-Equilibrium Systems; Wiley: New York, NY, USA, 1977. [Google Scholar]
- Prigogine, I.; Stengers, I. Order Out of Chaos: Man’S New Dialogue with Nature; Bantam Books: New York, NY, USA, 1984. [Google Scholar]
- Kondepudi, D.; Kay, B.; Dixon, J. End-directed evolution and the emergence of energy-seeking behavior in a complex system. Phys. Rev. E 2015, 91, 050902. [Google Scholar] [CrossRef] [Green Version]
- Kondepudi, D.; Kay, B.; Dixon, J. Dissipative structures, machines, and organisms: A perspective. Chaos 2017, 27, 104607. [Google Scholar] [CrossRef]
- Dixon, J.A.; Kay, B.A.; Davis, T.J.; Kondepudi, D. End-directedness and context in nonliving dissipative systems. In Contextuality from Quantum Physics to Psychology; Dzhafarov, E., Jordan, S., Zhang, R., Cervantes, V., Eds.; World Scientific: Singapore, 2016. [Google Scholar]
- Chung, B.J.; McDermid, K.; Vaidya, A. On the affordances of the MaxEP principle. Eur. Phys. J. B 2014, 87, 20. [Google Scholar] [CrossRef]
- Doll, W.E., Jr. Foundations for a post-modern curriculum. J. Curric. Stud. 1989, 21, 243–253. [Google Scholar] [CrossRef]
- Braithwait, R.B. Causal and teleological explanation. In Purpose in Nature; Canfield, J.V., Ed.; Prentice Hall: Hoboken, NJ, USA, 1966; pp. 27–47. [Google Scholar]
- Feynman, R.P.; Robert, B.L.; Matthew, S. The principle of least action. Feynman Lect. Phys. 1964, 2, 19-1. [Google Scholar]
- Wiener, N.; Rosenbluth, A.; Bigelow, J. Behavior, Purpose and Teleogie. Philos. Sci. 1943, 1, 18–24. [Google Scholar]
- Kugler, P.N.; Shaw, R.E.; Vincente, K.J.; Kinsella-Shaw, J. Inquiry into intentional systems I: Issues in ecological physics. Psychol. Res. 1990, 52, 98–121. [Google Scholar] [CrossRef]
- Schoemaker, P.J. The quest for optimality: A positive heuristic of science? Behav. Brain Sci. 1991, 14, 205–215. [Google Scholar] [CrossRef]
- Wiener, N. Cybernetics or Control and Communication in the Animal and the Machine; MIT Press: Cambridge, MA, USA, 1961; Volume 25. [Google Scholar]
- De Bari, B.; Paxton, A.; Kondepudi, D.K.; Kay, B.A.; Dixon, J.A. Functional Interdependence in Coupled Dissipative Structures: Physical Foundations of Biological Coordination. Entropy 2021, 23, 614. [Google Scholar] [CrossRef] [PubMed]
- De Bari, B.; Dixon, J.A.; Kay, B.A.; Kondepudi, D. Oscillatory dynamics of an electrically driven dissipative structure. PLoS ONE 2019, 14, e0217305. [Google Scholar] [CrossRef]
- De Bari, B.; Dixon, J.; Pateras, J.; Rusling, J.; Satterwhite-Warden, J.; Vaidya, A. A thermodynamic analysis of end-directed particle flocking in chemical systems. Commun. Nonlinear Sci. Numer. Simul. 2021, 106, 106107. [Google Scholar] [CrossRef]
- Martyushev, L.M.; Seleznev, V.D. Maximum entropy production principle in physics, chemistry and biology. Phys. Rep. 2006, 426, 1–45. [Google Scholar] [CrossRef]
- Prigogine, I.; Isabelle, S. Order out of Chaos: Man’s New Dialogue with Nature; Verso Books: London, UK, 2018. [Google Scholar]
- Chen, T.; Kondepudi, D.K.; Dixon, J.A.; Rusling, J.F. Particle Flock Motion at Air–Water Interface Driven by Interfacial Free Energy Foraging. Langmuir 2019, 35, 11066–11070. [Google Scholar] [CrossRef]
- Chung, B.J.; Ashwin, V. An optimal principle in fluid–structure interaction. Phys. D Nonlinear Phenom. 2008, 237, 2945–2951. [Google Scholar] [CrossRef]
- Chung, B.J.; Ashwin, V. Non-equilibrium pattern selection in particle sedimentation. Appl. Math. Comput. 2011, 218, 3451–3465. [Google Scholar] [CrossRef]
- Chung, B.J.; Blas, O.; Ashwin, V. Entropy production in a fluid–solid system far from thermodynamic equilibrium. Eur. Phys. J. E 2017, 40, 105. [Google Scholar] [CrossRef] [PubMed]
- Onsager, L. Reciprocal relations in irreversible processes. I. Phys. Rev. 1931, 37, 405. [Google Scholar] [CrossRef]
- Onsager, L. Reciprocal relations in irreversible processes. II. Phys. Rev. 1931, 38, 450. [Google Scholar] [CrossRef] [Green Version]
- Ziegler, H. An Introduction to Thermomechanics; North-Holland: Amsterdam, The Netherlands, 1983. [Google Scholar]
- Bodenschatz, E.; Werner, P.; Guenter, A. Recent developments in Rayleigh–Benard convection. Annu. Rev. Fluid Mech. 2000, 32, 709–778. [Google Scholar] [CrossRef] [Green Version]
- Busse, F.H. Non-linear properties of thermal convection. Rep. Prog. Phys. 1929, 41, 1929. [Google Scholar] [CrossRef]
- Chatterjee, A.; Mears, N.; Yadati, Y.; Iannacchione, G.S. An overview of emergent order in far-from-equilibrium driven systems: From kuramoto oscillators to rayleigh–bénard convection. Entropy 2020, 22, 561. [Google Scholar] [CrossRef]
- Cross, M.C.; Hohenberg, P.C. Pattern formation outside of equilibrium. Rev. Mod. Phys. 1993, 65, 851. [Google Scholar] [CrossRef] [Green Version]
- Bejan, A. Shape and Structure, from Engineering to Nature; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar]
- Bejan, A.; Marden, J.H. Unifying constructal theory for scale effects in running, swimming and flying. J. Exp. Biol. 2006, 209, 238–248. [Google Scholar] [CrossRef] [Green Version]
- Bejan, S.; Lorente, S. The constructal law of design and evolution in nature. Philos. Trans. R. Soc. B 2010, 365, 1335–1347. [Google Scholar] [CrossRef] [Green Version]
- File: Benard Cells Convection.ogv. (23 September 2013). Wikimedia Commons, the Free Media Repository. Available online: https://commons.wikimedia.org/w/index.php?title=File:B%C3%A9nard_cells_convection.ogv\&oldid=105140182 (accessed on 2 July 2018).
- Makela, T.; Annila, A. Natural patterns of energy dispersal. Phys. Life Rev. 2010, 7, 477–498. [Google Scholar] [CrossRef] [PubMed]
- Galdi, G.P. On the Motion of a Rigid Body in a Viscous Fluid: A Mathematical Analysis with Applications. In Handbook of Mathematical Fluid Mechanics; Elsevier Science: Amsterdam, The Netherlands, 2002; pp. 653–791. [Google Scholar]
- Chung, B.J.; Cohrs, M.; Ernst, W.; Galdi, G.P.; Vaidya, A. Wake-cylinder interaction at low and intermediate Reynolds numbers. Arch. Appl. Mech. 2016, 86, 627–641. [Google Scholar] [CrossRef]
- Segre, G.; Silberberg, A. Radial particle displacements in Poiseuille flow of suspensions. Nature 1961, 189, 209–210. [Google Scholar] [CrossRef]
- Choi, C.R.; Kim, C.N. Inertial migration and multiple equilibrium positions of a neutrally buoyant spherical particle in Poiseuille flow. Korean J. Chem. Eng. 2010, 27, 1076–1086. [Google Scholar] [CrossRef]
- Mitchell, H.W.; Spagnolie, S.E. Sedimentation of spheroidal bodies near walls in viscous fluids: Glancing, reversing, tumbling, and sliding. J. Fluid Mech. 2015, 772, 600–629. [Google Scholar] [CrossRef] [Green Version]
- Shao, X.; Yu, Z.; Sun, B. Inertial migration of spherical particles in circular Poiseuille flow at moderately high Reynolds numbers. Phys. Fluids 2008, 20, 103307. [Google Scholar] [CrossRef] [Green Version]
- Yang, B.H.; Wang, J.; Joseph, D.D.; Hu, H.H.; Pan, T.W.; Glowinski, R. Migration of a sphere in tube flow. J. Fluid Mech. 2005, 540, 109–131. [Google Scholar] [CrossRef] [Green Version]
- Morita, Y.; Itano, T.; Sugihara-Seki, M. Equilibrium radial positions of neutrally buoyant spherical particles over the circular cross-section in Poiseuille flow. J. Fluid Mech. 2017, 813, 750–767. [Google Scholar] [CrossRef]
- Happel, J.; Brenner, H. Low Reynolds Number Hydrodynamics: With Special Applications to Particulate Medial; Springer Science and Business Media: New York, NY, USA, 2012; Volume 1. [Google Scholar]
- Satterwhite-Warden, J.E.; Kondepudi, D.K.; Dixon, J.A.; Rusling, J.F. Co-operative motion of multiple benzoquinone disks at the air–water interface. Phys. Chem. Chem. Phys. 2015, 17, 29891–29898. [Google Scholar] [CrossRef]
- Satterwhite-Warden, J.E.; Kondepudi, D.K.; Dixon, J.A.; Rusling, J.F. Thermal-and Magnetic-Sensitive Particle Flocking Motion at the Air–Water Interface. J. Phys. Chem. B 2019, 123, 3832–3840. [Google Scholar] [CrossRef]
- Kohira, M.I.; Hayashima, Y.; Nagayama, M.; Nakata, S. Synchronized self-motion of two camphor boats. Langmuir 2001, 17, 7124–7129. [Google Scholar] [CrossRef]
- Howe, H.F.; Smallwood, J. Ecology of seed dispersal. Annu. Rev. Ecol. Syst. 1982, 13, 201–228. [Google Scholar] [CrossRef]
- Minami, S.; Azuma, A. Various flying modes of wind-dispersal seeds. J. Theor. Biol. 2003, 225, 1–14. [Google Scholar] [CrossRef]
- Kaila, V.R.; Annila, A. Natural selection for least action. R. Soc. Lond. Math. Phys. Eng. Sci. 2008, 464, 3055–3070. [Google Scholar] [CrossRef] [Green Version]
- Sharma, V.; Annila, A. Natural process? Natural selection. Biophys. Chem. 2007, 127, 123–128. [Google Scholar] [CrossRef] [Green Version]
- Vallino, J.J.; Huber, J.A. Using maximum entropy production to describe microbial biogeochemistry over time and space in a meromictic pond. Front. Environ. Sci. 2018, 6, 100. [Google Scholar] [CrossRef] [Green Version]
- Kondepudi, D. Self-organization, entropy production, and physical intelligence. Ecol. Psychol. 2012, 24, 33–45. [Google Scholar] [CrossRef]
- Adler, J. Chemotaxis in bacteria. Science 1966, 153, 708–716. [Google Scholar] [CrossRef]
- Dobovišek, A.; Županović, P.; Brumen, M.; Juretić, D. Maximum entropy production and maximum Shannon entropy as germane principles for the evolution of enzyme kinetics. In Beyond the Second Law; Springer: Berlin/Heidelberg, Germany, 2014; pp. 361–382. [Google Scholar]
- Goldbeter, A. Dissipative structures and biological rhythms. Chaos 2017, 27, 104612. [Google Scholar] [CrossRef]
- Goldbeter, A. Biochemical Oscillations and Cellular Rhythms. In The Molecular Bases of Periodic and Chaotic Behaviour; Cambridge University Press: Cambridge, UK, 1996. [Google Scholar]
- Horne, C.; Smith, C.A.; Karamcheti, K. Aeroacoustic and Aerodynamic Applications of the Theory of Nonequilibrium Thermodynamics; NASA Technical Paper 3118; NASA: Washington, DC, USA, 1991. [Google Scholar]
- Ghesselini, R. Elastic free energy of an upper convected maxwell fluid undergoing fully developed planar poiseuille flow: A variational result. J. Non-Newton. Fluid Mech. 1993, 46, 229–241. [Google Scholar] [CrossRef]
- Niven, R.K. Steady state of a dissipative flow-controlled system and the maximum entropy production principle. Phys. Rev. E 2009, 80, 2. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Vaidya, A. MaxEP and Stable Configurations in Fluid Solid Interactions, Beyond the Second Law: Entropy Production and Non-Equilibrium Systems; Book Series: Understanding Complex Systems; Dewar, R.C., Lineweaver, C., Niven, R., Regenauer-Lieb, K., Eds.; Springer: New York, NY, USA, 2013. [Google Scholar]
- Galdi, G.P.; Vaidya, A.; Pokorný, M.; Joseph, D.D.; Feng, J. Orientation of symmetric bodies falling in a second-order liquid at nonzero Reynolds number. Math. Models Methods Appl. Sci. 2002, 12, 1653–1690. [Google Scholar] [CrossRef] [Green Version]
- Allaire, R.; Guerron, P.; Nita, B.; Nolan, P.; Vaidya, A. On the equilibrium configurations of flexible fibers in a flow. Int. J. Non-Linear Mech. 2015, 69, 157–165. [Google Scholar] [CrossRef]
- Joseph, D.D.; Liu, Y.J.; Poletto, M.; Feng, J. Aggregation and dispersion of spheres falling in viscoelastic liquids. J. Non-Newton. Fluid Mech. 1994, 54, 45–86. [Google Scholar] [CrossRef]
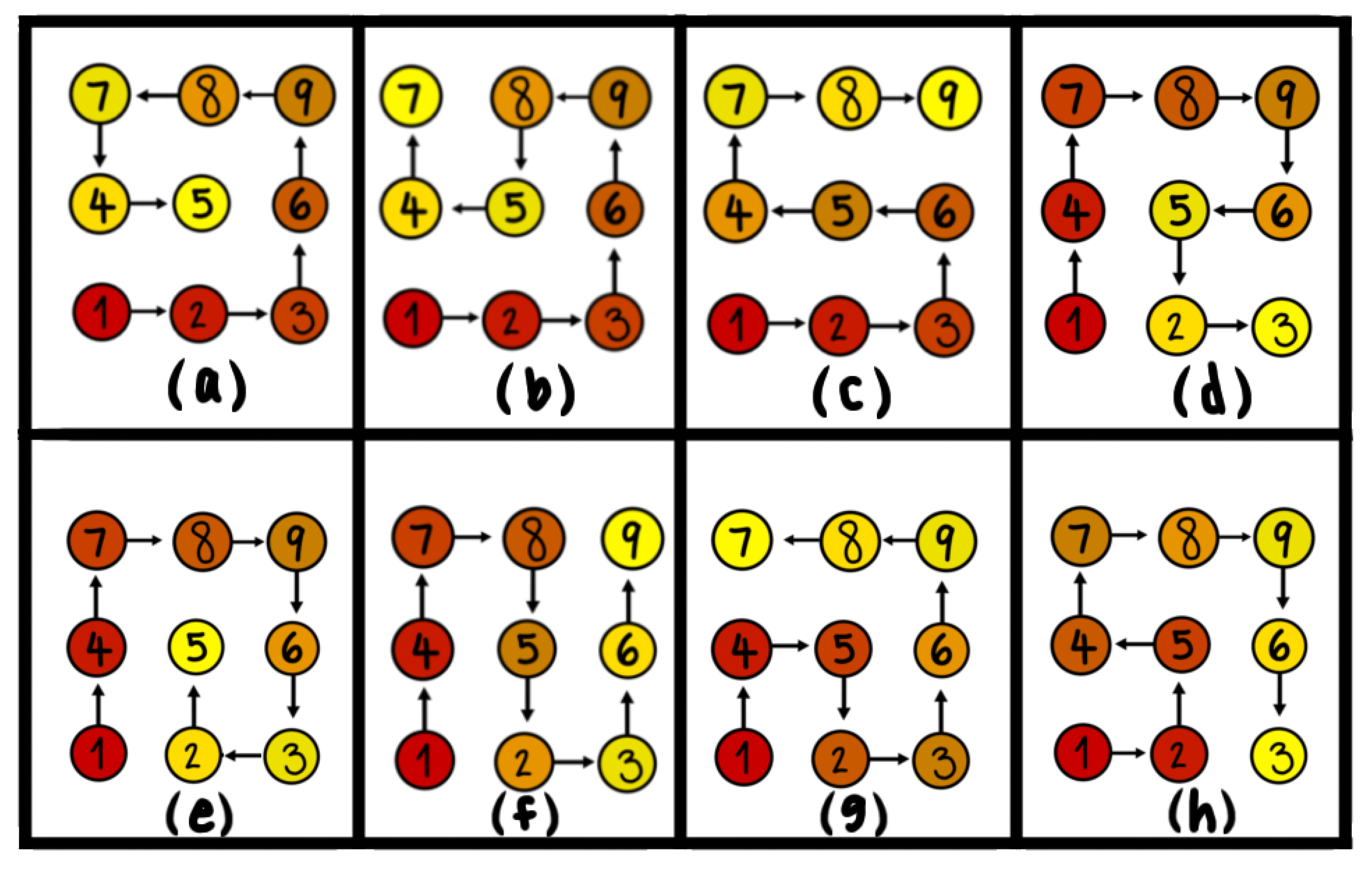

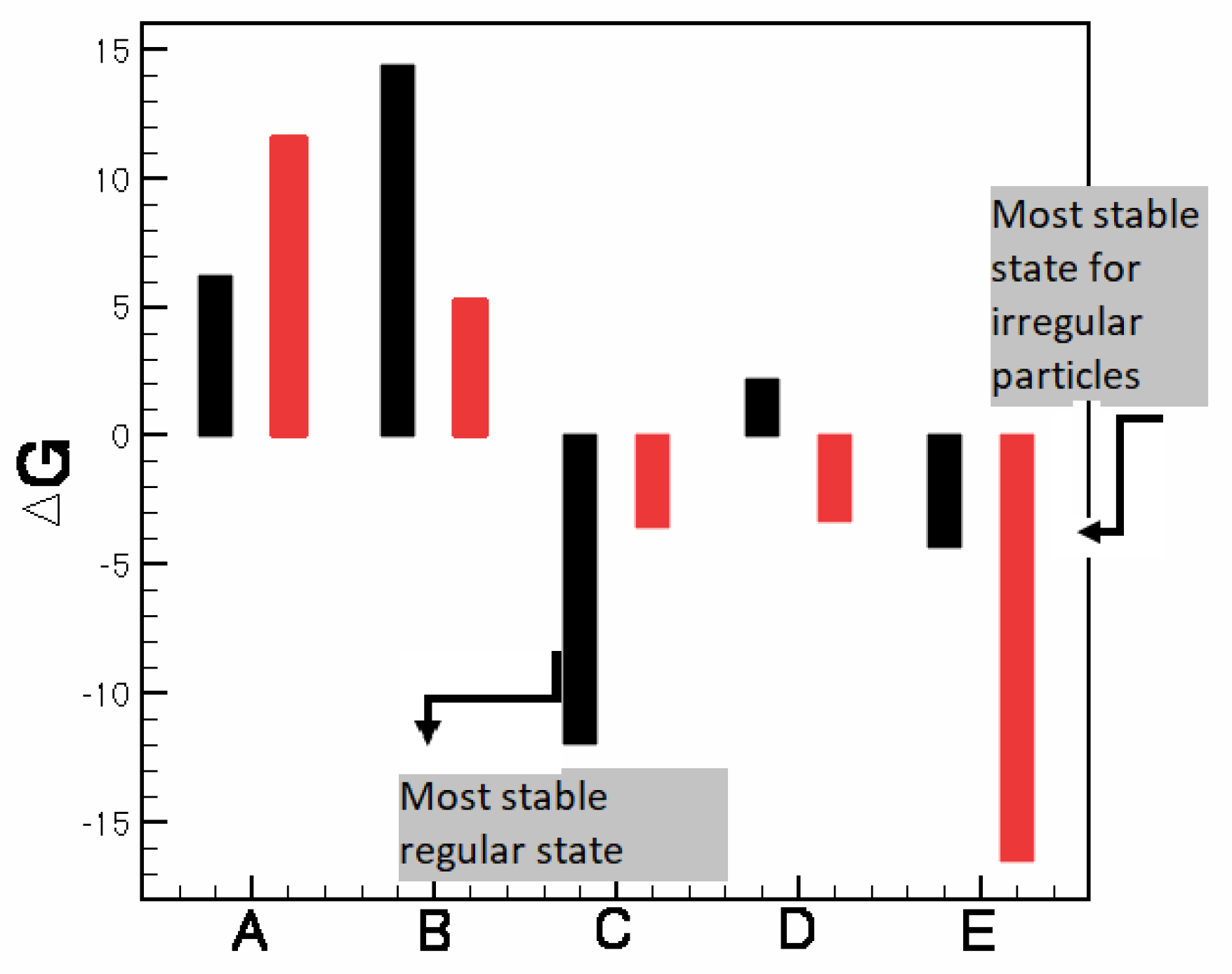
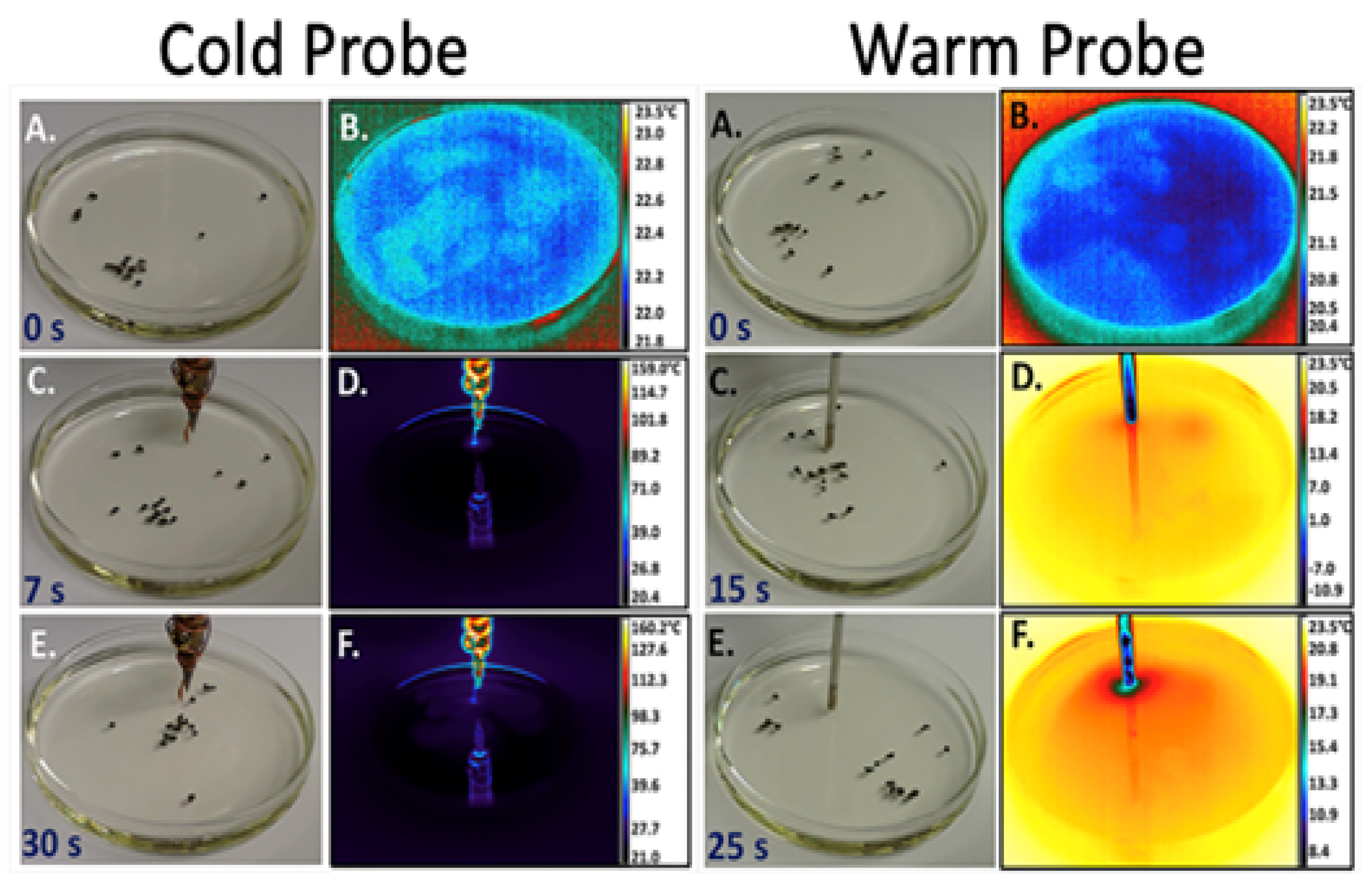
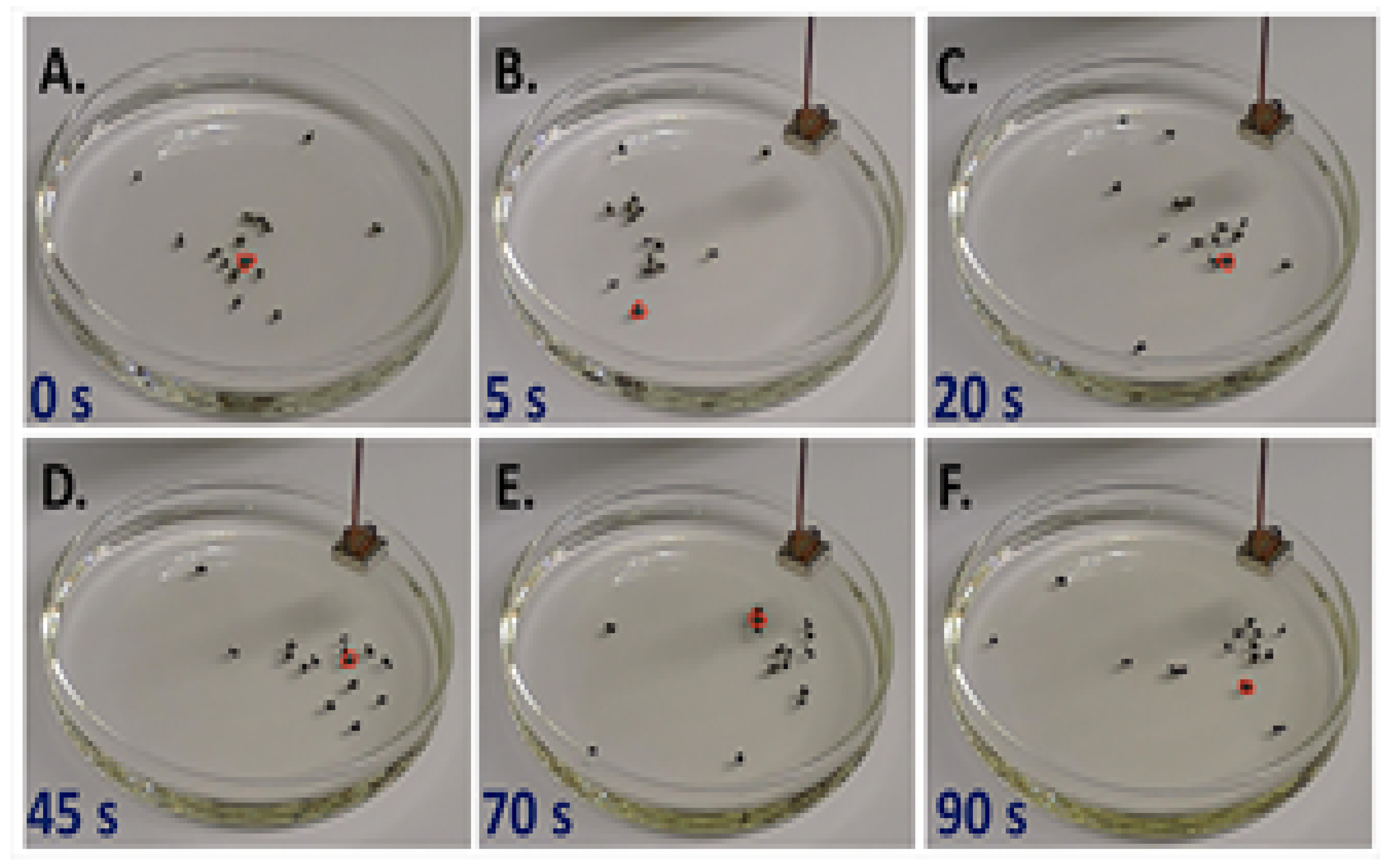

| n (→) m (↓) | 2 | 3 | 4 | 5 |
| 2 | 2 | 3 | 4 | 5 |
| 3 | 3 | 8 | 13 | 76 |
| 4 | 4 | 13 | 52 | 165 |
| 5 | 4 | 76 | 165 | 702 |
| Physical Problem | Forces | Gradient | References | Thermodynamic Principle | |
|---|---|---|---|---|---|
| 1. | Channel Flow | viscous | velocity | [67,68,69] | MEP |
| 2. | Orientation in a NF | viscous | velocity | [30,40,70] | MEP, CT |
| 3. | Orientation in a VEF | viscous, elastic | velocity | [70,71] | MEP |
| 4. | Deformable body in a NF | viscous, spring | velocity | [16,72] | MEP |
| 5. | Orientation of two spheres in a NF | viscous | velocity | [31,73] | MEP |
| 6. | Sphere falling near a wall in NF | viscous | velocity | Section 3.3 | MEP |
| 7. | Chemical flocking | viscous, chemical | Surface tension | [24,26] | MFE |
| 8. | Flocking in E&M field | viscous, magnetic | charge distribution | [25,26] | MEP |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chung, B.J.; De Bari, B.; Dixon, J.; Kondepudi, D.; Pateras, J.; Vaidya, A. On the Thermodynamics of Self-Organization in Dissipative Systems: Reflections on the Unification of Physics and Biology. Fluids 2022, 7, 141. https://doi.org/10.3390/fluids7040141
Chung BJ, De Bari B, Dixon J, Kondepudi D, Pateras J, Vaidya A. On the Thermodynamics of Self-Organization in Dissipative Systems: Reflections on the Unification of Physics and Biology. Fluids. 2022; 7(4):141. https://doi.org/10.3390/fluids7040141
Chicago/Turabian StyleChung, Bong Jae, Benjamin De Bari, James Dixon, Dilip Kondepudi, Joseph Pateras, and Ashwin Vaidya. 2022. "On the Thermodynamics of Self-Organization in Dissipative Systems: Reflections on the Unification of Physics and Biology" Fluids 7, no. 4: 141. https://doi.org/10.3390/fluids7040141
APA StyleChung, B. J., De Bari, B., Dixon, J., Kondepudi, D., Pateras, J., & Vaidya, A. (2022). On the Thermodynamics of Self-Organization in Dissipative Systems: Reflections on the Unification of Physics and Biology. Fluids, 7(4), 141. https://doi.org/10.3390/fluids7040141








