A Multi-Node Lumped Parameter Model Including Gravity and Real Gas Effects for Steady and Transient Analysis of Heat Pipes
Abstract
:1. Introduction and State of the Art
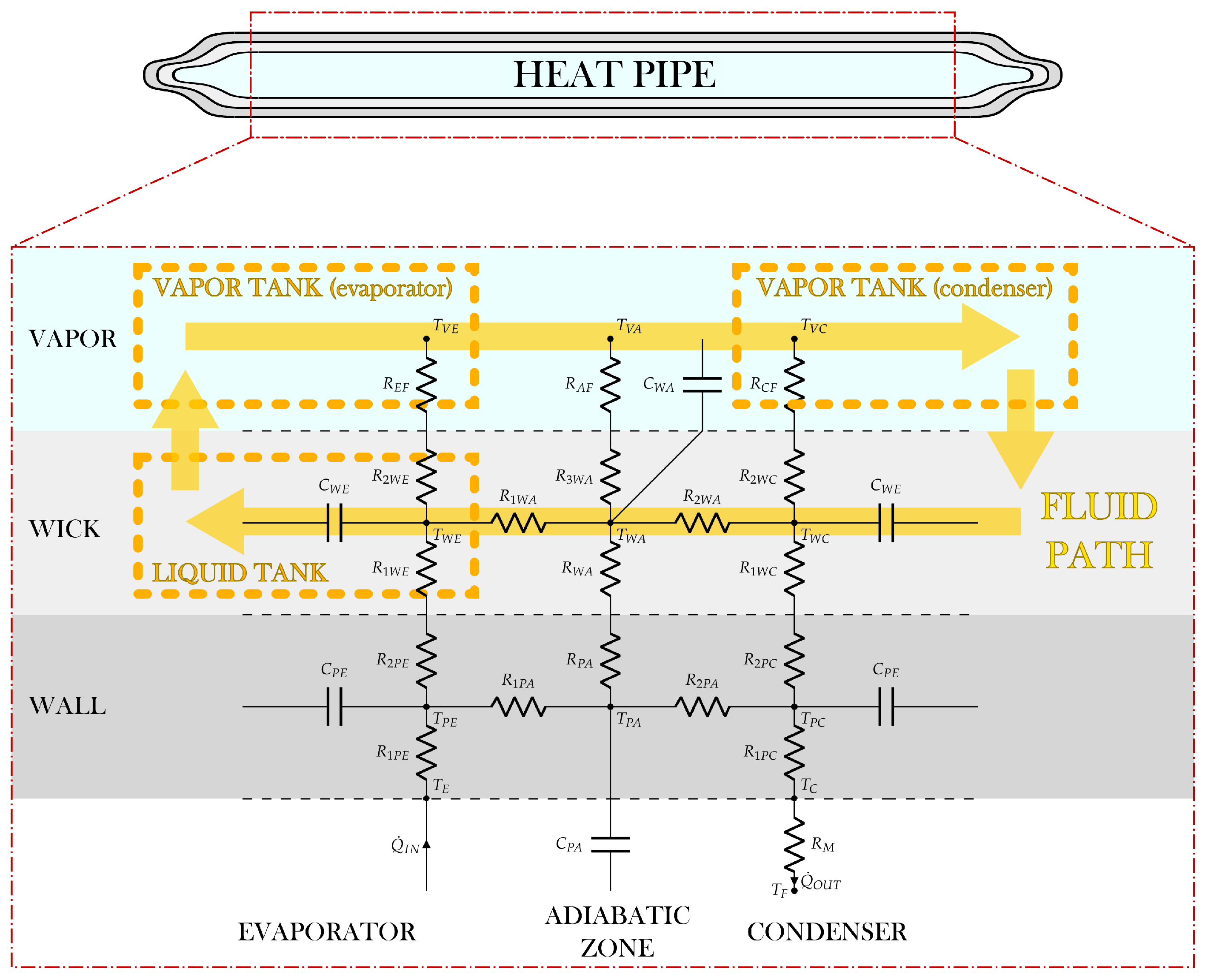
2. Heat Pipe Model Description
2.1. Solid Network
2.2. Fluid Network
- the fluid flow is one-dimensional and laminar for both phases;
- vapor transformations in the evaporator/condenser tanks are isoentropic;
- evaporation and condensation only occur in the evaporator and condenser zones, therefore, the mass flow rates of the phases do not change in the adiabatic region;
- the liquid temperature is exactly equal to the wick temperature for each point along the wick length;
- the thermophysical properties of the liquid, the surface tension, and the latent heat of vaporization are evaluated in saturation conditions;
- All the condensing fluid reaches the wick, with no “accumulation” before it.
2.2.1. Condensed Liquid
2.2.2. Vapor Tanks
2.2.3. Vapor Pressure–Temperature Relation
2.2.4. Evaporation and Condensation
2.2.5. Vapor Line
2.2.6. Liquid Line
2.3. Solid–Fluid Coupling
2.4. Liquid–Vapor Coupling
2.5. Implementation of the Complete Set
- Ten temperatures: ;
- Four pressures: ;
- Four mass flow rates: ;
- Two heat transfer rates: .
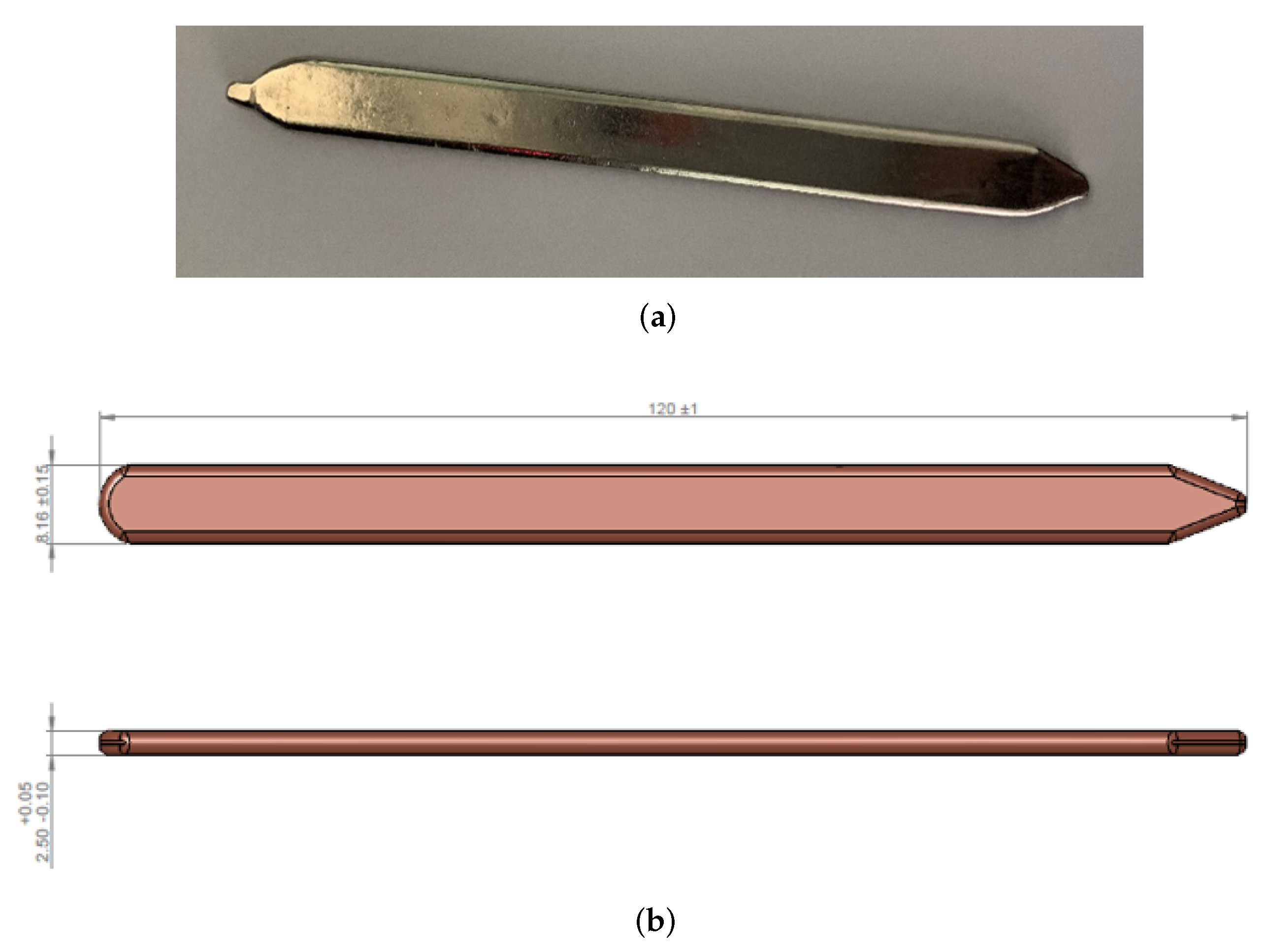
3. Case Study
- The thickness of the wall is equal to ;
- The thickness of the wick is equal to ;
- The wick porosity, , is approximately equal to ;
- The average grain radius is approximately equal to 100 μm.
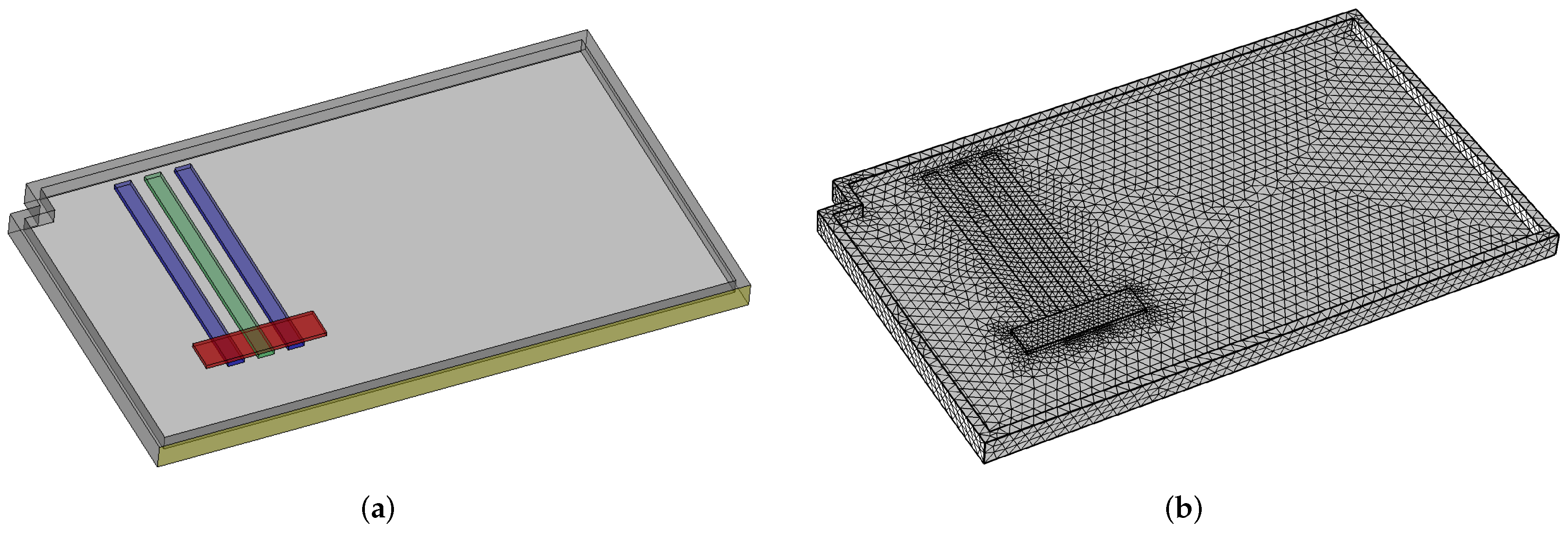
3.1. Description of the Experiment
3.2. Model Adaptation
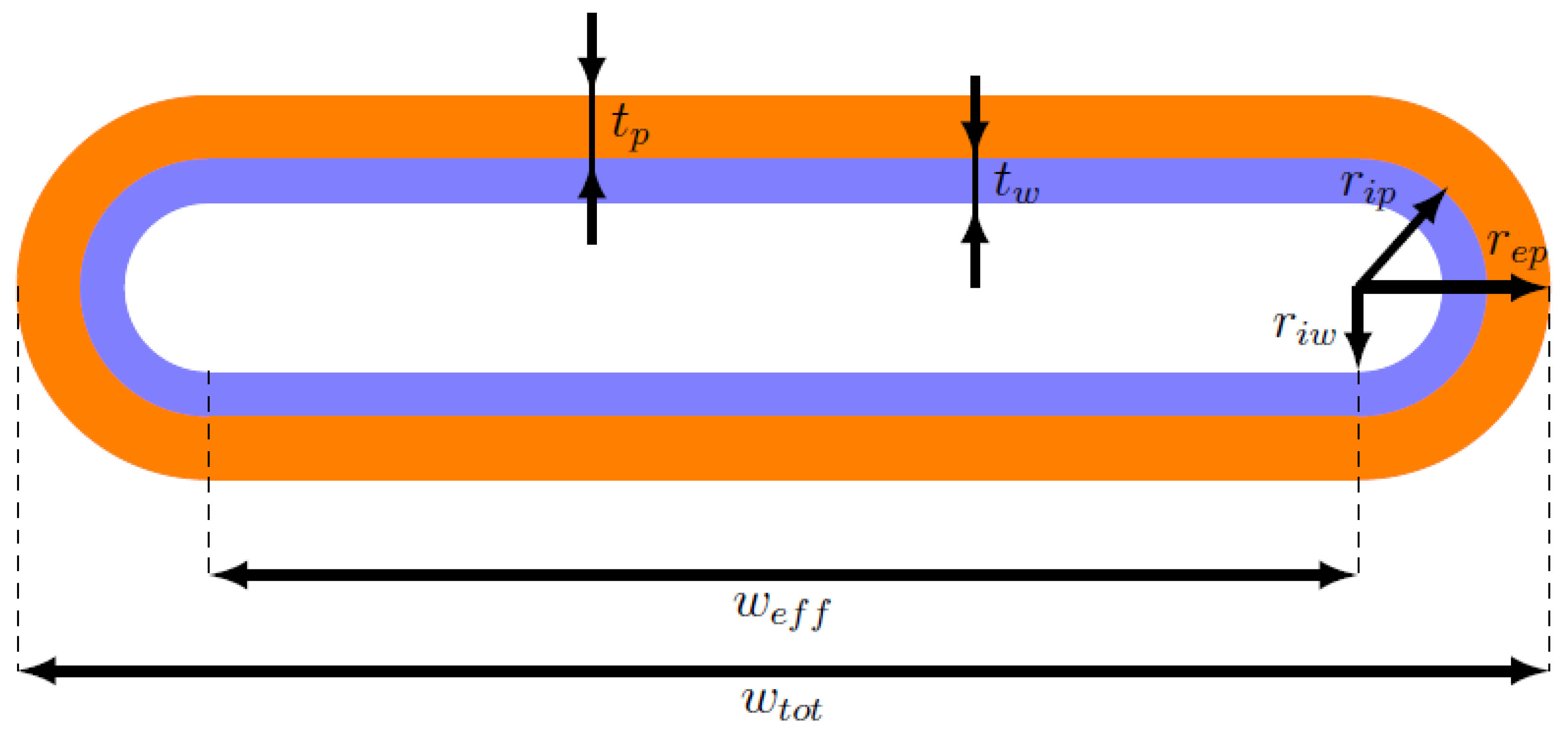
- , , and are the external wall, internal wall, and internal wick radii of the circular regions at the ends of the section, respectively;
- is the effective width of the section, which corresponds to the total width minus twice the external wall radius: ;
- is the wall thickness: ;
- is the wick thickness: .
3.2.1. Solid Network
3.2.2. Fluid Network
3.3. Extraction of Input Data
- is the saturation pressure evaluated at temperature ;
- is the liquid surface tension evaluated at temperature ;
- is the liquid molar volume, which is computed dividing the molar mass of the working fluid by the liquid density evaluated at temperature ;
- R is the universal gas constant;
- is the mean radius of curvature of the liquid–gas interface, and for this application, it is equal to the capillary radius, .
- An equivalent mass, computed as the sum of the masses of the solid, liquid, and vapor parts;
- An equivalent thermal conductivity, estimated by a preliminary run of the heat pipe model in a basic case;
- An equivalent thermal capacitance, computed as weighted average of the capacitances of the solid, liquid, and vapor parts.
- Their sides and rear face can exchange by conduction with the chassis cover;
- Their front face can exchange by natural convection with the air in the room;
- Their front face can exchange also by radiation with the walls of the room.
- The input thermal power, , is constant and equal for all the thermal charge phases, and it is null for all the thermal discharge phases;
- The value of the resistance is constant during the phases of charge and discharge of the same cycle, while it changes between the cycles to take into account the equivalent convective coefficient variation with temperature.
- For the thermal charge phases: ;
- For the thermal discharge phases: .
4. Results
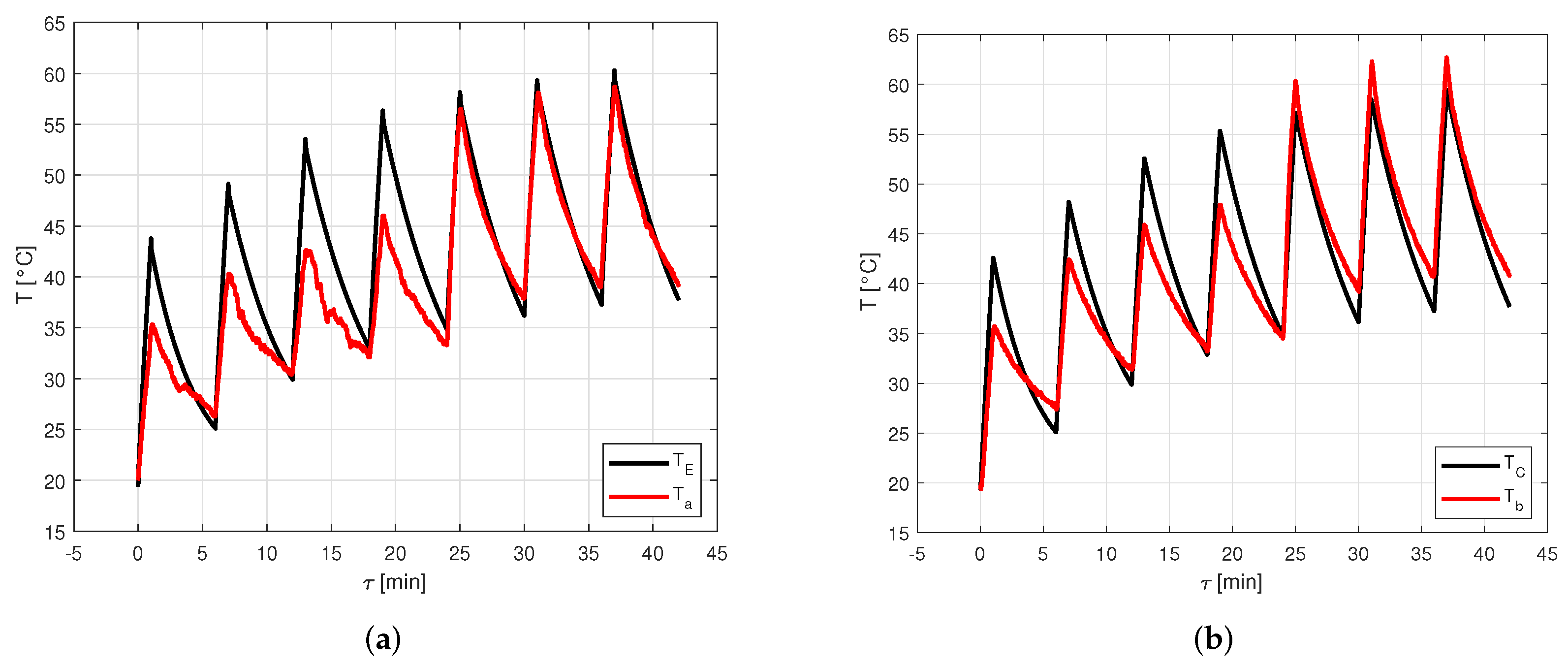
4.1. Model Validation
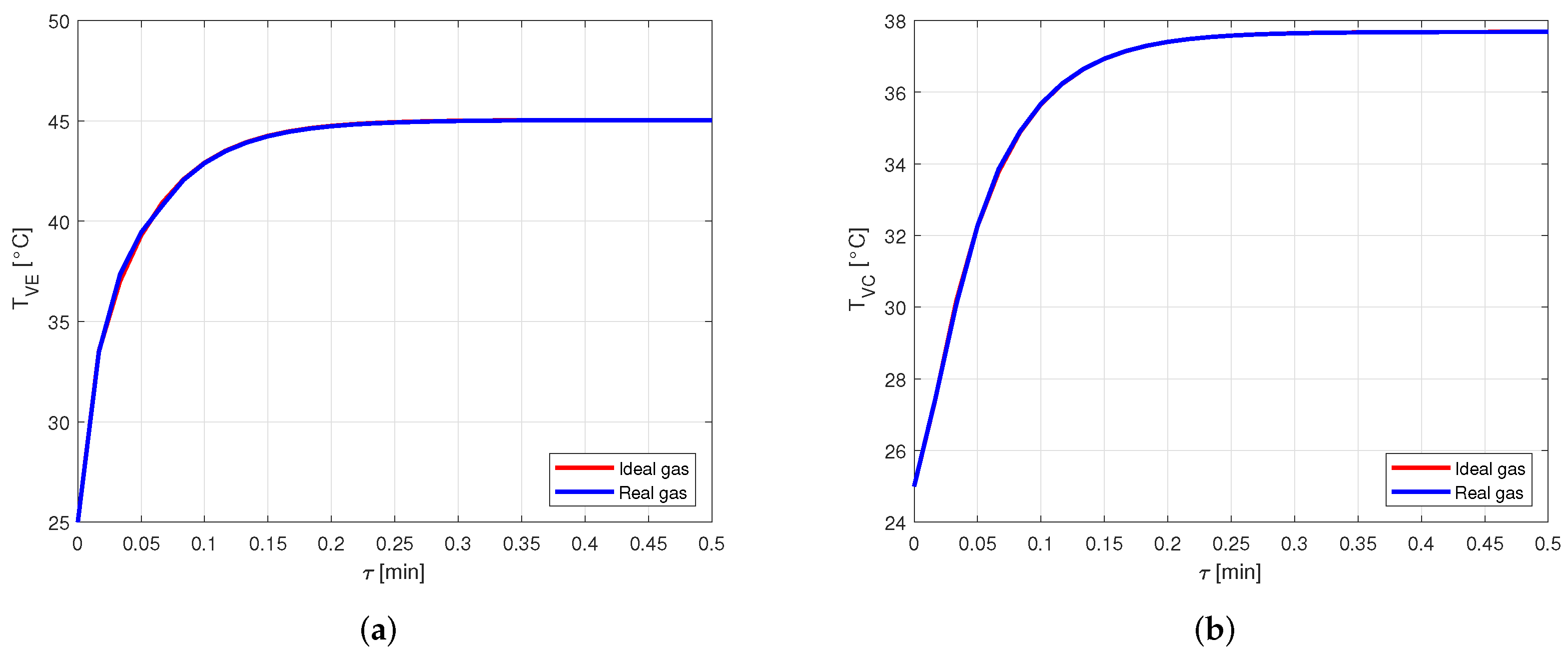
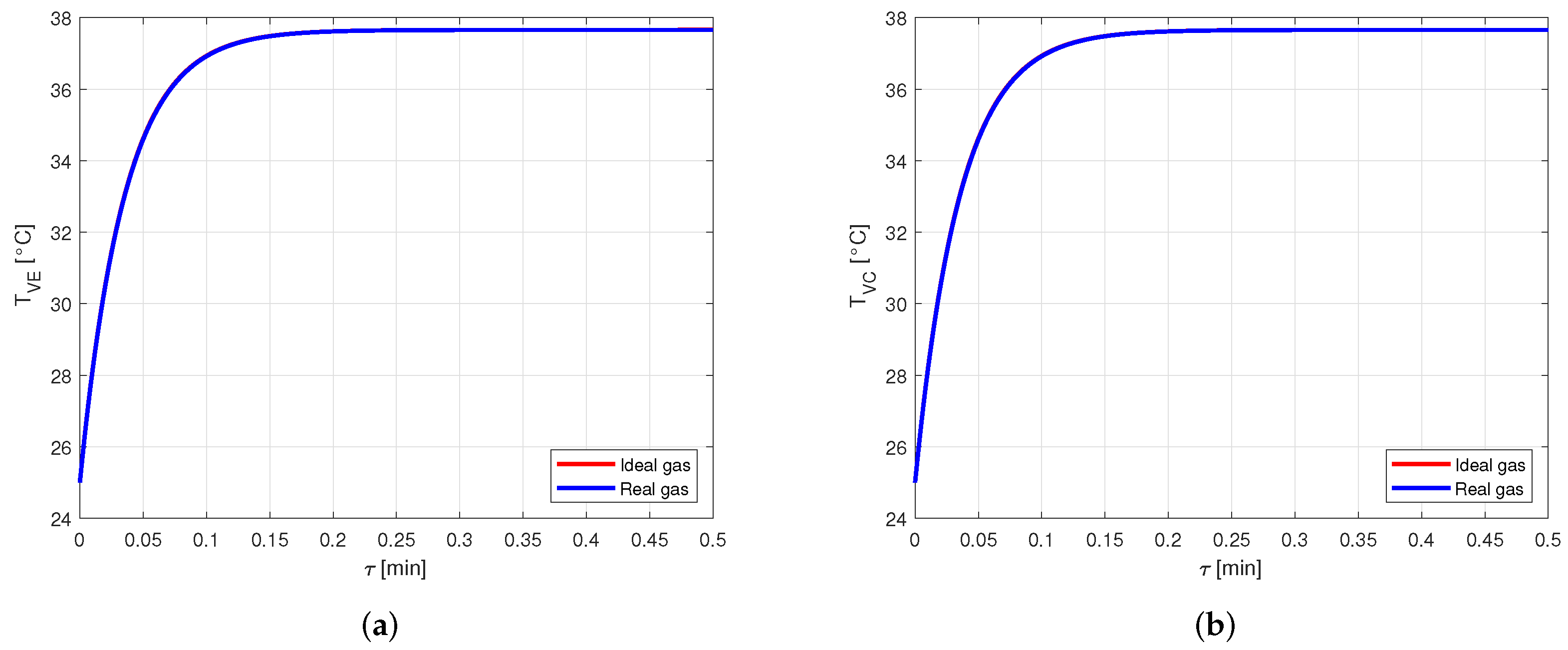
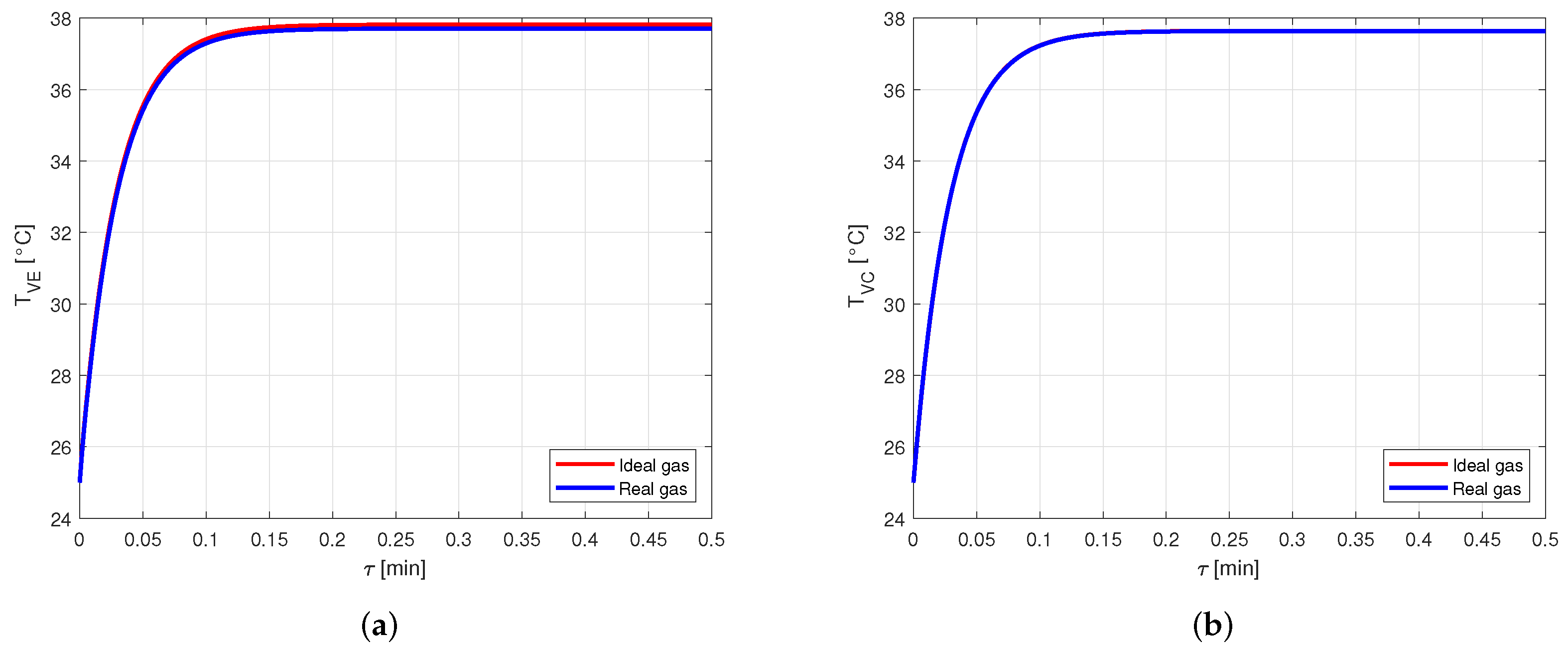
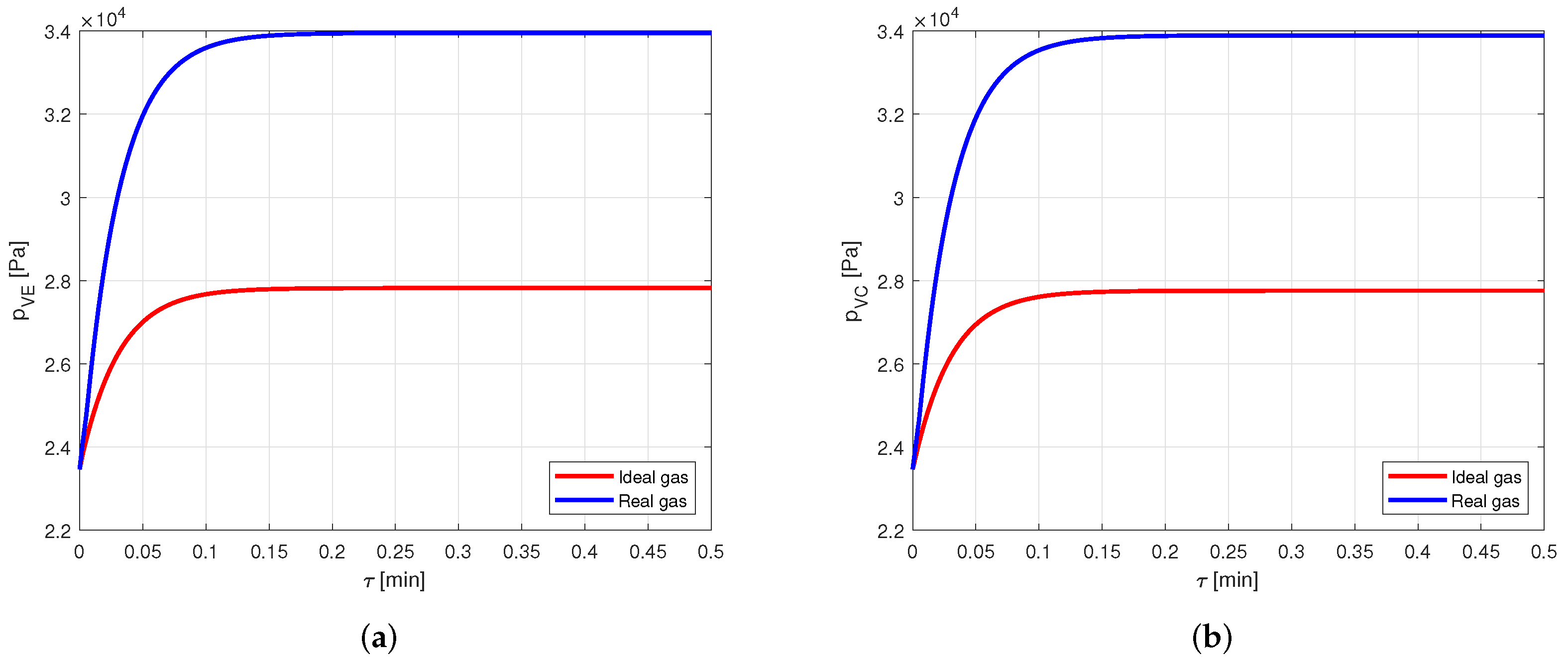
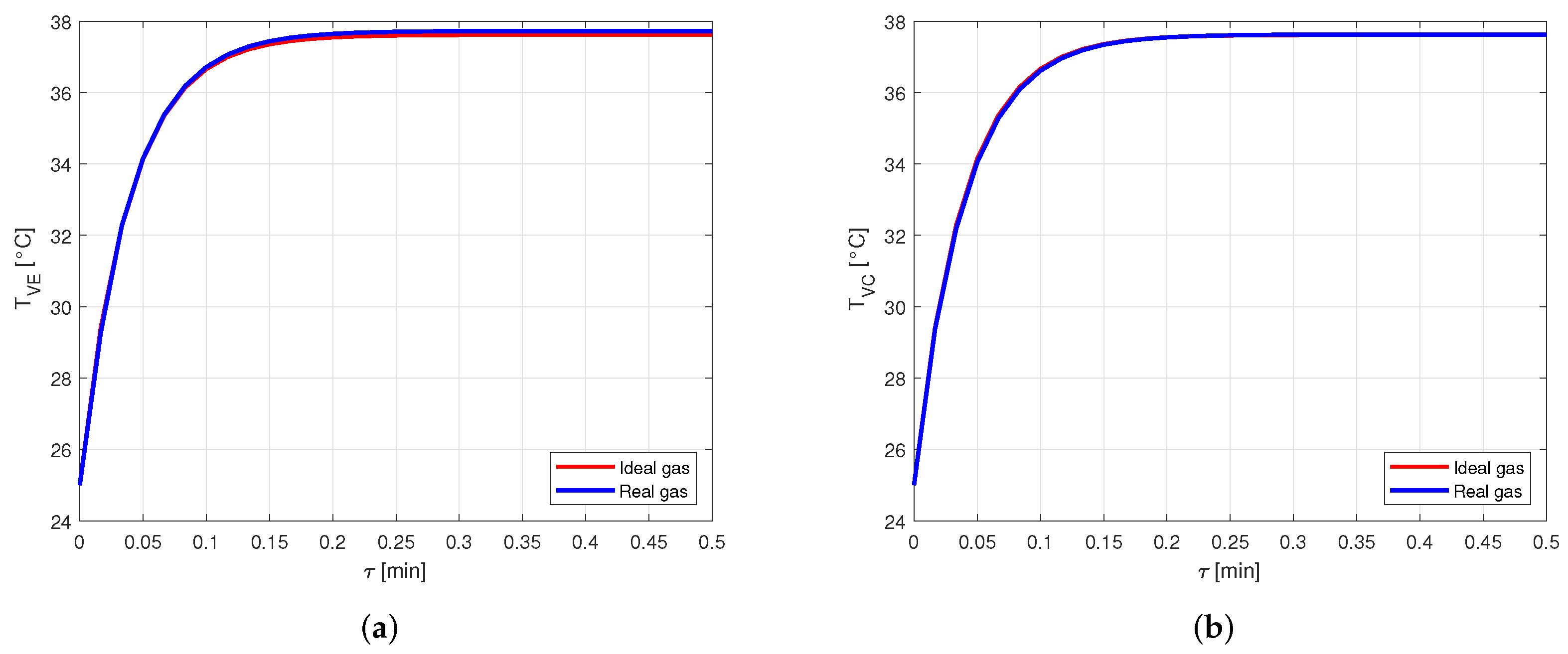
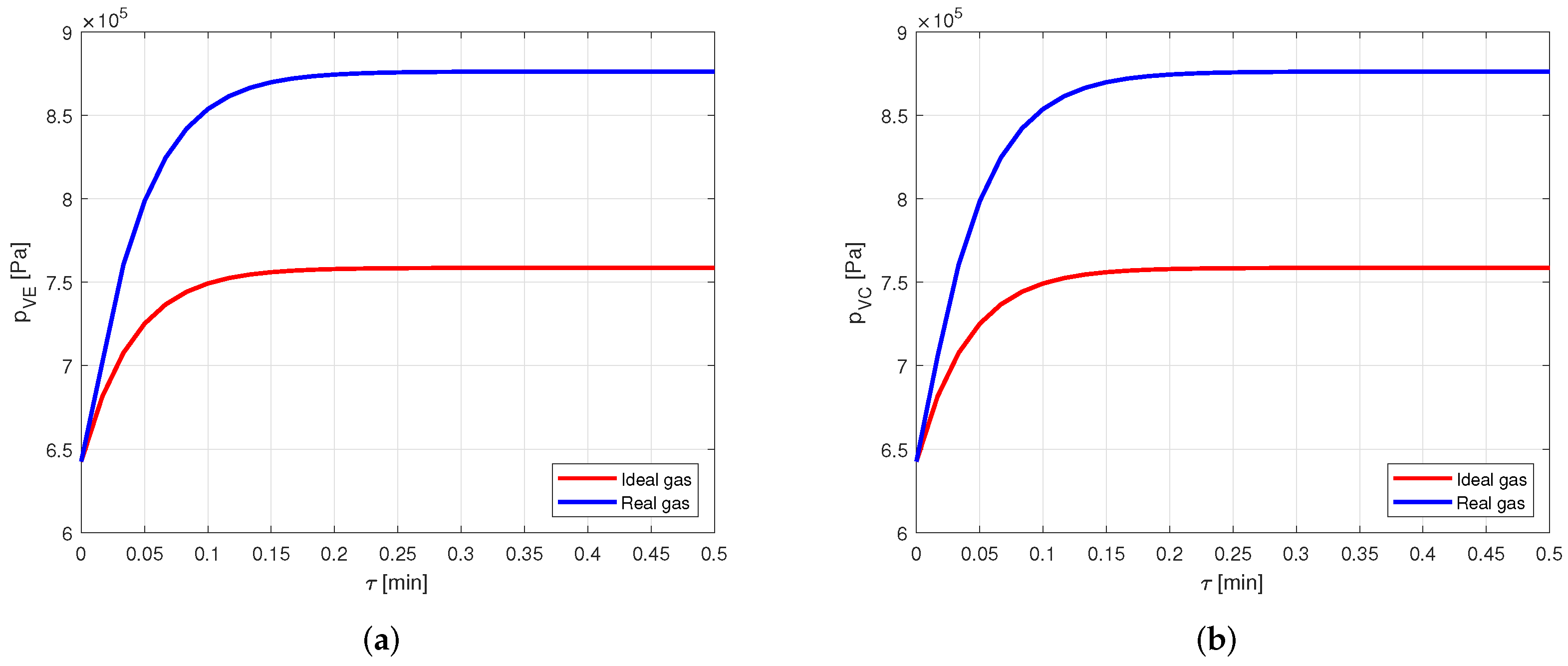
4.2. Comparison between Ideal and Real Gas
4.2.1. Copper-Water
4.2.2. Aluminum–Ammonia
4.2.3. Aluminum–Acetone
4.2.4. Copper–HFC134a Refrigerant
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Symbols: | |
| A | Area [m2] |
| diagonal matrix of the coefficients multiplying the | |
| derivatives of the solid network unknowns [WsK−1] | |
| a | Speed of sound [ms−1] |
| symmetric matrix of the coefficients multiplying the | |
| solid network unknowns [KW−1] | |
| C | Capacitance of a thermal equivalent condenser [WsK−1] |
| vector of the constant terms | |
| c | Specific heat [J kg−1 K−1] |
| Specific heat at constant pressure [J kg−1 K−1] | |
| Hydraulic diameter | |
| f | Body force per unit volume [Nm−3] |
| g | Gravity acceleration [ms−2] |
| h | Convective coefficient [Wm−2 K−1] |
| Latent heat of vaporization [J kg−1] | |
| K | Wick permeability [m2] |
| Isobaric expansion coefficient [K−1] | |
| identity matrix | |
| L | Length |
| l | Variable of integration |
| Mass flow rate [kg s−1] | |
| N | Total number of vapor mass flow rates crossing the vapor |
| tank boundaries | |
| n | Current time step |
| P | Perimeter |
| p | Pressure |
| Heat power | |
| R | Resistance of a thermal equivalent resistor [WK−1] |
| r | Radius |
| Reynolds number | |
| s | Specific entropy [J kg−1 K−1] |
| T | Temperature |
| t | Thickness |
| u | Vapor velocity [ms−1] |
| V | Volume [m3] |
| w | Weight/Width |
| x | One-dimensional coordinate |
| vector of the temperatures of the solid network | |
| Heat pipe orientation angle | |
| Wick porosity | |
| Contact angle | |
| Thermal conductivity [Wm−1 K−1] | |
| Dynamic viscosity | |
| Density [kgm−3] | |
| Surface tension [Nm−1] | |
| Time | |
| Subscripts: | |
| 0 | Initial |
| 1 | Node 1 |
| 2 | Node 2 |
| A | Adiabatic zone/Axial |
| C | Condenser zone/Condensing/Circular |
| c | Capillary |
| E | Evaporator zone/Evaporating |
| Effective | |
| External wall | |
| Equivalent | |
| External wick | |
| F | Referred to a thermal exchange by convection |
| i | Summation index |
| Entering the system | |
| Internal wall | |
| Internal wick | |
| L | Liquid/Linear |
| M | Mixed |
| m | Mean/Molar |
| n | Referred to a generic node |
| Exiting the system | |
| P | Solid wall |
| p | Pore/Solid wall |
| R | Radial |
| r | Reduced |
| Saturation | |
| Total | |
| V | Vapor zone/Vapor |
| W | Wick |
| w | Wick |
| x | One dimensional coordinate |
Appendix A. Details about the Numerical Implementation
- is the diagonal matrix which contains the coefficients that multiplies the derivatives of the unknowns, namely the capacitances;
- is the symmetric matrix which contains the coefficients that multiplies the unknowns, namely the inverse of the solid resistances;
- is the vector of the constant terms, namely the one representing the heat transfer rates entering and exiting the solid network.
References
- Shukla, K. Heat pipe for aerospace applications—An overview. J. Electron. Cool. Therm. Control 2015, 5, 1. [Google Scholar] [CrossRef] [Green Version]
- Jouhara, H.; Chauhan, A.; Nannou, T.; Almahmoud, S.; Delpech, B.; Wrobel, L.C. Heat pipe based systems-Advances and applications. Energy 2017, 128, 729–754. [Google Scholar] [CrossRef]
- Elnaggar, M.H.; Edwan, E. Heat pipes for computer cooling applications. In Electronics Cooling; InTech: Rijeka, Croatia, 2016; p. 51. [Google Scholar]
- Velardo, J.; Singh, R.; Date, A.; Date, A. An investigation into the effective thermal conductivity of vapour chamber heat spreaders. Energy Procedia 2017, 110, 256–261. [Google Scholar] [CrossRef]
- Cullimore, B.; Baumann, J. Steady State and Transient Loop Heat Pipe Modeling. In Proceedings of the International Conference On Environmental Systems, Toulouse, France, 10 July 2000. [Google Scholar]
- Suman, B.; De, S.; DasGupta, S. Transient modeling of micro-grooved heat pipe. Int. J. Heat Mass Tran. 2005, 48, 1633–1646. [Google Scholar] [CrossRef]
- Ferrandi, C.; Marengo, M.; Zinna, S. Influence of Tube Size on Thermal Behaviour of Sintered Heat Pipe. In Proceedings of the 2nd European Conference on Microfluidics, Toulouse, France, 8–10 December 2010. [Google Scholar]
- Ferrandi, C.; Iorizzo, F.; Mameli, M.; Zinna, S.; Marengo, M. Lumped parameter model of sintered heat pipe: Transient numerical analysis and validation. Appl. Therm. Eng. 2013, 50, 1280–1290. [Google Scholar] [CrossRef]
- Bernagozzi, M.; Charmer, S.; Georgoulas, A.; Malavasi, I.; Michè, N.; Marengo, M. Lumped parameter network simulation of a Loop Heat Pipe for energy management systems in full electric vehicles. Appl. Therm. Eng. 2018, 141, 617–629. [Google Scholar] [CrossRef]
- Wang, X.; Li, B.; Yan, Y.; Gao, N.; Chen, G. Predicting of thermal resistances of closed vertical meandering pulsating heat pipe using artificial neural network model. Appl. Therm. Eng. 2019, 149, 1134–1141. [Google Scholar] [CrossRef]
- Guichet, V.; Khordehgah, N.; Jouhara, H. Experimental investigation and analytical prediction of a multi-channel flat heat pipe thermal performance. Int. J. Thermofluids 2020, 5, 100038. [Google Scholar] [CrossRef]
- Zimmermann, S.; Dreiling, R.; Nguyen-Xuan, T.; Pfitzner, M. An advanced conduction based heat pipe model accounting for vapor pressure drop. Int. J. Heat Mass Tran. 2021, 175, 121014. [Google Scholar] [CrossRef]
- Guilizzoni, M. La Fisica Tecnica e il Rasoio di Ockham; Maggioli Editore: Santarcangelo di Romagna (RM), Italy, 2017. [Google Scholar]
- Payne, L.; Rodrigues, J.; Straughan, B. Effect of anisotropic permeability on Darcy’s law. Math. Method Appl. Sci. 2001, 24, 427–438. [Google Scholar] [CrossRef]
- Byon, C.; Kim, S.J. Capillary performance of bi-porous sintered metal wicks. Int. J. Heat Mass Tran. 2012, 55, 4096–4103. [Google Scholar] [CrossRef]
- Pal, R. Teach second law of thermodynamics via analysis of flow through packed beds and consolidated porous media. Fluids 2019, 4, 116. [Google Scholar] [CrossRef] [Green Version]
- de Laplace, P.S. Traité De Mécanique Céleste: Supplément au Dixième Livre du Traité de Méchanique Céleste sur l’action Capillaire; Duprat: Paris, France, 1808; Volume 6. [Google Scholar]
- National Institute of Standards and Technology. 2021. Available online: https://www.nist.gov (accessed on 13 December 2021).
- Incropera, F.P.; Lavine, A.S.; Bergman, T.L.; DeWitt, D.P. Fundamentals of Heat and Mass Transfer; Wiley: Hoboken, NJ, USA, 2007. [Google Scholar]
- Mitropoulos, A.C. The Kelvin equation. J. Colloid Interface Sci. 2008, 317, 643–648. [Google Scholar] [CrossRef] [PubMed]
- Comsol—Software for Multiphysics Simulation. 2021. Available online: https://www.comsol.com/ (accessed on 26 December 2021).
- Nezbeda, I. Simulations of vapor–liquid equilibria: Routine versus thoroughness. J. Chem. Eng. Data 2016, 61, 3964–3969. [Google Scholar] [CrossRef]






Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Caruana, R.; Gallazzi, L.; Iazurlo, R.; Marcovati, M.; Guilizzoni, M. A Multi-Node Lumped Parameter Model Including Gravity and Real Gas Effects for Steady and Transient Analysis of Heat Pipes. Fluids 2022, 7, 109. https://doi.org/10.3390/fluids7030109
Caruana R, Gallazzi L, Iazurlo R, Marcovati M, Guilizzoni M. A Multi-Node Lumped Parameter Model Including Gravity and Real Gas Effects for Steady and Transient Analysis of Heat Pipes. Fluids. 2022; 7(3):109. https://doi.org/10.3390/fluids7030109
Chicago/Turabian StyleCaruana, Roberta, Luciano Gallazzi, Romano Iazurlo, Maurizio Marcovati, and Manfredo Guilizzoni. 2022. "A Multi-Node Lumped Parameter Model Including Gravity and Real Gas Effects for Steady and Transient Analysis of Heat Pipes" Fluids 7, no. 3: 109. https://doi.org/10.3390/fluids7030109
APA StyleCaruana, R., Gallazzi, L., Iazurlo, R., Marcovati, M., & Guilizzoni, M. (2022). A Multi-Node Lumped Parameter Model Including Gravity and Real Gas Effects for Steady and Transient Analysis of Heat Pipes. Fluids, 7(3), 109. https://doi.org/10.3390/fluids7030109