Convective Velocity Perturbations and Excess Gain in Flame Response as a Result of Flame-Flow Feedback
Abstract
1. Introduction
2. Materials and Methods
2.1. Flow Dynamics
2.1.1. Governing Equations
2.1.2. Conformal Mapping-Based Modeling Approach
2.1.3. Flow-Field Singularities
2.1.4. Kutta Condition
2.2. Flame Dynamics
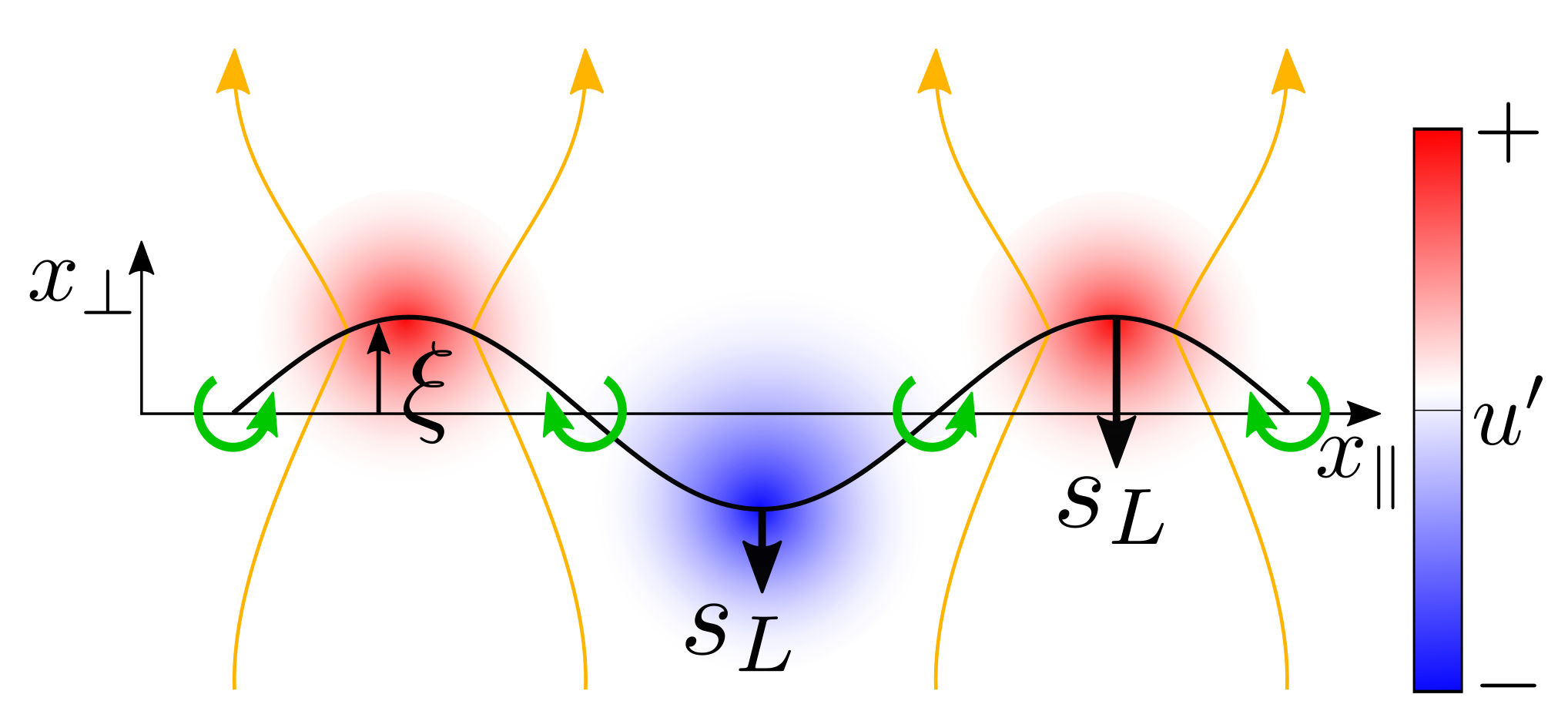
2.2.1. Linearized G-Equation for the Flame Front
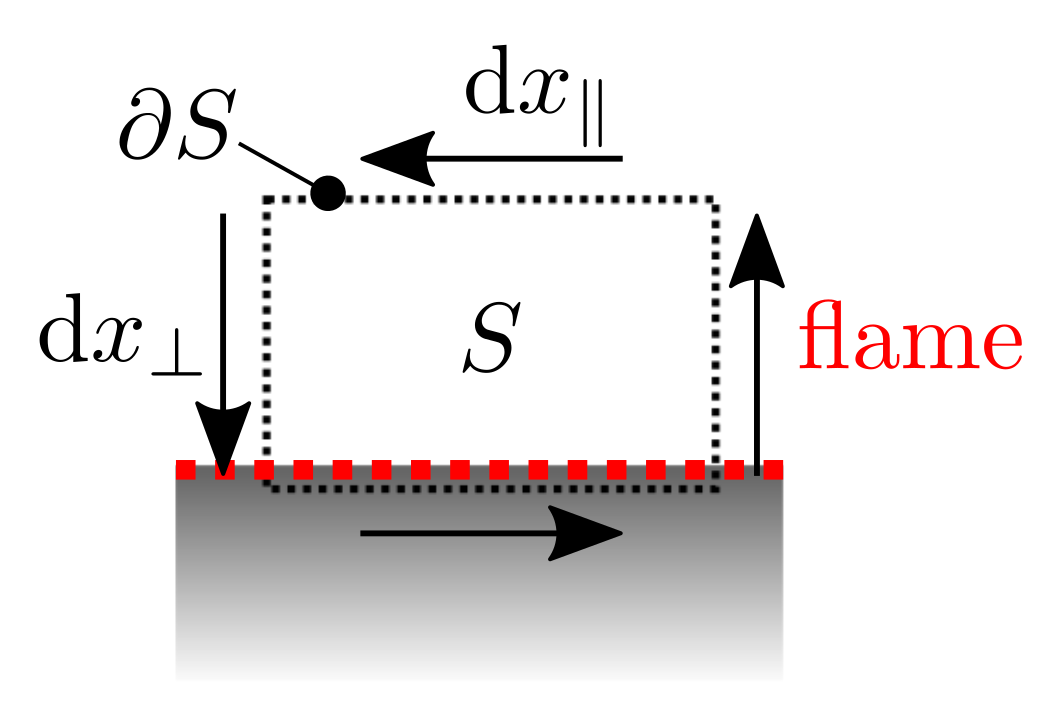
2.2.2. Jump Conditions across a Flame Sheet
2.2.3. Modeling of Flame Generated Vorticity
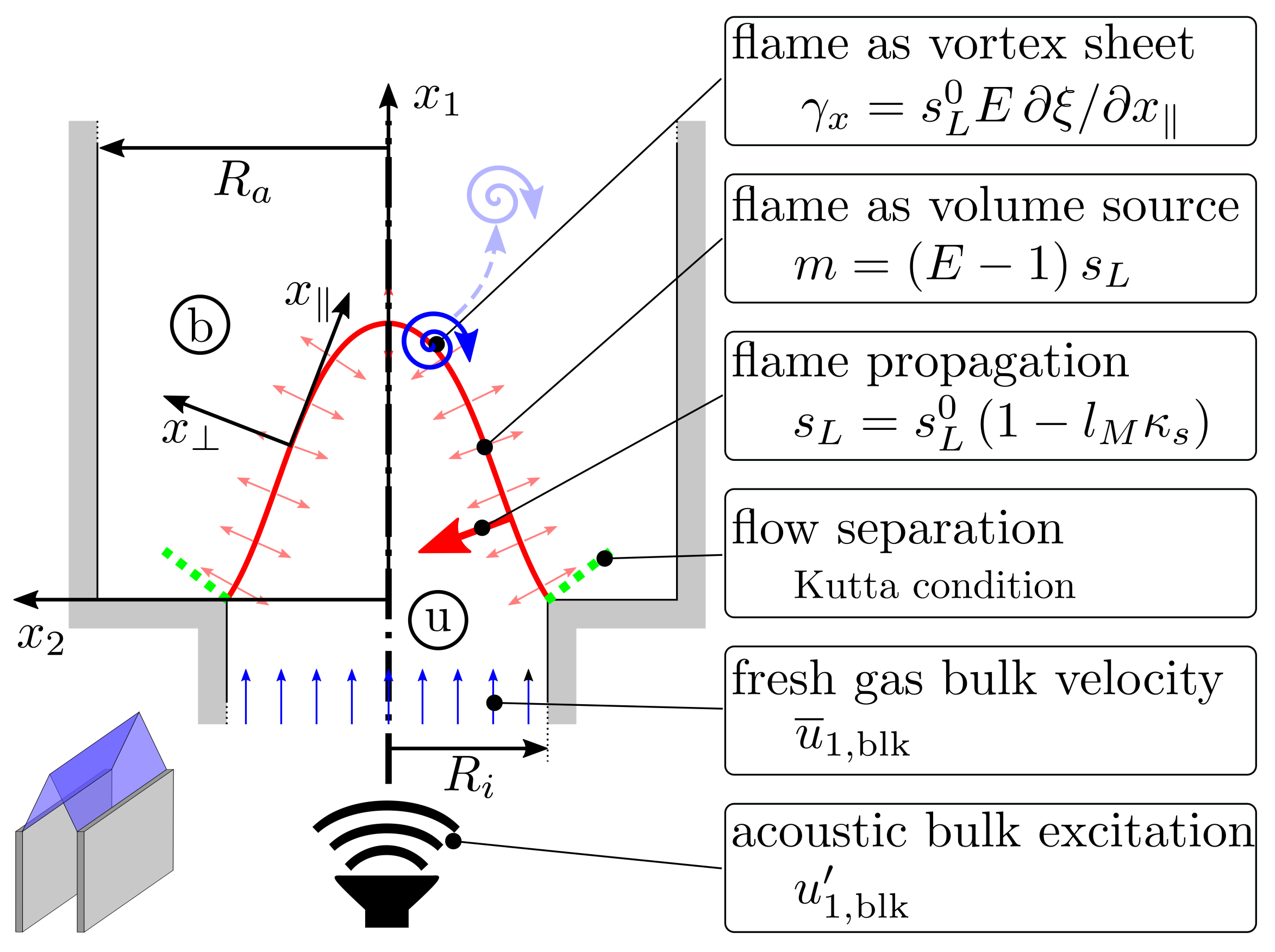
2.3. Test Case Setup
 ). An estimate of the shear layer position (
). An estimate of the shear layer position ( ) as well as the steady state flame front of length , as predicted by the 1D linearized G-equation approach, (
) as well as the steady state flame front of length , as predicted by the 1D linearized G-equation approach, ( ) are indicated. Note, that other than for conical configurations [13], the confinement ratio does not impact the flame height of 2D Slit flames [54].
) are indicated. Note, that other than for conical configurations [13], the confinement ratio does not impact the flame height of 2D Slit flames [54].3. Results—Analysis of Flow/Flame Interactions
3.1. Unidirectional Coupling
3.1.1. Harmonic Forcing
 ).
). ) and the resulting flame front displacements (
) and the resulting flame front displacements ( ) generated by the velocity perturbations are also shown. It is evident that the flame front displacements provoked by the irrotational velocity are significantly larger than those generated by the vortical contribution. In other words, vorticity shed at the backward facing step contributes in the present case only in a negligible manner to the response of the flame. Furthermore, it is seen that irrotational velocity perturbations are large near the flame base, and weaken significantly towards the tip of the flame.
) generated by the velocity perturbations are also shown. It is evident that the flame front displacements provoked by the irrotational velocity are significantly larger than those generated by the vortical contribution. In other words, vorticity shed at the backward facing step contributes in the present case only in a negligible manner to the response of the flame. Furthermore, it is seen that irrotational velocity perturbations are large near the flame base, and weaken significantly towards the tip of the flame.3.1.2. Impulse Forcing
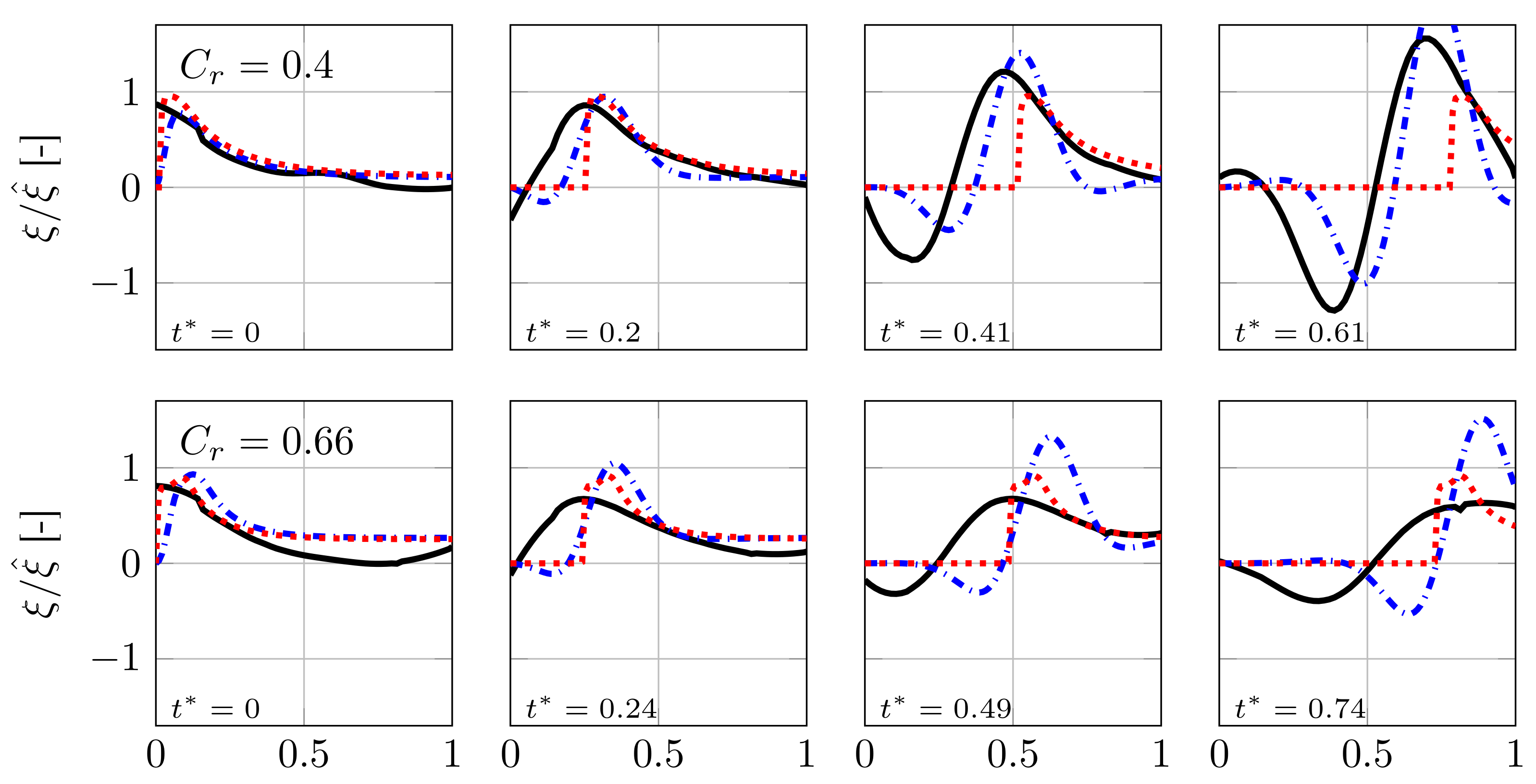
 ) and the CFD results (
) and the CFD results ( ) is indeed observed for the initial time .
) is indeed observed for the initial time . ) occurs at the right time, however its magnitude is under-predicted. More importantly, subsequent negative and positive strong excursions of the IR shown by the CFD results (
) occurs at the right time, however its magnitude is under-predicted. More importantly, subsequent negative and positive strong excursions of the IR shown by the CFD results ( ) are completely absent in the UD model. One must expect [10,11] that these discrepancies in IR have significant consequences also for the FR. Indeed, while the CFD model develops pronounced “excess gain” with a magnitude of the the frequency response (FR) above unity for frequencies , the FR predicted by the UD model (
) are completely absent in the UD model. One must expect [10,11] that these discrepancies in IR have significant consequences also for the FR. Indeed, while the CFD model develops pronounced “excess gain” with a magnitude of the the frequency response (FR) above unity for frequencies , the FR predicted by the UD model ( ) shows a low pass behavior without excess gain. An almost linearly decaying phase of the FR is captured by all models.
) shows a low pass behavior without excess gain. An almost linearly decaying phase of the FR is captured by all models.3.2. Bidirectional Coupling
 ) with CFD results (
) with CFD results ( ). The authors of the present study attribute these discrepancies to flame-flow feedback, which is not taken into account by the unidirectional coupling of the UD model. Furthermore, such a feedback may also be responsible for the CVPs, which would run contrary to established G-equation models that impose CVPs of presumed functional form. Support for these hypotheses will be presented in this subsection.
). The authors of the present study attribute these discrepancies to flame-flow feedback, which is not taken into account by the unidirectional coupling of the UD model. Furthermore, such a feedback may also be responsible for the CVPs, which would run contrary to established G-equation models that impose CVPs of presumed functional form. Support for these hypotheses will be presented in this subsection. ) develops negative and positive secondary excursions, which grow in amplitude while they are advected towards the flame tip. This is in good qualitative agreement with the CFD results (
) develops negative and positive secondary excursions, which grow in amplitude while they are advected towards the flame tip. This is in good qualitative agreement with the CFD results ( ). However, the negative and positive excursions are found slightly too far downstream, and the amplitudes of the excursions are over-predicted by the BD model. Overall, BD model predictions for match the respective CFD results better than for . The damping, presumably imposed by the presence of a rather narrow confinement, seems to be under-predicted for the setup.
). However, the negative and positive excursions are found slightly too far downstream, and the amplitudes of the excursions are over-predicted by the BD model. Overall, BD model predictions for match the respective CFD results better than for . The damping, presumably imposed by the presence of a rather narrow confinement, seems to be under-predicted for the setup. ) and BD (
) and BD ( ) predictions agree well, only small deviations in phase/gain and the impulse response are observed. This supports the hypothesis that flame generated vorticity represents an important mechanism of flame-flow feedback that makes an important contribution to the response of a flame to acoustic perturbations. For , on the other hand, the IR predicted by the BD model deviates significantly from the CFD data right from the beginning, see Figure 11b. Accordingly, the corresponding FTF gain curves are qualitatively different. This suggests that the effects of confinement are not properly captured by the comparatively simplistic implementation of flame-flow feedback, which does not include all possibly important effects [29].
) predictions agree well, only small deviations in phase/gain and the impulse response are observed. This supports the hypothesis that flame generated vorticity represents an important mechanism of flame-flow feedback that makes an important contribution to the response of a flame to acoustic perturbations. For , on the other hand, the IR predicted by the BD model deviates significantly from the CFD data right from the beginning, see Figure 11b. Accordingly, the corresponding FTF gain curves are qualitatively different. This suggests that the effects of confinement are not properly captured by the comparatively simplistic implementation of flame-flow feedback, which does not include all possibly important effects [29].4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| CFD | Computational Fluid Dynamics |
| CVP | Convective Velocity Perturbation |
| BD | BiDirectional coupling |
| FTF | Flame Transfer Function |
| FR | Frequency Response |
| IR | Impulse Response |
| UD | UniDirectional coupling |
References
- Lieuwen, T.; Yang, V. (Eds.) Combustion Instabilities in Gas Turbine Engines: Operational Experience, Fundamental Mechanisms and Modeling; Number v. 210 in Progress in Astronautics and Aeronautics; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2005. [Google Scholar]
- Candel, S. Combustion Dynamics and Control: Progress and Challenges. Proc. Combust. Inst. 2002, 29, 1–28. [Google Scholar] [CrossRef]
- Culick, F.E.C. Unsteady Motions in Combustion Chambers for Propulsion Systems; Number AC/323(AVT-039)TP/103 in RTO AGARDograph AG-AVT-039, AGARD/NATO; NATO Research and Technology Organization: Neuilly-Sur-Seine, France, 2006. [Google Scholar]
- Poinsot, T. Prediction and Control of Combustion Instabilities in Real Engines. Proc. Combust. Inst. 2017, 36, 1–28. [Google Scholar] [CrossRef]
- Renard, P.H.; Thevenin, D.; Rolon, J.C.; Candel, S. Dynamics of Flame/Vortex Interactions. Prog. Energy Combust. Sci. 2000, 26, 225–282. [Google Scholar] [CrossRef]
- Lieuwen, T.C. Unsteady Combustor Physics; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar]
- Merk, H. Analysis of Heat-Driven Oscillations of Gas Flows. I. General Considerations. Appl. Sci. Res. Sect. A 1957, 6, 317–336. [Google Scholar] [CrossRef]
- Fleifil, A.; Annaswamy, A.M.; Ghoneim, Z.A.; Ghoniem, A.F. Response of a Laminar Premixed Flame to Flow Oscillations: A Kinematic Model and Thermoacoustic Instability Results. Combust. Flame 1996, 106, 487–510. [Google Scholar] [CrossRef]
- Schuller, T.; Durox, D.; Candel, S. A Unified Model for the Prediction of Laminar Flame Transfer Functions: Comparisons Between Conical and V-Flame Dynamics. Combust. Flame 2003, 134, 21–34. [Google Scholar] [CrossRef]
- Blumenthal, R.S.; Subramanian, P.; Sujith, R.; Polifke, W. Novel Perspectives on the Dynamics of Premixed Flames. Combust. Flame 2013, 160, 1215–1224. [Google Scholar] [CrossRef]
- Polifke, W. Modeling and Analysis of Premixed Flame Dynamics by Means of Distributed Time Delays. Prog. Energy Combust. Sci. 2020, 79, 100845. [Google Scholar] [CrossRef]
- Preetham; Santosh, H.; Lieuwen, T. Dynamics of Laminar Premixed Flames Forced by Harmonic Velocity Disturbances. J. Propuls. Power 2008, 24, 1390–1402. [Google Scholar] [CrossRef]
- Cuquel, A.; Durox, D.; Schuller, T. Scaling the Flame Transfer Function of Confined Premixed Conical Flames. Proc. Combust. Inst. 2013, 34, 1007–1014. [Google Scholar] [CrossRef]
- Baillot, F.; Durox, D.; Prud’homme, R. Experimental and Theoretical Study of a Premixed Vibrating Flame. Combust. Flame 1992, 88, 149–168. [Google Scholar] [CrossRef]
- Disselhorst, J.H.M.; Wijngaarden, L.V. Flow in the Exit of Open Pipes During Acoustic Resonance. J. Fluid Mech. 1980, 99, 293–319. [Google Scholar] [CrossRef][Green Version]
- Peters, M.C.A.M.; Hirschberg, A. Acoustically Induced Periodic Vortex Shedding At Sharp Edged Open Channel Ends: Simple Vortex Models. J. Sound Vib. 1993, 161, 281–299. [Google Scholar] [CrossRef]
- Hirsch, C.; Fanaca, D.; Reddy, P.; Polifke, W.; Sattelmayer, T. Influence of the Swirler Design on the Flame Transfer Function of Premixed Flames. In ASME Turbo Expo 2005: Power for Land, Sea, and Air; GT2005-68195; ASME: Reno, NV, USA, 2005; pp. 151–160. [Google Scholar] [CrossRef]
- Schlimpert, S.; Hemchandra, S.; Meinke, M.; Schröder, W. Hydrodynamic Instability and Shear Layer Effect on the Response of an Acoustically Excited Laminar Premixed Flame. Combust. Flame 2015, 162, 345–367. [Google Scholar] [CrossRef]
- Steinbacher, T.; Albayrak, A.; Ghani, A.; Polifke, W. Response of Premixed Flames to Irrotational and Vortical Velocity Fields Generated by Acoustic Perturbations. Proc. Combust. Inst. 2019, 37, 5367–5375. [Google Scholar] [CrossRef]
- Pindera, M.Z.; Talbot, L. Some Fluid Dynamic Considerations in the Modeling of Flames. Combust. Flame 1988, 73, 111–125. [Google Scholar] [CrossRef]
- Rhee, C.W.; Talbot, L.; Sethian, J.A. Dynamical Behaviour of a Premixed Turbulent Open V-Flame. J. Fluid Mech. 1995, 300, 87–115. [Google Scholar] [CrossRef][Green Version]
- Birbaud, A.; Durox, D.; Candel, S. Upstream Flow Dynamics of a Laminar Premixed Conical Flame Submitted to Acoustic Modulations. Combust. Flame 2006, 146, 541–552. [Google Scholar] [CrossRef]
- Kornilov, V.N.; Schreel, K.R.A.M.; de Goey, L.P.H. Experimental Assessment of the Acoustic Response of Laminar Premixed Bunsen Flames. Proc. Combust. Inst. 2007, 31, 1239–1246. [Google Scholar] [CrossRef]
- Blanchard, M.; Schuller, T.; Sipp, D.; Schmid, P.J. Response Analysis of a Laminar Premixed M-Flame to Flow Perturbations Using a Linearized Compressible Navier-Stokes Solver. Phys. Fluids 2015, 27, 043602. [Google Scholar] [CrossRef]
- Mehta, P.G.; Soteriou, M.C. Combustion Heat Release Effects on the Dynamics of Bluff Body Stabilized Premixed Reacting Flows. In Proceedings of the 41st Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 6–9 January 2003. [Google Scholar]
- Erickson, R.; Soteriou, M.; Mehta, P. The Influence of Temperature Ratio on the Dynamics of Bluff Body Stabilized Flames. In Proceedings of the 44th AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 9–12 January 2006. [Google Scholar] [CrossRef]
- Chu, B.T.; Kovasznay, L.S.G. Non-Linear Interactions in a Viscous Heat-Conducting Compressible Gas. J. Fluid Mech. 1957, 3, 495–514. [Google Scholar] [CrossRef]
- Boyer, L.; Quinard, J. On the Dynamics of Anchored Flames. Combust. Flame 1990, 82, 51–65. [Google Scholar] [CrossRef]
- Steinbacher, T. Analysis and Low-Order Modeling of Interactions between Acoustics, Hydrodynamics and Premixed Flames. Ph.D. Thesis, TU München, München, Germany, 2019. [Google Scholar]
- Pindera, M.Z.; Talbot, L. Flame Induced Vorticity: Effects of Stretch. Symp. Int. Combust. 1988, 21, 1357–1366. [Google Scholar] [CrossRef][Green Version]
- Landau, L. On the Theory of Slow Combusiton. Acta Physicochim. 1944, 19, 403–411. [Google Scholar] [CrossRef]
- Sivashinsky, G.; Clavin, P. On the Nonlinear Theory of Hydrodynamic Instability in Flames. J. Phys. 1987, 48, 193–198. [Google Scholar] [CrossRef]
- Frankel, M.L. An Equation of Surface Dynamics Modeling Flame Fronts as Density Discontinuities in Potential Flows. Phys. Fluids A Fluid Dyn. 1990, 2, 1879–1883. [Google Scholar] [CrossRef]
- Zimmermann, A. Modeling the Influence of Gas Expansion on the Linear Response of Laminar Premixed Flames. Bachelor’s Thesis, Technische Universität München, München, Germany, 2018. [Google Scholar]
- Matalon, M.; Cui, C.; Bechtold, J.K. Hydrodynamic Theory of Premixed Flames: Effects of Stoichiometry, Variable Transport Coefficients and Arbitrary Reaction Orders. J. Fluid Mech. 2003, 487, 179–210. [Google Scholar] [CrossRef]
- Brown, J.W.; Churchill, R.V. Complex Variables and Applications, 8th ed.; Brown and Churchill Series; McGraw-Hill Higher Education: Boston, MA, USA, 2009. [Google Scholar]
- Batchelor, G. An Introduction to Fluid Mechanics; Cambridge University Press: Cambridge, UK, 1967. [Google Scholar]
- Pullin, D.I. The Large-Scale Structure of Unsteady Self-Similar Rolled-up Vortex Sheets. J. Fluid Mech. 1978, 88, 401–430. [Google Scholar] [CrossRef]
- Hoeijmakers, H.W.M. Computational Aerodynamics of Ordered Vortex Flows. Ph.D. Thesis, TU Delft, Delft, The Netherlands, 1989. [Google Scholar]
- Ducruix, S.; Durox, D.; Candel, S. Theoretical and Experimental Determinations of the Transfer Function of a Laminar Premixed Flame. Proc. Combust. Inst. 2000, 28, 765–773. [Google Scholar] [CrossRef]
- Wang, H.Y.; Law, C.K.; Lieuwen, T. Linear Response of Stretch-Affected Premixed Flames to Flow Oscillations. Combust. Flame 2009, 156, 889–895. [Google Scholar] [CrossRef]
- Markstein, G.H. Experimental and Theoretical Studies of Flame-Front Stability. J. Aeronaut. Sci. 1951, 18, 199–209. [Google Scholar] [CrossRef]
- Lieuwen, T. Theoretical Investigation of Unsteady Flow Interactions with a Premixed Planar Flame. J. Fluid Mech. 2001, 435, 289–303. [Google Scholar] [CrossRef]
- Class, A.G.; Matkowsky, B.J.; Klimenko, a.Y. A Unified Model of Flames as Gasdynamic Discontinuities. J. Fluid Mech. 2003, 491, 11–49. [Google Scholar] [CrossRef]
- Hayes, W.D. The Vorticity Jump Across a Gasdynamic Discontinuity. J. Fluid Mech. 1957, 2, 595–600. [Google Scholar] [CrossRef]
- Ashurst, W.M.T.; Sivashinsky, G.I.; Yakhot, V. Flame Front Propagation in Nonsteady Hydrodynamic Fields. Combust. Sci. Technol. 1988, 62, 273–284. [Google Scholar] [CrossRef]
- Matalon, M. Flame Dynamics. Proc. Combust. Inst. 2009, 32, 57–82. [Google Scholar] [CrossRef]
- The OpenFOAM Foundation. OpenFOAM 2.3.1. 2017. Available online: https://openfoam.org/ (accessed on 11 May 2017).
- Bibrzycki, J.; Poinsot, T.; Zajdel, A. Investigation of Laminar Flame Speed of CH4/N2/O2 and CH4/CO2/O2 Mixtures Using Reduced Chemical Kinetic Mechanisms. Arch. Combust. 2010, 30, 287–296. [Google Scholar]
- Duchaine, F.; Boudy, F.; Durox, D.; Poinsot, T. Sensitivity Analysis of Transfer Functions of Laminar Flames. Combust. Flame 2011, 158, 2384–2394. [Google Scholar] [CrossRef]
- Jaensch, S.; Merk, M.; Gopalakrishnan, E.; Bomberg, S.; Emmert, T.; Sujith, R.; Polifke, W. Hybrid CFD/Low-Order Modeling of Nonlinear Thermoacoustic Oscillations. Proc. Combust. Inst. 2017, 36, 3827–3834. [Google Scholar] [CrossRef]
- Steinbacher, T.; Meindl, M.; Polifke, W. Modeling the Generation of Temperature Inhomogeneities by a Premixed Flame. Int. J. Spray Combust. Dyn. 2018, 10, 111–130. [Google Scholar] [CrossRef]
- Steinbacher, T.; Albayrak, A.; Ghani, A.; Polifke, W. Consequences of Flame Geometry for the Acoustic Response of Premixed Flames. Combust. Flame 2019, 199, 411–428. [Google Scholar] [CrossRef]
- Mehta, P.; Soteriou, M.; Banaszuk, A. Impact of Exothermicity on Steady and Linearized Response of a Premixed Ducted Flame. Combust. Flame 2005, 141, 392–405. [Google Scholar] [CrossRef]
- Clements, R.R. An Inviscid Model of Two-Dimensional Vortex Shedding. J. Fluid Mech. 1973, 57, 321–336. [Google Scholar] [CrossRef]
- Hirschberg, A.; Hoeijmakers, H. Comments on the Low Frequency Radiation Impedance of a Duct Exhausting a Hot Gas. J. Acoust. Soc. Am. 2014, 136, EL84–EL89. [Google Scholar] [CrossRef] [PubMed]
- Langtangen, H.P.; Linge, S. 3.1 an Explicit Method for the 1D Diffusion Equation. In Finite Difference Computing with PDEs: A Modern Software Approach; Number 16 in Texts in Computational Science and Engineering; Springer Open: Cham, Switzerland, 2017. [Google Scholar]
- Nelson, P.A.; Halliwell, N.A.; Doak, P.E. Fluid Dynamics of a Flow Excited Resonance, Part II: Flow Acoustic Interaction. J. Sound Vib. 1983, 91, 375–402. [Google Scholar] [CrossRef]
- Darrieus, G. Propagation d’un Front de Flamme. Presented at La Technique Moderne Paris, Paris, France, 6–15 March 1938. Unpublished Work. [Google Scholar]
- Saffman, P.G. Vortex Dynamics; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Goodwin, D.G.; Moffat, H.K.; Speth, R.L. Cantera: An Object-Oriented Software Toolkit for Chemical Kinetics, Thermodynamics, and Transport Processes. 2016. Available online: http://www.cantera.org (accessed on 13 December 2021).
- Searby, G.; Truffaut, J.M.; Joulin, G. Comparison of Experiments and a Nonlinear Model Equation for Spatially Developing Flame Instability. Phys. Fluids 2001, 13, 3270–3276. [Google Scholar] [CrossRef]
- Lee, D.H.; Lieuwen, T.C. Acoustic Near-Field Characteristics of a Conical, Premixed Flame. J. Acoust. Soc. Am. 2003, 113, 167–177. [Google Scholar] [CrossRef]
- Lee, D.; Lieuwen, T. Premixed Flame Kinematics in a Longitudinal Acoustic Field. J. Propuls. Power 2003, 19, 837–846. [Google Scholar] [CrossRef]
- Hemchandra, S. Premixed Flame Response to Equivalence Ratio Fluctuations: Comparison Between Reduced Order Modeling and Detailed Computations. Combust. Flame 2012, 159, 3530–3543. [Google Scholar] [CrossRef]
- Oberleithner, K.; Schimek, S.; Paschereit, C.O. Shear Flow Instabilities in Swirl-Stabilized Combustors and Their Impact on the Amplitude Dependent Flame Response: A Linear Stability Analysis. Combust. Flame 2015, 162, 86–99. [Google Scholar] [CrossRef]
- Oberleithner, K.; Paschereit, C.O. Modeling Flame Describing Functions Based on Hydrodynamic Linear Stability Analysis. In Proceedings of the ASME Turbo Expo 2016: Turbomachinery Technical Conference and Exposition, Seoul, Korea, 13–17 June 2016; p. V04BT04A009. [Google Scholar] [CrossRef]
- Class, A.G.; Matkowsky, B.J.; Klimenko, A.Y. Stability of Planar Flames as Gasdynamic Discontinuities. J. Fluid Mech. 2003, 491, 51–63. [Google Scholar] [CrossRef]
- Howe, M.S. Hydrodynamics and Sound; Cambridge University Press: Cambridge, UK; New York, NY, USA, 2007. [Google Scholar]
- Joulin, G. Nonlinear Hydrodynamic Instability of Expanding Flames: Intrinsic Dynamics. Phys. Rev. E 1994, 50, 2030–2047. [Google Scholar] [CrossRef] [PubMed]
- Denet, B. Frankel Equation for Turbulent Flames in the Presence of a Hydrodynamic Instability. Phys. Rev. E 1997, 55, 6911–6916. [Google Scholar] [CrossRef]
- Joulin, G.; Denet, B.; El-Rabii, H. Potential-Flow Models for Channelled Two-Dimensional Premixed Flames around near-Circular Obstacles. Phys. Rev. E 2010, 81, 016314. [Google Scholar] [CrossRef] [PubMed]
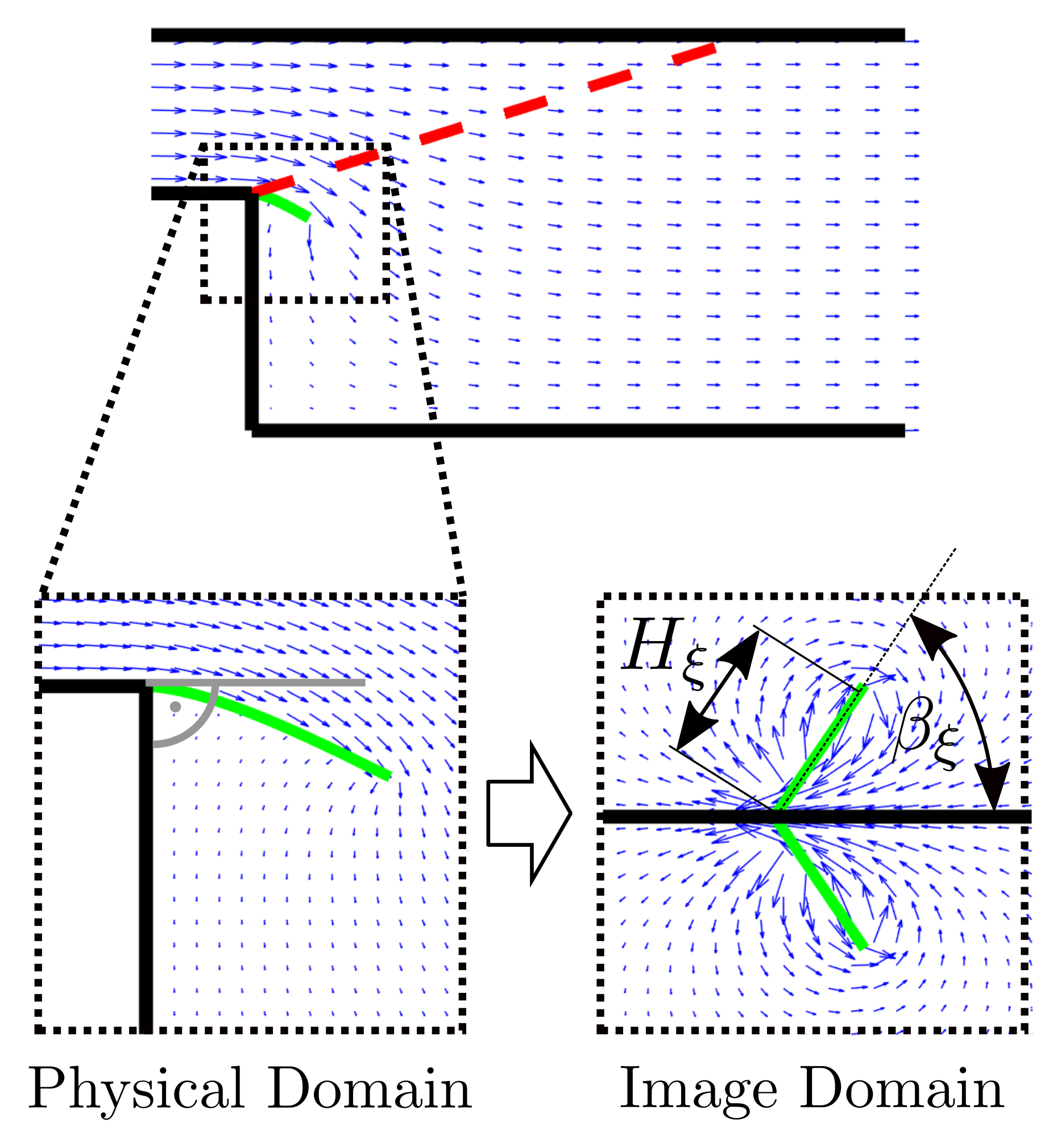
 ) and straight lines radiating from the origin of the image domain (
) and straight lines radiating from the origin of the image domain ( ), each in the physical domain (left) and the image domain (right).
), each in the physical domain (left) and the image domain (right).
 ) and straight lines radiating from the origin of the image domain (
) and straight lines radiating from the origin of the image domain ( ), each in the physical domain (left) and the image domain (right).
), each in the physical domain (left) and the image domain (right).

 ), the analytically predicted mean flame front (
), the analytically predicted mean flame front ( ) and the approximate location of the shear layer (
) and the approximate location of the shear layer ( ) are also shown.
) are also shown.
 ), the analytically predicted mean flame front (
), the analytically predicted mean flame front ( ) and the approximate location of the shear layer (
) and the approximate location of the shear layer ( ) are also shown.
) are also shown.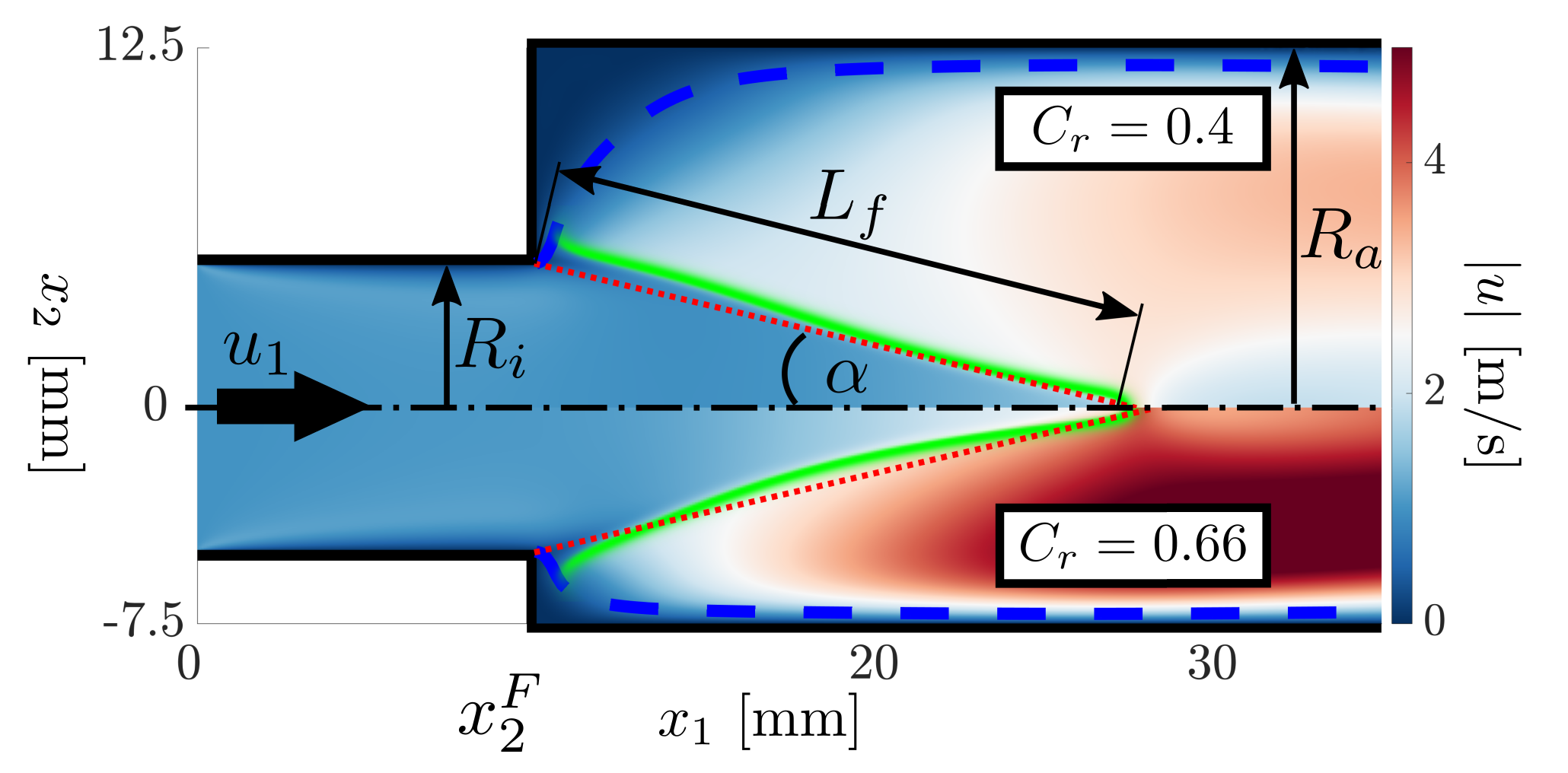
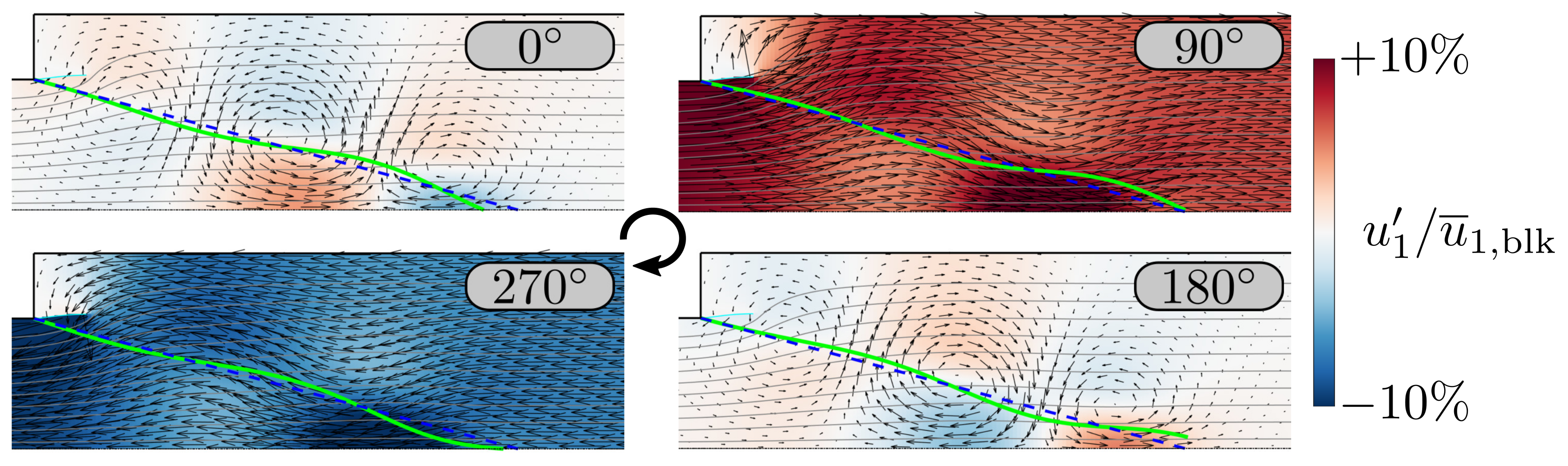
 ) is scaled by a factor of 7. Top: vortical flow component, with color along the shear layer indicating the strength of vorticity fluctuations. Bottom: irrotational flow component. The flame normal velocity is represented by green arrows (scaled by a factor of 4 for better visibility).
) is scaled by a factor of 7. Top: vortical flow component, with color along the shear layer indicating the strength of vorticity fluctuations. Bottom: irrotational flow component. The flame normal velocity is represented by green arrows (scaled by a factor of 4 for better visibility).
 ) is scaled by a factor of 7. Top: vortical flow component, with color along the shear layer indicating the strength of vorticity fluctuations. Bottom: irrotational flow component. The flame normal velocity is represented by green arrows (scaled by a factor of 4 for better visibility).
) is scaled by a factor of 7. Top: vortical flow component, with color along the shear layer indicating the strength of vorticity fluctuations. Bottom: irrotational flow component. The flame normal velocity is represented by green arrows (scaled by a factor of 4 for better visibility).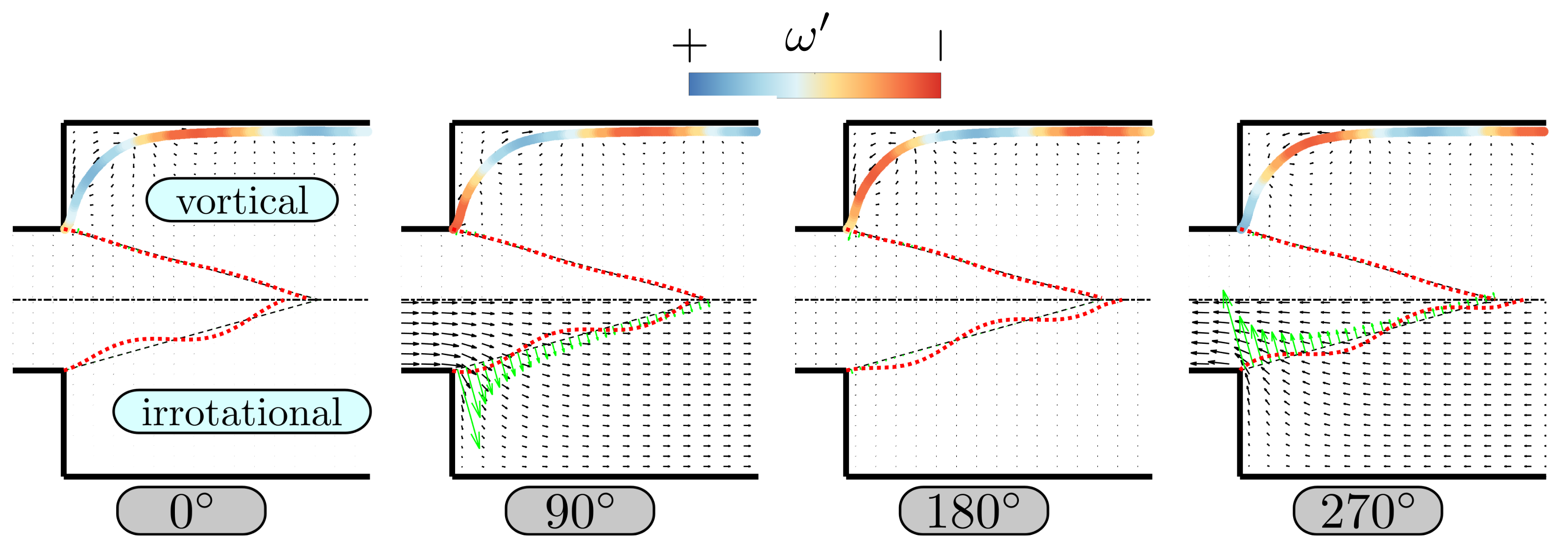
 ), (
), ( ), (
), ( ) and (
) and ( ). is set to for (c.f. Figure 4) and to for , respectively.
). is set to for (c.f. Figure 4) and to for , respectively.
 ), (
), ( ), (
), ( ) and (
) and ( ). is set to for (c.f. Figure 4) and to for , respectively.
). is set to for (c.f. Figure 4) and to for , respectively.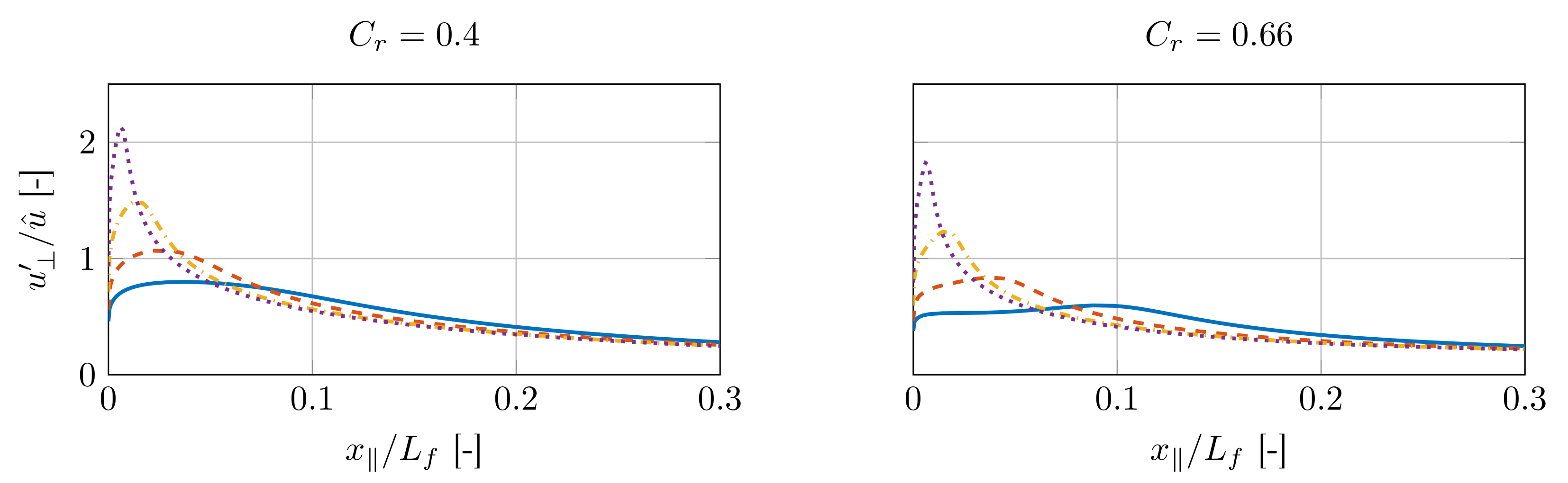
 ); UD model (
); UD model ( ); BD model (
); BD model ( ).
).
 ); UD model (
); UD model ( ); BD model (
); BD model ( ).
).
 ) vs. predictions of the unidirectional (
) vs. predictions of the unidirectional ( ) and bidirectional (
) and bidirectional ( ) models, that is without and with consideration of flame-generated vorticity ().
) models, that is without and with consideration of flame-generated vorticity ().
 ) vs. predictions of the unidirectional (
) vs. predictions of the unidirectional ( ) and bidirectional (
) and bidirectional ( ) models, that is without and with consideration of flame-generated vorticity ().
) models, that is without and with consideration of flame-generated vorticity ().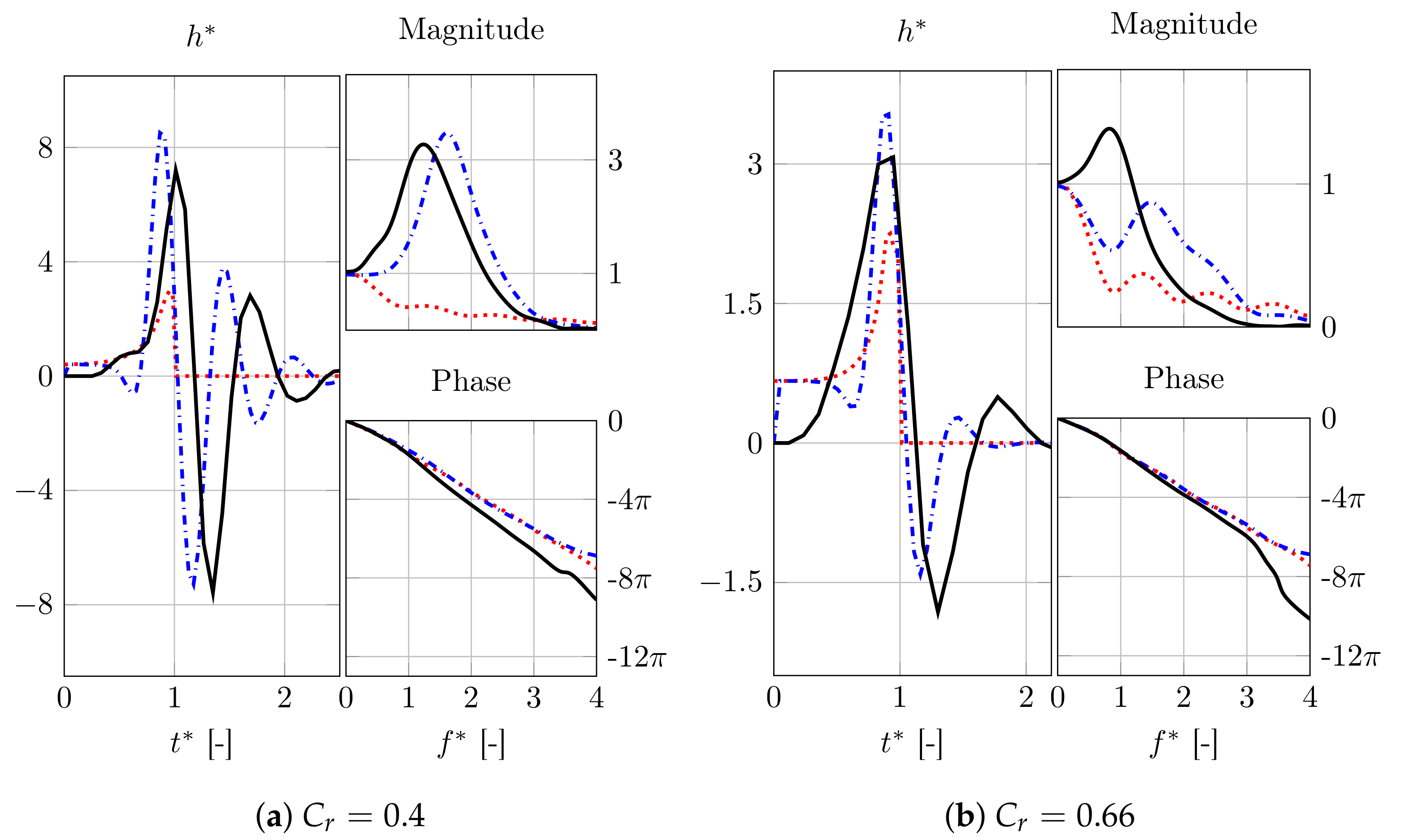

 ); perturbed flame (
); perturbed flame ( ). Minimum vortex kernel radius .
). Minimum vortex kernel radius .
 ); perturbed flame (
); perturbed flame ( ). Minimum vortex kernel radius .
). Minimum vortex kernel radius .
| Parameter | Value |
|---|---|
| Expansion ratio e | 6.68 |
| Flame speed | 0.2686 |
| Flame thickness | 83.95 m |
| Markstein number | 4 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Steinbacher, T.; Polifke, W. Convective Velocity Perturbations and Excess Gain in Flame Response as a Result of Flame-Flow Feedback. Fluids 2022, 7, 61. https://doi.org/10.3390/fluids7020061
Steinbacher T, Polifke W. Convective Velocity Perturbations and Excess Gain in Flame Response as a Result of Flame-Flow Feedback. Fluids. 2022; 7(2):61. https://doi.org/10.3390/fluids7020061
Chicago/Turabian StyleSteinbacher, Thomas, and Wolfgang Polifke. 2022. "Convective Velocity Perturbations and Excess Gain in Flame Response as a Result of Flame-Flow Feedback" Fluids 7, no. 2: 61. https://doi.org/10.3390/fluids7020061
APA StyleSteinbacher, T., & Polifke, W. (2022). Convective Velocity Perturbations and Excess Gain in Flame Response as a Result of Flame-Flow Feedback. Fluids, 7(2), 61. https://doi.org/10.3390/fluids7020061







