Numerical Simulation of Velocity Field around Two Columns of Tandem Piers of the Longitudinal Bridge
Abstract
1. Introduction
2. Model Setup
2.1. Governing Equations in the Hydrodynamic Model
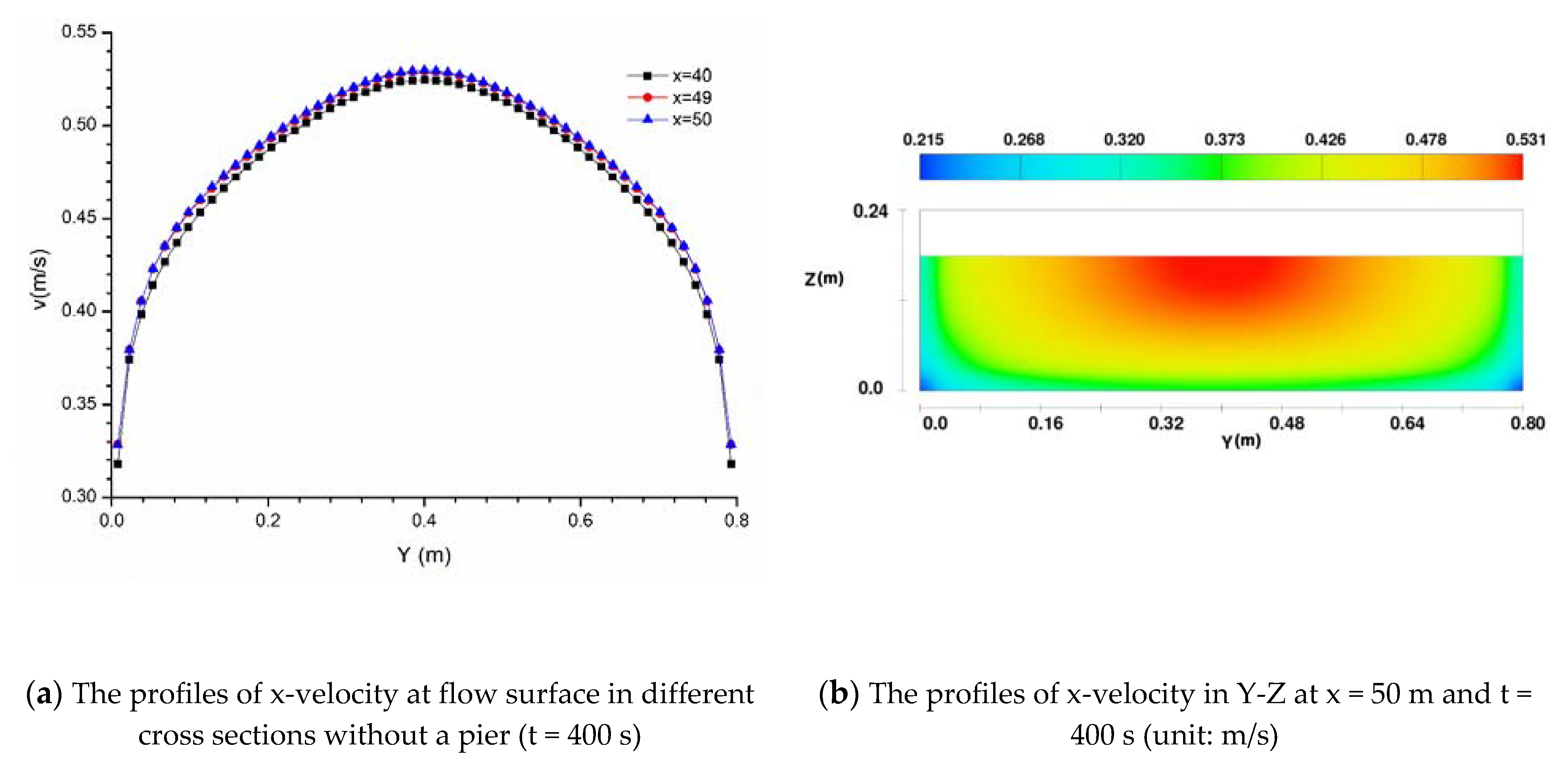
2.2. Model Setup without Piers
2.3. Model Setup with Piers
2.3.1. Parameters of the Model with Piers
2.3.2. Layout of the Model with Piers
3. Results and Discussion
3.1. Simulation of each Model
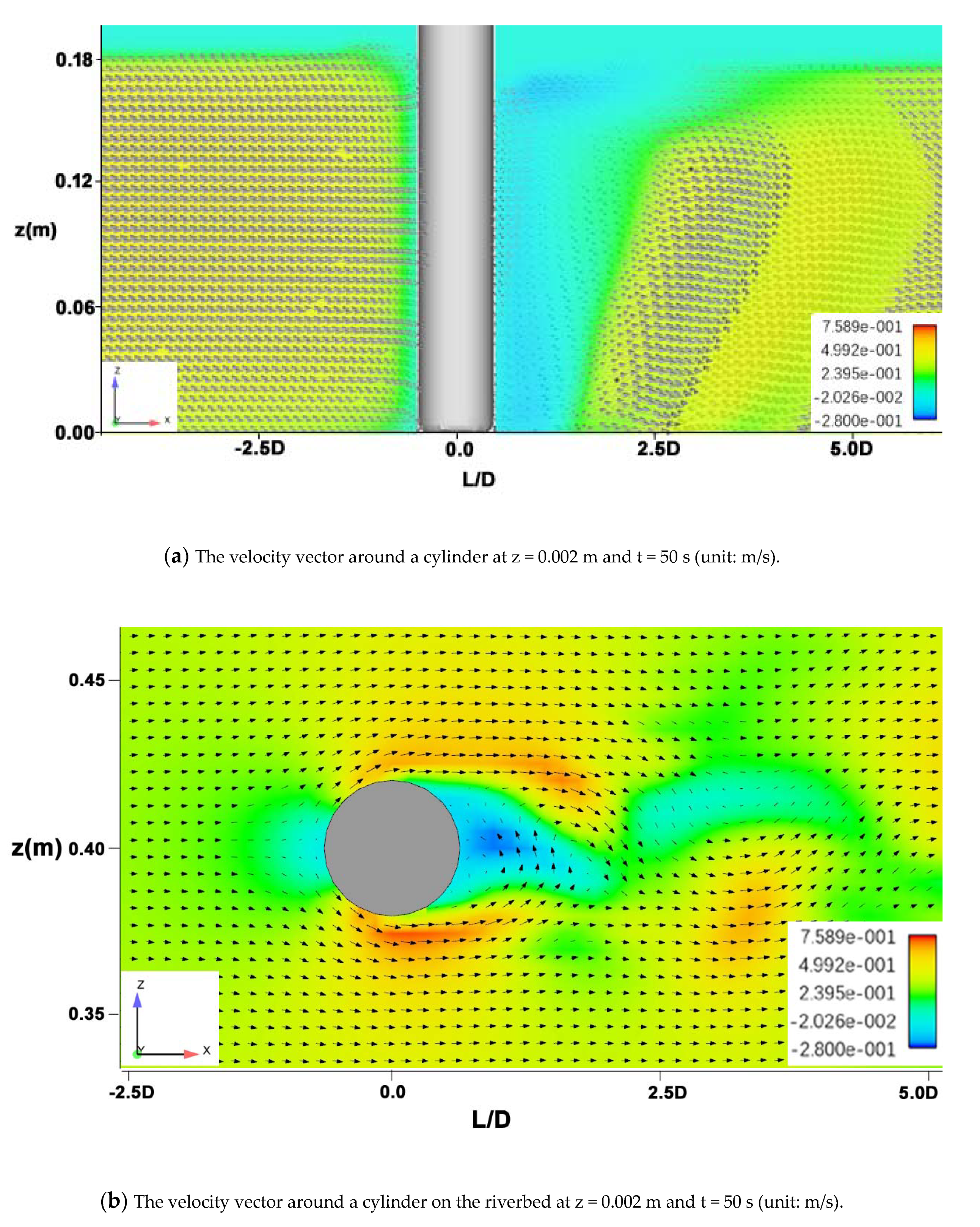
3.1.1. The X-Velocity Field abound Two Piers Arranged Side by Side
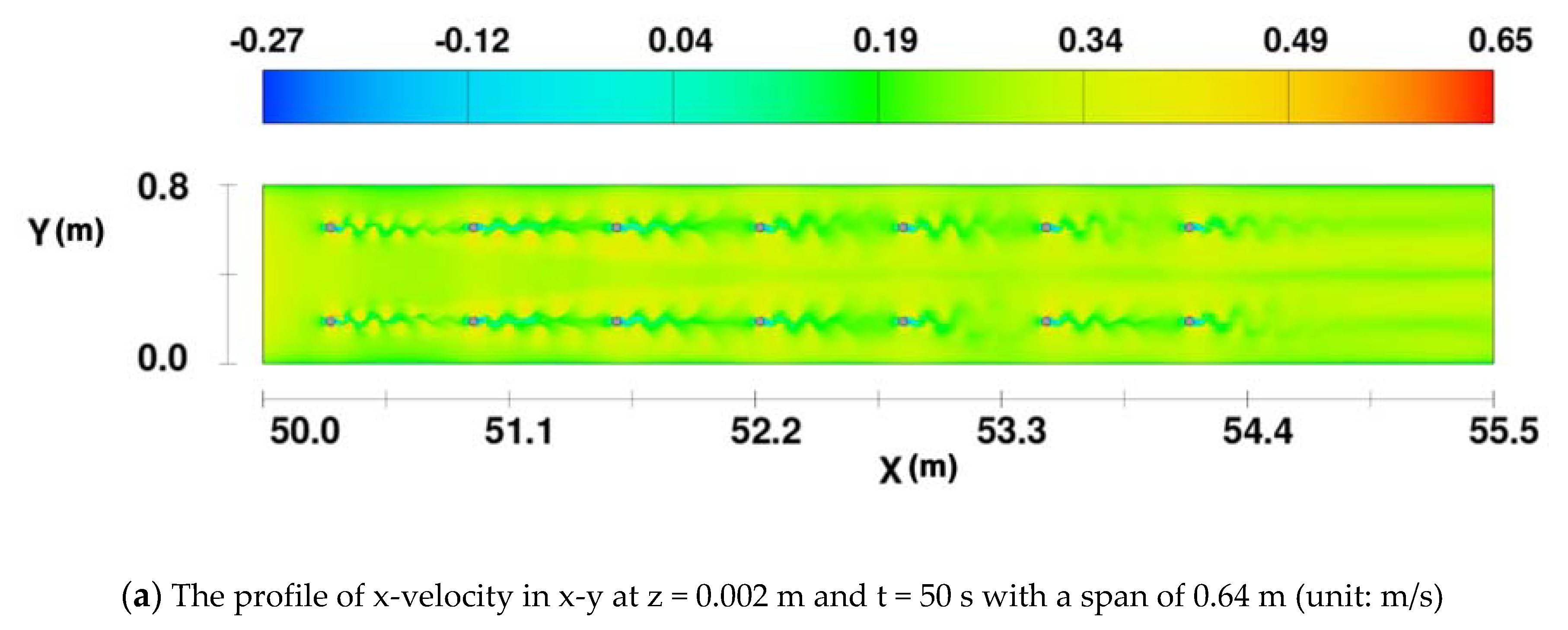
3.1.2. The X-Velocity Field around Two Columns of Tandem Piers of the Longitudinal Bridge
3.2. The X-Velocity of Cross-Sections in the Y-Direction
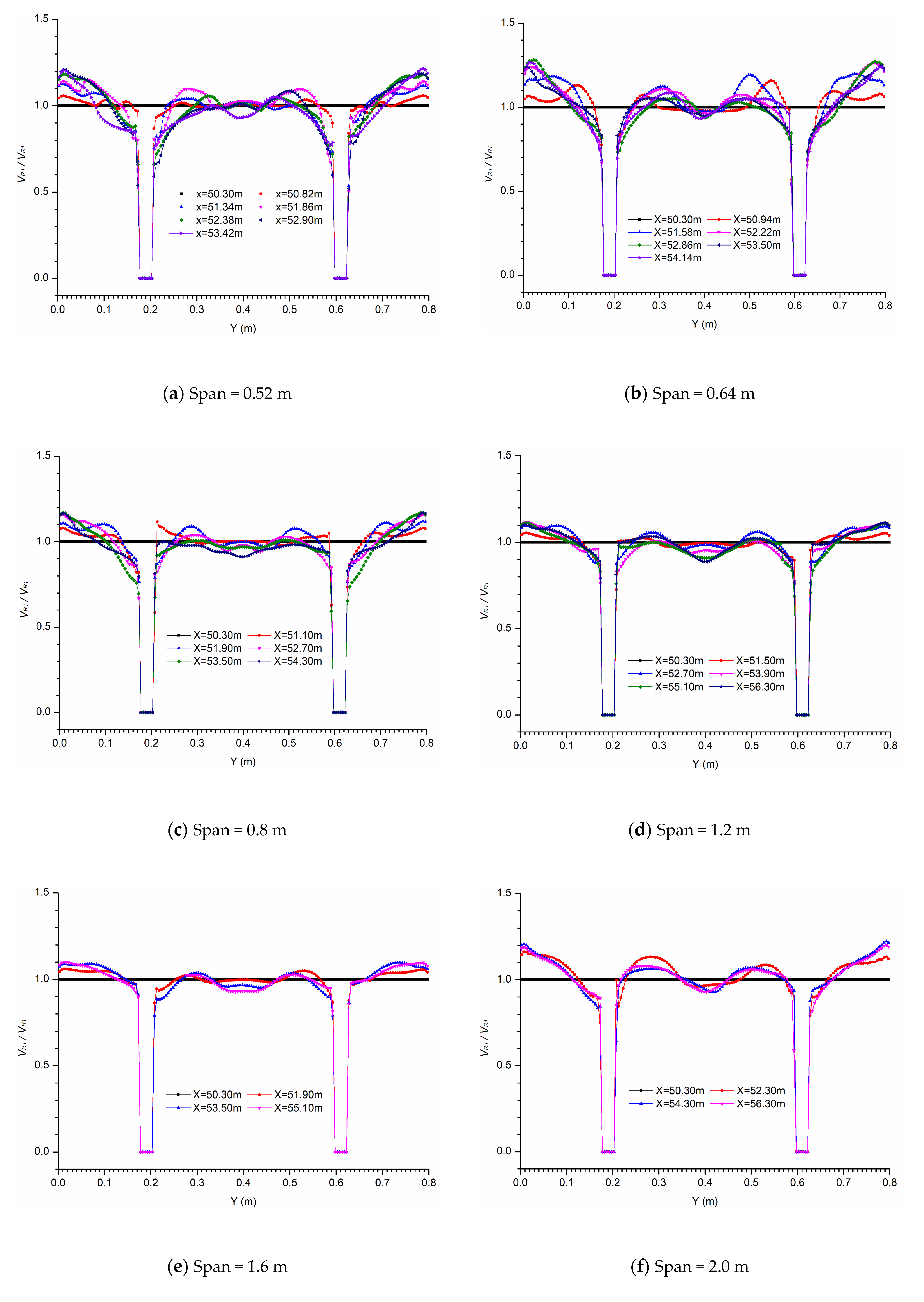
3.3. The Relationship between X-Velocity and the Span of the Pier
4. Conclusions
- With a span shorter than 27.5D, the shape and the lateral range of the x-velocity increase with the increase of distance downwards the x-direction. The accumulative influence of the superposition of Kármán Vortex Streets is obvious, and the shorter the span is, the more obvious the influence of the span on the shape and width of the x-velocity is. When the span is longer than 27.5D, the accumulative effects reduce and x-velocity fields become visually almost independent of each other.
- For the area between the piers and the wall, the VRi/VR1 near the wall increases up to 1.26. If the span is shorter than 27.5D, the largest VRi/VR1 is around 1.17–1.26, and it is about 1.10 when the span is between 27.5D and 40D. When the span is 50D, the largest VRi/VR1 is around 1.20. For the area between the two columns of tandem piers, the profile of the VRi/VR1 changes from a “∩-shape” to an “M-shape” in each model, which indicates that the value of x-velocity in the middle of each model becomes smaller in the x direction.
- RAVC increases gradually and tends to be stable with the increases of the span. The largest RAVC is about −17.66% with a span of 0.52 m and gets close to that of two piers arranged side by side gradually with the increase of span.
- The maximum x-velocity reduces rapidly from the 1st pier to the 3rd pier if the span is shorter than 27.5D, then slowly reduces from the 4th pier to the last one. If the span is longer than 27.5D, the maximum x-velocity is close from the 2nd pier and finally becomes stable.
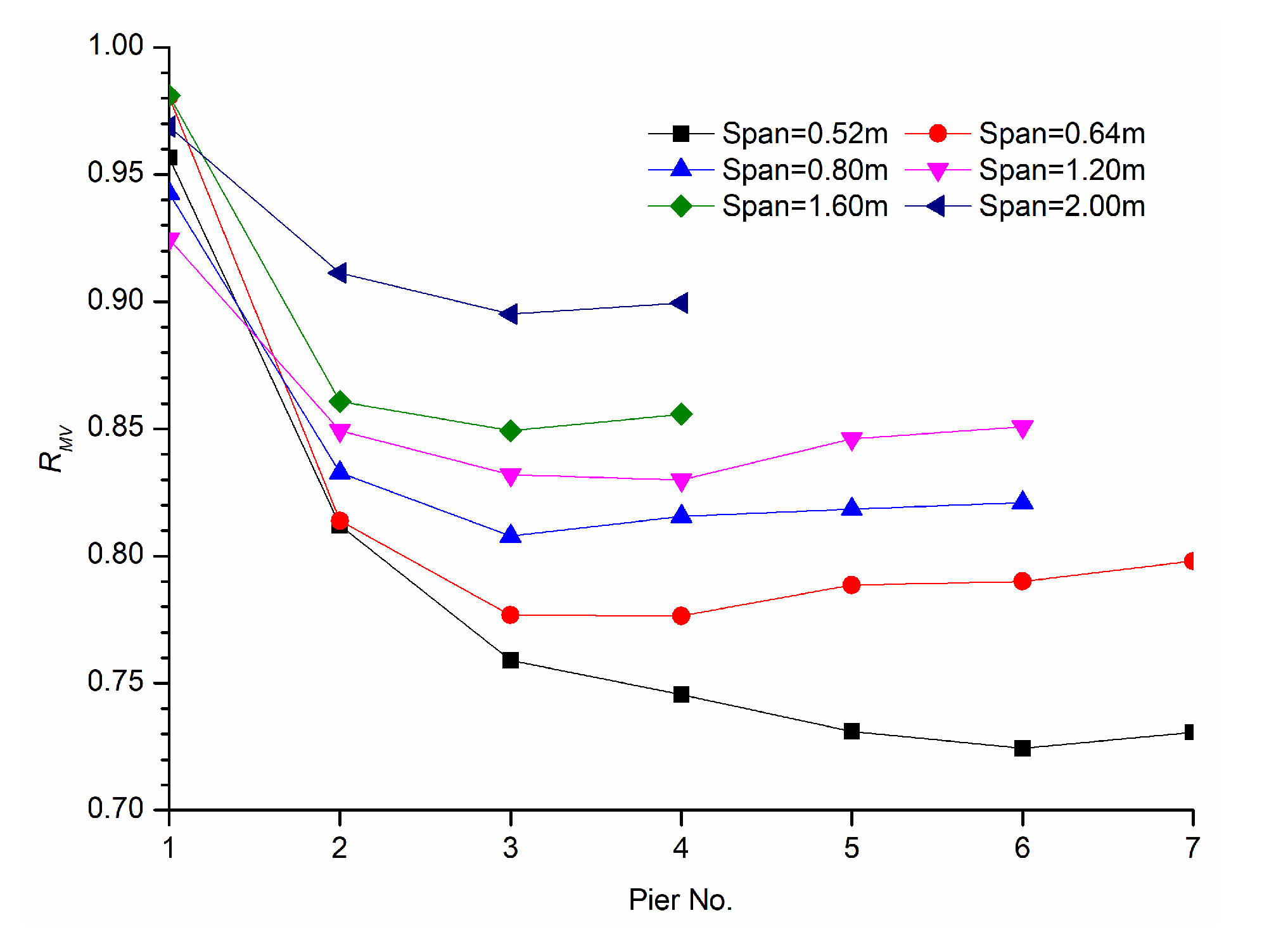
- The RMV of the piers in the first row of different models is around 0.95, and then reduces sharply to the second pier in each model, which is around 0.82 when the span is shorter than 27.5D and up to 0.91 if the span is longer than 27.5D. If the span is longer than 27.5D, the RMV is close to each other from the 2nd pier to the last one.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Julio, S. An investigation of the wake of a circular cylinder using a video-based digital cross-correlation particle image velocimetry technique. Exp. Therm. Fluid Sci. 1996, 12, 221–233. [Google Scholar]
- Lin, J.C.; Rockwell, D. Horizontal oscillation of a cylinder beneath a free surface vortex formation and loading. J. Fluid Mech. 1999, 389, 1–26. [Google Scholar] [CrossRef]
- Breuer, M.S.; Jørgen, F. Hydrodynamics around Cylindrical Structures; World Scientific Press: Copenhagen, Denmark, 2006. [Google Scholar]
- Lin, J.C.; Yang, Y.; Rockwell, D. Flow past two cylinders in tandem: Instantaneous and averaged flow structure. J. Fluids Struct. 2002, 16, 1059–1071. [Google Scholar] [CrossRef]
- Tian, W.P.; Shen, B. Circling flow characteristics around cylinder pier. J. Xi’an Highw. Univ. 2003, 23, 54–57. [Google Scholar]
- He, G.J.; Fang, H.W.; Fu, R.S. Three-dimensional numerical analyses on water flow affected by piers. J. Hydrodyn. Ser. A 2007, 22, 345–351. [Google Scholar]
- Li, B.; Sun, D.P.; Lai, G.W. The influence of bridge pier arrangement on circling flow around bridge pier and local velocity field. China Rural Water Hydropower 2013, 129–132, 134. [Google Scholar]
- Kim, H.J.; Durbin, P.A. Investigation of the flow between a pair of cylinders in the flopping regime. J. Fluid Mech. 1988, 196, 431–448. [Google Scholar] [CrossRef]
- Gu, Z.F. On interference between two circular cylinders at supercritical Reynolds number. J. Wind Eng. Ind. Aerodyn. 1996, 62, 175–190. [Google Scholar] [CrossRef]
- Sumner, D.; Price, S.J.; Paidoussis, M.P. Flow-pattern identification for two staggered circular cylinders in cross-flow. J. Fluid Mech. 2000, 411, 263–303. [Google Scholar] [CrossRef]
- Meneghini, J.R.; Saltara, F.; Siqueira, C.L.R.; Ferrarijr, J.A. Numerical simulation of flow interference between two circular cylinders in tandem and side-by-side arrangements. J. Fluids Struct. 2001, 15, 327–350. [Google Scholar] [CrossRef]
- Li, W.W.; Huang, B.S.; Hou, J. Experimental research on hydraulic characteristics of pile wharf upon the flow of the channel. J. Xinjiang Agric. Univ. 2004, 27, 78–81. [Google Scholar]
- Ataie-Ashtiani, B.; Aslani-Kordkandi, A. Flow field around side-by-side piers with and without a scour hole. Eur. J. Mech. B Fluids 2012, 36, 152–166. [Google Scholar] [CrossRef]
- Yan, J.K.; Jiao, C.; Long, T. Bed fixed experimental study on velocity distribution of bridge piers with different intersection angles between bridge axle and flow direction. J. Xi’an Univ. Archit. Technol. 2013, 45, 822–828. [Google Scholar]
- Igarashi, T. Characteristics of the flow around two circular cylinders arranged in tandem. Bull. JSME 1981, 24, 323–331. [Google Scholar] [CrossRef]
- Mahbub, A.M.; Zhou, Y. Strouhal numbers, forces and flow structures around two tandem cylinders of different diameters. J. Fluids Struct. 2008, 24, 505–526. [Google Scholar] [CrossRef]
- Ataie-Ashtiani, B.; Aslani-Kordkandi, A. Flow field around single and tandem piers. Flow Turbul. Combust. 2013, 90, 471–490. [Google Scholar] [CrossRef]
- Wang, H.; Tang, H.W.; Xiao, J.F.; Jiang, S. Clear-water local scouring around three piers in a tandem arrangement. Sci. China Technol. Sci. 2016, 59, 888–896. [Google Scholar] [CrossRef]
- Chavan, R.; Kumar, B. Experimental investigation on flow and scour characteristics around tandem piers in sandy channel with downward seepage. J. Mar. Sci. Appl. 2017, 16, 313–322. [Google Scholar] [CrossRef]
- Beg, M.; Beg, S. Scour hole characteristics of two unequal size bridge piers in tandem arrangement. ISH J. Hydraul. Eng. 2015, 21, 85–96. [Google Scholar] [CrossRef]
- Schindfessel, L.; Creëlle, S.; De Mulder, T. Flow patterns in an open channel confluence with increasingly dominant tributary inflow. Water 2015, 7, 4724–4751. [Google Scholar] [CrossRef]
- Ersoy, H.; Karahan, M.; Gelişli, K.; Akgün, A.; Anılan, T.; OğuzSünnetci, M.; Yahşi, B.K. Modelling of the landslide-induced impulse waves in the Artvin Dam reservoir by empirical approach and 3D numerical simulation. Eng. Geol. 2019, 249, 112–128. [Google Scholar] [CrossRef]
- Oscar, H.G.; Stanisław, W.K. Numerical and physical modeling of water flow over the ogee weir of the new Niedów barrage. J. Hydrol. Hydromech. 2016, 64, 67–74. [Google Scholar]
- Quaresma, A.L.; Romão, F.; Branco, P.; Maria, T.F.; António, N.P. Multi slot versus single slot pool-type fishways: A modeling approach to compare hydrodynamics. Ecol. Eng. 2018, 22, 197–206. [Google Scholar] [CrossRef]
- Thompson, J.M.; Hathaway, J.M.; Schwartz, J.S. Three-dimensional modeling of the hydraulic function and channel stability of regenerative stormwater conveyances. J. Sustain. Water Built Environ. 2018, 4, 1–12. [Google Scholar] [CrossRef]
- Veksler, A.B.; Safin, S.Z. Hydraulic regimes and downstream scour at the Kama Hydropower Plant. Power Technol. Eng. 2018, 51, 491–500. [Google Scholar] [CrossRef]
- Morovati, K.; Eghbalzadeh, A. Study of inception point, void fraction and pressure over pooled stepped spillways using FLOW-3D. Int. J. Numer. Methods Heat Fluid Flow 2018, 28, 982–998. [Google Scholar] [CrossRef]
- Movahedi, A.; Kavianpour, M.R.; Aminoroayaie, Y.O. Evaluation and modeling scouring and sedimentation around downstream of large dams. Environ. Earth Sci. 2018, 77, 320–327. [Google Scholar] [CrossRef]
- Song, Y.; Zhang, L.L.; Li, J.; Chen, M.; Zhang, Y.W. Mechanism of the influence of hydrodynamics on Microcystis aeruginosa, a dominant bloom species in reservoirs. Sci. Total Environ. 2018, 15, 230–239. [Google Scholar] [CrossRef]
- Yang, S.L.; Yang, W.L.; Qin, S.Q.; Li, Q.; Yang, B. Numerical study on characteristics of a dam-break wave. Ocean. Eng. 2018, 159, 358–371. [Google Scholar] [CrossRef]
- Palau-Salvador, G.; Stoesser, T.; Rodi, W. LES of the flow around two cylinders in tandem. J. Fluids Struct. 2008, 24, 1304–1312. [Google Scholar] [CrossRef]
- Mohamed, H.I. Numerical simulation of flow and local scour at two submerged-emergent tandem cylindrical piers. J. Eng. Sci. 2013, 41, 1–19. [Google Scholar]
- Jafari, M.; Ayyoubzadeh, S.A.; Esmaeilivaraki, M.; Rostami, M. Simulation of flow pattern around inclined bridge group pier using FLOW-3D Software. J. Water Soil 2017, 30, 1860–1873. [Google Scholar]
- Bayon, A.; Valero, D.; García-Bartual, R.; Vallés-Morána, F.J.; López-Jiméneza, P.A. Performance assessment of Open FOAM and FLOW-3D in the numerical modeling of a low Reynolds number hydraulic jump. Environ. Model. Softw. 2016, 80, 322–335. [Google Scholar] [CrossRef]
- Ali, K.H.M.; Karim, O. Simulation of flow around piers. J. Hydraul. Res. 2002, 40, 161–174. [Google Scholar] [CrossRef]
- Calomino, F.; Tafarojnoruz, A.; De Marchis, M.; Gaudio, R. Experimental and numerical study on the flow field and friction factor in a pressurized corrugated pipe. J. Hydraul. Eng. 2015, 141, 1–23. [Google Scholar] [CrossRef]
- Duguay, J.M.; Lacey, R.W.J.; Gaucher, J. A case study of a pool and weir fishway modeled with open foam and FLOW-3D. Ecol. Eng. 2017, 103, 31–42. [Google Scholar] [CrossRef]
- Ran, D.J.; Wang, W.E.; Hu, X.T. Three-dimensional numerical simulation of flow in trapezoidal cutthroat flumes based on FLOW-3D. Front. Agric. Sci. Eng. 2018, 5, 168–176. [Google Scholar] [CrossRef]
- Nguyen, V.T. 3D numerical simulation of free surface flows over hydraulic structures in natural channels and rivers. Appl. Math. Model. 2015, 39, 6285–6306. [Google Scholar] [CrossRef]
- Sarker, M.A. Flow Measurement around scoured bridge piers using Acoustic-Doppler Velocimeter (ADV). Flow Meas. Instrum. 1998, 9, 217–227. [Google Scholar] [CrossRef]
- Breuer, M. Numerical and modeling influences on large-eddy simulations for the flow past a circular cylinder. Int. J. Heat Fluid Flow 1998, 19, 512–521. [Google Scholar] [CrossRef]
- Roulund, A. Three-Dimensional Numerical Modeling of Flow around a Bottom-Mounted Pile and Its Application to Scour. Ph.D. Thesis, Technical University of Denmark, Kongens Lyngby, Denmark, 2000. [Google Scholar]
- Roulund, A.; Sumer, B.M.; Fredsøe, J.; Michelsen, J. Numerical and experimental investigation of flow and scour around a circular pile. J. Fluid Mech. 2005, 534, 351–401. [Google Scholar] [CrossRef]









| Source | Arrangements of Piers | Results |
|---|---|---|
| Ataie-Ashtiani et al. [17] | Two circular piers arranged in tandem |
|
| Wang et al. [18] | Three piers arranged in tandem under steady clear-water conditions. |
|
| Item | Width of Channel (m) | Height of Channel (m) | Average Velocity of Cross-Section (m/s) | Initial Flow Depth (m) | Pier Diameter (m) | Shape of Cross-Section |
|---|---|---|---|---|---|---|
| Parameters | 20D (0.8) | 6D (0.24) | 0.5 | 4D (0.16) | 0.04 | Rectangle |
| Item | Xmin (Upstream) | Xmax (Downstream) | Ymin | Ymax | Zmin | Zmax |
|---|---|---|---|---|---|---|
| Boundary conditions | velocity (0.5 m/s) | outflow | wall | wall | wall | pressure |
| Factors | Levels | |||||
|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | |
| Plane of river | straight | |||||
| Plane of bridge | straight | |||||
| Span of pier (m) | 0.52 | 0.64 | 0.80 | 1.20 | 1.60 | 2.00 |
| Position in the river (Distance between the centerline of the model and the channel bank) (m) | 0.4 | |||||
| Span | 0.52 m | 0.64 m | 0.80 m | 1.20 m | 1.60 m | 2.00 m | 2 Piers |
|---|---|---|---|---|---|---|---|
| V2 (m/s) | 0.275 | 0.279 | 0.280 | 0.289 | 0.297 | 0.299 | 0.302 |
| V1 (m/s) | 0.334 | ||||||
| RAVC (%) | −17.66 | −16.47 | −16.17 | −13.47 | −11.08 | −10.48 | −9.58 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qi, H.; Zheng, J.; Zhang, C. Numerical Simulation of Velocity Field around Two Columns of Tandem Piers of the Longitudinal Bridge. Fluids 2020, 5, 32. https://doi.org/10.3390/fluids5010032
Qi H, Zheng J, Zhang C. Numerical Simulation of Velocity Field around Two Columns of Tandem Piers of the Longitudinal Bridge. Fluids. 2020; 5(1):32. https://doi.org/10.3390/fluids5010032
Chicago/Turabian StyleQi, Hongliang, Junxing Zheng, and Chenguang Zhang. 2020. "Numerical Simulation of Velocity Field around Two Columns of Tandem Piers of the Longitudinal Bridge" Fluids 5, no. 1: 32. https://doi.org/10.3390/fluids5010032
APA StyleQi, H., Zheng, J., & Zhang, C. (2020). Numerical Simulation of Velocity Field around Two Columns of Tandem Piers of the Longitudinal Bridge. Fluids, 5(1), 32. https://doi.org/10.3390/fluids5010032





