Modeling Flow and Pressure Fields in Porous Media with High Conductivity Flow Channels and Smart Placement of Branch Cuts for Variant and Invariant Complex Potentials
Abstract
1. Introduction
2. Methodology: Swapping Complex Potentials
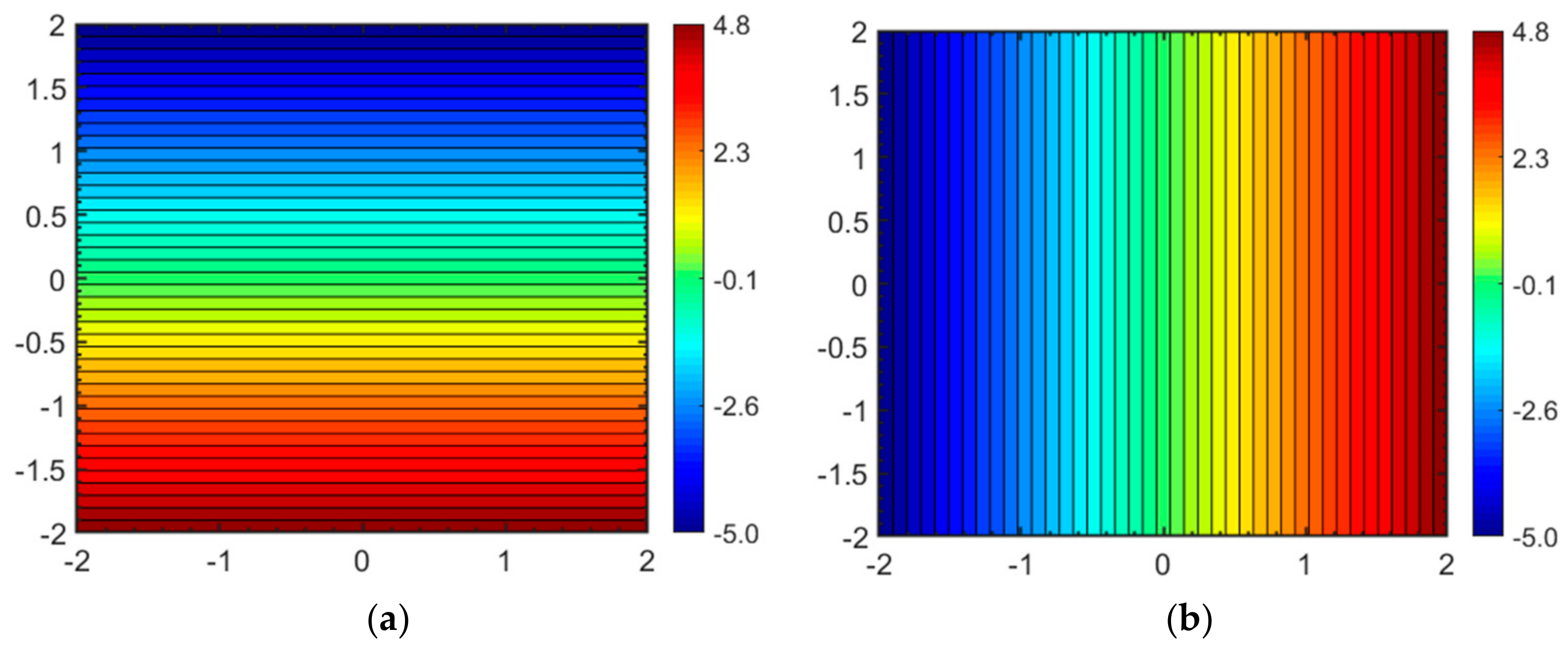
2.1. Simple Invariant Complex Potential: The Far-Field Flow
2.2. Simple Variant Complex Potential: The Source/Sink and Vortex Flows
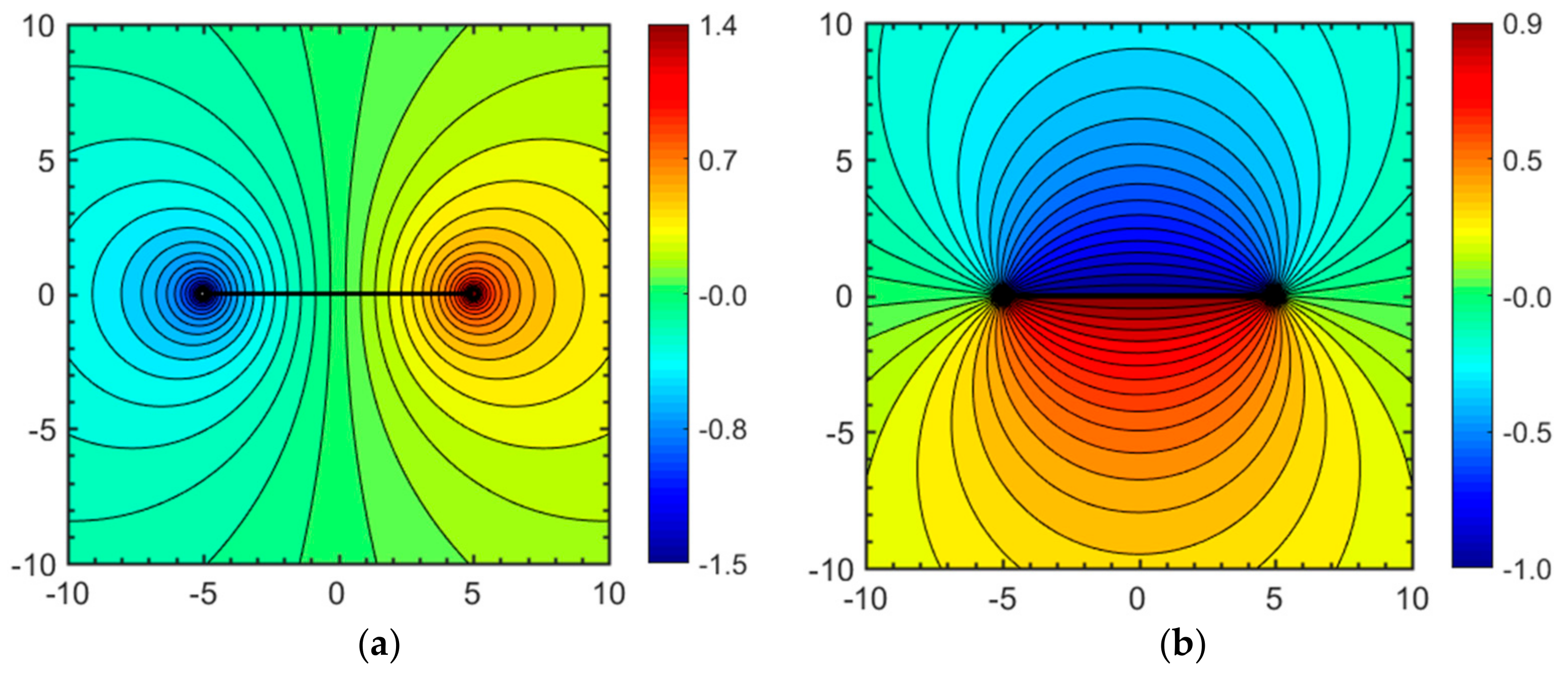
2.2.1. Source/Sink Flows
2.2.2. Vortex
2.2.3. Branch Cuts
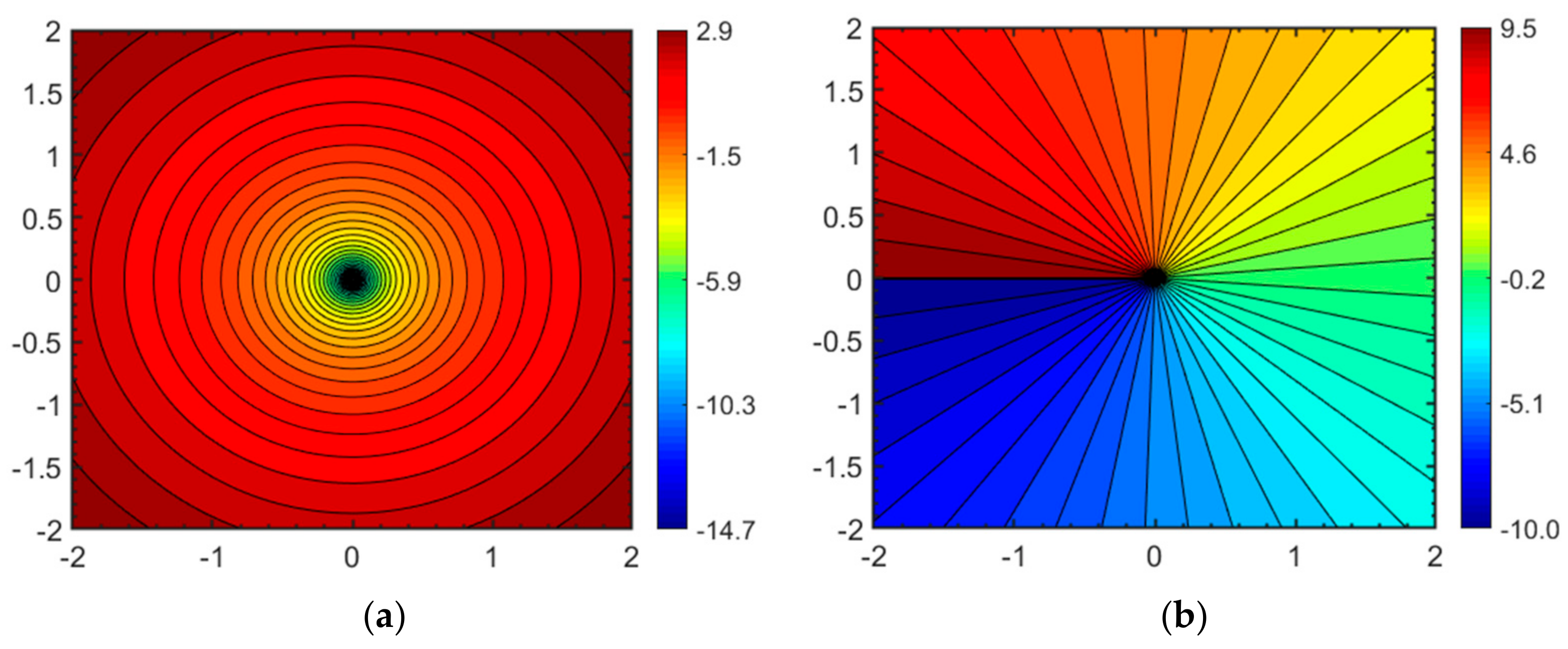
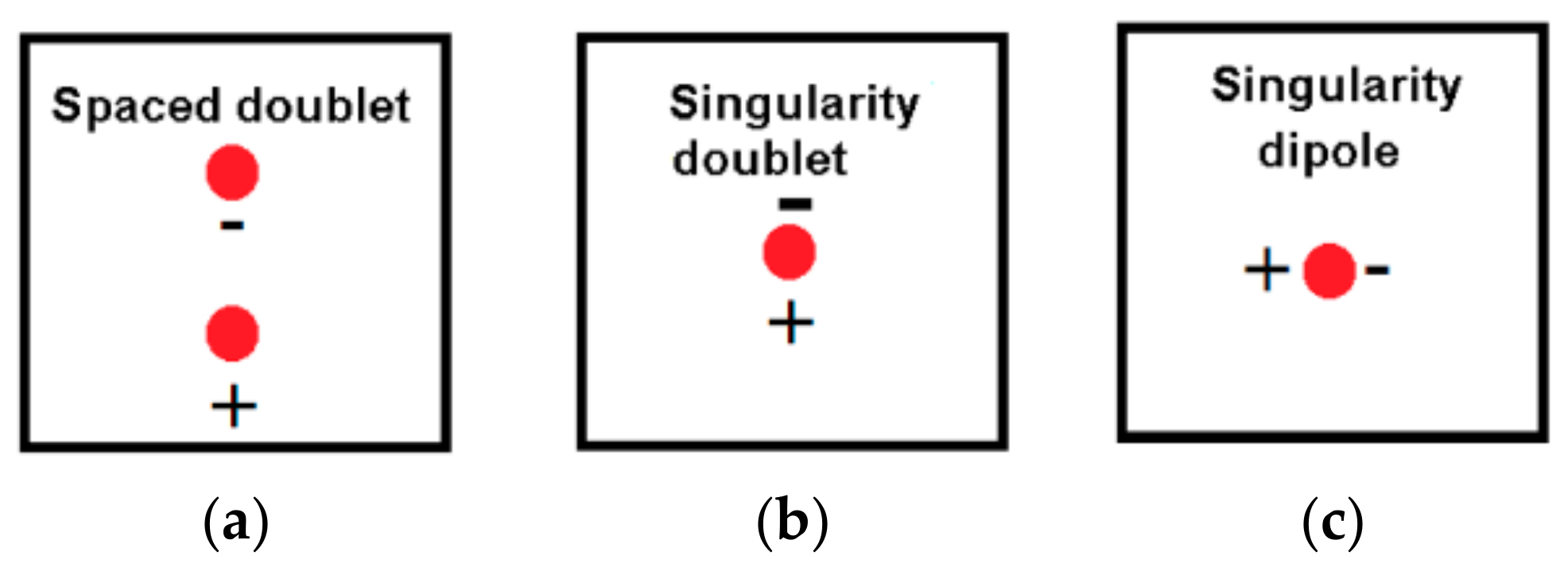
2.3. Superposed Invariant Complex Potentials: Doublet Flow
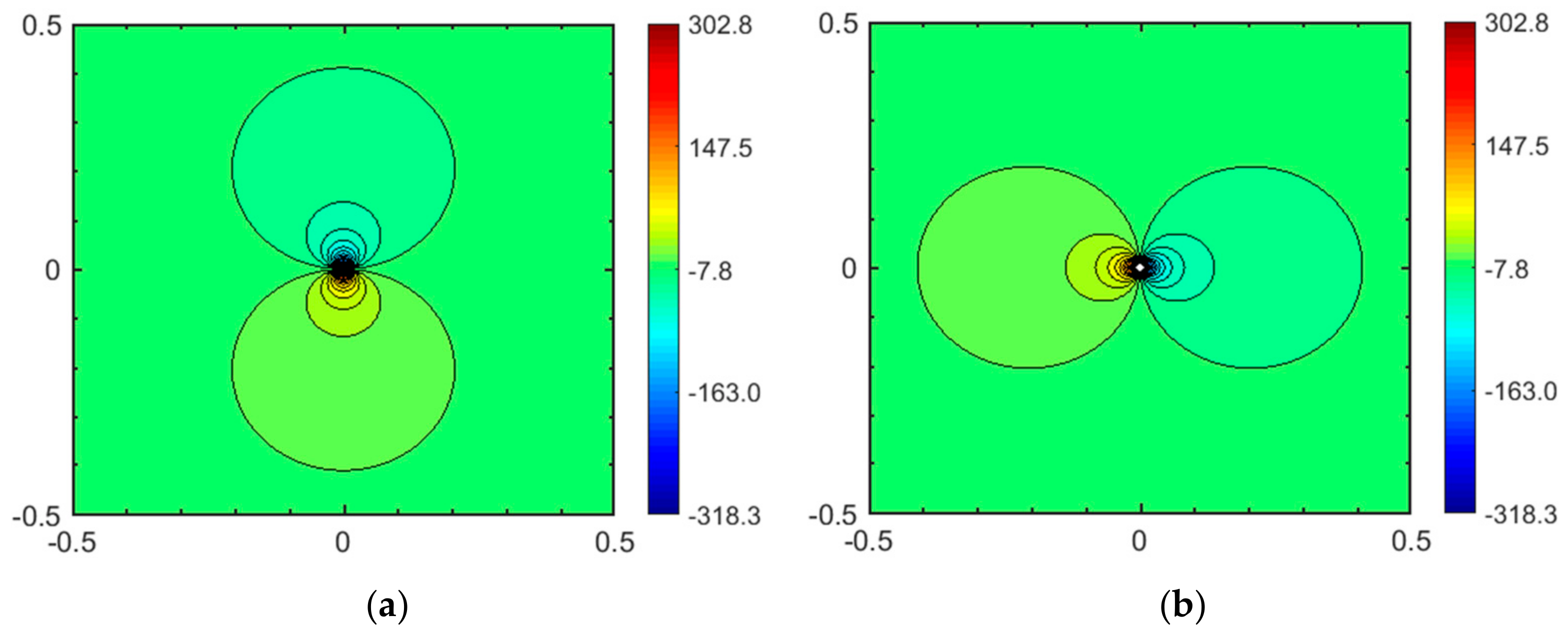
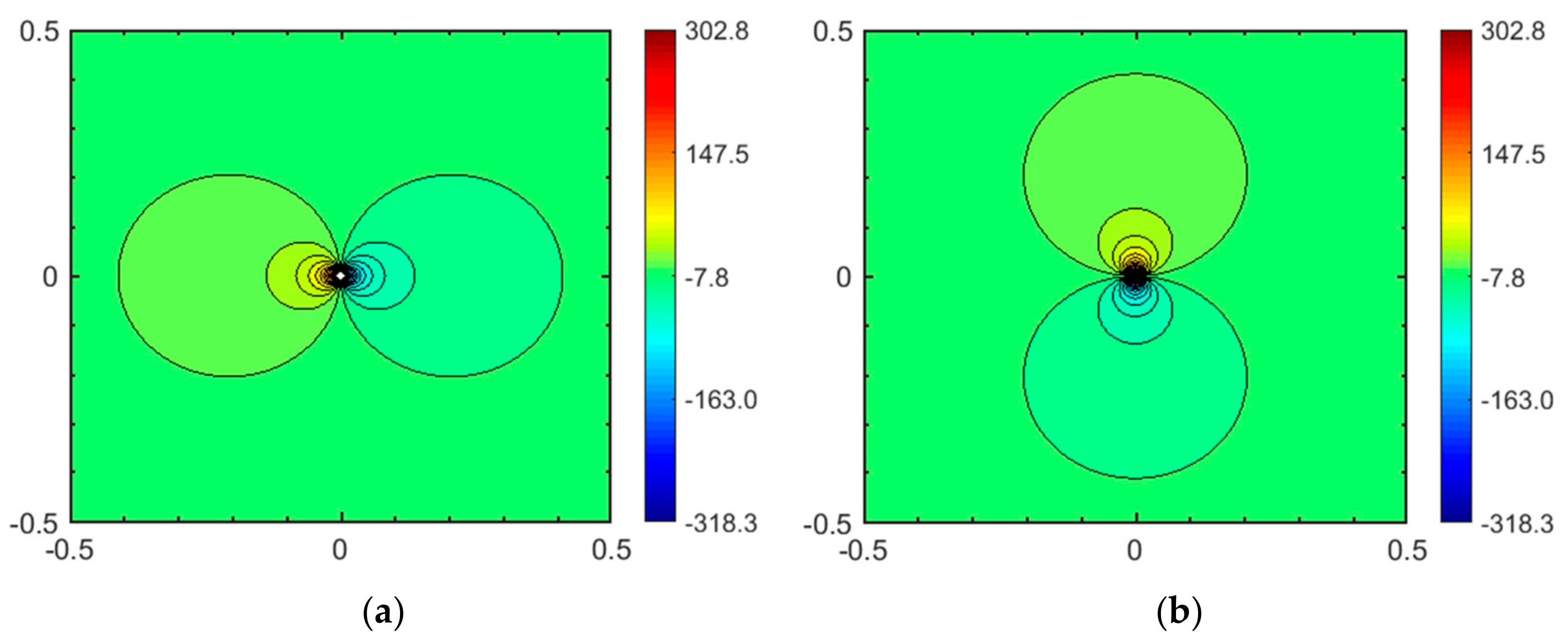
2.3.1. Singularity Doublet
2.3.2. Singularity Dipole
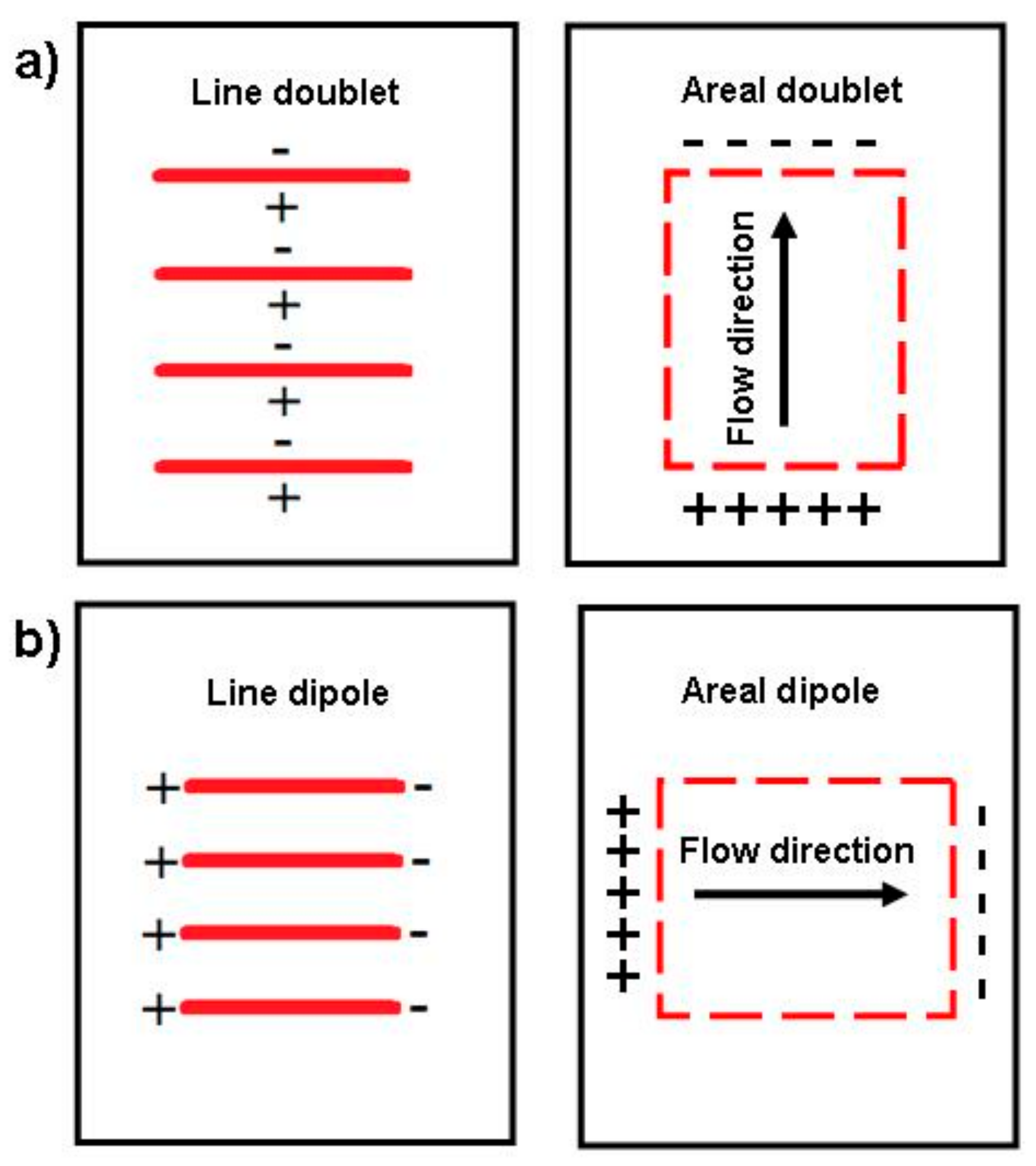
2.4. Superposed Variant Complex Potential: Line Doublets and Line Dipoles
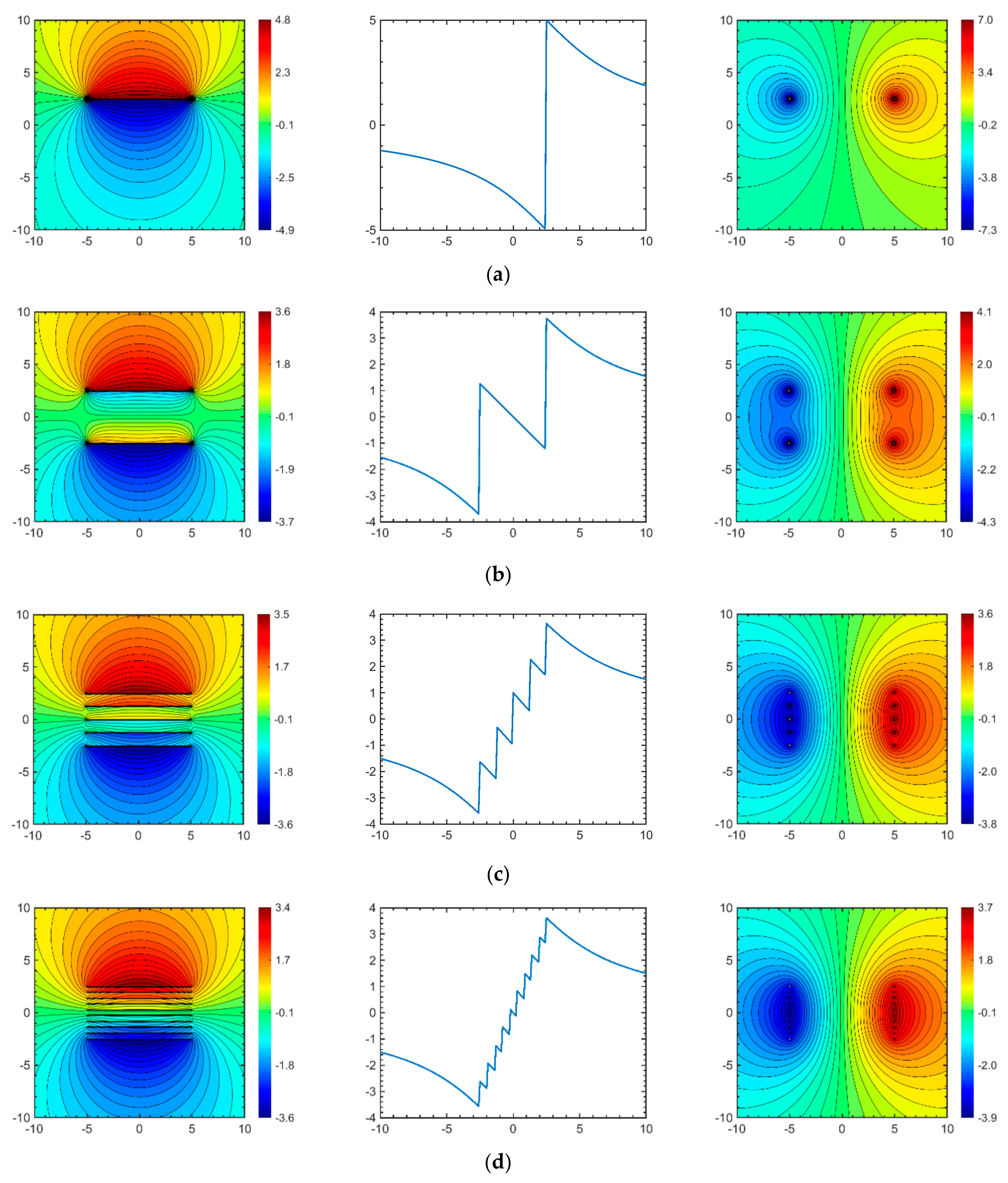
2.4.1. Line Doublets
2.4.2. Line Dipoles
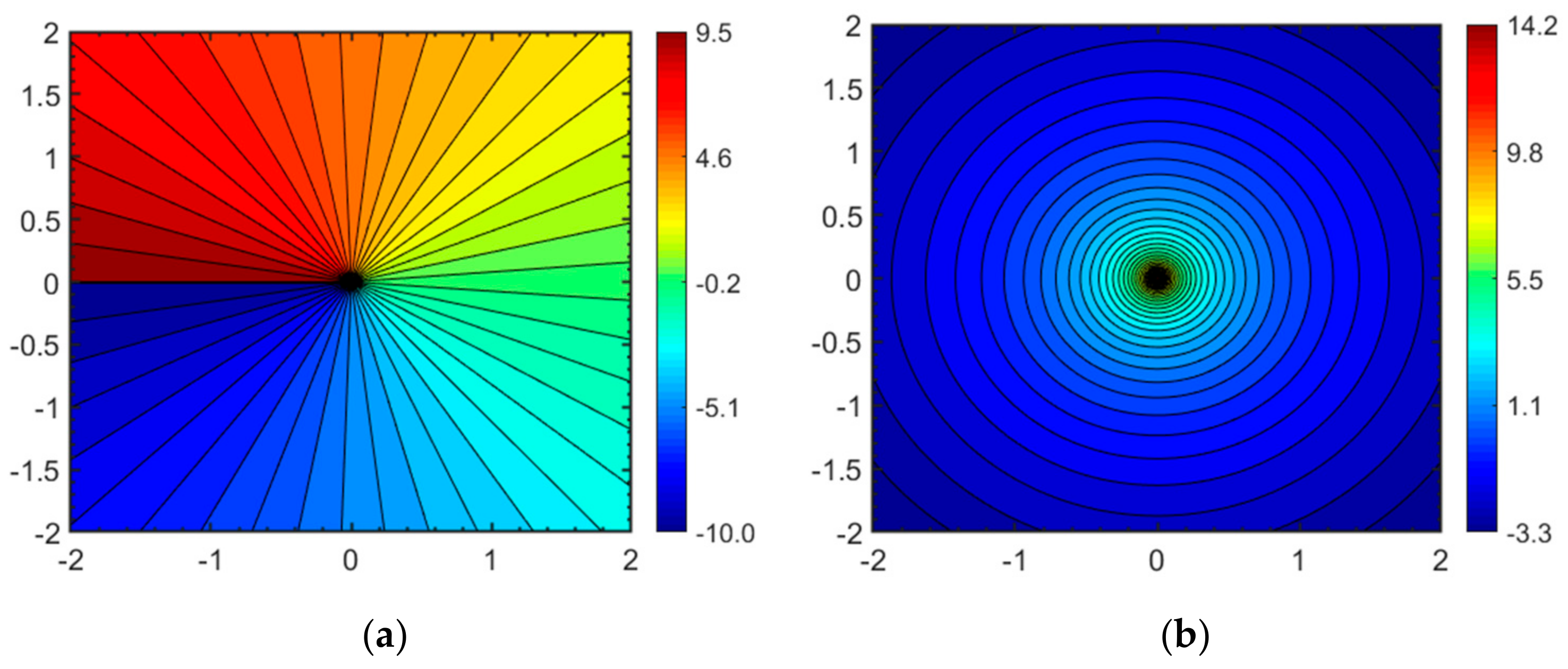
3. Complex Potentials to Describe Flow in Enhanced Permeability Flow Channels—Invariant Complex Potentials
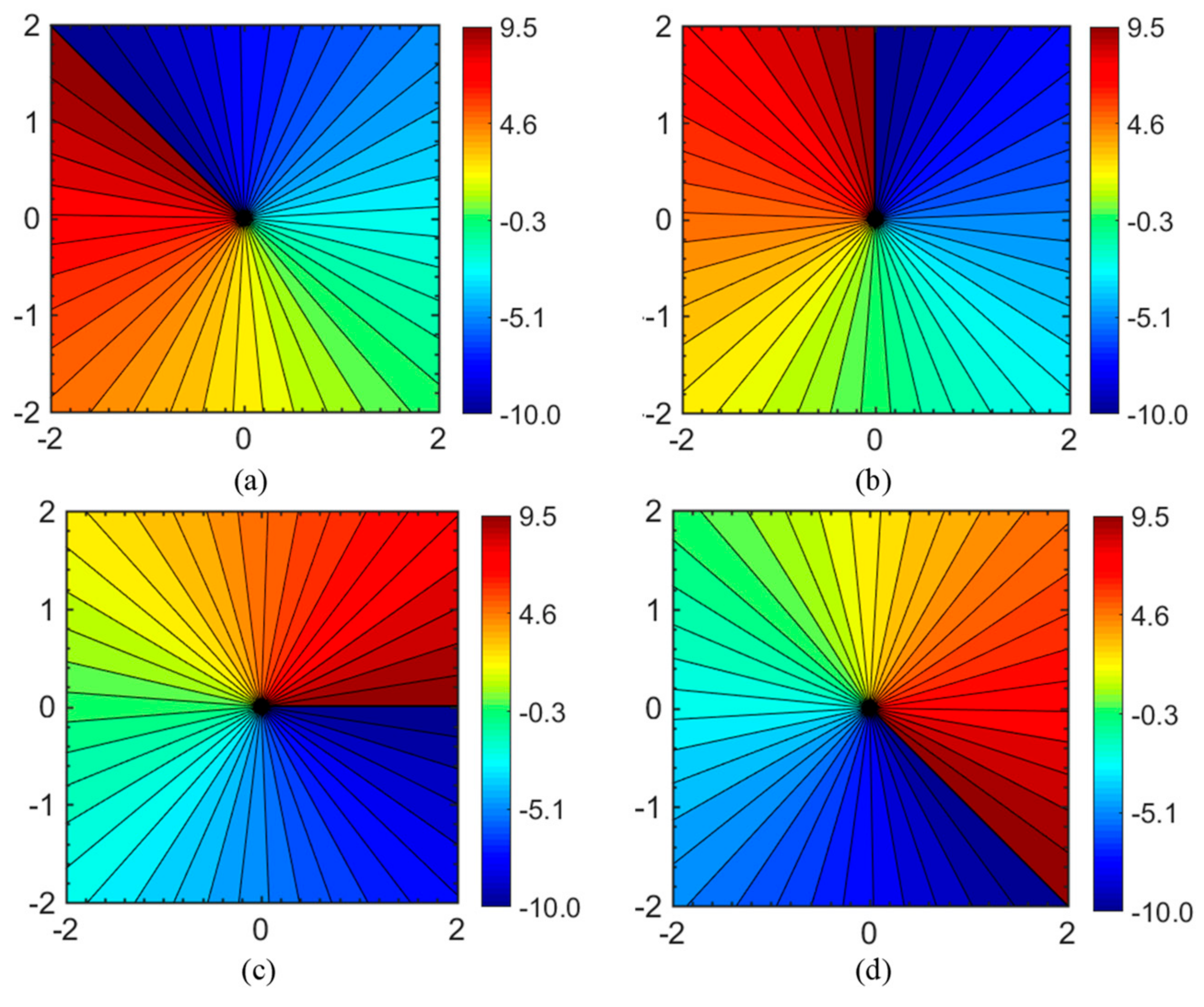
3.1. Branch Cut Issues
3.2. Areal Doublet
3.3. Branch Cut Choices for Areal Doublet
3.4. Areal Dipole
4. Application
4.1. Scaling of Pressure Field Solutions
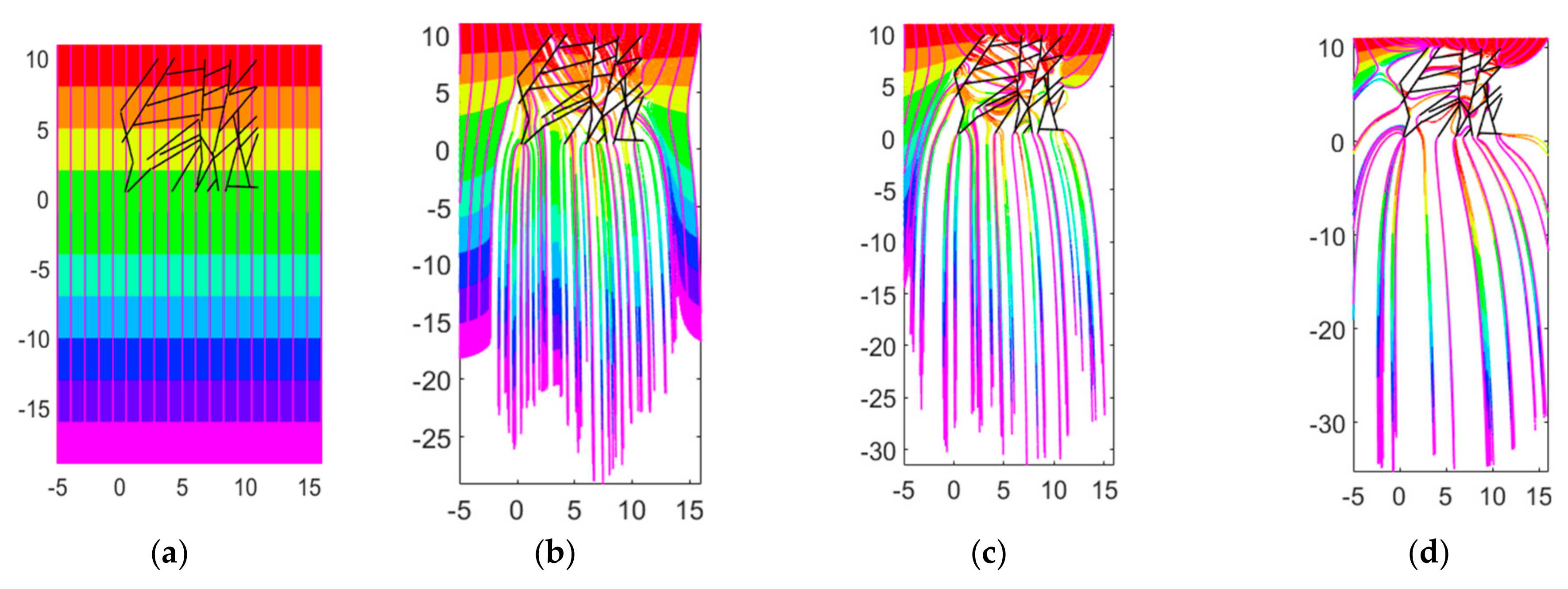
4.2. Particle Tracking and Time-of-Flight Contours
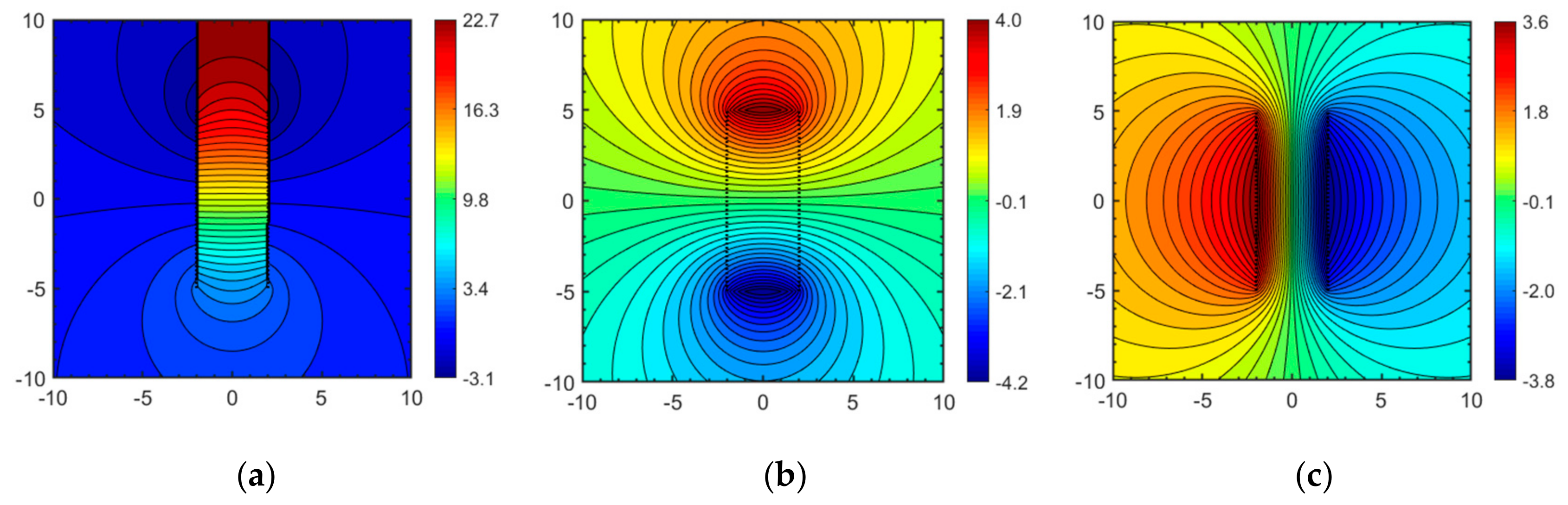
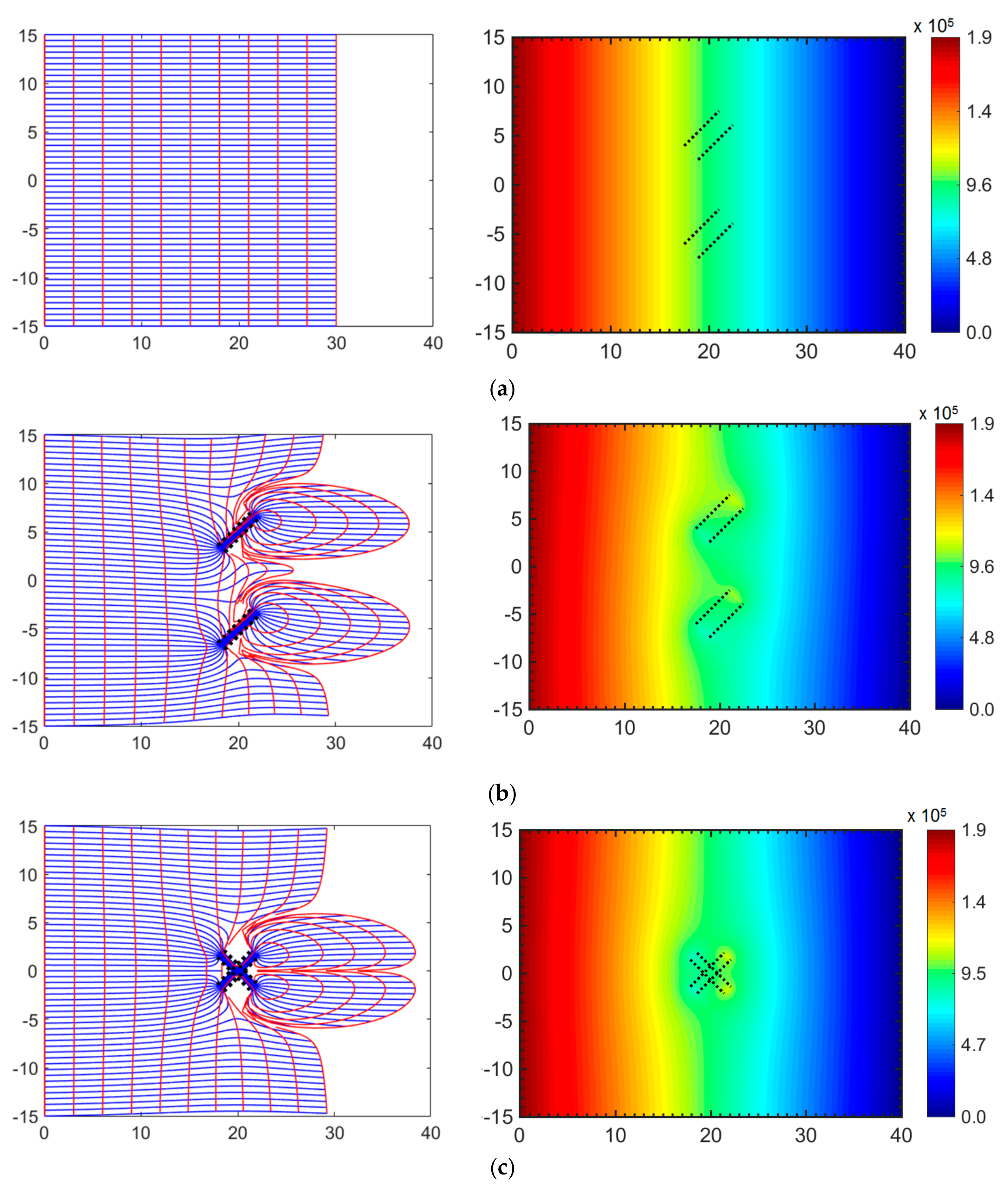
4.3. Synthetic Cases of Wide Flow Channels (Cases A–B)
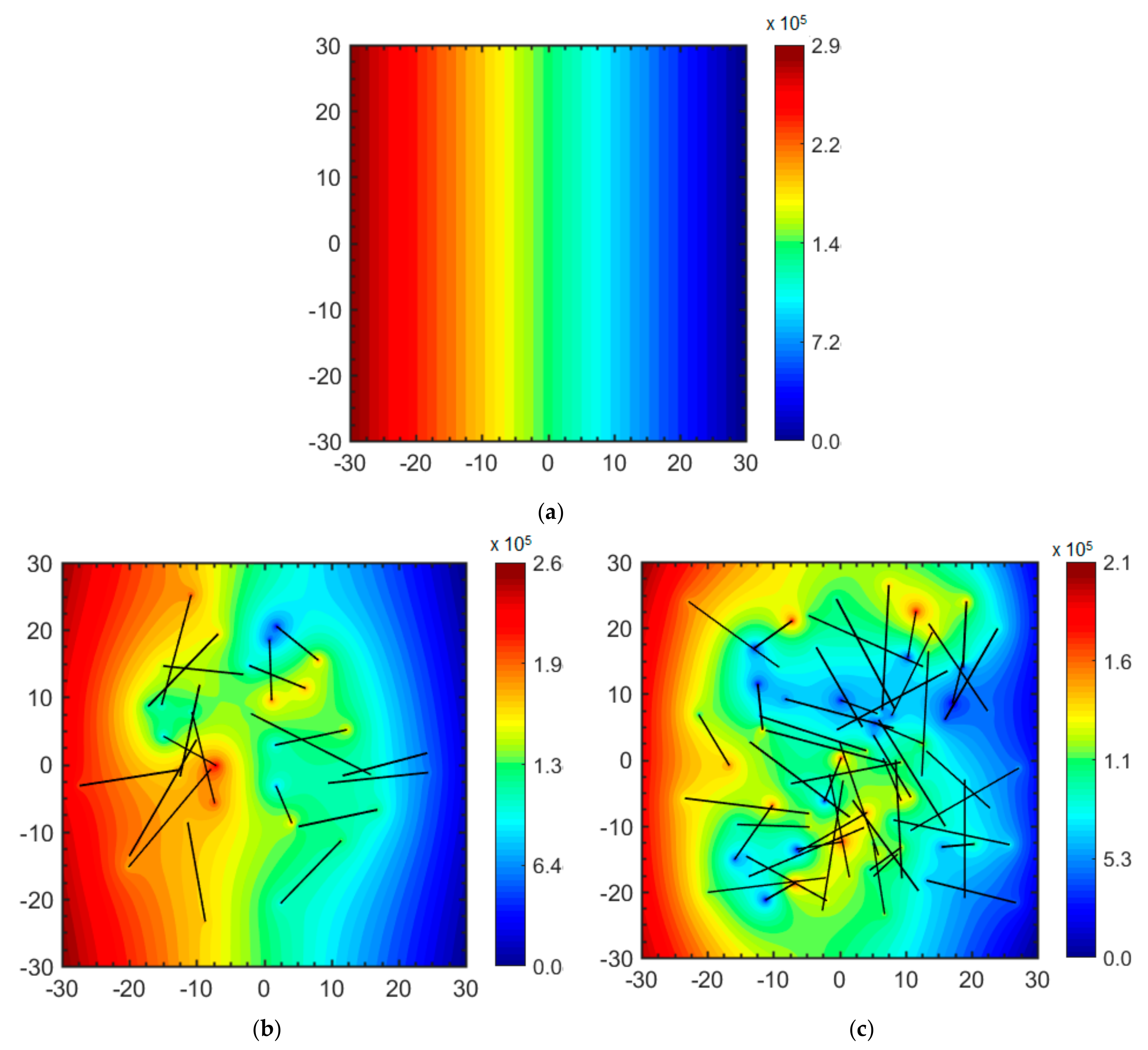
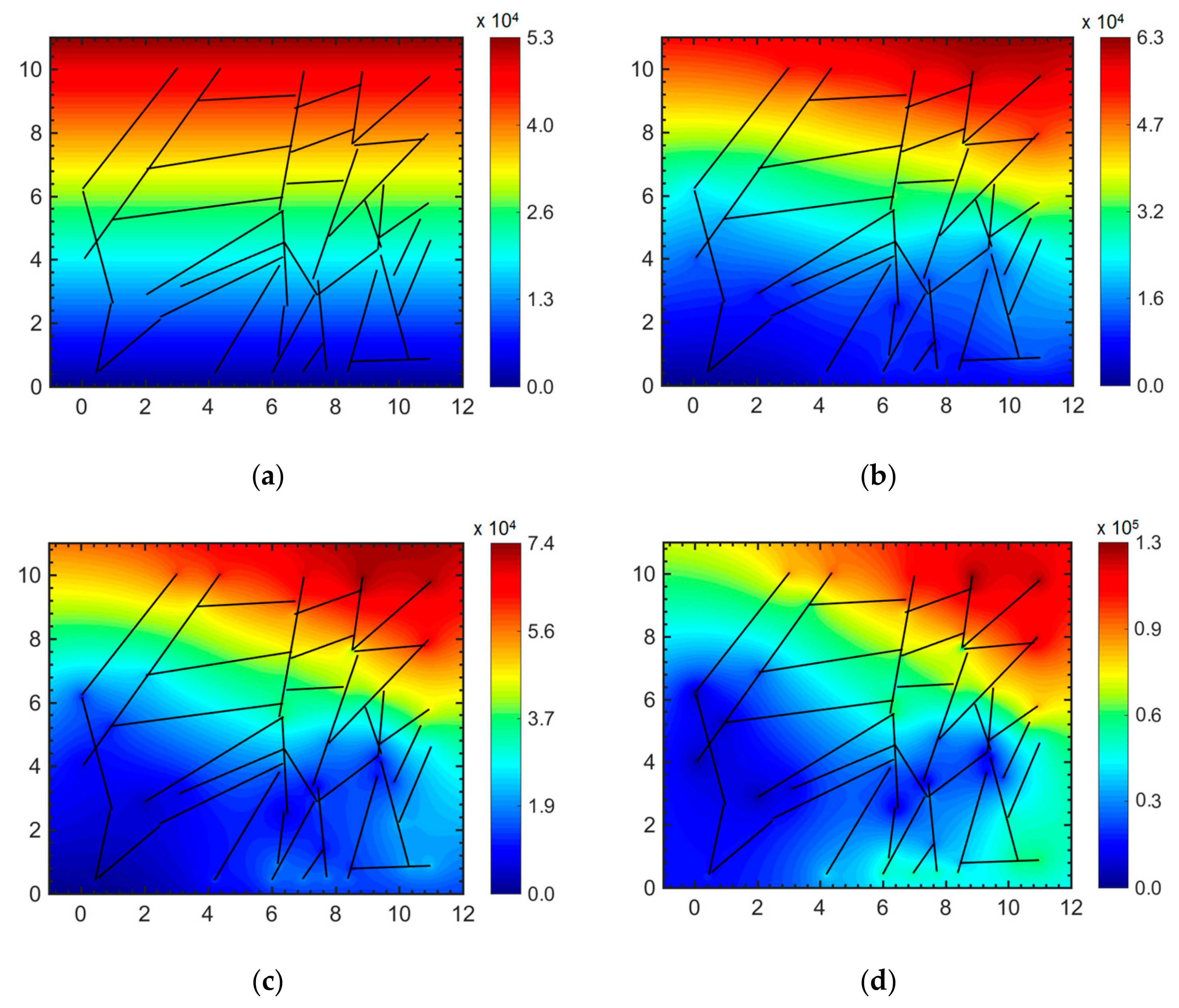
4.4. Effect of Spatial Complexity in the Location of the Flow Channels (Cases C–D)
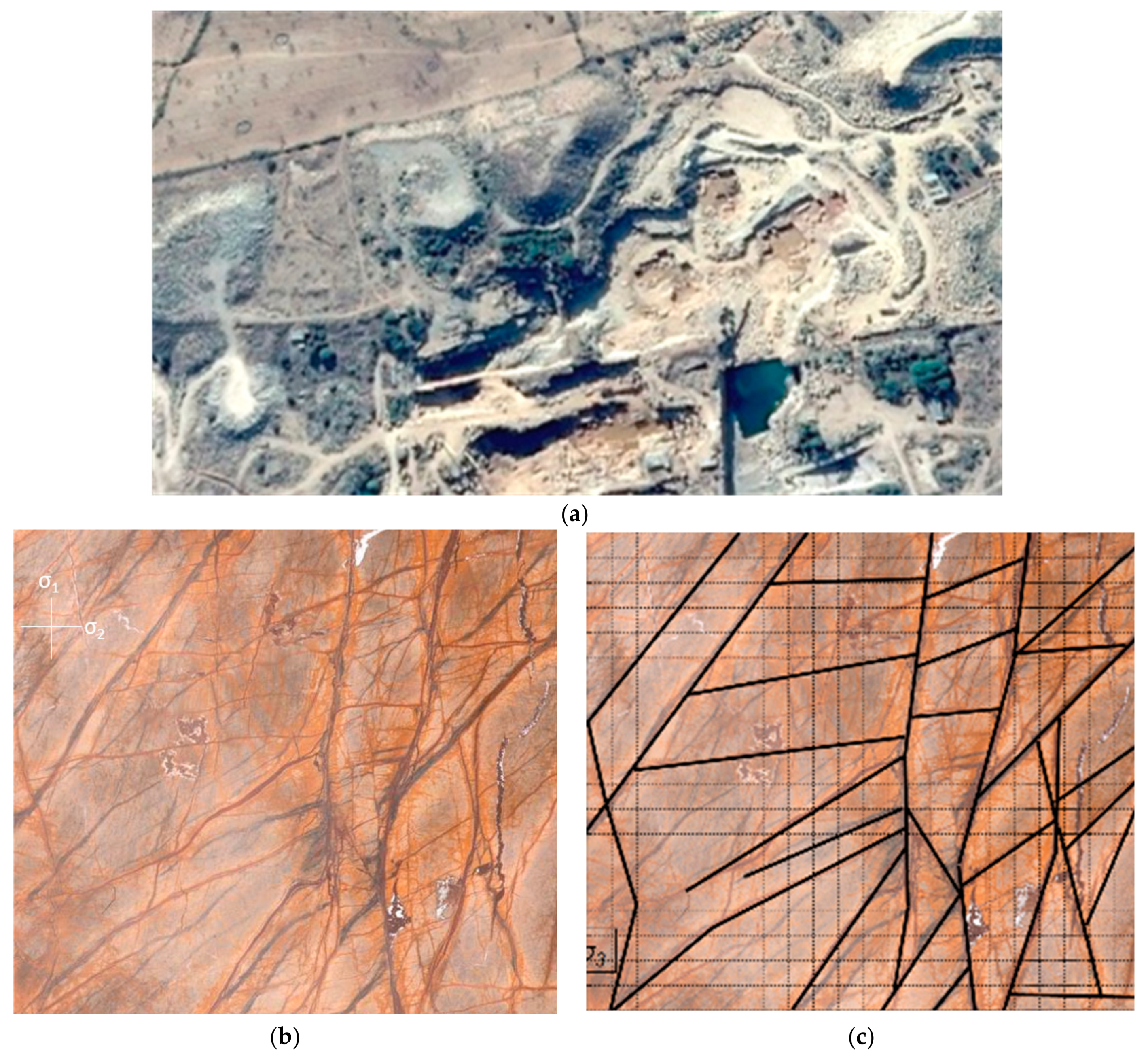
4.5. Field Example from Aravalli Supergroup—Rajasthan (India) (Case E)
5. Discussion
5.1. Variant and Invariant Complex Potentials
5.2. Trade-Offs in Branch Cut Choices
5.3. Applicability and Accuracy of Current Approach
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
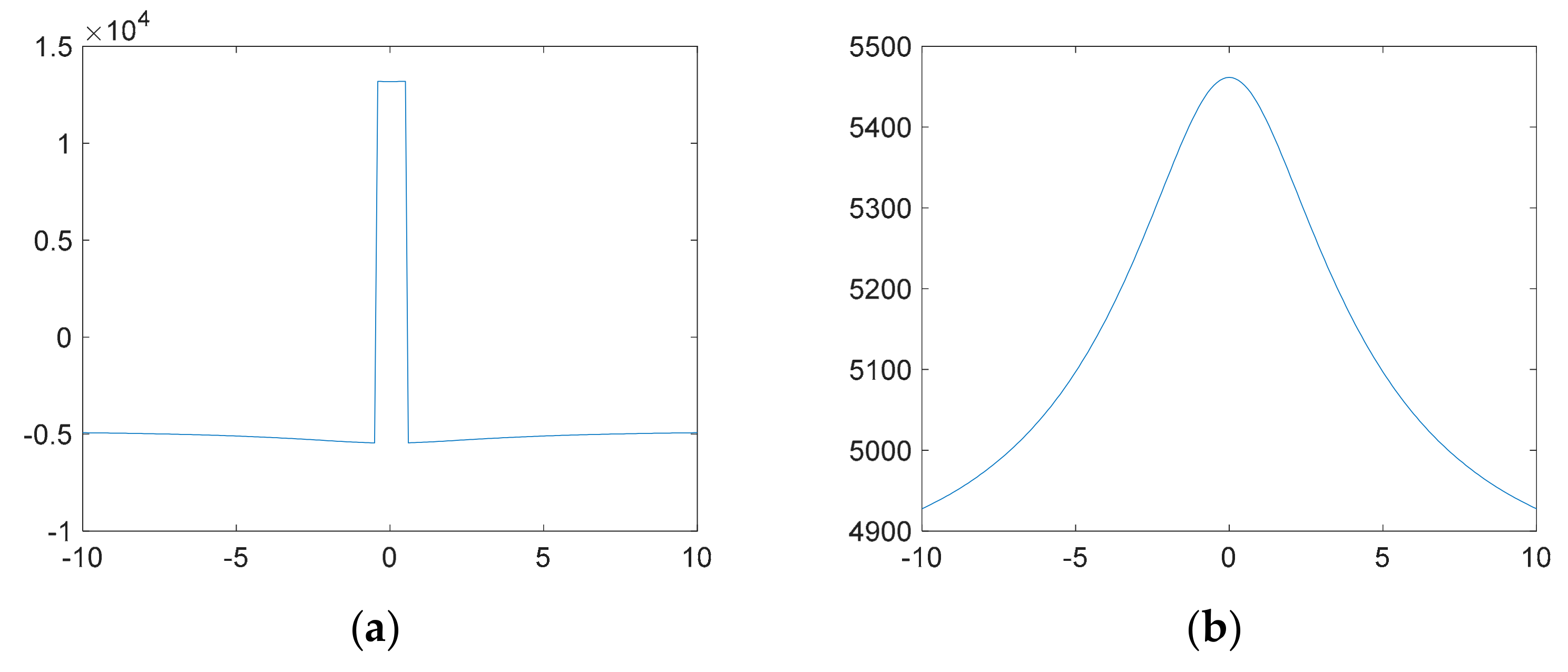
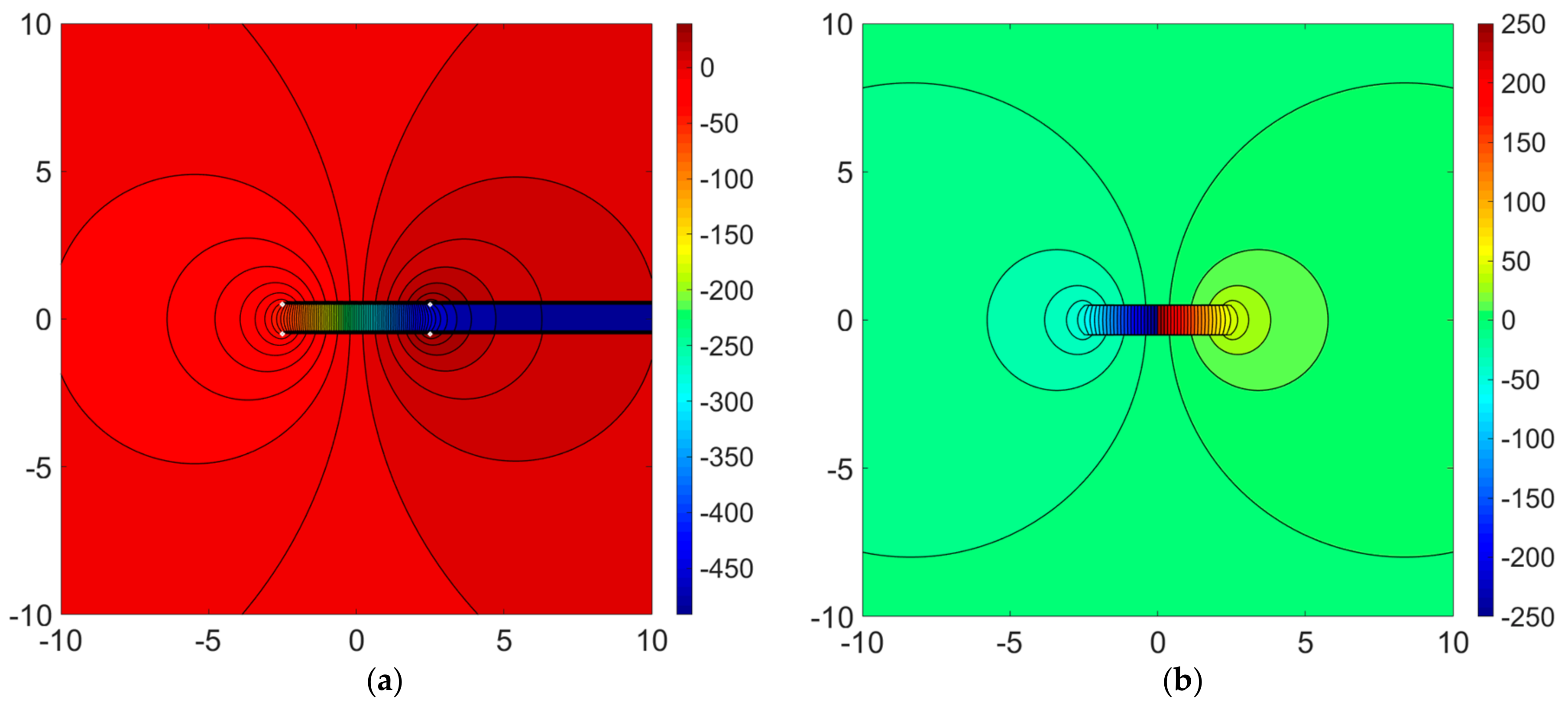
Appendix A. Branch Cuts in Pressure Plots
Appendix A1. Background
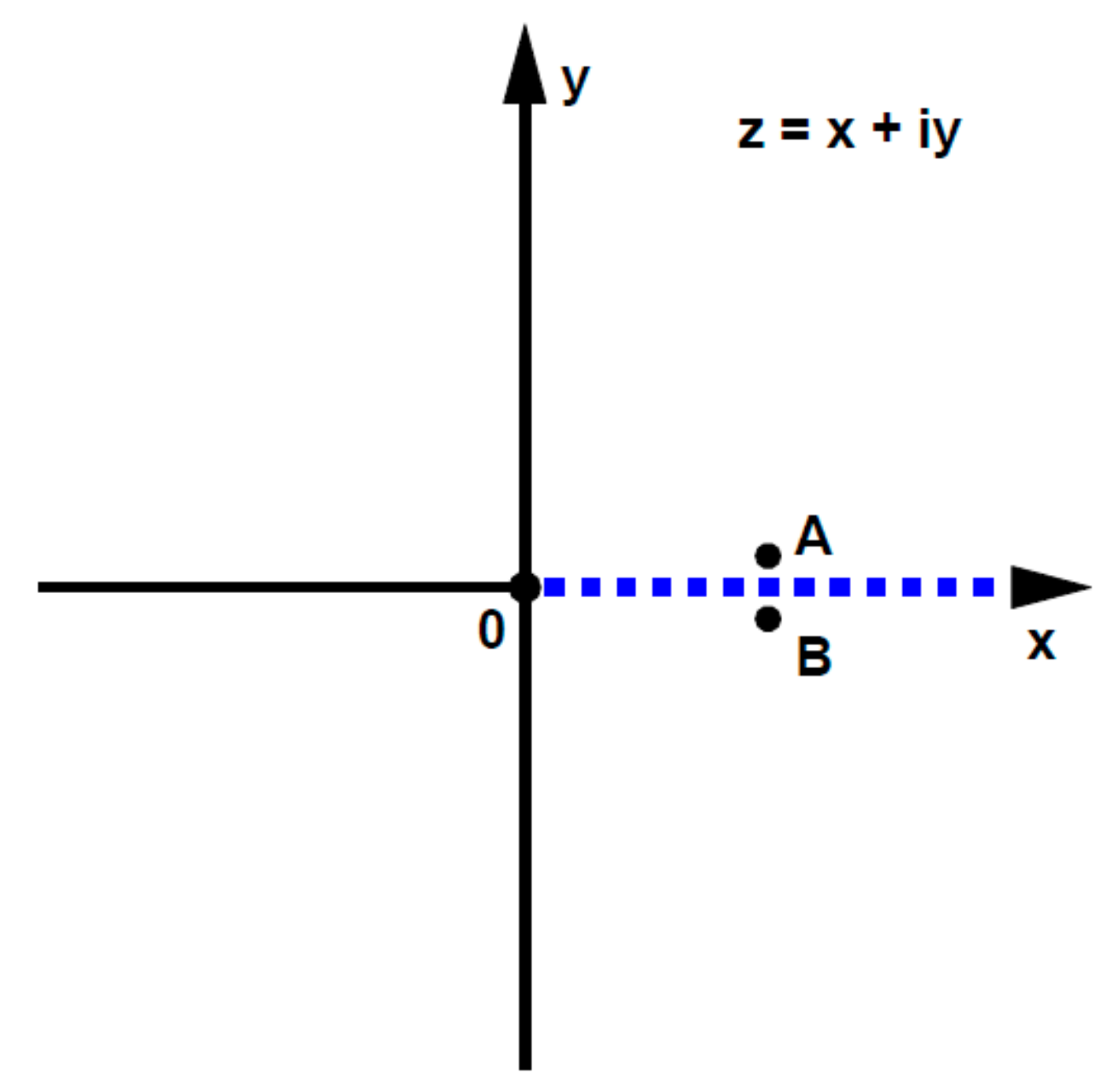
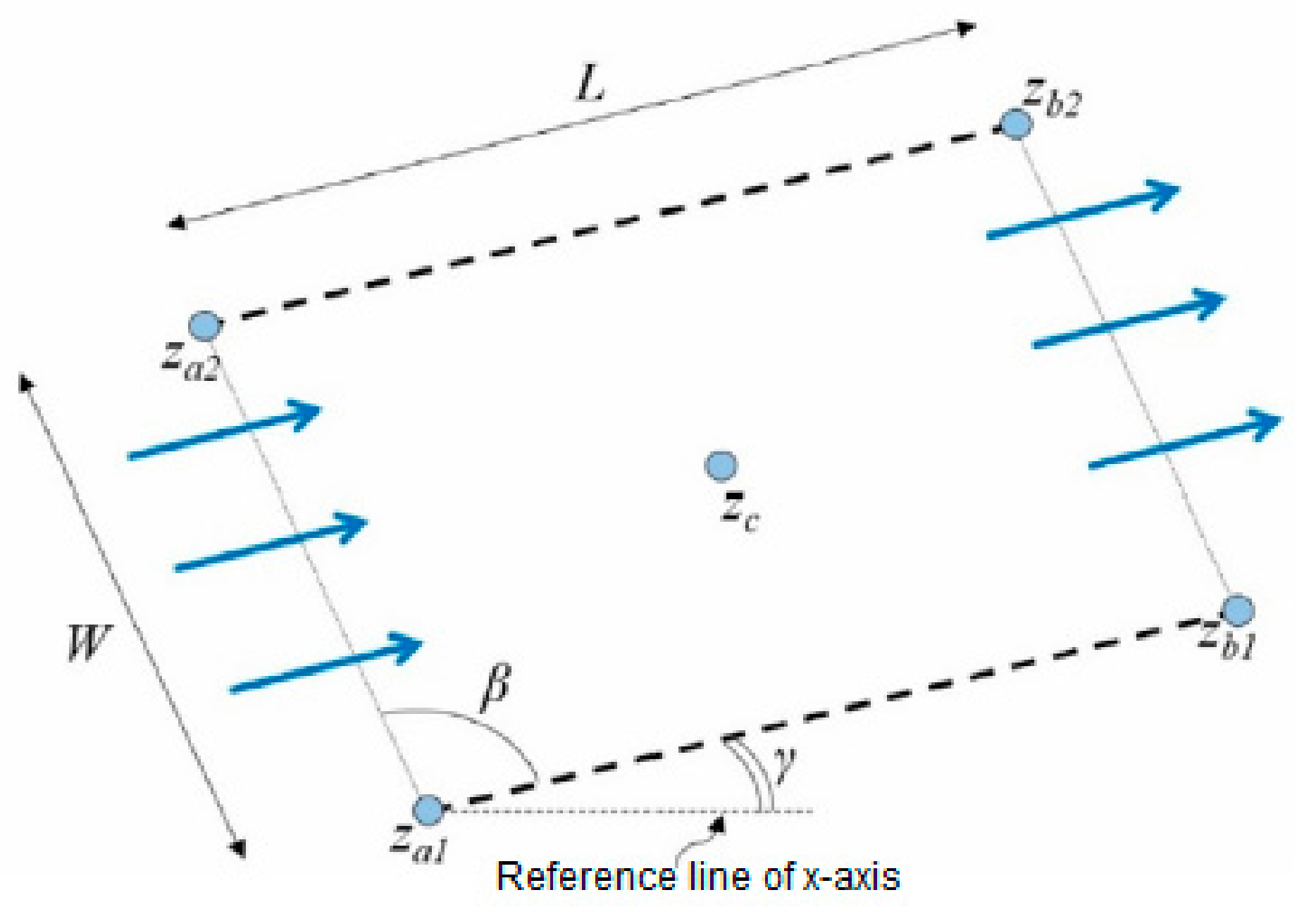
Appendix A2. Demonstration of Branch Cut for a Simple Case
| Quantity | Symbol | Value | Units |
|---|---|---|---|
| Depth | h | 1 | m |
| Porosity | n | 20 | % |
| Permeability | k | 9.87 × 10−16 | m2 |
| Viscosity | µ | 0.01 | Pa.s |
| Far-field velocity | ux | 9.5 × 10−9 | m·s−1 |
| Angle of far-field flow | α | 0 | ° |
| Fracture center | zc | 0 | m |
| Fracture length | L | 5 | m |
| Fracture width | W | 1 | m |
| Angle | γ | 0 | ° |
| Angle between the corner points | β | 90 | ° |
| Strength of fracture | ν | 9.5 × 10−9 | m4·s−1 |

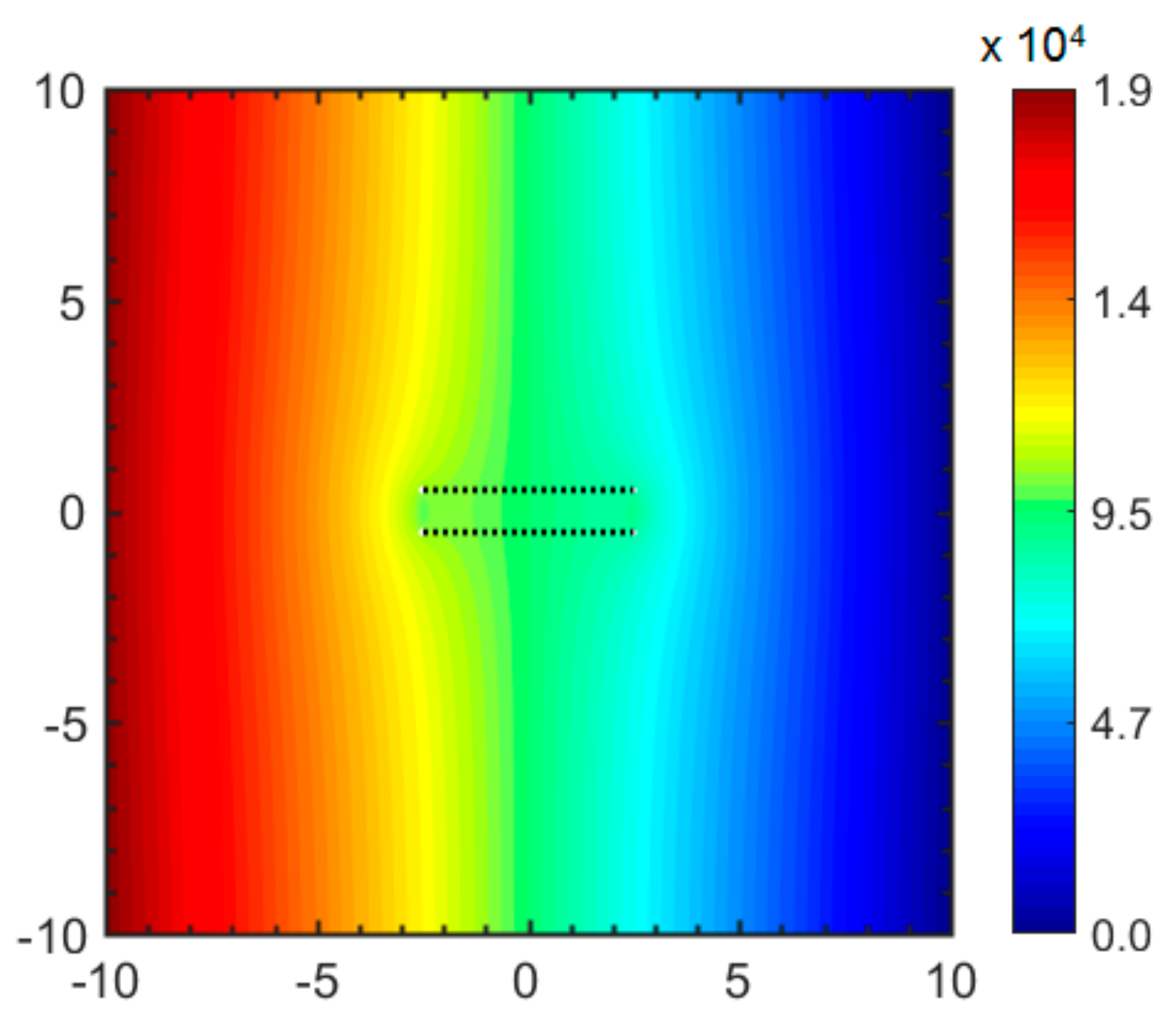
Appendix A3. Proposed Solution to the Branch Cut Placement
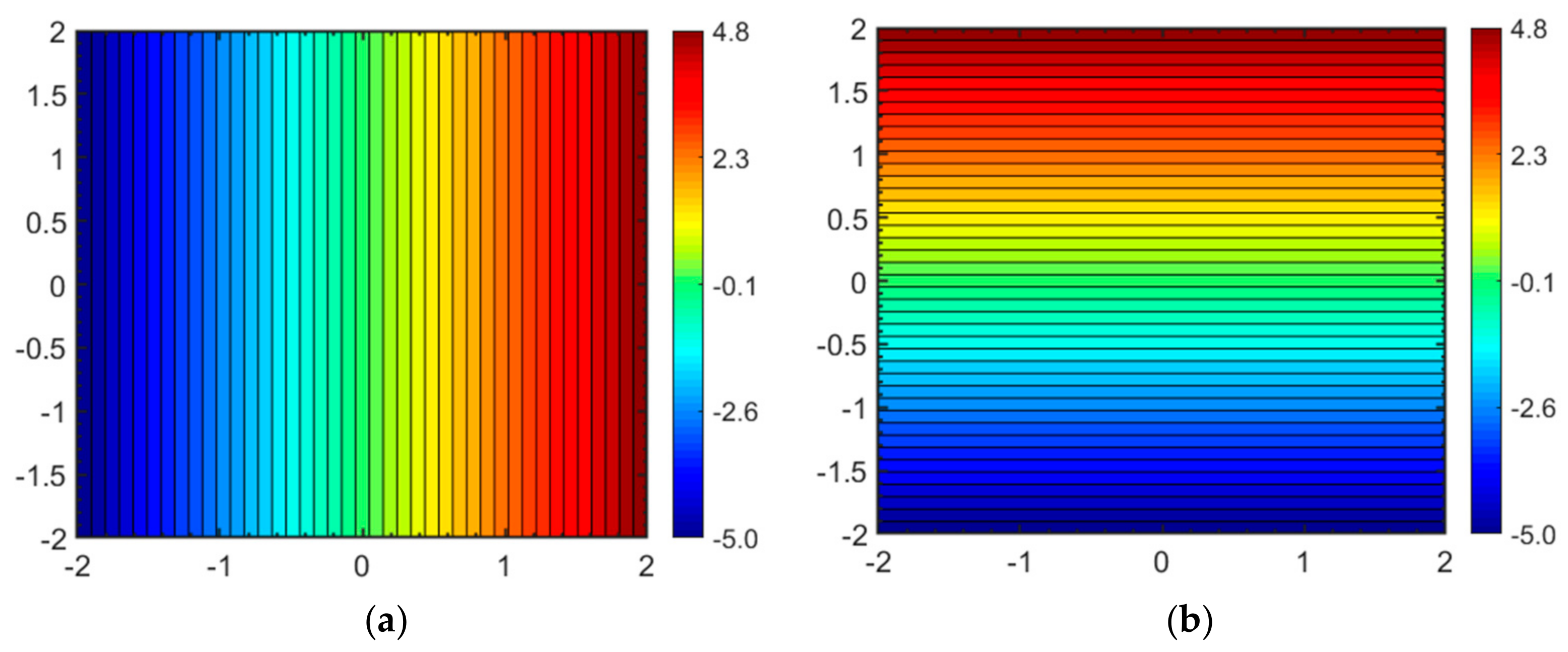
Appendix B. Inputs for Synthetic and Field Cases
| Inputs for Cases A through E | |
|---|---|
| Flow channel permeability (k) | 9.87 × 10−15 m2(10 mD) |
| Matrix Porosity | 0.1 |
| Viscosity (µ) | 0.001 Pa.s (1 cP) |
| Simulation time for Euler’s particle tracking | 6.31 × 108 s (20 years) |
| Time steps for particle tracking (Δt) | 8640 s (0.1 days) |
| Location | Width (m) | Length (m) | Angle (°) | Strength (m4·s−1) | Far-Field Velocity (m·s−1) | |
|---|---|---|---|---|---|---|
| 20 + 5i | 1 | 5 | 45 | 2.38 | ×10−6 | 4.75 × 10−9 |
| 20 − 5i | 1 | 5 | 45 | 2.38 | ×10−6 | |
| Location | Width (m) | Length (m) | Angle (°) | Strength (m4·s−1) | Far-Field Velocity (m·s−1) | |
|---|---|---|---|---|---|---|
| 20 | 1 | 5 | 45 | 2.38 | ×10−6 | 4.75 × 10−9 |
| 20 | 1 | 5 | 315 | 2.38 | ×10−6 | |
| Location | Width (m) | Length (m) | Angle (°) | Strength | (m4·s−1) |
|---|---|---|---|---|---|
| 7 + 3i | 0.043 | 20 | −27 | 3.95 | ×10−7 |
| −15 − 5i | 0.072 | 20 | 60 | 1.81 | ×10−7 |
| 18 + 0i | 0.093 | 13 | 15 | 0.29 | ×10−7 |
| −20 − 2i | 0.099 | 15 | 9 | 3.90 | ×10−7 |
| −1317i | 0.043 | 17 | 75 | 8.22 | ×10−7 |
| 5 + 18i | 0.057 | 8 | −39 | 8.32 | ×10−7 |
| −12 + 14i | 0.096 | 15 | 46 | 7.65 | ×10−7 |
| 7 − 16i | 0.050 | 13 | 46 | 5.23 | ×10−7 |
| 2 + 13i | 0.069 | 9 | −22 | 6.56 | ×10−7 |
| 11 + −8i | 0.098 | 12 | 12 | 6.46 | ×10−7 |
| −9 + 1i | 0.042 | 14 | −76 | 7.75 | ×10−7 |
| −10 − 16i | 0.051 | 15 | −80 | 1.28 | ×10−7 |
| 17 − 2i | 0.063 | 15 | 6 | 1.66 | ×10−7 |
| −14 − 8i | 0.028 | 19 | 50 | 6.70 | ×10−7 |
| −11 + 5i | 0.086 | 14 | 78 | 3.37 | ×10−7 |
| 3 − 6i | 0.032 | 6 | −67 | 5.99 | ×10−7 |
| 7 + 4i | 0.056 | 11 | 12 | 7.89 | ×10−7 |
| −9 + 14i | 0.062 | 12 | −6 | 2.47 | ×10−7 |
| 1 + 14i | 0.039 | 9 | −88 | 8.22 | ×10−7 |
| −11 + 2i | 0.011 | 9 | −29 | 9.03 | ×10−7 |
| Location | Width (m) | Length (m) | Angle (°) | Strength | (m4·s−1) |
|---|---|---|---|---|---|
| −19 + 3i | 0.042 | 9 | −59 | 4.47 | ×10−7 |
| 3 + 8i | 0.025 | 6 | −20 | 4.42 | ×10−7 |
| 20 + 13i | 0.096 | 16 | 60 | 5.80 | ×10−7 |
| −13 − 11i | 0.047 | 10 | 55 | 9.13 | ×10−7 |
| 0 − 10i | 0.011 | 16 | −79 | 2.71 | ×10−7 |
| −4 + 4i | 0.077 | 17 | −18 | 8.89 | ×10−7 |
| 18 − 13i | 0.090 | 5 | 5 | 2.47 | ×10−7 |
| 07i | 0.078 | 17 | −15 | 7.46 | ×10−7 |
| 8 + 9i | 0.071 | 19 | 28 | 2.95 | ×10−7 |
| −5 − 14i | 0.050 | 19 | 23 | 2.61 | ×10−7 |
| −6 − 3i | 0.073 | 19 | −37 | 1.43 | ×10−7 |
| 17 − 11i | 0.036 | 18 | −12 | 9.36 | ×10−7 |
| 9 − 9i | 0.061 | 18 | −87 | 0.62 | ×10−7 |
| 137i | 0.024 | 19 | 87 | 7.13 | ×10−7 |
| 0 + 11i | 0.078 | 14 | −60 | 2.90 | ×10−7 |
| 3 − 6i | 0.026 | 18 | −71 | 6.56 | ×10−7 |
| 9 + 4i | 0.026 | 8 | −23 | 6.13 | ×10−7 |
| 7 − 13i | 0.077 | 17 | −54 | 1.19 | ×10−7 |
| −10 − 10i | 0.034 | 11 | −2 | 4.18 | ×10−7 |
| −8 − 18i | 0.040 | 14 | −29 | 2.71 | ×10−7 |
| −1 − 13i | 0.029 | 20 | 81 | 1.28 | ×10−7 |
| 18 + 12i | 0.032 | 7 | 76 | 5.18 | ×10−7 |
| 6 − 18i | 0.010 | 11 | −81 | 3.18 | ×10−7 |
| 7 − 16i | 0.031 | 5 | 43 | 0.67 | ×10−7 |
| 18 − 3i | 0.021 | 13 | −42 | 0.86 | ×10−7 |
| 20 − 20i | 0.068 | 14 | −14 | 3.90 | ×10−7 |
| −3 − 13i | 0.027 | 7 | 9 | 6.99 | ×10−7 |
| 11 + 19i | 0.013 | 7 | 80 | 8.03 | ×10−7 |
| −2 + 2i | 0.079 | 19 | −15 | 3.90 | ×10−7 |
| 7 + 17i | 0.062 | 19 | 87 | 6.65 | ×10−7 |
| −16 + 19i | 0.010 | 17 | −36 | 4.14 | ×10−7 |
| 7 − 15i | 0.045 | 6 | 36 | 2.09 | ×10−7 |
| −9 − 20i | 0.098 | 5 | 30 | 6.84 | ×10−7 |
| −11 − 19i | 0.014 | 18 | 7 | 3.95 | ×10−7 |
| −10 + 19i | 0.095 | 7 | 36 | 8.75 | ×10−7 |
| 19 − 6i | 0.013 | 19 | 30 | 6.13 | ×10−7 |
| 11 − 2i | 0.063 | 19 | −58 | 3.66 | ×10−7 |
| 8 − 3i | 0.023 | 7 | −67 | 3.42 | ×10−7 |
| 19 − 12i | 0.020 | 18 | 90 | 1.62 | ×10−7 |
| 8 − 1i | 0.057 | 11 | −59 | 5.51 | ×10−7 |
| −12 + 8i | 0.092 | 7 | −84 | 6.61 | ×10−7 |
| 1 − 2i | 0.056 | 17 | 11 | 5.80 | ×10−7 |
| −1 − 3i | 0.044 | 7 | 69 | 8.98 | ×10−7 |
| −1 − 11i | 0.046 | 12 | 30 | 7.22 | ×10−7 |
| 18 + 14i | 0.048 | 16 | −56 | 2.00 | ×10−7 |
| 4 + 18i | 0.039 | 19 | −24 | 5.85 | ×10−7 |
| −14 − 7i | 0.065 | 19 | −7 | 6.70 | ×10−7 |
| 19 + 19i | 0.096 | 10 | 87 | 8.13 | ×10−7 |
| 4 + 16i | 0.092 | 19 | −62 | 4.71 | ×10−7 |
| 11 + 13i | 0.011 | 14 | 64 | 8.22 | ×10−7 |
| Location | Width (m) | Length (m) | Angle (°) | Strength | (m4·s−1) |
|---|---|---|---|---|---|
| 1.53 + 8.13i | 0.01 | 4.84 | 231 | 2.3 | ×10−7 |
| 2.23 + 7.03i | 0.01 | 7.38 | 235 | 3.51 | ×10−7 |
| 0.51 + 4.39i | 0.01 | 3.66 | 285 | 1.74 | ×10−7 |
| 0.69 + 1.52i | 0.01 | 2.26 | 253 | 1.07 | ×10−7 |
| 5.2 + 9.09i | 0.01 | 3.09 | 188 | 1.47 | ×10−7 |
| 4.34 + 7.22i | 0.01 | 4.65 | 195 | 2.21 | ×10−7 |
| 3.65 + 5.61i | 0.01 | 5.45 | 188 | 2.59 | ×10−7 |
| 4.2 + 4.22i | 0.01 | 5.08 | 215 | 2.41 | ×10−7 |
| 4.74 + 3.84i | 0.01 | 3.55 | 204 | 1.69 | ×10−7 |
| 4.42 + 3.14i | 0.01 | 4.32 | 209 | 2.05 | ×10−7 |
| 7.76 + 9.14i | 0.01 | 2.26 | 202 | 1.07 | ×10−7 |
| 7.58 + 7.74i | 0.01 | 2.11 | 202 | 1 | ×10−7 |
| 7.35 + 6.44i | 0.01 | 1.81 | 188 | 0.86 | ×10−7 |
| 6.91 + 3.71i | 0.01 | 1.97 | 305 | 0.94 | ×10−7 |
| 6.67 + 1.66i | 0.01 | 2.81 | 239 | 1.34 | ×10−7 |
| 5.22 + 2.12i | 0.01 | 3.97 | 236 | 1.89 | ×10−7 |
| 6.29 + 1.74i | 0.01 | 1.63 | 258 | 0.77 | ×10−7 |
| 8.39 + 3.6i | 0.01 | 2.32 | 218 | 1.1 | ×10−7 |
| 7.28 + 0.92i | 0.01 | 1.11 | 230 | 0.53 | ×10−7 |
| 8.85 + 2.07i | 0.01 | 3.33 | 254 | 1.58 | ×10−7 |
| 9.73 + 0.83i | 0.01 | 2.51 | 358 | 1.19 | ×10−7 |
| 9.86 + 2.51i | 0.01 | 3.4 | 285 | 1.62 | ×10−7 |
| 10.5 + 3.42i | 0.01 | 2.59 | 248 | 1.23 | ×10−7 |
| 10.26 + 4.38i | 0.01 | 1.98 | 225 | 0.94 | ×10−7 |
| 10.17 + 5.24i | 0.01 | 1.88 | 222 | 0.89 | ×10−7 |
| 9.36 + 6.35i | 0.01 | 4.52 | 227 | 2.15 | ×10−7 |
| 9.77 + 8.72i | 0.01 | 3.22 | 227 | 1.53 | ×10−7 |
| 8.69 + 8.77i | 0.01 | 2.32 | 265 | 1.1 | ×10−7 |
| 9.7 + 7.7i | 0.01 | 2.24 | 195 | 1.06 | ×10−7 |
| 7.58 + 1.93i | 0.01 | 2.85 | 279 | 1.35 | ×10−7 |
| 7.99 + 5.43i | 0.01 | 4.34 | 256 | 2.06 | ×10−7 |
| 6.62 + 7.74i | 0.01 | 4.46 | 267 | 2.12 | ×10−7 |
| 6.41 + 4.04i | 0.01 | 3.03 | 274 | 1.44 | ×10−7 |
| 1.51 + 1.31i | 0.01 | 2.54 | 222 | 1.21 | ×10−7 |
| 9.19 + 5.11i | 0.01 | 1.56 | 282 | 0.74 | ×10−7 |
| 9.43 + 5.34i | 0.01 | 2.06 | 272 | 0.98 | ×10−7 |
Symbols
| Ω | Complex potential |
| Far-field velocity in the x-direction | |
| Far-field velocity in the y-direction | |
| Strength of point sources/sinks | |
| Strength of vortex | |
| µ | Strength of singularity doublet |
| µ* | Strength of singularity dipole |
| m | Strength of line doublet/dipole |
| υ | Strength of the areal doublet |
| η | Viscosity of fluid |
| k | Reservoir permeability |
| Potential Function | |
| Stream Function | |
| Velocity on x-direction | |
| Velocity on y-direction | |
| Time step |
References
- Needham, T. Visual Complex Analysis; Clarendon Press: Oxford, UK, 1997. [Google Scholar]
- Sato, K. Complex Analysis for Practical Engineering; Springer International Publishing: Cham, Switzerland, 2015. [Google Scholar] [CrossRef]
- Van Harmelen, A.; Weijermars, R. Complex analytical solutions for flow in hydraulically fractured hydrocarbon reservoirs with and without natural fractures. Appl. Math. Model. 2018, 56, 137–157. [Google Scholar] [CrossRef]
- Weijermars, R.; Khanal, A. High-Resolution Streamline Models of Flow in Fractured Porous Media using Discrete Fractures: Implications for Upscaling of Permeability Anisotropy. Earth-Sci. Rev. 2019, 194, 399–448. [Google Scholar] [CrossRef]
- Weijermars, R.; Khanal, A. Elementary pore network models based on complex analysis methods (CAM): fundamental insights for shale field development. Energies 2019. [Google Scholar] [CrossRef]
- Khanal, A.; Weijermars, R. Visualization of drained rock volume (DRV) in hydraulically fractured reservoirs with and without natural fractures using complex analysis methods (CAMs). Pet. Sci. 2019, 16, 550. [Google Scholar] [CrossRef]
- Khanal, A.; Weijermars, R. Pressure depletion and drained rock volume near hydraulically fractured parent and child wells. J. Pet. Sci. Eng. 2019, 172, 607–626. [Google Scholar] [CrossRef]
- Khanal, A.; Weijermars, R. Production Interference of Hydraulically Fractured Hydrocarbon Wells: New Tools for Optimization of Productivity and Economic Performance of Parent and Child Wells. In Proceedings of the 2019 SPE Europec featured at 81st EAGE Conference and Exhibition, London, UK, 3–6 June 2019. [Google Scholar]
- Khanal, A.; Weijermars, R. Impact of Natural Fractures on the Shape and Location of Drained Rock Volumes in Unconventional Reservoirs: Case Studies from the Permian Basin. In Proceedings of the 2019 SPE/AAPG/SEG Unconventional Resources Technology Conference, Denver, CO, USA, 22–24 July 2019. [Google Scholar]
- Batycky, R.P.; Blunt, M.J.; Thiele, M.R.; Stanford, U. A 3D Field Scale Streamline Based Reservoir Simulator. Spe Reserv. Eng. 1997. [Google Scholar] [CrossRef]
- Thiele, M.R.; Batycky, R.P.; Blunt, M.J.; Orr, F.M., Jr. Simulating Flow in Heterogeneous Systems Using Streamtubes and Streamlines. Spe Reserv. Eng. 1996. [Google Scholar] [CrossRef]
- Tanaka, S.; Kam, D.; Datta-Gupta, A.; King, M.J. Streamline-Based History Matching of Arrival Times and Bottomhole Pressure Data for Multicomponent Compositional Systems. In Proceedings of the SPE Annual Technical Conference and Exhibition, Houston, TX, USA, 28–30 September 2015; Society of Petroleum Engineers: London, UK, 2015. [Google Scholar] [CrossRef]
- Kahan, W. Branch Cuts for Complex Elementary Functions, or Much Ado about Nothing’s Sign Bit. In The State of the Art in Numerical Analysis, Proceedings of the Joint IMA/SIAM Conference on the State of the Art in Numerical Analysis Held at the UN, Oxford, UK, 1 September 1987; Iserles, A., Powell, M.J.D., Eds.; Clarendon Press: New York, NY, USA, 1987; pp. 165–211. [Google Scholar]
- Weijermars, R.; Van Harmelen, A. Breakdown of doublet recirculation and direct line drives by far-field flow in reservoirs: Implications for geothermal and hydrocarbon well placement. Geophys. J. Int. 2016, 206, 19–47. [Google Scholar] [CrossRef]
- Strack, O.D.L. Groundwater Mechanics; Prentice-Hall: Englewood Cliffs, NJ, USA, 1989. [Google Scholar]
- Weijermars, R.; Van Harmelen, A.; Zuo, L. Flow Interference Between Frac Clusters (Part 2): Field Example from the Midland Basin (Wolfcamp Formation, Spraberry Trend Field) With Implications for Hydraulic Fracture Design. In Proceedings of the SPE/AAPG/SEG Unconventional Resources Technology Conference, Austin, TX, USA, 24–26 July 2017. [Google Scholar] [CrossRef]
- Weijermars, R.; van Harmelen, A.; Zuo, L.; Nascentes, I.A.; Yu, W. High- Resolution Visualization of Flow Interference Between Frac Clusters (Part 1): Model Verification and Basic Cases. In Proceedings of the SPE/AAPG/SEG Unconventional Resources Technology Conference, Austin, TX, USA, 24–26 July 2017. [Google Scholar]
- Zuo, L.; Weijermars, R. Rules for Flight Paths and Time of Flight for Flows in Porous Media with Heterogeneous Permeability and Porosity. Geofluids 2017. [Google Scholar] [CrossRef]
- Pradhan, V.R.; Meert, J.G.; Pandit, M.K.; Kamenov, G.; Mondal, M.d.E.A. Paleomagnetic and geochronological studies of the mafic dyke swarms of Bundelkhand craton, central India: Implications for the tectonic evolution and paleogeographic reconstructions. Precambrian Res. 2012, 198–199, 51–76. [Google Scholar] [CrossRef]
- Kilaru, S.; Goud, B.K.; Rao, V.K. Crustal structure of the western Indian shield: Model based on regional gravity and magnetic data. Geosci. Front. 2013, 4, 717–728. [Google Scholar] [CrossRef]
- McKenzie, N.R.; Hughes, N.C.; Myrow, P.M.; Banerjee, D.M.; Deb, M.; Planavsky, N.J. New age constraints for the Proterozoic Aravalli-Delhi successions of India and their implications. Precambrian Res. 2013, 238, 120–128. [Google Scholar] [CrossRef]
- Mukhopadhyay, S.; Bhattacharya, A.K. Bidasar ophiolite suite in the trans-Aravalli region of Rajasthan: A new discovery of geotectonic significance. Indian J. Geosci. 2009, 63, 345–350. [Google Scholar]
- Sanmiguel Vila, C.; Örlü, R.; Vinuesa, R.; Schlatter, P.; Ianiro, A.; Discetti, S. Adverse-Pressure-Gradient Effects on Turbulent Boundary Layers: Statistics and Flow-field Organization. Flow Turbul. Combust 2017, 99, 589. [Google Scholar] [CrossRef] [PubMed]
- Holzbecher, E. Streamline Visualization of Potential Flow with Branch Cuts, With Applications to Groundwater. J. Flow Vis. Image Process. 2018, 25, 119–144. [Google Scholar] [CrossRef]
- Gale, J.; Laubach, S.; Olson, J.; Eichhubl, P.; Fall, A. Natural Fractures in Shale: A Review and New Observations. Aapg Bull. 2014, 98, 2165–2216. [Google Scholar] [CrossRef]
- Dunn, T.L.; Bernabe, A.; Humphreys, J.; Surdam, R.C. Cements and In-situ Widths of Natural Fractures, Almond Formation, Green River Basin, Wyoming; WGA Field Conference Guide Book. 1995, pp. 255–269. Available online: http://www.searchanddiscovery.com/abstracts/html/1995/rms/abstracts/0917a.htm (accessed on 5 August 2019).
- Rosales, R.R. Branch Points and Branch Cuts; MIT: Cambridge, MA, USA, 1999. [Google Scholar]



| Invariant | Variant |
|---|---|
| Far-field flow | Source/sink flow |
| Singularity dipole/doublet | Vortex |
| Areal doublet | Line doublet |
| Areal dipole | Line dipole |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khanal, A.; Weijermars, R. Modeling Flow and Pressure Fields in Porous Media with High Conductivity Flow Channels and Smart Placement of Branch Cuts for Variant and Invariant Complex Potentials. Fluids 2019, 4, 154. https://doi.org/10.3390/fluids4030154
Khanal A, Weijermars R. Modeling Flow and Pressure Fields in Porous Media with High Conductivity Flow Channels and Smart Placement of Branch Cuts for Variant and Invariant Complex Potentials. Fluids. 2019; 4(3):154. https://doi.org/10.3390/fluids4030154
Chicago/Turabian StyleKhanal, Aadi, and Ruud Weijermars. 2019. "Modeling Flow and Pressure Fields in Porous Media with High Conductivity Flow Channels and Smart Placement of Branch Cuts for Variant and Invariant Complex Potentials" Fluids 4, no. 3: 154. https://doi.org/10.3390/fluids4030154
APA StyleKhanal, A., & Weijermars, R. (2019). Modeling Flow and Pressure Fields in Porous Media with High Conductivity Flow Channels and Smart Placement of Branch Cuts for Variant and Invariant Complex Potentials. Fluids, 4(3), 154. https://doi.org/10.3390/fluids4030154