1. Introduction
Vortex shedding is the oscillating flow pattern that can be observed when a fluid flows past a body, and is characterized by a regular pattern of staggered vortices (so-called
vortex street) that form and periodically detach at the back of the body. Vortex shedding is rather ubiquitous in practical applications, and can, for example, be observed with suspension cables, transmission lines, chimneys and slender structures in wind; risers and mooring cables in marine applications; bridge piers; tubes and rods in fluid machinery; aircraft and missiles under maneuvering conditions; and vegetation in wind or water flow. As is well known, the periodic shedding of vortices induces a periodic fluctuation of the fluid forces acting on the body, which in turn can trigger vortex-induced vibration if the body is flexible or otherwise free to oscillate. The practical relevance of vortex shedding and vortex-induced vibration for so many applications has motivated extensive investigation in the last decades, as synthetized for example in [
1,
2,
3]. Vortex shedding is a rather involved phenomenon that depends on a multitude of factors, including the body cross sectional shape, size, aspect ratio and surface roughness; the orientation of the body with respect to the incoming flow; density and viscosity of the fluid; free-stream flow velocity and turbulence intensity. Additional influencing parameters include the speed of sound for non-subsonic flows; end-effects such as the junction between the body and a wall or other surfaces, or free-ends and tips; and structural vibrations or body movement when present. Transverse vibrations with frequency close to the vortex shedding frequency, in particular, can have a profound effect on vortex shedding, and can cause the synchronization (so-called lock-in) between the structural vibration and the vortex shedding.
Vortex street wakes tend to be rather similar regardless of the geometry of the body [
1]. As a consequence, the majority of the vortex shedding investigations documented to date has been carried out using circular cross section cylinders, which in most studies, have been oriented with the axis normal to the incoming flow. As a matter of fact, the circular cylinder in cross-flow has become a paradigmatic flow problem for the study of vortex shedding and vortex-induced vibrations, and one of the most extensively investigated problems in fluid mechanics to date. At a practical level, the most important parameter that characterizes vortex shedding is the vortex shedding frequency
. In the case of a smooth and stationary circular cylinder exposed to a subsonic cross-flow with mild turbulence intensity (usually within 1–2%), the vortex shedding frequency essentially depends on the fluid density
and viscosity
, on the free-stream flow velocity
, and on the cylinder diameter
[
1]. The dimensionless groups that correspondingly characterize vortex shedding in this configuration are the Strouhal number
and the Reynolds number
:
the Strouhal number scales the vortex shedding frequency with the free-stream flow velocity and cylinder diameter, and is a function of the Reynolds number that characterizes the flow. End-effects are normally present near the junction of the cylinder with walls or other surfaces, or near end tips. However, these are normally rather confined and do not extend for more than a few diameters along the cylinder.
Despite the fact that actual slender structures are frequently not in cross-flow, but otherwise inclined with respect to the free-stream, most of the available literature on vortex shedding and vortex-induced vibration is concerned with circular cylinders in cross-flow, and comparatively fewer studies have investigated the effect of the cylinder inclination [
4,
5,
6,
7,
8,
9,
10,
11,
12,
13,
14,
15,
16,
17,
18,
19]. Recently, studies of inclined cylinders in flow at relatively low Reynolds numbers (
< 1000) have experienced renewed interest in connection with flow sensing [
20,
21], flow control [
22,
23,
24], and energy harvesting [
25,
26] applications involving slender flexible structures that reconfigure or bend due to fluid loads. The cylinder inclination angle
is normally defined as the angle between the incoming flow direction and the cylinder axis, so that
= 90° corresponds to normal incidence and therefore to cross-flow, whilst when
= 0° the cylinder axis is aligned with the free-stream velocity and the flow is purely axial. This convention will be followed here. As previously noted, the vortex shedding flow physics for stationary circular cylinders in cross-flow has been extensively investigated and is currently rather well characterized and understood. At the other extreme, the flow along a stationary circular cylinder aligned with the free-stream is purely axial, and is therefore controlled by the development of the boundary layer along the cylinder. This, in turn, depends on the Reynolds number and on the cylinder length and aspect ratio, which together control the growth of the boundary layer along the cylinder. In addition, as commonly happens with boundary layer flows, the purely axial-flow strongly depends on the upstream boundary condition, whose effect is not localized but rather transported along the cylinder by the axial flow itself. In the more general case of a stationary circular cylinder with arbitrary inclination with respect to the incoming flow, the flow field can be regarded as intermediate between the two asymptotic limiting cases of pure cross-flow and pure axial-flow, and therefore combines elements of both. Notably, the flow now includes an axial-flow component resembling a boundary layer flow that gradually develops along the cylinder. It follows that vortex shedding from a stationary, arbitrarily inclined circular cylinder cannot be simply characterized in terms of Strouhal and Reynolds number values, as happens in the cross-flow configuration. Vortex shedding is now also affected by the inclination of the cylinder, which determines the relative strength of the axial flow component. It is also affected by the cylinder aspect ratio, which controls the growth of the boundary layer axial-flow along the cylinder, and by end-conditions and particularly, by the upstream boundary condition. This latter, in fact, tends to affect the flow globally, and not locally as happens in the cross-flow case, because of the axial-flow component which transports any upstream flow disturbance downstream along the cylinder. To complicate things further, the available data indicate that the effects of the Reynolds number, cylinder inclination, aspect ratio, and end- conditions on vortex shedding are quite tightly coupled, and therefore difficult to separate. Moreover, the dependence on the upstream boundary condition tends to make experimental evidence to some extent problem-specific, and the corresponding observations difficult to generalize. Notwithstanding the investigations carried out to date, therefore, the fundamental understanding of vortex shedding from stationary inclined circular cylinders is far less satisfactory than what has been achieved with the cross-flow configuration.
In an early attempt to achieve a better control of the upstream boundary condition, Ramberg [
7] proposed the bent cylinder as a potentially superior configuration to investigate vortex shedding from stationary inclined circular cylinders. Specifically, several experiments were performed with inclined cylinders, covering Reynolds numbers in the range of 160–1100, yaw angles from −10° to 60° (note that the terms inclination angle and yaw angle will be used interchangeably), aspect ratios from 20 to 100, and a variety of cylinder end-conditions with and without endplates to control the flow. The results indicated a strong dependence of vortex shedding upon the cylinder end-conditions, which controlled the three-dimensional flow features in the wake. This was an effect that was apparently not sufficiently recognized in previous research. With a view at better controlling end-effects, a series of tests were carried out using a V-shaped bent cylinder with an aperture of 120° between the two legs, each 44 diameters long. Having no upstream end, Ramberg [
7] argued that the bent cylinder would be less affected by end-conditions, and could therefore help better clarifying the flow physics of vortex shedding behind inclined cylinders. In particular, the vortex shedding frequency behind one leg of the bent cylinder (about midway along the leg) at two Reynolds number values (160 and 460) and inclinations with respect to the free-stream variable between 90° and 30° were measured. Even though these tests with the bent cylinder were rather limited in scope (only 26 data points in total), the measurements were consistent (to within 15%) with cross-flow vortex shedding, surprisingly indicating that the vortex shedding frequency was not affected by the inclination of the bent cylinder with respect to the flow. The bulk of the literature on vortex shedding from stationary inclined circular cylinders generally indicates a reduction in vortex shedding frequency due to the cylinder inclination [
1,
2]. The results provided by Ramberg [
7], therefore, stand out and suggest that bent cylinders might indeed be a configuration worth investigating further to better clarify the flow physics of vortex shedding from stationary inclined circular cylinders, with a view at better controlling the influence of the upstream boundary condition. To the best of our knowledge, however, bent cylinders have not received any further attention, and this motivated the present study. The main objective of the present work was to experimentally investigate, via hot-wire anemometry, the vortex shedding frequency behind bent cylinders, with a view at corroborating the findings of Ramberg [
7] and extend the available vortex shedding frequency databank on bent cylinders to wider Reynolds numbers and yaw angle ranges. In particular, this study tested a bent cylinder comprising two legs, but with different apertures between the legs, that is, a configuration that yields a better control of the upstream boundary condition with respect to the bent cylinder design adopted by Ramberg [
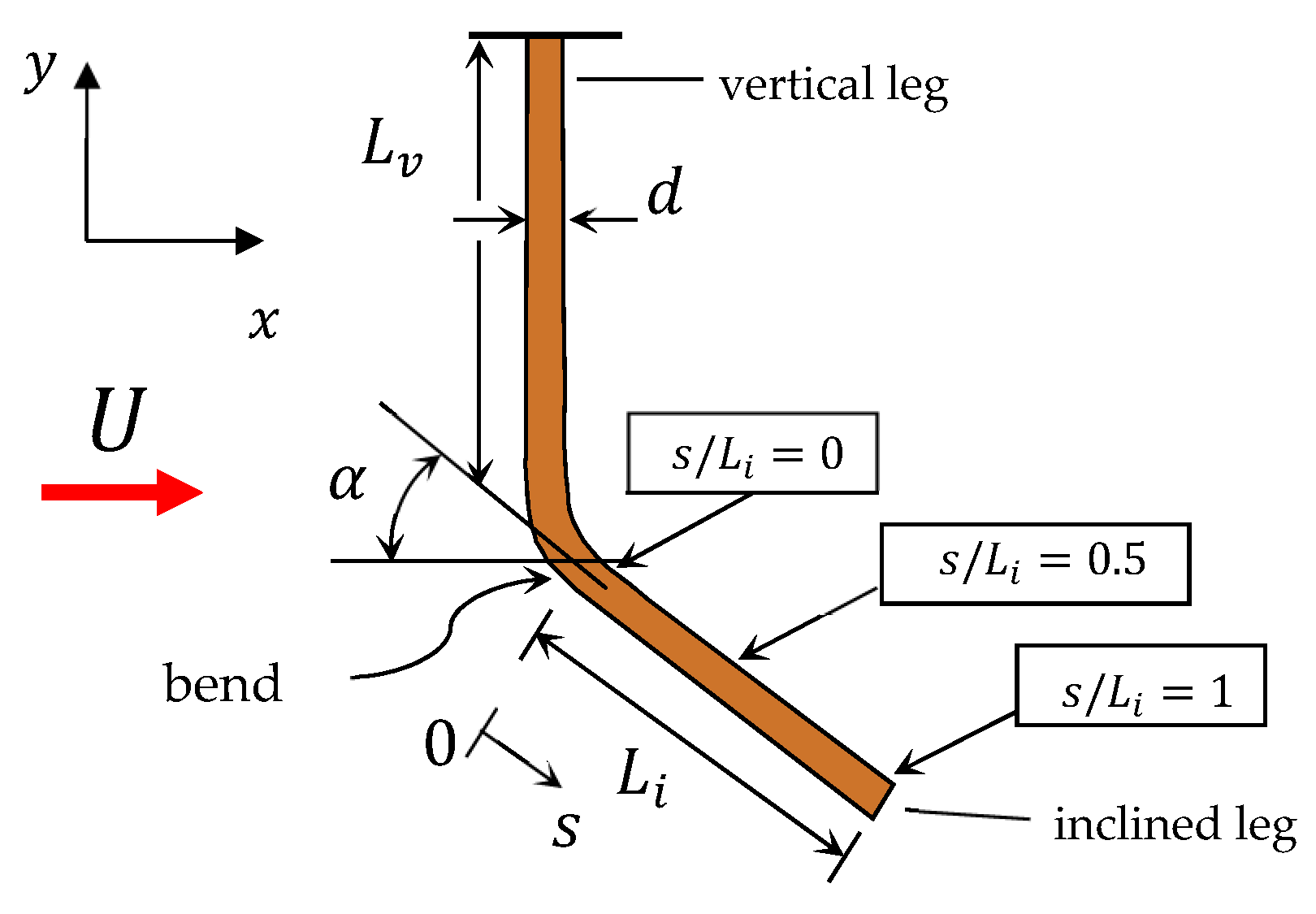
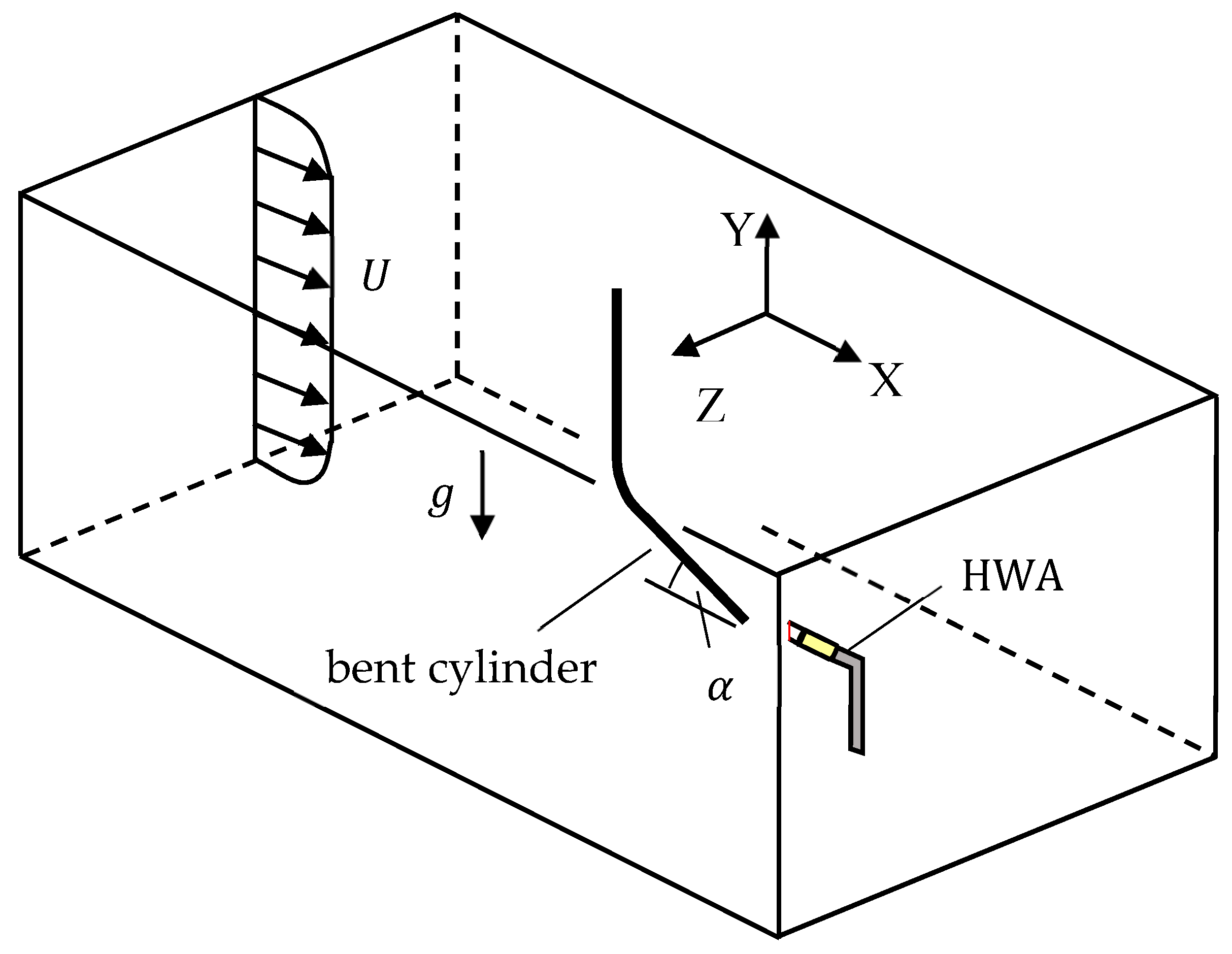
7]. As schematically shown in
Figure 1 and
Figure 2, and better discussed later on, in our tests the bent cylinder was placed in a vertical plane in the middle of a wind tunnel, with one leg vertically oriented and rigidly connected to the ceiling of the wind tunnel. The vertical leg of the bent cylinder was therefore always at normal incidence with respect to the incoming flow. The inclined leg, on the other hand, was yawed with respect to the incoming flow and was positioned in the middle of the wind tunnel downstream of the vertical leg. In this way, the upstream boundary condition acting upon the inclined leg was determined by the vertical leg, and corresponded to the well-known vortex shedding from a stationary circular cylinder in cross-flow. The influence of inclination on the vortex shedding frequency was then explored by systematically varying the yaw angle of the inclined leg.
The rest of this paper is organized as follows. The experimental setup and the experimental methodology are presented in
Section 2; whilst the measurement results are presented and discussed in
Section 3.
3. Results and Discussion
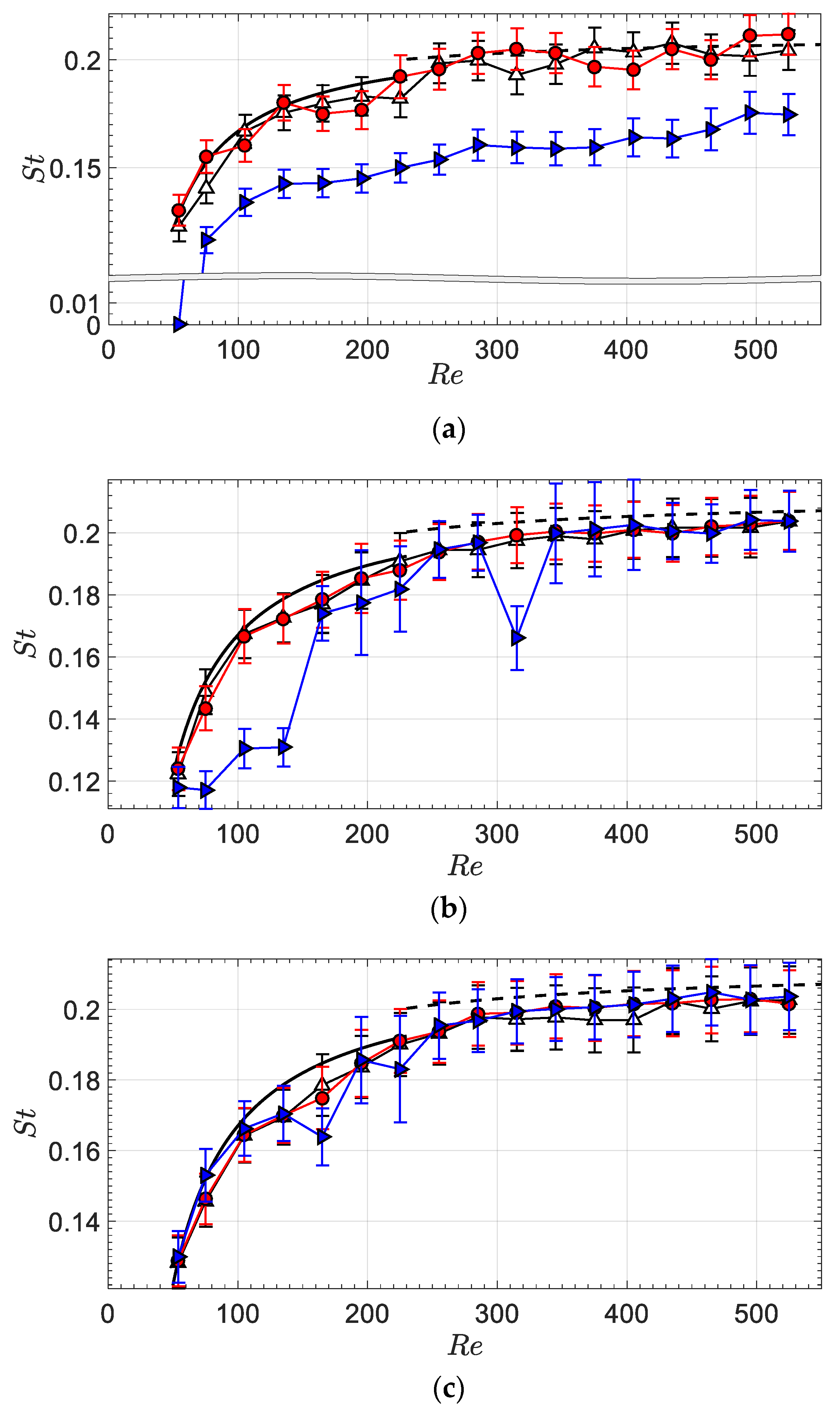
Representative power spectra measured at two Reynolds number values (75 and 285) and three inclinations (90°, 45°, and 15°) are provided in
Figure 6, and include the measurements taken at the three locations along the bent cylinder inclined leg (
). Besides measuring the vortex shedding frequency behind the inclined leg of the bent cylinder, for the sake of verifying the stability and reproducibility of the experimental setup, one additional vortex shedding frequency was measured behind the vertical leg of the bent cylinder. This was always oriented in cross-flow with respect to the flow (this additional measurement was taken midway through the vertical leg, i.e., 30 mm above the bent cylinder bend). This additional measurement is included in the power spectra in
Figure 6 and labeled for convenience
. All raw measurements are provided as
Supplementary Material.
Following common practice for better visual analysis, the power spectra in
Figure 6 have been normalized with respect to the maximum power of the signal. The blue-color vertical plane that can be noticed in the power spectra corresponds to the cross-flow vortex shedding frequency predicted with Equations (3) and (4), and has been included for a qualitative visual comparison.
It is evident, from inspecting
Figure 6, that the power spectral peaks measured at the lower Reynolds number (
= 75) are very narrow, whereas the peaks measured at the higher Reynolds number (
= 285) are broader. On the basis of the previously commented validation results presented in
Figure 5a, in the present experimental setup the laminar to turbulent flow transition in the vortex street begins at a Reynolds number of about
= 230. The vortex street can therefore be expected to be laminar at
= 75 and turbulent at
= 285, so that the broadening of the power spectral peaks observed at the higher Reynolds number in
Figure 6 can be regarded as the consequence of the turbulence in the vortex street that somewhat degrades the coherence of the vortex shedding [
10].
In all cases included in
Figure 6, the measurements taken behind the vertical leg (
) yield a power spectral peak that is very close to the blue plane, thus indicating vortex shedding at a frequency that compares well (i.e., within a few percent) with cross-flow expectations. This provides further confidence in the presented results.
Surprisingly, the measurements included in
Figure 6 taken behind the inclined leg of the bent cylinder yield a power spectral peak that, in most cases, is very close to the blue plane. This indicates that, for the conditions covered in
Figure 6, the inclined leg of the bent cylinder is most of the times, shedding vortices at a frequency that is very close (i.e., within a few percent) to the cross-flow frequency for that particular flow velocity. The only exceptions are firstly, a reduction in vortex shedding frequency (of approximately 20% with respect to the corresponding cross-flow frequency) at the free-end of the inclined leg (
) for both Reynolds number values for the 90° inclination, which can be regarded as a free-end effect. Secondly, a suppression of vortex shedding at the free-end of the inclined leg (
) at the lower Reynolds number (75) for the 15° inclination, which again can be regarded as a free-end effect (as a matter of fact, a small peak near the blue plane is still noticeable in the power spectrum, though most of the energy is concentrated at lower frequency). The additional, smaller peak that can be noticed at
for the lower Reynolds number (75) and 45° inclination is a harmonic of the dominant frequency, because its frequency is twice the dominant frequency.
For the conditions covered in
Figure 6, therefore, the frequency of vortex shedding behind the inclined leg is for the most part not affected by the yaw angle, and the systematic reduction in shedding frequency normally associated in the literature with inclination effects is therefore not present. The results included in
Figure 6 are only a representative subset of the present measurements, so that it is not yet possible to draw any definite conclusions. Analyzing the present databank in terms of power spectra for all Reynolds number values tested (17 values in the range of 50–500) and all inclinations would be impractical. Therefore, the measurements are presented in a condensed and more useful format. The measurements taken at each location along the inclined leg are first presented in contour maps that provide the frequency ratio (i.e., the measured vortex shedding frequency normalized to the corresponding cross-flow vortex shedding frequency, this latter predicted from Equations (3) and (4)), displayed versus the Reynolds number and the inclination. It is noted that a frequency ratio value close to 1 indicates that the measured frequency of vortex shedding is very close to the corresponding cross-flow shedding frequency for that particular flow velocity. One contour map is provided, in
Figure 7, for each location along the inclined leg of the bent cylinder (
). In addition, the data are also provided, in
Figure 8 and
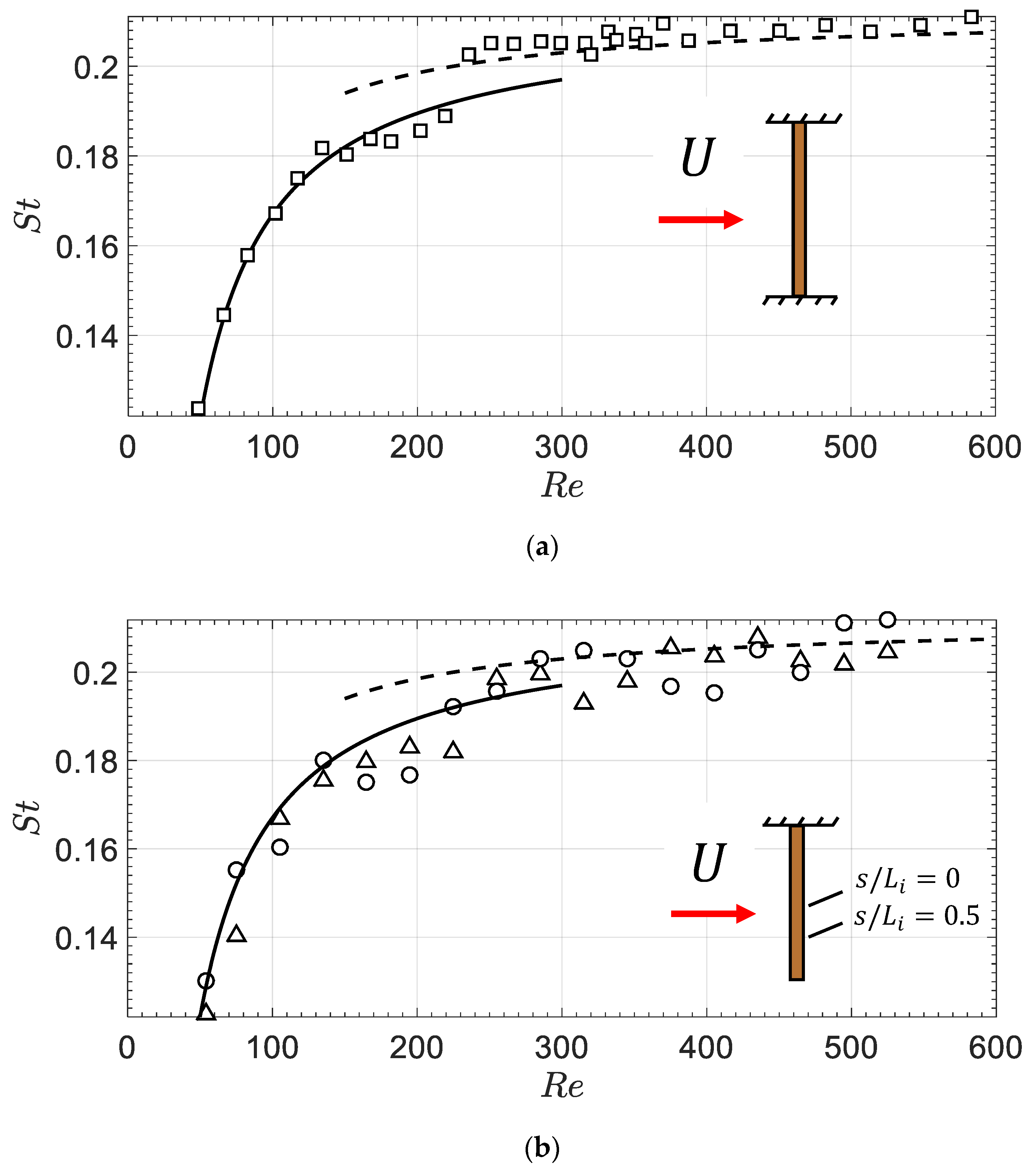
Figure 9, in the more familiar form of Strouhal-Reynolds numbers plot. There is one such plot for each inclination (90°, 75°, 60°, 45°, 30°, and 15°). It is noted that, for the convenience of the reader, the measurements taken at 90° inclination previously presented in
Figure 5b are also included in
Figure 8 and
Figure 9. The contour maps provide a clear visual representation of the vortex shedding and its dependence on the Reynolds number and inclination, allowing extrapolating the results to Reynolds number values and inclinations intermediate between those tested. The Strouhal-Reynolds number plots, on the other hand, provide the data in the form that is most frequently encountered in the literature, and allow a direct quantitative comparison of the present data with the cross-flow vortex shedding prediction methods in Equations (3) and (4).
The results in
Figure 7,
Figure 8 and
Figure 9 confirm and generalize the observations based on the power spectral densities presented in
Figure 6 and previously discussed. That is, most of the times, the inclined leg of the bent cylinder sheds vortices at a frequency that is very close (within a few percent) to the cross-flow frequency for that particular flow velocity. The only exceptions are noticeable at the inclined leg free-end (
) for certain inclinations and Reynolds number values, and can therefore be regarded as free-end effects. In particular:
For the limiting inclination of 90° (
Figure 8a), vortex shedding frequencies at the inclined leg free-end are reduced with respect to cross-flow predictions by about 15–20% at all Reynolds number values tested, and vortex shedding is suppressed altogether for the lowest Reynolds number tested (50). Note that the suppression of vortex shedding is here indicated with a null value of the vortex shedding frequency and Strouhal number.
For the inclination of 75° (
Figure 8b), vortex shedding frequencies at the inclined leg free-end are reduced with respect to cross-flow predictions by about 20–25% in the Reynolds number range of 75–140, and by about 20% at a Reynolds number of 320. At the inclination of 75°, the vortex shedding frequencies measured at the inclined leg free-end for Reynolds numbers within 190–230 and within 350–410 agree well with the cross-flow predictions, though the respective error bars are slightly larger, indicating that the vortex shedding is slightly less coherent. As previously discussed, the absolute uncertainty of the vortex shedding frequency is here estimated as one-half of the full-width-at-half-maximum of the corresponding peak in the power spectrum. An increase in the relative uncertainty of the Strouhal number in
Figure 8, therefore, originates from the broadening of the dominant peak in the power spectrum, which in turn indicates that vortex shedding is losing coherence [
10].
Finally, for the inclination of 15° (
Figure 9c), vortex shedding is suppressed altogether at the inclined leg free-end for Reynolds numbers below 225.
For the operating conditions covered in the present study, and with the exception of the free-end effects just discussed, the frequency of vortex shedding behind the inclined leg of the bent cylinder is not affected by the yaw angle. Deviations with respect to the corresponding cross-flow vortex shedding frequency are in fact on the order of a few percent, which is comparable with the present measuring error. This is noteworthy and indicates that, with the bent cylinder considered here, inclination effects are not present. The present results are in stark contrast with the available literature on vortex shedding behind inclined cylinders, where a systematic reduction in vortex shedding frequency is normally associated with inclination effects. As previously discussed, the flow field that develops around a cylinder with arbitrary inclination with respect to the incoming flow is three-dimensional, and includes an axial-flow component resembling a boundary layer flow that gradually develops along the cylinder. This, in turn, makes the flow field around the inclined cylinder strongly dependent upon the upstream boundary condition, whose effect is not localized but rather transported along the cylinder by the axial boundary layer flow. With the bent cylinder design proposed here, the upstream boundary condition acting upon the inclined leg is imposed by the vertical leg, and corresponds to a fully-established cross-flow vortex shedding. The absence of inclination effects on vortex shedding behind the inclined leg, therefore, suggests that the flow that develops around the inclined leg of the present bent cylinder is entirely controlled by the upstream boundary condition.
As previously noted, the only experiment on vortex shedding from bent cylinders currently available is documented in the study by Ramberg [
7], who tested a cylinder bent into a V-shape with a fixed aperture of 120° between the two legs. A disadvantage of adopting a bent cylinder design with a fixed aperture between the cylinder legs is that the upstream boundary condition acting upon the cylinder leg where the shedding frequency is measured varies with the inclination of the leg with respect to the incoming flow, and is therefore not the same at different yaw angles. The bent cylinder tested here, on the other hand, had different apertures between the legs, so that one leg was always at normal incidence with respect to the free-stream. The upstream boundary condition was therefore always the same, irrespective of the yaw angle of the inclined leg. Despite this improvement in the present bent cylinder design, the present results can be compared with the data from Ramberg [
7], who measured the vortex shedding frequency behind one leg of his bent cylinder (about midway along the leg) at two Reynolds number values (160 and 460) for inclinations between 90° and 30°. Even though the tests by Ramberg [
7] with the bent cylinder were rather limited in scope (only 26 data points in total), the measurements were consistent (to within 15%) with cross-flow vortex shedding, indicating no major inclination effects on the vortex shedding frequency. The present results, therefore, confirm the significance of the bent cylinder configuration originally proposed by Ramberg [
7], and corroborate his observations to wider scope (the present databank includes 306 data points in total), tighter tolerance (on account of the improved bent cylinder design adopted here with a better control over the upstream boundary condition, the present data agree with cross-flow predictions to within a few percent, excluding free-end effects), and wider Reynolds number (50–500) and inclination angle (90°–15°) ranges.
Within the limits of the present study, the axial boundary layer flow seems effective at transporting the upstream boundary condition along the inclined leg of the bent cylinder, but does not seem to otherwise affect the flow. It is worth noting that this would no longer be necessarily the case for a bent cylinder with a longer inclined leg. If the inclined leg was longer, in fact, the axial boundary layer flow would have more time to develop and might even separate, and could therefore more profoundly affect the flow. This clearly motivates further work into vortex shedding behind bent cylinders, focusing in particular on bent cylinders with a longer inclined leg. Analyzing the evolution of the axial boundary layer flow would necessarily require some sort of flow visualization, such as a PIV (Particle Image Velocimetry). Depending on the size of the bent cylinder and on the operating conditions, a high-frequency micro-PIV might be required (the present bent cylinder was 0.5 mm in diameter, and vortex shedding frequencies were as large as 6.86 kHz). High-fidelity CFD (Computational Fluid Dynamics) simulations might be a viable and effective alternative to an experimental flow visualization.