On the Steady-State Flow and Yielding Behaviour of Lubricating Greases
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Rheological Characterization
2.2.1. Stepped Shear Rate and Shear Stress Ramps
2.2.2. Transient Experiments at Constant Shear Rate
2.2.3. Creep Tests
2.2.4. SAOS Experiments
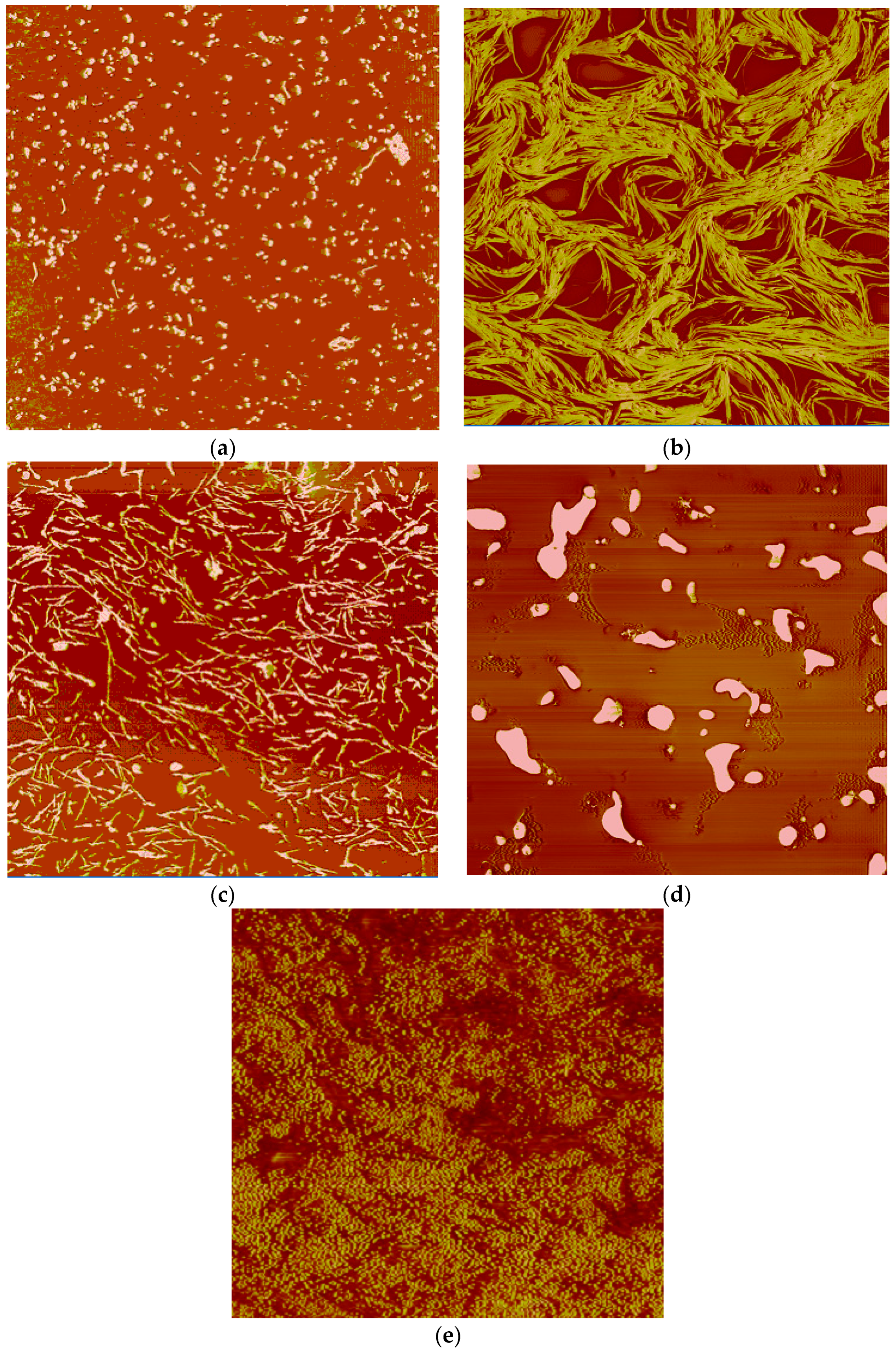
2.3. Atomic Force Microscopy
3. Results
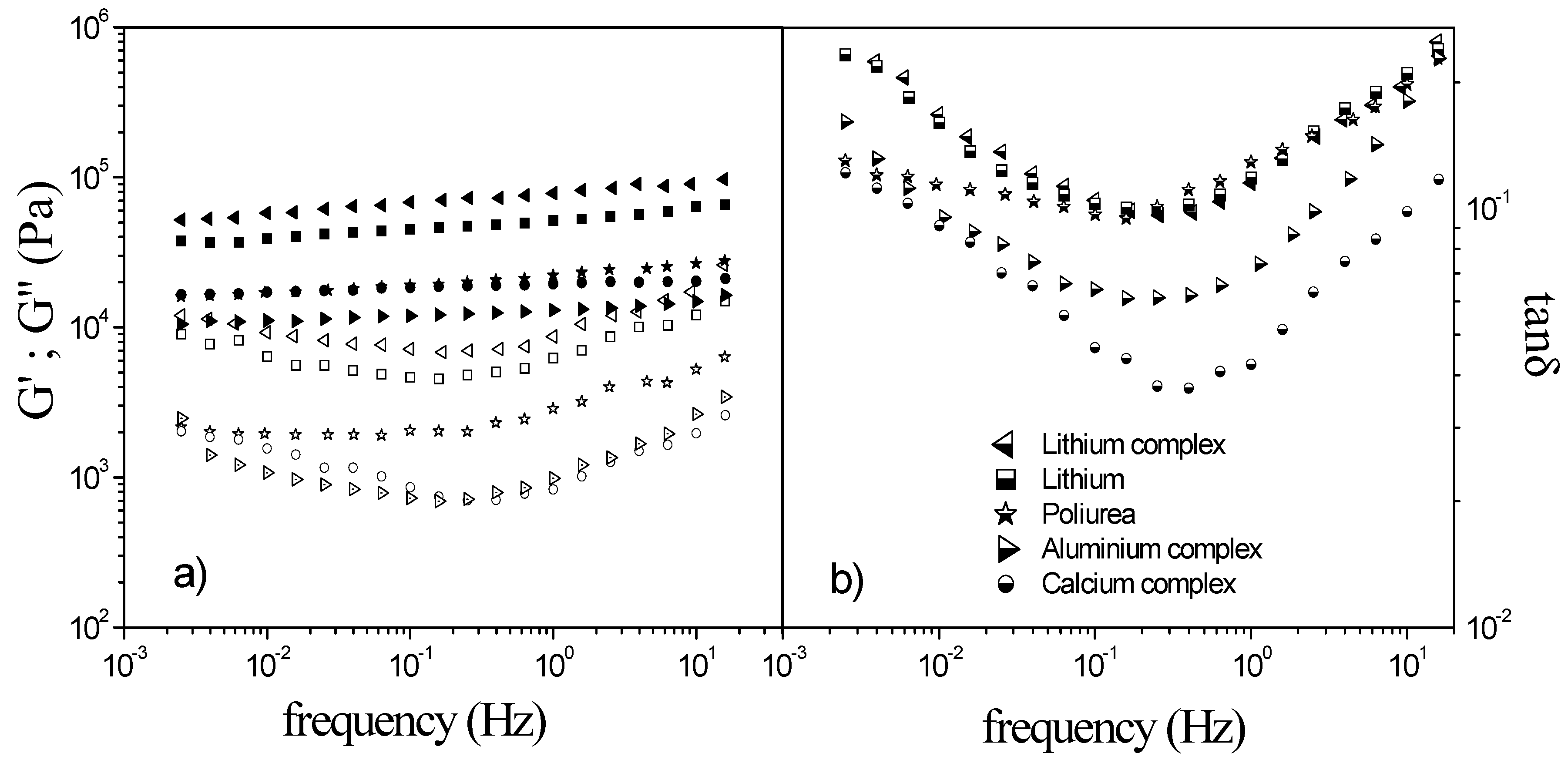
3.1. Linear Viscoelastic Response and Microstructure
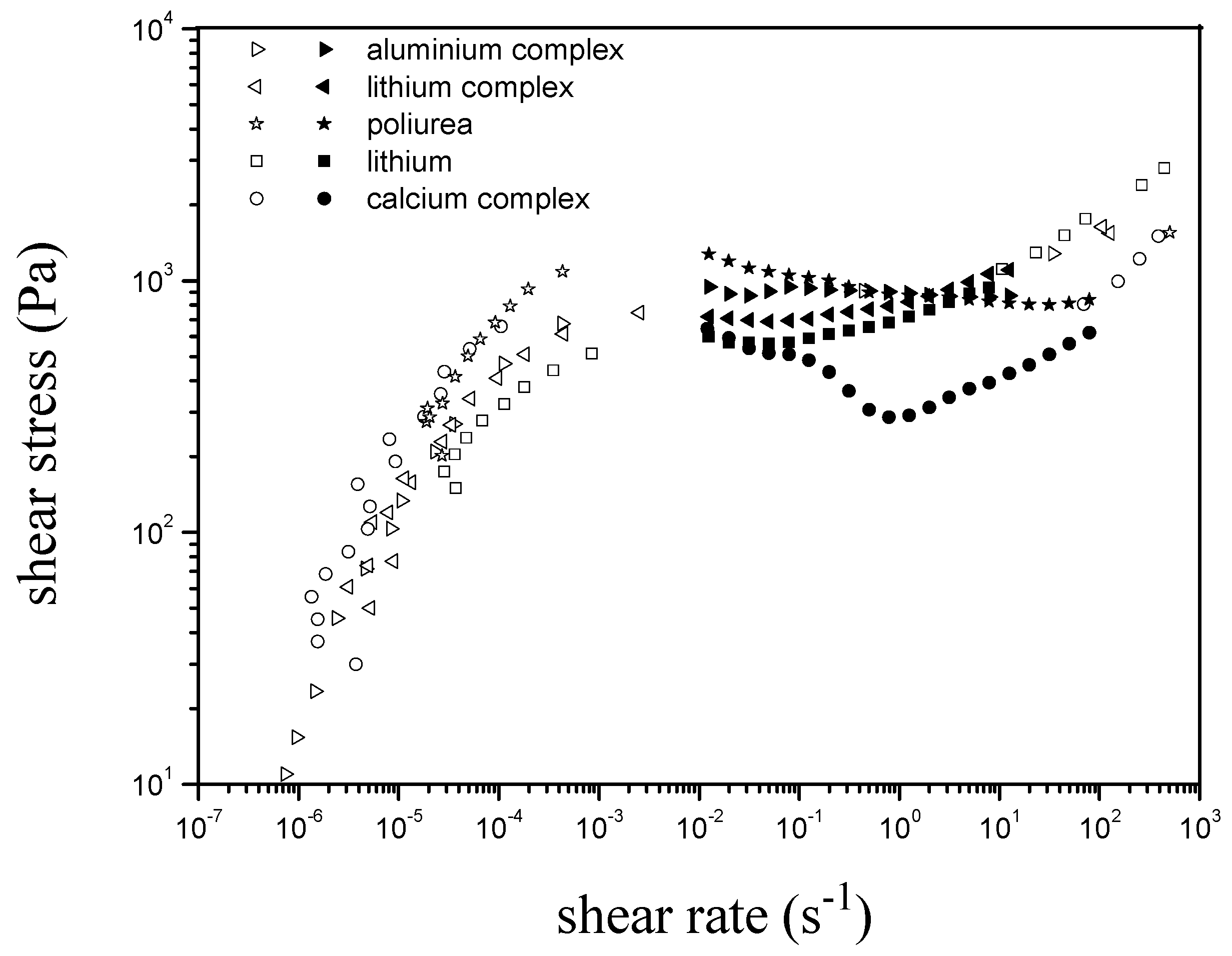
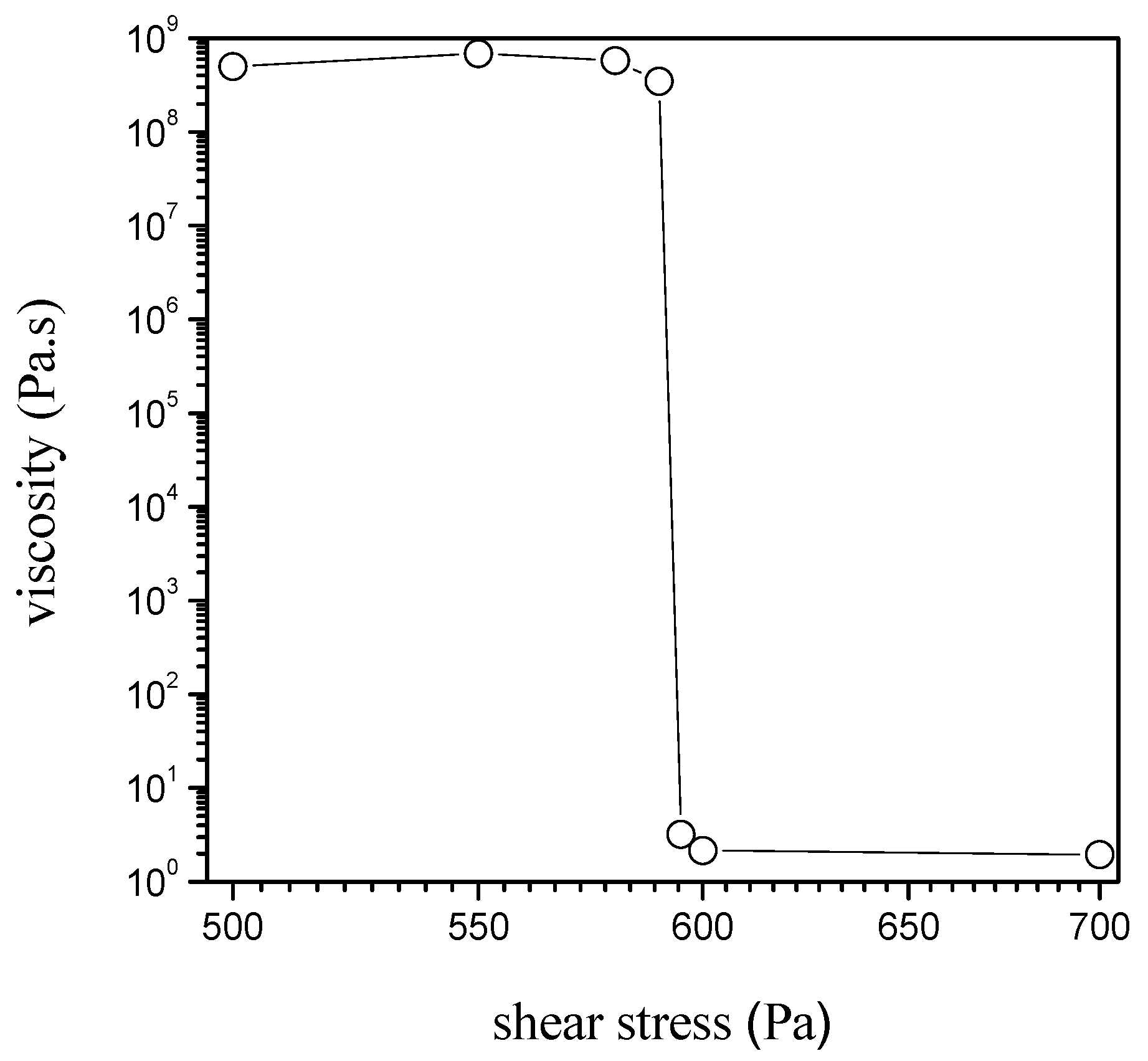
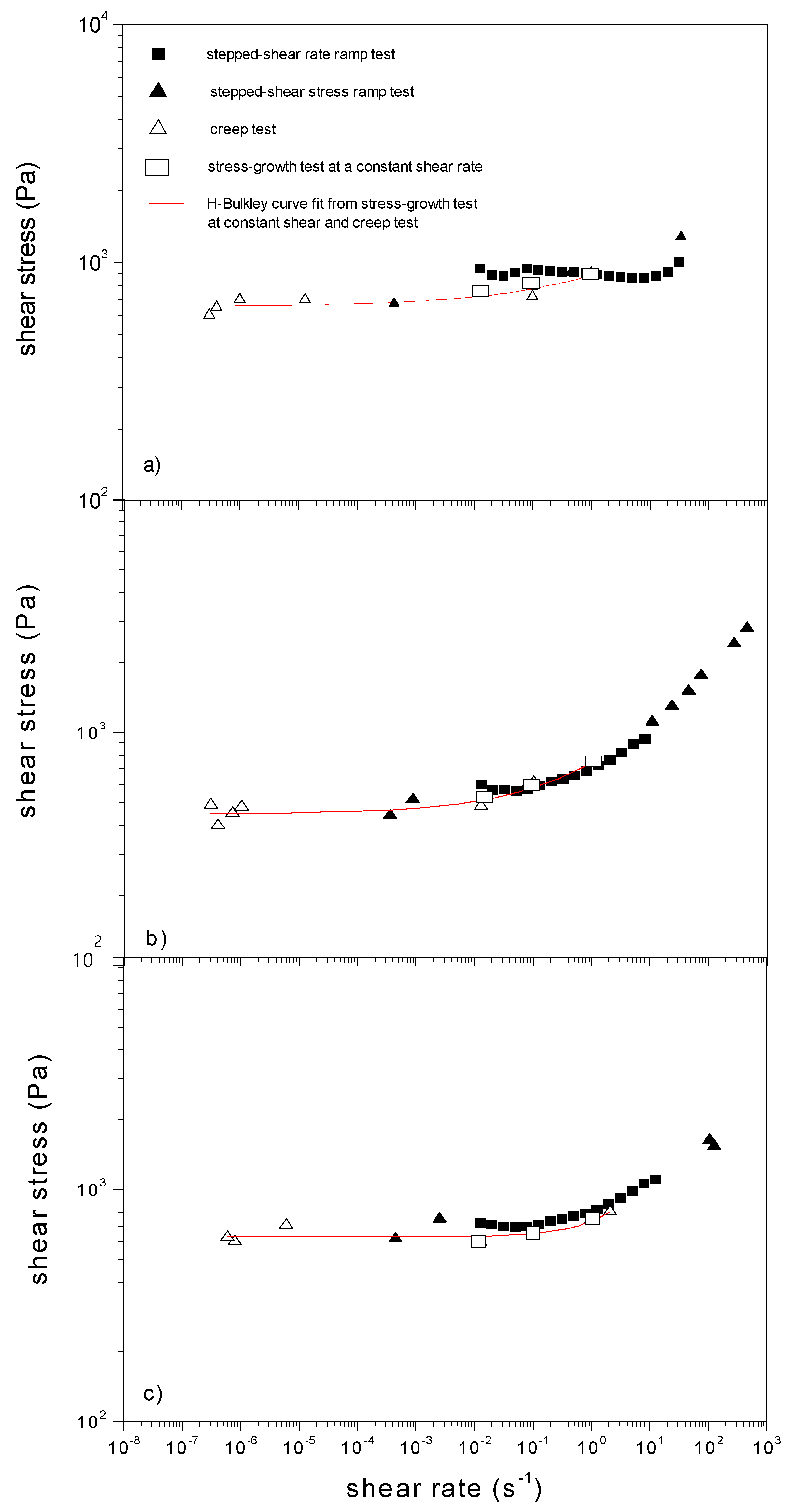
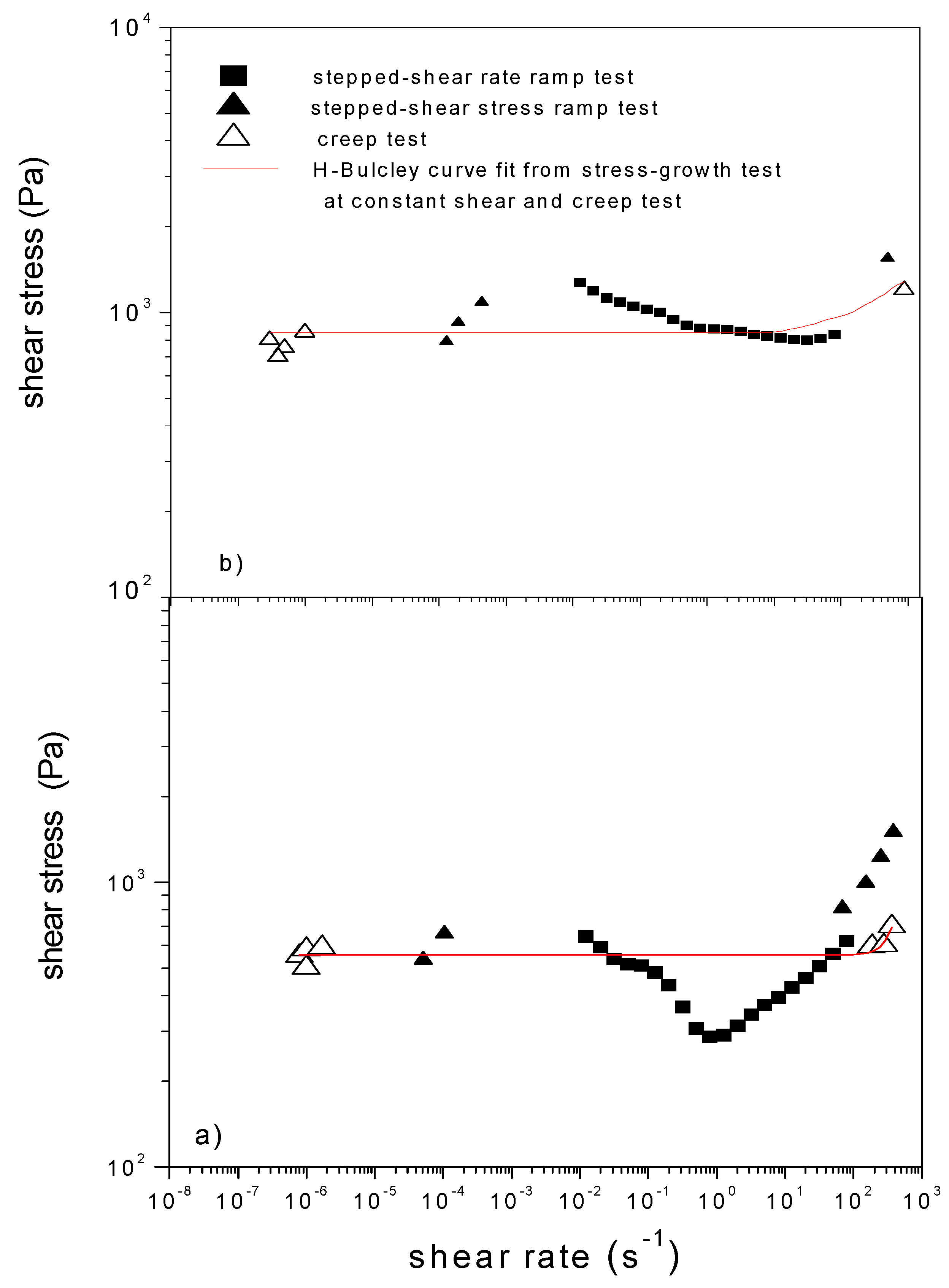
3.2. Stepped-Shear Rate and Stress Ramps: The “Practical” Steady-State Flow Curve
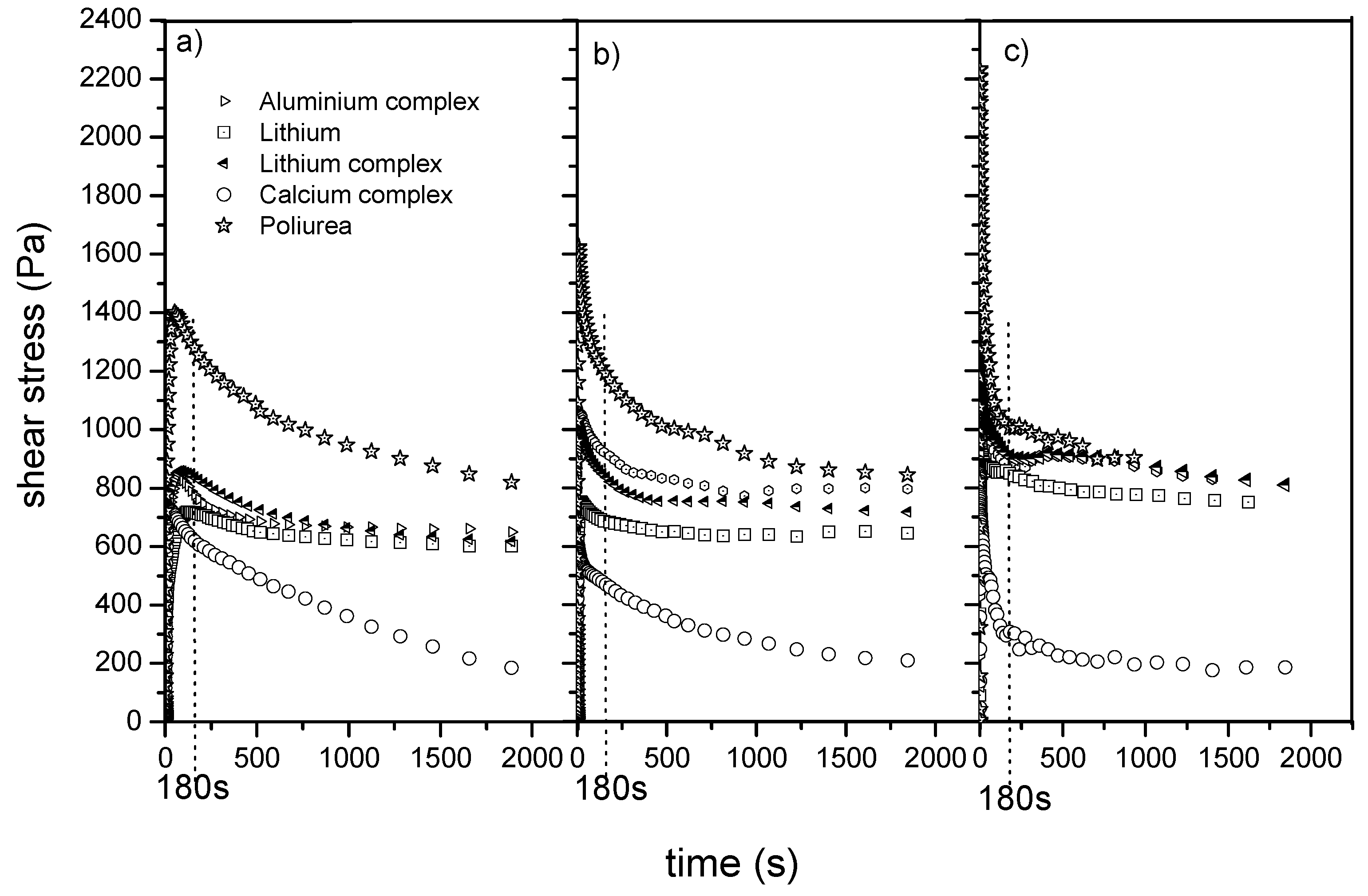
3.3. Transient Flow
3.4. Correction of the “Practical” Steady-State Flow Curve
4. Concluding Remarks
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Dawtray, S. Lubricating greases. In Modern Petroleum Technology; Hobson, G.D., Pohl, W., Eds.; Applied Science: Essex, UK, 1975. [Google Scholar]
- NLGI. Lubricating Greases Guide; National Lubricating Grease Institute: Kansas City, MO, USA, 1994. [Google Scholar]
- Gow, G. Lubricating grease. In Chemistry and Technology of Lubricants, 2nd ed.; Mortier, R.M., Orszulik, S.T., Eds.; Blackie Academic & Professional: London, UK, 1997; pp. 306–319. [Google Scholar]
- Mas, R.; Magnin, A. Rheology of colloidal suspensions: Case of Lubricating Greases. J. Rheol. 1994, 38, 889–908. [Google Scholar] [CrossRef]
- Cho, Y.I.; Choi, E.; Kirkland, W.H. The rheology and hydrodynamic analysis of grease flows in a circular pipe. Tribol. Trans. 1993, 36, 545–554. [Google Scholar] [CrossRef]
- Delgado, M.A.; Valencia, C.; Sánchez, M.C.; Franco, J.M.; Gallegos, C. Thermorheological behaviour of a lithium lubricating grease. Tribol. Lett. 2006, 23, 47–54. [Google Scholar] [CrossRef]
- Bondi, A. Theory and Applications. In Rheology, 3rd ed.; Eirich, F.R., Ed.; Academic Press: New York, NY, USA, 1960; p. 443. [Google Scholar]
- Madiedo, J.M.; Franco, J.M.; Valencia, C.; Gallegos, C. Modeling of the nonlinear rheological behavior of lubricating grease at low shear rates. J. Tribol. 2000, 122, 590–596. [Google Scholar] [CrossRef]
- Yeong, S.K.; Luckhama, P.F.; Tadros, T.F. Steady flow and viscoelastic properties of lubricating grease containing various thickener concentration. J. Colloid Interface Sci. 2004, 274, 285–293. [Google Scholar] [CrossRef] [PubMed]
- Balan, C.; Franco, J.M. Influence of the geometry on the rotational rheometry of lubricating greases. In The Rheology of Lubricating Greases; Balan, C., Ed.; ELGI: Amsterdam, The Netherlands, 2000; pp. 43–66. [Google Scholar]
- Westerberg, L.G.; Sarkar, C.; Farre-Llados, J.; Lundstrom, T.S.; Hoglund, E. Lubricating grease flow in a double restriction seal geometry: A computational fluid dynamics approach. Tribol. Lett. 2017, 65, 82. [Google Scholar] [CrossRef]
- Westerberg, L.G.; Farre-Llados, J.; Sarkar, C.; Casals-Terre, J. Contaminant particle motion in lubricating grease flow: A computational fluid dynamics approach. Lubricants 2018, 6, 10. [Google Scholar] [CrossRef]
- Yu, R.F.; Li, P.; Chen, W. Study of grease lubricated journal bearing with partial surface texture. Ind. Lubr. Technol. 2016, 68, 149–157. [Google Scholar] [CrossRef]
- Wu, Z.H.; Xu, Y.Q.; Deng, S.E. Analysis of dynamic characteristics of grease-lubricated tapered roller bearings. Shock Vibr. 2018, 2018, 7183042. [Google Scholar] [CrossRef]
- Sarkar, C.; Westerberg, L.G.; Hoglund, E.; Lundstrom, T.S. Numerical simulations of lubricating grease flow in a rectangular channel with and without restrictions. Tribol. Trans. 2018, 61, 144–156. [Google Scholar] [CrossRef]
- Dobrowolski, J.D.; Gawlinski, M.; Paszkowski, M.; Westerberg, L.G.; Hoglund, E. Experimental study of lubricating grease flow inside the gap of a labyrinth seal using microparticle image velocimetry. Tribol. Trans. 2018, 61, 31–40. [Google Scholar] [CrossRef]
- Li, J.X.; Westerberg, L.G.; Hoglund, E.; Lugt, P.M.; Baart, P. Lubricating grease shear flow and boundary layers in a concentric cylinder configuration. Tribol. Trans. 2014, 57, 1106–1115. [Google Scholar] [CrossRef]
- Cyriac, F.; Lugt, P.M.; Bosman, R. On a new method to determine the yield stress in lubricating grease. Tribol. Trans. 2015, 58, 1021–1030. [Google Scholar] [CrossRef]
- Cyriac, F.; Lugt, P.M.; Bosman, R. Yield stress and low-temperature start-up torque of lubricating greases. Tribol. Lett. 2016, 63, 6. [Google Scholar] [CrossRef]
- Møller, P.C.F.; Mewis, J.; Bonn, D. Yield stress and thixotropy: On the difficulty of measuring yield stresses in practice. Soft Matter 2006, 2, 274–283. [Google Scholar] [CrossRef]
- Barrnes, H.A. The Yield Stress-a review or “παντα ρει”-everything flow? J. Non-Newtonian Fluid Mech. 1999, 81, 133–178. [Google Scholar] [CrossRef]
- Magnin, A.; Piau, J.M. Shear rheometry of fluids with a yield stress. J. Non-Newtonian Fluid Mech. 1987, 23, 91–106. [Google Scholar] [CrossRef]
- Hartnett, J.P.; Hu, R.Y.Z. The yield stress - an engineering reality. J. Rheol. 1989, 33, 671–679. [Google Scholar] [CrossRef]
- Almdal, K.; Dyre, J.; Hvidt, S.; Kramer, O. Towards a phenomenological definition of the term “gel”. Polym. Gels Netw. 1993, 1, 5–17. [Google Scholar] [CrossRef]
- Sánchez, M.C.; Franco, J.M.; Valencia, C.; Gallegos, C.; Urquiola, F.; Urchegui, R. Atomic force microscopy and thermo-rheological characterization of lubricating greases. Tribol. Lett. 2011, 41, 463–470. [Google Scholar] [CrossRef]
- Delgado, M.A.; Sánchez, M.C.; Valencia, C.; Franco, J.M.; Gallegos, C. Relationship among microstructure, rheology and processing of a lithium lubricating grease. Chem. Eng. Res. Des. 2005, 83, 1085–1092. [Google Scholar] [CrossRef]
- Coussot, P. Slow flows of yield stress fluids: Yielding liquids or flowing solids? Rheol. Acta 2018, 57, 1–14. [Google Scholar] [CrossRef]
- Britton, M.M.; Callagham, P.T. Nuclear magnetic resonance visualization of anomalous flow in cone-and-plate rheometry. J. Rheol. 1997, 41, 1365. [Google Scholar] [CrossRef]
- Coussot, P.; Nguyen, Q.D.; Huynh, H.T.; Bonn, D. Avalanche behavior in yield stress fluids. Phys. Rev. Lett. 2002, 88, 175–207. [Google Scholar] [CrossRef] [PubMed]
- Coussot, P. Yield stress fluid flows: A review of experimental data. J. Non-Newtonian Fluid Mech. 2014, 211, 31–49. [Google Scholar] [CrossRef]
- Ovarlez, G.; Rodts, S.; Chateau, X.; Coussot, P. Phenomenology and physical origin of shear localization and shear banding in complex fluids. Rheol. Acta 2009, 48, 831–834. [Google Scholar] [CrossRef]
- Rubio-Hernandez, F.J.; Paez-Flor, N.M.; Velazquez-Navarro, J.F. Why monotonous and non-monotonous steady-flow curves can be obtained with the same non-Newtonian fluid? A single explanation. Rheol. Acta 2018, 57, 389–396. [Google Scholar] [CrossRef]
- Mewis, J. Thixotropy—A general review. J. Non-Newtonian Fluid Mech. 1979, 6, 1–20. [Google Scholar] [CrossRef]
- Barnes, H.A. Thixotropy—A review. J. Non-Newtonian Fluid Mech. 1997, 70, 1–33. [Google Scholar] [CrossRef]
- Delgado, M.A.; Franco, J.M.; Valencia, C.; Kuhn, E.; Gallegos, C. Transient shear flow of model lithium lubricating greases. Mech. Time-Depend. Mater. 2009, 13, 63–80. [Google Scholar] [CrossRef]
- Papenhuijzen, J.M.P. The role of particle interactions in the rheology of dispersed systems. Rheol. Acta 1972, 11, 73–88. [Google Scholar] [CrossRef]
- Kuhn, E. Analysis of a grease-lubricated contact from an energy point of view. Int. J. Mater. Prod. Technol. 2010, 38, 5–15. [Google Scholar] [CrossRef]
- Coussot, P.; Lenov, A.I.; Piau, J.M. Rheology of concentrated dispersed systems in a low molecular weight matrix. J. Non-Newtonian Fluid Mech. 1993, 46, 179–217. [Google Scholar] [CrossRef]
- Delgado, M.A.; Valencia, C.; Sánchez, M.C.; Franco, J.M.; Gallegos, C. Influence of soap concentration and oil viscosity on the rheology and microstructure of lubricating greases. Ind. Eng. Chem. Res. 2006, 45, 1902–1910. [Google Scholar] [CrossRef]

| Soap | Oil Viscosity at 40 °C (cP) | NLGI Grade | Drop Point (°C) |
|---|---|---|---|
| Aluminum Complex | 130 | 2 | >250 |
| Lithium | 150 | 2 | >150 |
| Lithium Complex | 160 | 2 | >250 |
| Calcium Complex | 150 | 1 | >250 |
| Polyurea | 110 | 2 | >240 |
| Lubricating Greases | 0.0125 s−1 | 0.1 s−1 | 1 s−1 | |||
|---|---|---|---|---|---|---|
| S+ | tmax (s) | S+ | tmax (s) | S+ | tmax (s) | |
| Aluminum Complex | 0.282 | 74 | 0.321 | 16.8 | 0.439 | 1.91 |
| Lithium | 0.172 | 128 | 0.157 | 22.1 | 0.238 | 3.08 |
| Lithium complex | 0.392 | 85 | 0.397 | 15.7 | 0.351 | 1.67 |
| Calcium complex | 2.946 | 26 | 1.635 | 2.6 | 3.043 | 0.24 |
| Polyurea | 0.708 | 52 | 0.890 | 6.8 | 1.477 | 0.65 |
| Lubricating Greases | τ0 (Pa) | kH (Pa·sn) | n |
|---|---|---|---|
| Aluminum Complex | 654 | 232 | 0.28 |
| Lithium | 448 | 301 | 0.35 |
| Lithium Complex | 626 | 105 | 0.72 |
| Calcium Complex | 543 | 40 | 0.15 |
| Polyurea | 859 | 47 | 0.20 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Delgado, M.A.; Secouard, S.; Valencia, C.; Franco, J.M. On the Steady-State Flow and Yielding Behaviour of Lubricating Greases. Fluids 2019, 4, 6. https://doi.org/10.3390/fluids4010006
Delgado MA, Secouard S, Valencia C, Franco JM. On the Steady-State Flow and Yielding Behaviour of Lubricating Greases. Fluids. 2019; 4(1):6. https://doi.org/10.3390/fluids4010006
Chicago/Turabian StyleDelgado, Miguel A., Sebastien Secouard, Concepción Valencia, and José M. Franco. 2019. "On the Steady-State Flow and Yielding Behaviour of Lubricating Greases" Fluids 4, no. 1: 6. https://doi.org/10.3390/fluids4010006
APA StyleDelgado, M. A., Secouard, S., Valencia, C., & Franco, J. M. (2019). On the Steady-State Flow and Yielding Behaviour of Lubricating Greases. Fluids, 4(1), 6. https://doi.org/10.3390/fluids4010006







