Sustaining Vaccine Potency in Cold Chain Logistics: Numerical Analysis of Extended Cooling Duration in Glycerol-Infused n-Tetradecane Phase-Change Materials

Abstract

Vaccination cold chains depend critically on maintaining temperatures within the 2–8 °C range, with phase-change materials (PCMs) like n-tetradecane offering substantial potential due to their high latent heat and optimal melting characteristics. Despite extensive research on PCM melting enhancement, strategies to extend melting duration and thermal stability remain underexplored. This pioneering numerical study investigates the impact of incorporating 5% glycerol additive in n-tetradecane, aiming to decelerate the melting rate and sustain the desired temperature range over prolonged periods. This study numerically assesses the effect of a 5% glycerol additive on n-tetradecane, revealing a substantial 20.6 h extension in safe temperature maintenance, from 123.3 h in pure n-tetradecane (T) to 143.9 h with the additive (T + G). Although T reaches full melting in 121.7 h, the air temperature inside the cold box breaches 8 °C only 1.6 h after; in contrast, T + G reaches this threshold 2.2 h before full melting, resulting in an effective extension of 20.6 h. Entropy analysis shows a delayed rise in T + G, indicating enhanced thermal stability, while temperature contours confirm T + G sustains cooling until day 6, a full day beyond T. These findings highlight glycerol’s potential to modulate thermal dynamics within PCM-based cold boxes, offering a cost-effective improvement in vaccine transport sustainability.

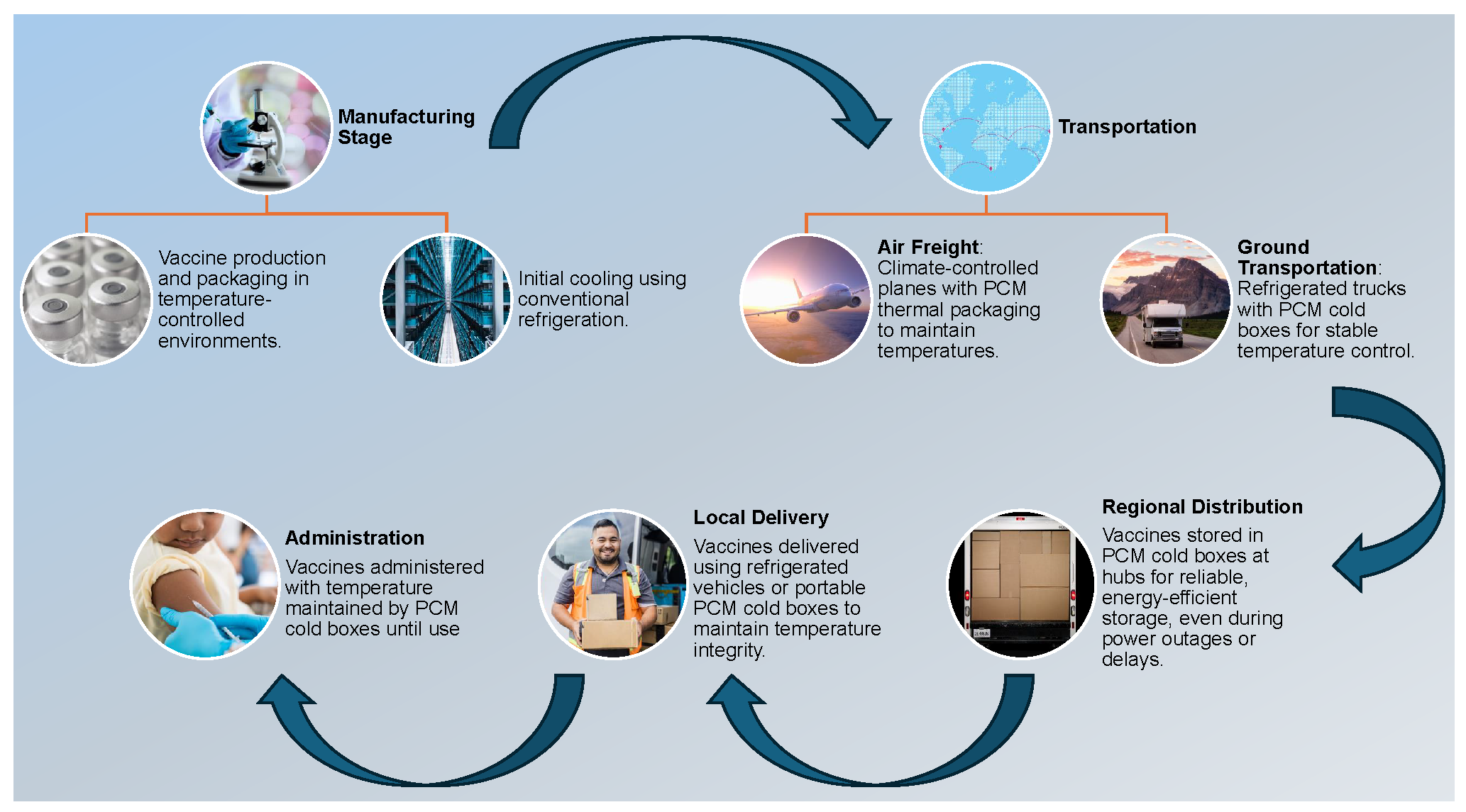
1. Introduction

The transportation of vaccines has become a pivotal aspect of global health, particularly in the wake of the COVID-19 pandemic. Vaccines are sensitive biological products that require strict temperature control throughout their journey from manufacturers to end users. The World Health Organization (WHO) emphasizes the importance of maintaining the cold chain to ensure vaccine efficacy, as deviations can lead to reduced potency or complete loss of effectiveness [1]. For instance, the Pfizer-BioNTech COVID-19 vaccine, once produced in Belgium, had to be shipped to various countries, including Indonesia. This journey typically involved refrigerated containers transported to major air cargo hubs like Changi Airport in Singapore before being flown to their final destination. The entire process could take up to five days, during which maintaining the required ultra-cold temperature was crucial. To ensure viability, phase-change materials (PCMs) are utilized for temperature regulation; however, these materials may require recharging at designated points along the route to maintain the necessary temperature throughout the journey [2].

As vaccine distribution increasingly operates on an intercontinental scale, logistical challenges—such as limited transport capacity and varying infrastructure—pose significant threats to timely and safe delivery. PCMs have emerged as a promising solution to enhance cold chain logistics for vaccines. These materials can absorb and release large amounts of thermal energy, effectively maintaining specific temperature ranges for extended periods. For example, TCP’s PC-21 solution can keep vaccines at ultra-cold temperatures for significantly longer durations than traditional methods like dry ice, which present safety hazards and logistical complications [3].

Recent advances in thermal insulation technologies, such as vacuum insulation panels (VIPs), also contribute significantly to cold chain efficiency. VIPs, widely recognized for their excellent adiabatic performance, have been explored in various applications, including cold chain containers, where they demonstrate remarkable thermal insulation properties [4]. Research has shown that the use of VIPs in multi-layer insulation systems, combined with advanced cooling technologies like semiconductor devices, can extend the duration of temperature control during transit [5]. Moreover, nano-aerogel composites used in VIP cores have been found to optimize thermal conductivity and enhance overall energy efficiency in cold chain systems [6]. These advancements underscore the potential of integrating innovative thermal management materials into vaccine logistics.

While extensive research has focused on accelerating PCM melting using passive techniques like fins and nanoparticles [7,8,9,10,11,12,13,14,15], and active methods such as vibrations and magnetic fields [16,17,18,19,20], limited efforts have been made to slow down the melting process.

The potential for extending the melting duration of PCM-lined containers could revolutionize vaccine transportation. This extension not only provides a buffer against unforeseen delays but also enhances the reliability of maintaining optimal temperatures during transit. By ensuring that vaccines remain within their required temperature range longer, we can significantly reduce the risk of wastage and improve overall vaccination rates globally [21].

This study presents a novel approach to extending the melting duration of PCMs, enabling vaccines to remain within their optimal temperature range for longer periods. By focusing on delaying the phase-change process, it introduces a unique perspective that could transform cold chain logistics and significantly enhance vaccine preservation during transportation. Unlike the existing literature, which primarily emphasizes accelerating PCM melting, this work explores the largely unexamined area of delaying PCM melting. Specifically, it investigates the impact of organic nanoparticles on pure PCMs, offering a fresh and innovative contribution to the field.

Figure 1 illustrates a comprehensive vaccine supply chain framework utilizing PCM technology for thermal regulation. This diagram delineates the critical stages of vaccine production, transportation, and administration, emphasizing the integration of PCM-lined containers to maintain the cold chain. Key components include initial refrigeration in temperature-controlled environments, air freight via climate-controlled aircraft, refrigerated ground transport with PCM cold boxes, regional distribution in energy-efficient hubs, and local delivery through temperature-maintained vehicles, ensuring optimal stability and efficacy throughout the entire logistics process.

Moreover, as global vaccination efforts expand—particularly in low- and middle-income countries where infrastructure may be lacking—optimizing PCM technology becomes crucial. The ability to transport larger quantities of vaccines safely and effectively can facilitate equitable access to immunization, ultimately contributing to global health security. This paper explores advancements in PCM technology and its implications for the prolonged cooling of vaccines, highlighting its role in overcoming logistical barriers in vaccine distribution.

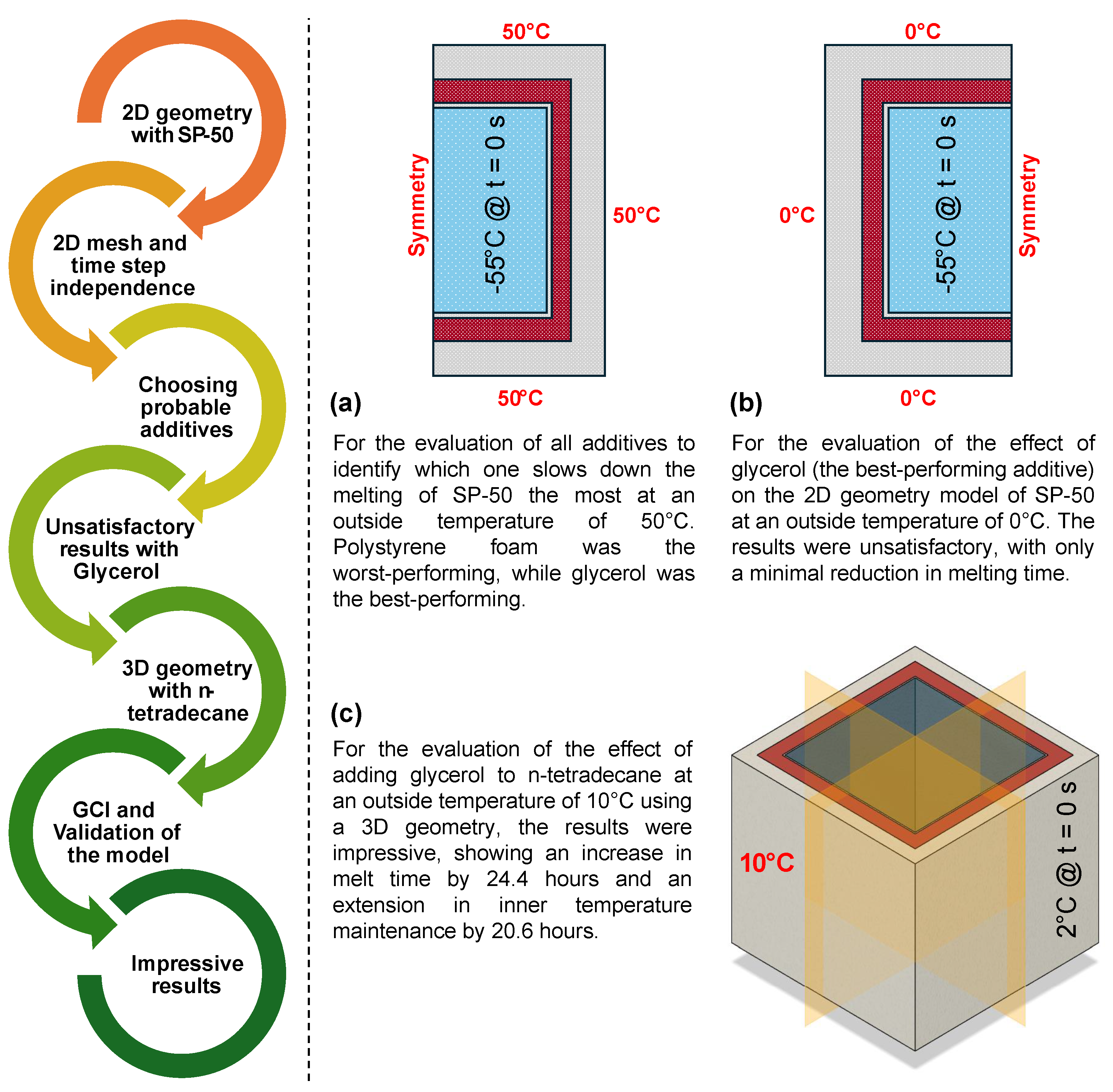
2. Identifying Suitable Additives

To optimize PCMs for vaccine transport, the possibility of the modification of their properties was explored to extend the melting time. An intriguing approach involved adding specific additives to decrease the thermal conductivity and/or increase the latent heat and specific heat capacity. This passive, cost-effective solution could effectively reduce the melting rate, providing a more sustainable method for maintaining stable temperatures during vaccine delivery.

The search for additives aimed to identify materials that could increase the duration of the maintenance of temperature inside the cold box for vaccine delivery for longer duration. Simulations were conducted using ANSYS Fluent v 2022 with a mixture of 5% additive and 95% PCM to study the melting process and identify effective candidates for slowing melting. The simulations were conducted on a system equipped with 16 GB of RAM, 6 logical cores, and a memory speed of 3200 MT/s. The model used SP-50 as the PCM and was structured with a 2D geometry comprising an outer insulation layer (20 mm), a 10 mm SP-50 layer, an inner insulation layer (2 mm), and a central air cavity, as depicted in Figure 2. In this initial pilot study, the primary objective was to identify which additive effectively slows down the melting rate of the PCM. To reduce computation time, only one half of the geometry was simulated, assuming symmetry along the vertical plane passing through the center of the geometry.

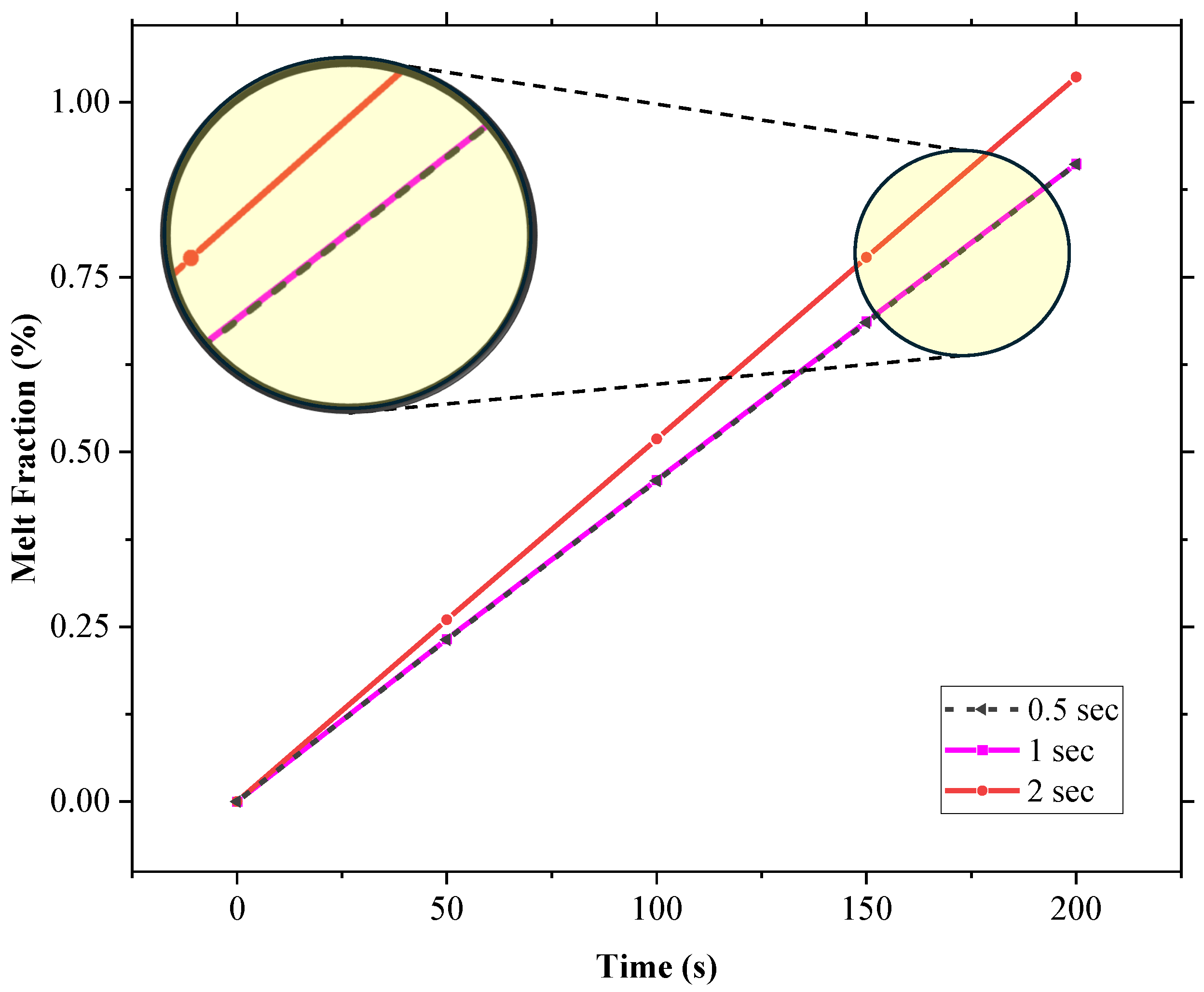
2.1. Mesh and Time Step Independence

A mesh independence study tested element sizes from 0.01 mm to 1 mm, monitoring the temperature at the top left vertex of SP-50 after 15,000 s, as depicted in Figure 3. It was, therefore, concluded that a mesh size of 0.1 mm was optimal, and a time step independence analysis found a 1 s time step as appropriate for the initial conditions, as shown in Figure 4.

With the parameters optimized, various additives were tested at a 5% volume fraction—polypropylene co-polymer, ethylene glycol (30% and 50%), glycerol, expanded polystyrene (EPS), and polystyrene foam, whose properties are taken from

Table 1. Thermal properties of additives [22].

Material	k (W/m·K)	${\mathit{C}}_{\mathit{p}}$ (J/kg·K)	$\mathit{\rho }$ (kg/m3)
Polypropylene Co-polymer	0.126	2343	910
Ethylene Glycol (30%)	0.484	3714	1037
Ethylene Glycol (50%)	0.425	3287	1056
EPS (Expanded Polystyrene)	0.033	1130	38
Polystyrene Foam	0.026	1130	46

.

The melting performance was compared for SP-50 and SP-50 with additives, with the properties of SP-50 sourced from Ray et al. [23]. These specific additives were chosen due to their low thermal conductivity. As the focus was on deriving qualitative insights rather than quantitative data, harsher boundary conditions were deliberately employed. With an initial temperature of −55 °C, the external temperature ($T_{ext}$) was set to 350 K, coupled with natural convection characterized by a heat transfer coefficient of h = 8.5 W/m²·K. These conditions were imposed for rapid melting, allowing for a faster evaluation of the additives and their relative impact on PCM performance.

The MF versus time plot for SP-50, with all the additives at a 5% volume fraction concentration, was plotted (Figure 5) and analyzed, and it was found that glycerol performed the best, as its MF vs. time curve was the lowest, indicating the greatest reduction in melting speed. The performance ranking of the additives, in terms of their effectiveness at reducing melting speed, was observed to be as follows: glycerol > expanded polystyrene ≈ ethylene glycol (30%) ≈ ethylene glycol (50%) > polypropylene co-polymer. Interestingly, polystyrene foam had a counterproductive effect, accelerating the melting process faster than pure SP-50.

To further study the performance of glycerol in reducing the melting speed of the PCM, refined simulations under better ambient conditions ($T_{ext}=$ 0 °C, h = 8.5 W/m²·K and the same initial temperature of −55 °C) were conducted.

2.2. Initial Results with SP-50

Figure 6 presents the melt fraction (MF) vs. time curve for the melting of pure SP-50 compared to SP-50 with a 5% volume fraction of glycerol added. Additionally, Figure 7 illustrates the temperature vs. time curve for both cases within the PCM domain, as well as the air cavity located inside the PCM domain (refer to Figure 2). The simulation results show that SP-50 melted completely in 17,150 s, while SP-50 mixed with 5% volume fraction of glycerol took slightly longer, melting in 17,400 s. This indicates that the improvement provided by the addition of glycerol was unsatisfactory. The increase in melting time—only 250 s above the base case of 17,150 s—was minimal. The 250 s increment over 17,150 s due to the addition of glycerol suggests that even if the results were simply linearly interpolated, for a typical 120 h vaccine transport duration, we would only gain about 1.75 h of extended cooling time—a marginal improvement at best. Despite these underwhelming results, glycerol was tested again with 3D geometry and higher external temperature conditions.

3. Methods

3.1. Numerical Formulation

Computational simulations were conducted utilizing the pressure-based model due to its compatibility with the melting and solidification processes in ANSYS Fluent v 2022. Transient simulations were performed to accurately depict temporal changes. The SIMPLE pressure–velocity coupling method was used, alongside the PRESTO! pressure solver, and a second-order upwind scheme was employed for the precise calculation of momentum and energy equations. The under-relaxation factors for the pressure correction, density, body forces, momentum, liquid fraction, and thermal energy were specified as 0.3, 1, 1, 0.7, 0.9, and 0.95, respectively. Convergence criteria were established at ${10}^{-3}$ for the continuity and momentum equations, and at ${10}^{-6}$ for the energy equation.

The numerical simulation of PCM melting dynamics, both with and without additives, was based on the following fundamental assumptions:

• Isotropic and Homogeneous Medium: The PCM matrix is assumed to be isotropic and homogeneous, neglecting microscale variations in density, viscosity, or composition due to their minimal influence under steady-state and transient conditions with a homogeneous distribution of the additives (if present) in the PCM domain.
• Negligible Volumetric Expansion: Volumetric changes during the phase transition are assumed to be inconsequential due to the limited expansion or contraction of the PCM, rendering their impact negligible within the temporal resolution of the computational domain.
• Omission of Radiative Heat Transfer: Radiative heat transfer mechanisms are disregarded, as conduction is the primary mode of heat transfer within the PCM due to its low thermal diffusivity.
• Laminar Flow Assumption: Flow within the PCM is modeled as a closed system with negligible kinetic energy, assuming laminar flow due to a low Reynolds number.
• Absence of Internal Heat Generation: Internal heat generation is assumed to be absent within the PCM matrix, as there are no volumetric or chemical heat sources.
• Air Instead of Vaccines: The PCM box is assumed to contain air instead of vaccines for a conservative analysis; as air heats faster, it yields a more cautious temperature reading.

3.2. Mathematical Model

In taking into account the assumptions mentioned above, the following equations were used: [24]

• Continuity:

  $${\displaystyle \frac{\partial \left(\rho u\right)}{\partial x}}+{\displaystyle \frac{\partial \left(\rho v\right)}{\partial y}}+{\displaystyle \frac{\partial \left(\rho w\right)}{\partial z}}=0$$

  (1)
• Momentum:

  $$\rho {\displaystyle \frac{\partial u}{\partial t}}+\rho u{\displaystyle \frac{\partial u}{\partial x}}+\rho v{\displaystyle \frac{\partial u}{\partial y}}+\rho w{\displaystyle \frac{\partial w}{\partial z}}=\nabla (\mu \nabla u)-{\displaystyle \frac{\partial P}{\partial x}}$$

  (2)

  $$\rho {\displaystyle \frac{\partial v}{\partial t}}+\rho u{\displaystyle \frac{\partial v}{\partial x}}+\rho v{\displaystyle \frac{\partial v}{\partial y}}+\rho w{\displaystyle \frac{\partial w}{\partial z}}=\nabla (\mu \nabla v)-{\displaystyle \frac{\partial P}{\partial y}}$$

  (3)

  $$\rho {\displaystyle \frac{\partial w}{\partial t}}+\rho u{\displaystyle \frac{\partial w}{\partial x}}+\rho v{\displaystyle \frac{\partial w}{\partial y}}+\rho w{\displaystyle \frac{\partial w}{\partial z}}=\nabla (\mu \nabla w)-{\displaystyle \frac{\partial P}{\partial z}}-\rho g$$

  (4)
• Energy:

  $${\displaystyle \frac{\partial \left(\rho cT\right)}{\partial t}}+{\displaystyle \frac{\partial \left(\rho ucT\right)}{\partial x}}+{\displaystyle \frac{\partial \left(\rho vcT\right)}{\partial y}}+{\displaystyle \frac{\partial \left(\rho wcT\right)}{\partial z}}=\nabla (k\nabla T)$$

  (5)

In the equations, $\rho$ denotes the density of the fluid; $u,v,$ and w represent the velocity components in the x, y, and z directions, respectively; t indicates time; P signifies the pressure within the fluid; c denotes the specific heat capacity; T represents the temperature; and k refers to the thermal conductivity of the fluid.

To calculate the properties of ‘PCM + additives’, the following equations are utilized [25,26]:

• Density:

  $${\rho }_{\mathrm{mix}}=\chi {\rho }_{\mathrm{add}}+(1-\chi ){\rho }_{\mathrm{pcm}}$$

  (6)
• Specific Heat Capacity:

  $$C_{p_{,\mathrm{mix}}}={\displaystyle \frac{\chi C_{p_{,\mathrm{add}}}{\rho }_{\mathrm{add}}+(1-\chi )C_{p_{,\mathrm{pcm}}}{\rho }_{\mathrm{pcm}}}{{\rho }_{\mathrm{mix}}}}$$

  (7)
• Thermal Conductivity:

  $$k_{\mathrm{mix}}=\chi k_{\mathrm{add}}+(1-\chi )k_{\mathrm{pcm}}$$

  (8)
• Melting point:

  $$\begin{array}{cc}\hfill & T_m=\sum _{i=1}^n{\displaystyle \frac{T_i{L}_i}{L_i-T_{i}Rln{\chi }_i}}\hfill \end{array}$$

  (9)
• Latent Heat:

  $$\begin{array}{cc}\hfill & L_{\mathrm{m}}=T_{\mathrm{m}}\sum _{\mathrm{i}=1}^{\mathrm{n}}{\displaystyle \frac{{\chi }_{\mathrm{i}}{L}_{\mathrm{i}}}{T_{\mathrm{i}}}}\hfill \end{array}$$

  (10)

where $\chi$ is the volume fraction, $\rho$ is the density, k is the thermal conductivity, T is the temperature, L is the melting heat, and R is the gas constant, R = 8.314 J/(mol·K).

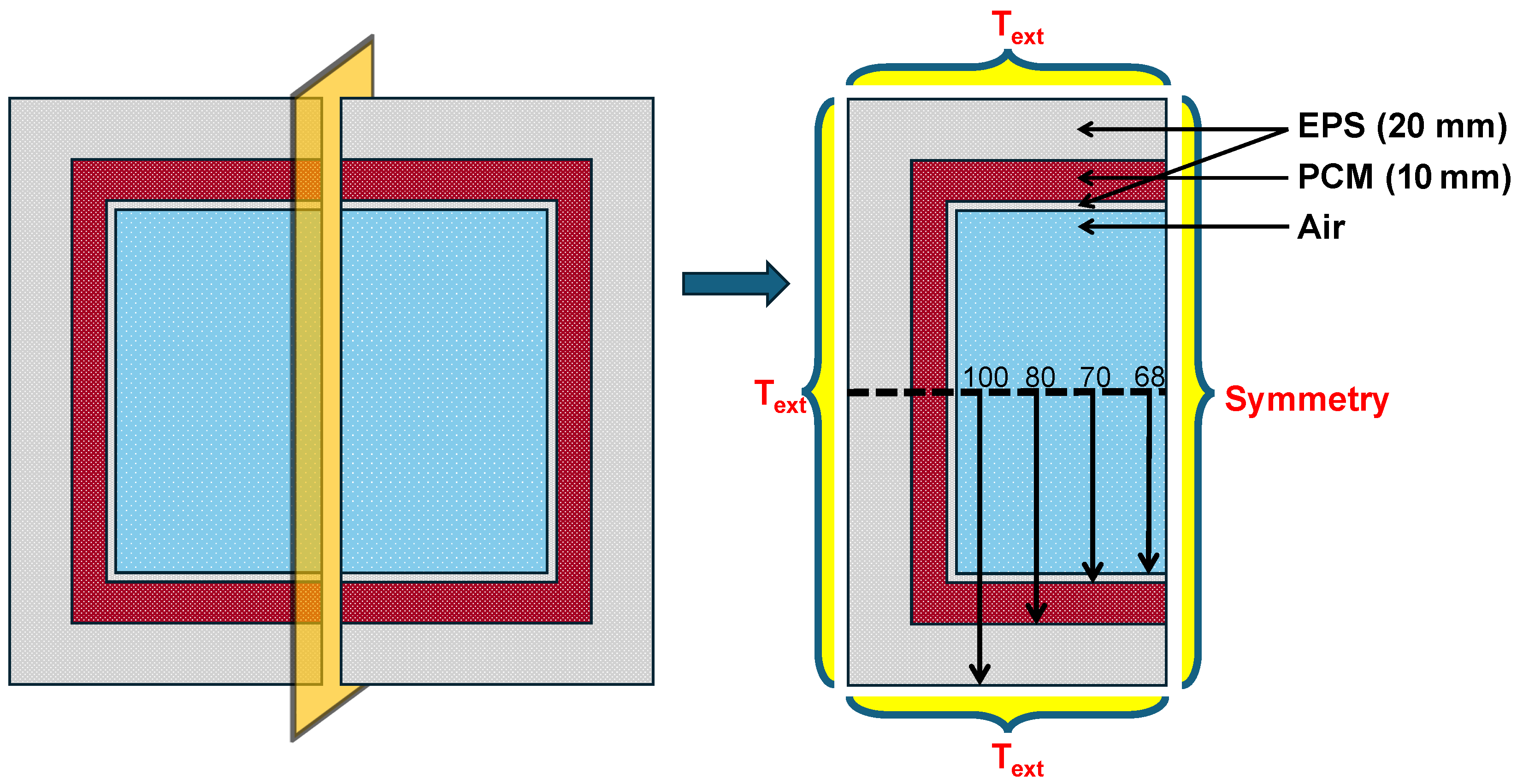
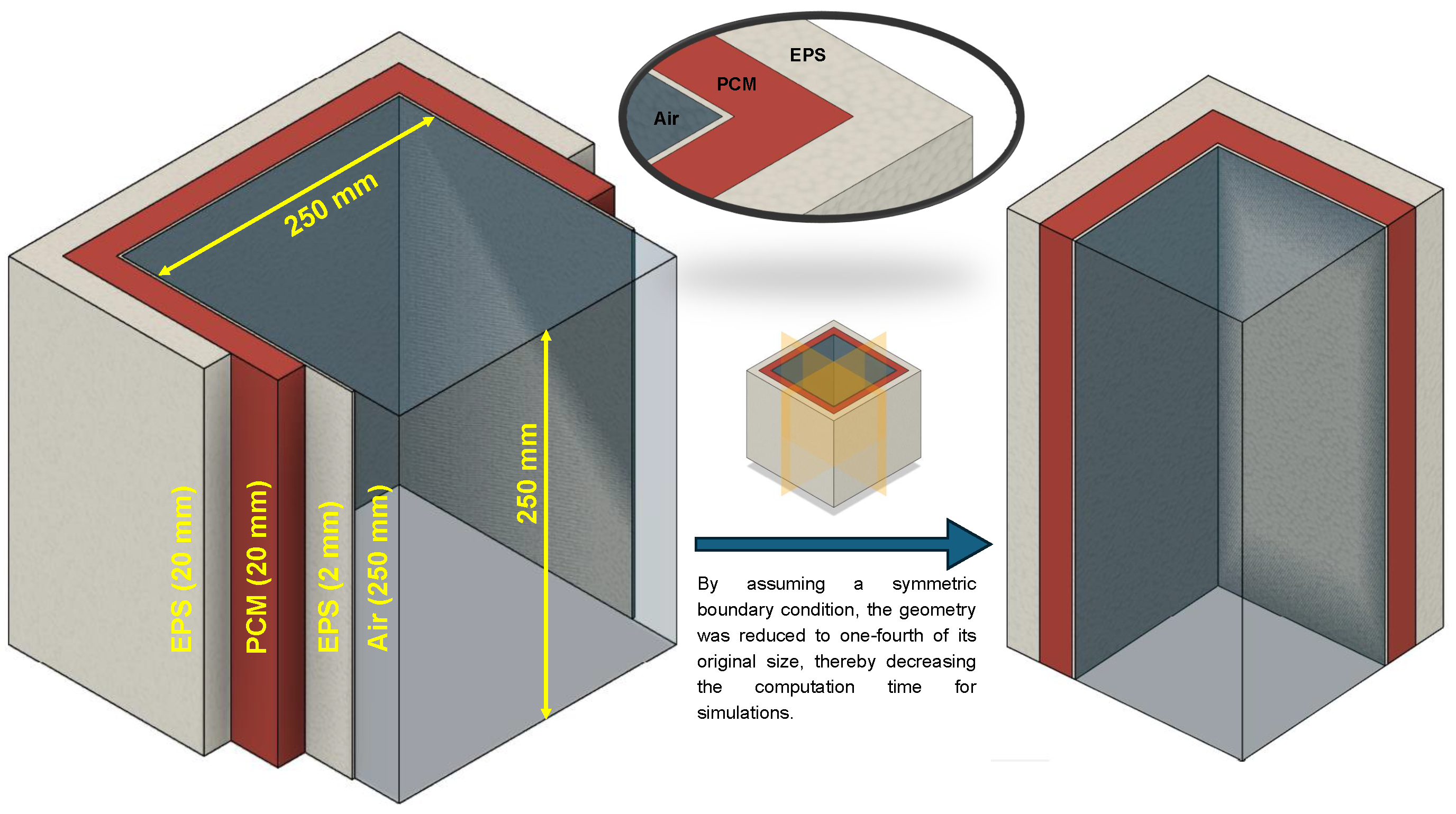
4. Three-Dimensional Model Formulation

After the initial tests, glycerol was re-evaluated under smaller temperature gradients to simulate actual vaccine storage conditions (2–8 °C), and n-tetradecane was chosen as the new PCM instead of SP-50 due to its favorable melting point (5–6 °C) for vaccine transport, high latent heat of fusion, and use in prior studies [27,28,29]. The thermophysical properties of n-tetradecane (T), glycerol, and a mixture containing 5% glycerol (T + G) are provided in

Table 2. Thermophysical properties of n-tetradecane (T), glycerol, and the mixture of 5% volume fraction of glycerol to n-tetradecane (T + G) [22,30,31].

Property	(T)	(G)	(T + G)
${\rho }_{\mathrm{solid}}$ (kg/m3)	775	1261.3	799.32
${\rho }_{\mathrm{liquid}}$ (kg/m3)	762.8	1273.07	788.31
$k_{\mathrm{solid}}$ (W/m·K)	0.35	0.49	0.357
$k_{\mathrm{liquid}}$ (W/m·K)	0.16	0.283	0.16615
$C_{p,\mathrm{solid}}$ (J/kg·K)	2180	1980	2164.22
$C_{p,\mathrm{liquid}}$ (J/kg·K)	2180	2290	2188.88
$L_{\mathrm{mol}}$ (J/mol)	41,959.485	18,280	42,730.03
$L_{\mathrm{kg}}$ (J/kg)	211,500	198,501.466	235,459.07
$T_{\mathrm{solidus}}$ (K)	279.01	291.8	279.39
$T_{\mathrm{liquidus}}$ (K)	279.01	291.8	279.39
$T_{\mathrm{solidus}}$ (°C)	5.86	18.65	6.24
$T_{\mathrm{liquidus}}$ (°C)	5.86	18.65	6.24
M (kg/mol)	0.19839	0.09209	0.18
${\varphi }_{\mathrm{vol}}$	0.95	0.05	1

.

A 3D geometry (see Figure 8) was utilized, with an external temperature of 10 °C and a time step of 10 s (validated through time step independence analysis (see Figure 9) and grid convergence index (GCI), discussed in Section 4.2). Under these conditions, glycerol’s performance was promising, as discussed in detail in the subsequent sections. Similar geometries have also been used in the literature previously [32].

4.1. Initial and Boundary Conditions

Equations (1)–(5) utilize the following initial and boundary conditions:

• The cold box is subjected to an external environment with a temperature of 10 °C across all walls.
• The air contained within the box is maintained at a lower temperature of 2 °C from t = 0.
• The system is subjected to a forced convection current from outside characterized by a heat transfer coefficient of h = 8.5 $\mathrm{W}/{\mathrm{m}}^2·\mathrm{K}$.

4.2. Grid Convergence Index (GCI)

To strenghten our numerical model, a GCI analysis was performed following the guidelines recommended by ASME [33] to assess the accuracy of the numerical solution and ensure grid independence. It relies on the discretization error estimation method known as Richardson extrapolation (RE). For the accurate estimation of discretization errors, the solution must be within the asymptotic range. The RE method requires the exact solution, which is often unavailable in most practical applications [33]. Despite this limitation, the method remains widely adopted in contemporary research and was originally introduced by Richardson in the early 20th century [34,35]. It quantifies the sensitivity of the solution to further mesh refinement and provides an indication of how close the solution is to the asymptotic range. A smaller GCI value suggests that the solution is closer to achieving asymptotic convergence [36].

A representative mesh size is calculated as follows: [37]

$$H={\left[{\displaystyle \frac{1}{N}}\sum _{i=1}^N(\Delta V_i)\right]}^{1/3}$$

(11)

where $\Delta V_i$ represents the volume of the ith cell, and N denotes the total number of cells in the computational domain. Three distinct mesh sizes ($H_1<H_2<H_3$, where 1, 2, and 3 denote fine, medium, and coarse meshes, respectively) were selected, with a refinement factor (r) of 2. Simulations were then performed for variable $\varphi$, which represents the MF here. Typically, r should exceed 1.3, a value determined through practical experience. The refinement should be conducted systematically based on the refinement factor r, even when dealing with an unstructured grid. The refinement factor can be calculated by

$$r={\displaystyle \frac{H_{coarse}}{H_{fine}}}$$

(12)

Following this, an apparent order of accuracy (p) was defined:

$$p={\displaystyle \frac{1}{ln\left(r\right)}}\left|ln\left({\displaystyle \frac{{ϵ}_{32}}{{ϵ}_{21}}}\right)\right|$$

(13)

Here, ${ϵ}_{32}$ = ${\varphi }_3$−${\varphi }_2$ and ${ϵ}_{21}$ = ${\varphi }_2$−${\varphi }_1$. The convergence behavior based on the ratio of errors ${ϵ}_{21}/{ϵ}_{32}$ is as follows: Monotonic convergence occurs when $0<{ϵ}_{21}/{ϵ}_{32}<1$, while oscillatory convergence happens for $-1<{ϵ}_{21}/{ϵ}_{32}<0$. ${ϵ}_{21}/{ϵ}_{32}<-1$ indicates oscillatory divergence, and ${ϵ}_{21}/{ϵ}_{32}>1$ signals monotonic divergence. The grid in this case is made up of uniformly sized rectangular cells with no cell sizes approaching 0. So, the next step is to calculate the error estimates.

For the approximate relative error,

$$e_a^{21}=\left|{\displaystyle \frac{{\varphi }_1-{\varphi }_2}{{\varphi }_1}}\right|$$

(14)

For the extrapolated relative error,

$$e_{ext}^{21}=\left|{\displaystyle \frac{{\varphi }_{ext}^{21}-{\varphi }_1}{{\varphi }_{ext}^{21}}}\right|$$

(15)

Finally, for the fine grid convergence index,

$$GC{I}_{21}^{fine}={\displaystyle \frac{1.25e_{21}^a}{r^p-1}}$$

(16)

Here, 1.25 is the factor of safety. The $GC{I}_{21}^{fine}$ is characterized by the percentage numerical error, with no iterative or rounding errors. The medium grid with 27,306 elements yielded a GCI of 0.34%, demonstrating that the numerical solution exhibits minimal discretization error.

The ratio ${\displaystyle \frac{{ϵ}_{21}}{{ϵ}_{32}}}=0.466$ indicates a decrease in error as the mesh is refined, confirming that the results are converging as expected. The apparent order of convergence $p=1.1025$ also suggests moderate convergence behavior. These findings confirm that the grid refinement successfully minimized numerical errors and the solution was sufficiently accurate using the medium grid for the present study. These findings are presented in

Table 3. Grid convergence table for $F_S=1.25$.

	Grid Convergence Details			Error and GCI
Grid Number	$\mathit{r}={\displaystyle \frac{{\mathit{d}}_{\mathit{k}}}{{\mathit{d}}_{\mathit{k}+\mathbf{1}}}}$	$\mathit{p}={\displaystyle \frac{\mathbf{1}}{\mathit{ln}\left(\mathit{r}\right)}}\left|\mathit{ln}{\displaystyle \frac{{\mathit{ϵ}}_{\mathbf{32}}}{{\mathit{ϵ}}_{\mathbf{21}}}}\right|$	$\mathit{\varphi }$	${\displaystyle \frac{{\mathit{ϵ}}_{\mathbf{21}}}{{\mathit{ϵ}}_{\mathbf{32}}}}$	GCI (%)
3990			0.101
27,306	2	1.1025	0.1017	0.466	0.34
201,042			0.102

Note: The grid convergence index (GCI) helps determine the proximity of the solution to the asymptotic range.

.

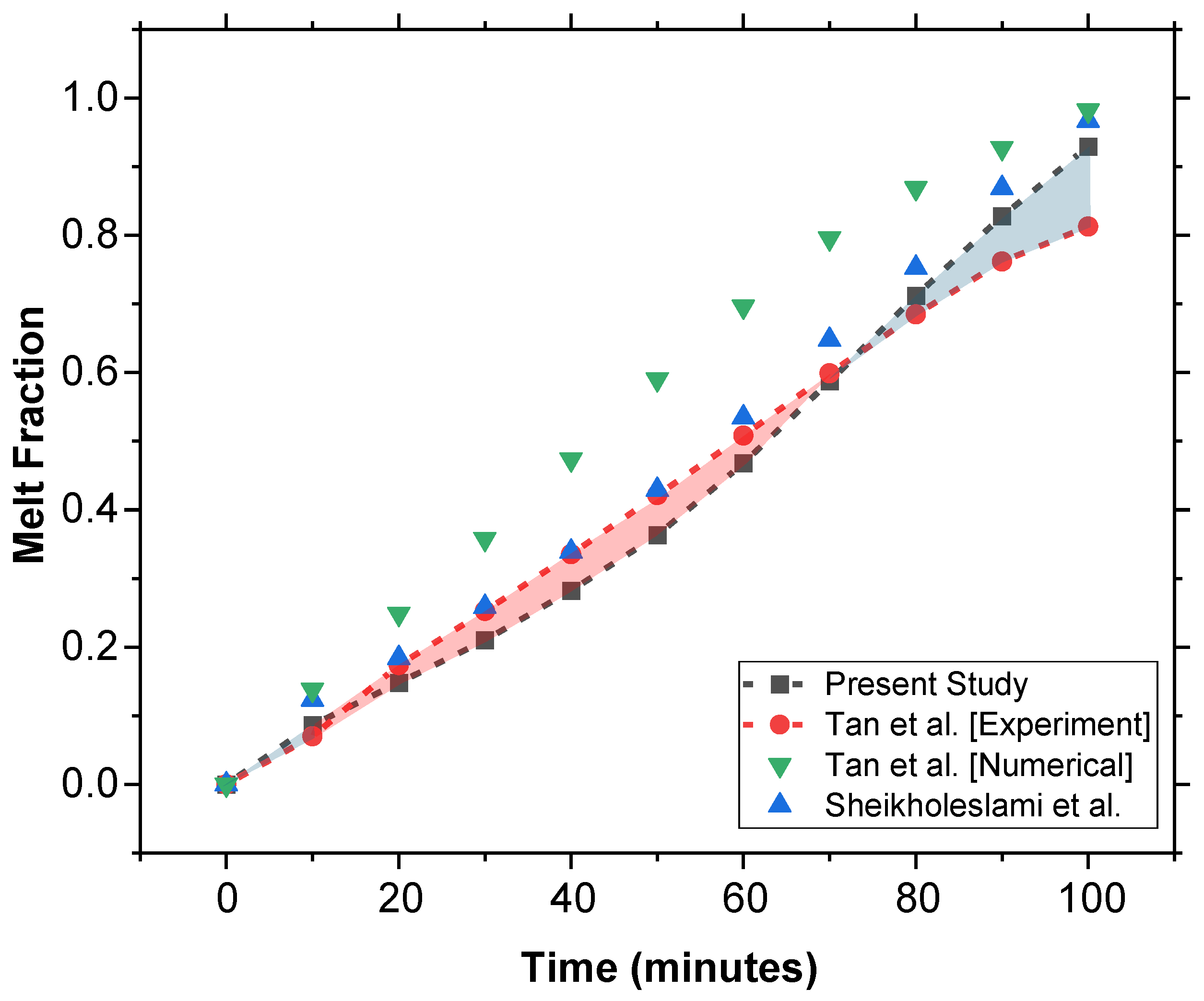
4.3. Validation

The present study was validated using the experimental and computational study on constrained melting of PCM in a spherical cavity in [38]. As shown in Figure 10, the MF vs. time results of both the experimental and numerical data from [38], as well as the numerical results from Sheikholeslami et al. [39], have been plotted.

Our numerical model shows better agreement compared to the numerical model of Tan et al. [38]. This improvement in our results highlights the accuracy of our model in predicting the phase change behavior of PCM and thus validates our approach.

5. Discussion

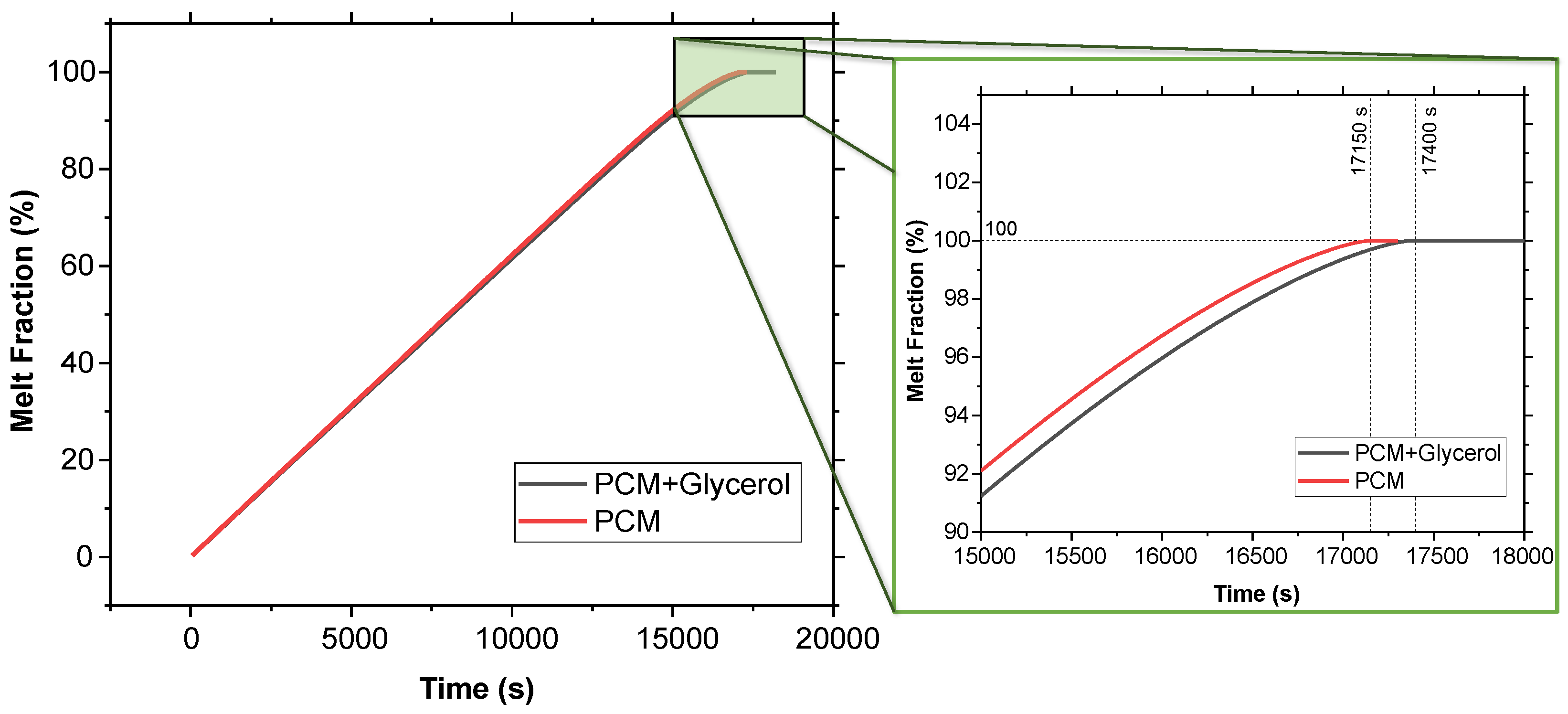
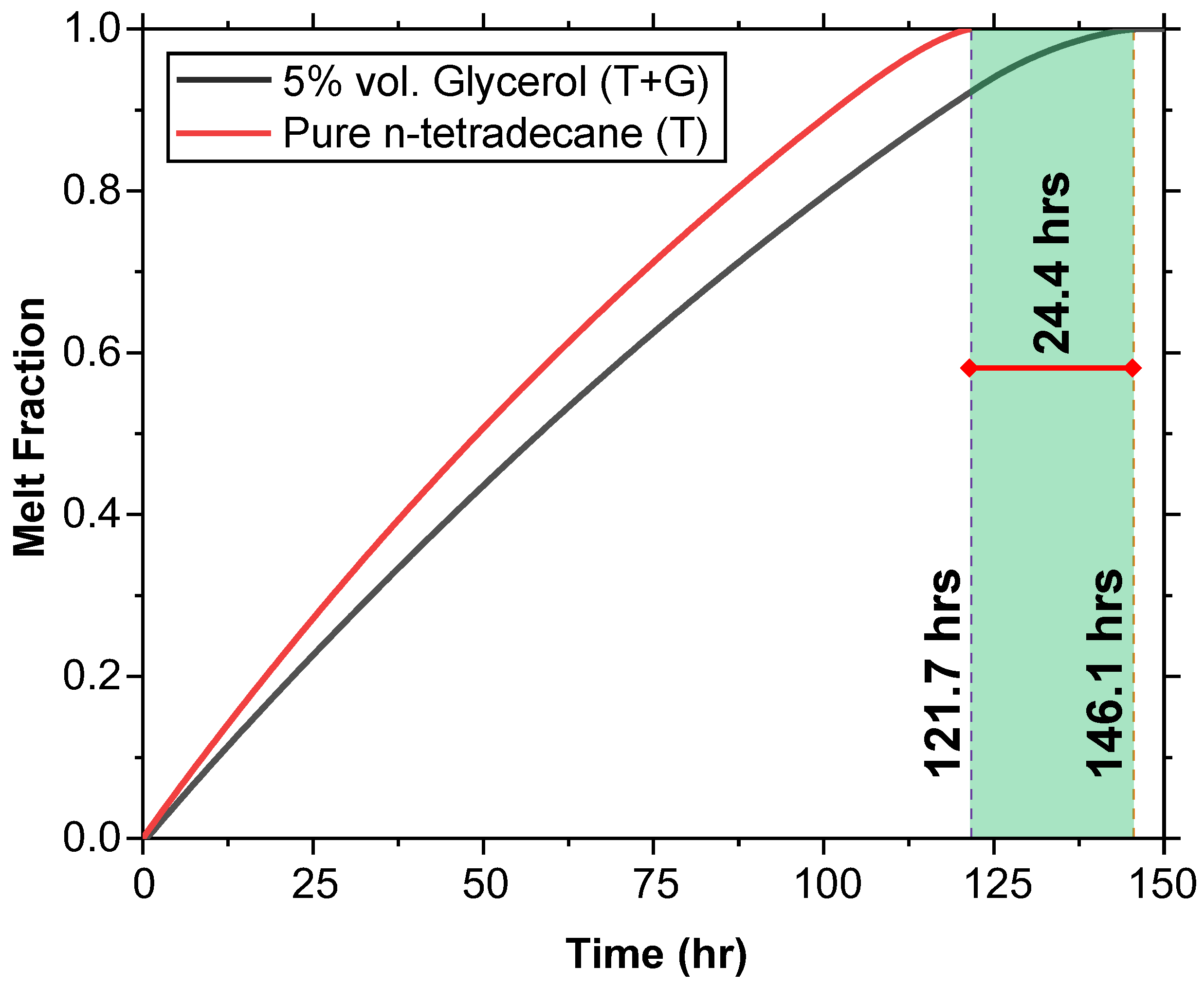
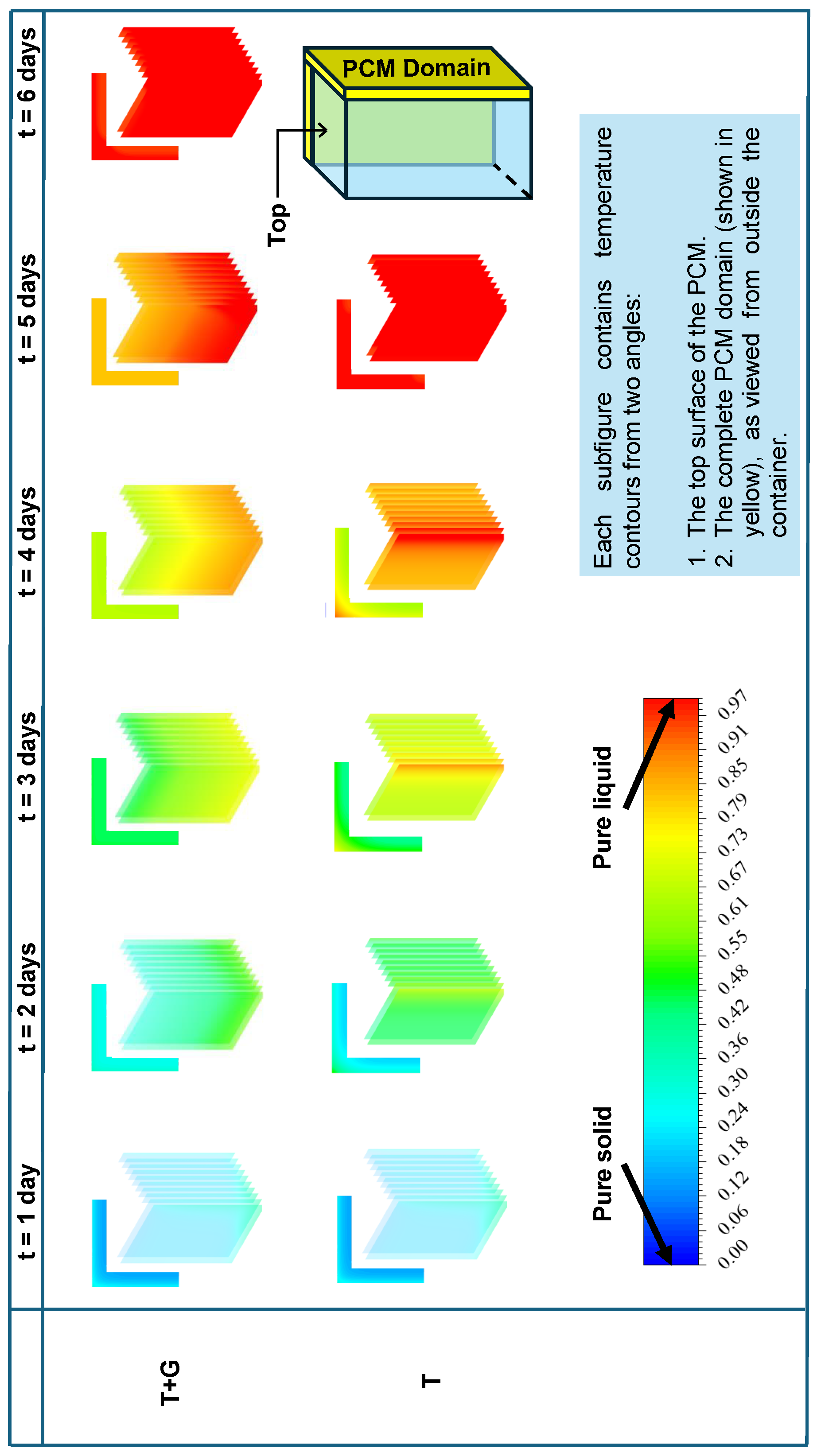
5.1. Melting Behavior of n-Tetradecane and n-Tetradecane with Glycerol

Pure n-tetradecane (T) and n-tetradecane with 5% glycerol (T + G) were heated from an initial temperature of 2 °C to observe their melting behavior under external heat. The melt fraction (MF) versus time curve (Figure 11) shows that T fully melts in 121.7 h, while T + G takes 146.1 h, providing an extension of 24.4 h.

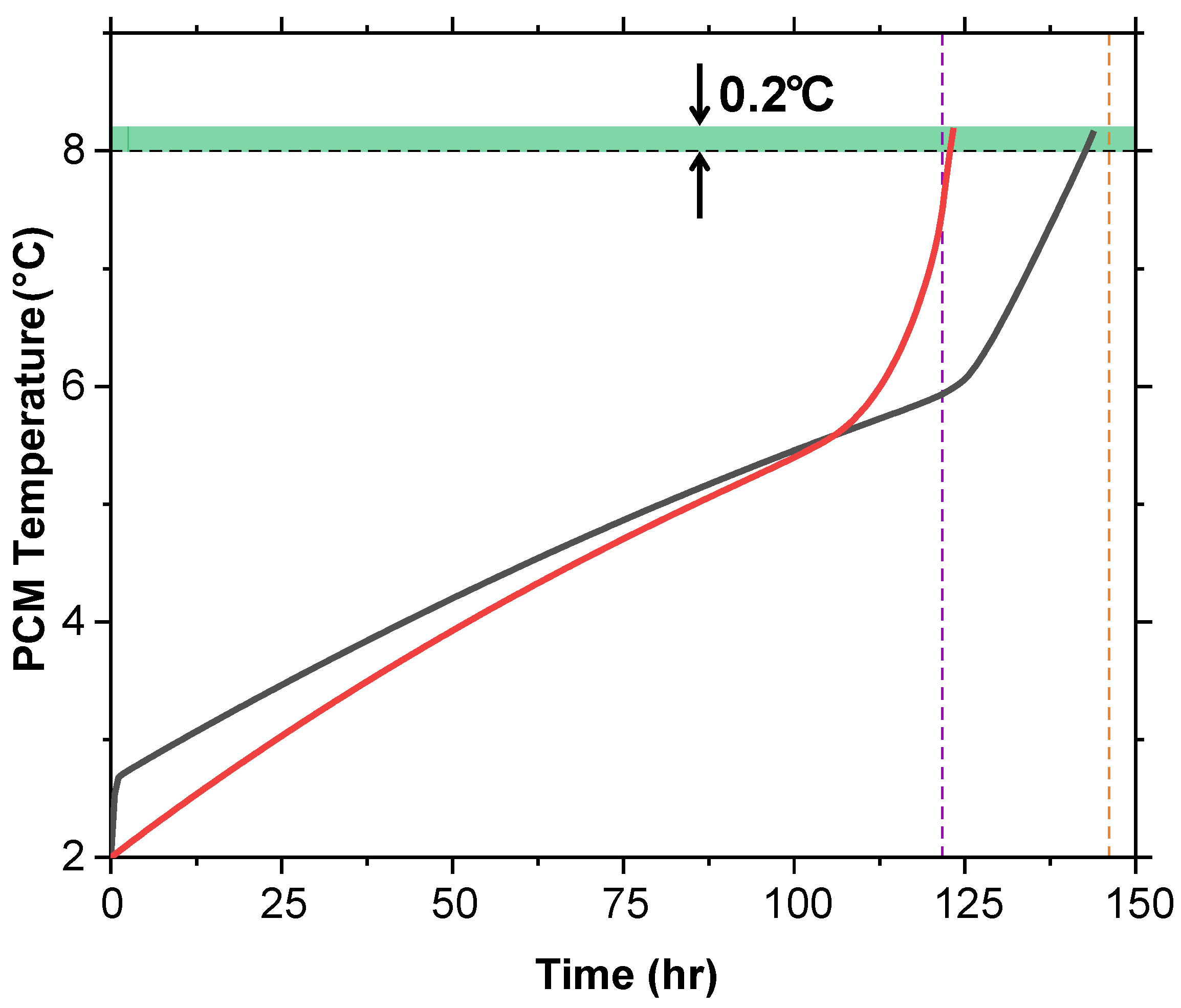
5.2. Internal Air Temperature and True Extension of Cooling

Although the MF reaching 100% does not guarantee the preservation of vaccine efficacy, the internal air temperature offers a more accurate measure of temperature control within the cold box. The average air temperature during the melting process is shown in Figure 12. It was observed that for T, the internal air temperature reaches the critical 8 °C threshold 1.6 h after the MF reaches 100%. For T + G, the air temperature reaches 8 °C approximately 2.2 h before the MF reaches 100%. Therefore, the true extension provided by T + G is approximately 20.6 h, not the full 24.4 h indicated by the MF.

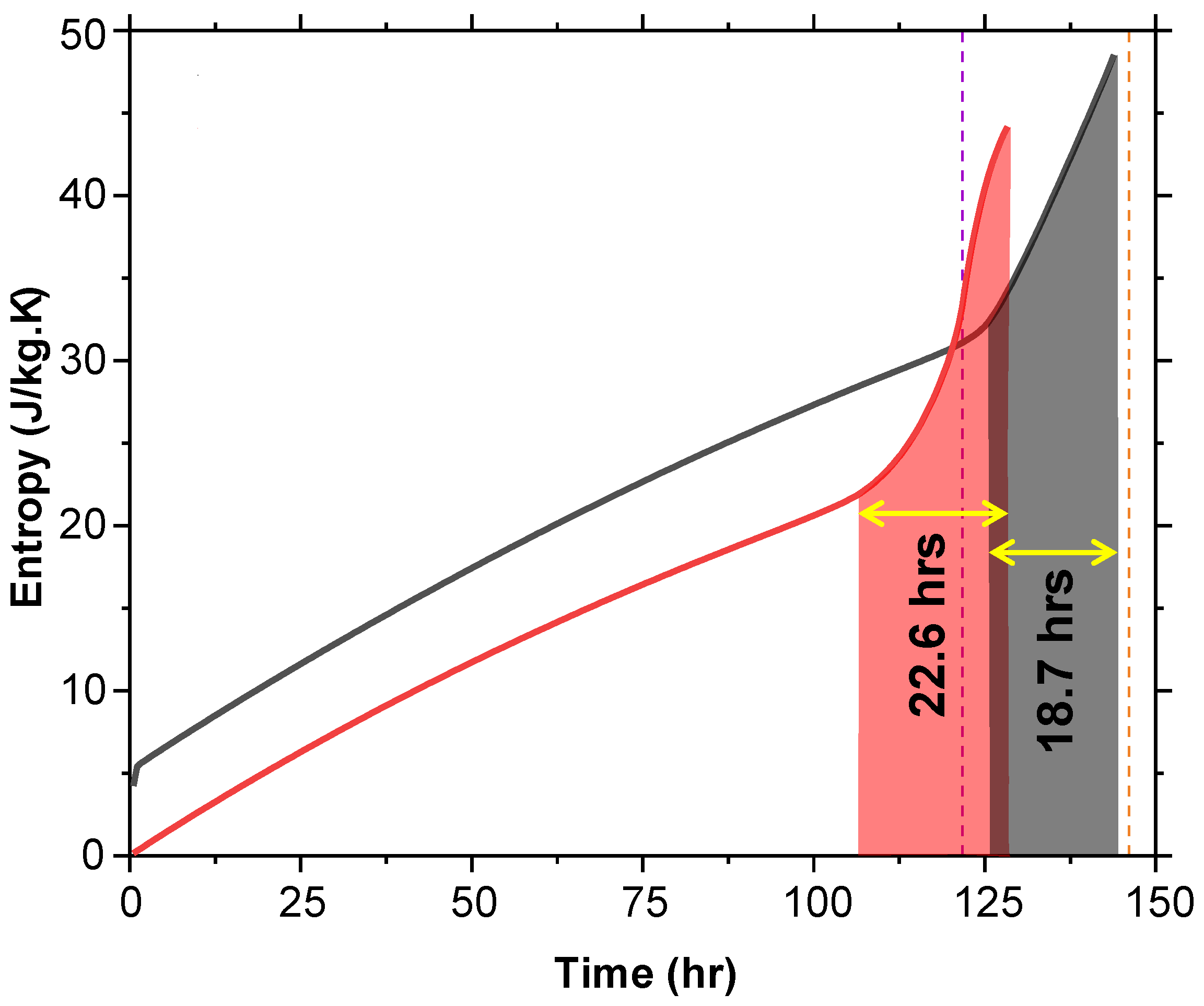
5.3. Entropy Analysis

Entropy, a measure of molecular disorder, was analyzed over time (Figure 13). Initially, T + G has a higher entropy value, starting 4.1 J/kg·K above T at time zero. This initial disorder is due to the 5% glycerol content. As melting progresses, entropy increases steadily, with a steep rise observed as the system approaches 100% MF or when the air temperature nears 8 °C. For T, the steep rise in entropy begins approximately 22.6 h before the air temperature reaches 8 °C, whereas for T + G, this rise occurs around 18.7 h before reaching the same threshold. The nearly 4-h difference suggests that glycerol delays the onset of the rapid entropy increase, effectively extending the duration of thermal stability in T + G.

5.4. Temperature Dynamics of the PCM Domain

The average temperature of the PCM domain was plotted against time during the melting process (Figure 14). Both T and T + G slightly exceed 8 °C by around 0.2 °C before the internal air temperature catches up. This is due to the PCM being on the exterior of the cold box, absorbing more heat from the surroundings than the internal air cavity.

5.5. Temperature Contours of the Cold Box

Figure 15 presents the temperature contours at the end of each day for both the T and T + G cases. To reduce computation time, a quarter geometry was employed for the 3D model. The contours are shown from two perspectives: the top adiabatic surface and the external side of the PCM domain. The results indicate that T reaches complete melting by the end of day 5, whereas T + G continues to sustain cooling until the end of day 6.

5.6. Potential Limitations of Glycerol as an Additive

While our study primarily focuses on the thermal performance improvements achieved with glycerol as an additive in n-tetradecane, we acknowledge the importance of addressing its broader implications for practical implementation. Below, we discuss key considerations:

(a) Cost–Benefit Analysis: Glycerol is an economically viable and widely available substance, often produced as a byproduct of biodiesel production. However, the long-term economic feasibility, particularly for large-scale applications in cold chain logistics, warrants further exploration.

(b) Feasibility of Large-Scale Production: With global production exceeding 5 million metric tons annually, glycerol is readily available for industrial applications. Nevertheless, challenges such as transportation, storage, and integration into PCM systems on a large scale need additional study.

(c) Long-Term Effects on Thermophysical Properties: Additives like glycerol may influence the thermophysical properties of PCMs over multiple thermal cycles, including latent heat, thermal conductivity, and phase-change behavior. While glycerol’s chemical stability is well established, further investigations using techniques such as differential scanning calorimetry (DSC) and thermal cycling experiments are essential to assess potential phase separation or degradation over extended usage cycles.

Addressing these aspects in future research will enhance the practical applicability of glycerol-enhanced PCMs and facilitate their integration into sustainable vaccine transport systems.

5.7. Long-Term Stability of the PCM–Additive Mixture

The current study focuses on the immediate thermal and melting behavior of the PCM–additive mixture. However, assessing its performance over multiple melting and solidification cycles is critical for practical applicability. To explore this, the following analyses can be considered for future work:

(a) Cyclic Thermal Stability Tests: The PCM (pure and with 5% glycerol additive) can be subjected to repeated thermal cycles simulating real-world conditions. Critical properties such as melting point, solidification point, latent heat, and thermal conductivity can be monitored over 50–100 cycles.

(b) Chemical Stability Assessment: Potential chemical degradation or phase separation could be evaluated using techniques like Fourier transform infrared spectroscopy (FTIR) and differential scanning calorimetry (DSC) to detect changes in chemical composition or latent heat.

(c) Performance Analysis Post Cycling: The melting duration, temperature maintenance, and entropy behavior could be reassessed after multiple cycles to determine if the additive retains its efficacy over time.

(d) Comparison with Baseline PCM: The long-term stability of the PCM with glycerol could be compared to that of the pure PCM to identify any adverse effects introduced by the additive.

These supplementary investigations could provide valuable insights into the additive’s long-term reliability, enhancing the practical relevance of this study. Although beyond the scope of the current work, these aspects could form a key focus of future research.

5.8. Regulatory Compliance of Glycerol-Modified PCM

The glycerol-modified PCM is designed to align with established regulatory guidelines to ensure adherence to health and safety standards:

(a) World Health Organization (WHO) Guidelines: The WHO’s Vaccine Storage and Handling Toolkit emphasizes maintaining recommended temperature ranges to preserve vaccine efficacy. The glycerol-modified PCM is engineered to maintain these temperature ranges during transportation. (https://www.cdc.gov/vaccines/hcp/admin/storage/toolkit/storage-handling-toolkit.pdf (CDC Toolkit) accessed on 25 January 2025).

(b) U.S. Food and Drug Administration (FDA) Recommendations: Glycerol is classified as Generally Recognized as Safe (GRAS) by the FDA, indicating its suitability for applications related to human health. (https://www.fda.gov/food/gras-notice-inventory/recently-published-gras-notices-and-fda-letters (FDA GRAS Notice Inventory) accessed on 25 January 2025).

(c) International Air Transport Association (IATA) Regulations: The PCM complies with the IATA Temperature Control Regulations (TCR), which provide comprehensive requirements for the transportation of temperature-sensitive healthcare products. This ensures the integrity of vaccines during air transport. (https://www.iata.org/en/publications/manuals/temperature-control-regulations/ (IATA TCR) accessed on 25 January 2025).

In adhering to these guidelines, the glycerol-modified PCM complies with current regulatory frameworks and maintains the highest standards of health and safety for vaccine transportation.

6. Conclusions

• In n-tetradecane, 5% glycerol extends the melting time and improves the temperature regulation for vaccine delivery.
• Glycerol increases cooling time by 20.6 h, maintaining the 2–8 °C range.
• Initial tests showed polystyrene foam as the least effective additive, while glycerol slowed melting the most.
• Glycerol’s effect on SP-50 at 0 °C showed minimal improvement.
• In 3D model tests with n-tetradecane at 10 °C, glycerol extended the melt time by 24.4 h, prolonging inner temperature maintenance by 20.6 h.
• Figure 16 outlines the step-by-step process of this study.