1. Introduction
Thorough analyses of satellite observations have repeatedly shown variations in the wind stress at the ocean surface on length scales comparable to mesoscale ocean eddies (e.g., [
1,
2,
3]). The range of processes responsible for these mesoscale wind stress variations, and the range of effects that these variations have on atmospheric and oceanic dynamics and on ocean biogeochemistry have been discussed by many authors; Small et al. [
4] and Chelton and Xie [
5] provide reviews and References [
6,
7,
8,
9] provide an incomplete sampling of more recent work. This investigation focuses on two causes of wind stress variations—ocean surface velocity and temperature anomalies—and their impact on the large-scale ocean circulation.
Pacanowski [
10] argued that the wind stress
in an ocean model should depend on both the winds
and the ocean surface velocity
through a relation of the form
where
is the density of air and
is a dimensionless drag coefficient. Duhaut and Straub [
11] and Dawe and Thompson [
12] found that this leads to a significant reduction in the net power input by the winds, and that the large-scale circulation is accordingly weakened when compared with the alternative formulation where
is assumed negligible. Duhaut and Straub [
11] showed that the variability in wind stress reduces the net power input by directly removing energy from the ocean mesoscale.
Hogg et al. [
13] modeled the effect of sea surface temperature (SST) on wind stress through a relation of the form
where
is the atmosphere–ocean temperature difference. They found that this also leads to a significant reduction in the strength of the gyre circulation. They argued that transient Ekman pumping anomalies near the western boundary current separation point destabilize the flow; the unstable jet then produces a downgradient flux of potential vorticity (PV) between the gyres, causing the circulation to weaken. More recently, Byrne et al. [
9] found that thermodynamic air–sea coupling at the ocean mesoscale in a coupled model enhances the transfer of wind energy to the ocean.
The current investigation considers both of these ocean influences on wind stress, ocean velocity and SST, and on how the resulting wind stress variations impact the large-scale ocean circulation. As in [
11,
13], we frame our investigation in the context of the classical wind-driven quasigeostrophic (QG) double-gyre. We find, as expected, that both the SST and ocean velocity dependences cause the net wind power input to decrease. The two dependences do not have an additive effect: the impact of the SST dependence is reduced when considered in combination with the ocean velocity dependence. We also find that SST dependence tends to shrink the subtropical gyre at the expense of the subpolar gyre, with an associated southward shift of the inter-gyre jet. This southward bias leads to a new explanation for the way in which SST dependence leads to reduced wind power input: the net wind power input is proportional to the product of the stress and the surface currents
, and the southward shift of the jet leads to a reduction in the stress–current alignment, which results in reduced power input. The southward shift of the jet itself is explained by an SST-induced wind stress anomaly that accelerates the southern edge of the jet and decelerates the northern edge, pushing the jet south.
In
Section 2, we describe the formulation and configuration of our QG model with active SST and interactive wind stress.
Section 3 analyzes the model output, and
Section 4 discusses the results.
3. Results
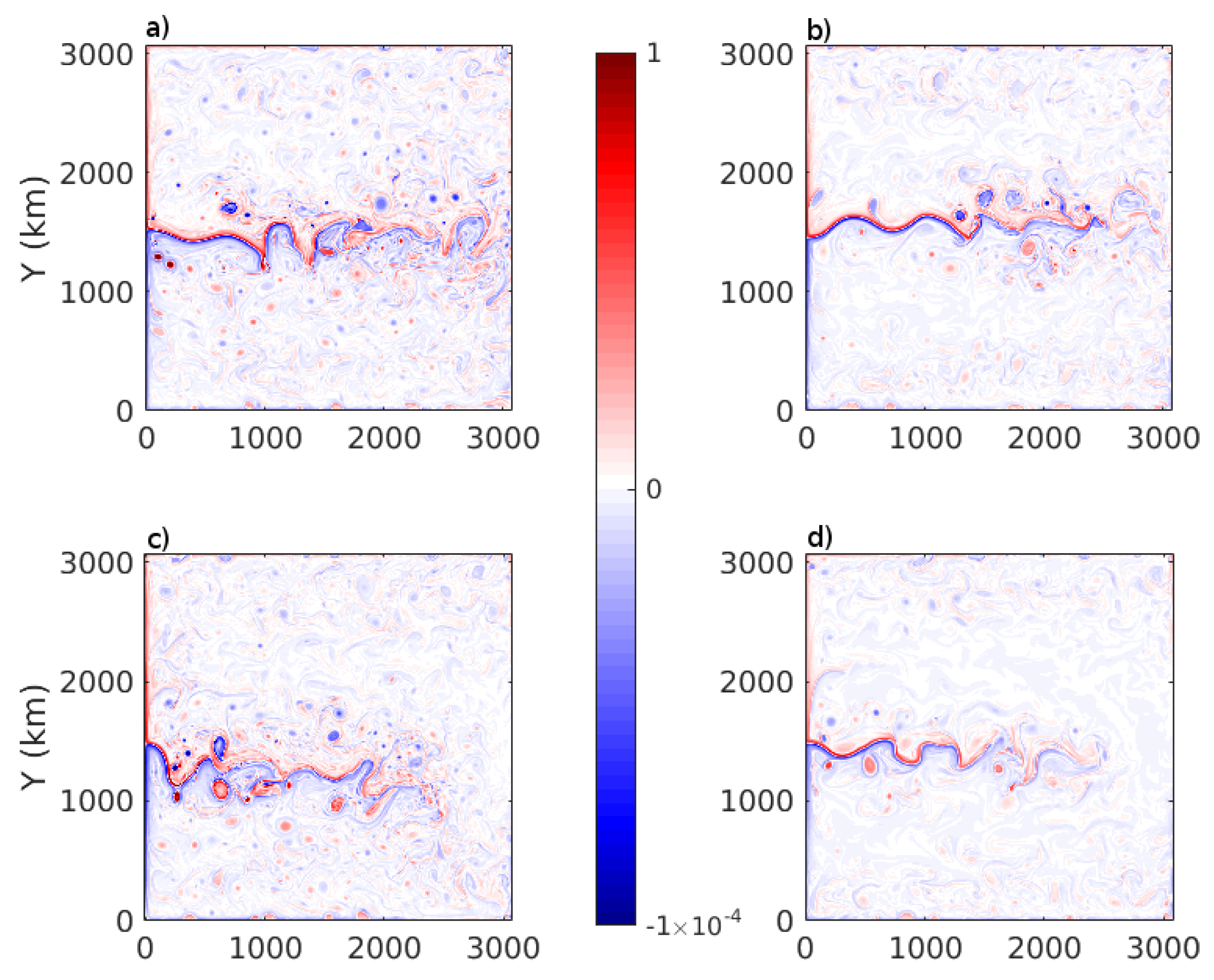
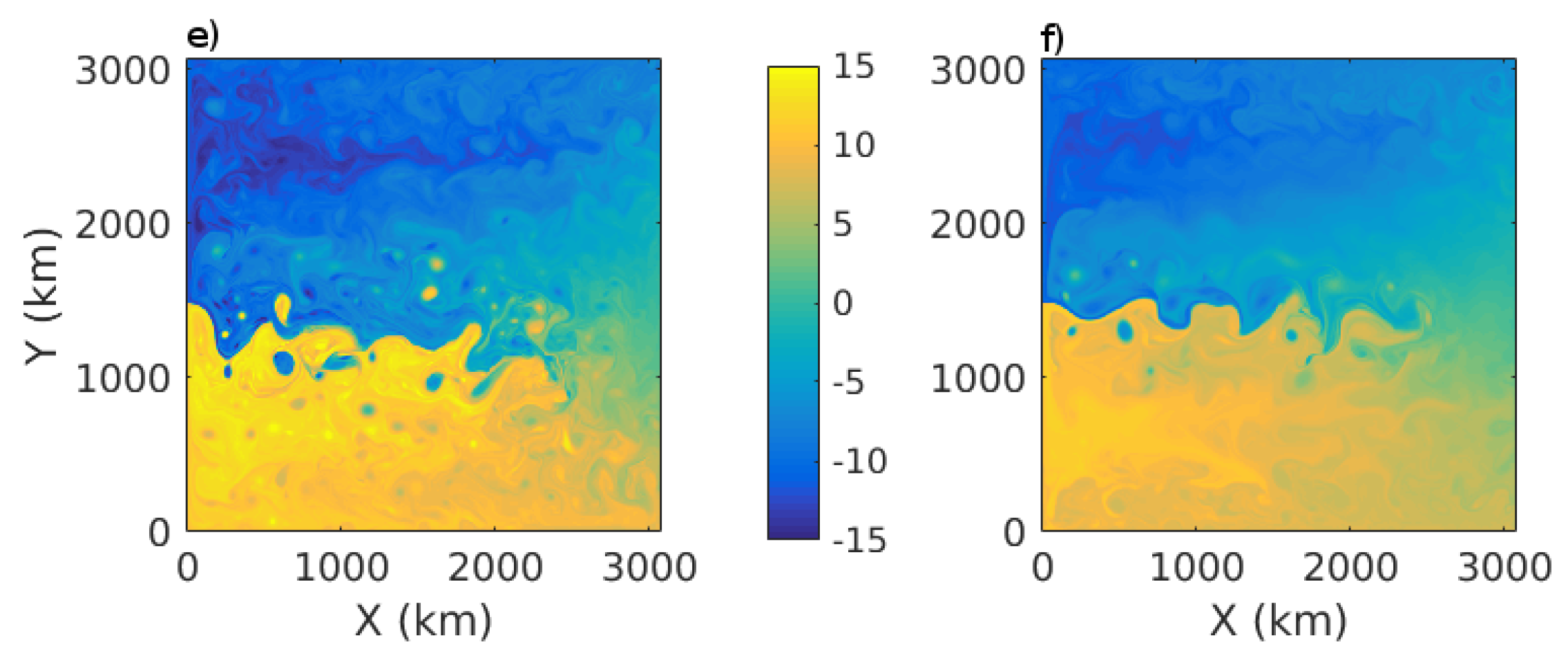
We begin our investigation by considering, in
Figure 1, typical snapshots from the four configurations. In each case, surface relative vorticity patterns (panels a–d) are characteristic of a double gyre flow: two western boundary currents merging into an inter-gyre jet, continually destabilized to produce a rich eddying field. The eastern extension of this meandering jet varies according to the wind stress formulation, decreasing from case
I to case
IV. The QG SST perturbation (
Figure 1e,f) covers a range of about 30
K, which corresponds roughly to the observed SST range of the polar/sub-polar gyre system of the Atlantic and Pacific. An asymmetry about
is visible on some snapshots of relative vorticity and SST perturbation in
Figure 1. This asymmetry will be discussed in more detail later in this section.
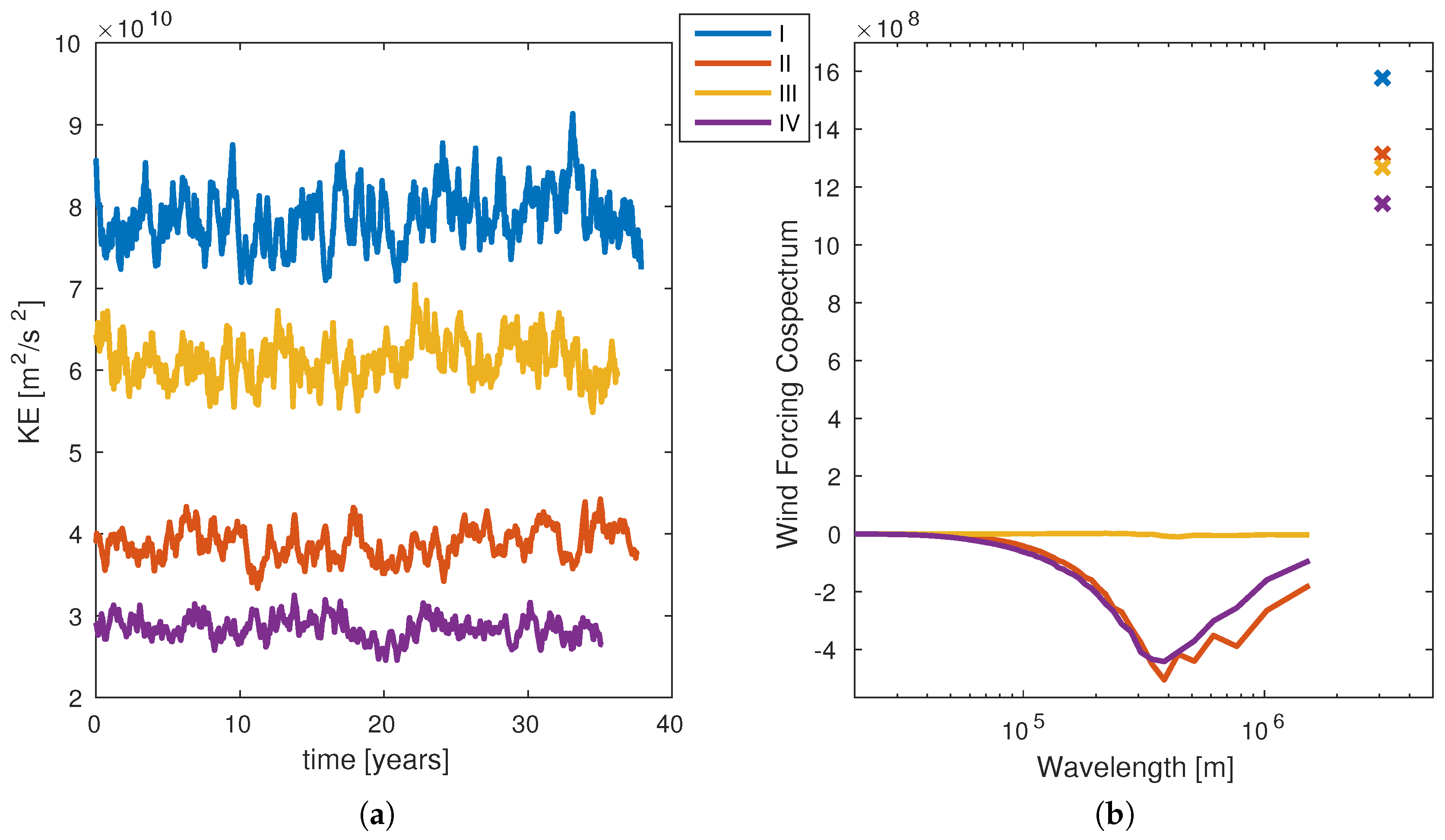
Figure 2a shows time series of the total kinetic energy at statistical equilibrium for all four cases. Consistent with the results of several previous studies, the use of the surface velocity dependent formulation (
1) decreases significantly the total kinetic energy—by roughly half. The SST dependent formulation (
2) has a similar but weaker effect.
To understand the origin of these differences in energy between each wind formulation, we now focus on the power input from the surface wind. Recall that the energy equation is obtained by multiplying the evolution equations for
,
and
by
, then summing over the layers and integrating over the domain. The wind power input is therefore
Expanding both
and
in Fourier series, we can write the wind power input as
up to an arbitrary normalization constant that can be absorbed into the definition of the Fourier coefficients
and
. Superscript
denotes the complex conjugate, and
are wavenumbers in the
x- and
y-directions. The rescaled wavenumbers
are integers, and the sum in Equation (
30) can be re-arranged into a sum over wavenumber shells as follows:
where
is the one-dimensional wind power cospectrum, shown for each case in
Figure 2b. By definition, the non-interactive wind stress (
I) only injects energy at the largest scale (notice that for each case, the value of the power input at the largest scale has been divided by 10 to fit the vertical axis on
Figure 2b). The cospectrum breaks the total wind power input into contributions from different spatial scales; and the total power input is obtained by summing the cospectrum. Negative values indicate that the wind is removing energy from the oceans at a particular length scale.
The ocean velocity dependent formulation (
II) has two effects on the energy input: it decreases the power input of the largest scale and it removes energy from the smaller scales. The peak of this energy sink occurs at a wavelength of about 300 km, slightly larger than the wavelength of the largest internal Rossby radius. In contrast,
Figure 2b shows that the SST dependent formulation does not remove energy at the smaller scales and only affects directly large-scale wind power input. In this case, the spectral decomposition of power input for independent snapshots have a non-zero contribution from the smaller scales. However, these contributions cancel each other when the time-mean is applied.
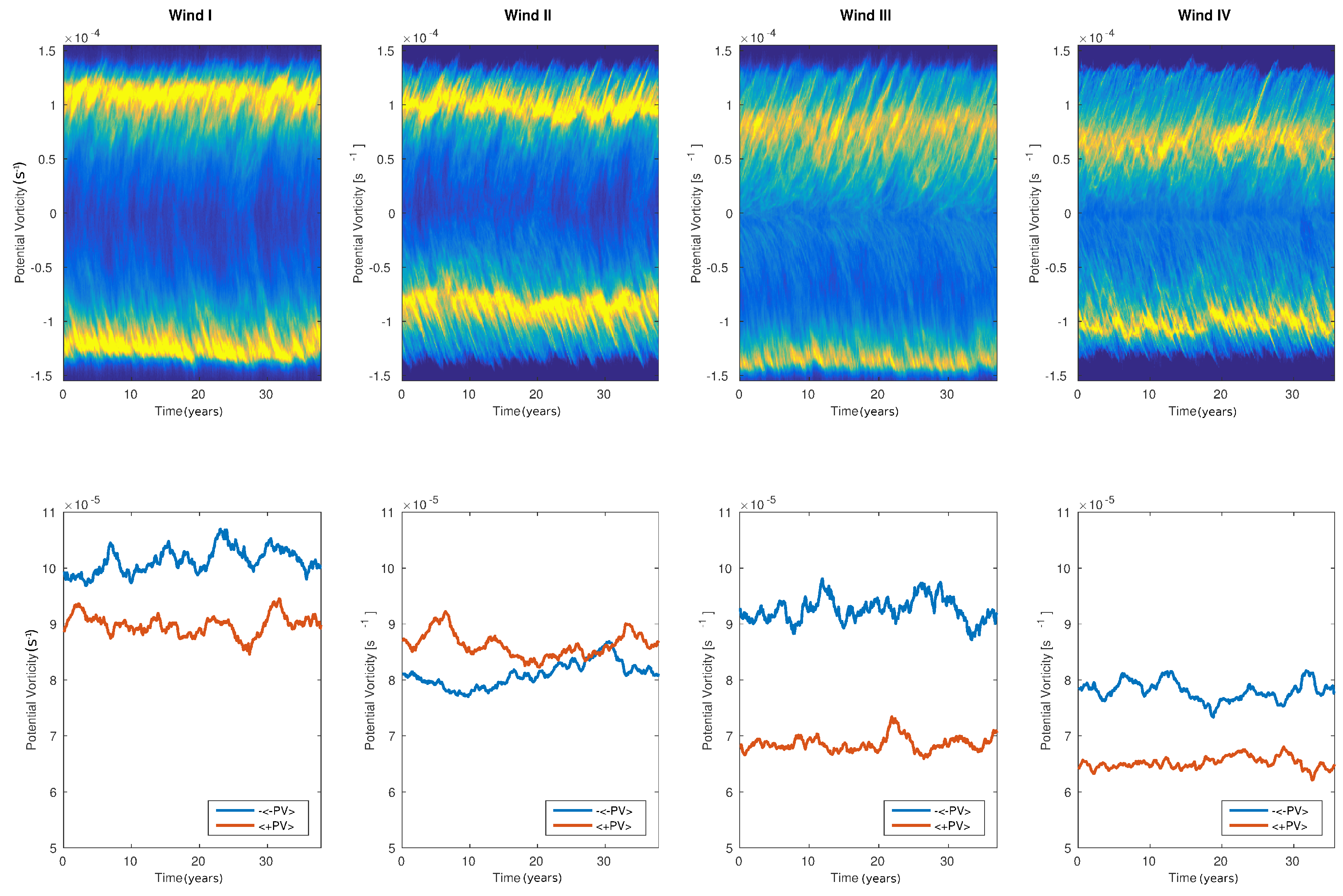
We now focus on the gyres asymmetry about
, visible in
Figure 1. As a useful way to quantify this asymmetry, we show in
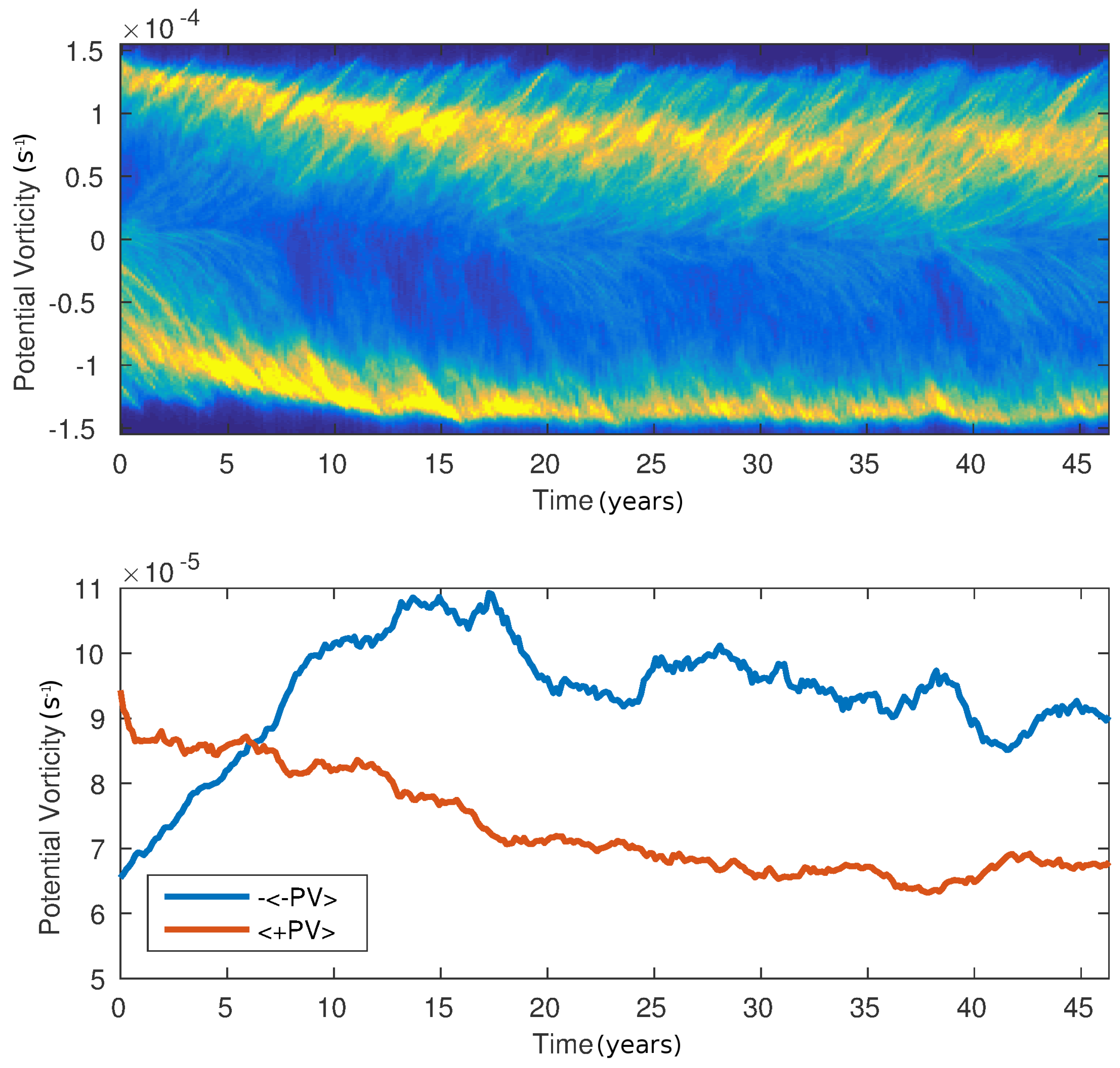
Figure 3, the temporal evolution of the distribution of surface pseudo PV,
. The lower panels shows the absolute value of the average positive and negative PV. In each case, distributions of positive and negative PV show some level of asymmetry. However, the details of the disparity between the mean and skewness of each distributions varies widely for each independent cases.
In the case of non-interactive wind stress, asymmetric solutions have been observed in several other studies with similar double gyre setup (e.g., [
24,
25,
26]). These studies show that although asymmetric solutions occur spontaneously, they are generally unstable for high Reynolds numbers and the system can thus oscillate between several “preferred phenomenological regimes” [
26]. The QG equations with symmetric wind forcing are invariant under the following symmetry:
,
(
). The different preferred states are associated with a spontaneous symmetry breaking, but because of the symmetric invariance of the QG equations, the solutions can oscillate equally between the northward-shifted and southward-shifted preferred states. These oscillations occur on a long-term scale, and are not visible in our simulations. Ocean velocity dependent wind stress does not break the symmetry of the equations, and Duhaut and Straub [
11] have shown that this feature of the circulation is not enhanced or modified in the case of ocean velocity dependent formulation of the wind stress (Wind
II). In contrast, the addition of SST dependence to the wind stress formulation breaks the aforementioned symmetry of the QG equations. In the following, we show that the SST dependent formulation (
2) induces a systematic asymmetry leading to a southward slanting and shift of the inter-gyre jet.
In principle, it could be the case that a northward-shifted regime still exists for the case with SST dependent wind stress, and that our simulation simply shows one of many possible regimes. To test this, we ran a simulation with SST-dependent wind stress (Wind
III), and with an initial condition taken from a previous simulations, but transformed according to
(flipped north–south) and
(reversed). If a northward-shifted regime exists for the SST dependent case, then this simulation should remain northward-shifted, perhaps after a period of adjustment.
Figure 4 shows a Hovmöller diagram similar to the ones of
Figure 3 but for the simulation with sign reversed and flipped initial conditions. The southern gyre rapidly shrinks and strengthens, as seen in the rapid increase of mean negative PV, while the northern gyre slowly dilates and weakens, as seen in the slow reduction of mean positive PV. After approximately 25 years, the asymmetry of the initial condition has reversed, and the simulation has returned to a southward-shifted state.
For comparison, we ran a simulation with independent wind stress (Wind I) where the initial condition was flipped and reversed. Unlike the simulation with SST dependent wind stress, this simulation remained in a northward-shifted state for the length of the simulation. In principle, the simulations with SST independent wind stress can transition between northward-shifted and southward-shifted states, but this happens on a very long-term scale. In contrast, the simulations with SST dependent wind stress have a clear preference for a southward-shifted state.
Physical Mechanism
We propose that SST dependence causes the jet to shift southward because the SST anomaly induces a wind stress anomaly over the jet that accelerates the southern flank and decelerates the northern flank. The jet is extremely unsteady; thus, to track the wind stress over the jet, we perform a jet-centered time average as follows. We first compute the instantaneous location of the maximum zonal velocity along a line 150 km east of the western boundary. This location is considered to be the center of the jet. The zonal velocity, SST perturbation
, zonal wind stress
, surface pseudo-PV
, and Ekman pumping
are all time-averaged in a moving coordinate frame centered on the jet.
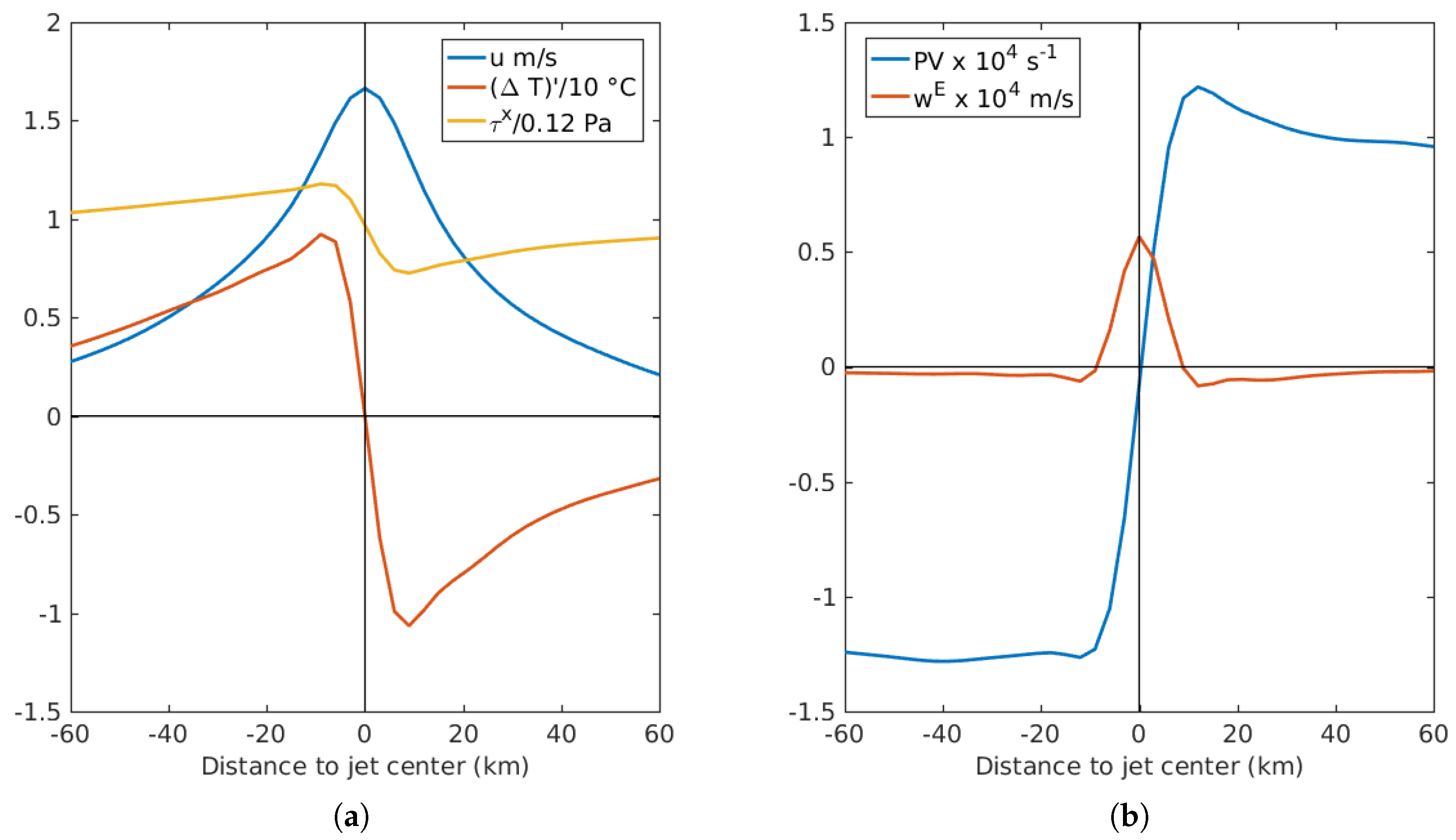
Figure 5a shows the jet-centered time-mean zonal velocity profile, SST perturbation, and zonal wind stress; a positive wind stress anomaly on the southern flank and a negative anomaly on the northern flank are clearly visible.
The wind stress anomaly is explained as follows. The SST front is centered on the jet, as shown in
Figure 5a, and the atmospheric zonal wind profile
varies on a much larger scale than the jet or the SST front. Equation (
2) then implies that the local wind stress anomaly takes the form
The SST perturbation is positive on the southern flank of the jet and negative on the northern flank, leading to a wind stress perturbation with the same pattern.
The effect can also be viewed from the perspective of PV.
Figure 5b shows the jet-centered time-mean surface pseudo-PV front and Ekman pumping. The Ekman pumping is derived from the meridional derivative of the zonal wind stress, which is dominated by the short-scale variation of the wind stress anomaly, and, as a result, there is a positive Ekman pumping anomaly centered on the PV front. The Ekman pumping appears as a forcing term in the surface PV equation; thus, the positive anomaly tends to shift the front to the south.
4. Discussion and Conclusions
The primary point of comparison for our results is the work of Hogg et al. [
13], who also found that SST dependent wind stress leads to reduced net wind power input, and who observed a southward shift of the jet. Though they also considered the impact of SST dependent wind stress on a QG double-gyre circulation, there are several differences in model configuration worth reviewing. The major differences in comparison with the present study are that they used an atmospheric mixed layer model and defined
as the atmosphere–ocean temperature difference, and that they used an ocean mixed-layer model for SST rather than identifying it with the QG surface buoyancy anomaly. Despite these and other differences in the models, the main qualitative results—reduced wind power input, and a southward-shifted jet—are the same.
We propose a mechanism to explain the reduced wind power input that is different from the mechanism proposed by Hogg et al. [
13]. The wind power input is proportional to the domain-integrated scalar product of surface velocity and wind stress
(up to small corrections due to boundary stresses). The wind forcing cospectra in
Figure 2 indicate that wind power input is dominated by the largest scales, and that the SST dependence causes a reduced wind power input at the largest scales, with no net effect on smaller scales. As shown in
Figure 5, SST dependence causes a wind stress anomaly that pushes the jet south and leads to asymmetric gyres. This wind stress anomaly is small-scale, and can not directly account for the reduced wind power input. Instead, the wind stress anomaly leads to asymmetric gyres whose surface currents are out of alignment with the wind stress, reducing the net power input.
Previous studies [
27,
28] have shown that the low-frequency variability found in the QG double-gyre problem with symmetric wind stress is qualitatively different from the variability in models with even a slightly asymmetric wind stress. The qualitative nature of the low frequency variability observed in our model, with symmetric zonal winds, is probably different from the variability that would be observed with a more realistic asymmetric wind field. Nevertheless, the mechanism proposed here (a wind stress anomaly shifting the jet southwards) is robust; Hogg et al. [
13] used an asymmetric wind stress and observed a southward jet shift. Shifting of the jet away from the region of maximum wind stress leads to an overall decrease in the wind power input, regardless of whether the wind field is symmetric or not.
Hogg et al. [
13] proposed a different mechanism to explain why SST dependent wind stress leads to a weaker gyre circulation. They argued that transient Ekman-pumping anomalies in the dynamically-sensitive region near the separation of the western boundary current destabilize the jet. The dynamics of the destabilized jet then lead to downgradient PV flux between the gyres, reducing their intensity. It is worth noting that this explanation is not directly at odds with the mechanism proposed here: both mechanisms could be operating simultaneously. However, the mechanism proposed by Hogg et al. [
13] is dynamically more complex, and therefore harder to observe directly in simulations.
Byrne et al. [
9] recently examined the effect of SST dependent wind stress in coupled atmosphere–ocean simulations of the South Atlantic. They found that SST dependence led to an increase of up to 10% in the wind power input, and furthermore that this input is directly into the ocean mesoscale rather than at large scales. These results appear to be in direct contradiction to ours, but the contradiction is only apparent. The mechanism they proposed requires a single sign of wind gradient: in the Southern Ocean, if the meridional wind gradient is positive the eddies are energized, and if the wind gradient is negative the eddies are de-energized. In our idealized QG double gyre, the wind gradient is positive over half the domain, and negative over the other half. The effects thus cancel each other out, leading to our observation of zero mean wind power input to the eddies.
To verify this cancellation of effects, we computed the eddy-scale part of the Ekman pumping velocity
and the eddy-scale part of the surface streamfunction
, where the prime
notation indicates application of the high-pass spatial filter. The eddy-scale contribution to the total wind power input is approximately
We computed this integral separately for the northern and southern halves of the domain and time-averaged the results (a similar result could presumably be obtained by computing the wind power cospectrum over the northern and southern halves independently.) In order to isolate the impact of SST dependence, we computed the results for the case where wind stress depends on SST but not on the ocean surface currents (Wind
III). The time-averaged eddy-scale wind power input for the southern half was 7.6 MW (megawatts), and for the northern half was
MW, leading to near-cancellation. The real ocean gyres are not symmetric so the effects from the subpolar and subtropical gyres are not likely to cancel as completely in the real ocean, or in a particular region of the ocean as in the study of Byrne et al. [
9].
The impact of SST dependent wind stress on jets is interesting in its own right, independent of its effect on the wind input to the circulation. The southward shift could potentially lead to improvements in eddy-permitting ocean model representations of the Gulf Stream separation, since many ocean models have a Gulf Stream that separates too far north [
29]. The mechanism proposed here could for example act on jets in the Southern Ocean, in addition to western-boundary current extensions. The SST fronts are reversed in the Southern Ocean, which would lead to a wind stress anomaly that accelerates the northern flank and decelerates the southern flank of a jet. Nevertheless, one hesitates to predict that this mechanism will cause Southern Ocean jets to drift north because the dynamics of jets are a complex and incompletely understood interplay between wind stress, Reynolds stresses, topography, and potential vorticity gradients (e.g., [
30,
31]).
In summary, we have investigated the effects of SST dependent wind stress on the wind-driven QG double gyre. The SST front co-located with the inter-gyre jet induces a wind stress anomaly that accelerates the southern flank of the jet, and decelerates its northern flank causing it to shift towards the south. The subtropical gyre shrinks and strengthens while the subpolar gyre dilates and weakens. This asymmetrizing of the gyre circulation, despite a symmetric wind stress forcing, leads to a reduction in the wind power input and an overall reduction of the strength of the circulation. The effects found in this simplified model should be verified in more complete, coupled atmosphere-ocean models.