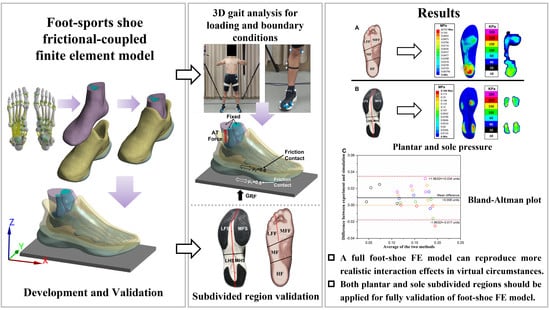
Development and Validation of a Subject-Specific Coupled Model for Foot and Sports Shoe Complex: A Pilot Computational Study
Abstract
1. Introduction
2. Materials and Methods
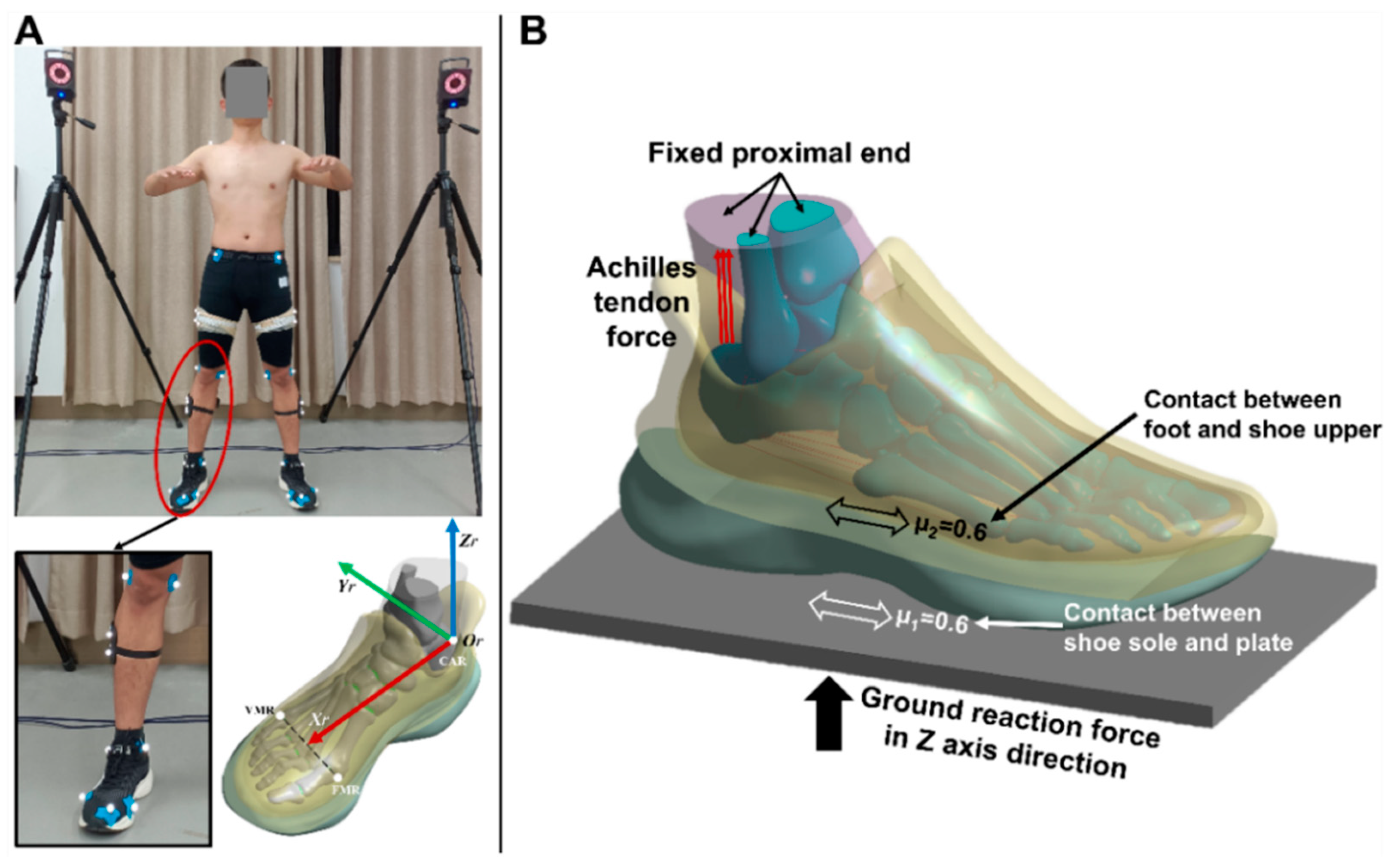
2.1. Participant Information
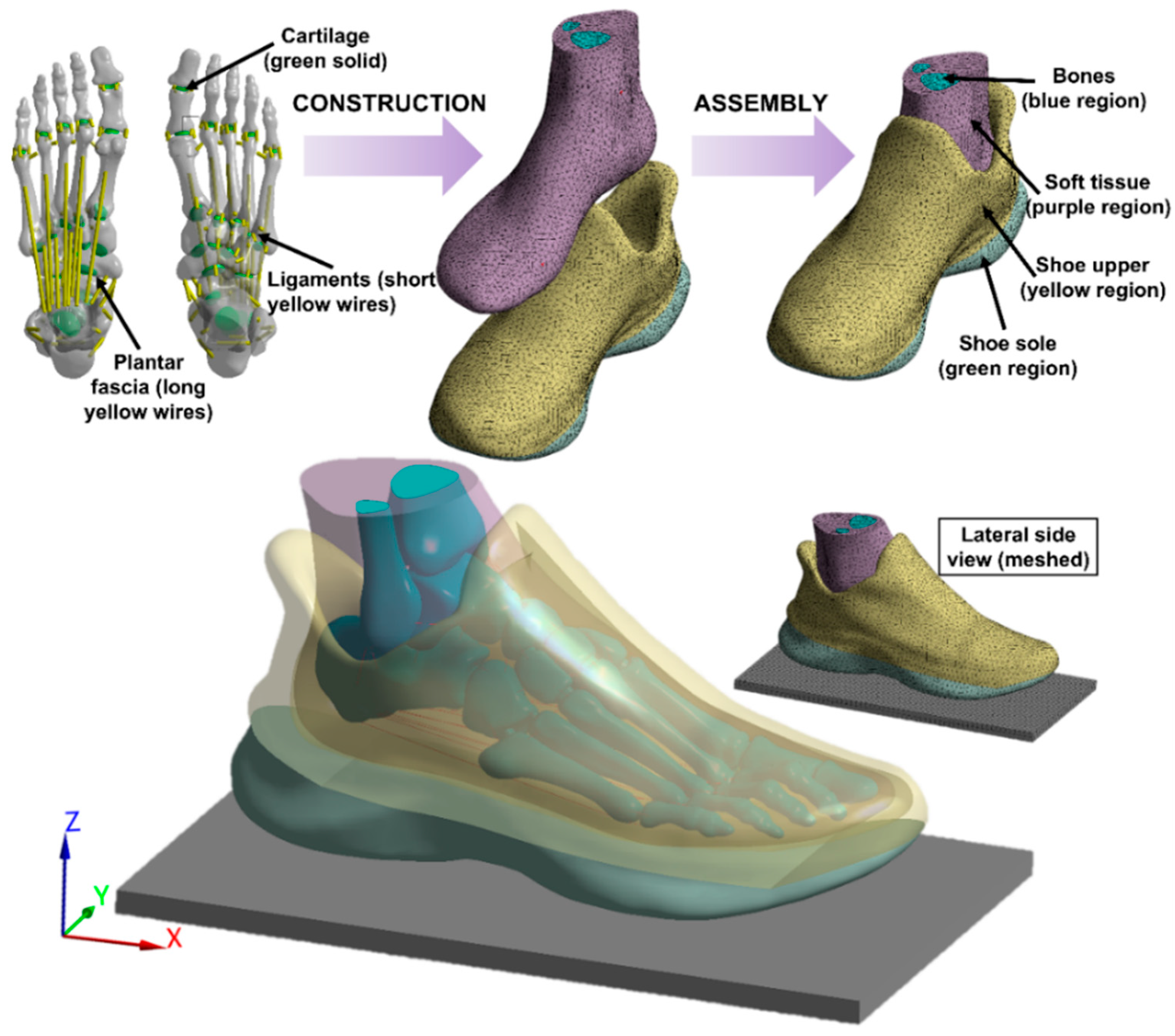
2.2. Model Construction
2.3. Material Properties
2.4. Boundary and Loading Conditions
2.5. Model Validation
3. Results
3.1. Plantar Pressure Validation
3.2. Sole Pressure Validation
3.3. Agreement Analysis
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Jiang, C. The Effect of Basketball Shoe Collar on Ankle Stability: A Systematic Review and Meta-Analysis. Phys. Act. Health 2020, 4, 11–18. [Google Scholar] [CrossRef][Green Version]
- Patra, R.; Das, H.C.; Jena, S. Evaluation of the mechanical behaviour of a bipolar hip prosthesis under transient loading. Int. J. Biomed. Eng. Technol. 2020, 12, 193–215. [Google Scholar] [CrossRef]
- Nigg, B.M.; Cigoja, S.; Nigg, S.R. Effects of running shoe construction on performance in long distance running. Footwear Sci. 2020, 12, 133–138. [Google Scholar] [CrossRef]
- Nigg, B.M.; Cigoja, S.; Nigg, S.R. Teeter-totter effect: A new mechanism to understand shoe-related improvements in long-distance running. Br. J. Sports Med. 2021, 55, 462–463. [Google Scholar] [CrossRef]
- Tavares, J.; Jost, T.; Drewelow, G.; Rylander, J. Do maximalist shoes mitigate risk factors for tibial stress fractures better than stability or flexible (marketed as minimalist) shoes? Footwear Sci. 2020, 12, 63–74. [Google Scholar] [CrossRef]
- Firminger, C.; Edwards, B. Effects of minimalist footwear and stride length reduction on the probability of metatarsal stress fracture: A weibull analysis with bone repair. Footwear Sci. 2017, 9, S127–S129. [Google Scholar] [CrossRef]
- Firminger, C.R.; Fung, A.; Loundagin, L.L.; Edwards, W.B. Effects of footwear and stride length on metatarsal strains and failure in running. Clin. Biomech. 2017, 49, 8–15. [Google Scholar] [CrossRef]
- Sun, X.; Lam, W.-K.; Zhang, X.; Wang, J.; Fu, W. Systematic review of the role of footwear constructions in running biomechanics: Implications for running-related injury and performance. J. Sport. Sci. Med. 2020, 19, 20. [Google Scholar]
- Cho, J.-R.; Park, S.-B.; Ryu, S.-H.; Kim, S.-H.; Lee, S.-B. Landing impact analysis of sports shoes using 3-D coupled foot-shoe finite element model. J. Mech. Sci. Technol. 2009, 23, 2583–2591. [Google Scholar] [CrossRef]
- Sun, D.; Mei, Q.; Baker, J.S.; Jia, X.; Gu, Y. A pilot study of the effect of outsole hardness on lower limb kinematics and kinetics during soccer related movements. J. Hum. Kinet. 2017, 57, 17–27. [Google Scholar] [CrossRef]
- Jiang, X.; Zhou, H.; Quan, W.; Hu, Q.; Baker, J.S.; Gu, Y. Ground reaction force differences between bionic shoes and neutral running shoes in recreational male runners before and after a 5 km run. Int. J. Environ. Res. Public Health 2021, 18, 9787. [Google Scholar] [CrossRef] [PubMed]
- Zhou, H.; Xu, D.; Quan, W.; Liang, M.; Ugbolue, U.C.; Baker, J.S.; Gu, Y. A pilot study of muscle force between normal shoes and bionic shoes during men walking and running stance phase using opensim. Actuators 2021, 10, 274. [Google Scholar] [CrossRef]
- Behforootan, S.; Chatzistergos, P.; Naemi, R.; Chockalingam, N. Finite element modelling of the foot for clinical application: A systematic review. Med. Eng. Phys. 2017, 39, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Cheung, J.T.-M.; Yu, J.; Wong, D.W.-C.; Zhang, M. Current methods in computer-aided engineering for footwear design. Footwear Sci. 2009, 1, 31–46. [Google Scholar] [CrossRef]
- Fekete, G. Computational study on lateral and medial wear characterization in knee implants by a multibody dynamic system. Acta Mech. 2021, 232, 1075–1086. [Google Scholar] [CrossRef]
- Zhang, Y.; Awrejcewicz, J.; Szymanowska, O.; Shen, S.; Zhao, X.; Baker, J.S.; Gu, Y. Effects of severe hallux valgus on metatarsal stress and the metatarsophalangeal loading during balanced standing: A finite element analysis. Comput. Biol. Med. 2018, 97, 1–7. [Google Scholar] [CrossRef]
- Li, S.; Zhang, Y.; Gu, Y.; Ren, J. Stress distribution of metatarsals during forefoot strike versus rearfoot strike: A finite element study. Comput. Biol. Med. 2017, 91, 38–46. [Google Scholar] [CrossRef]
- Gu, Y.; Ren, X.; Li, J.; Lake, M.; Zhang, Q.; Zeng, Y. Computer simulation of stress distribution in the metatarsals at different inversion landing angles using the finite element method. Int. Orthop. 2010, 34, 669–676. [Google Scholar] [CrossRef]
- Morales-Orcajo, E.; Becerro de Bengoa Vallejo, R.; Losa Iglesias, M.; Bayod, J.; Barbosa de Las Casas, E. Foot internal stress distribution during impact in barefoot running as function of the strike pattern. Comput. Methods Biomech. Biomed. Eng. 2018, 21, 471–478. [Google Scholar] [CrossRef]
- Li, Y.; Leong, K.; Gu, Y. Construction and finite element analysis of a coupled finite element model of foot and barefoot running footwear. Proc. Inst. Mech. Eng. Part P J. Sport. 2019, 233, 101–109. [Google Scholar] [CrossRef]
- Qiu, T.-X.; Teo, E.-C.; Yan, Y.-B.; Lei, W. Finite element modeling of a 3D coupled foot–boot model. Med. Eng. Phys. 2011, 33, 1228–1233. [Google Scholar] [CrossRef] [PubMed]
- Kim, S.; Cho, J.; Choi, J.; Ryu, S.; Jeong, W. Coupled foot-shoe-ground interaction model to assess landing impact transfer characteristics to ground condition. Interact. Multiscale Mech. 2012, 5, 75–90. [Google Scholar] [CrossRef]
- Chen, T.L.-W.; Wong, D.W.-C.; Wang, Y.; Lin, J.; Zhang, M. Foot arch deformation and plantar fascia loading during running with rearfoot strike and forefoot strike: A dynamic finite element analysis. J. Biomech. 2019, 83, 260–272. [Google Scholar] [CrossRef] [PubMed]
- Yu, L.; Mei, Q.; Mohamad, N.I.; Gu, Y.; Fernandez, J. An exploratory investigation of patellofemoral joint loadings during directional lunges in badminton. Comput. Biol. Med. 2021, 132, 104302. [Google Scholar] [CrossRef]
- Akrami, M.; Qian, Z.; Zou, Z.; Howard, D.; Nester, C.J.; Ren, L. Subject-specific finite element modelling of the human foot complex during walking: Sensitivity analysis of material properties, boundary and loading conditions. Biomech. Model. Mechanobiol. 2018, 17, 559–576. [Google Scholar] [CrossRef] [PubMed]
- Yu, J.; Cheung, J.T.-M.; Fan, Y.; Zhang, Y.; Leung, A.K.-L.; Zhang, M. Development of a finite element model of female foot for high-heeled shoe design. Clin. Biomech. 2008, 23, S31–S38. [Google Scholar] [CrossRef]
- Chow, J.C.; Boyd, S.K.; Lichti, D.D.; Ronsky, J.L. Robust self-supervised learning of deterministic errors in single-plane (monoplanar) and dual-plane (biplanar) X-ray fluoroscopy. IEEE Trans. Med. Imaging 2020, 39, 2051–2060. [Google Scholar] [CrossRef]
- Brandenburg, J.E.; Eby, S.F.; Song, P.; Zhao, H.; Brault, J.S.; Chen, S.; An, K.-N. Ultrasound elastography: The new frontier in direct measurement of muscle stiffness. Arch. Phys. Med. Rehabil. 2014, 95, 2207–2219. [Google Scholar] [CrossRef]
- Passmore, E.; Lai, A.; Sangeux, M.; Schache, A.G.; Pandy, M.G. Application of ultrasound imaging to subject-specific modelling of the human musculoskeletal system. Meccanica 2017, 52, 665–676. [Google Scholar] [CrossRef]
- Xiang, L.; Mei, Q.; Wang, A.; Shim, V.; Fernandez, J.; Gu, Y. Evaluating function in the hallux valgus foot following a 12-week minimalist footwear intervention: A pilot computational analysis. J. Biomech. 2022, 132, 110941. [Google Scholar] [CrossRef]
- Xu, D.; Quan, W.; Zhou, H.; Sun, D.; Baker, J.S.; Gu, Y. Explaining the differences of gait patterns between high and low-mileage runners with machine learning. Sci. Rep. 2022, 12, 2981. [Google Scholar] [CrossRef] [PubMed]
- Merry, C.; Baker, J.S.; Dutheil, F.; Ugbolue, U.C. Do Kinematic Study Assessments Improve Accuracy & Precision in Golf Putting? A Comparison between Elite and Amateur Golfers: A Systematic Review and Meta-Analysis. Phys. Act. Health 2022, 6, 108–123. [Google Scholar]


| Component | Element Type | Young’s Modulus E (MPa) | Poisson’s Ratio ν | Cross-Section Area (mm2) | Mass Density ρ (kg/m3) |
|---|---|---|---|---|---|
| Shoe upper | Tetrahedral solid | 11.76 | 0.35 | - | 9400 |
| Shoe sole (baseline) | Tetrahedral solid | 2.49 | 0.35 | - | 2300 |
| Bone | Tetrahedral solid | 7300 | 0.30 | - | 1500 |
| Cartilage | Tetrahedral solid | 1 | 0.40 | - | 1050 |
| Ligament | Tension-only truss | 260 | 0.40 | 18.4 | 937 |
| Plantar fascia | Tension-only truss | 350 | 0.40 | 58.6 | 937 |
| Soft tissue | Tetrahedral solid | 1.15 | 0.49 | - | 937 |
| Ground plate | Hexahedral solid | 17,000 | 0.10 | - | 5000 |
| Plantar Region | Peak Plantar Pressure (MPa) | ||
|---|---|---|---|
| Experiment | Simulation | Relative Error (%) | |
| MFF | 0.086 | 0.061 | −29.07 |
| LFF | 0.065 | 0.044 | −32.21 |
| MF | 0.047 | 0.043 | −8.51 |
| HF | 0.113 | 0.111 | −1.77 |
| Average error (%) | = 17.89 | ||
| Sole Region | Peak Sole Pressure (MPa) | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Experiment | Simulation (Baseline) | Simulation (Baseline + 10%) | Simulation (Baseline + 20%) | Simulation (Baseline − 10%) | Simulation (Baseline − 20%) | ||||||
| Value | Value | Relative Error % | Value | Relative Error % | Value | Relative Error % | Value | Relative Error % | Value | Relative Error % | |
| MFS | 0.182 | 0.186 | 2.20 | 0.198 | 8.79 | 0.207 | 13.74 | 0.176 | −3.30 | 0.166 | −8.79 |
| LFS | 0.120 | 0.112 | −6.67 | 0.117 | −2.50 | 0.120 | 0.00 | 0.108 | −10.00 | 0.104 | −13.33 |
| MHS | 0.189 | 0.173 | −8.47 | 0.182 | −3.70 | 0.190 | 0.53 | 0.165 | −12.70 | 0.157 | −16.93 |
| LHS | 0.154 | 0.142 | −7.79 | 0.150 | −2.60 | 0.155 | 0.65 | 0.136 | −11.69 | 0.131 | −14.94 |
| Average error (%) | = 6.28 | = 4.39 | = 3.73 | = 9.42 | = 13.49 | ||||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, Y.; Cen, X.; Zhang, Y.; Bíró, I.; Ji, Y.; Gu, Y. Development and Validation of a Subject-Specific Coupled Model for Foot and Sports Shoe Complex: A Pilot Computational Study. Bioengineering 2022, 9, 553. https://doi.org/10.3390/bioengineering9100553
Song Y, Cen X, Zhang Y, Bíró I, Ji Y, Gu Y. Development and Validation of a Subject-Specific Coupled Model for Foot and Sports Shoe Complex: A Pilot Computational Study. Bioengineering. 2022; 9(10):553. https://doi.org/10.3390/bioengineering9100553
Chicago/Turabian StyleSong, Yang, Xuanzhen Cen, Yan Zhang, István Bíró, Yulei Ji, and Yaodong Gu. 2022. "Development and Validation of a Subject-Specific Coupled Model for Foot and Sports Shoe Complex: A Pilot Computational Study" Bioengineering 9, no. 10: 553. https://doi.org/10.3390/bioengineering9100553
APA StyleSong, Y., Cen, X., Zhang, Y., Bíró, I., Ji, Y., & Gu, Y. (2022). Development and Validation of a Subject-Specific Coupled Model for Foot and Sports Shoe Complex: A Pilot Computational Study. Bioengineering, 9(10), 553. https://doi.org/10.3390/bioengineering9100553