Variation of Passive Biomechanical Properties of the Small Intestine along Its Length: Microstructure-Based Characterization
Abstract
1. Introduction
2. Materials and Methods
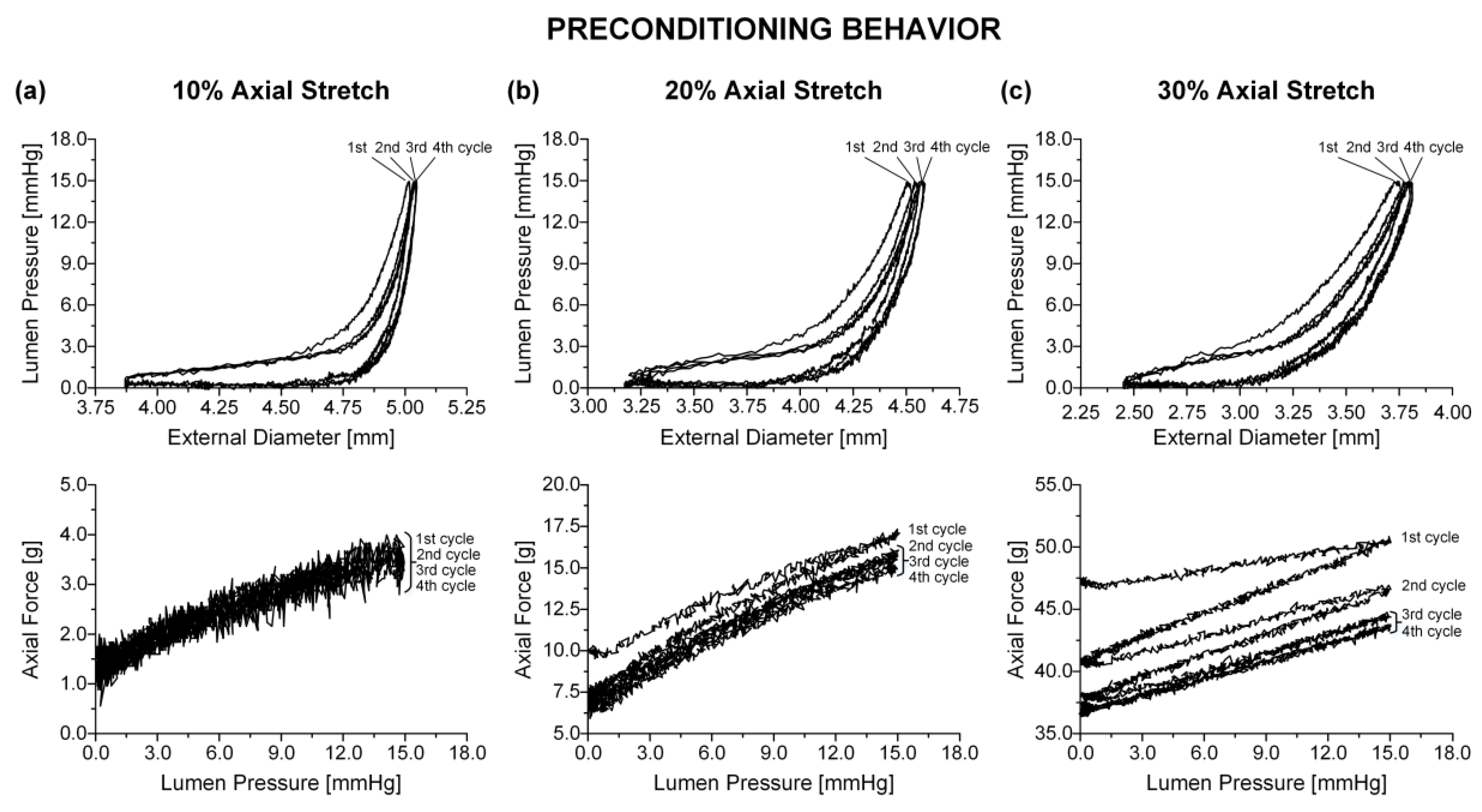
2.1. Biologic Tissue and Biomechanical Testing
2.2. Histomorphometric Evaluation
2.3. Microstructure-Based Material Models
2.4. Parameter Estimation
2.5. Statistical Analysis
3. Results
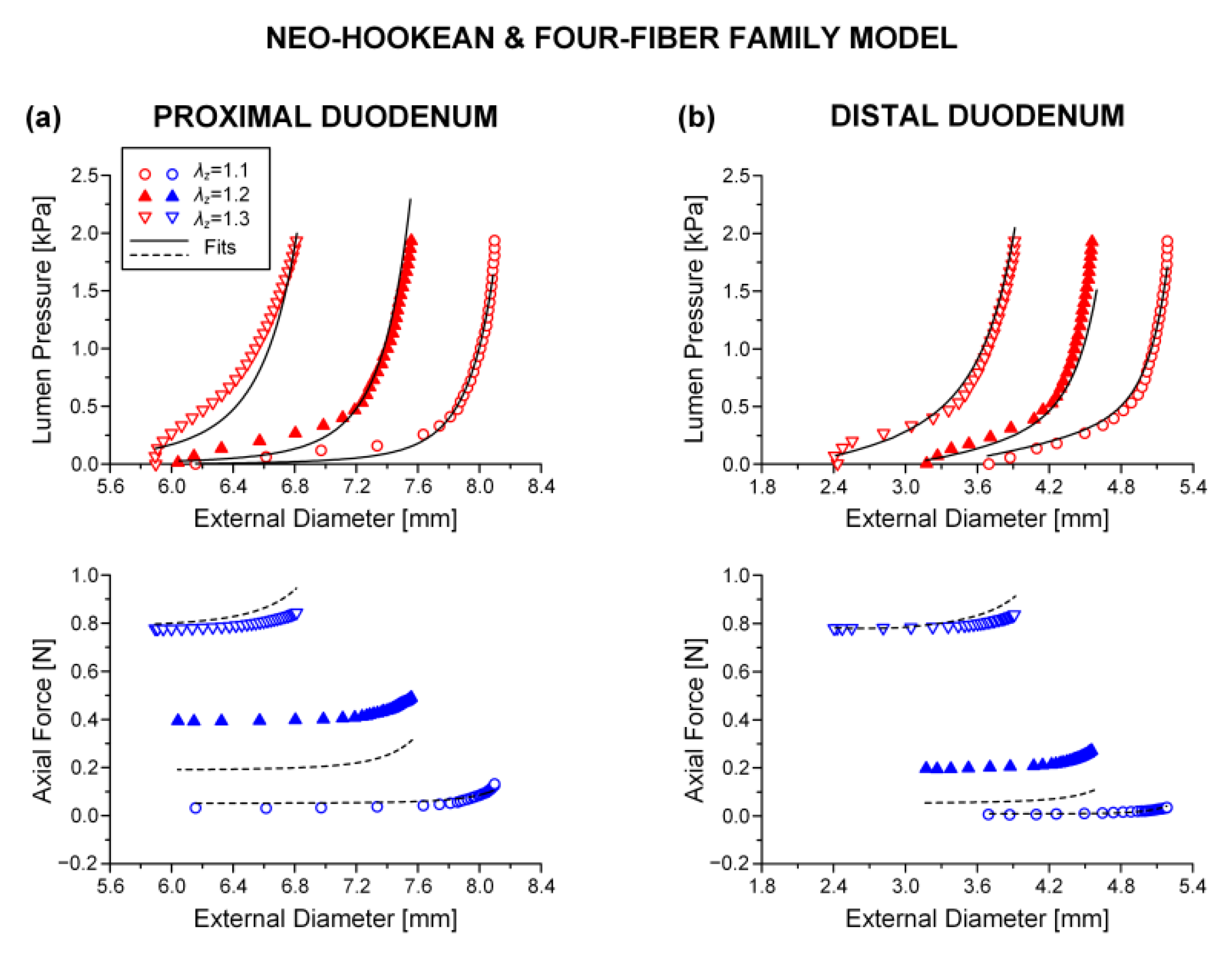
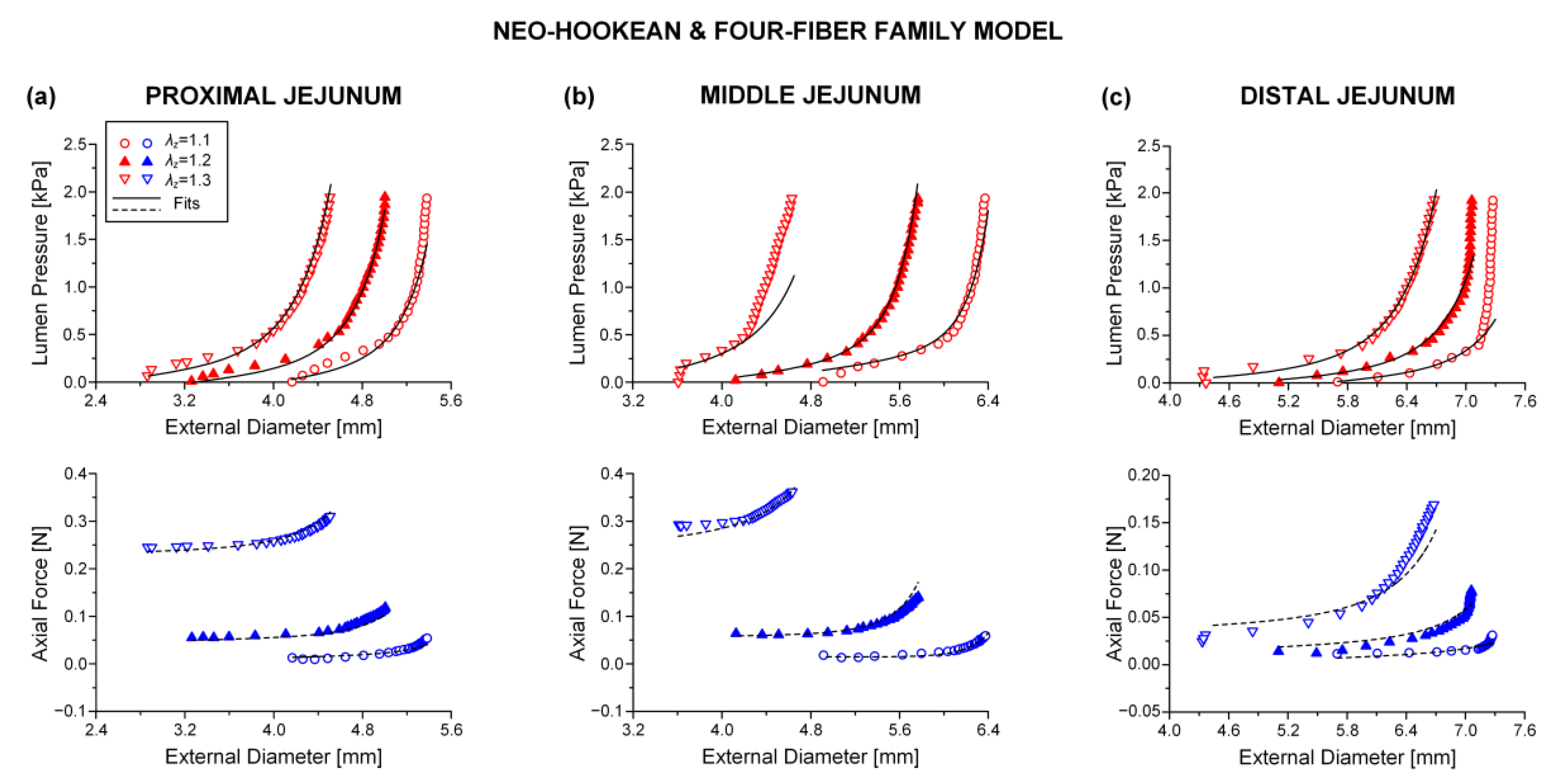
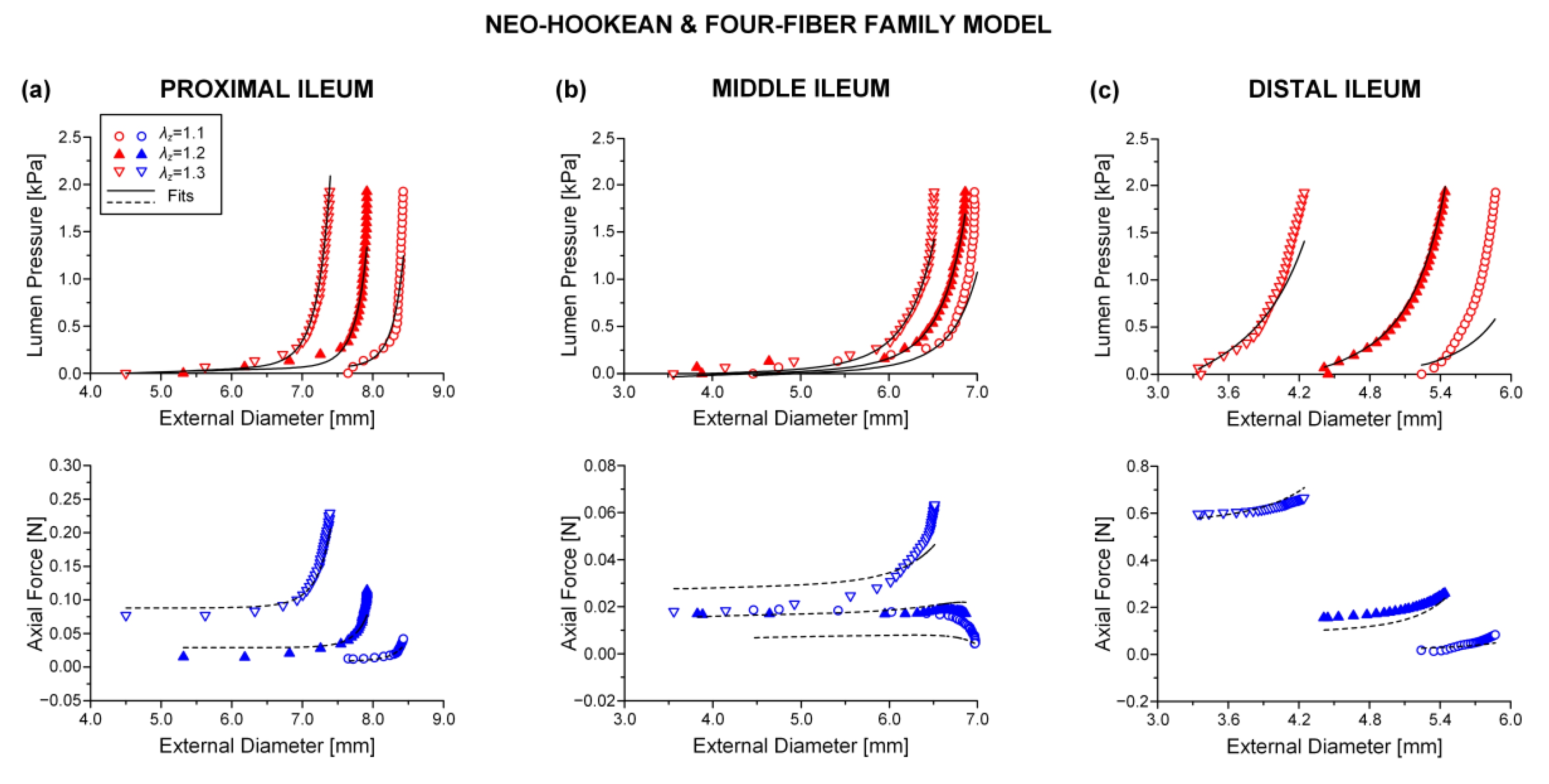
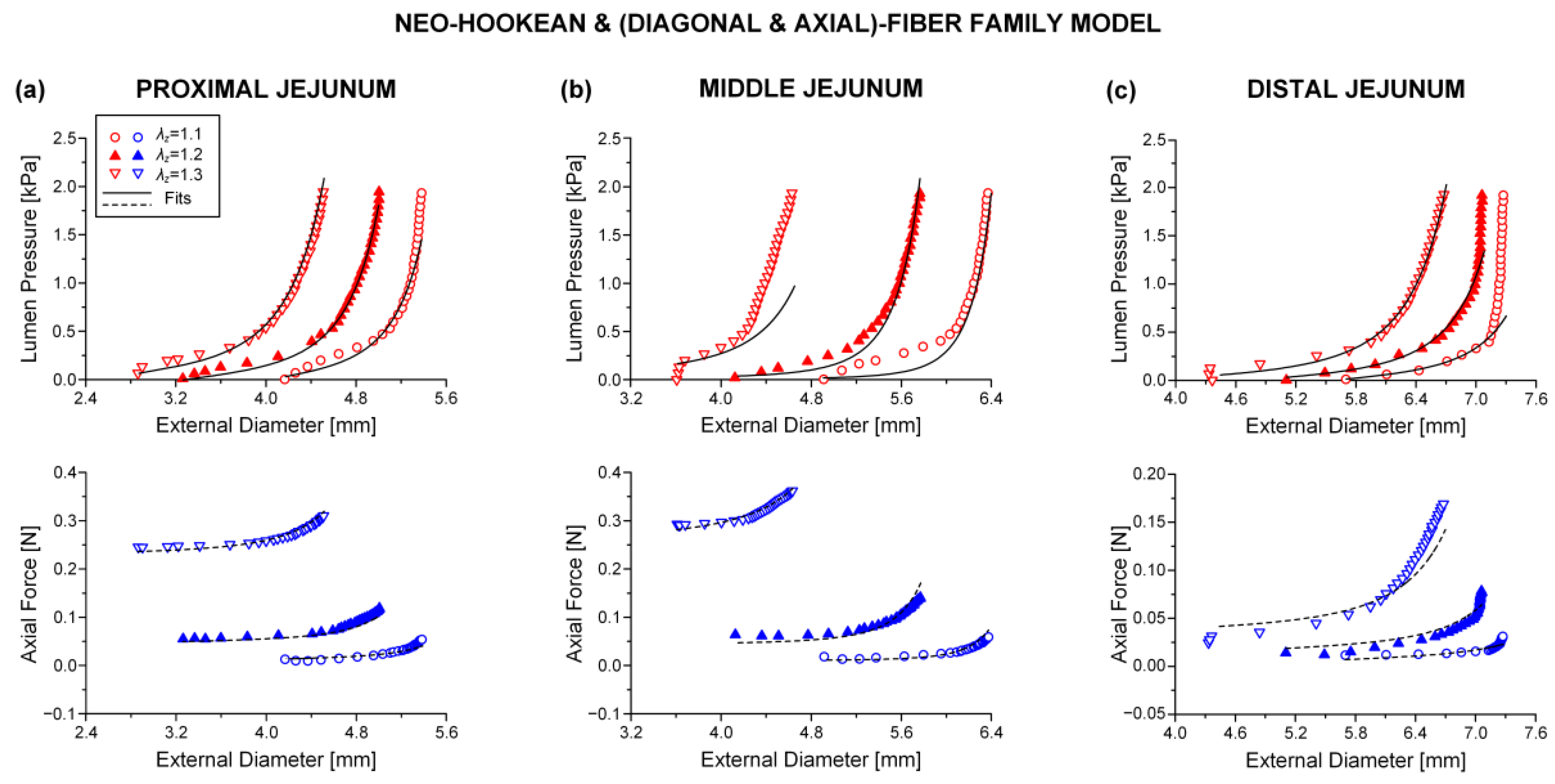
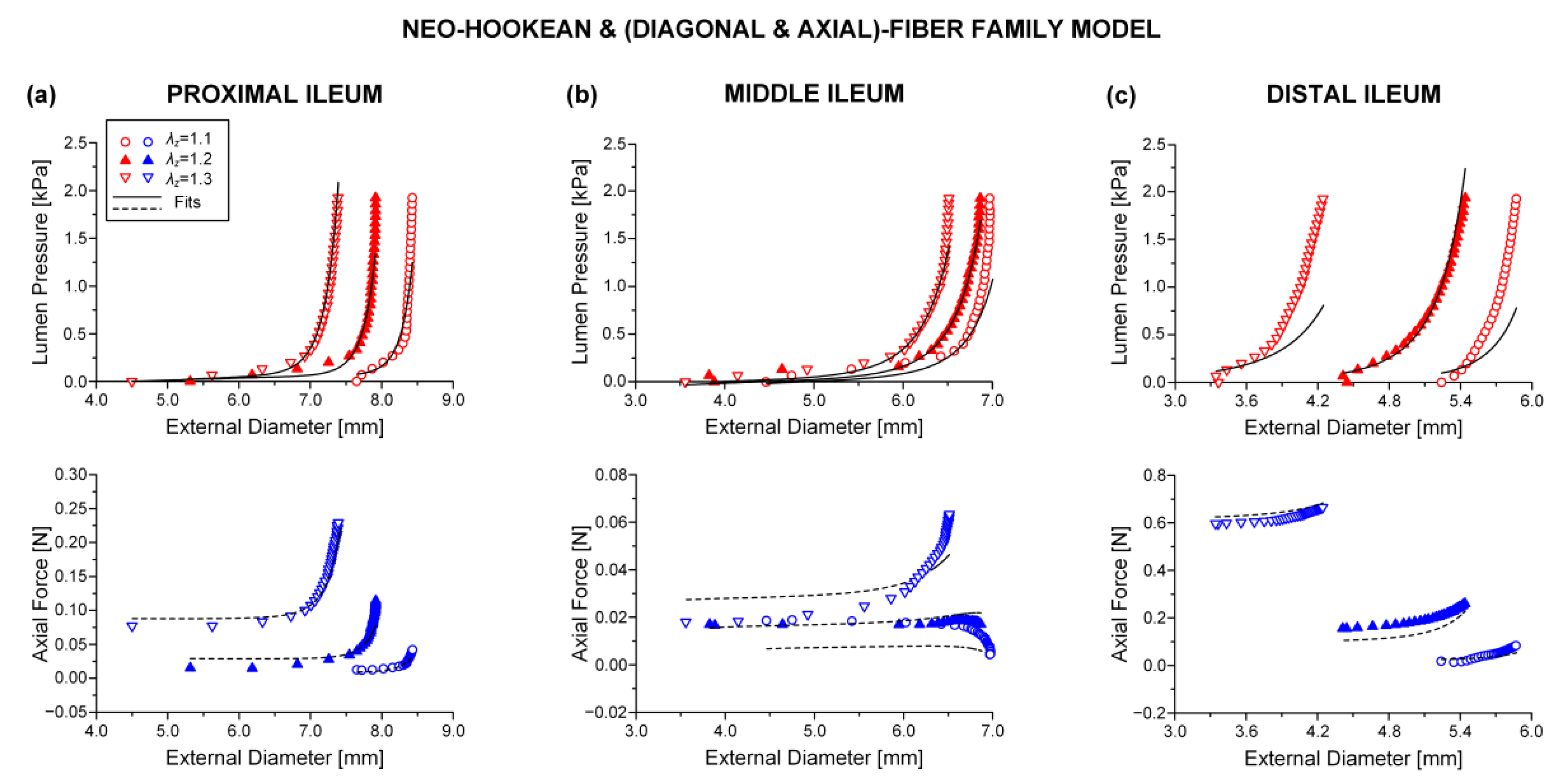
3.1. Comprehensive Model
3.2. Parametric Analysis
3.3. Reduced Model
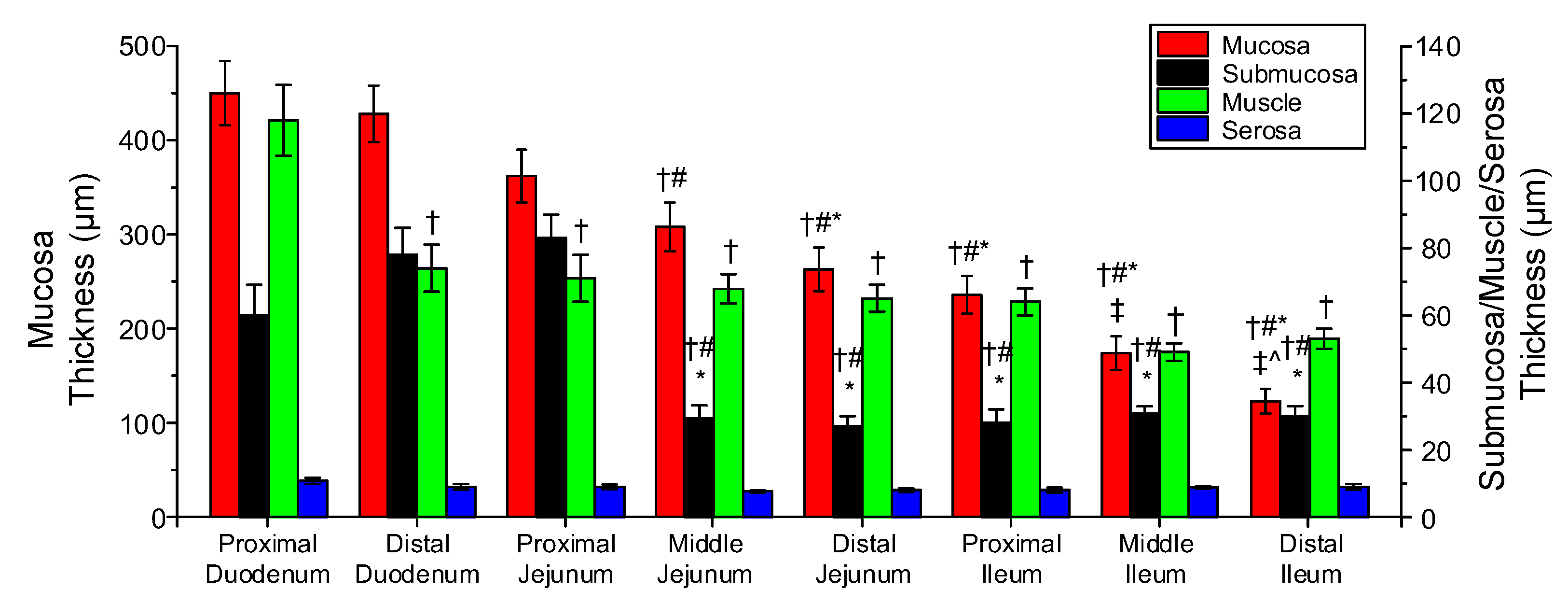
3.4. Histologic Findings
4. Discussion
4.1. General Findings
4.2. Consideration of Microstructure-Based Material Models for the Small Intestine
4.3. Structural Interpretation of Model Parameters: Consideration of Segmental Differences and Physiologic Implications
4.4. Limitations and Future Studies
5. Conclusions
Supplementary Materials
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Campbell, J.; Berry, J.; Liang, Y. Anatomy and physiology of the small intestine. In Shackelford’s Surgery of the Alimentary Tract, 8th ed.; Yeo, C.J., Ed.; Elsevier: Philadelphia, PA, USA, 2019; Volume 2, pp. 817–841. [Google Scholar]
- Gregersen, H. Biomechanics of the Gastrointestinal Tract. New Prospectives in Motility Research and Diagnostics, 1st ed.; Springer-Verlag London: London, UK, 2003. [Google Scholar] [CrossRef]
- Storkholm, J.H.; Villadsen, G.E.; Jensen, S.L.; Gregersen, H. Mechanical properties and collagen content differ between isolated guinea pig duodenum, jejunum, and distal ileum. Dig. Dis. Sci. 1998, 43, 2034–2041. [Google Scholar] [CrossRef]
- Gregersen, H.; Kassab, G.S.; Fung, Y.C. The zero-stress state of the gastrointestinal tract: Biomechanical and functional implications. Dig. Dis. Sci. 2000, 45, 2271–2281. [Google Scholar] [CrossRef]
- Dou, Y.; Zhao, J.; Gregersen, H. Morphology and stress-strain properties along the small intestine in the rat. J. Biomech. Eng. 2003, 125, 266–273. [Google Scholar] [CrossRef] [PubMed]
- Dou, Y.; Fan, Y.; Zhao, J.; Gregersen, H. Longitudinal residual strain and stress-strain relationship in rat small intestine. Biomed. Eng. Online 2006, 5, 37. [Google Scholar] [CrossRef] [PubMed]
- Sokolis, D.P.; Sassani, S.G. Microstructure-based constitutive modeling for the large intestine validated by histological observations. J. Mech. Behav. Biomed. Mater. 2013, 21, 149–166. [Google Scholar] [CrossRef] [PubMed]
- Carniel, E.L.; Gramigna, V.; Fontanella, C.G.; Stefanini, C.; Natali, A.N. Constitutive formulations for the mechanical investigation of colonic tissues. J. Biomed. Mater. Res. A 2014, 102, 1243–1254. [Google Scholar] [CrossRef]
- Patel, B.; Chen, H.; Ahuja, A.; Krieger, J.F.; Noblet, J.; Chambers, S.; Kassab, G.S. Constitutive modeling of the passive inflation-extension behavior of the swine colon. J. Mech. Behav. Biomed. Mater. 2018, 77, 176–186. [Google Scholar] [CrossRef]
- Zhao, Y.; Siri, S.; Feng, B.; Pierce, D.M. Computational modeling of mouse colorectum capturing longitudinal and through-thickness biomechanical heterogeneity. J. Mech. Behav. Biomed. Mater. 2021, 113, 104127. [Google Scholar] [CrossRef]
- Sokolis, D.P. Experimental study and biomechanical characterization for the passive small intestine: Identification of regional differences. J. Mech. Behav. Biomed. Mater. 2017, 74, 93–105. [Google Scholar] [CrossRef]
- Humphrey, J.D. Cardiovascular Solid Mechanics: Cells, Tissues, and Organs, 1st ed.; Springer-Verlag: New York, NY, USA, 2002. [Google Scholar]
- Fung, Y.C. Biomechanics: Mechanical Properties of Living Tissues, 1st ed.; Springer-Verlag: New York, NY, USA, 1993. [Google Scholar]
- Holzapfel, G.A.; Gasser, T.C.; Ogden, R.W. A new constitutive framework for arterial wall mechanics and a comparative study of material models. J. Elast. 2000, 61, 1–48. [Google Scholar] [CrossRef]
- Baek, S.; Gleason, R.L.; Rajagopal, K.R.; Humphrey, J.D. Theory of small on large: Potential utility in computations of fluid-solid interactions in arteries. Comput. Methods Appl. Mech. Eng. 2007, 196, 3070–3078. [Google Scholar] [CrossRef]
- Sokolis, D.P. Structurally-motivated characterization of the passive pseudo-elastic response of esophagus and its layers. Comput. Biol. Med. 2013, 43, 1272–1285. [Google Scholar] [CrossRef]
- Sokolis, D.P. Alterations with age in the biomechanical behavior of human ureteral wall: Microstructure-based modeling. J. Biomech. 2020, 109, 109940. [Google Scholar] [CrossRef]
- Sokolis, D.P. Experimental investigation and constitutive modeling of the 3d histomechanical properties of vein tissue. Biomech. Model. Mechanobiol. 2013, 12, 431–451. [Google Scholar] [CrossRef]
- Sokolis, D.P.; Sassani, S.; Kritharis, E.P.; Tsangaris, S. Differential histomechanical response of carotid artery in relation to species and region: Mathematical description accounting for elastin and collagen anisotropy. Med. Biol. Eng. Comput. 2011, 49, 867–879. [Google Scholar] [CrossRef]
- Fackler, K.; Klein, L.; Hiltner, A. Polarizing light microscopy of intestine and its relationship to mechanical behavior. J. Microsc. 1981, 124, 305–311. [Google Scholar] [CrossRef]
- Orberg, J.W.; Klein, L.; Hiltner, A. Scanning electron microscopy of collagen fibers in intestine. Connect. Tissue Res. 1982, 9, 187–193. [Google Scholar] [CrossRef] [PubMed]
- Klein, L.; Eichelberger, H.; Mirian, M.; Hiltner, A. Ultrastructural properties of collagen fibrils in rat intestine. Connect. Tissue Res. 1983, 12, 71–78. [Google Scholar] [CrossRef] [PubMed]
- Sacks, M.S.; Gloeckner, C.D. Quantification of the fiber architecture and biaxial mechanical behavior of porcine intestinal submucosa. J. Biomed. Mater. Res. 1999, 46, 1–10. [Google Scholar] [CrossRef]
- Shirazi, S.; Schulze-Delrieu, K.; Brown, C.K. Duodenal resistance to the emptying of various solutions from the isolated cat stomach. J. Lab. Clin. Med. 1988, 111, 654–660. [Google Scholar] [PubMed]
- Schulze-Delrieu, K. Intrinsic differences in the filling response of the guinea pig duodenum and ileum. J. Lab. Clin. Med. 1991, 117, 44–50. [Google Scholar] [PubMed]
- Soergel, K.H. Flow measurements of test meals and fasting contents in human small intestine. In Proceedings of the International Symposium on Motility of the Gastrointestinal Tract; Demling, L., Ed.; Thieme: Stuttgart, Germany, 1971; pp. 81–86. [Google Scholar]
- Lennernäs, H.; Regårdh, C.G. Regional gastrointestinal absorption of the beta-blocker Pafenolol in the rat an intestinal transit rate determined by movement of 14C-polyethylene glycol (PEG 4000). Pharm. Res. 1993, 10, 130–135. [Google Scholar] [CrossRef]
- Kararli, T.T. Comparison of the gastrointestinal anatomy, physiology, and biochemistry of humans and commonly used laboratory animals. Biopharm. Drug Dispos. 1995, 16, 351–380. [Google Scholar] [CrossRef] [PubMed]
- Ferreira, J.P.S.; Parente, M.P.L.; Jabareen, M.; Natal Jorge, R.M. A general framework for the numerical implementation of anisotropic hyperelastic material models including non-local damage. Biomech. Model. Mechanobiol. 2017, 16, 1119–1140. [Google Scholar] [CrossRef] [PubMed]
- Stavropoulou, E.A.; Dafalias, Y.F.; Sokolis, D.P. Biomechanical behavior and histological organization of the three-layered passive esophagus as a function of topography. Proc. Inst. Mech. Eng. H 2012, 226, 477–490. [Google Scholar] [CrossRef]
- Siri, S.; Maier, F.; Santos, S.; Pierce, D.M.; Feng, B. Load-bearing function of the colorectal submucosa and its relevance to visceral nociception elicited by mechanical stretch. Am. J. Physiol. Gastrointest. Liver Physiol. 2019, 317, G349–G358. [Google Scholar] [CrossRef] [PubMed]



| μ [kPa] | ε [-] | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| PD | 0.042 ± 0.042 | 1.256 ± 0.527 * | 17.118 ± 4.047 | 16.751 ± 6.176 | 3.788 ± 0.730 | 0.003 ± 0.003 & | 1.546 ± 0.605 | 0.651 ± 0.029 # | 0.325 ± 0.014 | 0.865 ± 0.011 | (14.1 ± 9.2) × 10−5 |
| DD | (8.3 ± 8.3) × 10−7 | 0.955 ± 0.216 * | 5.964 ± 0.641 | 10.592 ± 4.936 * | 5.235 ± 1.351 | 0.167 ± 0.031 | 0.057 ± 0.046 @ | 0.378 ± 0.028 | 0.332 ± 0.026 | 0.857 ± 0.024 | (9.0 ± 4.2) × 10−5 |
| PJ | (1.8 ± 1.2) × 10−12 | 3.760 ± 0.757 | 10.521 ± 1.926 | 49.491 ± 18.385 | 2.327 ± 0.566 | (1.7 ± 1.4) × 10−9 & | 0.607 ± 0.269 @ | 0.665 ± 0.028 # | 0.287 ± 0.019 | 0.883 ± 0.018 | 0.002 ± 0.002 |
| MJ | (2.8 ± 2.7) × 10−9 | 0.615 ± 0.131 * | 4.447 ± 1.006 | 19.571 ± 7.680 | 3.252 ± 0.988 | 0.045 ± 0.020 | 0.205 ± 0.186 @ | 0.560 ± 0.056 # | 0.263 ± 0.015 | 0.908 ± 0.009 | (6.2 ± 2.3) × 10−5 |
| DJ | (13.5 ± 8.9) × 10−11 | 1.064 ± 0.191 * | 10.974 ± 3.093 | 16.812 ± 6.229 | 2.836 ± 0.570 | 0.090 ± 0.063 | 1.191 ± 0.649 | 0.622 ± 0.031 # | 0.271 ± 0.019 | 0.898 ± 0.017 | (3.8 ± 1.5) × 10−4 |
| PI | 0.065 ± 0.048 | 0.642 ± 0.265 * | 9.651 ± 2.983 | 13.258 ± 4.926 * | 3.226 ± 0.420 | (3.4 ± 1.8) × 10−8 & | 0.095 ± 0.092 @ | 0.599 ± 0.050 # | 0.236 ± 0.021 | 0.919 ± 0.011 | (12.0 ± 5.1) × 10−5 |
| MI | (2.5 ± 1.7) × 10−7 | 1.261 ± 0.213 * | 18.490 ± 5.010 | 3.713 ± 0.668 * | 2.518 ± 0.966 | (1.6 ± 1.4) × 10−4& | 5.298 ± 2.608 | 0.704 ± 0.048 # | 0.319 ± 0.018 | 0.859 ± 0.019 | (5.7 ± 3.9) × 10−5 |
| DI | (6.2 ± 3.6) × 10−9 | 2.102 ± 0.658 | 14.938 ± 2.796 | 9.541 ± 2.091 * | 4.540 ± 0.664 | 0.240 ± 0.108 | 1.751 ± 0.283 | 0.639 ± 0.018 # | 0.304 ± 0.020 | 0.882 ± 0.017 | (9.8 ± 4.7) × 10−5 |
| μ [kPa] | ε [-] | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| Zero Neo-Hookean Term | |||||||||||
| D | 0 | 1.118 ± 0.330 | 11.320 ± 2.968 | 13.155 ± 4.550 | 4.557 ± 0.920 | 0.088 ± 0.033 | 0.857 ± 0.434 | 0.519 ± 0.051 | 0.357 ± 0.022 | 0.835 ± 0.019 | (3.4 ± 1.0) × 10−4 |
| J | 0 | 1.817 ± 0.480 | 8.709 ± 1.633 | 28.980 ± 10.048 | 2.857 ± 0.491 | 0.046 ± 0.027 | 0.738 ± 0.292 | 0.613 ± 0.029 | 0.272 ± 0.012 | 0.895 ± 0.012 | (8.4 ± 2.5) × 10−4 |
| I | 0 | 1.377 ± 0.319 | 14.084 ± 3.669 | 8.338 ± 2.182 | 3.438 ± 0.522 | 0.080 ± 0.051 | 2.542 ± 1.165 | 0.649 ± 0.030 | 0.290 ± 0.016 | 0.883 ± 0.012 | (5.6 ± 2.1) × 10−4 |
| Zero Diagonal-Fiber Families | |||||||||||
| D | 0.103 ± 0.103 | 0 | 0 | 20.853 ± 6.232 | 3.422 ± 0.701 | 0.161 ± 0.053 | 2.483 ± 1.105 | 0 | 0.665 ± 0.031 | 0.442 ± 0.038 | 0.031 ± 0.011 |
| J | (1.9 ± 1.8) × 10−9 | 0 | 0 | 36.421 ± 10.349 | 2.048 ± 0.347 | 0.185 ± 0.051 | 0.964 ± 0.279 | 0 | 0.664 ± 0.024 | 0.392 ± 0.041 | 0.018 ± 0.004 |
| I | 0.064 ± 0.047 | 0 | 0 | 14.093 ± 3.330 | 2.507 ± 0.400 | 0.259 ± 0.094 | 0.890 ± 0.244 | 0 | 0.673 ± 0.024 | 0.389 ± 0.037 | 0.013 ± 0.003 |
| Zero Axial-Fiber Family | |||||||||||
| D | 0.144 ± 0.014 | 1.939 ± 0.623 | 10.332 ± 3.270 | 0 | 0 | 0.110 ± 0.054 | 0.924 ± 0.424 | 0.487 ± 0.054 | 0.572 ± 0.041 | 0.562 ± 0.062 | 0.010 ± 0.004 |
| J | (1.3 ± 1.2) × 10−9 | 2.529 ± 0.563 | 7.478 ± 1.339 | 0 | 0 | 0.093 ± 0.065 | 0.496 ± 0.170 | 0.572 ± 0.029 | 0.577 ± 0.032 | 0.521 ± 0.055 | 0.011 ± 0.007 |
| I | (2.7 ± 2.7) × 10−4 | 2.979 ± 0.817 | 9.869 ± 3.525 | 0 | 0 | 0.265 ± 0.164 | 1.381 ± 0.346 | 0.562 ± 0.028 | 0.511 ± 0.023 | 0.645 ± 0.030 | 0.002 ± 0.001 |
| Zero Circumferential-Fiber Family | |||||||||||
| D | 0.025 ± 0.025 | 1.185 ± 0.320 | 11.016 ± 3.043 | 12.280 ± 4.562 | 5.127 ± 1.043 | 0 | 0 | 0.528 ± 0.049 | 0.375 ± 0.022 | 0.819 ± 0.020 | 0.007 ± 0.002 |
| J | (1.1 ± 1.1) × 10−9 | 1.804 ± 0.483 | 8.660 ± 1.630 | 28.639 ± 10.076 | 3.075 ± 0.568 | 0 | 0 | 0.619 ± 0.028 | 0.285 ± 0.014 | 0.886 ± 0.011 | 0.015 ± 0.003 |
| I | 0.026 ± 0.020 | 1.298 ± 0.311 | 14.431 ± 3.755 | 8.638 ± 2.228 | 3.329 ± 0.482 | 0 | 0 | 0.671 ± 0.031 | 0.302 ± 0.019 | 0.873 ± 0.015 | 0.008 ± 0.002 |
| μ [kPa] | ε [-] | ||||||||
|---|---|---|---|---|---|---|---|---|---|
| PD | 0.042 ± 0.042 | 1.237 ± 0.517 * | 17.196 ± 4.024 | 16.724 ± 6.175 | 3.822 ± 0.735 | 0.652 ± 0.028 # | 0.325 ± 0.014 | 0.865 ± 0.011 | 0.011 ± 0.002 |
| DD | (5.2 ± 5.2) × 10−7 | 1.127 ± 0.196 * | 5.145 ± 0.522 | 9.183 ± 4.722 * | 6.136 ± 1.497 | 0.397 ± 0.030 | 0.364 ± 0.024 | 0.827 ± 0.024 | 0.004 ± 0.002 |
| PJ | (1.8 ± 1.2) × 10−12 | 3.760 ± 0.757 | 10.521 ± 1.926 | 49.491 ± 18.385 | 2.327 ± 0.566 | 0.665 ± 0.028 # | 0.286 ± 0.019 | 0.883 ± 0.018 | 0.028 ± 0.005 |
| MJ | (2.9 ± 2.7) × 10−9 | 0.565 ± 0.130 * | 4.549 ± 1.012 | 19.244 ± 7.821 | 3.594 ± 1.140 | 0.570 ± 0.056 # | 0.281 ± 0.024 | 0.894 ± 0.016 | 0.006 ± 0.002 |
| DJ | (14.5 ± 9.3) × 10−11 | 1.086 ± 0.189 * | 10.816 ± 3.110 | 16.349 ± 6.061 | 3.030 ± 0.714 | 0.628 ± 0.029 # | 0.284 ± 0.019 | 0.888 ± 0.017 | 0.010 ± 0.002 |
| PI | 0.065 ± 0.048 | 0.642 ± 0.265 * | 9.650 ± 2.983 | 13.258 ± 4.927 * | 3.226 ± 0.420 | 0.599 ± 0.050 # | 0.236 ± 0.021 | 0.919 ± 0.011 | 0.004 ± 0.002 |
| MI | (2.6 ± 1.7) × 10−7 | 1.251 ± 0.214 * | 18.474 ± 5.005 | 3.669 ± 0.671 * | 2.520 ± 0.965 | 0.709 ± 0.045 # | 0.323 ± 0.020 | 0.855 ± 0.021 | 0.009 ± 0.002 |
| DI | (6.5 ± 3.9) × 10−9 | 1.967 ± 0.648 | 15.262 ± 3.629 | 9.918 ± 1.806 * | 4.396 ± 0.484 | 0.695 ± 0.027 # | 0.333 ± 0.028 | 0.858 ± 0.022 | 0.011 ± 0.003 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sokolis, D.P. Variation of Passive Biomechanical Properties of the Small Intestine along Its Length: Microstructure-Based Characterization. Bioengineering 2021, 8, 32. https://doi.org/10.3390/bioengineering8030032
Sokolis DP. Variation of Passive Biomechanical Properties of the Small Intestine along Its Length: Microstructure-Based Characterization. Bioengineering. 2021; 8(3):32. https://doi.org/10.3390/bioengineering8030032
Chicago/Turabian StyleSokolis, Dimitrios P. 2021. "Variation of Passive Biomechanical Properties of the Small Intestine along Its Length: Microstructure-Based Characterization" Bioengineering 8, no. 3: 32. https://doi.org/10.3390/bioengineering8030032
APA StyleSokolis, D. P. (2021). Variation of Passive Biomechanical Properties of the Small Intestine along Its Length: Microstructure-Based Characterization. Bioengineering, 8(3), 32. https://doi.org/10.3390/bioengineering8030032





