Comparison of Aerodynamic and Elastic Properties in Tissue and Synthetic Models of Vocal Fold Vibrations
Abstract
1. Introduction
2. Materials and Methods
2.1. Models Preparation
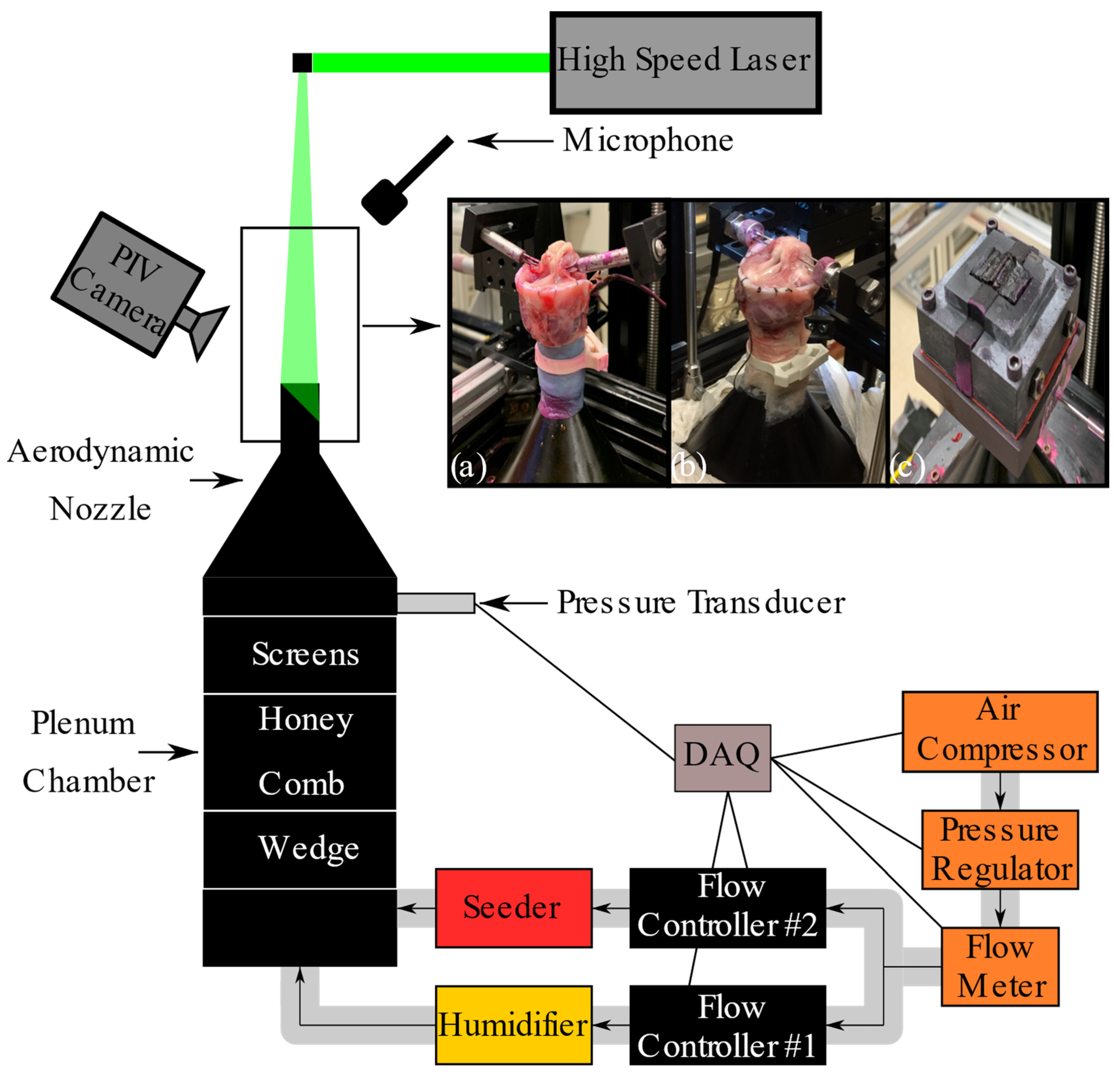
2.2. Experimental Setup for Aerodynamic Measurements
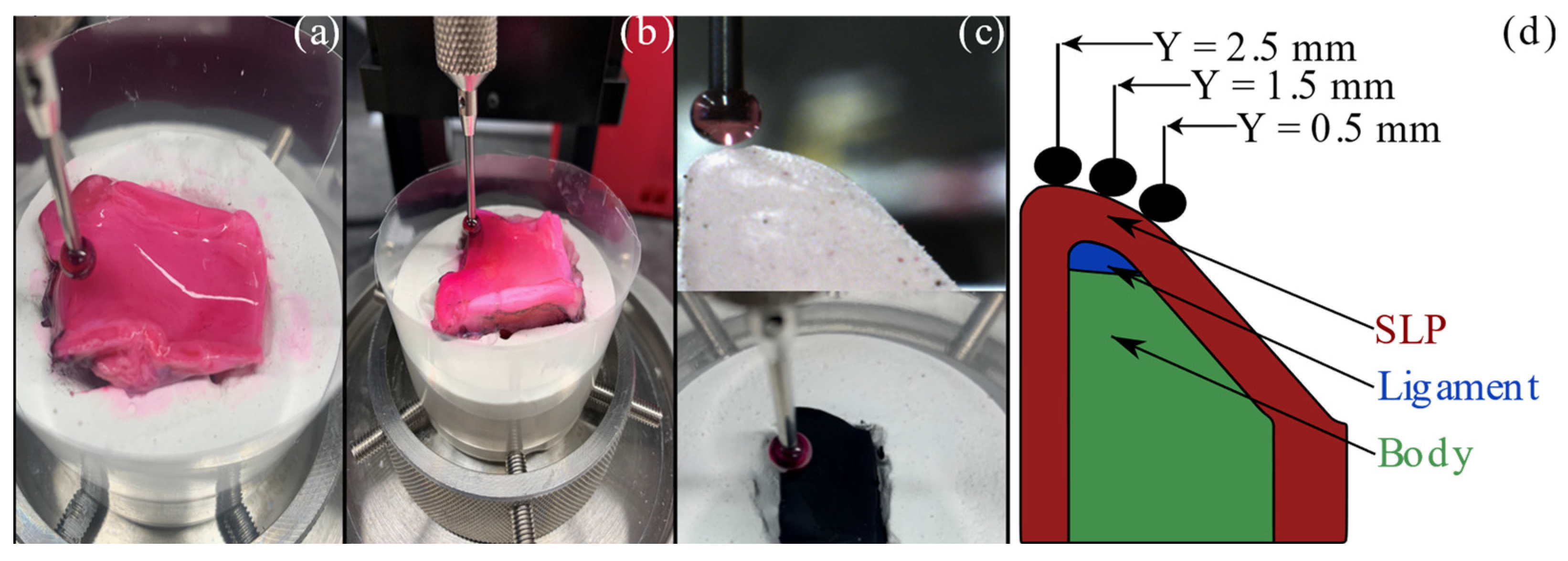
2.3. Experimental Setup for Elasticity Measurements
3. Results
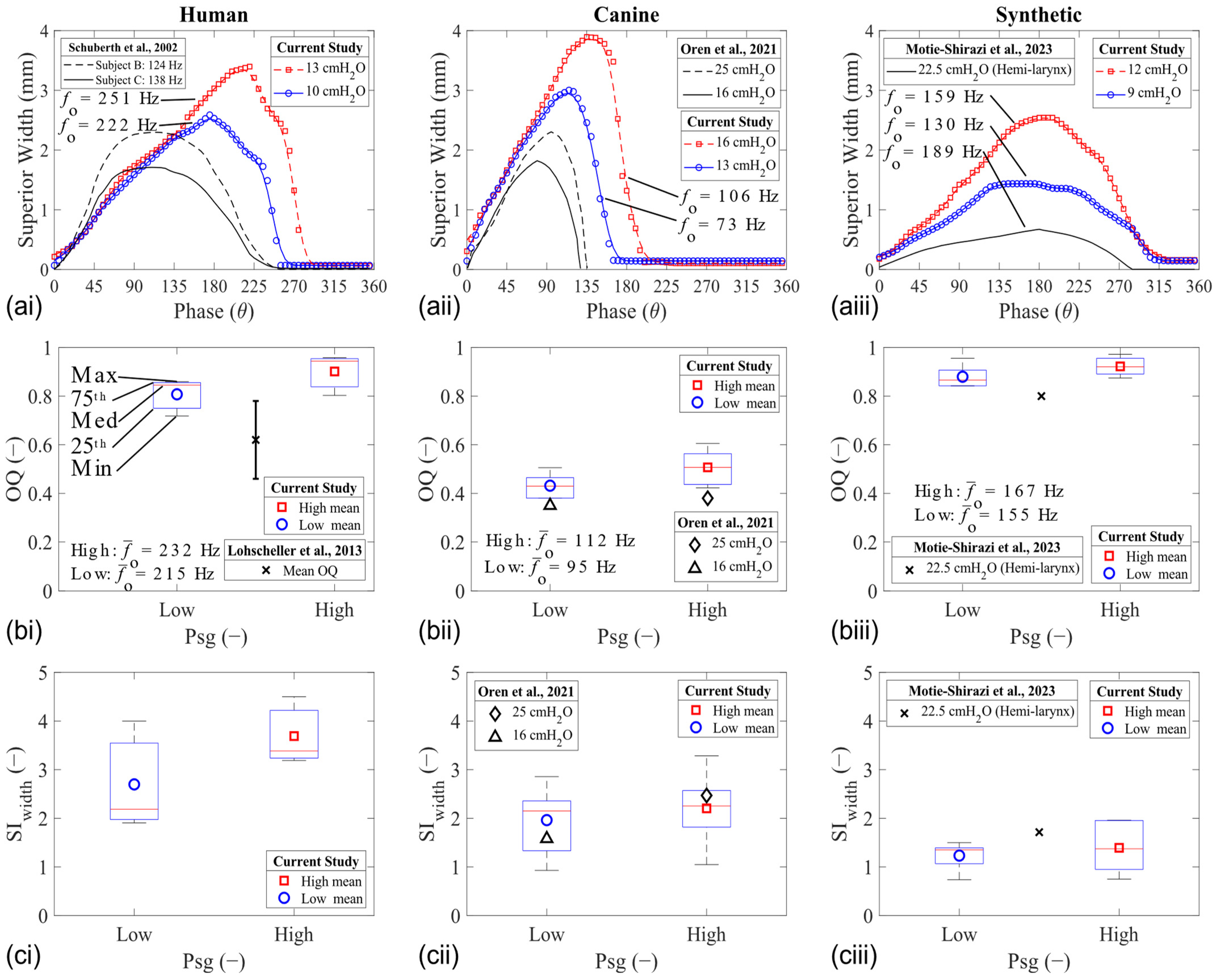
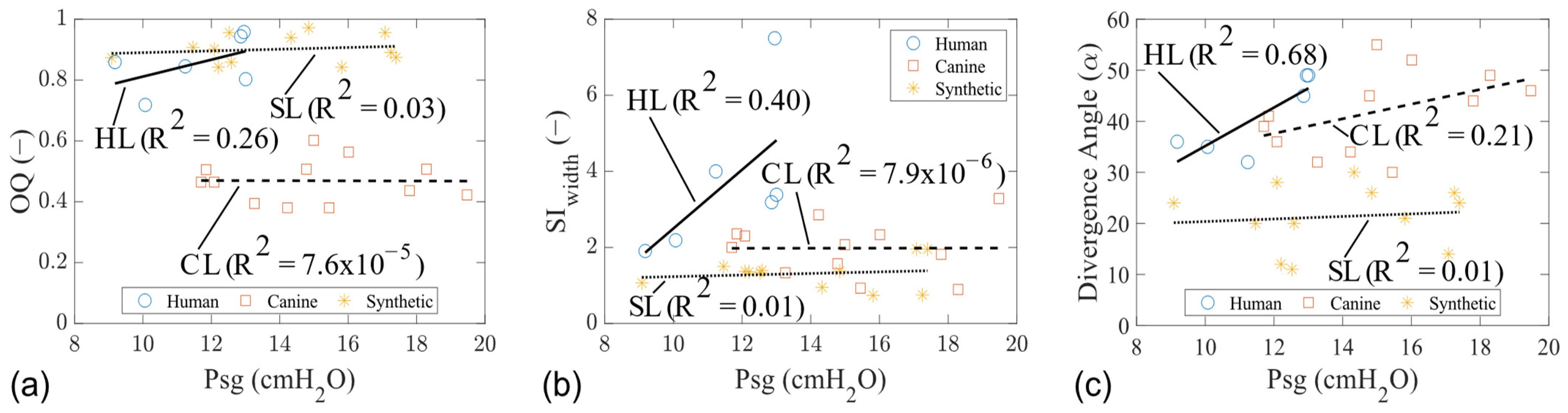
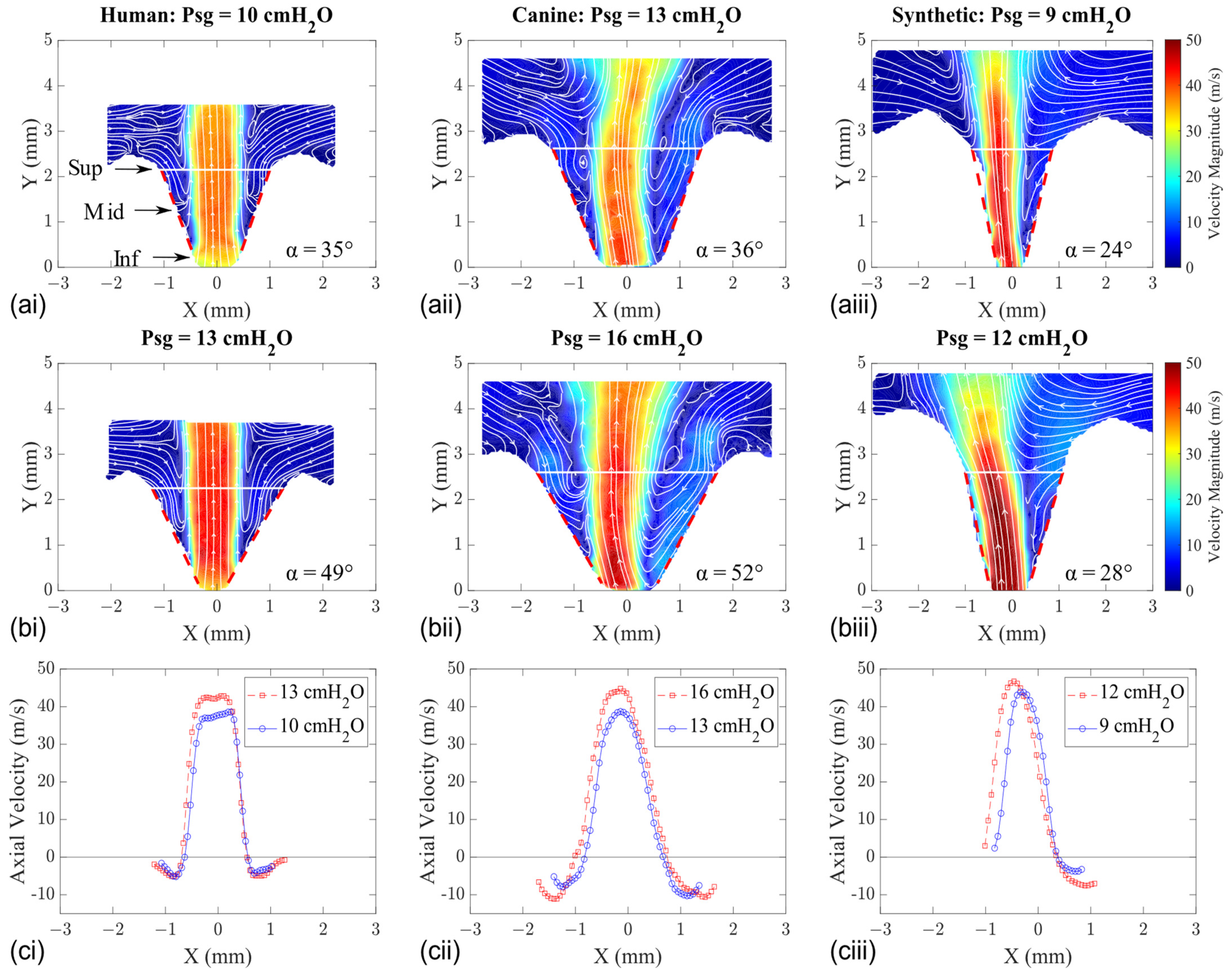
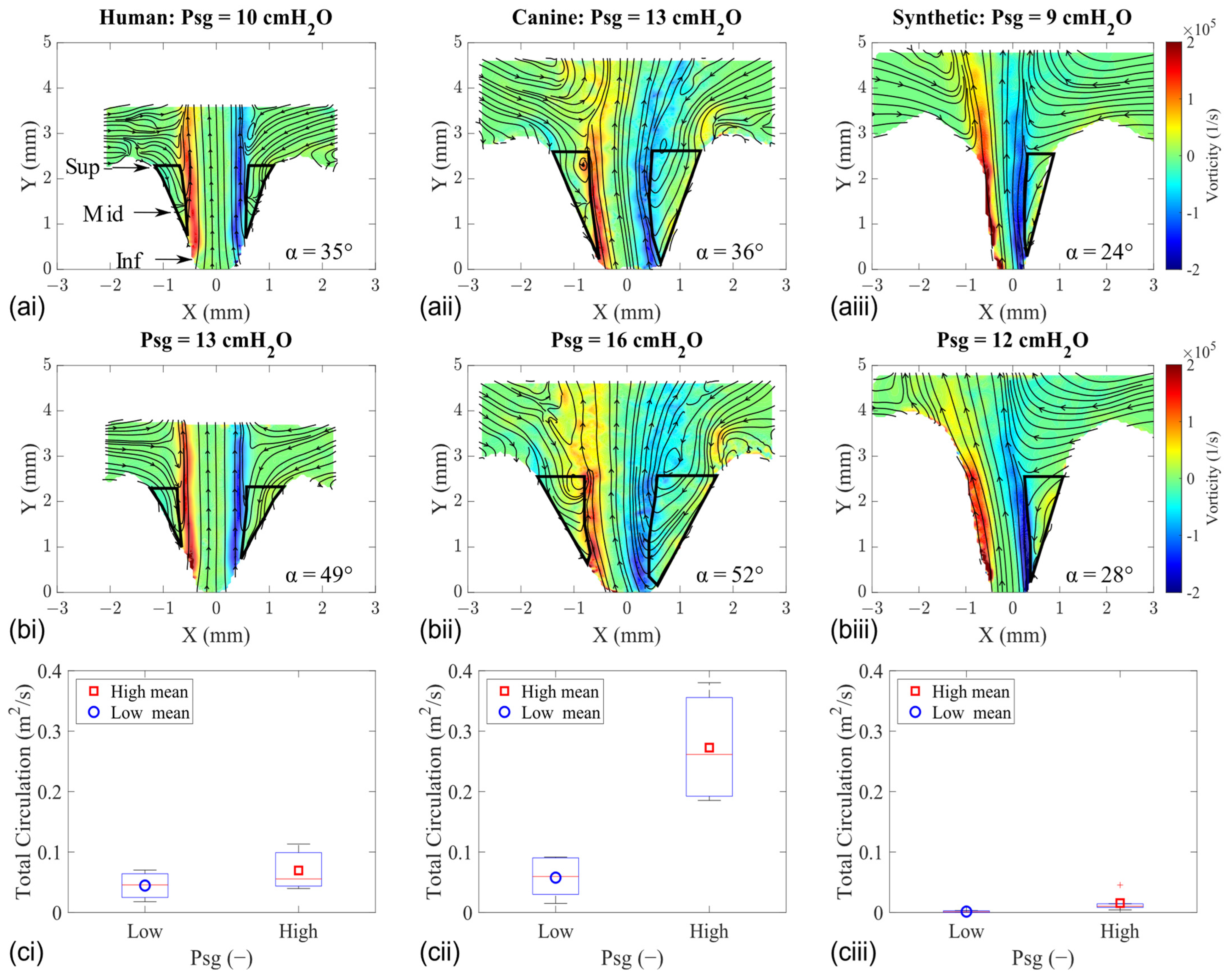
3.1. Folds’ Displacement and Glottal Geometry Characteristics
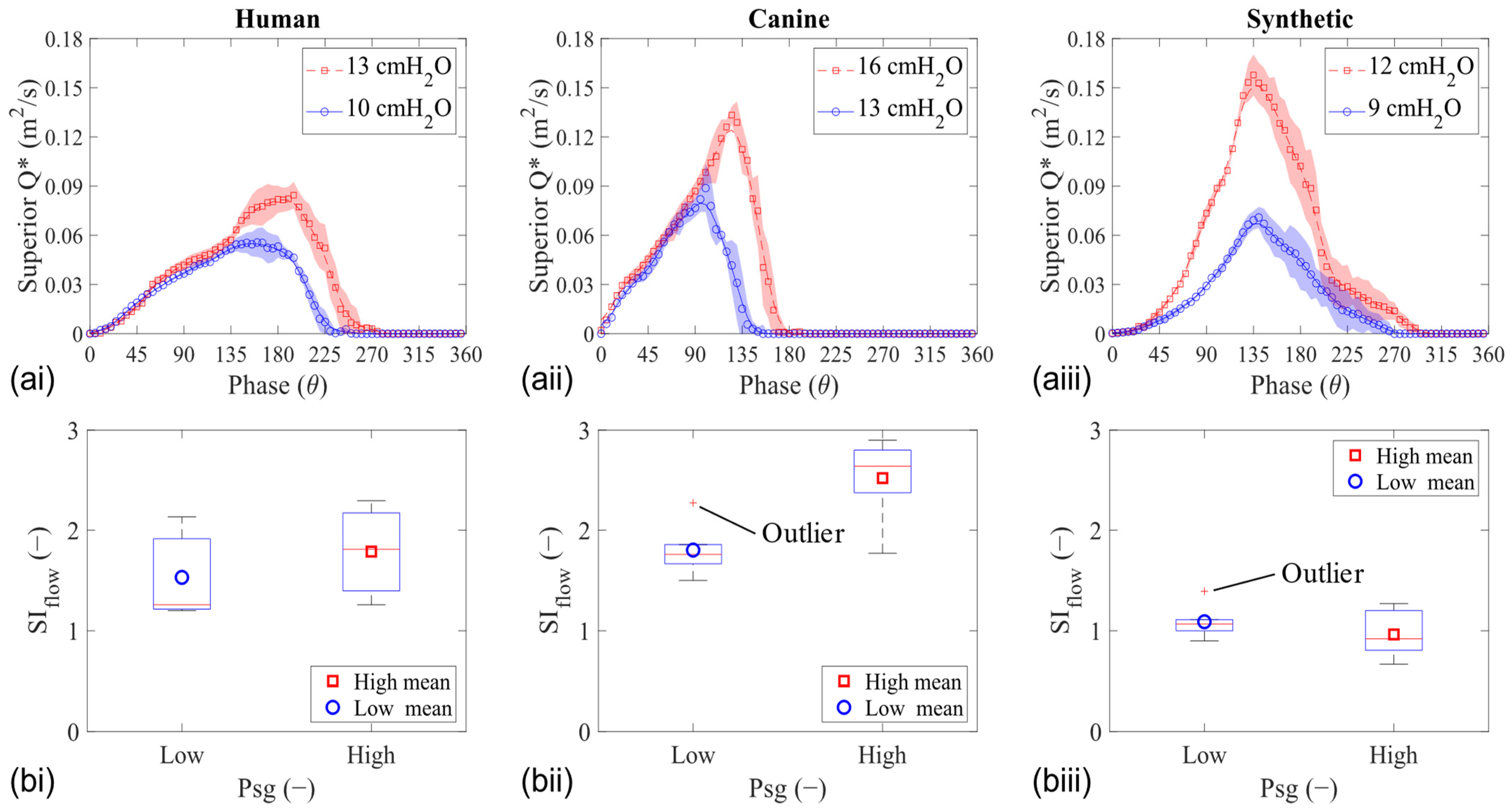
3.2. Glottal Flow Characteristics
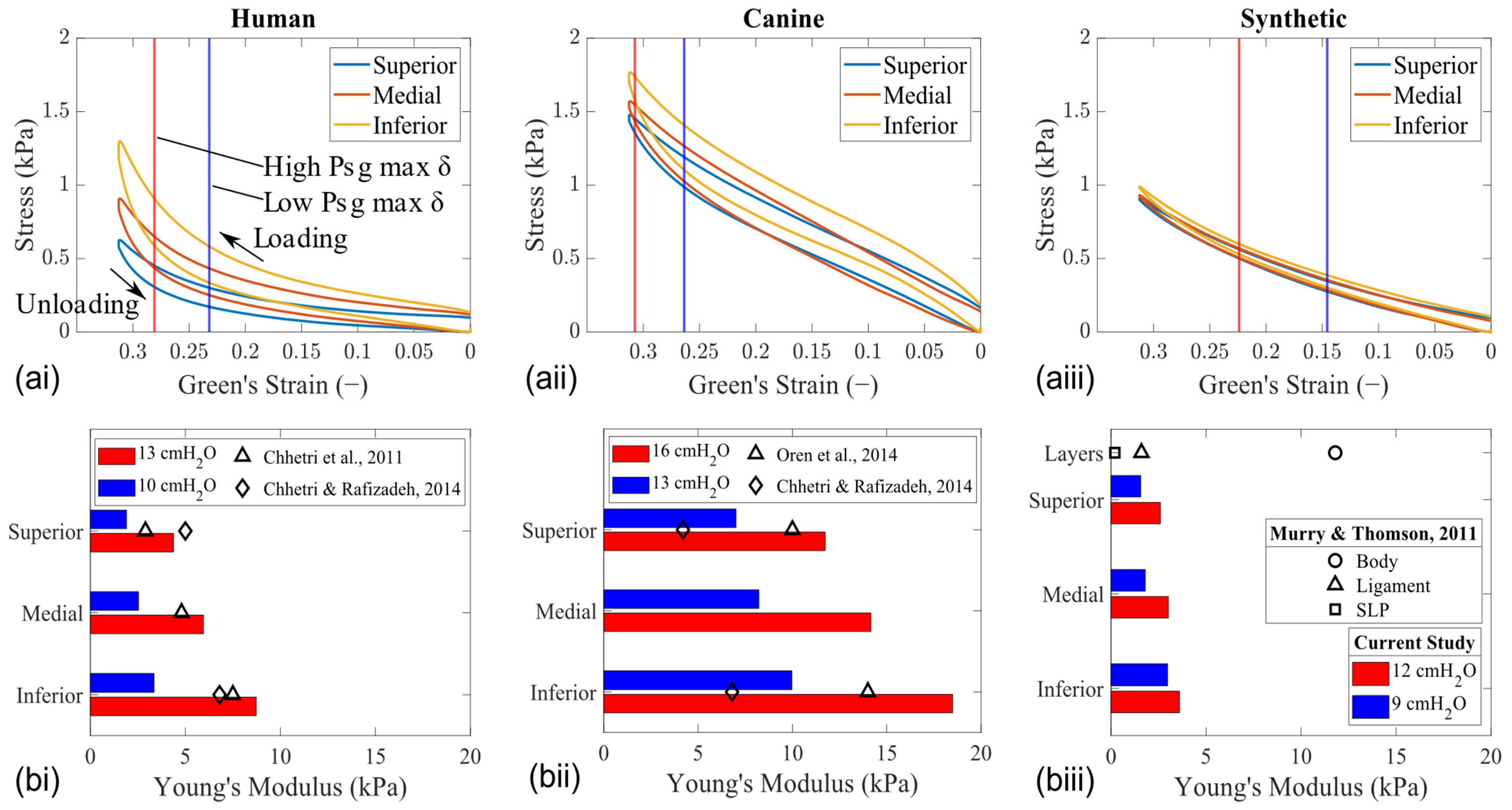
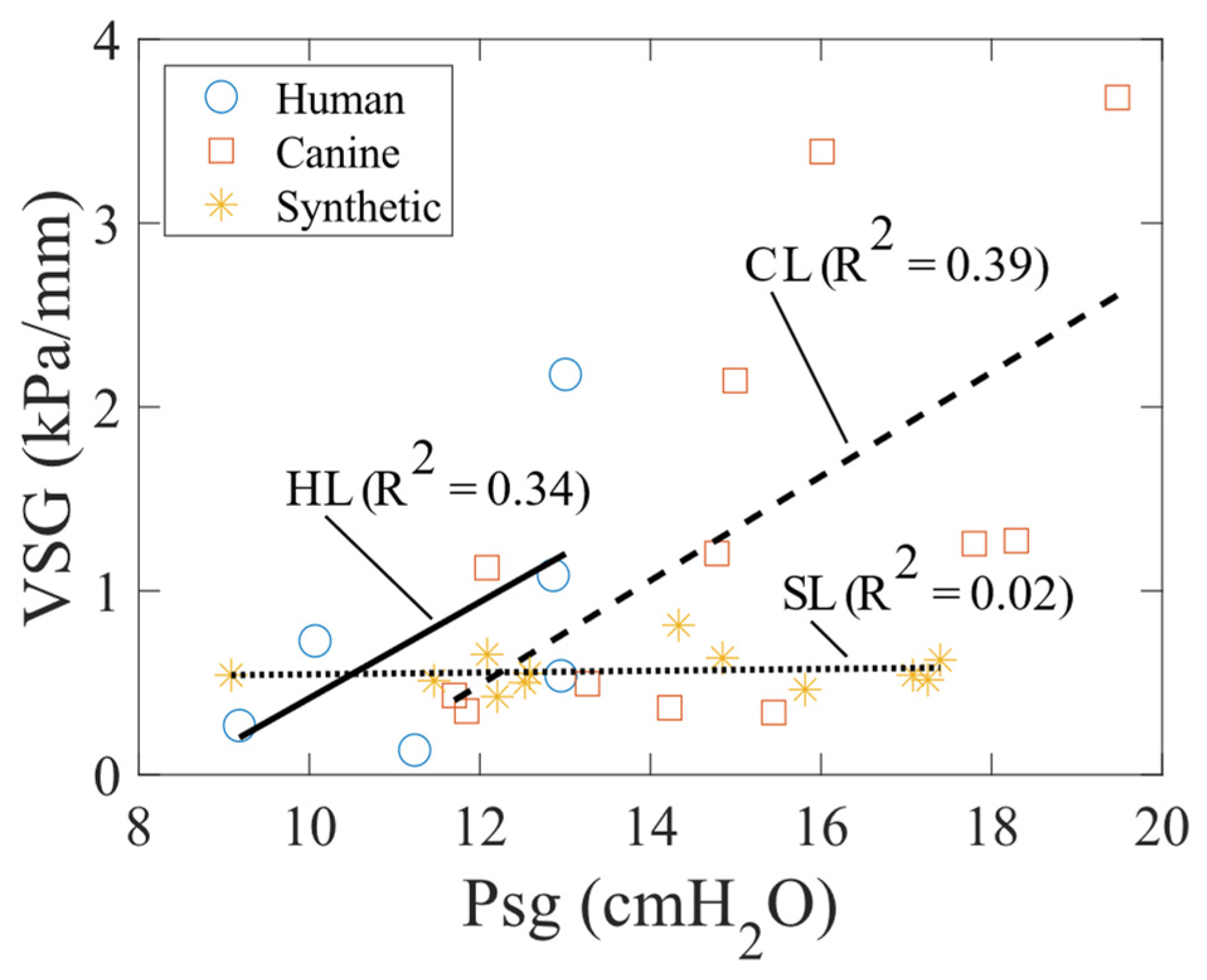
3.3. Elasticity Characteristics
3.4. Summary
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Flanagan, J.L. Speech Analysis Synthesis and Perception; Springer Science & Business Media: Berlin, Germany, 1972. [Google Scholar]
- Zhang, Z. Mechanics of human voice production and control. J. Acoust. Soc. Am. 2016, 140, 2614–2635. [Google Scholar] [CrossRef]
- Fant, G. The source filter concept in voice production. STL-QPSR 1981, 1, 21–37. [Google Scholar]
- Stevens, K.N. Acoustic Phonetics; MIT Press: Cambridge, MA, USA, 2000. [Google Scholar]
- de Luzan, C.F.; Oren, L.; Gutmark, E.; Khosla, S.M. Quantification of the intraglottal pressure induced by flow separation vortices using large eddy simulation. J. Voice 2021, 35, 822–831. [Google Scholar] [CrossRef] [PubMed]
- Jiang, W.; Zheng, X.; Farbos de Luzan, C.; Oren, L.; Gutmark, E.; Xue, Q. The effects of negative pressure induced by flow separation vortices on vocal fold dynamics during voice production. Bioengineering 2023, 10, 1215. [Google Scholar] [CrossRef]
- Oren, L.; Khosla, S.; Dembinski, D.; Ying, J.; Gutmark, E. Direct measurement of planar flow rate in an excised canine larynx model. Laryngoscope 2015, 125, 383–388. [Google Scholar] [CrossRef]
- Sundström, E.; Oren, L.; de Luzan, C.F.; Gutmark, E.; Khosla, S. Fluid-structure interaction analysis of aerodynamic and elasticity forces during vocal fold vibration. J. Voice 2022. [Google Scholar] [CrossRef]
- Barber, R.E.; Schultheiss, M.J. Effect of nozzle geometry on off-design performance of partial admission impulse turbines. Sundstrand Rep. AER 1967, 486, 49–52. [Google Scholar]
- Östlund, J. Flow Processes in Rocket Engine Nozzles with Focus on Flow Separation and Side-Loads. Doctoral Dissertation, Royal Institute of Technology, Stockholm, Sweden, 2002. [Google Scholar]
- Hirano, M. Clinical Examination of Voice; Springer: Berlin/Heidelberg, Germany, 1981. [Google Scholar]
- Khosla, S.; Murugappan, S.; Gutmark, E. What can vortices tell us about vocal fold vibration and voice production. Curr. Opin. Otolaryngol. Head Neck Surg. 2008, 16, 183–187. [Google Scholar] [CrossRef]
- Farbos de Luzan, C.; Maddox, A.; Oren, L.; Gutmark, E.; Howell, R.J.; Khosla, S.M. Impact of vertical stiffness gradient on the maximum divergence angle. Laryngoscope 2021, 131, E1934–E1940. [Google Scholar] [CrossRef]
- Titze, I.R. Vocal efficiency. J. Voice 1992, 6, 135–138. [Google Scholar] [CrossRef]
- Fraile, R.; Godino-Llorente, J.I. Cepstral peak prominence: A comprehensive analysis. Biomed. Signal Process. Control. 2014, 14, 42–54. [Google Scholar] [CrossRef]
- Hillenbrand, J.; Cleveland, R.A.; Erickson, R.L. Acoustic correlates of breathy vocal quality. J. Speech Lang. Hear. Res. 1994, 37, 769–778. [Google Scholar] [CrossRef] [PubMed]
- Ishizaka, K.; Flanagan, J.L. Synthesis of voiced sounds from a two-mass model of the vocal cords. Bell Syst. Tech. J. 1972, 51, 1233–1268. [Google Scholar] [CrossRef]
- Chhetri, D.K.; Zhang, Z.; Neubauer, J. Measurement of young’s modulus of vocal folds by indentation. J. Voice 2011, 25, 1–7. [Google Scholar] [CrossRef] [PubMed]
- Chhetri, D.K.; Rafizadeh, S. Young’s modulus of canine vocal fold cover layers. J. Voice 2014, 28, 406–410. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Oren, L.; Dembinski, D.; Gutmark, E.; Khosla, S. Characterization of the vocal fold vertical stiffness in a canine model. J. Voice 2014, 28, 297–304. [Google Scholar] [CrossRef] [PubMed]
- Dembinski, D.; Oren, L.; Gutmark, E.; Khosla, S.M. Biomechanical measurements of vocal fold elasticity. OA Tissue Eng. 2014, 2, 1–5. [Google Scholar]
- Geng, B.; Xue, Q.; Zheng, X. The effect of vocal fold vertical stiffness variation on voice production. J. Acoust. Soc. Am. 2016, 140, 2856–2866. [Google Scholar] [CrossRef] [PubMed]
- Kim, M.J.; Hunter, E.J.; Titze, I.R. Comparison of human, canine, and ovine laryngeal dimensions. Ann. Otol. Rhinol. Laryngol. 2004, 113, 60–68. [Google Scholar] [CrossRef] [PubMed]
- Titze, I.R. The Myoelastic Aerodynamic Theory of Phonation; National Center for Voice and Speech: Iowa City, IA, USA, 2006. [Google Scholar]
- Alipour, F.; Finnegan, E.M.; Jaiswal, S. Phonatory characteristics of the excised human larynx in comparison to other species. J. Voice 2013, 27, 441–447. [Google Scholar] [CrossRef]
- Murray, P.R.; Thomson, S.L. Synthetic, multi-layer, self-oscillating vocal fold model fabrication. J. Vis. Exp. 2011, 58, e3498. [Google Scholar]
- Murray, P.R.; Thomson, S.L. Vibratory responses of synthetic, self-oscillating vocal fold models. J. Acoust. Soc. Am. 2012, 132, 3428–3438. [Google Scholar] [CrossRef] [PubMed]
- Spencer, M.; Siegmund, T.; Mongeau, L. Determination of superior surface strains and stresses, and vocal fold contact pressure in a synthetic larynx model using digital image correlation. J. Acoust. Soc. Am. 2008, 123, 1089–1103. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z. Vibration in a self-oscillating vocal fold model with left-right asymmetry in body-layer stiffness. J. Acoust. Soc. Am. 2010, 128, EL279–EL285. [Google Scholar] [CrossRef]
- Pickup, B.A.; Thomson, S.L. Influence of asymmetric stiffness on the structural and aerodynamic response of synthetic vocal fold models. J. Biomech. 2009, 42, 2219–2225. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Hieu Luu, T. Asymmetric vibration in a two-layer vocal fold model with left-right stiffness asymmetry: Experiment and simulation. J. Acoust. Soc. Am. 2012, 132, 1626–1635. [Google Scholar] [CrossRef]
- Hilton, B.A.; Thomson, S.L. Aerodynamic-induced effects of artificial subglottic stenosis on vocal fold model phonatory response. J. Voice 2022, in press. [Google Scholar] [CrossRef]
- Morel, T. Comprehensive Design of Axisymmetric Wind Tunnel Contractions. J. Fluids Eng. 1975, 97, 225–233. [Google Scholar] [CrossRef]
- Mehta, R.D. Turbulent boundary layer perturbed by a screen. AIAA J. 1985, 23, 1335–1342. [Google Scholar] [CrossRef]
- Oren, L.; Khosla, S.; Gutmark, E. Intraglottal geometry and velocity measurements in canine larynges. J. Acoust. Soc. Am. 2014, 135, 380–388. [Google Scholar] [CrossRef]
- Dion, G.R.; Lavoie, J.; Coelho, P.; Amin, M.R.; Branski, R.C. Automated indentation mapping of vocal fold structure and cover properties across species. Laryngoscope 2019, 129, E26–E31. [Google Scholar] [CrossRef]
- Polyanin, A.D.; Manzhirov, A.V. Handbook of Mathematics for Engineers and Scientists; Chapman and Hall/CRC: Boca Raton, FL, USA, 2006. [Google Scholar]
- Schuberth, S.; Hoppe, U.; Döllinger, M.; Lohscheller, J.; Eysholdt, U. High-precision measurement of the vocal fold length and vibratory amplitudes. Laryngoscope 2002, 112, 1043–1049. [Google Scholar] [CrossRef] [PubMed]
- Motie-Shirazi, M.; Zañartu, M.; Peterson, S.D.; Mehta, D.D.; Kobler, J.B.; Hillman, R.E.; Erath, B.D. Toward development of a vocal fold contact pressure probe: Sensor characterization and validation using synthetic vocal fold models. Appl. Sci. 2019, 9, 3002. [Google Scholar] [CrossRef]
- Oren, L.; Khosla, S.; Gutmark, E. Medial surface dynamics as a function of subglottal pressure in a canine larynx model. J. Voice 2021, 35, 69–76. [Google Scholar] [CrossRef]
- Lohscheller, J.; Švec, J.G.; Döllinger, M. Vocal fold vibration amplitude, open quotient, speed quotient and their variability along glottal length: Kymographic data from normal subjects. Logop. Phoniatr. Vocol. 2013, 38, 182–192. [Google Scholar] [CrossRef]
- Baken, R.J.; Orlikoff, R.F. Clinical Measurement of Speech and Voice; Singular Thomson Learning: San Diego, CA, USA, 2000. [Google Scholar]
- Schutte, H.K. The Efficiency of Voice Production. Doctoral Dissertation, University of Groningen, Groningen, The Netherlands, 1980. [Google Scholar]
- Greenwood, T.E.; Thomson, S.L. Embedded 3D printing of multi-layer, self-oscillating vocal fold models. J. Biomech. 2021, 121, 110388. [Google Scholar] [CrossRef] [PubMed]
- Romero, R.G.; Colton, M.B.; Thomson, S.L. 3D-printed synthetic vocal fold models. J. Voice 2021, 35, 685–694. [Google Scholar] [CrossRef]
- Young, C.A.; O’Bannon, M.; Thomson, S.L. Three-dimensional printing of ultrasoft silicone with a functional stiffness gradient. 3D Print. Addit. Manuf. 2022, 11, 435–445. [Google Scholar] [CrossRef]

| Model | (cmH2O) | (Hz) | (LPM) | (−) | (−) | (Deg.) | flow(−) | (m/s·θ) | (m2/s) | (−) | (kPa/mm) |
|---|---|---|---|---|---|---|---|---|---|---|---|
| Human | 10.26 ± 1.03 | 215.67 ± 75.70 | 15.93 ± 8.80 | 0.81 ± 0.08 | 2.70 ± 1.14 | 37.00 ± 5.57 | 1.53 ± 0.52 | 8.66 × 10−4 ± 3.43 × 10−4 | 4.46 × 10−2 ± 2.62 × 10−2 | 1.64 × 10−5 ± 7.95 × 10−6 | 0.34 ± 0.27 |
| 12.94 ± 0.07 | 232.00 ± 79.23 | 24.48 ± 12.87 | 0.90 ± 0.09 | 3.69 ± 2.43 | 48.33 ± 3.06 | 1.79 ± 0.52 | 1.20 × 10−3 ± 5.24 × 10−4 | 6.95 × 10−2 ± 3.88 × 10−2 | 2.30 × 10−5 ± 1.10 × 10−5 | 0.90 ± 0.63 | |
| Canine | 13.23 ± 1.31 | 94.33 ± 25.91 | 24.16 ± 6.18 | 0.45 ± 0.45 | 1.96 ± 0.65 | 35.33 ± 3.82 | 1.80 ± 0.24 | 1.98 × 10−3 ± 3.53 × 10−4 | 5.77 × 10−2 ± 3.00 × 10−2 | 3.68 × 10−5 ± 1.72 × 10−5 | 0.49 ± 0.28 |
| 17.02 ± 1.70 | 111.67 ± 25.01 | 31.24 ± 5.91 | 0.49 ± 0.49 | 2.20 ± 0.73 | 48.50 ± 3.95 | 2.52 ± 0.38 | 2.73 × 10−3 ± 3.59 × 10−4 | 2.73 × 10−1 ± 7.89 × 10−2 | 5.40 × 10−5 ± 2.41 × 10−5 | 2.13 ± 1.05 | |
| Synthetic | 12.40 ± 1.80 | 155.67 ± 25.55 | 29.93 ± 8.26 | 0.87 ± 0.78 | 1.23 ± 0.26 | 19.00 ± 5.80 | 1.09 ± 0.15 | 9.99 × 10−4 ± 5.66 × 10−4 | 1.46 × 10−3 ± 1.16 × 10−3 | 1.30 × 10−5 ± 2.54 × 10−6 | 0.45 ± 0.14 |
| 15.48 ± 1.97 | 167.17 ± 17.10 | 39.85 ± 9.76 | 0.92 ± 0.82 | 1.39 ± 0.46 | 24.67 ± 5.12 | 0.96 ± 0.21 | 1.45 × 10−3 ± 3.20 × 10−4 | 1.55 × 10−2 ± 1.37 × 10−2 | 1.92 × 10−5 ± 3.31 × 10−6 | 0.54 ± 0.20 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Michaud-Dorko, J.; Farbos de Luzan, C.; Dion, G.R.; Gutmark, E.; Oren, L. Comparison of Aerodynamic and Elastic Properties in Tissue and Synthetic Models of Vocal Fold Vibrations. Bioengineering 2024, 11, 834. https://doi.org/10.3390/bioengineering11080834
Michaud-Dorko J, Farbos de Luzan C, Dion GR, Gutmark E, Oren L. Comparison of Aerodynamic and Elastic Properties in Tissue and Synthetic Models of Vocal Fold Vibrations. Bioengineering. 2024; 11(8):834. https://doi.org/10.3390/bioengineering11080834
Chicago/Turabian StyleMichaud-Dorko, Jacob, Charles Farbos de Luzan, Gregory R. Dion, Ephraim Gutmark, and Liran Oren. 2024. "Comparison of Aerodynamic and Elastic Properties in Tissue and Synthetic Models of Vocal Fold Vibrations" Bioengineering 11, no. 8: 834. https://doi.org/10.3390/bioengineering11080834
APA StyleMichaud-Dorko, J., Farbos de Luzan, C., Dion, G. R., Gutmark, E., & Oren, L. (2024). Comparison of Aerodynamic and Elastic Properties in Tissue and Synthetic Models of Vocal Fold Vibrations. Bioengineering, 11(8), 834. https://doi.org/10.3390/bioengineering11080834









