CARING: Cannula for Alleviation of Retinal Injury Caused by Needle Fluidic Gashing
Abstract
1. Introduction
2. Materials and Methods
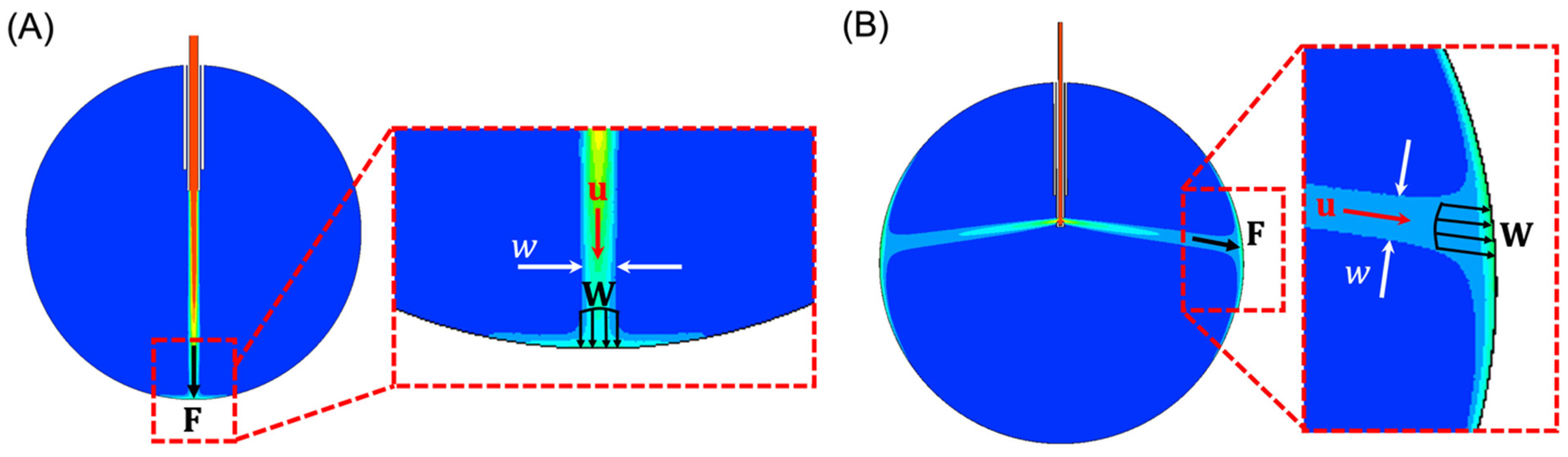
2.1. Fluidic Analytical Modeling
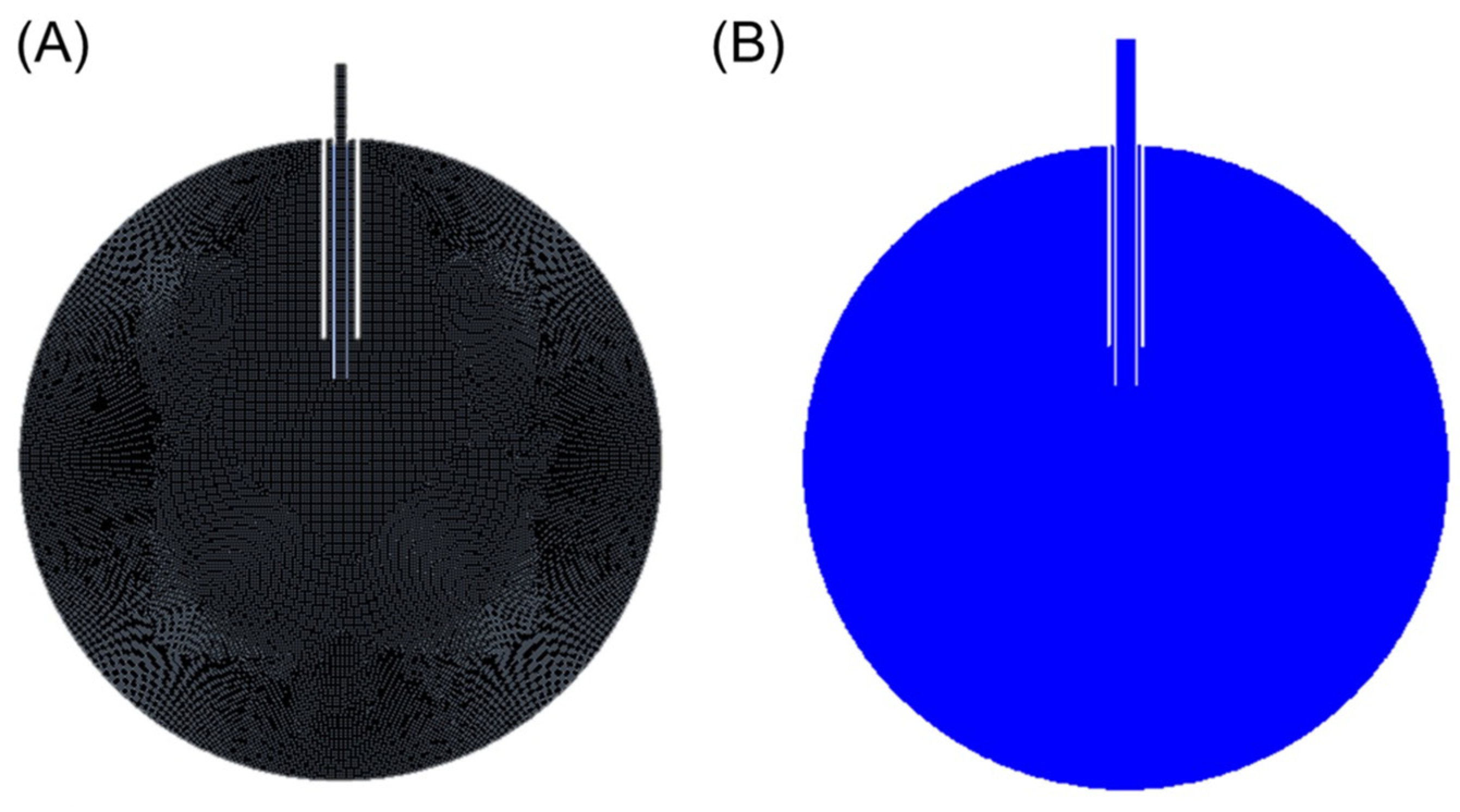
2.2. Fluidic FEA Simulation
- The cross-sectional area of the infusing fluid stream is assumed to have a circular cross-section with a diameter that is equivalent to the width, , of the infusing fluid stream in 2-D, as shown in Figure 1A,B.
- The total effective applied force, , is the cumulative sum of the distributed force, , induced by the infusing fluid stream across the area defined by the width, . Note that and are assumed to have the same direction as , written as , where is the magnitude of .
- The density of the infusing fluid stream striking the retinal wall is equal to the density of water multiplied by the water volume fraction provided by the FEA model.
3. Results
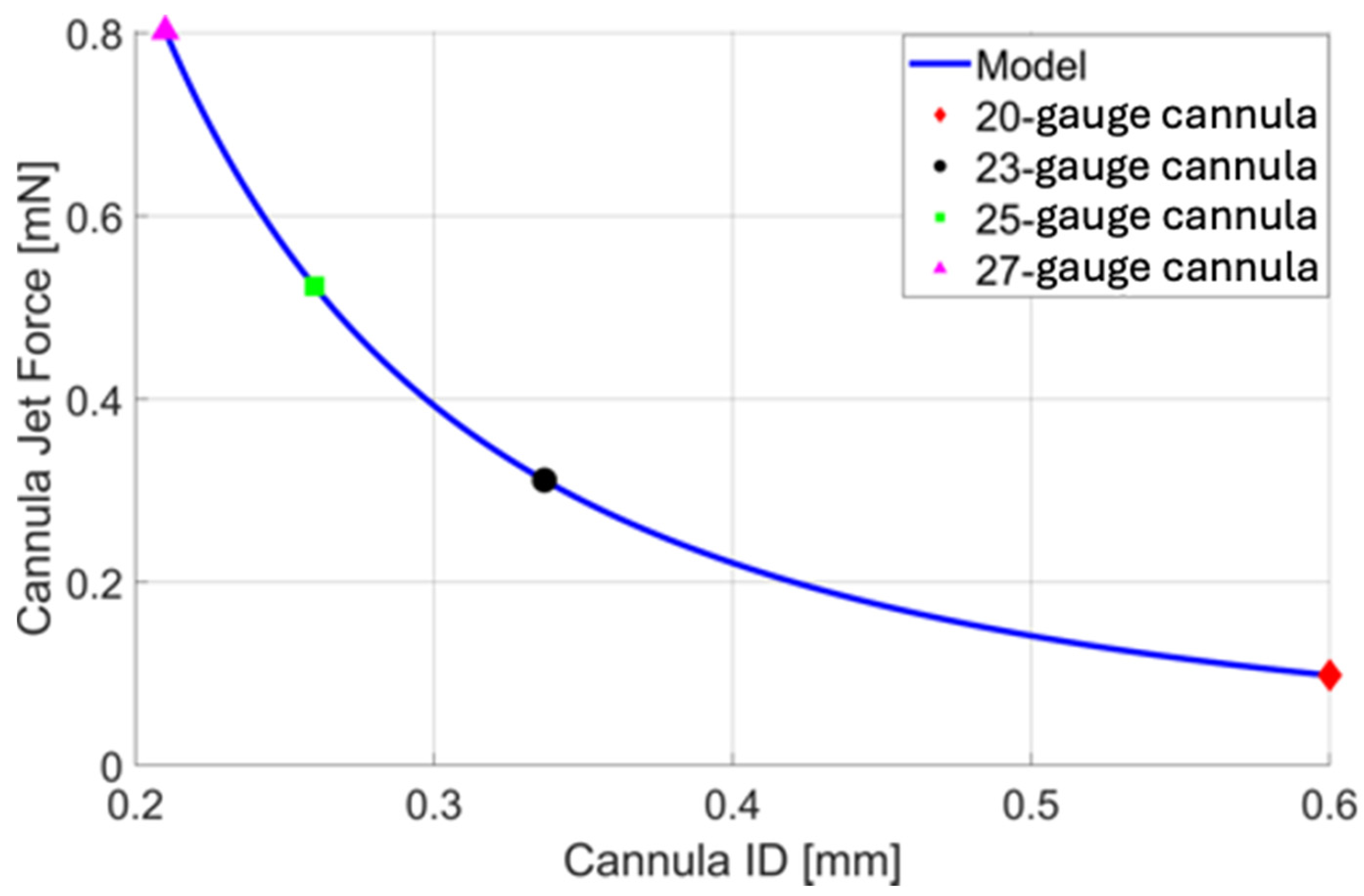
3.1. Analytical Evaluation of Nominal Cannulas
3.2. FEA Evaluation of Nominal Cannulas
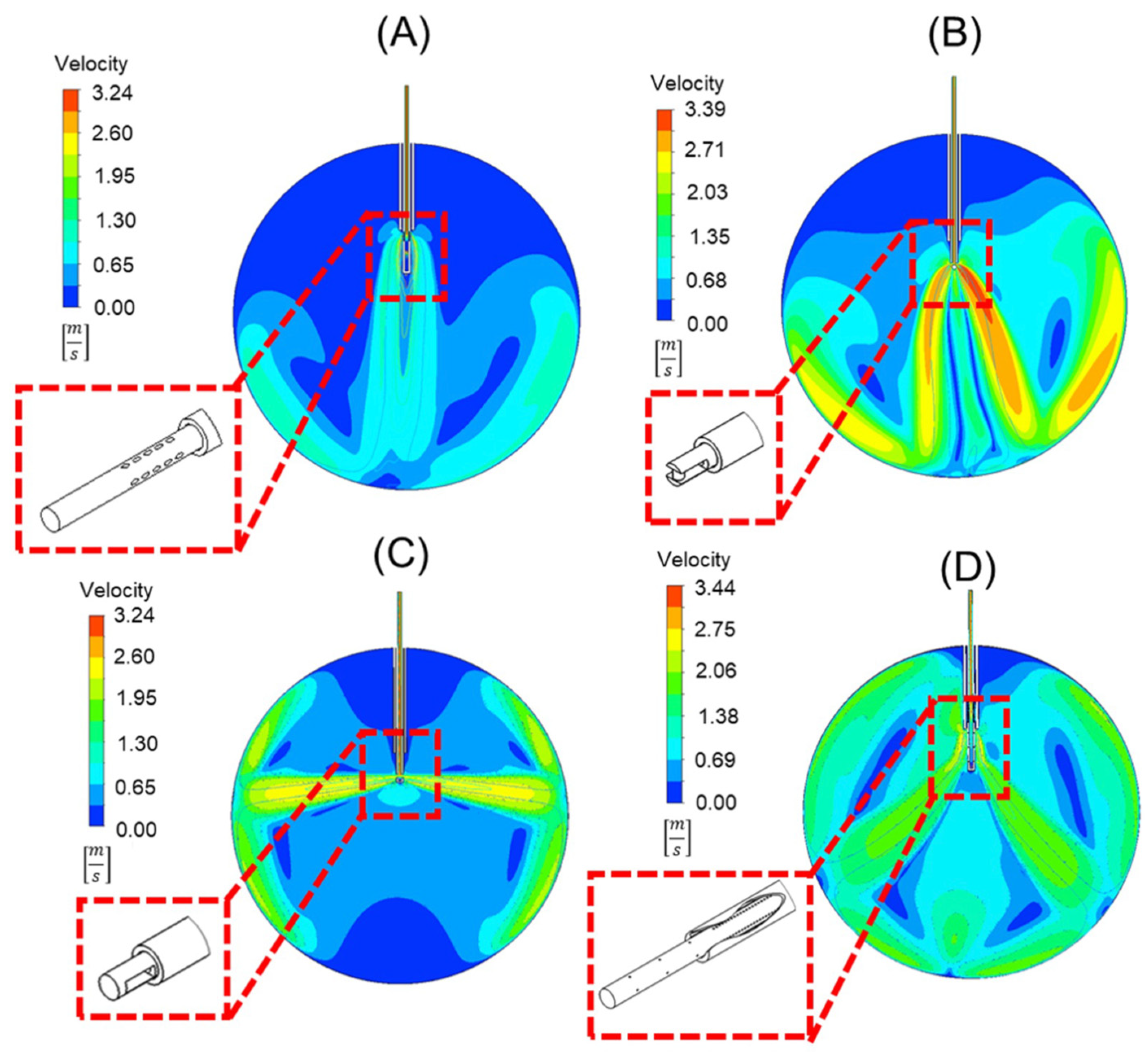
3.3. Alternative Cannula Designs
4. Discussion
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lalezary, M.; Kim, S.J.; Jiramongkolchai, K.; Recchia, F.M.; Agarwal, A.; Sternberg, P., Jr. Long-Term Trends in Intraocular Pressure after Pars Plana Vitrectomy. Retina 2011, 31, 679–685. [Google Scholar] [CrossRef] [PubMed]
- Scott, M.N.; Weng, C.Y. The Evolution of Pars Plana Vitrectomy to 27-G Microincision Vitrectomy Surgery. Int. Ophthalmol. Clin. 2016, 56, 97–111. [Google Scholar] [CrossRef] [PubMed]
- Mohamed, S.; Claes, C.; Tsang, C.W. Review of Small Gauge Vitrectomy: Progress and Innovations. J. Ophthalmol. 2017, 2017, 6285869. [Google Scholar] [CrossRef] [PubMed]
- Omari, A.; Mahmoud, T.H. Vitrectomy; StatPearls Publishing: Treasure Island, FL, USA, 2020. [Google Scholar]
- Chen, E. 25-Gauge Transconjunctival Sutureless Vitrectomy. Curr. Opin. Ophthalmol. 2007, 18, 188–193. [Google Scholar] [CrossRef] [PubMed]
- Spirn, M.J. Comparison of 25, 23, and 20-Gauge Vitrectomy. Curr. Opin. Ophthalmol. 2009, 20, 195–199. [Google Scholar] [CrossRef] [PubMed]
- Okamoto, F.; Okamoto, C.; Sakata, N.; Hiratsuka, K.; Yamane, N.; Hiraoka, T.; Kaji, Y.; Oshika, T. Changes in Corneal Topography after 25-gauge Transconjunctival Sutureless Vitrectomy versus after 20-Gauge Standard Vitrectomy. Ophthalmology 2007, 114, 2138–2141. [Google Scholar] [CrossRef] [PubMed]
- Williams, G.A. 25-, 23-, or 20-gauge Instrumentation for Vitreous Surgery. Eye 2008, 22, 1263–1266. [Google Scholar] [CrossRef] [PubMed]
- Gupta, O.P.; Weichel, E.D.; Regillo, C.D.; Fineman, M.S.; Kaiser, R.S.; Ho, A.C.; McNamara, J.A.; Vander, J.F. Postoperative Complications Associated with 25-Gauge Pars Plana Vitrectomy. Ophthalmic Surg. Lasers Imaging Retin. 2007, 38, 270–275. [Google Scholar] [CrossRef] [PubMed]
- Steel, D.H.; Charles, S. Vitrectomy Fluidics. Ophthalmologica 2011, 226, 27–35. [Google Scholar] [CrossRef] [PubMed]
- Zacharias, L.C.; Rezende, F.A.; Suzuki, A.C.; Tanaka, T.M.; Azevedo, B.M.; Takahashi, W.Y. Intraoperative Infusion-related Jet Stream Enlargement of Macular Hole. Retina 2016, 36, e31–e32. [Google Scholar] [CrossRef] [PubMed]
- Rishi, E.; Rishi, P.; Sharma, T.; Nagesha, C.K. Infusion Flow Related Retinal Breaks in 25G Vitrectomy. Acta Ophthalmol. 2018, 96, 100–102. [Google Scholar] [CrossRef] [PubMed]
- Bilgin, A.B.; Türkoğlu, E.B.; Ilhan, H.D.; Ünal, M.; Apaydin, K.C. Iatrogenic retinal breaks caused by infusion fluid during pars plana vitrectomy. Can. J. Ophthalmol. 2015, 50, 77–79. [Google Scholar] [CrossRef] [PubMed]
- Belenje, A.; Takkar, B.; Agarwal, K.; Tyagi, M.; Aggarwal, V.; Padhi, T.R.; Narayanan, R. Jet Stream-Related Iatrogenic Retinal Breaks during Vitreoretinal Surgery. Indian J. Ophthalmol. 2022, 70, 902–907. [Google Scholar] [CrossRef] [PubMed]
- Liu, W.; Wang, D.D.; Huang, S.Y.; Li, J.Q.; Wu, S.H.; Hang, L. A Bent Infusion Cannula for Vitreous Surgery. Am. J. Ophthalmol. 2005, 140, 151–152. [Google Scholar] [CrossRef] [PubMed]
- Hirata, A.; Yonemura, N.; Hasumura, T.; Murata, Y.; Negi, A.; Tanihara, H. New Infusion Cannula for Prevention of Retinal Damage by Infusion Air during Vitrectomy. Retina 2003, 23, 682–685. [Google Scholar] [CrossRef] [PubMed]
- Young, E. Numerical Simulations of the Mechanics of Vitrectomy. Ph.D. Thesis, Biomedical Engineering, University of California, Los Angeles, CA, USA, 2015. [Google Scholar]
- Henein, C.; Awwad, S.; Ibeanu, N.; Vlatakis, S.; Brocchini, S.; Khaw, P.T.; Bouremel, Y. Hydrodynamics of Intravitreal Injections into Liquid Vitreous Substitutes. Pharm 2019, 11, 371. [Google Scholar] [CrossRef] [PubMed]
- Hill, P.; Peterson, C. Mechanics and Thermodynamics of Propulsion, 2nd ed.; Pearson: London, UK, 2014. [Google Scholar]
- Fox, R.W.; McDonald, A.T.; Pritchard, P.J. Introduction to Fluid Mechanics, 6th ed.; John Wiley and Sons: Hoboken, NJ, USA, 2004. [Google Scholar]
- Rahman, R.; Murray, C.D.; Stephenson, J. Risk Factors for Iatrogenic Retinal Breaks Induced by Separation of Posterior Hyaloid Face during 23-gauge Pars Plana Vitrectomy. Eye 2013, 27, 652–656. [Google Scholar] [CrossRef] [PubMed]
- Gosse, E.; Newsom, R.; Lochhead, J. The Incidence and Distribution of Iatrogenic Retinal Tears in 20-Gauge and 23-Gauge Vitrectomy. Eye 2012, 26, 140–143. [Google Scholar] [CrossRef] [PubMed]
- Fujiwara, N.; Tomita, G.; Yagi, F. Incidence and Risk Factors of Iatrogenic Retinal Breaks: 20-Gauge versus 25-Gauge Vitrectomy for Idiopathic Macular Hole Repair. J. Ophthalmol. 2020, 2020, 5085180. [Google Scholar] [CrossRef] [PubMed]
- Tan, H.S.; Mura, M.; de Smet, M.D. Iatrogenic Retinal Breaks in 25-Gauge Macular Surgery. Am. J. Ophthalmol. 2009, 148, 427–430. [Google Scholar] [CrossRef] [PubMed]

| Paper | Patient | Procedure | Complication |
|---|---|---|---|
| Rishi et al. [12] | Case 1 | CEIOL * + 25 G PPV † for FTMH ‡ | Retinal break |
| Case 2 | 25 G PPV for FTMH | Retinal break > RRD § | |
| Case 3 | PPV for FTMH | Retinal break | |
| Case 4 | 25 G PPV for FTMH | Retinal break > RRD | |
| Bilgin et al. [15] | Case 1 | 25 G PPV for vitreous hemorrhage | Retinal break |
| Case 2 | 25 G PPV for silicone oil extraction, ERM || removal | Retinal break > RRD | |
| Case 3 | PPV for silicone oil extraction | Retinal break > RRD | |
| Belenje et al. [13] | Case 1 | 25 G PPV for FTMH | Retinal break > RRD |
| Zacharias et al. [11] | Case 1 | 23 G PPV for macular hole repair | Enlargement of macular hole |
| Needle | ID [mm] | OD [mm] | Reynold’s Number |
|---|---|---|---|
| 25-gauge | 0.26 | 0.52 | 917 |
| 20-gauge | 0.60 | 0.91 | 395 |
| 23-gauge | 0.34 | 0.64 | 707 |
| 27-gauge | 0.21 | 0.41 | 1135 |
| Gauge | 20 | 23 | 25 | 27 |
|---|---|---|---|---|
| Analytical Force [mN] | 0.098 | 0.311 | 0.523 | 0.802 |
| Model Force [mN] | 0.096 | 0.323 | 0.546 | 0.834 |
| Error [%] | 2.04% | 3.85% | 4.39% | 3.99% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rickels, K.L.; Gunderman, A.L.; McLellan, M.S.; Shamim, M.M.; Sanford, J.A.; Uwaydat, S.H. CARING: Cannula for Alleviation of Retinal Injury Caused by Needle Fluidic Gashing. Bioengineering 2024, 11, 718. https://doi.org/10.3390/bioengineering11070718
Rickels KL, Gunderman AL, McLellan MS, Shamim MM, Sanford JA, Uwaydat SH. CARING: Cannula for Alleviation of Retinal Injury Caused by Needle Fluidic Gashing. Bioengineering. 2024; 11(7):718. https://doi.org/10.3390/bioengineering11070718
Chicago/Turabian StyleRickels, Kaersti L., Anthony L. Gunderman, Mattie S. McLellan, Muhammad M. Shamim, Joseph A. Sanford, and Sami H. Uwaydat. 2024. "CARING: Cannula for Alleviation of Retinal Injury Caused by Needle Fluidic Gashing" Bioengineering 11, no. 7: 718. https://doi.org/10.3390/bioengineering11070718
APA StyleRickels, K. L., Gunderman, A. L., McLellan, M. S., Shamim, M. M., Sanford, J. A., & Uwaydat, S. H. (2024). CARING: Cannula for Alleviation of Retinal Injury Caused by Needle Fluidic Gashing. Bioengineering, 11(7), 718. https://doi.org/10.3390/bioengineering11070718






