Development of a Hydrogeological Conceptual Model of the Varaždin Alluvial Aquifer
Abstract
:1. Introduction
2. Materials and Methods
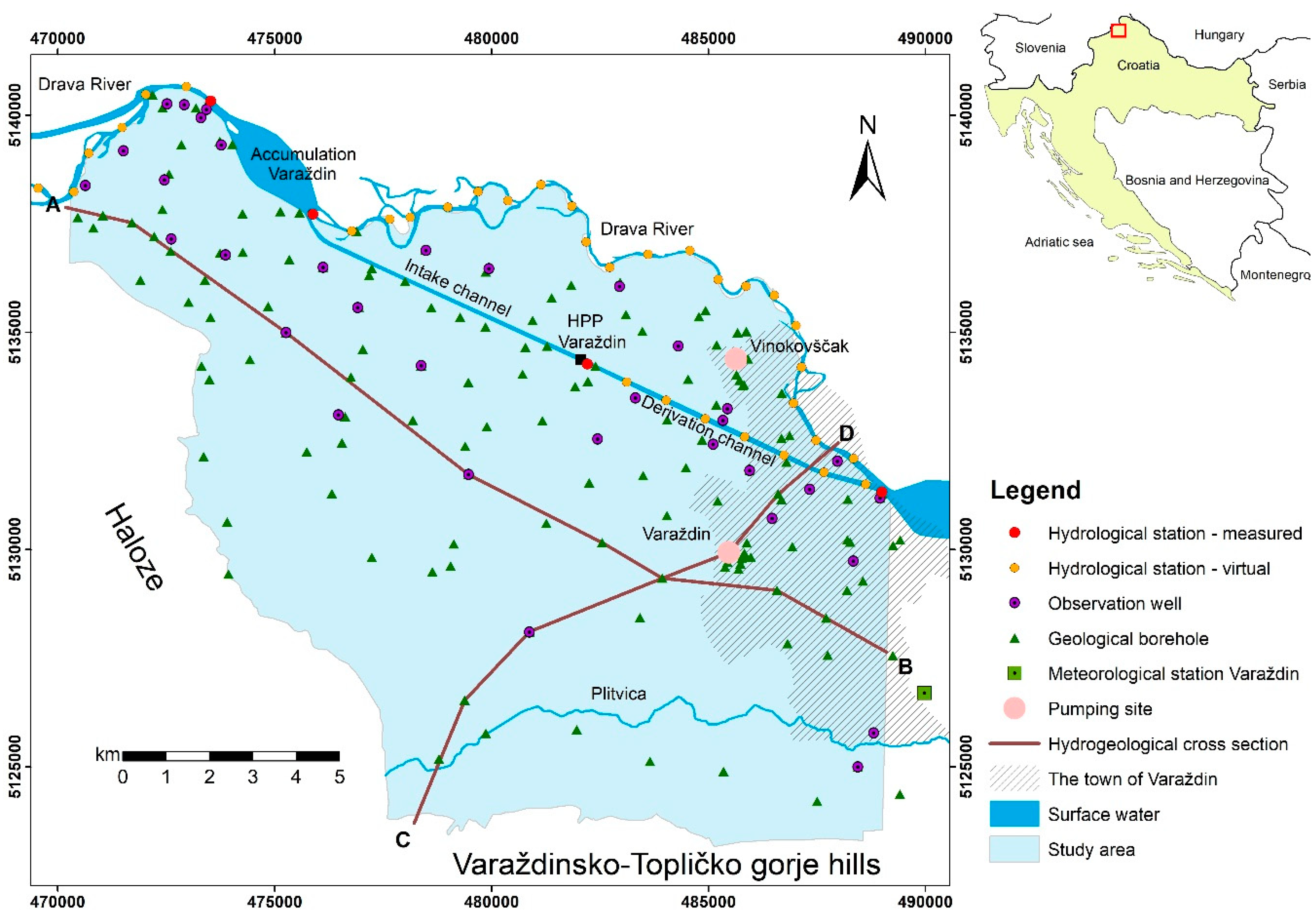
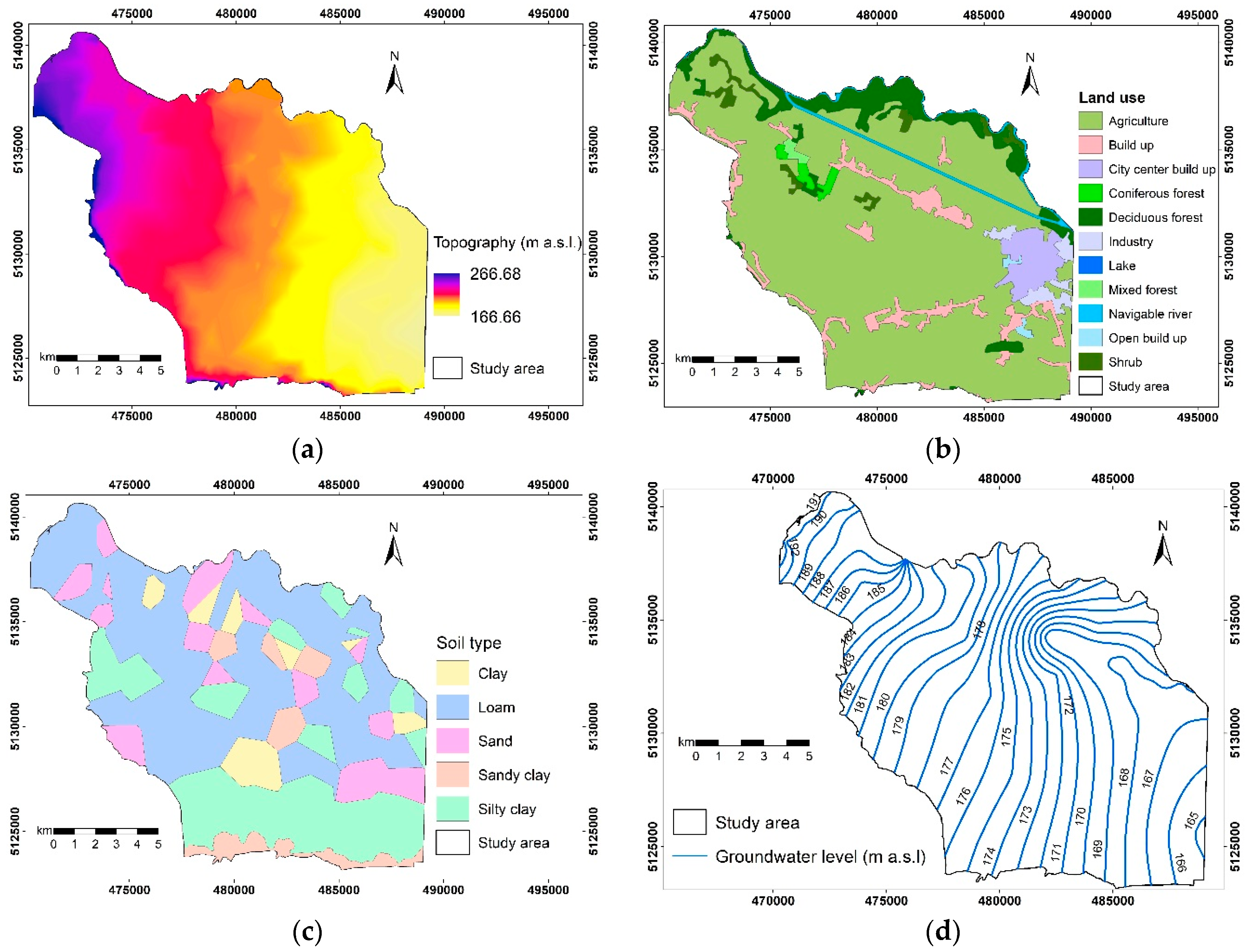
2.1. Study Area
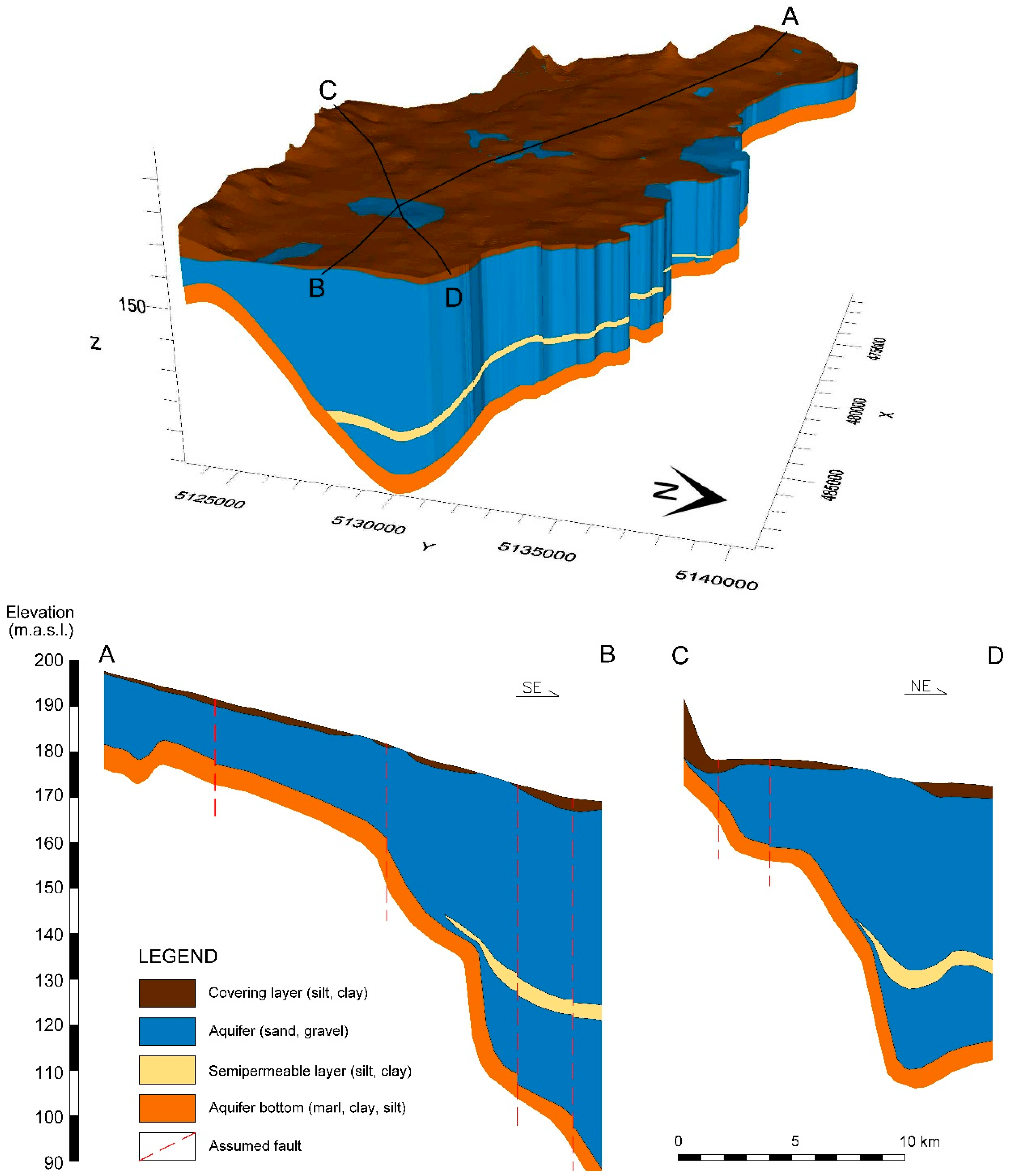
2.2. Aquifer Geometry
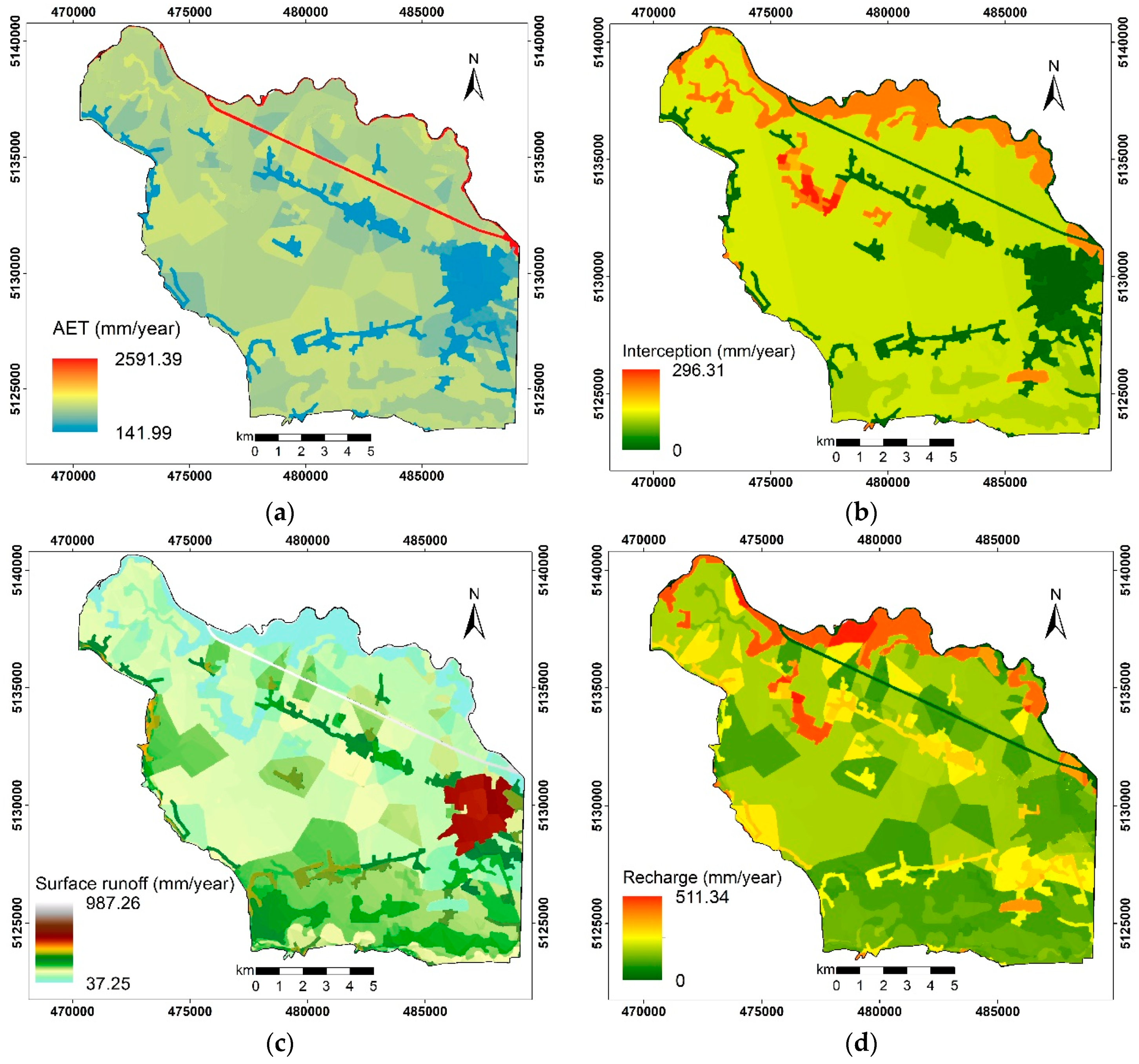
2.3. Groundwater Recharge from Precipitation
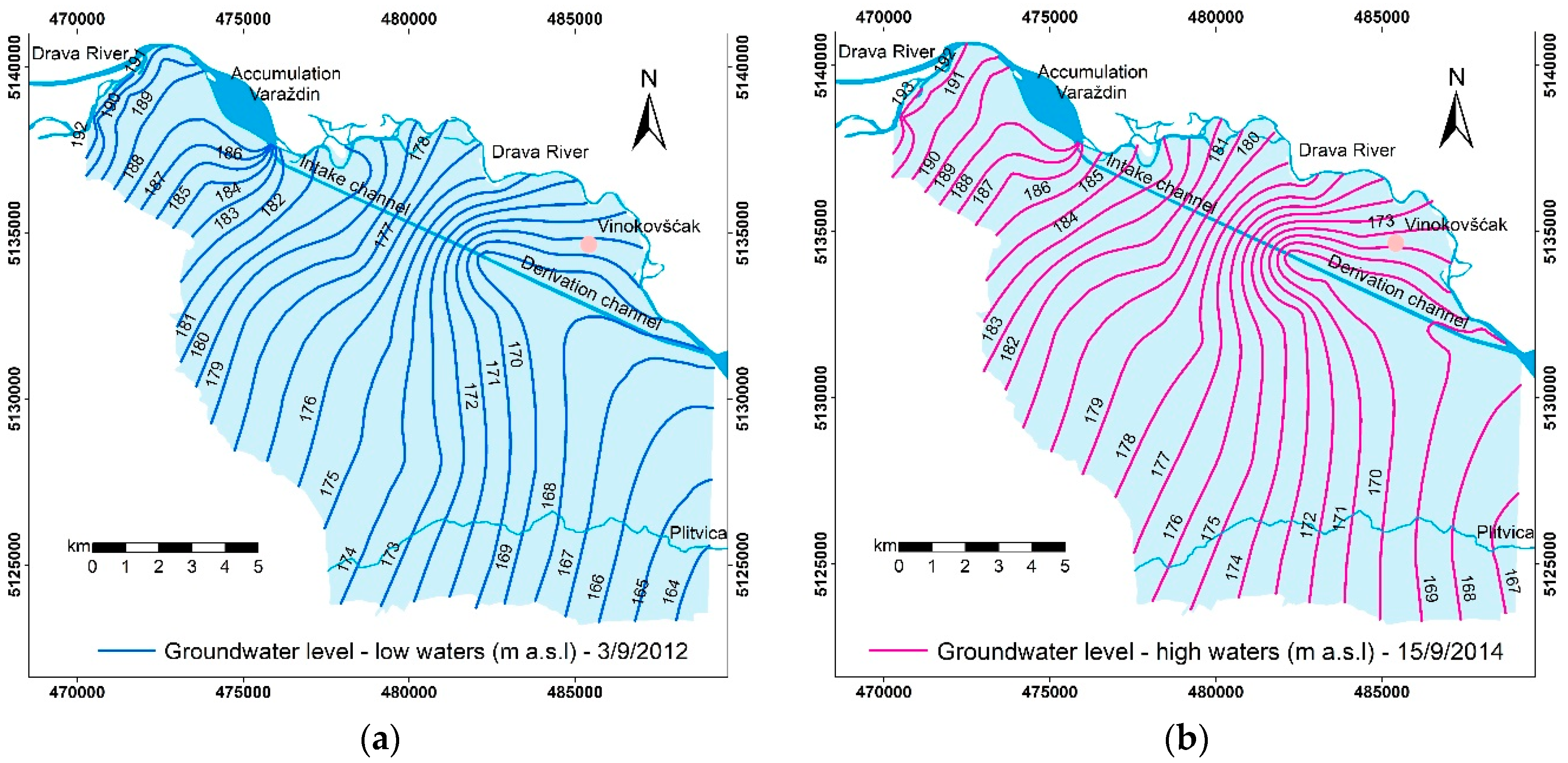
2.4. Boundary Conditions
3. Results and Discussion
3.1. Aquifer Geometry
3.2. Groundwater Recharge from Precipitation
3.3. Boundary Conditions
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Anderson, M.P.; Woessner, W.W.; Hunt, R.J. Modeling purpose and Conceptual Model. In Applied Groundwater Modeling—Simulation of Flow and Advective Transport, 2nd ed.; Academic Press, Inc.: San Diego, CA, USA, 2015; p. 630. [Google Scholar]
- Zheng, C.; Bennett, G.D. A Framework for Model Applications. In Applied Contaminant Transport Modeling, 2nd ed.; Wiley-Interscience: New York, NY, USA, 2002; p. 621. [Google Scholar]
- Enemark, T.; Peeters, L.J.M.; Mallants, D.; Batelaan, O. Hydrogeological conceptual model building and testing: A review. J. Hydrol. 2018, 569, 310–329. [Google Scholar] [CrossRef]
- Healy, R.W. Estimating Groundwater Recharge, 1st ed.; Cambridge University Press: New York, NY, USA, 2010; p. 256. [Google Scholar]
- Gebreyohannes, T.; De Smedt, F.; Walraevens, K.; Gebresilassie, S.; Hussien, A.; Hagos, M.; Amare, K.; Deckers, J.; Gebrehiwot, K. Application of a spatially distributed water balance model for assessing surface water and groundwater resources in the Geba basin, Tigray, Ethiopia. J. Hydrol. 2013, 499, 110–123. [Google Scholar] [CrossRef]
- Porretta-Brandyk, L.; Chormański, J.; Ignar, S.; Okruszko, T.; Brandyk, A.; Szymczak, T.; Krężałek, K. Evaluation and verification of the WetSpa model based on selected rural catchments in Poland. J. Water Land Dev. 2010, 14, 115–133. [Google Scholar] [CrossRef]
- Zarei, M.; Ghazavi, R.; Vali, A.; Abdollahi, K. Estimating Groundwater Recharge, Evapotranspiration and Surface Runoff using Land-use data: A Case Study in Northeast Iran. Biol. Forum Int. J. 2016, 8, 196–202. [Google Scholar]
- Zhang, Y.; Liu, S.; Cheng, F.; Shen, Z. WetSpass-Based Study of the Effects of Urbanization on the Water Balance Components at Regional and Quadrat Scales in Beijing, China. Water 2017, 10, 5. [Google Scholar] [CrossRef] [Green Version]
- Salem, A.; Dezső, J.; El-Rawy, M. Assessment of Groundwater Recharge, Evaporation, and Runoff in the Drava Basin in Hungary with the WetSpass Model. Hydrology 2019, 6, 23. [Google Scholar] [CrossRef] [Green Version]
- Patrčević, V. Hidrološka Analiza Vertikalne Vodne Bilance Podzemnih Voda na Prostoru Riječnog Aluvija (Hydrological Analysis of the Vertical Water Balance of Groundwater in the River Alluvium—in Croatian). Ph.D. Thesis, University of Zagreb, Zagreb, Croatia, 1995. [Google Scholar]
- Larva, O. Ranjivost Vodonosnika na Priljevnom Području Varaždinskih Crpilišta (Aquifer Vulnerability at Catchment Area of Varaždin Pumping Sites—in Croatian). Ph.D. Thesis, University of Zagreb, Zagreb, Croatia, 2008. [Google Scholar]
- Salem, A.; Dezső, J.; El-Rawy, M.; Lóczy, D. Hydrological Modeling to Assess the Efficiency of Groundwater Replenishment through Natural Reservoirs in the Hungarian Drava River Floodplain. Water 2020, 12, 250. [Google Scholar] [CrossRef] [Green Version]
- Prelogović, E.; Velić, I. Kvartarna tektonska aktivnost u zapadnom dijelu Dravske potoline. Geol. Vjesnik 1988, 41, 237–253. [Google Scholar]
- Marković, T.; Karlović, I.; Perčec Tadić, M.; Larva, O. Application of Stable Water Isotopes to Improve Conceptual Model of Alluvial Aquifer in the Varaždin Area. Water 2020, 12, 379. [Google Scholar] [CrossRef] [Green Version]
- Nimac, I.; Perčec Tadić, M. New 1981–2010 Climatological Normals for Croatia and Comparison to Previous 1961–1990 and 1971–2000 Normals. In Proceedings of the Geostatistics and Machine Learning Conference, Belgrade, Serbia, 23–24 June 2016; University of Belgrade—Faculty of Civil Engineering: Belgrade, Serbia, 2016; pp. 79–85. [Google Scholar]
- Zaninović, K.; Gajić-Čapka, M.; Perčec Tadić, M. Klimatski atlas Hrvatske. Climate atlas of Croatia: 1961–1990, 1971–2000; Državni Hidrometeorološki Zavod: Zagreb, Croatia, 2008; p. 200. [Google Scholar]
- Šimunić, A.; Pikija, M.; Hećimović, I. Osnovna Geološka Karta SFRJ 1:100.000. List Varaždin L33–69 (Basic Geological Map of SFRY, Scale 1:100.000, Sheet Varaždin L33–69—in Croatian); Federal Geological Survey: Belgrade, Serbia, 1982. [Google Scholar]
- Mioč, P.; Marković, S. Osnovna Geološka Karta SFRJ 1:100.000. List Čakovec L33–57 (Basic Geological Map of SFRY, Scale 1:100.000, Sheet Čakovec L33–57—in Croatian); Geological Survey, Zagreb and Geological, Geotechnical and Geophysical Survey: Ljubljana, Slovenia, 1998. [Google Scholar]
- Batelaan, O.; Smedt, F.D. WetSpass: A flexible, GIS based, distributed recharge methodology for regional groundwater modelling. In Impact of Human Activity on Groundwater Dynamics; Gehrels, H., Peters, J., Leibundgut, C., Eds.; International Association of Hydrological Sciences: Wallingford, UK, 2001; pp. 11–17. [Google Scholar]
- Batelaan, O.; De Smedt, F. GIS-based recharge estimation by coupling surface-subsurface water balances. J. Hydrol. 2007, 337, 337–355. [Google Scholar] [CrossRef]
- Rwanga, S. A Review on Groundwater Recharge Estimation Using Wetspass Model. In Proceedings of the International Conference on Civil and Environmental Engineering (CEE’2013), Johannesburg, South Africa, 27–28 November 2013. [Google Scholar]
- Abdollahi, K.; Bashir, I.; Verbeiren, B.; Harouna, M.R.; Griensven, A.V.; Husmans, M.; Batelaan, O. A distributed monthly water balance model: Formulation and application on Black Volta Basin. Environ. Earth Sci. 2017, 76, 198. [Google Scholar] [CrossRef]
- Allen, R.G.; Pereira, L.S.; Raes, D.; Smith, M. Crop Evapotranspiration—Guidelines for Computing Crop Water Requirements; FAO Irrigation and Drainage Paper 56; Food and Agriculture Organization: Rome, Italy, 1998. [Google Scholar]
- Djaman, K.; O’Neill, M.; Diop, L.; Bodian, A.; Allen, S.; Koudahe, K.; Lombard, K. Evaluation of the Penman-Monteith and other 34 reference evapotranspiration equations under limited data in a semiarid dry climate. Theor. Appl. Climatol. 2019, 137, 729–743. [Google Scholar] [CrossRef]
- Bogawski, P.; Bednorz, E. Comparison and Validation of Selected Evapotranspiration Models for Conditions in Poland (Central Europe). Water Resour. Manag. 2014, 28, 5021–5038. [Google Scholar] [CrossRef] [Green Version]
- Čadro, S.; Uzunović, M.; Žurovec, J.; Žurovec, O. Validation and calibration of various reference evapotranspiration alternative methods under the climate conditions of Bosnia and Herzegovina. Int. Soil Water Conserv. Res. 2017, 5, 309–324. [Google Scholar] [CrossRef]
- Song, X.; Lu, F.; Xiao, W.; Zhu, K.; Zhuou, Y.; Xie, Z. Performance of 12 reference evapotranspiration estimation methods compared with the Penman–Monteith method and the potential influences in northeast China. Meteorol. Appl. 2019, 26, 83–96. [Google Scholar] [CrossRef] [Green Version]
- FAO. ETo Calculator; Land and Water Digital Media Series No. 36; FAO: Rome, Italy, 2012. [Google Scholar]
- Nistor, M.M.; Porumb-Ghiurco, G.C. How to compute the land cover evapotranspiration at regional scale? A spatial approach of Emilia-Romagna region. Georeview 2015, 25, 38–54. [Google Scholar]
- Nistor, M.M.; Ronchetti, F.; Corsini, A.; Cheval, S.; Dumitrescu, A.; Rai, P.K.; Petrea, D.; Dezsi, Ş. Crop evapotranspiration variation under climate change in South East Europe during 1991–2050. Carpath. J. Earth Environ. Sci. 2017, 12, 571–582. [Google Scholar]
- Nistor, M.M.; Titus, M.; Benzaghta, M.A.; Dezsi, Ş.; Kizza, R. Land cover and temperature implications for the seasonal evapotranspiration in Europe. Geogr. Technol. 2018, 13, 85–108. [Google Scholar] [CrossRef] [Green Version]
- Batelaan, O.; De Smedt, F.; Triest, L. Regional groundwater discharge: Phreatophyte mapping, groundwater modelling and impact analysis of land-use change. J. Hydrol. 2003, 275, 86–108. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Karlović, I.; Marković, T.; Vujnović, T.; Larva, O. Development of a Hydrogeological Conceptual Model of the Varaždin Alluvial Aquifer. Hydrology 2021, 8, 19. https://doi.org/10.3390/hydrology8010019
Karlović I, Marković T, Vujnović T, Larva O. Development of a Hydrogeological Conceptual Model of the Varaždin Alluvial Aquifer. Hydrology. 2021; 8(1):19. https://doi.org/10.3390/hydrology8010019
Chicago/Turabian StyleKarlović, Igor, Tamara Marković, Tatjana Vujnović, and Ozren Larva. 2021. "Development of a Hydrogeological Conceptual Model of the Varaždin Alluvial Aquifer" Hydrology 8, no. 1: 19. https://doi.org/10.3390/hydrology8010019
APA StyleKarlović, I., Marković, T., Vujnović, T., & Larva, O. (2021). Development of a Hydrogeological Conceptual Model of the Varaždin Alluvial Aquifer. Hydrology, 8(1), 19. https://doi.org/10.3390/hydrology8010019







