Combined Modelling of Coastal Barrier Breaching and Induced Flood Propagation Using XBeach
Abstract
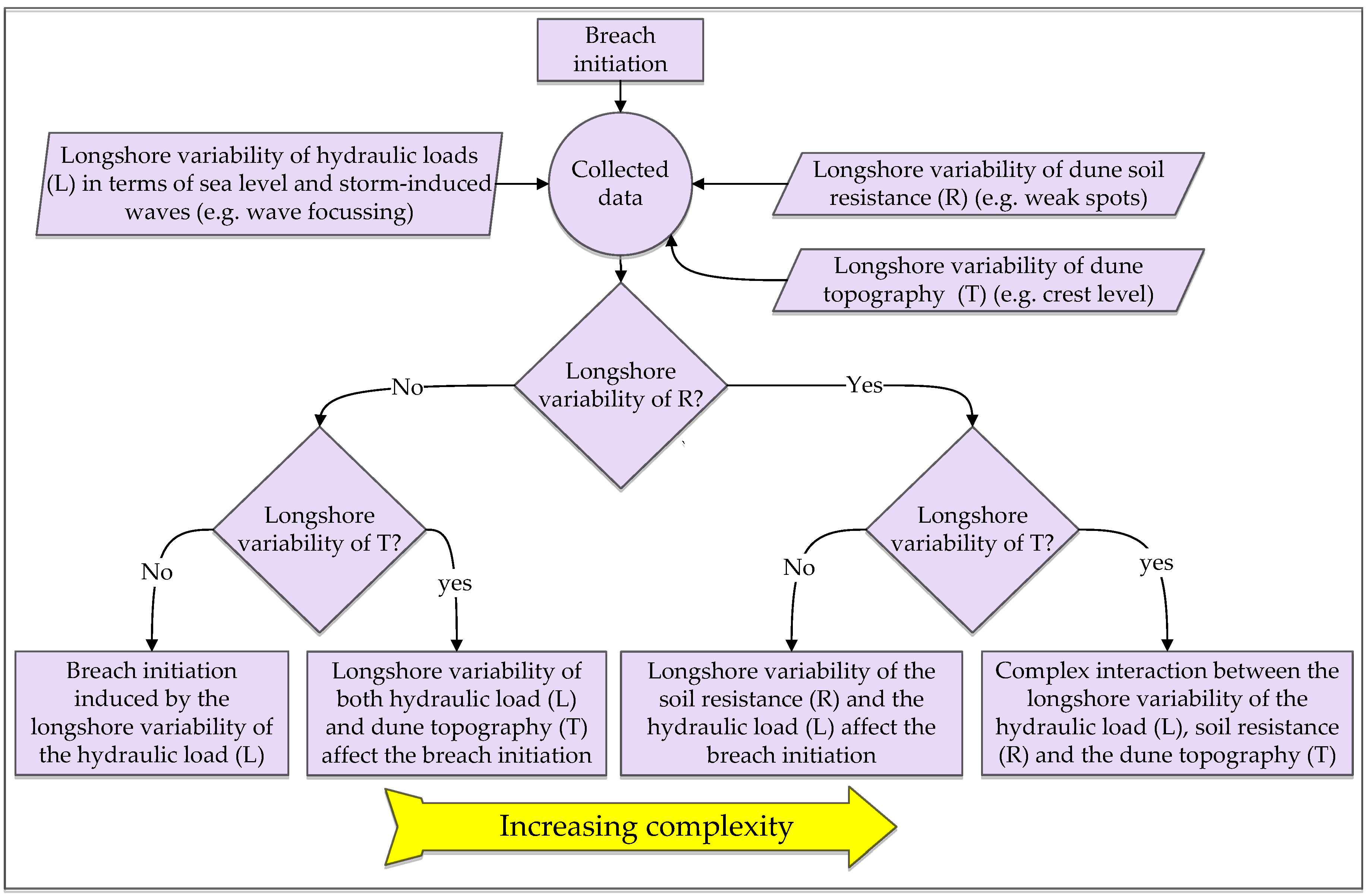
:1. Introduction
- (i)
- empirical model (e.g., EurOtop) or numerical model (e.g., XBeach) to predict overtopping rates along representative discrete transects for overland flow model input, and
- (ii)
- Computational Fluid Dynamics (CFD) solver used as inundation models to simulate the overland and surface runoff using the results from the overtopping models as input conditions (Wadey et al. 2012 [29]; Gallien, 2016 [27]; Worni et al. 2014 [30]). Such CFD models (e.g., River-2D, ISIS-2D, BASEMENT-2D, MIKE FLOOD, BreZo, DIVAST, TELEMAC-2D, TUFLOW or SOBEK) are generally based on the solution of the nonlinear shallow water equations (NLSWEs).
- (i)
- breaching model (e.g., XBeach) to calculate the flow rates through a breach-induced inlet and
- (ii)
- CFD model as described above to simulate the inundation resulting from the breach.
- (i)
- to extend the scope of using XBeach by examining its applicability for modelling coastal barrier breaching and inundation modelling in combination, instead of the current approach, which addresses the modelling of each of these two processes separately,
- (ii)
- at a comparative analysis of the results from the latter approach (decoupled approach) with the results of the proposed combined modelling approach using XBeach,
- (iii)
- to introduce a computing technique for calculating the flow through breaches reproduced by XBeach (inland hydrograph), and
- (iv)
- to improve the understanding of the processes and interactions associated with both breaching and subsequent flood propagation.
- (i)
- with the results from common 1D and 2D CFD models for flood propagation (e.g., Hydrologic Engineering Center’s River Analysis System (HEC-RAS)(1D) and River-2D)), using the calculated inland hydrograph through a breach-induced inlet(s) as the upstream inflow condition for these models and
- (ii)
- with observations for breaching and subsequent inundation from a real case study.
2. Methods
2.1. HEC-RAS
2.2. River-2D
2.3. XBeach
3. Test Cases
3.1. 1D Synthetic Cross-Shore Profile
- (i)
- Load case 1 (LC1): represents, in addition to the wave action described by a JONSWAP spectrum, a sudden sea level rise from 0.00 m to +5.00 m where the latter level persists over the entire storm duration (rectangular shape) and
- (ii)
- Load case 2 (LC2): represents, in addition to the wave action described by a JONSWAP spectrum, a linear sea level rise from 0.00 m to 6.00 m within half of the storm duration followed by a linear decrease at the same rate to level 0.00 m within the other half (triangular shape).
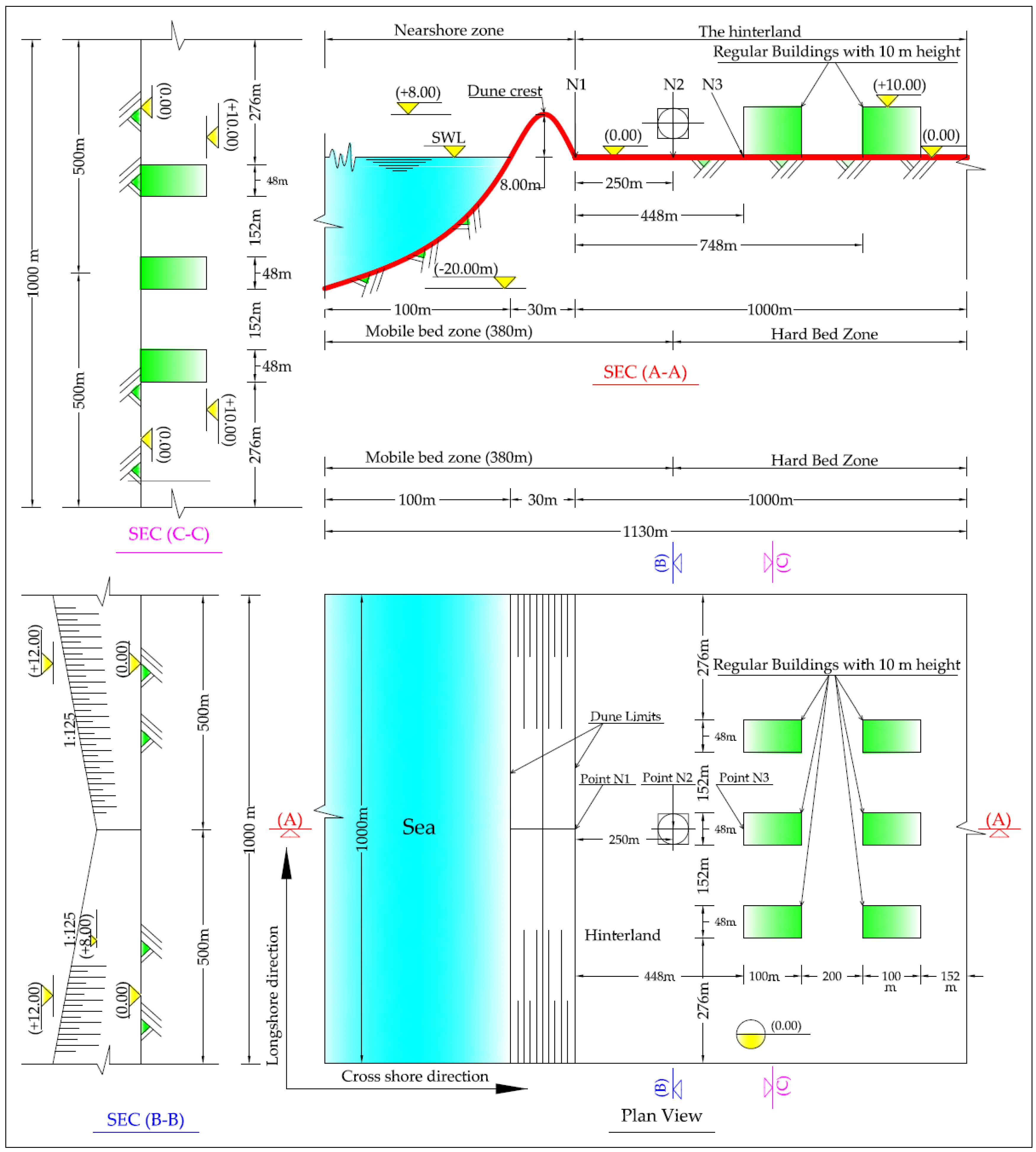
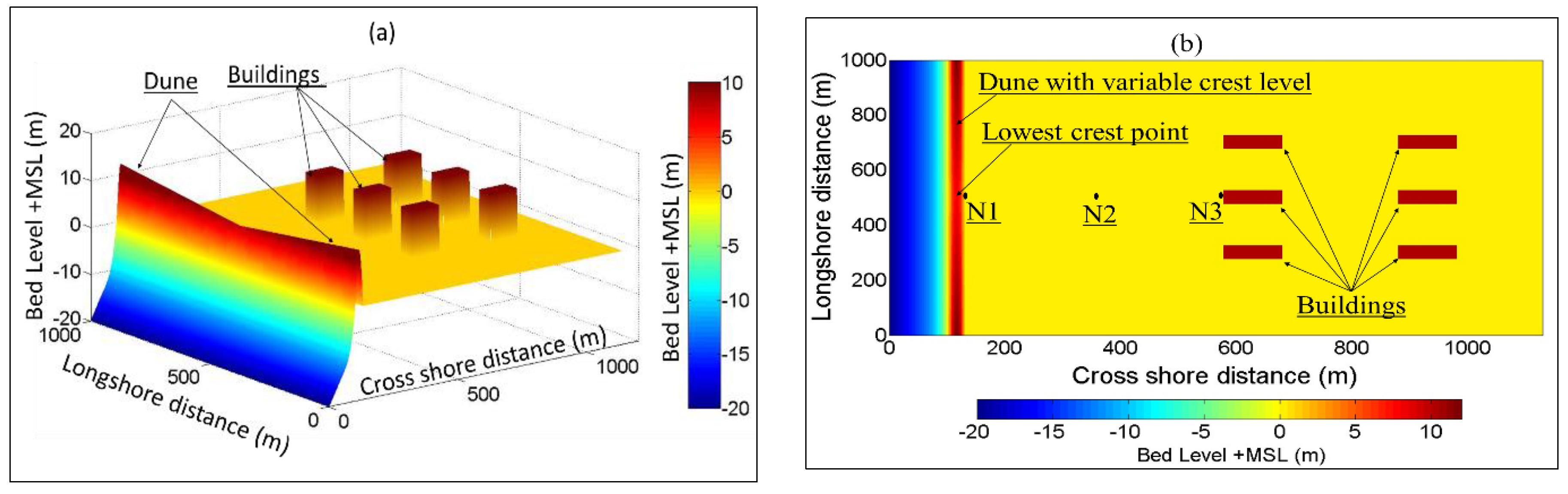
3.2. 2D Synthetic Coastal Zone
3.3. The Case of Het Zwin
- (i)
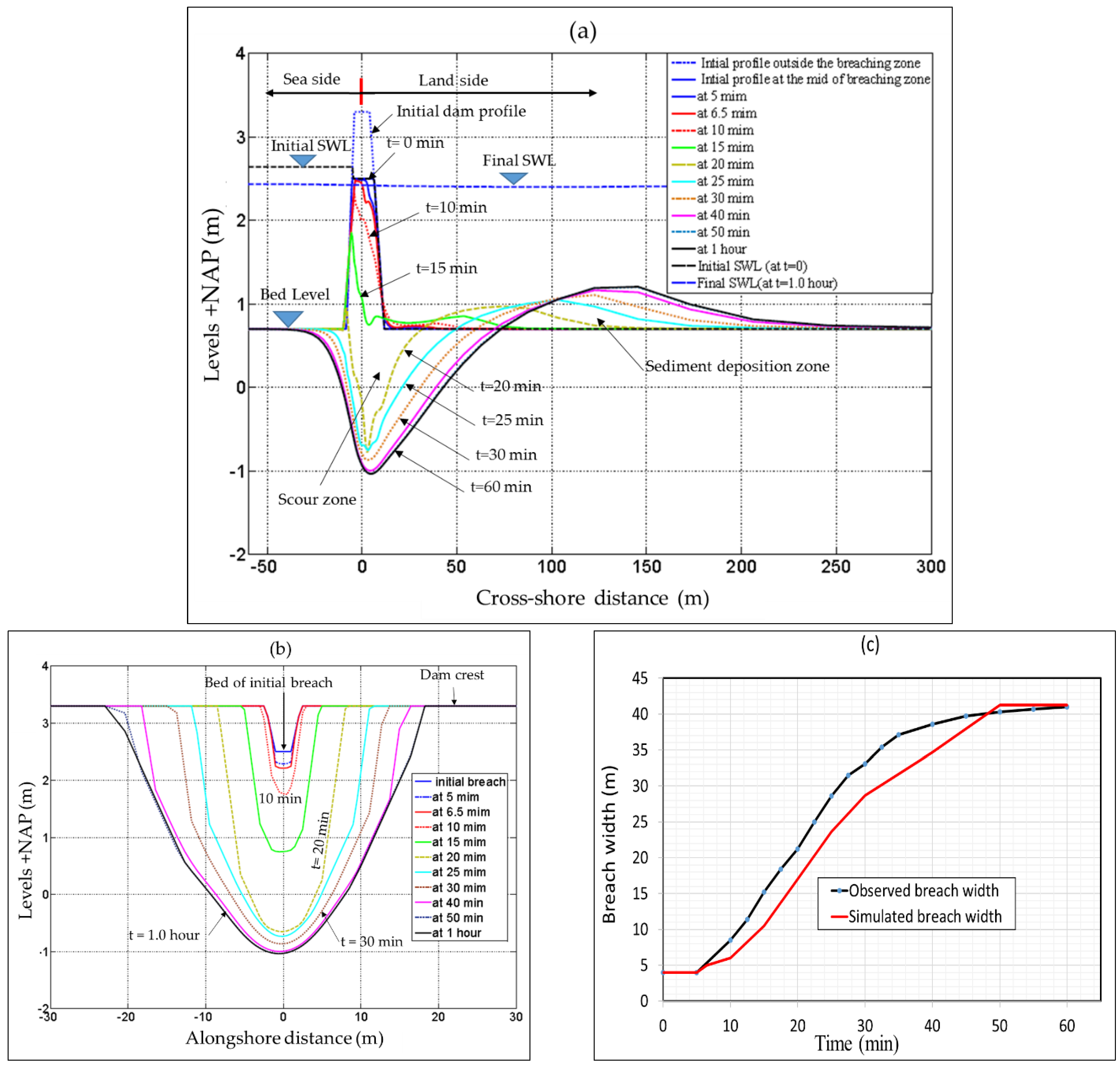
- The comparison between the observed and calculated breach widths to assess the capability of XBeach as a breaching model.
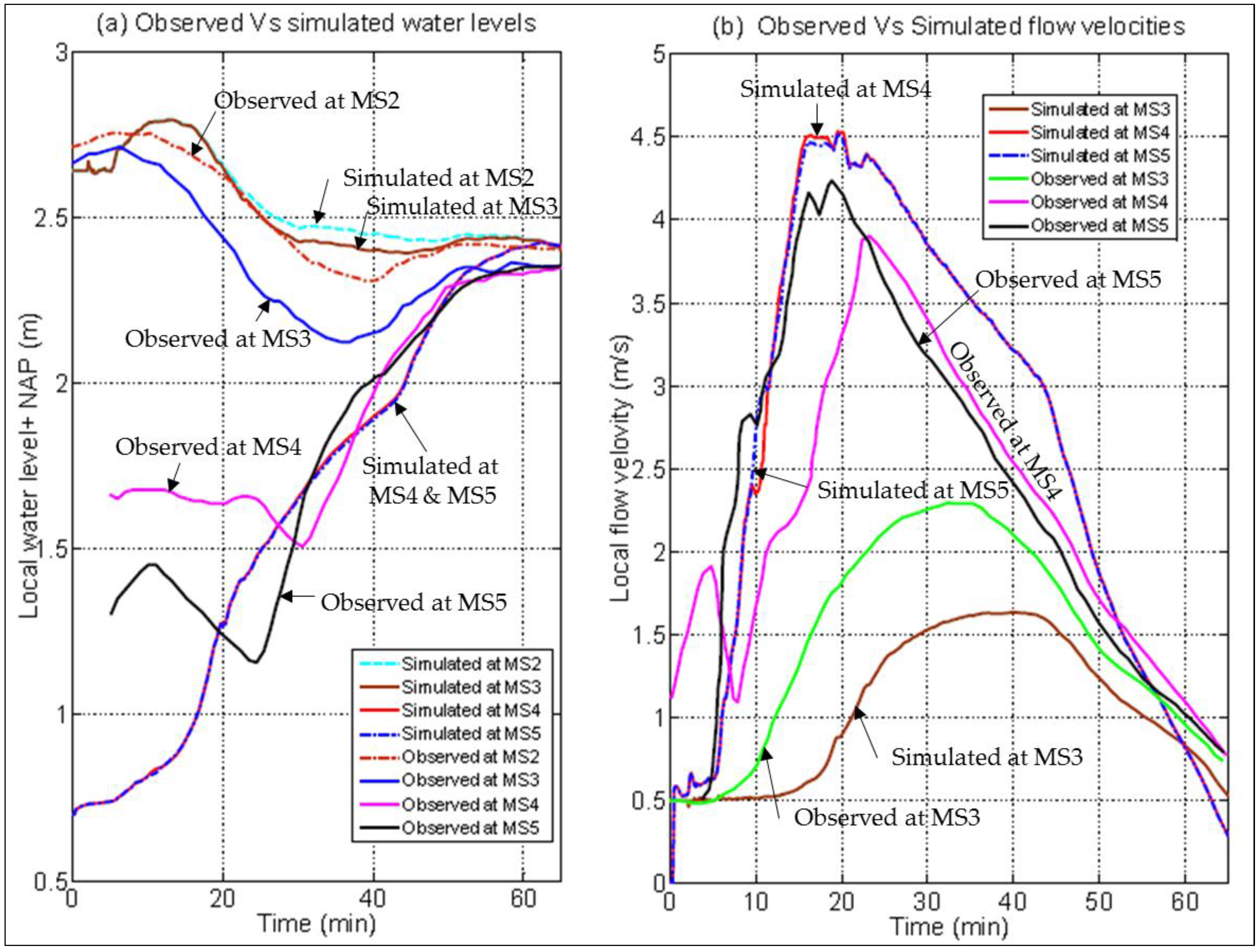
- (ii)
- The comparison between the observed and modelled water depths and flow velocities at the measuring stations MS4 and MS5 (located behind the dam) to show that XBeach correctly calculates the water depths and flow velocities in the hinterland.
- (iii)
- The comparison between the volume of the tidal prism and the total inflow discharge to prove that XBeach calculates the flood extent correctly.
4. Results
4.1. 1D Synthetic Cross-Shore Profile
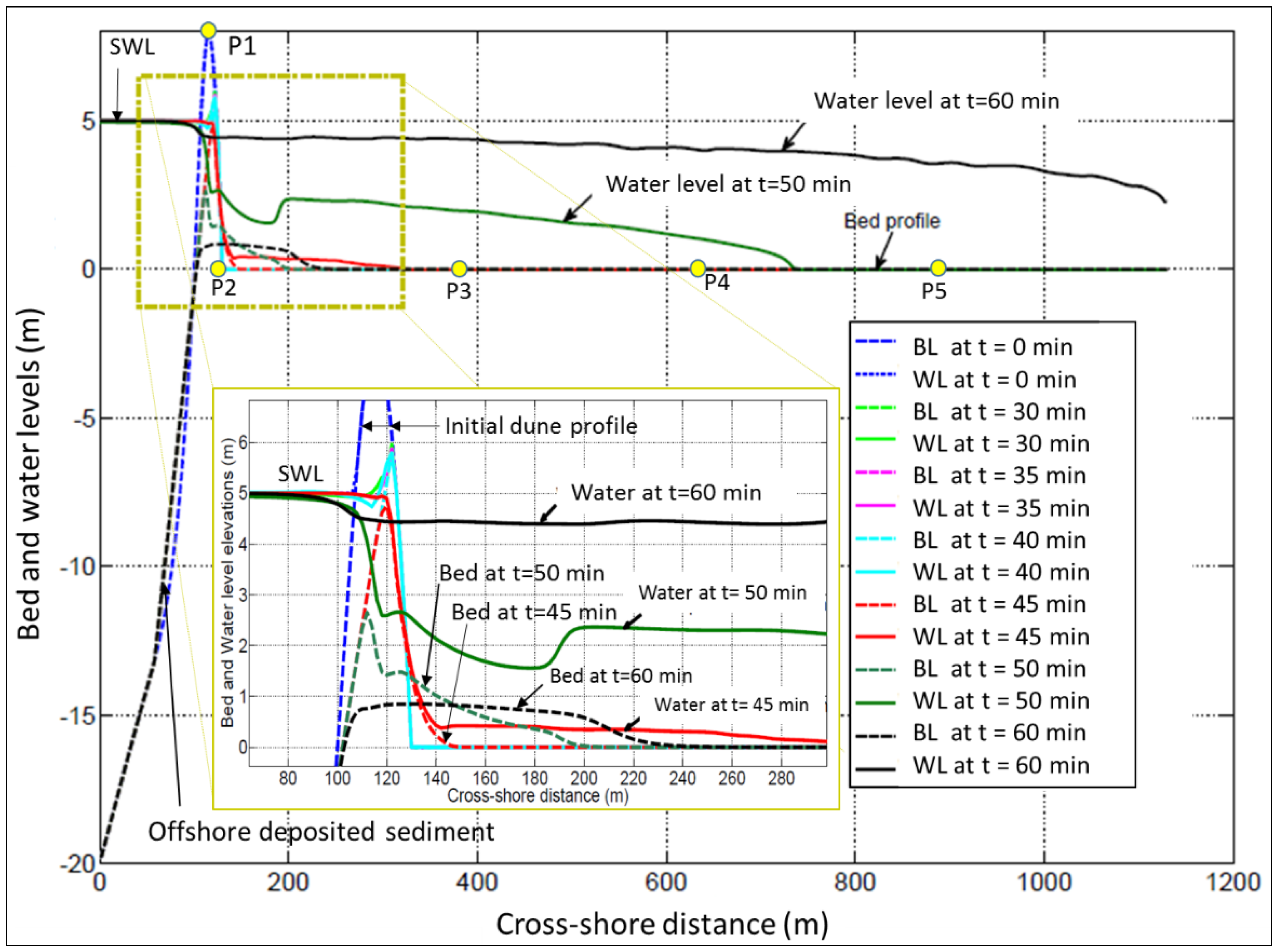
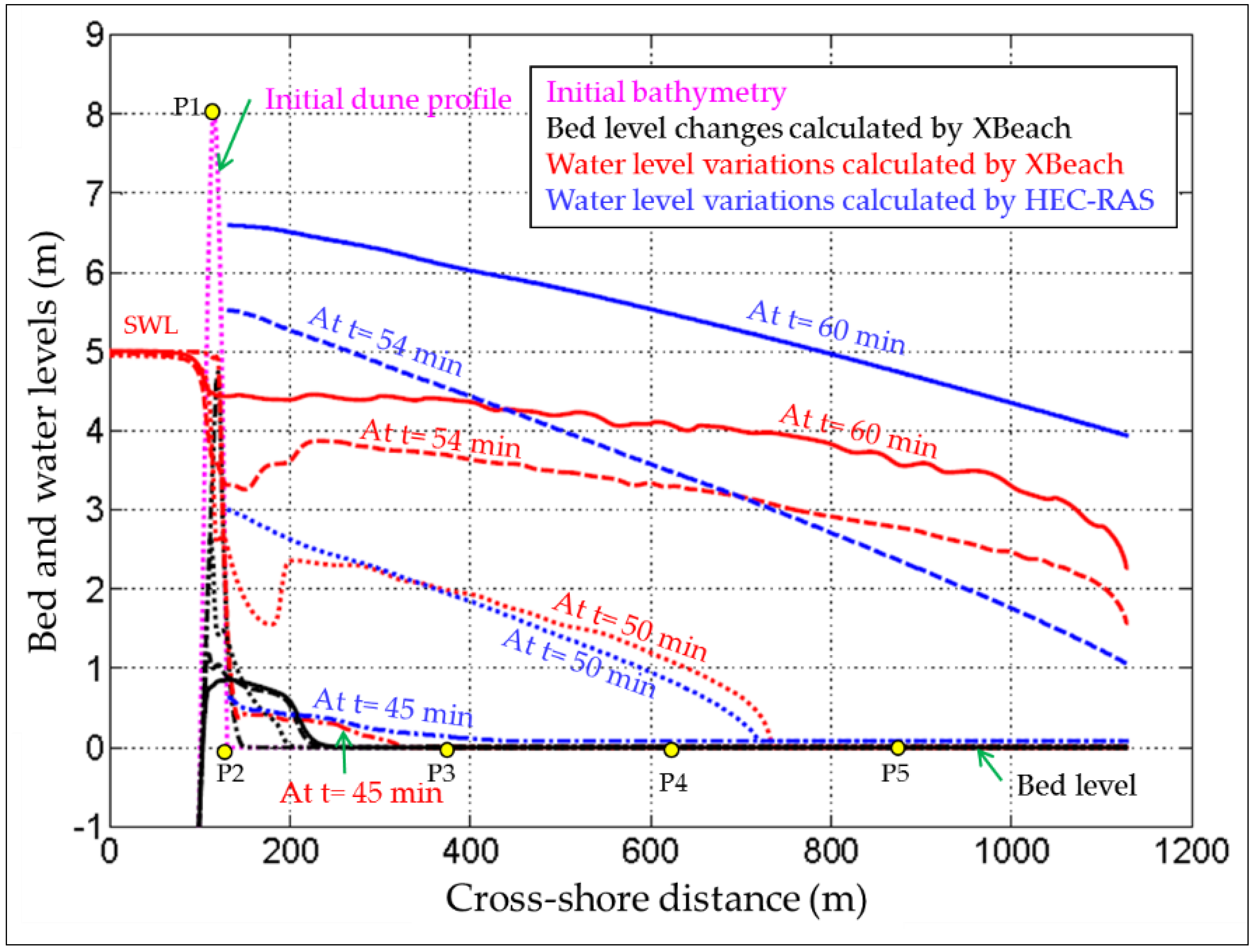
4.1.1. Bed Profile and Water Level Evolution under Load Case LC1 by XBeach
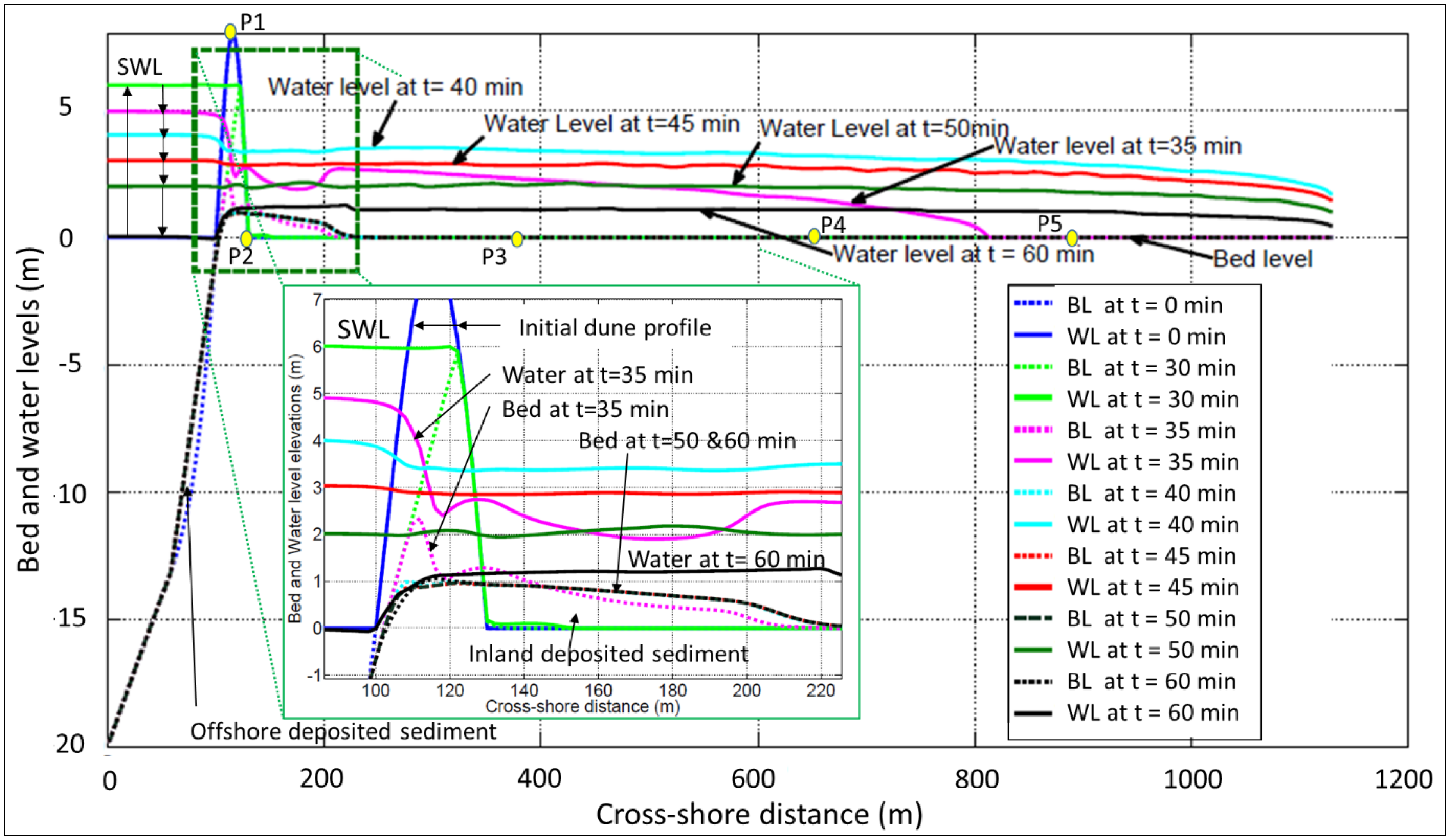
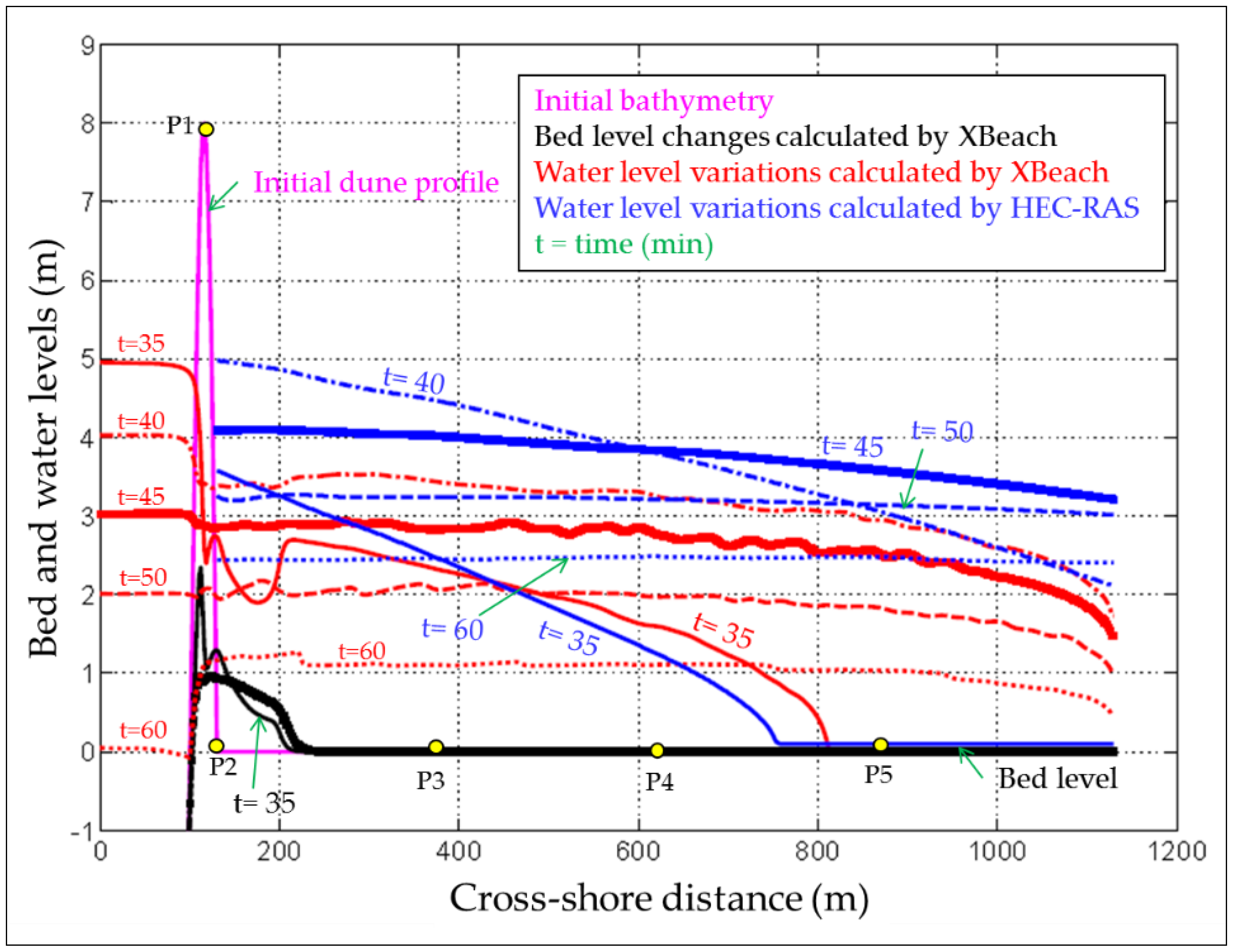
4.1.2. Bed Profile and Water Level Evolution under Load Case LC2 by XBeach
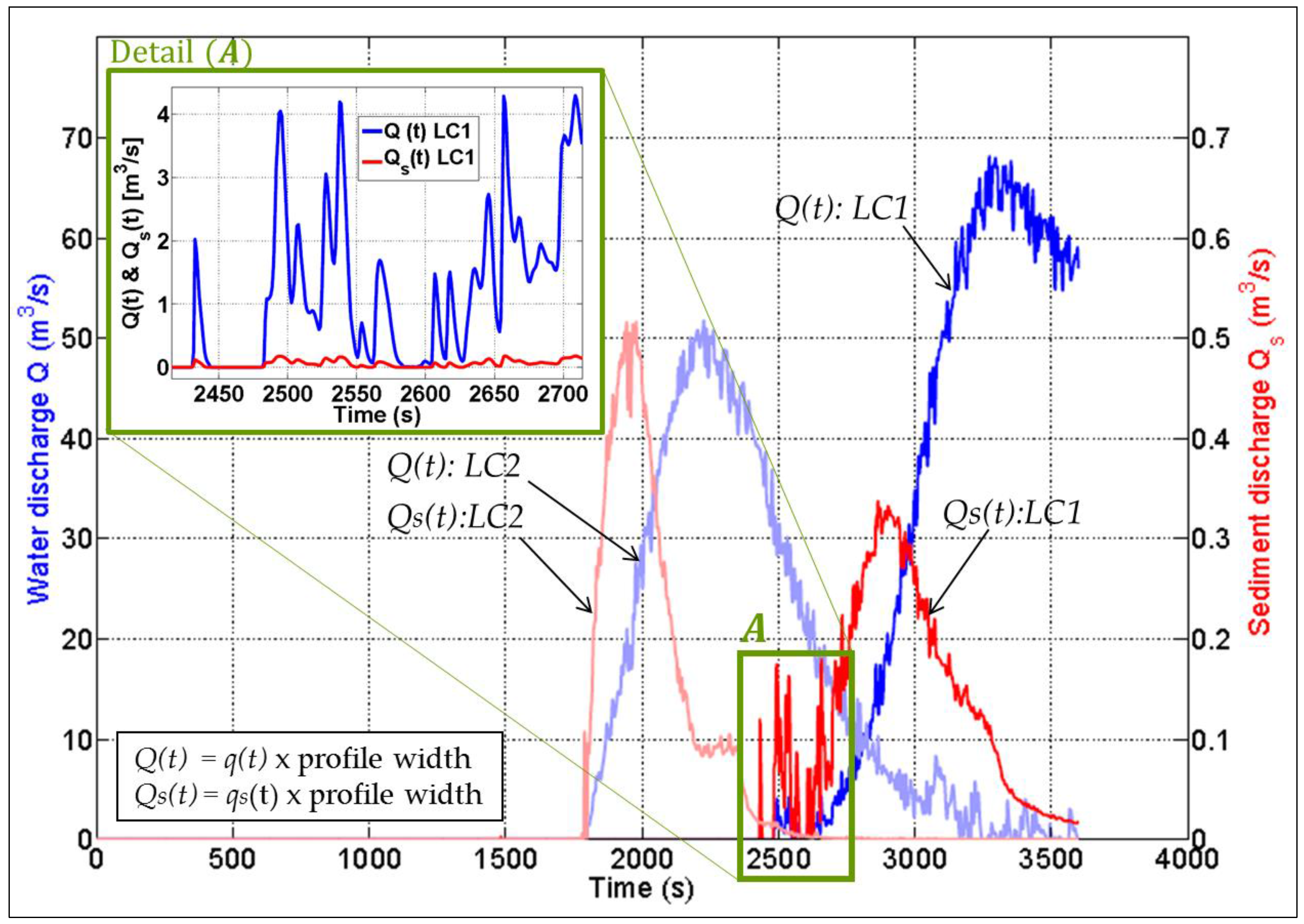
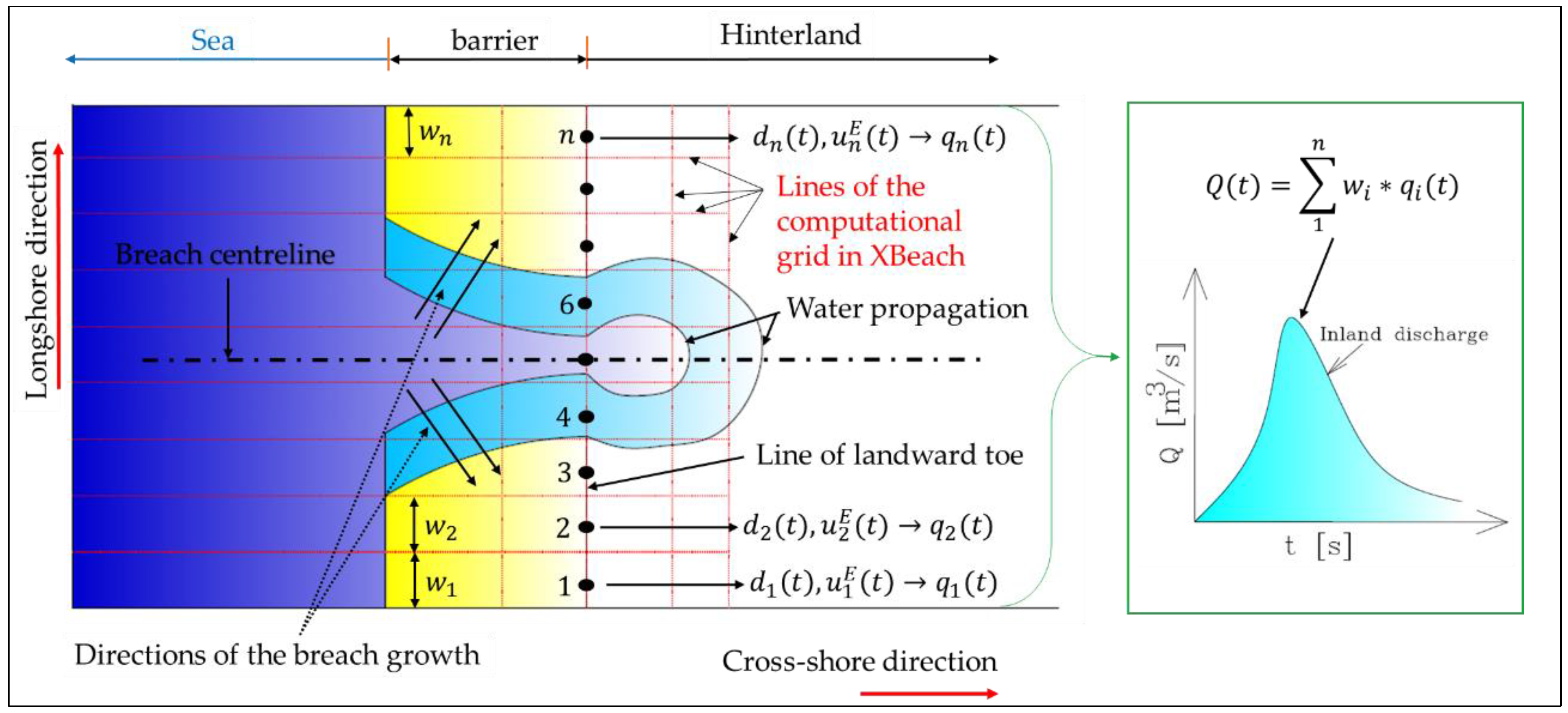
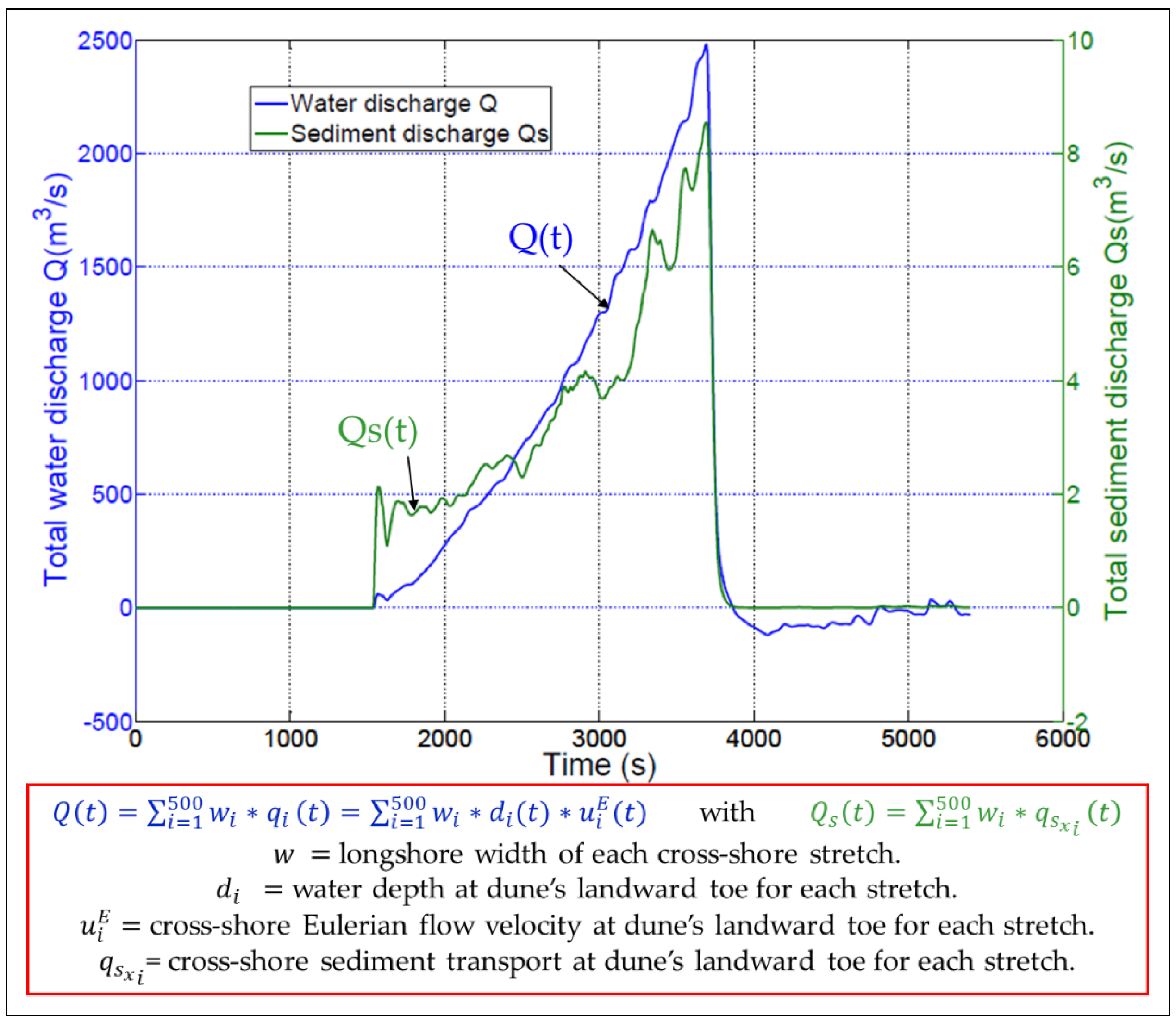
4.1.3. Water and Sediment Inflow Discharges to the Hinterland
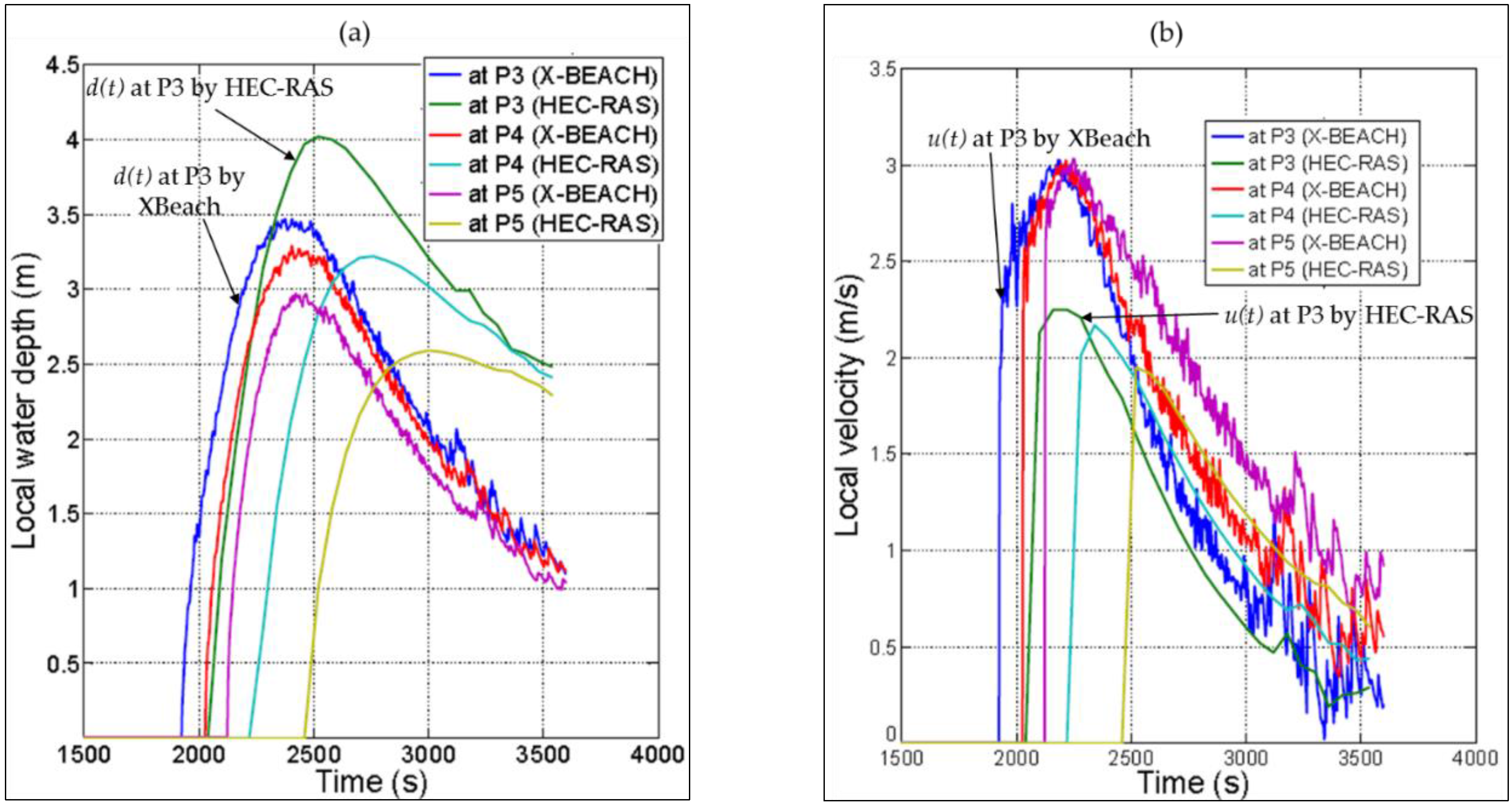
4.1.4. Comparison of Inundation Depths and Velocities Obtained from XBeach and HEC-RAS
4.2. 2D Synthetic Coastal Zone
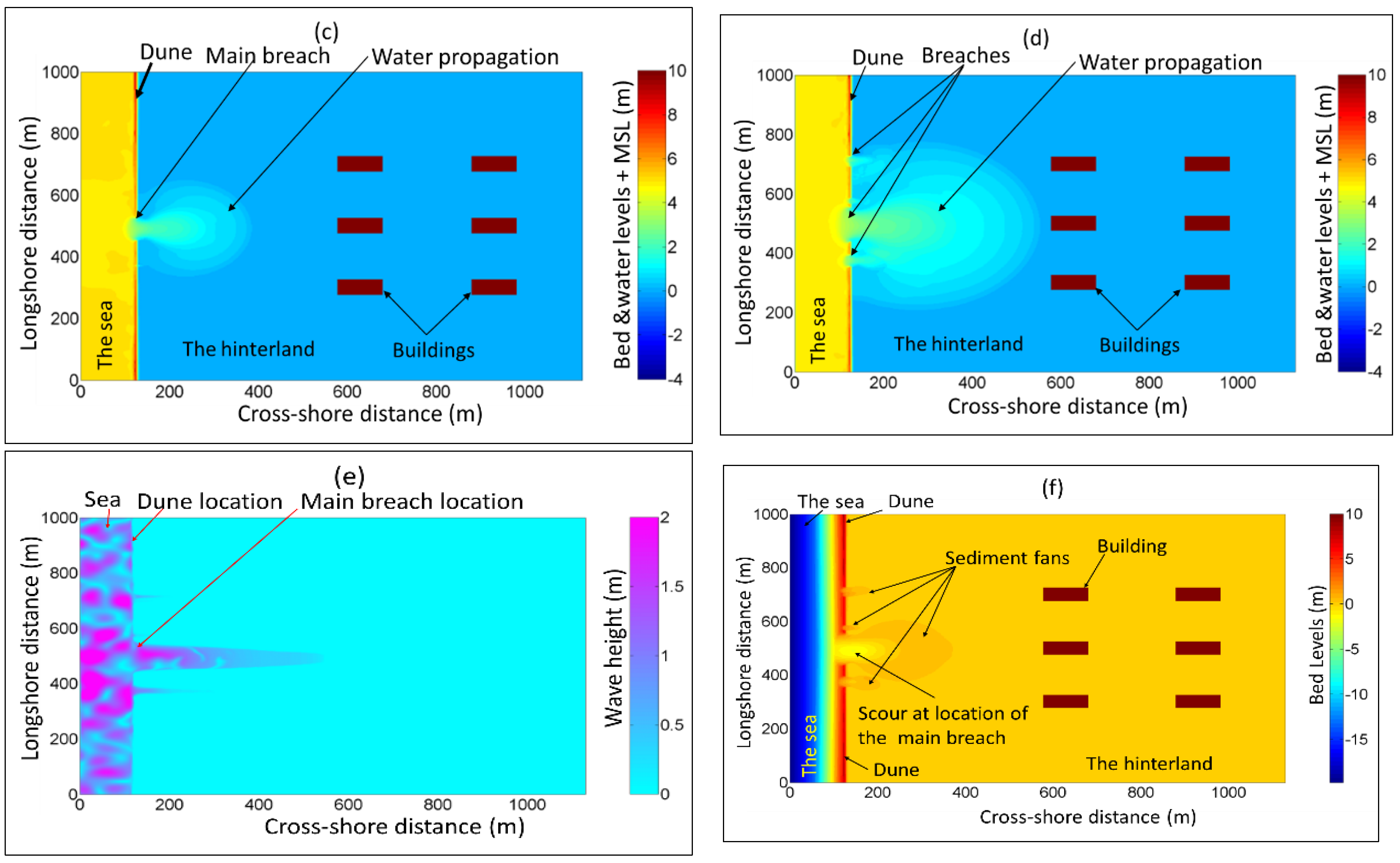
4.2.1. Breach and Flood Propagation Results from XBeach for Load Case LC1
4.2.2. Water and Sediment Inflow Discharges to the Hinterland
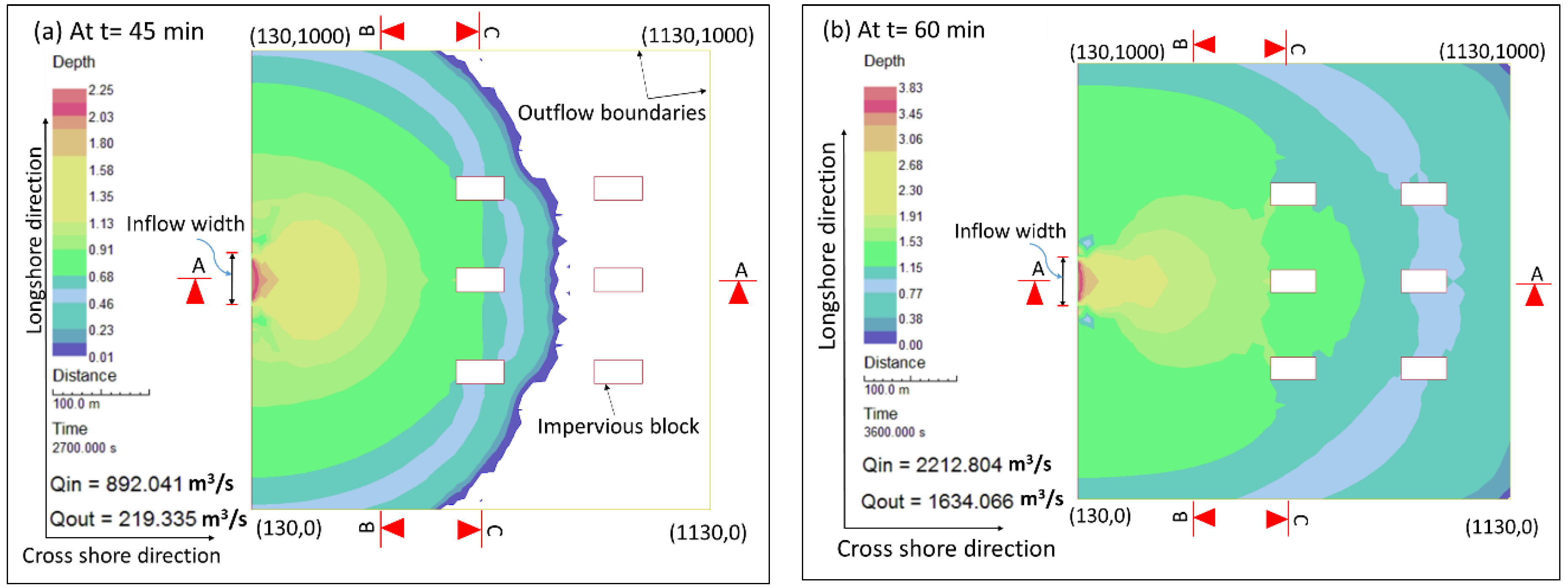
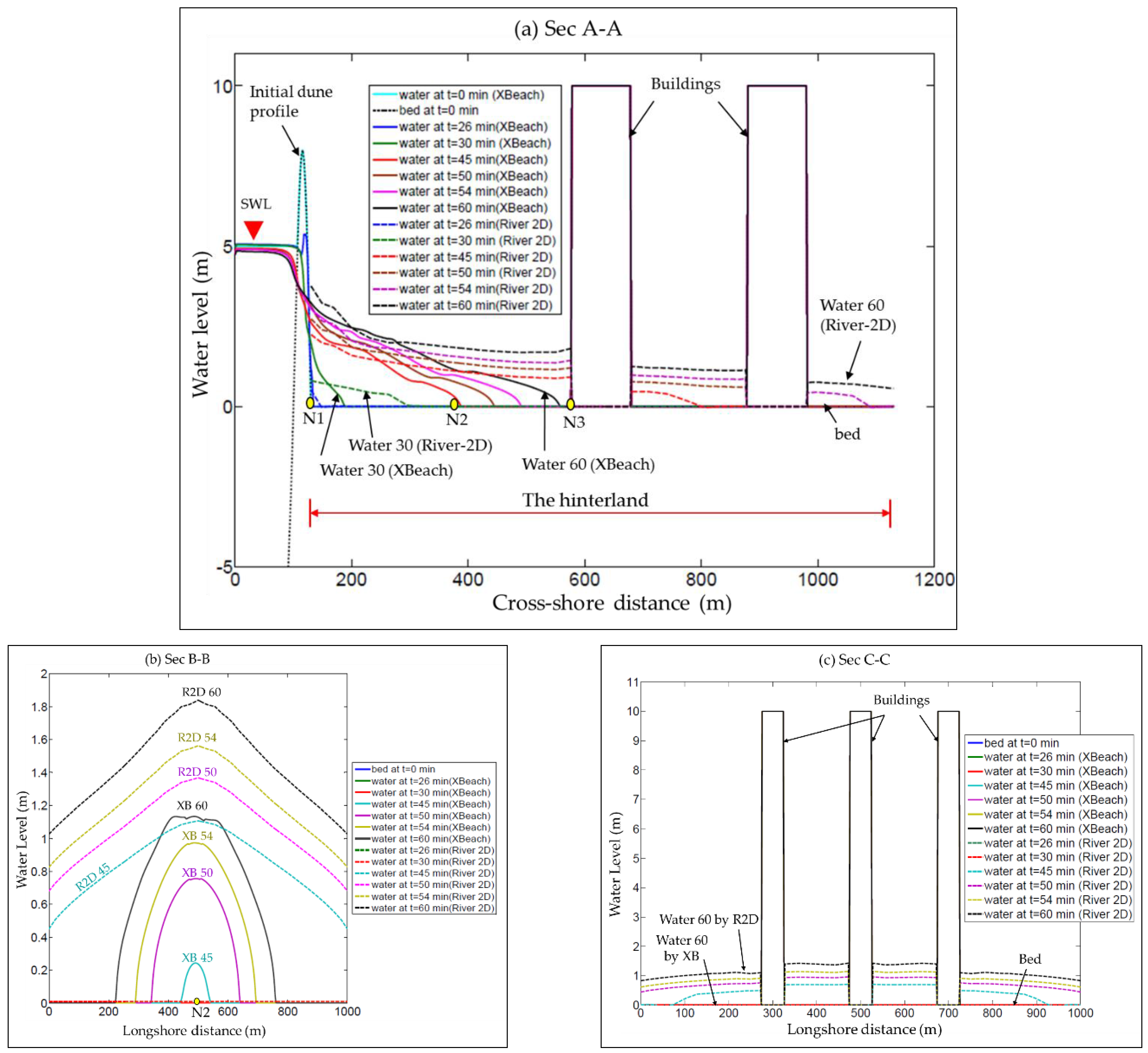
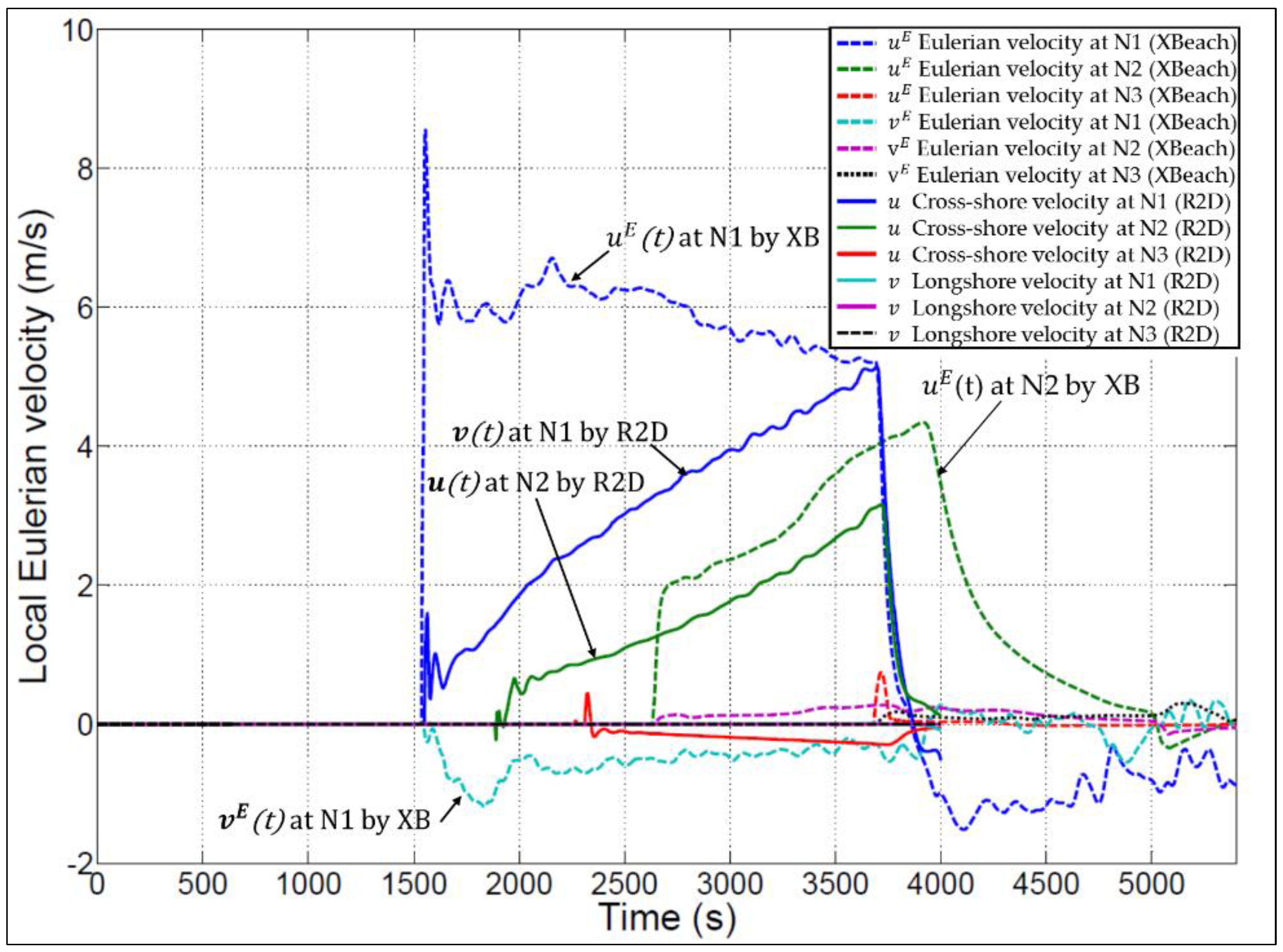
4.2.3. Comparative Analysis of Inundation Modelling Results from XBeach and River-2D
- (i)
- Assigning the inflow to River-2D through a fixed width (Figure 15) while omitting the evolution of the breach size dimensions as generated by XBeach. Such a fixed inflow width results in wider estimates of the flood extent from the beginning to the end of the simulation.
- (ii)
- The omission of the momentum conservation principle when passing the inflow hydrograph to River-2D, resulting in subcritical inflow behind the model inlet, which is in contrast to reality.
4.3. Case Study of Het Zwin Dam Breach
4.3.1. Reproduction of the Zwin Dam Breach by XBeach
4.3.2. Observed vs Modelled Water Depths and Flow Velocities at Het Zwin Breach
4.3.3. Water Discharge through the Dam Breach
5. Discussion of the Results
- (i)
- the results from the current modelling approach, applying separately an overtopping/breaching model to calculate the inflow hydrograph Q(t) and a common inundation NLSWEs-based model, such as HEC-RAS and River-2D, using Q(t) as an inflow boundary condition at the barrier to simulate the flood propagation in the hinterland, and
- (ii)
- the results of XBeach applied to simulate, together, both breaching and subsequent inundation, have clearly demonstrated the advantages of the latter modelling approach. In fact, among the available open-source hydro-morphodynamic models, XBeach is the most appropriate tool for the latter approach.
- (i)
- XBeach can generate real sea conditions through generating alongshore varying time series of wave energy (alongshore varying hydraulic loads), where the effect of waves is introduced as a source term in the NLSWEs;
- (ii)
- the CFD module can simulate wave overtopping and overflow processes in combination, the flow through the developing breach and the subsequent coastal inundation processes in a single simulation;
- (iii)
- the morphodynamic module can properly calculate sediment transport and the resulting morphological changes and also includes a soil avalanching algorithm making Xbeach capable of to properly simulating the evolution of barrier breaching.
- (i)
- The use of hydrograph Q(t) as inflow conditions to the common inundation models is in line with the mass conservation principle, but the flow velocity v(t) which cannot be accounted for in the inflow conditions is also crucial as it provides, together with Q(t) the momentum;
- (ii)
- (iii)
- No account of the evolution of the inflow width (see Figure 15) in the common inundation models.
6. Conclusions and Remarks
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Smith, K. Environmental Hazards: Assessing Risk and Reducing Disaster, 6th ed.; Routledge: London, UK, 2013. [Google Scholar]
- Xu, L.; He, Y.; Huang, W. A multi-dimensional integrated approach to assess flood risks on a coastal city, induced by sea-level rise and storm tides. Environ. Res. Lett. 2016, 11, 014001. [Google Scholar]
- Kraus, N.C.; Wamsley, T.V. Coastal Barrier Breaching. Part 1. Overview of Breaching Processes. DTIC Document. 2003. Available online: http://oai.dtic.mil/oai/oai?verb=getRecord&metadataPrefix=html&identifier=ADA588872 (accessed on 5 December 2015).
- Roger, S.; Dewals, B.; Schuettrumpf, H.; Erpicum, S.; Archambeau, P.; Pirotton, M.; Schwanenberg, D.; Dewals, B. Hybrid modelling of dike-break induced flows. In River Flow 2010, Proceedings of the International Conference on Fluvial Hydraulics, Braunschweig, Germany, 8–10 September 2010; Dittrich, A., Koll, K., Aberle, J., Geisenhainer, P., Eds.; Karlsruhe: Bundesanstalt für Wasserbau, 2010; pp. 523–531. [Google Scholar]
- Bisschop, F.; Visser, P.; van Rhee, C.; Verhagen, H.J. Erosion due to high flow velocities: A description of relevant processes. In Proceedings of 32nd Conference on Coastal Engineering, Shanghai, China, 30 June–5 July 2010; Lynett, P., Ed.; International Conference on Coastal Engineering (ICCE), 2010; Volume 1, p. 24. [Google Scholar]
- Wu, W.; Altinakar, M.S.; Song, C.R.; Al-Riffai, M.; Bergman, N.; Bradford, S.F.; Cao, Z.; Chen, Q.J.; Constantinescu, S.G.; Duan, J.G. Earthen embankment breaching. J. Hydraul. Eng. 2011, 137, 1549–1564. [Google Scholar]
- Sills, G.; Vroman, N.; Wahl, R.; Schwanz, N. Overview of New Orleans levee failures: Lessons learned and their impact on national levee design and assessment. J. Geotech. Geoenviron. Eng. 2008, 134, 556–565. [Google Scholar] [CrossRef]
- Yang, J.; Graf, T.; Herold, M.; Ptak, T. Modelling the effects of tides and storm surges on coastal aquifers using a coupled surface-subsurface approach. J. Contam. Hydrol. 2013, 149, 61–75. [Google Scholar] [CrossRef] [PubMed]
- Yang, J.; Graf, T.; Ptak, I.T. Sea level rise and storm surge effects in a coastal heterogeneous aquifer: A 2d modelling study in northern Germany. Grundwasser Z. Fachsekt. Hydrogeol. 2015, 20, 39–51. [Google Scholar] [CrossRef]
- Christensen, B.B.; Drønen, N.; Klagenberg, P.; Jensen, J.; Deigaard, R.; Sørensen, P. Multiscale modelling of coastal flooding. In Proceedings of 7th International Conference for Coastal Dynamics, Arcachon Convention Centre, Arcachon, France, 24–28 June 2013; Jörg-Olaf, W., Ed.; Springer, 2013; pp. 339–350. [Google Scholar]
- He, Z.; Hu, P.; Zhao, L.; Wu, G.; Pähtz, T. Modeling of breaching due to overtopping flow and waves based on coupled flow and sediment transport. Water 2015, 7, 4283–4304. [Google Scholar] [CrossRef]
- Hinkel, J.; Lincke, D.; Vafeidis, A.T.; Perrette, M.; Nicholls, R.J.; Tol, R.S.; Marzeion, B.; Fettweis, X.; Ionescu, C.; Levermann, A. Coastal flood damage and adaptation costs under 21st century sea-level rise. Proc. Natl. Acad. Sci. USA 2014, 111, 3292–3297. [Google Scholar] [CrossRef] [PubMed]
- Cheung, K.; Phadke, A.; Wei, Y.; Rojas, R.; Douyere, Y.-M.; Martino, C.; Houston, S.; Liu, P.-F.; Lynett, P.; Dodd, N. Modelling of storm-induced coastal flooding for emergency management. Ocean Eng. 2003, 30, 1353–1386. [Google Scholar] [CrossRef]
- Bates, P.D.; Dawson, R.J.; Hall, J.W.; Horritt, M.S.; Nicholls, R.J.; Wicks, J.; Hassan, M.A.A.M. Simplified two-dimensional numerical modelling of coastal flooding and example applications. Coast. Eng. 2005, 52, 793–810. [Google Scholar] [CrossRef]
- Chini, N.; Stansby, P.; Rogers, B.; Vacondio, R.; Mignosa, P. State-of-the-art coastal inundation models applied to the 2007 Norfolk storm. In Comprehensive Flood Risk Management: Research for Policy and Practice. Proceedings of the 2nd European Conference on Flood Risk Management FLOODrisk2012, Rotterdam, The Netherlands, 20–22 November 2012; Klijn, F., Schweckendiek, T., Eds.; CRC Press/Balkema: London, UK, 2012; p. 8. [Google Scholar]
- Smith, R.A.; Bates, P.D.; Hayes, C. Evaluation of a coastal flood inundation model using hard and soft data. Environ. Model. Softw. 2012, 30, 35–46. [Google Scholar] [CrossRef]
- McCabe, M.; Stansby, P.; Apsley, D. Random wave runup and overtopping a steep sea wall: Shallow-water and Boussinesq modelling with generalised breaking and wall impact algorithms validated against laboratory and field measurements. Coast. Eng. 2013, 74, 33–49. [Google Scholar] [CrossRef]
- Chini, N.; Stansby, P. Extreme values of coastal wave overtopping accounting for climate change and sea level rise. Coast. Eng. 2012, 65, 27–37. [Google Scholar] [CrossRef]
- Gallien, T.; Sanders, B.; Flick, R. Urban coastal flood prediction: Integrating wave overtopping, flood defenses and drainage. Coast. Eng. 2014, 91, 18–28. [Google Scholar] [CrossRef]
- Tsoukala, V.K.; Chondros, M.; Kapelonis, Z.G.; Martzikos, N.; Lykou, A.; Belibassakis, K.; Makropoulos, C. An integrated wave modelling framework for extreme and rare events for climate change in coastal areas–the case of Rethymno, Crete. Oceanologia 2016, 58, 71–89. [Google Scholar] [CrossRef]
- Pullen, T.; Allsop, N.; Bruce, T.; Kortenhaus, A.; Schuttrumpf, H.; van der Meer, J. EurOtop (European overtopping manual): Wave overtopping of sea defences and related structures: Assessment manual. Available online: Http://www.Overtopping-manual.Com/manual.Html (accessed on 20 May 2016).
- Vousdoukas, M.I.; Voukouvalas, E.; Annunziato, A.; Giardino, A.; Feyen, L. Projections of extreme storm surge levels along Europe. Clim. Dynam. 2016. [Google Scholar] [CrossRef]
- Roelvink, D.; Reniers, A.; van Dongeren, A.; de Vries, J.V.T.; McCall, R.; Lescinski, J. Modelling storm impacts on beaches, dunes and barrier islands. Coast. Eng. 2009, 56, 1133–1152. [Google Scholar] [CrossRef]
- Giardino, A.; Briére, C.; Deserti, M.; Malmonado, V.M.; Reggiani, P.; van Ormondt, M.; Valentini, A.; van der Werf, J. Operational modelling applications for coastal engineering: New developments. In MEDCOAST 2011. Proceedings of the 10th International Conference on the Mediterranean Coastal Environment, Rhodes, Greece, 25–29 October 2011; Özhan, E., Ed.; Mediterranean Coastal Foundation, 2011. [Google Scholar]
- Barnard, P.L.; van Ormondt, M.; Erikson, L.H.; Eshleman, J.; Hapke, C.; Ruggiero, P.; Adams, P.N.; Foxgrover, A.C. Development of the coastal storm modelling system (COSMOS) for predicting the impact of storms on high-energy, active-margin coasts. Nat. Hazards 2014, 74, 1095–1125. [Google Scholar] [CrossRef]
- Gallien, T.; Guza, R. Modelling and observations of wave overtopping flooding on a southern California beach. In Proceedings of the 36th IAHR World Congress, The Hague, The Netherlands, 28 June–3 July 2015; International Association for Hydro-Environment Engineering and Research-IAHR: Madrid, Spain, 2015; p. 2. [Google Scholar]
- Gallien, T. Validated coastal flood modelling at Imperial Beach, California: Comparing total water level, empirical and numerical overtopping methodologies. Coast. Eng. 2016, 111, 95–104. [Google Scholar] [CrossRef]
- Palmsten, M.L.; Splinter, K.D. Observations and simulations of wave runup during a laboratory dune erosion experiment. Coast. Eng. 2016, 115, 58–66. [Google Scholar] [CrossRef]
- Wadey, M.P.; Nicholls, R.J.; Hutton, C. Coastal flooding in the Solent: An integrated analysis of defences and inundation. Water 2012, 4, 430–459. [Google Scholar] [CrossRef]
- Worni, R.; Huggel, C.; Clague, J.J.; Schaub, Y.; Stoffel, M. Coupling glacial lake impact, dam breach, and flood processes: A modeling perspective. Geomorphology 2014, 224, 161–176. [Google Scholar] [CrossRef]
- Elsayed, S.M. Comparative study of different scenarios for the morphological evolution in a river stream. Master’s Thesis, Politecnico di Milano, Milan, Italy, 2013. [Google Scholar]
- Radice, A.; Elsayed, S.M. Hydro-Morphologic Modelling for Different Calamitous Scenarios in a Mountain Stream. In Proceedings of the River Flow 2014: 7th International Conference on Fluvial Hydraulics, Lausanne, Switzerland, 3–5 September 2014; Schleiss, A.J., de Cesare, G., Franca, M.J., Pfister, M., Eds.; CRC Press/Balkema: Leiden, The Netherlands, 2014. [Google Scholar]
- Fan, X.; Tang, C.; van Westen, C.; Alkema, D. Simulating dam-breach flood scenarios of the Tangjiashan Landslide dam-induced by the Wenchuan Earthquake. Nat. Hazards Earth Syst. Sci. 2012, 12, 3031–3044. [Google Scholar] [CrossRef]
- Popescu, I.; Jonoski, A.; van Andel, S.; Onyari, E.; Moya Quiroga, V. Integrated modelling for flood risk mitigation in Romania: Case study of the Timis–Bega river basin. Int. J. River Basin Manag. 2010, 8, 269–280. [Google Scholar] [CrossRef]
- Tadesse, Y.B.; Fröhle, P. An integrated approach to simulate flooding due to river dike breach. In Proceedings of 11th International Conference on Hydroinformatics (HIC 2014), New York, NY, USA, 17–21 August 2014; CUNY Academic Works, 2014. [Google Scholar]
- Bertin, X.; Li, K.; Roland, A.; Zhang, Y.J.; Breilh, J.F.; Chaumillon, E. A modelling based analysis of the flooding associated with Xynthia, Central Bay of Biscay. Coast. Eng. 2014, 94, 80–89. [Google Scholar] [CrossRef]
- Roland, A.; Zhang, Y.J.; Wang, H.V.; Meng, Y.; Teng, Y.C.; Maderich, V.; Brovchenko, I.; Dutour-Sikiric, M.; Zanke, U. A fully coupled 3D wave-current interaction model on unstructured grids. J. Geophys. Res. Oceans 2012. [Google Scholar] [CrossRef]
- Vousdoukas, M.I.; Voukouvalas, E.; Mentaschi, L.; Dottori, F.; Giardino, A.; Bouziotas, D.; Bianchi, A.; Salamon, P.; Feyen, L. Developments in large-scale coastal flood hazard mapping. Nat. Hazards Earth Syst. Sci. 2016, 16, 1841–1853. [Google Scholar] [CrossRef]
- Elsayed, S.M.; Oumeraci, H. Effect of beach slope and grain-stabilization on coastal sediment transport: An attempt to overcome the erosion overestimation by XBeach. Coast. Eng. 2016. submitted for publication. [Google Scholar]
- Bendoni, M. Salt Marsh Edge Erosion due to Wind-Induced Waves. Ph.D. Thesis, Technische Universität Braunschweig & University of Florence, Braunschweig, Germany, 2015. [Google Scholar]
- De Vet, P. Modelling Sediment Transport and Morphology during Overwash and Breaching Events. Master’s Thesis, Delft University of Technology, Delft, The Netherlands, 2014. [Google Scholar]
- Hartanto, I.M.; Beevers, L.; Popescu, I.; Wright, N.G. Application of a coastal modelling code in fluvial environments. Environ. Model. Softw. 2011, 26, 1685–1695. [Google Scholar] [CrossRef]
- Beevers, L.; Popescu, I.; Pan, Q.; Pender, D. Applicability of a coastal morphodynamic model for fluvial environments. Environ. Model. Softw. 2016, 80, 83–99. [Google Scholar] [CrossRef]
- Vreugdenhil, C.B. Numerical Methods for Shallow-Water Flow; Springer Science & Business Media: Dordrecht, The Netherlands, 2013; Volume 13. [Google Scholar]
- Weiyan, T. Shallow Water Hydrodynamics: Mathematical Theory and Numerical Solution for a Two-Dimensional System of Shallow-Water Equations; Water&Power Press and Elsevier Science Publishers: Beijing, China, 1992; Volume 55. [Google Scholar]
- Saint-Venant, A.D. Theorie du mouvement non permanent des eaux, avec application aux crues des rivieres et a l’introduction de marees dans leurs lits. Comptes rendus des seances de l’Academie des Sciences 1871, 36, 174–154. [Google Scholar]
- Steffler, P.; Blackburn, J. River 2D Two-Dimensional Depth-Averaged Model of River Hydrodynamics and Fish Habitat: Introduction to Depth Averaged Modelling and User’s; University of Alberta. Available online: http://www.river2d.ualberta.ca/ (accessed on 2 March 2016).
- Szymkiewicz, R. Numerical stability of implicit four-point scheme applied to inverse linear flow routing. J. Hydrol. 1996, 176, 13–23. [Google Scholar] [CrossRef]
- Meyer-Peter, E.; Müller, R. Formulas for Bed-Load Transport: Report on Second Meeting of International Association for Hydraulics Research (IAHR); IAHR: Stockholm, Sweden, 1948. [Google Scholar]
- Pender, D.; Patidar, S.; Haynes, H. Incorporating river bed level changes into flood risk modelling. In Proceedings of the 36th IAHR World Congress, The Hague, The Netherlands, 28 June–3 July 2015; Tailor & Francis Groop: The Hague, The Netherlands, 2015. [Google Scholar]
- Brunner, G.W. HEC-RAS River Analysis System. Hydraulic Reference Manual. Version 5.0; United States Army Corps of Engineers: Davis, CA, USA, 2016. [Google Scholar]
- Roelvink, D.; van Dongeren, A.; McCall, R.; Hoonhout, B.; van Rooijen, A.; van Geer, P.; de Vet, P.; Nederhoff, K.; Quataert, E. XBeach Technical Reference: Kingsday Release (Technical Report: Model Description and Reference Guide to Functionalities); Deltares, UNESCO-IHE Institute of Water Education and Delft University of Technology: Delft, The Netherlands, 2015; p. 141. [Google Scholar]
- Holthuijsen, L.; Booij, N.; Herbers, T. A prediction model for stationary, short-crested waves in shallow water with ambient currents. Coast. Eng. 1989, 13, 23–54. [Google Scholar] [CrossRef]
- Buckley, M.; Lowe, R.; Hansen, J. Evaluation of nearshore wave models in steep reef environments. Ocean Dyn. 2014, 64, 847–862. [Google Scholar] [CrossRef]
- Sultan, N.J. Irregular Wave-Induced Velocities in Shallow Water; Ocean Engineering Department Texas A&M University: College Station, Texas, USA, 1992. [Google Scholar]
- Vetsch, D.; Müller, R.; Rousselot, P.; Volz, C.; Vonwiller, L.; Farshi, D.; Veprek, R.; Fäh, R. Basement Reference Manual; the Laboratory of Hydraulics, Hydrology and Glaciology (VAW) of the Swiss Federal Institute of Technology (ETH): Zurich, Switzerland, 2016; Available online: http://www.basement.ethz.ch/ (accessed on 30 May 2016).
- Andrews, D.G.; McIntyre, M. An exact theory of nonlinear waves on a lagrangian-mean flow. J. Fluid Mech. 1978, 89, 609–646. [Google Scholar] [CrossRef]
- Walstra, D.; Roelvink, D.; Groeneweg, J. Calculation of wave-driven currents in a 3d mean flow model. In Proceedings of the 27th International Conference on Coastal Engineering ICCE, Sydney, Australia, 16–21 July 2000; Edge, B.L., Ed.; ASCE, 2001; Volume 2, pp. 1050–1063. [Google Scholar]
- Ambrosio, B.; Siegle, E. Wave energy levels at inlet channel margins: The effects of the ebb-tidal delta morphology. In Proceedings of the 17th Physics of Estuaries and Coastal Seas (PECS) Conference, Porto de Galinhas, Pernambuco, Brazil, 19–23 October 2014; FCERM.net: Pernambuco, Brazil, 2014; p. 4. [Google Scholar]
- Swartenbroekx, C.; Soares-Frazão, S.; Staquet, R.; Zech, Y. Two-dimensional operator for bank failures induced by water-level rise in dam-break flows. J. Hydraul. Res. 2010, 48, 302–314. [Google Scholar] [CrossRef]
- Evangelista, S.; Greco, M.; Iervolino, M.; Leopardi, A.; Vacca, A. A new algorithm for bank-failure mechanisms in 2d morphodynamic models with unstructured grids. Int. J. Sediment Res. 2015, 30, 382–391. [Google Scholar] [CrossRef]
- Wainwright, D.; Baldock, T. Measurement and modelling of an artificial coastal lagoon breach. Coast. Eng. 2015, 101, 1–16. [Google Scholar] [CrossRef]
- Vellinga, P. Beach and Dune Erosion During Storm Surges. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 1986. [Google Scholar]
- Visser, P. Breach Growth in Sand-Dikes. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 1998. [Google Scholar]
- Bakker, W.; van der Graaff, J.; Kraak, A.; Smit, M.; Snip, D.; Steetzel, H.; Visser, P. Het Zwin, Successen en Lessen: Bresgroeiexperimenten 6 en 7 Oktober 1994 “Het Totale Experiment Geslaagd”; TU Delft, Department Hydraulic Engineering: Delft, The Netherlands, 1996. [Google Scholar]
- Elsayed, S.M.; Oumeraci, H. Breaching of Coastal Barriers Under Extreme Storm Surges and Implications for Groundwater Contamination: Application of XBeach in Coastal Flood Propagation; TU Braunschweig: Internal report; Leichtweiß-Institut für Wasserbau: Braunschweig, Germany, 2016. [Google Scholar]
- Morris, M.W. Breaching of Earth Embankments and Dams. Ph.D. Thesis, Open University, UK, 2011. [Google Scholar]






| Parameter | Value | Meaning | Note |
|---|---|---|---|
| D50 | 2 mm | Median grain size | |
| dx | 2 m | Cross-shore spatial step | Regular spatial step |
| dy | 5 m | Profile width | |
| Bedfriction | n | Manning parameter (selected as representative for the bed friction) | |
| bedfriccoef | 0.03 m-1/3·s | Value of Manning coefficient | This value is generalised over the whole model |
| instat | 4 or jons | Standard Joint North Sea Wave Project (JONSWAP) JONSWAP spectrum is selected as an upstream wave boundary condition | The selected spectrum coefficients are: Hs (significant wave height) = 1.5 m and Tp (peak period) = 6.6 s |
| front | 0 | Absorbing–generating weakly-reflective boundary is used as a 1D inflow boundary | |
| back | 1 | Absorbing–generating weakly-reflective boundary is used as a 1D outflow boundary | |
| left | 1 | Impermeable wall is a lateral flow boundary | Left side wall of the numerical wave flume |
| right | 1 | Impermeable wall is a lateral flow boundary | Right side wall of the numerical wave flume |
| lateralwave | Neumann | Neumann boundary is a lateral wave boundary in both lateral sides | The alongshore gradient of the wave energy is zero at the lateral boundaries |
| asabeta | 11.30 | Average slope angle accounts for the beach slope effect on wave nonlinearity | See Elsayed and Oumeraci, (2016 [39]) |
| facpi | 1 | Grain-stabilization effect is not considered | See Elsayed and Oumeraci, (2016 [39]) |
| form | 2 | Sediment transport is calculated according to Van Thiel–Van Rijn formulation | See Roelvink et al. (2015 [52]) |
| tstop | 3600 s | Simulation time | |
| tint | 1 s | Output time step |
| Parameter | Value | Meaning | Note |
|---|---|---|---|
| front | 1 | Absorbing–generating weakly-reflective boundary is used as a 2D inflow boundary | |
| left | 0 | Neumann boundary is a lateral flow boundary | Neumann means that gradient of the lateral outflow is zero |
| right | 0 | Neumann boundary is a lateral flow boundary | |
| back | 2 | Absorbing–generating weakly-reflective boundary is used as a 2D outflow boundary | |
| lateralwave | Neumann | Neumann boundary is a lateral wave boundary in both lateral sides of the model | |
| dy | 2 m | The spatial step in the longshore direction | Regular spatial step |
| morfac | 10 | Factor in Exner equation to accelerate the calculations of the morphological evolution | See Roelvink et al. (2015 [52]) |
| tstop | 5400 s | Simulation time | |
| tint | 5 s | Output time step |
| Parameter | Value | Meaning | Note |
|---|---|---|---|
| facpi | 1.3 | Grain-stabilization effect is considered by increasing the critical stirring velocity by 30% | See Elsayed and Oumeraci, (2016 [39]) |
| form | 2 | Sediment transport is calculated according to Van Thiel–Van Rijn formulation | See Roelvink et al. (2015 [52]) |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Elsayed, S.M.; Oumeraci, H. Combined Modelling of Coastal Barrier Breaching and Induced Flood Propagation Using XBeach. Hydrology 2016, 3, 32. https://doi.org/10.3390/hydrology3040032
Elsayed SM, Oumeraci H. Combined Modelling of Coastal Barrier Breaching and Induced Flood Propagation Using XBeach. Hydrology. 2016; 3(4):32. https://doi.org/10.3390/hydrology3040032
Chicago/Turabian StyleElsayed, Saber M., and Hocine Oumeraci. 2016. "Combined Modelling of Coastal Barrier Breaching and Induced Flood Propagation Using XBeach" Hydrology 3, no. 4: 32. https://doi.org/10.3390/hydrology3040032
APA StyleElsayed, S. M., & Oumeraci, H. (2016). Combined Modelling of Coastal Barrier Breaching and Induced Flood Propagation Using XBeach. Hydrology, 3(4), 32. https://doi.org/10.3390/hydrology3040032





