Adaptive Operating Rules for Flood Control of a Multi-Purpose Reservoir

Abstract

Almost all multipurpose reservoirs in Romania were put into operation 30–50 years ago or even earlier. Meanwhile, a large volume of hydrologic data has been collected, and the initial design flood should be reconsidered. Consequently, the operating rules of flow control structures (bottom gates and weir gates) should be re-examined, mainly for medium and low-frequency floods. The design flood is not unique, being characterized by different shapes and time to peak, which has consequences for flood mitigation rules. Identifying the critical design flood is an important preliminary step, although it is usually neglected in flood management. Simulating the operation of the Stânca–Costești reservoir on the Prut River, it was found that the design flood corresponding to the maximum value of the compactness coefficient is the most difficult to mitigate considering the specific conditions of the dam and the reservoir: the prescribed conservation level in the reservoir, and the design flood volume of medium and rare floods that far exceeds the flood control volume. These conditions can jeopardize both dam safety and downstream flood protection. The main steps of the proposed approach are as follows: (1) developing the hydraulic model; (2) statistical processing of the registered floods and defining critical design floods for different AEPs (Annual Exceedance Probabilities); (3) deriving optimal operation rules based on a simulation-optimization model; (4) implementing real-time adaptive operation of the mechanical outlets; and (5) critically assessing the operating rules after the event. Based on the hydrological forecast, if necessary, new outlets are put into operation while keeping the ones already activated. Based on the hydrological forecast and properly operated, the safety of the Stânca–Costești dam is guaranteed even in the event of a 0.1% CC (Climate Change) flood. However, for floods greater than 1% magnitude, the carrying capacity of the downstream riverbed is exceeded. The main gaps addressed in this paper are the following: (1) the establishment of critical design floods, and (2) the adaptive operating rules of outlet devices aimed at optimizing flood control results, using short-term flood forecasts.

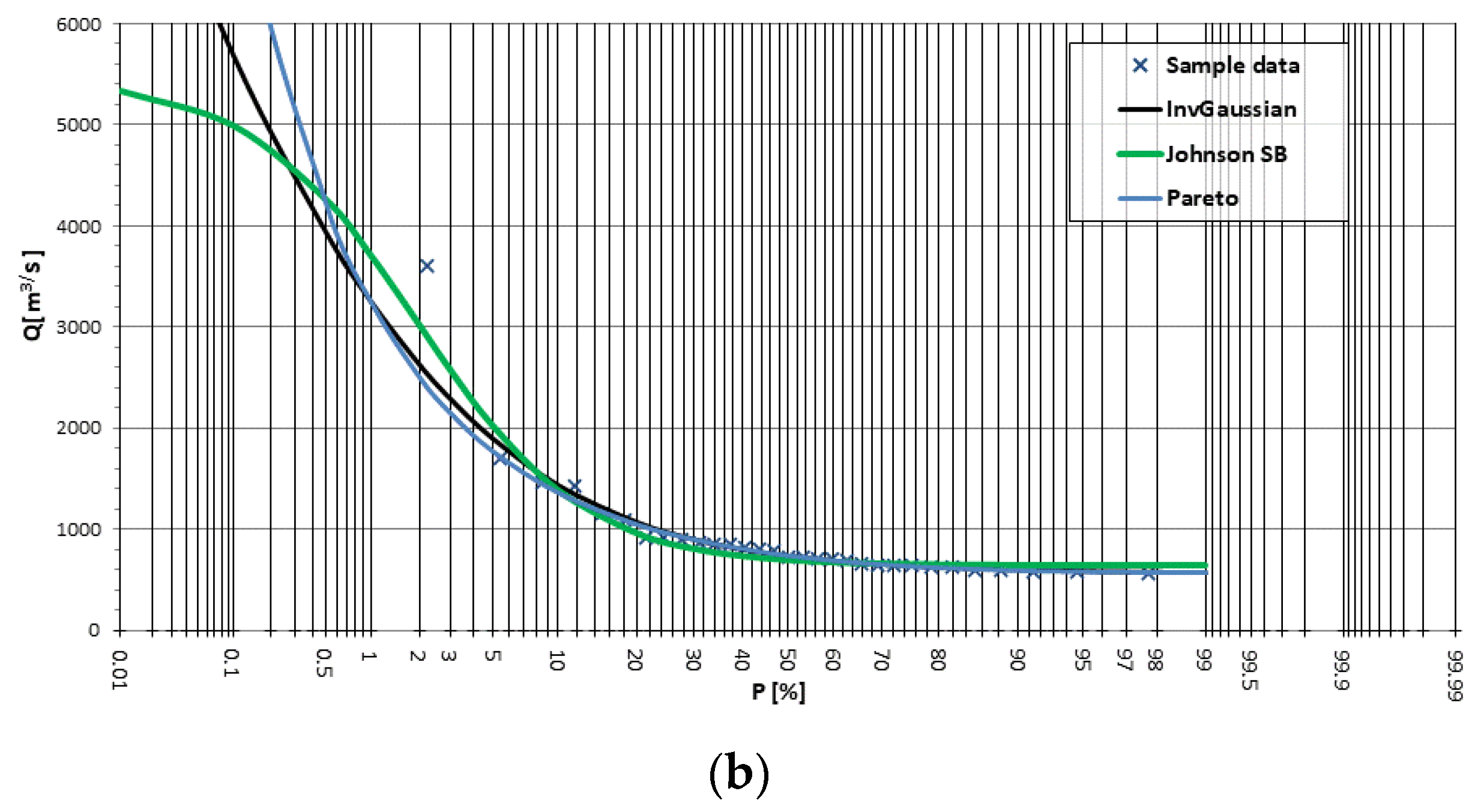
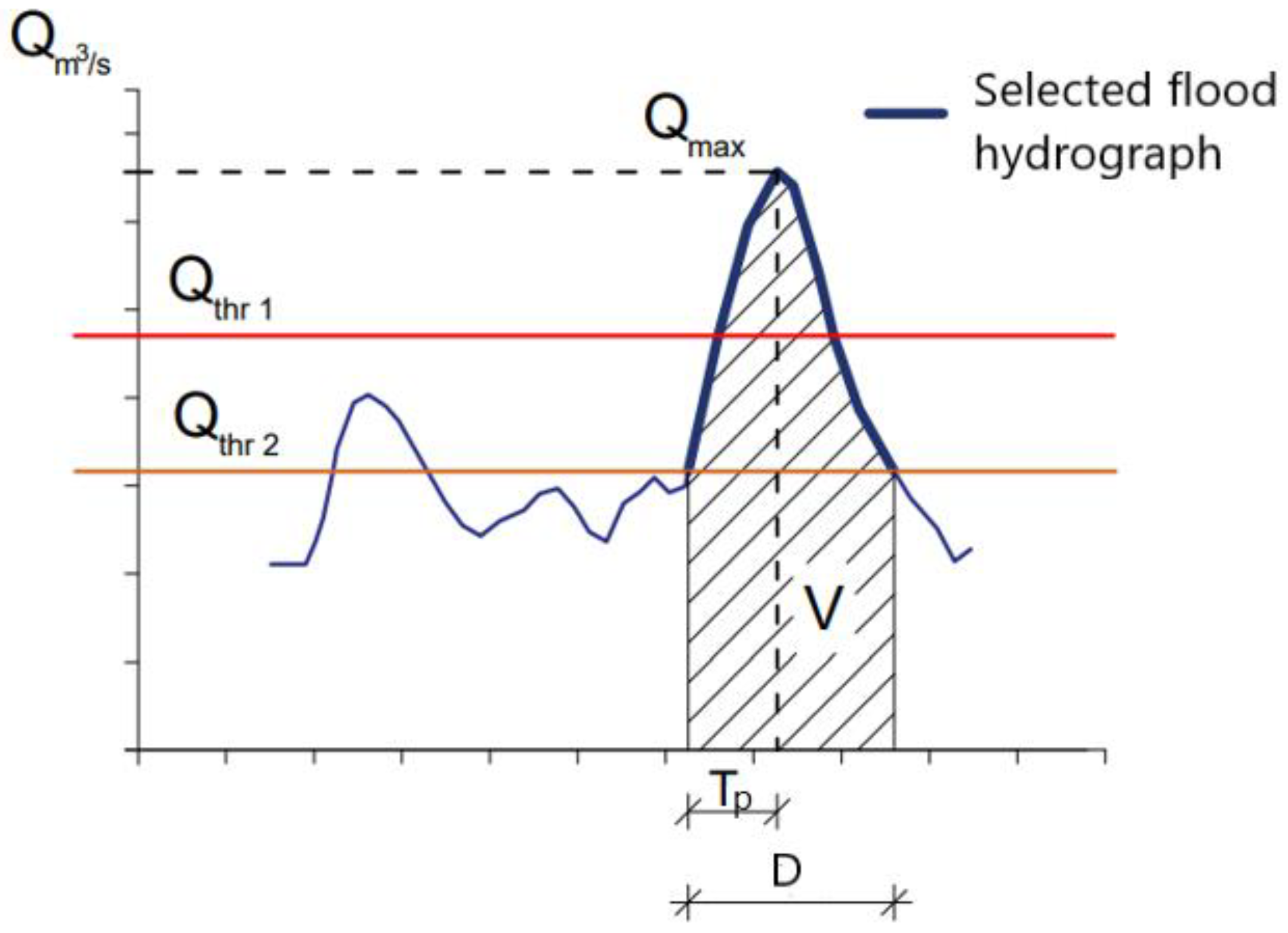
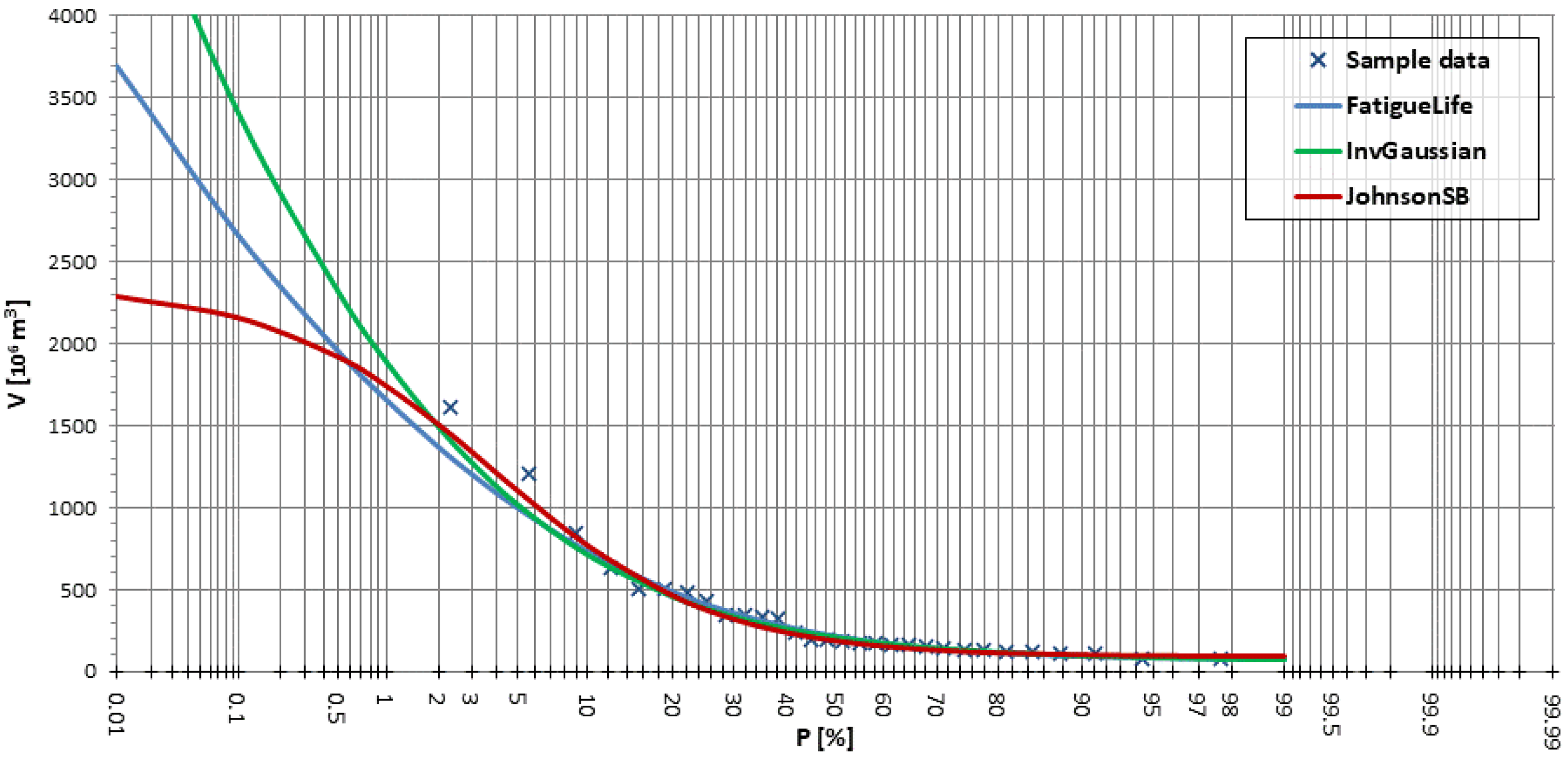
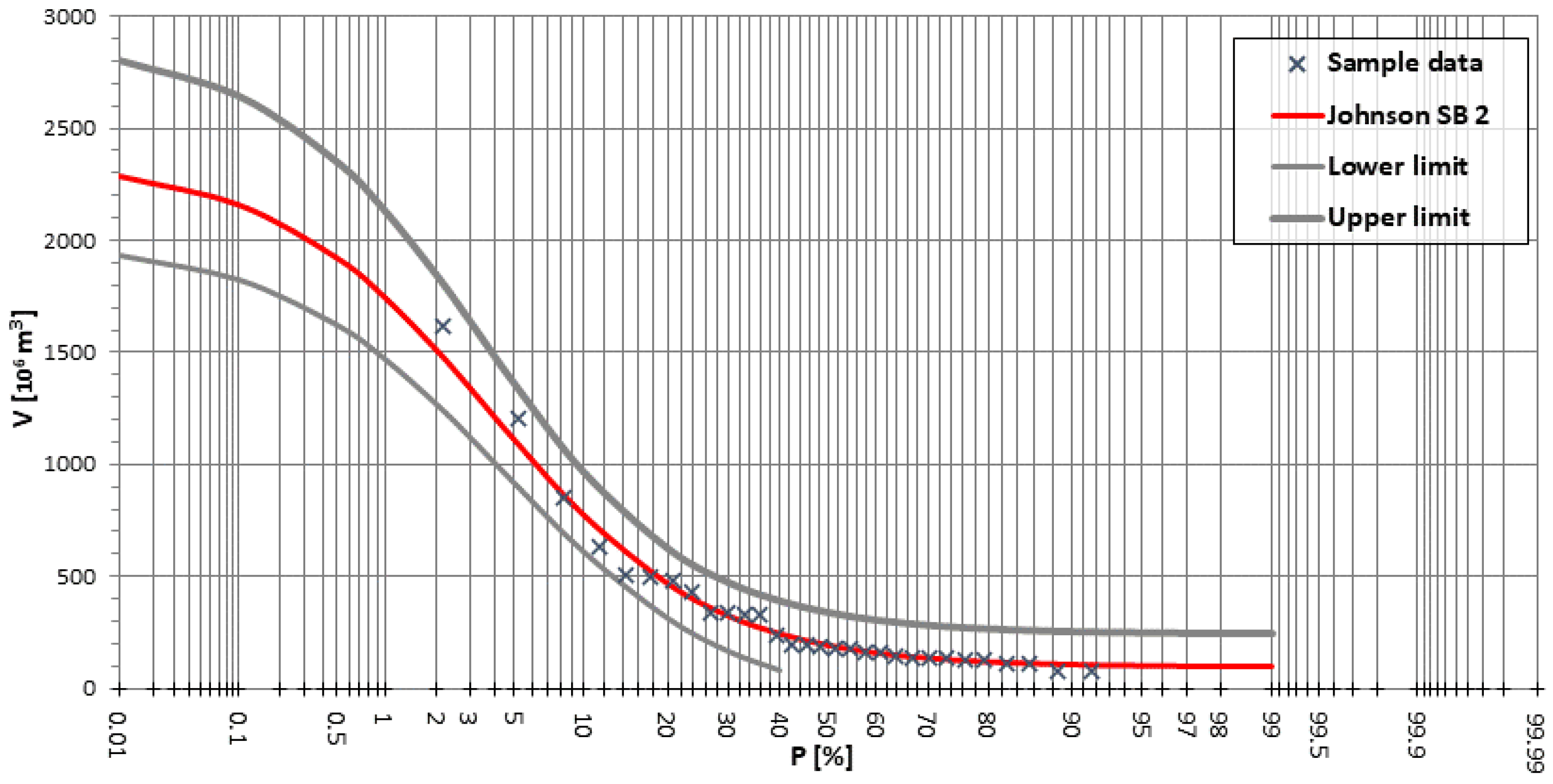
1. Introduction

Floods are a dangerous natural phenomenon that leads to serious consequences for human health and life, the environment, cultural heritage, economic activity, and infrastructure associated with flood protection [1]. Floods are the most frequent natural disasters with up to 1000 fatalities per event. A comprehensive global analysis of flood fatalities over spatial, temporal, and economic scales was made available by Jonkman et al. [2]. On the global scale, due to flood management practices, the average number of fatalities per event has declined over time, despite population growth and increasing flood hazards [2]. Due to urbanization on a global scale and the continuous increase in impervious surfaces in urban areas, maximum flood flows and volume are amplified [3]. Cities are becoming hotspots capable of generating much more severe flooding downstream than before urbanization. At the same time, urban areas concentrate valuable assets, making urban flood management a priority of flood risk basin management [3]. Flood damage to buildings and their contents in urban areas depends not only on depth, but also on water velocity, water contact time, associated pollution, and warning time [4]. Understanding the close link between hydroclimatic extremes and societal components provides stakeholders and authorities with a scientific basis for planning sustainable strategies and ensuring human well-being [5]. Due to climate change, the threat of floods continuously evolves [6]. More frequent and severe floods are expected in the future in some parts of Europe [7], while in other parts a decrease in flood magnitude or frequency could happen [6].

The flood protection level is usually defined as the probability of overtopping or exceeding the design limits of a hydraulic structure. Flood protection level can also be defined as the probability of occurrence/exceedance of significant socio-economic impacts within a subnational region [8]. Flood protection can be achieved through structural or non-structural measures [1,9] (pp. 1–20) [10]. Structural measures consist of hydraulic engineering works, while non-structural measures are all other types of interventions [11]. Structural measures are linked to the river, while non-structural measures generally extend throughout the river basin.

The classic structural solution for the protection of flood zones is represented by dikes. Made locally, they have favourable effects by protecting urban areas and industrial sites. However, the construction of dykes over long stretches amplifies peak water levels. Thus, the expansion of high dyke construction in the upper Mekong Delta from 2000 to 2011 resulted in a significant increase in flood water levels of up to +68 cm in the upper delta [12]. If the levee fails due to extraordinary flooding, the damage can be considerable. In Germany during the 2002 floods, more than 130 dike breaches occurred along the Elbe and its tributaries, causing total damage of approximately EUR 15 billion [13].

Dams and corresponding reservoirs represent major structural measures [14] providing basic protection for flood management. River reservoirs (on-line reservoirs) and/or bank-side reservoirs (off-line reservoirs) are used with high efficiency for flood mitigation [15]. Dams stimulate economic and social development worldwide [16]. Many dams are under construction [16], especially in developing countries that need to conserve water resources for water supply, energy production, and flood control. Reservoir and dam operation reduces both the extent and frequency of floods, as well as the extent of flooded areas [16] and thereby flood damage in downstream areas. Thus, the operation of the Zaung Tu Dam on the Bago River in Myanmar reduces the flood area by about 10% and the flood damage to buildings, assets, and agriculture by about 40%, 60%, and 10%, respectively [17]. Existing dams will face new weather conditions and flood patterns in the future that increase the risk of structural failure. Increasing flood volumes or higher discharges require new dam release operations to maintain desired levels of flood protection under climate change [16]. Dams play an undeniable role in reducing the risk of flooding due to climate change. Despite their positive effects, dams fragment rivers, leading to severe environmental impact in some cases [18]. Consequently, the promotion of new dam works must also consider their negative environmental and social effects to ensure the sustainable development of society [16].

Diversions that transfer excess water from a river basin to a neighbouring one are another solution used with reliable results in mitigating the consequences of flooding. Formerly flooded areas, now protected by dikes, can also be converted into controlled flooded polders to reduce flood peaks [19].

In the case of medium (1% AEP) or rare floods (0.1% AEP), defined according to European legislation [1], the structural measures, especially the dams and the reservoirs behind them, provide protection against flooding corresponding to the design standards. Reservoirs retain floodwater, with the objective of minimizing the peak flow downstream of the dam, while keeping the water level in the reservoir under control [20,21,22]. Despite their essential role in flood protection, structural measures represent an amplifier of hazard in case of failure [23].

Non-structural measures include not only traditional approaches such as land use planning, dike relocation, building codes, floodproofing, detection and early warning, evacuation plans, and risk transfer instruments [11], but also virtual databases and flood management Decision Support Systems [24]. In Europe, the use of non-structural measures increased rapidly in the 21st century following the catastrophic floods that occurred after 2002 on the Elbe and Danube rivers [11]. For already existing hydraulic works, updating the operating rules in accordance with the new hydrological data, recorded sometimes decades after the commissioning of the dams, represents a necessary non-structural measure that is easy to implement and that also provides flood reduction [25].

According to the operating rules, the water released from the reservoir depends on the current reservoir water level (representing the state variable of the reservoir) and the hydro-meteorological conditions, as well as the accuracy of the forecasted inflow [26,27,28,29]. The determination of optimal rules aimed at maximising net benefits in reservoir operation can be treated as a direct problem in which the parameters of the operating rules are obtained by simulation through a trial-and-error procedure [30] (pp. 59–78), or as an inverse problem based on simulation-optimization [31].

Non-structural measures generally represent solutions in full alignment with natural processes. However, non-structural measures alone cannot provide complete flood protection. Floods have been recorded throughout history even when human interventions in natural processes were almost negligible. Non-structural measures are effective for small upstream catchments (10–100 km²) for flood protection up to 2% AEP [32]. For lower AEPs and larger catchments, they represent necessary complementary measures to structural engineering solutions [24].

There are different categories of reservoirs used for flood mitigation: flood storage reservoirs and multi-purpose reservoirs. Flood storage reservoirs are designed for flood protection only by temporarily storing water during flood events [33]. Multi-purpose reservoirs have two main objectives: providing water to the water users and flood attenuation [34,35]. According to ICOLD statistics, more than 58,000 large dams (dam height > 15 m and volume > 1 million m³) have been built worldwide for flood control and water conservation for irrigation, public and industrial water supply, or hydropower generation [36,37]. The conservation volume, used for reliable water supply, is computed based on hydrological data and water demands on a monthly scale. The attenuation in the flood protection volume is based on hydrological data on a much smaller temporal scale, usually an hour or a few hours. A 24 h time step is used only for exceptionally large reservoirs.

The calculation of the flood defence works is based on the design flood, which is the “flood that a dam facility should be able to withstand and convey safely without being seriously damaged” [38]. A similar definition, which also includes the check flood, is provided by Patterson [33]: “the dam structures will need to be designed to accommodate the design flood with an appropriate freeboard, and the dam must be capable of withstanding passage of the safety check flood without failure”. The hydrological safety of dams and the safe design of flood spillways are major concerns, especially now under climate change [18].

The most widely used design flood estimation technique for gauged catchments is at-site flood frequency analysis [39]. Usually, the flood protection level is associated with the annual probability of exceedance $P\%$ of the maximum discharge. The average exceedance period $T=1/P$ is also used to characterise the maximum annual discharge, where T represents the average number of years (on a geological scale) in which a maximum flow equal to or greater than $Q_{P\%}$ occurs only once. Usually, the average exceedance period is known as the return period, although this name suggests the occurrence of a flood with the maximum discharge $Q_{P\%}$ exactly when the T years are completed, which is obviously not true.

Maximum discharge cannot be considered the only important parameter of the flood. Flood volume also plays a significant role in determining flood operation rules, especially when the flood protection volume is small compared to the design flood volume [40,41]. Relatively recently, design hydrographs have been characterized by maximum annual discharge, volume, and duration [42,43]. Time to peak is also added [43]. If these variables are uncorrelated, a univariate frequency analysis can be used for each of them in the design process. However, peak discharge and flood volume, as well as flood volume and duration, are correlated variables making the univariate statistical analysis unsatisfactory. The traditional univariate flood frequency analysis does not reflect the complexity of floods [44]. Therefore, the design flood should be defined in terms of at least two variables [45]. A bivariate analysis will lead to more appropriate estimates than a univariate analysis [46]. Dam overtopping represents more than 40% of dam failures worldwide according to ASCE/USCOLD, cited by [18]. Not only the maximum discharge but also the flood volume that cannot be accommodated in the reservoir during the flood event contributes to dam accidents.

The type and dimensions of the outlet devices are critical to the sizing and operation of the flood volume [33]. There are two main types of control devices: fixed flow control structures without moving parts, and variable flow control structures which have moving parts like gates [33]. In the case of fixed flow control structures, the attenuation is strictly unsupervised [22], without any human intervention. The excess volume is being discharged downstream through uncontrolled spillways, regardless of whether the carrying capacity of the riverbed is exceeded or not. The main concern of the designer is to guarantee the integrity of the dam. In the case of variable flow control structures, human intervention is required to decide the operating rules of the bottom gates and weirs. Depending on the hydrological forecast and the water level in the reservoir, the outlets are opened, closed, or held in an intermediate position. When the flood volume far exceeds the available storage volume, the excess water must be released to prevent dam overtopping [33].

Various constraints must be met. Flood discharges downstream of the dam must be reduced to less than the carrying capacity of a downstream watercourse or the floodplain between the dikes (if applicable). At the same time, the maximum water level in the reservoir must be kept below the safety level [47] to prevent dam failure, while the gradient of rise and fall of the water level in the reservoir is limited due to possible landslides of contour dikes or surrounding slopes. The flood-carrying capacity of the downstream channel can be a key factor in flood control decisions [48] for current floods (10% or higher AEP of the peak flow), while the maximum water level in the reservoir is a key factor for medium and rare floods (below 1% and 0.1%, respectively).

Old dams have bottom gates of small dimensions for structural security reasons. Consequently, their discharge capacity is small, which limits efficient pre-release of the reservoir to create additional attenuation volume. On the other hand, many reservoirs have the conservation level (known also as full supply level) at the crest of their spillway, the attenuation occurring due to temporary storage above the crest. Under these conditions, in many cases the downstream flow cannot be kept below the bankfull discharge. Improved flood attenuation is reached when a special volume for flood control is reserved below the spillway crest, although a strong opposition of the other users, like hydroelectricity, can be met.

Real-time reservoir operation for flood control refers to the optimal operation of an existing reservoir based on flood forecast information [27]. Forecast-informed management based on hydrologic and hydraulic models has the potential to maximize the value of reservoir operations facing conflicting objectives [49], increasing variability [50] and more frequent hydrological extremes [51] under climate change. Although hydrological forecast predictability decreases with longer lead times [50], and the physical constraints can limit the value of forecast information [49], the real-time operation of many reservoirs is based on flood forecasts and a forecast update procedure [27]. The forecast accuracy of predicting future flow significantly affects the decisions that must be made for reservoir operation [52]. Early pre-release creates an effective buffer volume for flood control [50]. Lead time can be increased by coupling weather forecasting with rainfall–runoff models.

Optimum operation of reservoirs means deciding when to open/close the variable flow control structures according to the distinct phases of the flood: pre-flood, during flood, and post-flood [25,28]. As previously mentioned, the pre-release increases the available flood storage capacity. During the flood event, a delicate balance must be maintained between storing water in the reservoir and releasing it. In the recession phase, retained water must be released downstream in preparation for the next flood [28]. The efficiency of the pre-release depends on the discharge capacity of the bottom openings of the dam. The post-flood decision to retain or release water depends on the hydrological forecast and the economic value of the water retained in flood protection volume (mainly for hydroelectricity production). Thus, in flood control, the focus must be directed to operating rules around the flood peak. The operating framework rules are defined in the design stage for the standard AEPs: 10%, 1%, 0.1%, and 0.01%. In general, national guidelines or standards do not specify whether the probability of exceedance refers only to the peak flow, the flood volume, or the entire design flood [53]. Since the dams were put into operation, sometimes decades ago, significant volumes of hydrological data have been accumulated, and the initial design flood should be reconsidered. Consequently, the operating rules of flow control structures should also be re-examined. Among the many possible design floods of different shapes, the critical design flood corresponding to the maximum value of the compactness coefficient decides the operating rules for flood mitigation.

In this work, the critical design flood hydrograph (including the CC scenario) is obtained for each AEP. The corresponding optimal operating rules for these AEPs are obtained based on a simulation-optimization model, where the bottom outlets and weir gates are individually actuated. The outlets are opened or closed during the event with the aim of flattening the downstream flood as much as possible, without exceeding the prescribed maximum water level in the reservoir. However, when the flood warning is issued the exceedance probability of the flood magnitude is not known. At the same time, the actual flood shape is always different from the idealized design flood shape. Consequently, water managers must adapt the operating rules in real-time, trying to fit the flood into one of the predefined operating framework rules. Increases in peak flows and flood volume due to climate change exacerbate the situation, especially when the flood volume far exceeds the flood control volume.

The main gaps addressed in this paper are as follows: (1) the establishment of critical design floods, and (2) the development of adaptive operating rules of outlet devices aimed at optimizing flood control results, using short-term flood forecasts. The paper is organized as follows: Section 2 deals with the methods used in this study; Section 3 provides a case study and the obtained results; Section 4 contains the discussion, and Section 5 presents the main conclusions.

2. Materials and Methods

2.1. Case Study

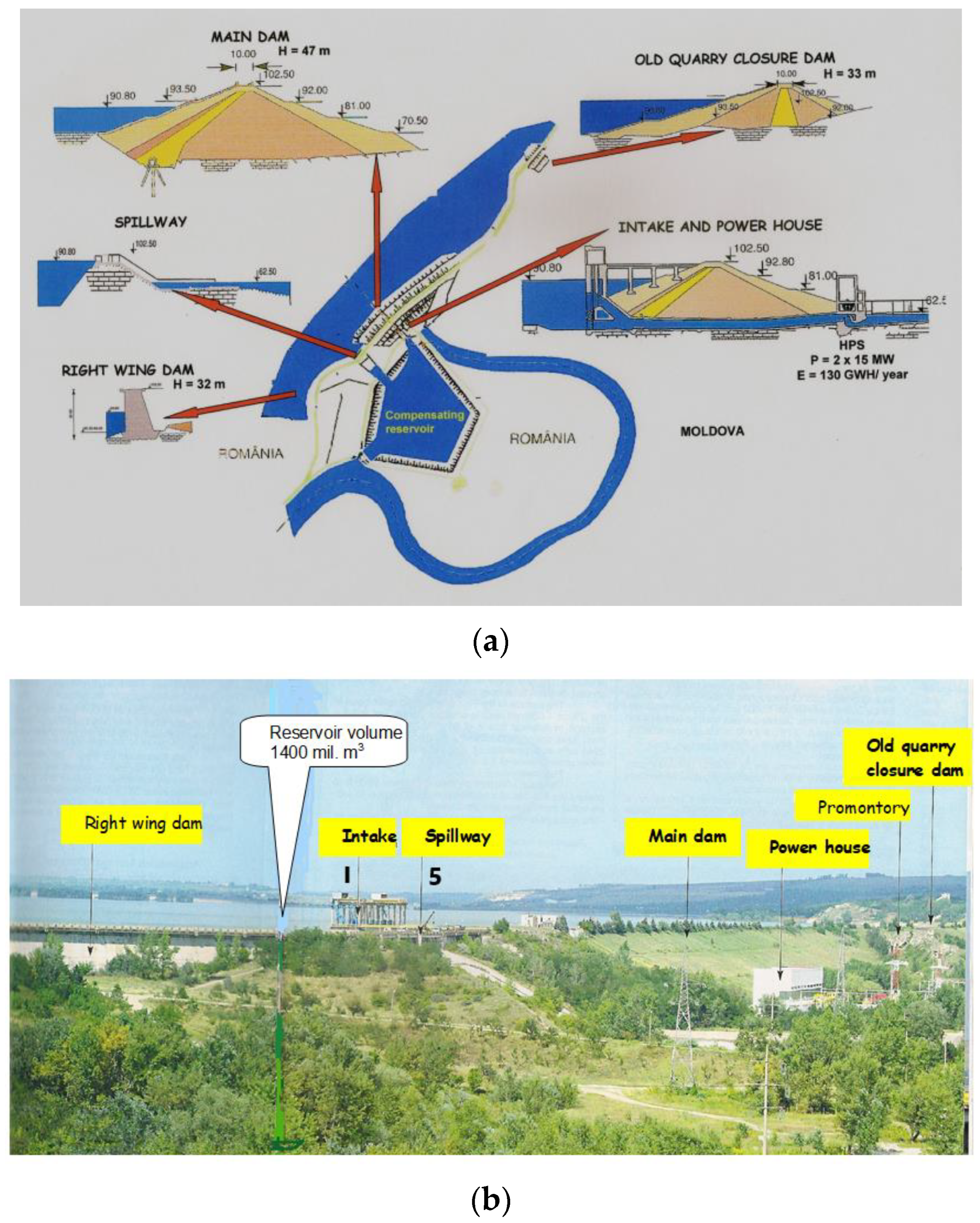
The Prut River Basin is in the northeastern part of the Danube Basin and is bordered by the following river basins: the Tisa to the northeast, the Siret to the west, and the Dniester to the north and east. The basin covers a total area of 28,433 km², with approximately 39% of it situated in Romania. The Stânca–Costești dam and appurtenant works (Figure 1) are in northeastern Romania on the border between Romania and the Republic of Moldova.

The Prut River (991.2 km) is the second longest tributary of the Danube. It forms the border between Ukraine and Romania for 39 km and between Romania and the Republic of Moldova for 713 km. Compared to the entire Prut River basin, the drainage basin of the Stânca–Costești reservoir (12,212 km²) is much more rugged. Thus, the average slope of the river is 4.2‰ upstream of the reservoir (1.7‰ at the level of the entire hydrographic basin), the average elevation is 446 m (227 m for Prut R.B.), and the average slope of the basin is 12.2% (9.7% for Prut R.B.). The Rădăuți–Prut gauge station, upstream of the reservoir, monitors the drained flow over an area of 9296 km².

Among the 247 large dams in Romania, the Stânca–Costești Dam ranks 49th in terms of dam height and second, after the Iron Gates on the Danube, in terms of reservoir volume. The main purposes of the reservoir are flood control, hydroelectricity production, water supply, irrigation, and fish farming. The construction period was between 1973 and 1979. The surface area of the reservoir is 77 km², whilst its length, at maximum water level during floods, is about 120 km. The retention front is approximately 3 km long and is composed of different types of dams connected between them [54] (pp. 272–276): a buttress dam (370 m long), continued by a gravity dam (650 m), an earthfill dam (representing the main dam—750 m), a reinforced concrete dam (180 m long), and another earthfill dam (235 m). All dams have the crest elevation at 102.50 MASL.

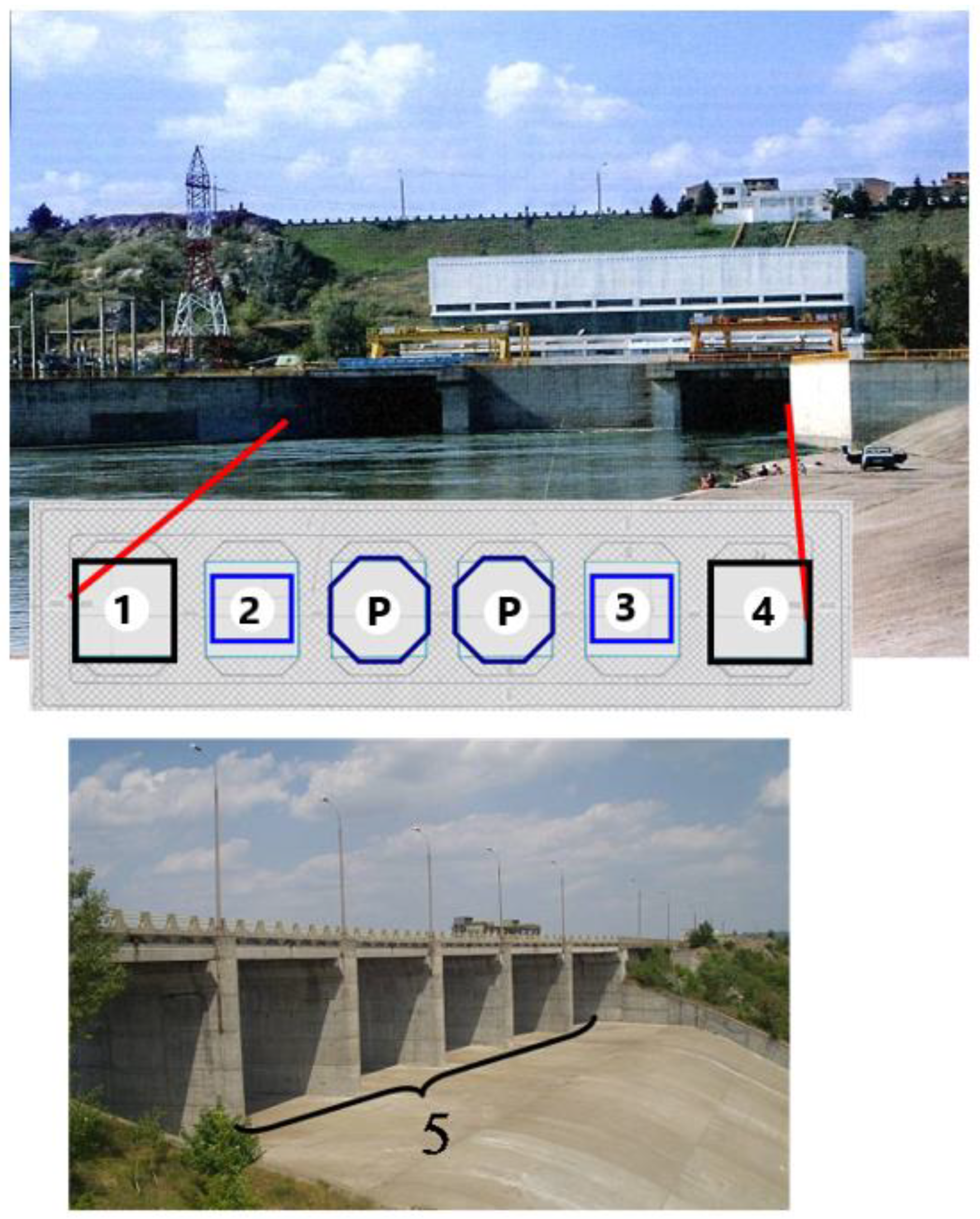
The dam has the following discharge equipment: two power galleries (P in Figure 2); a backup intake equipped with a hydraulically operated sluice gate (I in Figure 1b); two central conduits and narrow bottom outlets with a section of 9 m² each (no. 2 and no. 3 in Figure 2); two lateral conduits and bottom outlets with a section of 15.76 m² each (no. 1 and no. 4 in Figure 2); and an overflowing weir of curved profile, with 6 bays equipped with flap gates, 16 × 2.70 m², each hydraulically operated (no. 5 in Figure 1b and Figure 2).

The main characteristics of the reservoir are presented in

Table 1. Stânca–Costești characteristics.

Level	Elevation (MASL)	Volume (mil. m3)
Dead volume level	78.00	175
Minimum storage level	81.50	285
Conservation level	90.80	735
Spillway crest level	95.50	1060
Flap gates level = 1% water level *	98.20	1285
0.1% water level *	99.50	1400
Dam crest level	102.50	1709

* According to the design phase.

.

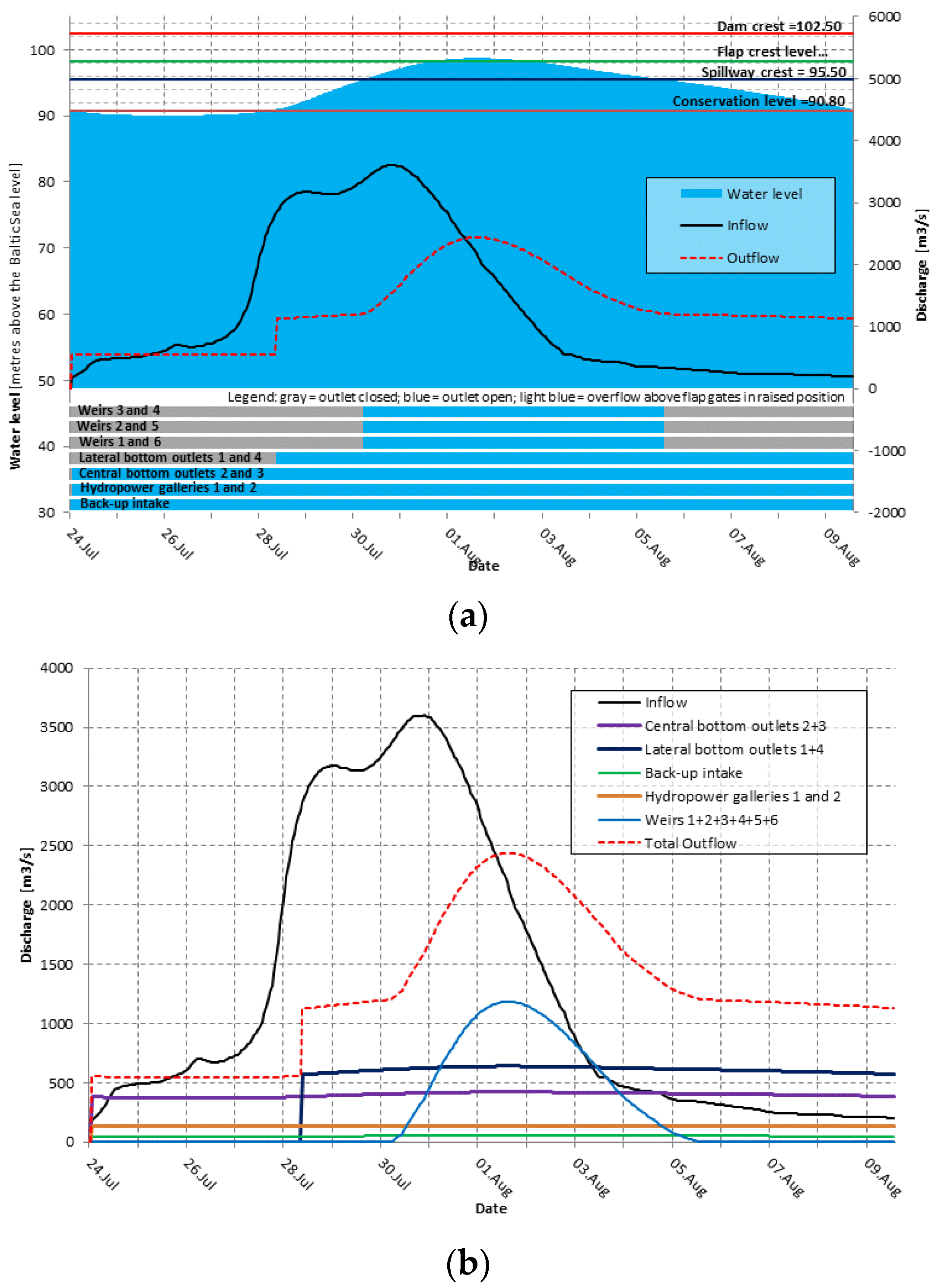
The flood control volume between the top of the conservation level and the top of the surcharge pool (the maximum allowed water level in the reservoir during extraordinary floods, corresponding to 0.1% AEP of the maximum discharge) amounts to 665 mil. m³. This includes 325 mil. m³ between the level of the spillway crest and the conservation level, 225 mil. m³ between the flap gates level and spillway crest, and 115 mil. m³ between the 0.1% water level and flap gates level.

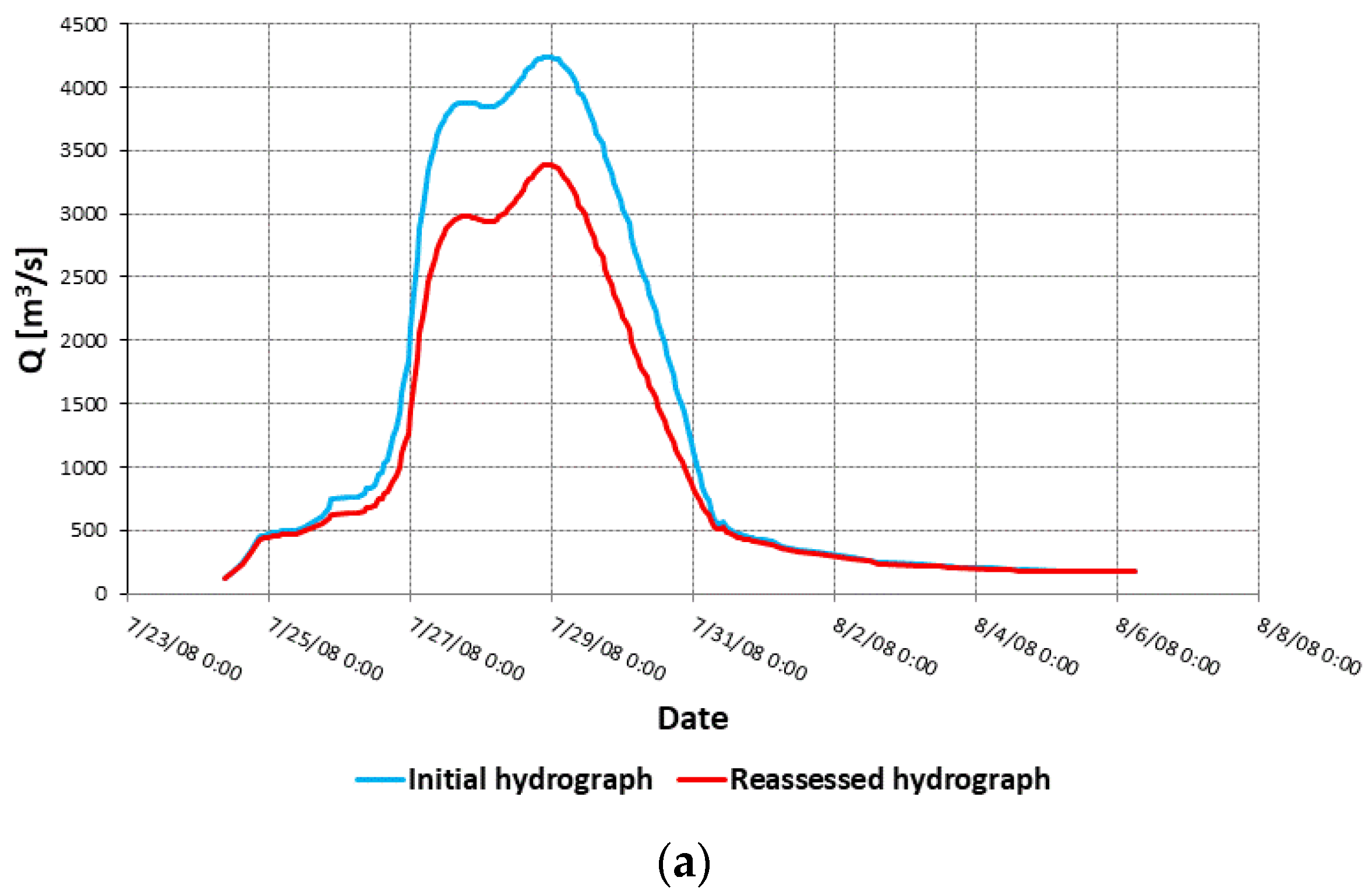
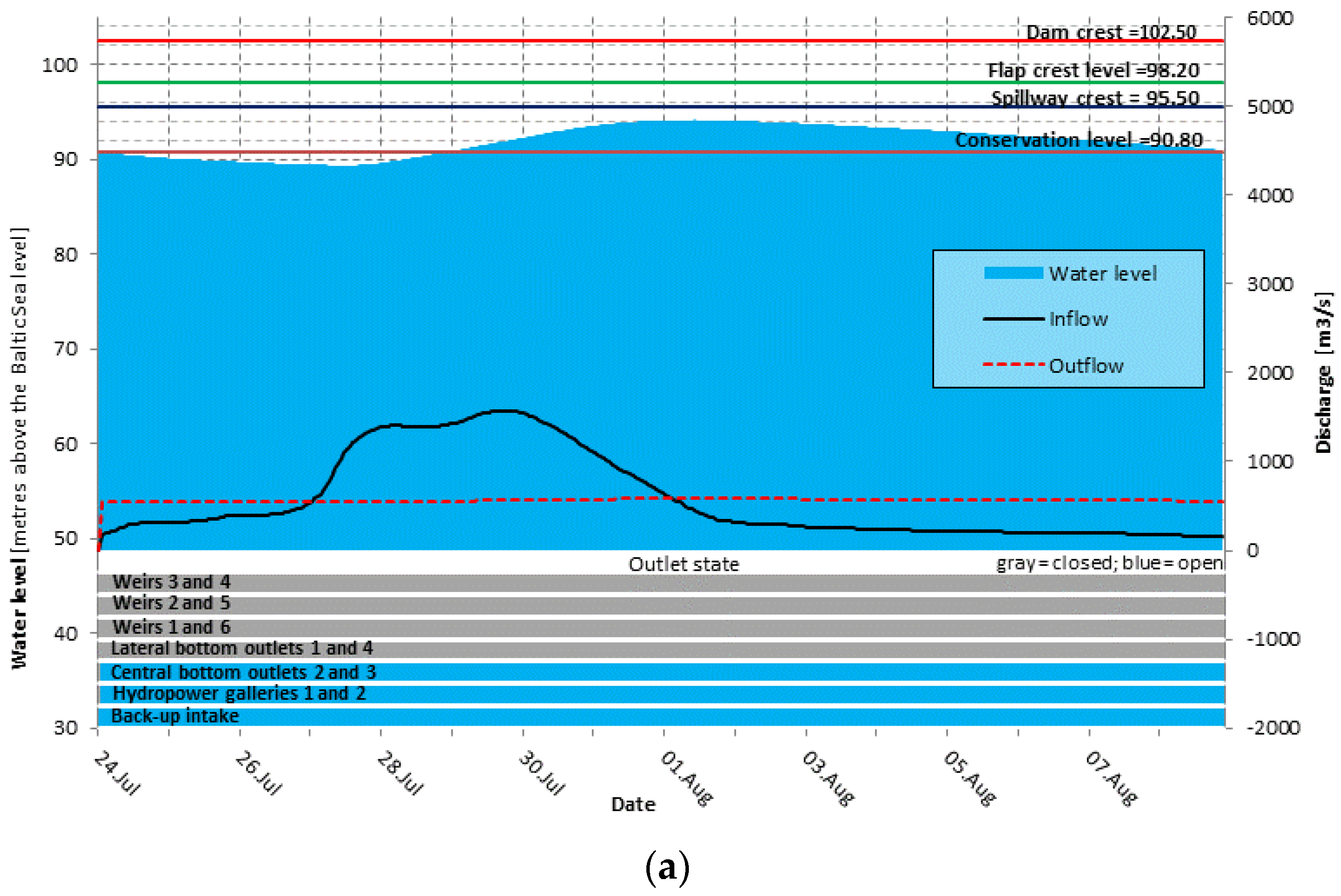
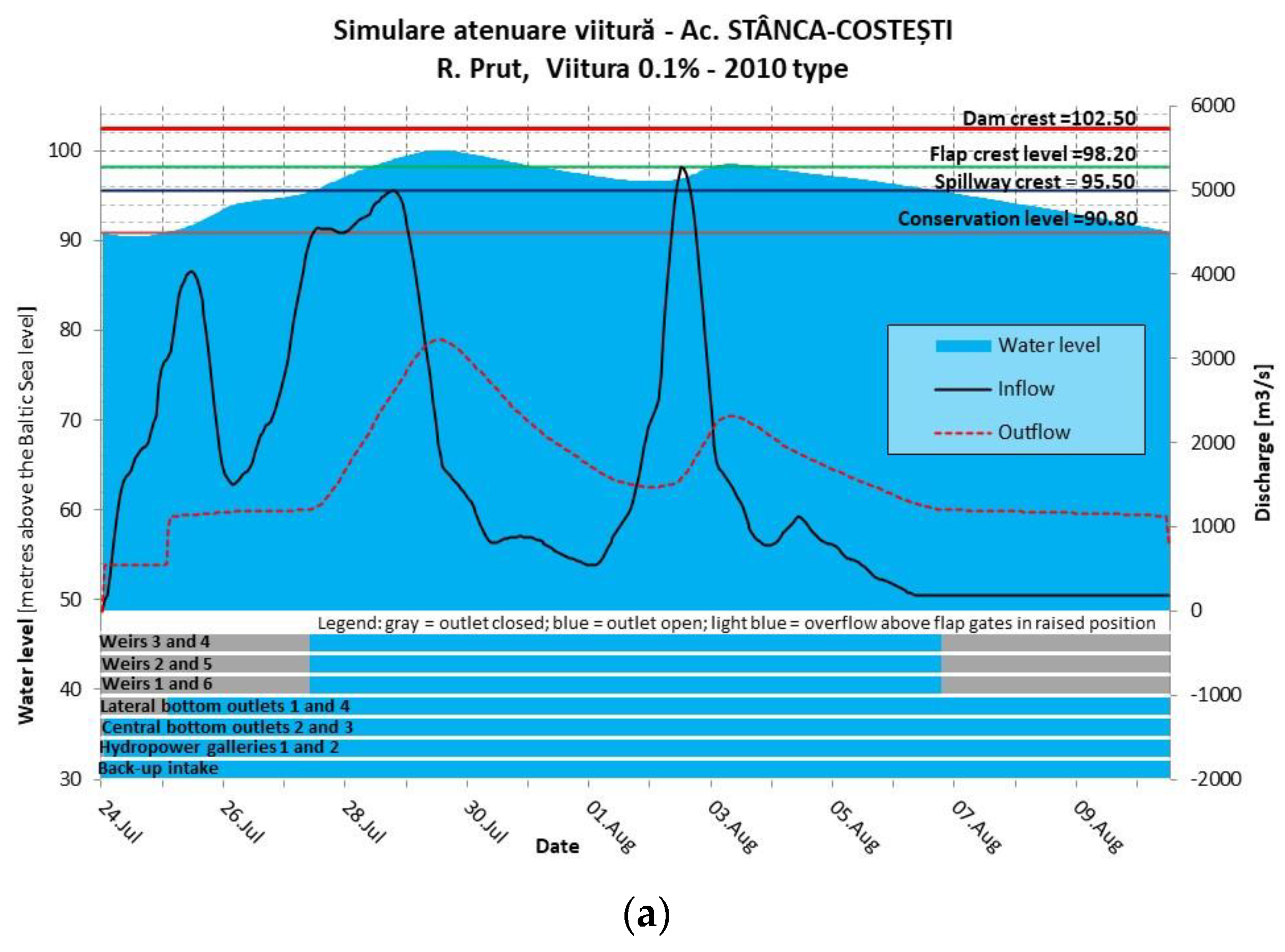
There have been major floods in the past 20 years. A remarkable flood occurred between 25 July and 2 August 2008, with a peak discharge of approximately 3300 m³/s (15% greater than the maximum discharge of the design flood during the project phase). The water level in the reservoir exceeded the upper limit of the flap gates (Figure 3). Other large floods occurred in 2005, 2010, and 2020, raising concerns about the design values of peak flow and especially the flood volume.

The flood that occurred from 22 to 28 July 2008 was caused by a significant amount of torrential rainfall, which fell primarily between 23 and 27 July 2008 in the upper parts of the Siret and Prut River basins. In Ukraine, where the upper course of the Prut River is located, precipitation levels ranged from 63 to 260 mm during this period. Maximum daily precipitation exceeded 120 mm in several areas, including Verhovina (186 mm), Putila (128 mm), and Kuti (133 mm). Abundant precipitation was also recorded in the tributary basins of the Prut River in the Republic of Moldova. The highest registered amount was 225 mm in Ocniţa, which is over ten times the ten-year average. A significant amount of precipitation was also observed at hydrometric stations in Romania; for instance, the Cotnari weather station recorded 203.2 mm in 24 h. The 2008 flood on Prut River has a historical character justified by the magnitude of the main flood parameters, such as peak flow and the volume.

On the Prut River near the city of Chernivtsi (Cernăuți) in Ukraine, the flood peaked at 7.9 m above the pre-flood water level. On 26 July 2008, a maximum flow of 3985 m³/s was recorded. At the Rădăuți Prut hydrometric station, the maximum flow rate recorded was 4240 m³/s (3300 m³/s after reassessment). The Prut River flood of 2008 began on July 24 at 6:00 p.m., when the flow at the Cernăuți gauge station suddenly increased to a value of 387 mc/s and the water level reached 314 cm, which was 186 cm above the Warning Level (WL). On the same day, at the Rădăuți–Prut station, at 8:00 p.m., a flow rate of 434 m³/s and a water level of 290 cm were recorded, triggering the Attention Level (AL). The Stânca–Costești reservoir, at that time, recorded a low volume of water due to a prolonged drought. The Danger Level (DL) at the Rădăuți–Prut gauge station, set at 600 cm above the river’s reference level, started on 26 July 2008, at 10:00 p.m. and lasted until 30 July 2008, at 6:00 p.m. The highest water levels were recorded on 28 July 2008, between 9:00 p.m. and 12:00 p.m., when the water level reached 1100 cm (11 m)

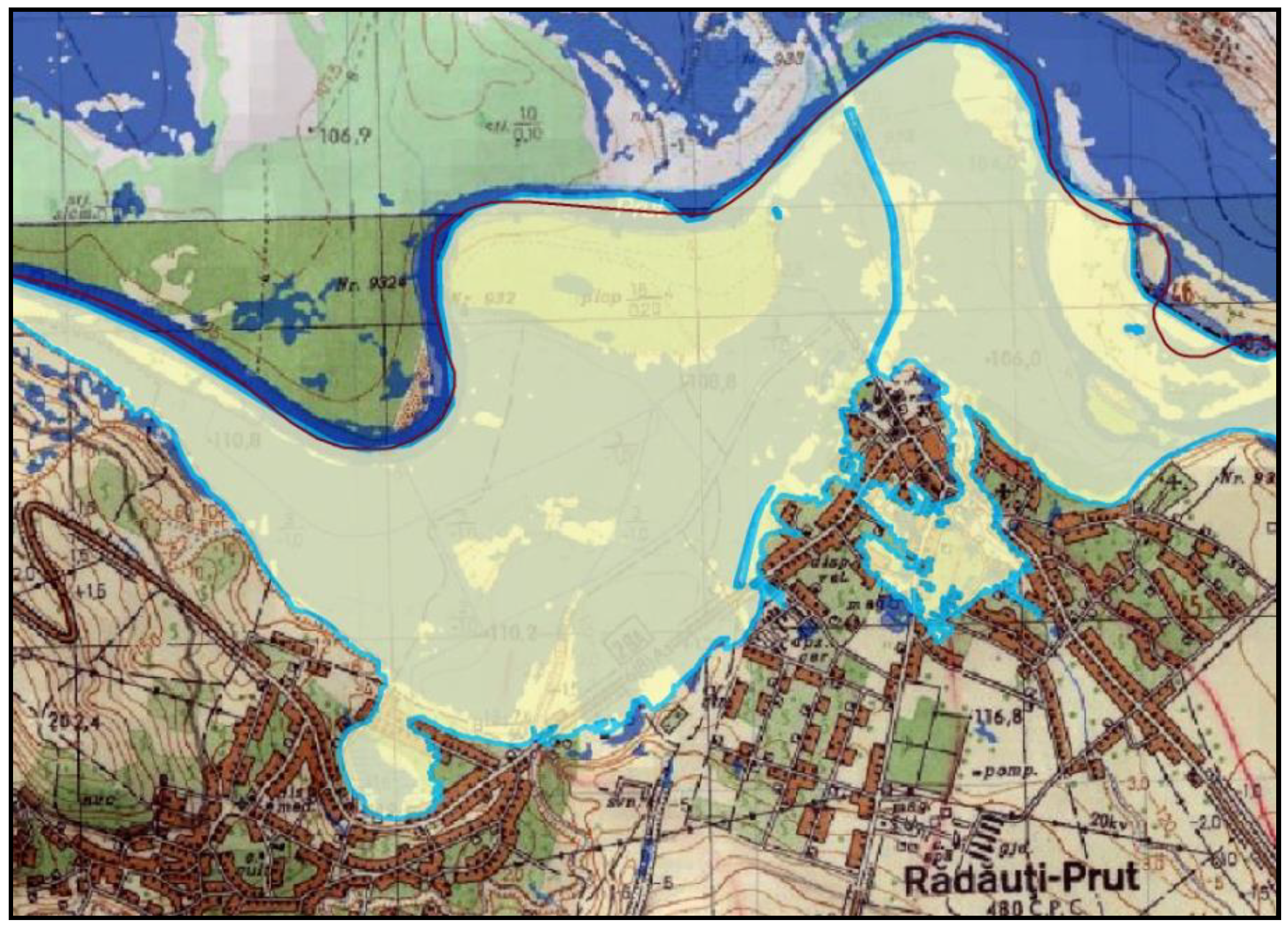
The most affected area was the town of Rădăuți–Prut, located upstream of the Stânca–Costești Reservoir (Figure 4). The flood had significant consequences, resulting in the total collapse of 127 houses. Additionally, 52 houses were more than 50% damaged, 62 houses were slightly damaged, 23 km of county roads were flooded, 4877 hectares of agricultural land were affected, and 11 riverbank protection works were destroyed, among other damages.

The Stânca–Costești reservoir played a significant role in flood mitigation. The water level in the reservoir remained high for approximately 20 days, due to the storage of a large volume of water and its gradual release downstream over an extended period. The floodings that occurred downstream of the Stânca–Costești reservoir are caused by the high discharges from the reservoir, exceeding the carrying capacity of the riverbed.

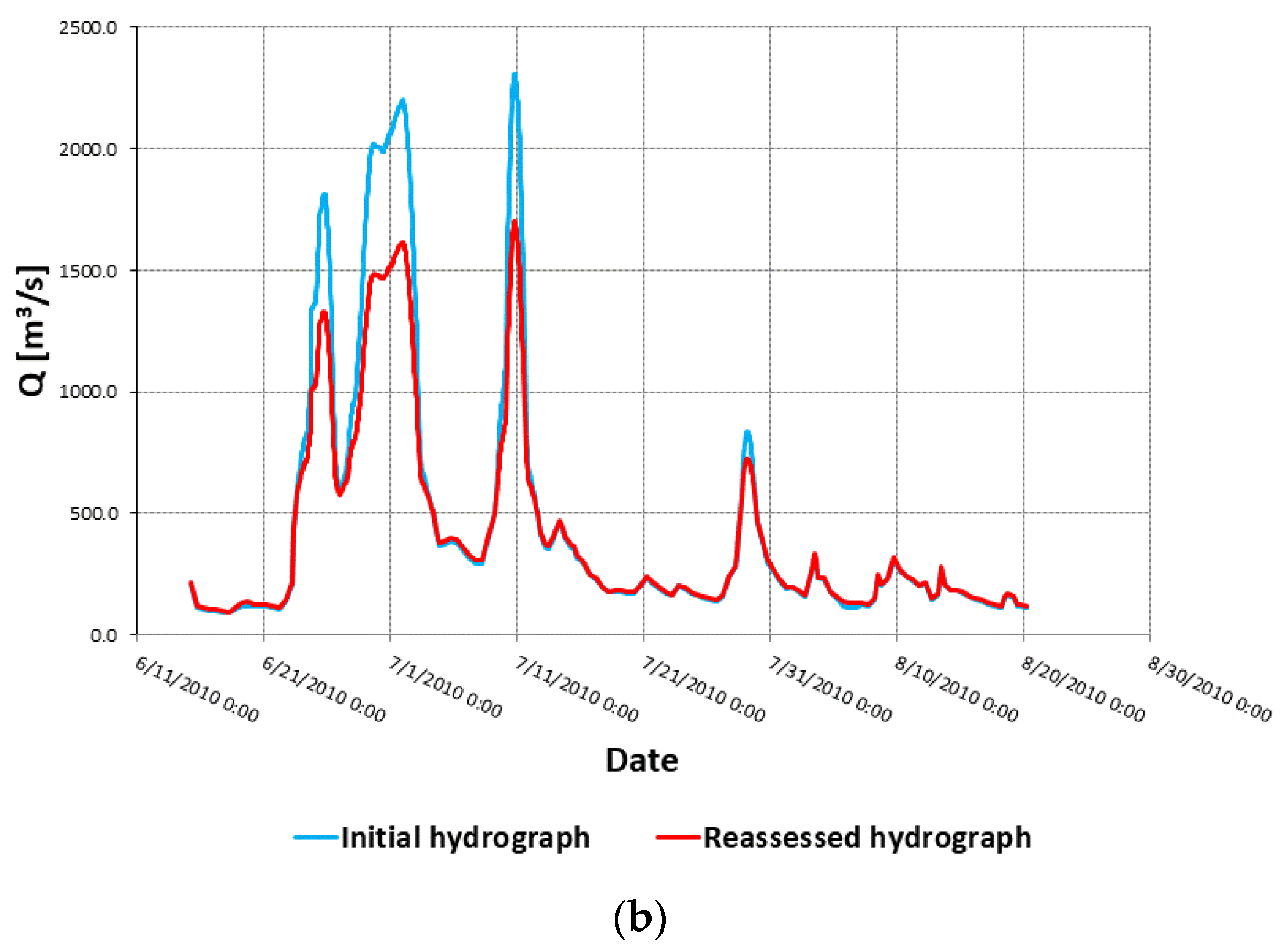
The floods that occurred in June and July 2010 affected both the Siret and Prut River basins. The common characteristic of the 2008 and 2010 floods was their transboundary nature. The 2010 floods were triggered by heavy rains caused by the unusual convergence of two weather fronts over the eastern Carpathian Mountains, including western Ukraine. In this region, large amounts of precipitation were recorded across three intervals: 21–24 June, 26–27 June, and 28 June–1 July. This led to consecutive floods occurring at short intervals, with high maximum flow values. During these periods, precipitation amounts ranged from 50 to 150 mm. Unlike the 2008 event, the 2010 flood mainly affected the middle part of the Prut River basin, including the Republic of Moldova, where a significant amount of precipitation fell. The precipitation recorded both in the upper basin of the Prut River in Ukraine and on the territory of Romania led to a significant water level increase, exceeding the Danger Levels in 3 successive intervals (25–26 June with a maximum flow of 1330 m³/s, 28 June–2 July with a maximum flow of 1560 m³/s, and 10–11 July with a maximum flow of 1705 m³/s). At the Rădăuți–Prut gauge station, the maximum flow recorded was lower than during the historical event of 2008, but the characteristic of this period was the formation of a multimodal flood with 3 consecutive peaks at short time intervals.

The large amounts of precipitation that fell in the upper part of the Prut River Basin favoured the occurrence of a historic volume of the flood upstream of the Stânca–Costești reservoir. Despite the progressive release of the large volume of water downstream of the dam, the unprotected areas of the lower Prut meadow were flooded. However, the damage recorded was significantly lower than in 2008.

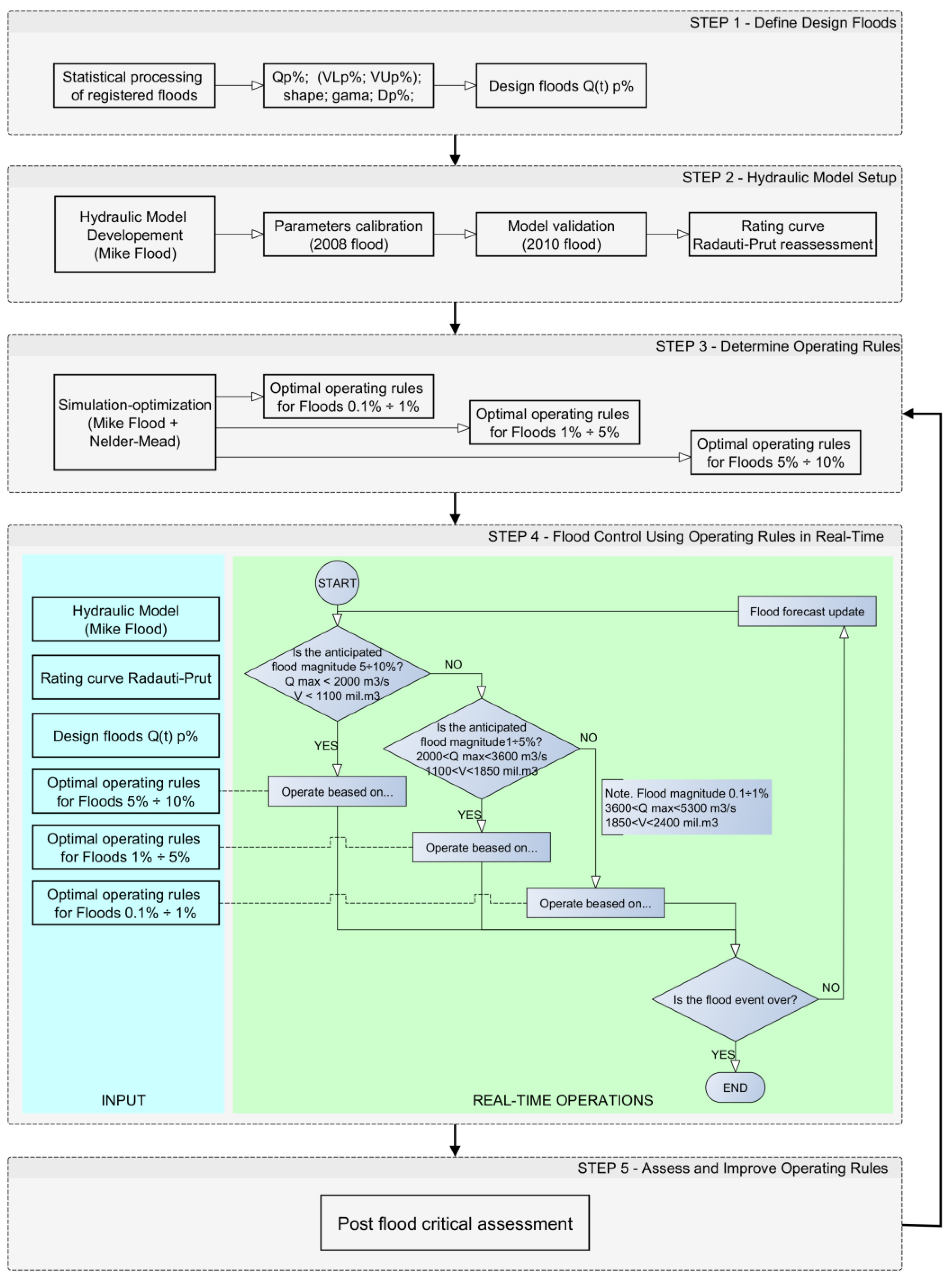
2.2. Flowchart of the Methodological Approach

The flow chart of the methodological approach is presented in Figure 5.

The main steps are as follows:

Step 1: Set up the hydraulic model and re-evaluate the Rădăuți–Prut rating curve.

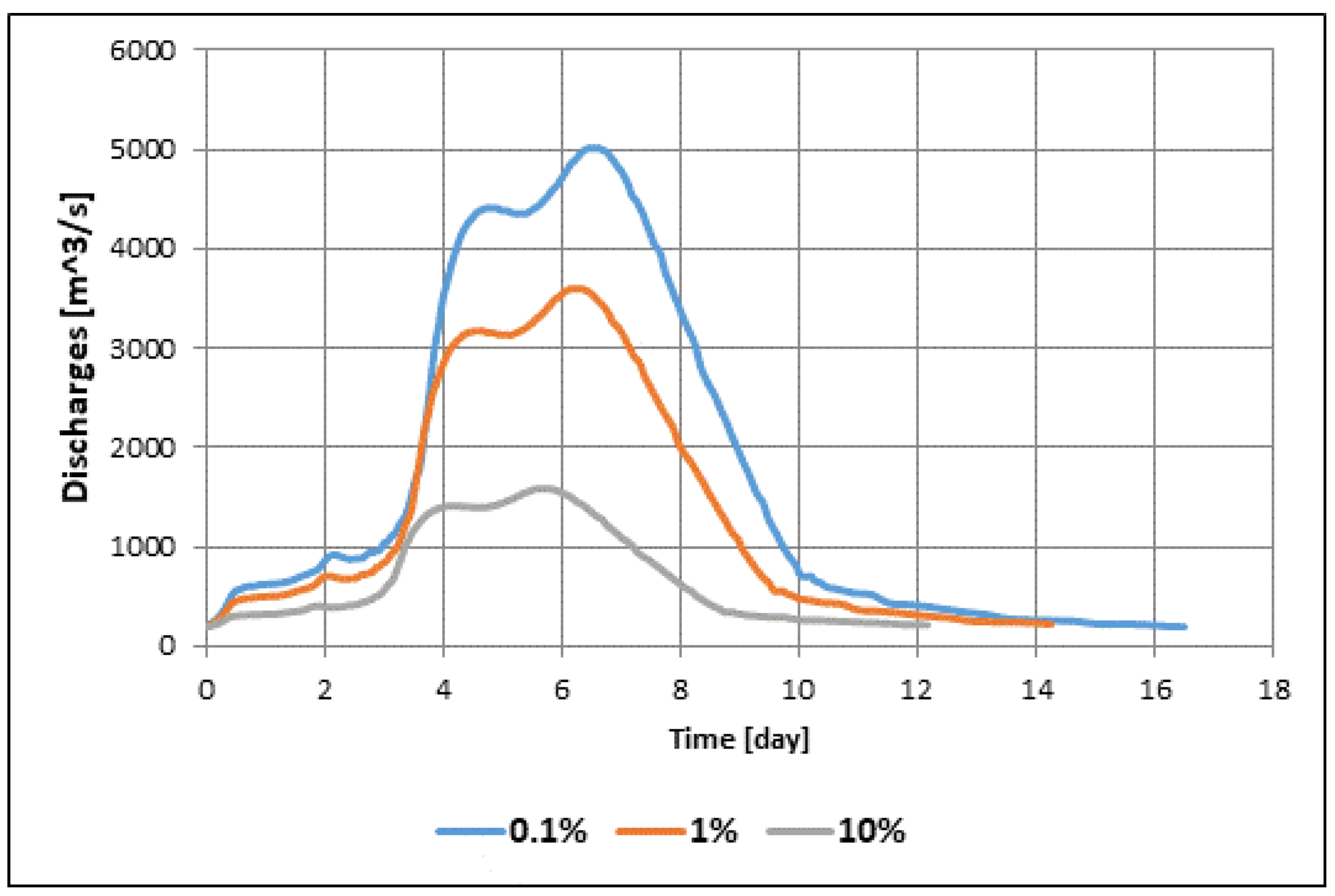
Step 2: Conduct statistical analysis of adjusted daily flows to develop design floods $Q{\left(t\right)}_{P\%}$, considering both maximum discharge $Q_{P\%}^{max}$ and volume $V_{P\%}$, realistic flood shape, and other parameters, exceeding the conventional synthetic flood design approach.

Step 3: Develop a simulation-optimization model to derive the operating rules for design floods, grouped by their common operating rules into 3 classes according to the exceedance probabilities: (5–10%), (1–5%), and (0.1–1%).

Step 4: Flood control using operating rules in real-time.

Step 5: Post-flood critical assessment.

For flood control, the following questions should be answered [55] (pp. 29–34): “What is the optimum size of flood release? What is the optimum duration of flood release? When should flood releases be made?”. The answers to all these questions are closely related to the design flood, defined not only by the maximum flow, flood volume, and duration, but also by its shape [28] which influences the synchronization of different manoeuvres. In fact, the full hydrograph is of interest in the case of dam design [53], as well as for flood control. 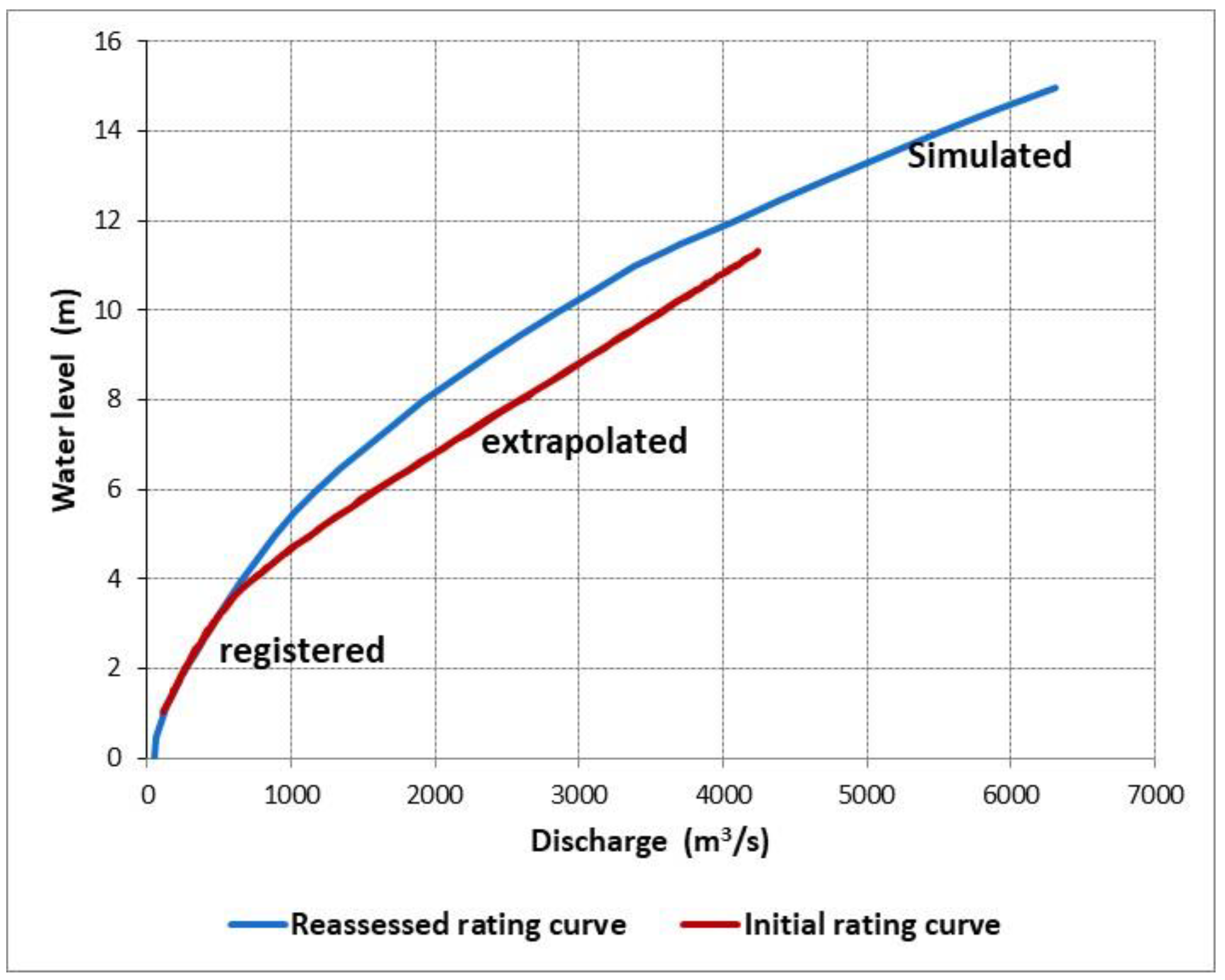Flow measurements at Rădăuți–Prut are only made up to 500 m³/s, while for higher flows the rating curve initially used was obtained by extrapolation. Further analysis of the rating curve was required due to differences in peak flow values provided by various sources for major floods. At the same time, significant differences were calculated between the volume of the flood at Rădăuți–Prut and Stânca–Costești. In order to solve these problems, it was necessary to create a hydraulic model on the Prut River between Rădăuți–Put and Stânca–Costești downstream of the stilling reservoir.

2.3. Hydraulic Model

The Mike Flood by DHI (release 2016 SP 3) software was used to set up a flood routing model between the Rădăuți–Prut gauge station and a section downstream of the Stânca–Costești dam. The calibration of the hydraulic parameters on this river reach took place simultaneously with the re-evaluation of the rating curve at Rădăuți–Prut based on an iterative process. To evaluate the calibration accuracy, the following information was considered: the time series of water level in the reservoir and the outflows from the reservoir, respectively, and the water balance between the two ends of the river reach. The simulations were performed using the same outlet operating schedule as during the registered flood events. The upstream boundary condition is the temporal series of water levels recorded at the Rădăuți–Prut gauge station, while the downstream boundary condition is the average slope of the Prut River downstream of the compensation reservoir.

The unsteady state routing model has the following characteristics:

• The length of the analysed sector, characterized by a high sinuosity, is 159.74 km. The river geometry is described by means of 79 cross-sections at variable partial distances (minimum 38 m, average 2079 m, and maximum 6155 m). These are supplemented by interpolation, finally resulting in 360 cross-sections (minimum distance 38 m, average value 442.6 m, and maximum value 498 m).
• Number of flow control structures (dam structures) = 13
• Number of cross sections in the reservoir = 35.

The purpose of the hydraulic simulations was to identify the most appropriate rating curve at the Rădăuți–Prut gauge station and calibrate the roughness coefficients on the analysed section. A comparison of the rating curves before and after simulations is shown in Figure 6. Following the calibration of the model parameters, the roughness coefficients have the following values: 0.032 for the riverbed and 0.085 for the floodplain. The water balance components for the 2008 and 2010 floods are shown in

Table 2. Water balance components for the 2008 flood.

Location	Time Span	Volume (mil. m3)
Rădăuți–Prut gauge station	24.07.900–30.08.1800	1164
Additional storage in the reservoir	25.07.900–31.08.1800	46
Stânca–Costești (downstream)	25.07.900–31.08.1800	1172

Water balance: (1172 + 46) − 1164 = 54 mil. m³.

and

Table 3. Water balance components for the 2010 flood.

Location	Time Span	Volume (mil. m3)
Rădăuți–Prut gauge station	23.06.600–30.08.1800	2334
Additional storage in the reservoir	24.06.600–31.08.1800	13
Stânca–Costești (downstream)	24.06.600–31.08.1800	2383

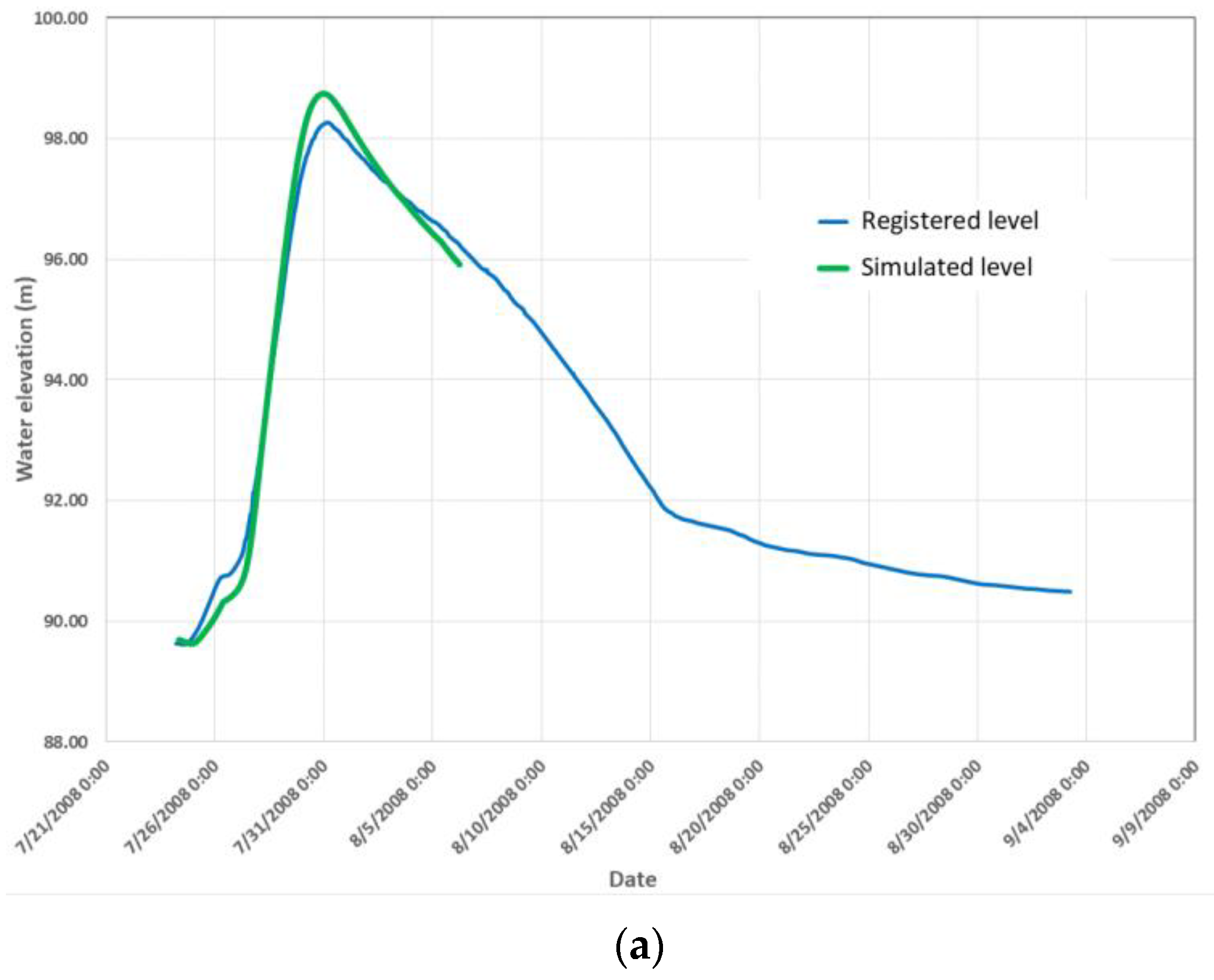
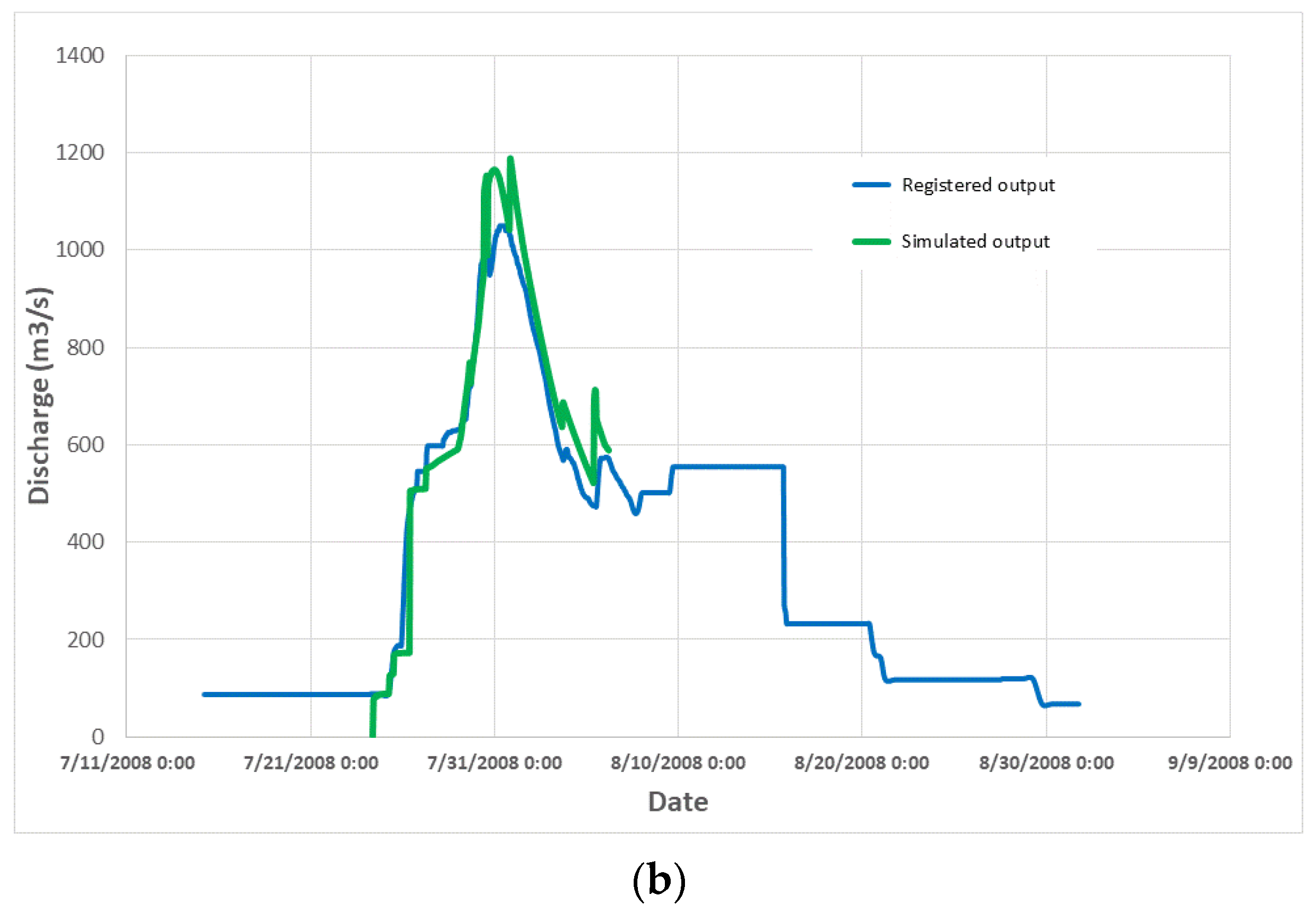
Water balance: (2383 + 13) − 2334 = 62 mil. m³.

, respectively. Hydrographs of the reservoir water levels and discharges downstream of the stilling basin during the 2008 flood are shown in Figure 7.

The difference between the output and input volumes can be explained by the contribution of the rest of the basin. The Rădăuți–Prut gauge station controls a basin of approximately 9000 km², while the upstream Stânca–Costești basin is about 12,000 km², resulting in a difference of approximately 3000 km². Small tributaries are present in this sector, but their contribution is not significant.

The operating rules used by the operators of the National Administration Romanian Waters (also known as ANAR) during the 2008 flood event are presented in Figure 7. A comparison, reflecting the good fit of the recorded and simulated values of water levels of the reservoir and outflow discharges can be followed in Figure 8. As a result, the calibration and validation of the rating curve from Rădăuți–Prut, along with the roughness coefficients on the river reach between the gauge station and the dam, can be considered completed.

2.4. Statistical Background

According to Romanian legislation, the dams are classified into five classes of importance based on various characteristics. The dams in the first category of importance are designed for a 0.1% AEP of the maximum discharge and/or flood volume during normal operating conditions (the design flood) and a 0.01% AEP under special operating conditions corresponding to more severe floods (the check flood). In the dams in the second category of importance, AEPs are 1% and 0.1%, respectively. Finally, for the fifth category of importance, corresponding to temporary hydraulic works, the following AEPs are considered: 10% and 3%, respectively [56] (pp. 1–2).

A design flood cannot be defined simultaneously by the same probability of exceedance $P\%$ of peak discharge $Q_{P\%}^{max}$ and flood volume $V_{P\%}$ [57] (pp. 174–194). The hydrograph of such a flood would represent the “maximum possible hydrograph $P\%$” [57]. Consequently, the design flood $P\%$ should be characterized by lower values at least for one of the parameters $(Q_{P\%}^{max}$ or $V_{P\%})$, or even for both.

Bivariate analysis [58] (pp. 165–166), [59] and copula-based bivariate flood frequency analysis [46] are widely used to obtain the quantiles of the pair ${\left(Q^{max}; V\right)}_{P\%}$ corresponding to the flood hydrograph $P\%$. Unlike univariate analysis, where a single design quantile is obtained for the maximum discharge for the probability of exceedance $P\%$ and for the flood volume, respectively, the solution in bivariate analysis is located on the isoline $P\%$ in the $(Q^{max}; V)$ coordinates [43,60,61,62]. That means that all points ${(Q^{max}, V)}_{P\%}$ and corresponding set of pairs (maximum flow; flood volume) located on the contour line $P\%$ are characterized by the same probability of exceedance $P\%$. A further step leading to a more complex analysis was to link peak flow, volume, and duration in a trivariate statistical approach [63].

In the bivariate analysis, the four different joint probability distribution functions of special interest include the following two [43,45,64]:

[a] simultaneous exceedance: $P\%=Pr \left(Q^{max}\ge q \mathrm{AND} V\ge v\right)$

[b] simultaneous non-exceedance: either the peak flow or the flood volume (only one of them) is equalled or exceeded: $P\%=Pr \left(Q^{max}\ge q \mathrm{OR} V\ge v\right)$

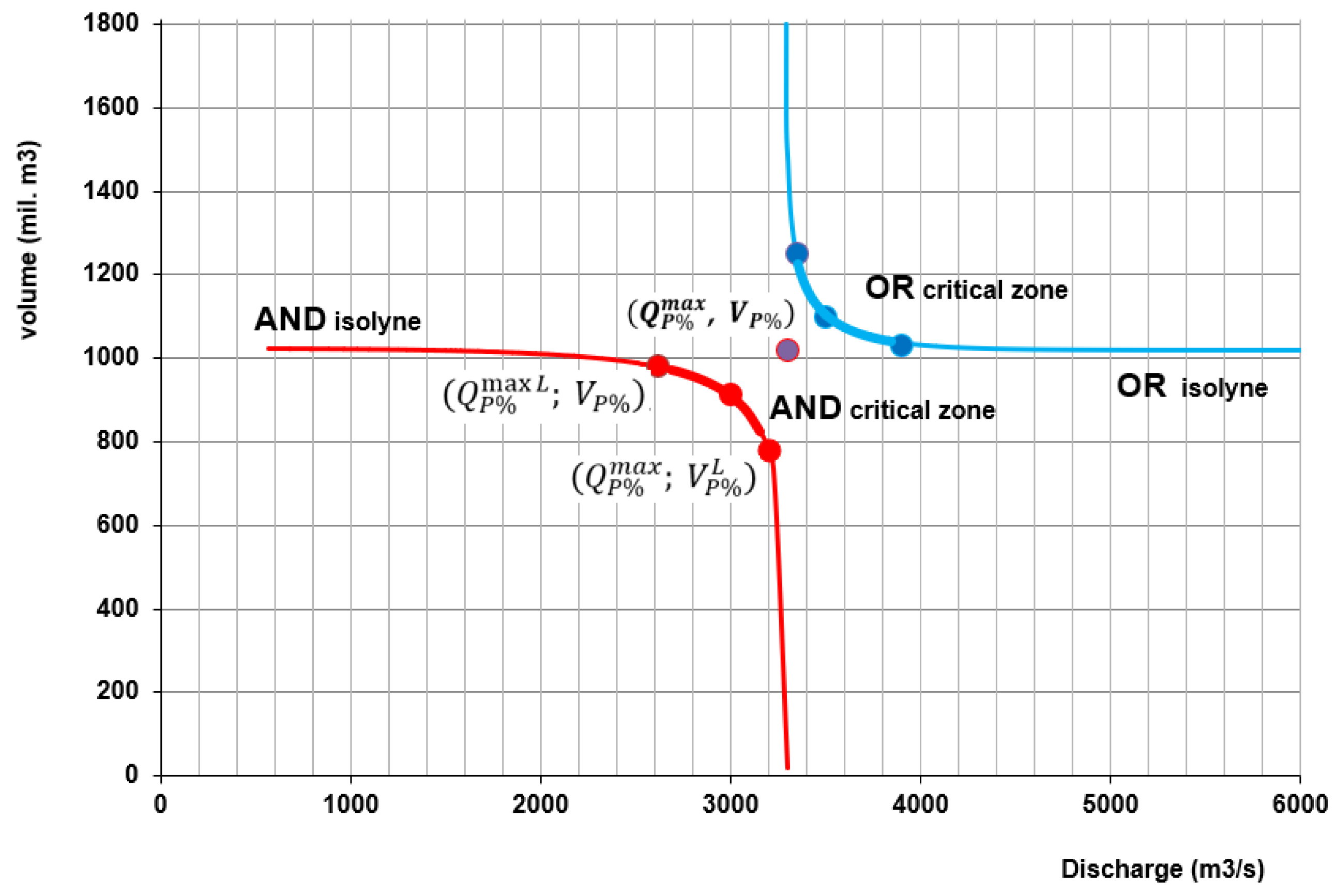
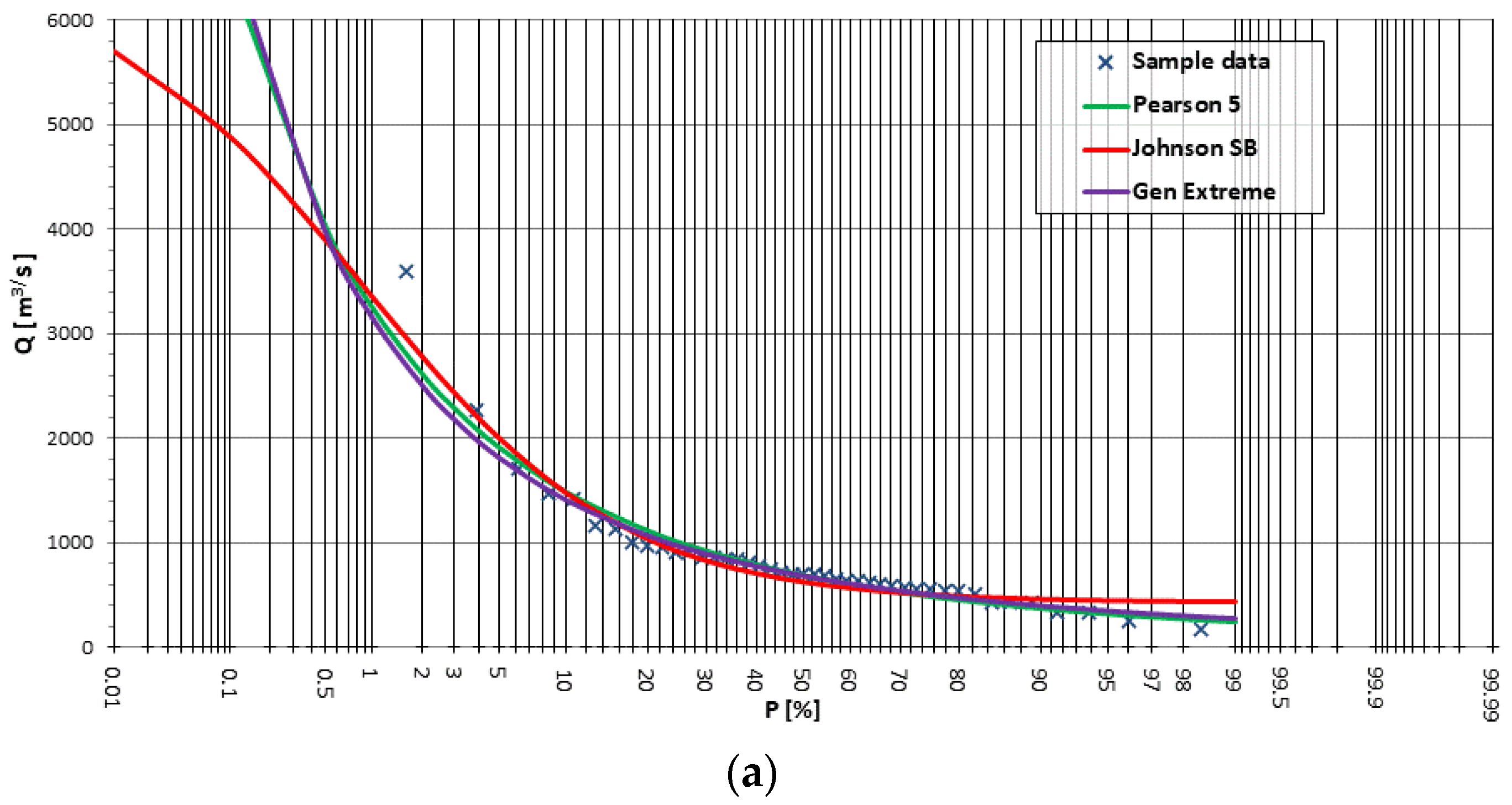
In the first case, all possible sets of pairs ${\left(Q^{max}, V\right)}_{P\%}$ belong to the joint AND isoline, while in the latter corresponds to a joint OR isoline. Figure 9 shows an example of the AND (red line) and OR (blue line) isolines for $P\%$ probability of exceedance. Accepting that the flood characterized by ($Q_{P\%}^{max};$ $V_{P\%}$) corresponds to the maximum possible flood hydrograph $P\%$ [57], still considered unlikely, the AND approach is much more credible in defining the parameters of the design flood [40]. In this way, lower values than $Q_{P\%}^{max}$ and $V_{P\%}$ or equal to one of them are obtained for maximum discharge or flood volume of the design flood. At the same time, it should be noticed that the OR isolines provide higher design quantiles than the AND contour lines for a given probability of exceedance [43]. OR quantiles (either maximum discharge or flood volume) could be critical if, according to the operation rules in the long term, the conservation level is at the spillway crest level. Otherwise, for lower conservation levels, the AND approach is recommended.

The isoline $P\%$ can be divided into a proper (central) and a naïve (tail) part [64]. The tail part is defined by the intersection of the contour line $P\%$ with the lines of the equation $Q=Q_{P\%}^{max}$ and $V=V_{P\%}.$ The intersection points are $(Q_{P\%}^{max}; V_{P\%}^L)$ and $(Q_{P\%}^{\max L}; V_{P\%})$, which at the same time represent the limits of the critical zone (Figure 9). The index L (abbreviation from “lower”) in the notations $V_{P\%}^L$ and $Q_{P\%}^{\max L}$ indicates the lower values of the volume and the peak discharge, respectively, in the central zone. Corresponding to the central part, the peak discharge takes values in the range $\left(Q_{P\%}^{\max L}, Q_{P\%}^{max}\right)$ and the volume can be in the range $\left(V_{P\%}^L, V_{P\%}\right)$.

Each pair ${(Q^{max}, V)}_{P\%}$ located in the central part of the $P\%$ AND isoline represents a combination of both peak discharge and flood volume corresponding to the joint probability of exceedance $P\%$. Therefore, there is not a single $P\%$ synthetic hydrograph, but an infinite number of such flood hydrographs. From this infinity, those hydrographs corresponding to the most unfavourable combinations of maximum discharge and volume must be chosen. Either a single design realization [65] or a limited subset belonging to the set of critical combinations [61] can be selected for design purposes. The extreme limits of the critical zone: $(Q_{P\%}^{max}; V_{P\%}^L)$ and $(Q_{P\%}^{\max L}; V_{P\%})$ are leading to the maximum discharge of flood $P\%$ (acronym MDF) and maximum volume flood $P\%$, respectively (acronym MVF) [66]. The univariate variables $V_{P\%}^L$ and $Q_{P\%}^{\max L}$ in the copula approach can be replaced by the lower limit of the confidence interval of the survival function for flood volumes and peak flows, respectively [66]. Usually, the dimensioning of spillways is based on the MDF flood, while for the flood protection volume the MVF flood is necessary. However, depending on the conservation water level in the reservoir and dam characteristics, both MDF and MVF could be necessary for sizing spillways and flood protection volume.

An important index for the characterization of the flood hydrograph is the compactness coefficient $\gamma$ [67,68], which incorporates all the parameters of the flood wave:

$$\gamma ={\displaystyle \frac{V}{D \left(Q^{max}-Q_b\right) }}$$

(1)

where $Q_b$ is baseflow, $V$—volume above baseflow, $D$—flood duration, and $Q^{max}$ is peak flow.

In addition to maximum discharge and volume, the design flood is also defined by duration and shape. The shape of the design flood can be obtained by normalizing the registered floods, grouping them into classes of similar shape, calculating the average normalized flood in each class and then scaling it with the maximum discharge $Q_{P\%}^{max}$ and the corresponding duration [66]. An alternative to obtaining design floods is to scale extreme floods that have occurred in the past, thus preserving their shape.

2.5. Flood Control Model

Once the design flood for various AEPs is determined, the next step is flood mitigation modelling. Over time, in addition to simulation models, many flood control optimization models have been developed for single reservoirs or reservoir systems. Early algorithms and methods used for flood mitigation included linear programming, dynamic programming, and nonlinear programming [69]. Later, novel approaches were adopted, such as evolutionary algorithms or machine learning. Among the many works related to flood control, models of linear programming [70,71], stochastic dynamic programming [72], nonlinear programming [73], evolutionary algorithms [74,75,76], and machine learning [77] are just a few examples of optimization models.

The real-time operation of the dam outlets during the flood period is affected not only by the inflow uncertainties [78], but also by the outflow uncertainties as well as the non-updated storage capacity of the reservoir [79]. A special case of uncertainty is related to the blocking of outlet equipment, which requires real-time adaptation to unforeseen situations.

The operating rules to be derived need to be a reasonable trade-off between dam safety, flood control, and operability [80]. In many countries, flood control is based on fixed predefined operating rules without considering inflow forecasts [80]. They are usually obtained through simulation techniques during the planning stage [81]. Operating rules are presented as graphs or tables to guide reservoir release [69], making them easy for system operators to use [26,81]. Predefined operating rules can be the result of trial-and-error simulations, optimization models, or mixed simulation-optimization approaches. The simulation, based on the continuity equation in the reservoir, describes the evolution of state variables and outputs under the forcing variables (input hydrograph and outlets manoeuvres). The initial condition of the model is the water level in both the reservoir and river sectors (obtained from previous simulations using the average discharge). The boundary conditions are the flood hydrograph at the nearest upstream gauge station, and the rating curve at the downstream end of the modelled sector. The flood operation is mathematically represented as a multi-objective control problem, characterized by several objective functions aimed at minimizing downstream flooding, the danger of overtopping the dam crest, the number of gate operations, unproductive discharges, and energy losses [80,82].

As previously mentioned, release decisions are generally made based on reservoir water levels only, without flood forecasts. Consequently, during real-time flood control, dam managers face uncertainties regarding the magnitude of the floods that occur. The real-time flood operation becomes more realistic when considering the short-term forecasts. A sequential approach combines at each stage a flood-routing simulation with a nonlinear programming model [73]. Based on repeatedly updated flood forecasts, new simulation-optimizations are considered during a flood event. In this way, flood control is represented by a series of successive deterministic optimization problems [83]. The sequential simulation-optimization leads to the best state variables (water level and volume in the reservoir) and outflow trajectories (downstream discharge) for each stage of optimization. However, the obtained results are less favourable than in the case of perfect knowledge from the beginning of the entire flood hydrograph. An alternative to repetitive simulation-optimization is assimilating the predicted flood at each stage with the closest standard design flood (10%, 1%, 0.1%, or 0.01% AEP) and adopting the corresponding operating rules up to the next forecast.

The reservoir flood control model developed in this work is based on scheduling the activation or deactivation of bottom outlets and weir gates [84,85,86], depending on the water level in the reservoir and the short-term flood forecast. The aim is to derive the operating rules for the outflow devices and reduce the downstream outflows as much as possible while limiting the maximum water level in the reservoir. The developed nonlinear simulation-optimization model uses the Nelder–Mead algorithm, which does not require the evaluation of the derivatives of the objective function.

The continuity equation in the reservoir is:

$$Q\left(t\right)-q\left(t\right)={\displaystyle \frac{dV\left(t\right)}{dt}}$$

(2)

where $Q\left(t\right)$ is the inflow, $q\left(t\right)$—the outflow and $V\left(t\right)$—the volume of water in the reservoir at time $t$. The outflow $q\left(t\right)$ can be expressed as follows [75] (pp. 249–253):

$$q\left(t\right)={{\displaystyle \sum }}_{j=1}^N{s}_j\left(H\left(t\right)\right) q_j\left(H\left(t\right)\right)$$

(3)

where:

$N$ is the number of equivalence classes in which the outflow devices are grouped.

$j=\overline{1,N}$—current number of the equivalence class.

$q_j\left(H\right)$—outflow of a single outlet belonging to class j.

$s_j\left(H\right)$—number of active outflow devices in class j.

H = H(t)—water level in the reservoir.

The same type of outlet may belong to different equivalence classes depending on its operating regime. All outlets in class $j$ have the same operating rules. If class j is not active, $s_j=0$.

Combining Equations (2) and (3) yields:

$$Q\left(t\right)-{\displaystyle \sum }_{j=1}^N{s}_j\left(t\right) q_j\left(t\right)={\displaystyle \frac{dV\left(t\right)}{dt}}$$

(4)

Outlets belonging to class $j$ are active in the range of levels $\left(H_j^{\prime } ; H_j^{″}\right)$. Depending on the operating rules, outlets are classified into the following two types (Figure 10):

(a) First type outlets: at the level $H_j^{\prime }$, the gates are opened to allow water release, while at the level $H_j^{″}$ (which can be lower, equal, or higher than $H_j^{\prime }$), the gates are closed. The maximum level of water in the reservoir always exceeds $H_j^{″}.$

(b) Second type outlets: $H_j^{\prime }$ and $H_j^{″}$ have the same meaning during resevoir filling. The outlet device is closed before the maximum water level in the reservoir is reached and is opened again during the emptying phase when the water level decreses below $H_j^{″}$.

After discretization, relation (4) is written as follows:

$${\displaystyle \frac{Q_{i-1}+Q_i}{2}}-{\displaystyle \sum }_{j=1}^N{\displaystyle \frac{s_{i-1,j}\cdot q_{i-1,j}+s_{i,j}\cdot q_{i,j}}{2}}={\displaystyle \frac{V_i-V_{i-1}}{\mathit{\Delta }t}}$$

(5)

After rearranging the terms, this becomes:

$$V_i=V_{i-1}+\left({\displaystyle \frac{Q_{i-1}+Q_i}{2}}-{\displaystyle \sum }_{j=1}^N{\displaystyle \frac{s_{i-1,j}\cdot q_{i-1,j}+s_{i,j}\cdot q_{i,j}}{2}}\right) \mathit{\Delta }t$$

(6)

where $i=\overline{1,n}$ represents the current value of the variables and $j=\overline{1,N}$ is the equivalence class; $s_{i,j}=s_j\left(H_i\right)$; $H_i=H\left(t=i\mathit{\Delta }t\right)$; $\mathit{\Delta }t$ is the time step.

In a simulation model, the decision variables $H_j^{\prime }$ and $H_j^{″}$ are proposed by the water manager. Through successive iterations, the volumes $\{ V_i \}$, the downstream discharge $\left\{ q_i=\sum _{j=1}^N{q}_{i,j} \right\}$ and the water levels in the reservoir $\{ H_i \}$ are obtained.

In a simulation-optimization approach, in addition to the dynamic Equation (6), constraints and the objective function(s) are added. The downstream hydrograph should have an almost rectangular shape, with the maximum flow value limited to $q_{adm}$ which represents the carrying capacity of the river channel. Since the flood wave must pass through the reservoir in the shortest possible time, the outlet discharges must be as close as possible to the $q_{adm}$ value. Therefore, the objective function is:

$$\left(min\right) Z ={\sum }_{i=1}^n{\left({\sum }_{j=1}^N{s}_{i,j}{q}_{i,j}-q_{adm}\right)}^2$$

(7)

The following constraints are added:

(a)

The water height $h_i$ above spillway elevation $H_s$ is limited to the maximum value $h_{adm}$ established in the design phase.

$$h_i=H_i-H_s<h_{adm}; i=\overline{1,n}$$

(8)

(b)

Successive outflow values $q_{i-1}$ and $q_i$ must be as close as possible to avoid significant variation of downstream discharges.

$$\left|q_i-q_{i-1}\right|<\mathit{\Delta }{q}_{adm}; i=\overline{1,n}$$

(9)

where $\mathit{\Delta }{q}_{adm}$ is the maximum acceptable difference between two successive outlet discharges from the reservoir. This constraint also ensures a minimum number of manoeuvres of the outlet equipment during the flood event.

(c)

The end-of-simulation volume $V_N$ must be close to the prescribed volume $V_f$ to accommodate a new flood wave or to increase the resources available to water users.

$$\left|V_N-V_f\right|<\mathit{\Delta }{V}_{adm}$$

(10)

where $\mathit{\Delta }{V}_{adm}$ is the maximum acceptable difference between the volume at the end of the simulation and the prescribed volume.

However, the last constraint can be removed, the decision regarding the volume kept in the reservoir in the post-flood period being at the discretion of the water managers.

These constraints are transformed into partial objective functions, being then added to the function (7):

$$\begin{gathered} \left(min\right) Z ={\sum }_{i=1}^n{\left({\sum }_{j=1}^N{s}_{i,j}{q}_{i,j}-q_{adm}\right)}^2+w_1 {\sum }_{i=1}^n{\left(h_i-h_{adm} \right)}^2 \\ +w_2 {\sum }_{i=1}^n{\left(q_i-q_{i-1} \right)}^2 +w_3 {\left(V_N-V_f \right)}^2 \end{gathered}$$

(11)

where $w_1$, $w_2$, and $w_3$ are weighting factors expressing penalties associated with deviations from the constraints.

The optimal control model consists of the objective function (11) and the dynamic Equation (6). The model unknowns are the 2$n$ decision variables $H_j^{\prime }$ and $H_j^{″}$, representing the lower and upper limits of the operating range for each class of outlet devices. Other constraints can be added, such as limiting the water curtain overflowing the weir flap gates in the raised position, setting a maximum gradient of water level increase or decrease expressed in m/day, etc.

3. Results

3.1. Design Flood

According to national regulations, the operating rules of the reservoirs are revised every 10 years. Following major floods in 2005, 2008, 2010, and 2020, a new statistical analysis was deemed necessary. On this occasion, the rating curve at the Rădăuți–Prut gauge station was reassessed.

3.1.1. Univariate Statistical Analysis

Based on the revised rating curve, all recorded floods have been reassessed. Floods up to 800 m³/s are identical regardless of the rating curve. The differences start to be visible up to 1000 m³/s, and are important above this value. Only six recorded floods have maximum flow rates higher than 1000 m³/s, but their influence on the statistical parameters is important. Figure 11 shows the reassessed discharges for two of the most important floods that occurred during the analysed period.

(a)

Analysis of maximum discharges

The statistical processing was carried out for the period 1978–2020, i.e., for 43 years. The calculation of peak flow quantiles corresponding to different AEPs was carried out by both the BM method and the POT method.

The unknown theoretical distribution of the population is estimated by several distributions that satisfactorily fit the empirical values according to different statistical criteria (Kolmogorov–Smirnov, Anderson–Darling, etc.). The range of extreme values for the accepted distributions represents “uncertainty bounds” [87] or “uncertainty interval” [68]. This interval highlights epistemic uncertainty and is different from the confidence interval, which reflects the aleatory uncertainty associated with a chosen distribution function.

The selected distributions for maximum discharges can be examined comparatively in Figure 12. They model well the empirical values for medium or high AEPs but differ strongly for low probabilities. Thus, for 0.1% AEP, the maximum discharges have values between 4878 m³/s and 7806 m³/s, i.e., a difference of more than 37%. For 1% AEP, the $Q_{1\%}^{max}$ discharges are in a range of (3160–3720) m³/s. The maximum discharges $Q_{P\%}^{max}$ obtained by the BM and POT methods are shown in

Table 4. Maximum discharges $Q_{P\%}^{max}$.

${\mathit{Q}}_{\mathit{P}\mathit{\%}}^{\mathit{m}\mathit{a}\mathit{x}}$ (BM)					${\mathit{Q}}_{\mathit{P}\mathit{\%}}^{\mathit{m}\mathit{a}\mathit{x}}$ (POT)
P%	Johnson SB	Pearson5	Gen Logistic	Gen Extreme	P%	Inv Gaussian	Johnson SB	Pareto
0.1	4878	6468	7518	6671	0.1	5691	4998	7806
0.5	3887	4032	4129	3971	0.5	3943	4268	4243
1	3352	3261	3199	3160	1	3258	3719	3263
2	2774	2616	2484	2503	2	2627	3025	2509
3	2430	2289	2143	2178	3	2287	2581	2152
5	2007	1921	1779	1820	5	1894	2029	1773
10	1479	1489	1379	1411	10	1433	1394	1364
20	1039	1119	1056	1070	20	1065	967	1049

.

The Johnson SB distribution (BM approach) was chosen as the best fit for matching the empirical distribution. It shows a flattening tendency of the maximum discharges for low exceedance probabilities, which would correspond to reaching the maximum hydrological potential of the basin.

Figure 13 shows the Johnson SB distribution as well as its confidence interval. Corresponding discharges can be seen in

Table 5. Maximum flow rates $Q_{P\%}^{max}$ (Johnson SB) and confidence limits.

$\mathit{P}\mathit{\%}$	${\mathit{Q}}_{\mathit{P}\mathit{\%}}^{\mathit{m}\mathit{a}\mathit{x}}$(m3/s)	Lower Limit (m3/s)	Upper Limit (m3/s)	Climate Change Value (m3/s)
0.10%	4878	4272	5715	5300
1%	3352	2946	3901	3600
10%	1479	1262	1732	1575

, along with climate change values. The maximum discharges considered in the design stage had lower values, which justifies the update of the operating rule during flood events. Thus: $Q_{1\%}^{\max design}$ = 2800 m³/s and $Q_{0.1\%}^{\max check}$ = 4300 m³/s, both lower compared to the values in Table 5.

(b)

Analysis of flood volumes

Statistical processing of flood volumes was performed using the POT (Peak Over Threshold) method. A first threshold $Q_{thr1}$ is chosen for flood selection, while a second threshold $Q_{thr2}$ is proposed for the flood volume calculation (Figure 14). If the value of $Q_{thr1}$ is chosen appropriately, the number of selected floods is practically equal to the number of years in the analysed period, and the empirical probability of exceedance associated with each measured value can be interpreted as an AEP [88] (pp. 4–26). The total flood volume [66,89] consists of the volume above the lower threshold $Q_{thr2}$ plus the volume below this threshold corresponding to the period for which the flows of the Prut River exceed $Q_{thr2}$ value (Figure 14).

By trial and error, the following values were chosen: the upper threshold $Q_{thr1}$ = 400 m³/s, and the lower threshold $Q_{thr2}$ = 190 m³/s. The $V_{P\%}$ values considering the uncertainty bounds are shown in

Table 6. Flood volumes $V_{P\%}$ (mil. m³).

P%	Fatigue Life	Inv Gaussian	Johnson SB
0.1	2661	3410	2158
0.5	1956	2323	1920
1	1659	1893	1744
2	1368	1491	1508
3	1202	1273	1341
5	997	1017	1106
10	730	709	770

, while the graphical representations of these distributions can be examined comparatively in Figure 15. It is also found that the selected distributions fit well the empirical values for high probabilities but are strongly divergent for low probabilities. For example, for 0.1% AEP the volumes are in the range (2158–3410) mil. m³, i.e., a difference of over 37%.

As with maximum flows, the Johnson SB (BM) distribution was chosen due to its flattening tendency for low probabilities. Figure 16 shows the plot of the Johnson SB distribution as well as its confidence limits. Corresponding values of flood volumes are provided in

Table 7. Flood volumes $V_{P\%}$ (Johnson SB) and confidence limits.

$\mathit{P}\mathit{\%}$	${\mathit{V}}_{\mathit{P}\mathit{\%}}$ (Mil. m3)	Lower Limit (Mil. m3)	Upper Limit (Mil. m3)	Climate Change Value (Mil. m3)
0.10%	2158	1826	2642	2400
1%	1744	1473	2132	1850
10%	770	613	963	770

. The $V_{P\%}$ values characterize the current hydrological regime, while the values in the last column in Table 7 were adopted for the Climate Change (CC) scenario.

3.1.2. Shape of the Design Flood

An ensemble of design flood hydrographs of different shapes was previously obtained for the Rădăuți–Prut gauge station [66]. The shape of the flood has an important influence on the optimal operating rules, especially in the case of extraordinary floods. The worst situation was registered during the 2008 flood, which took place between 25 July and 2 August and was characterized by a particularly compact shape. The flood volume in 2008 was about 1100 mil. m³, while the flood control volume of the reservoir is 665 mil. m³. Considering the entire duration of the flood (the period for which the flow $Q\left(t\right)$ > $Q_{thr2}=190$ m³/s), the average inflow in the reservoir during the 2008 flood was 108 mil. m³/day or 1245 m³/s. These values are almost double the similar values of the next dangerous flood according to the maximum discharge. This second dangerous event took place in 2010. The flood volume was 1600 mil. m³, but due to its long duration, the average inflow was only 65 million m³/day or 750 m³/s.

Due to its compactness, the 2008 flood was considered the most dangerous flood ever recorded. Accordingly, the design floods replicate its shape. From relation (1), where $Q_b$ is replaced by $Q_{thr2}$ the duration of the design floods is computed as follows:

$$D={\displaystyle \frac{V}{\gamma \left(Q^{max}-Q_{thr2}\right) }}$$

(12)

where $\gamma$ is the compactness coefficient of the 2008 flood, while $Q^{max}$ and $V$ are replaced by the univariate quantiles of the corresponding probabilities of exceedance. As an example, Figure 17 shows the design floods 10%, 1%, and 0.1% AEP for the CC scenario.

3.2. Operating Rules

3.2.1. Design Phase Operating Rules

The proposed simulation-optimization model was tested on the design hydrograph, defined by the maximum discharge $Q_{0.1\%}^{max}$ = 4300 m³/s (Figure 18). Hydrological data are available at a 12 h resolution.

The design flood was obtained by scaling the 1969 flood hydrograph with $Q_{0.1\%}^{max}=4300$ m³/s, keeping the same flood duration. The flood event in 1969 was the highest maximum flood recorded up to the time the dam was designed.

According to Figure 18a, using the operating rules from the design phase, the downstream flows exceed 2300 mc/s for the first and last peaks [90] (p. 210). The operating rules resulting from the optimization model (Figure 18b) lead to downstream discharges much lower (1400 m³/s compared to 2300 m³/s).

3.2.2. Operating Rules According to Current and Climate Change Hydrology

During the design phase, the following constraints were established for reservoir operation:

• carrying capacity of the riverbed downstream of the dam = 550 m³/s.
• carrying capacity between the dikes with a minimum freeboard = 700 m³/s
• maximum overflow above the flap gates = 0.50 m
• design flood level for medium floods (1%) = 98.20 MASL.
• design flood level for rare floods (0.1%) = 99.50 MASL.

The design flood levels correspond to the maximum water elevation in the reservoir during the routing of the flood characterized by 1% and 0.1% AEP of the maximum discharge, respectively. Up to a 1% flood, the goal is not to exceed the carrying capacity of the riverbed, while for a rare flood (such as the 0.1% flood), the structural safety of the dam is the main priority.

The conservation level was set in the design phase at 90.80 MASL for hydroelectricity production and water demands for population and irrigation. Pre-release of the reservoir is allowed based on a flood warning, which usually does not exceed 3 days lead time. However, the maximum pre-release volume for this timespan, considering all bottom outlets in operation (power galleries, backup intake and central bottom outlets) does not exceed 100 mil. m³. Due to the limited flood storage (665 mil. m³) compared to the design flood volume (up to 2400 mil.m³), the operating rules depend not only on the water level in the reservoir, but also on the flood volume forecast for the next 3 days at Rădăuți–Prut gauge station.

The decision to gradually put into operation the outlet discharges (power galleries, backup intake, and lateral bottom outlets) is taken when the flow at Rădăuți–Prut reaches the value of 200 m³/s, and the hydrological forecast anticipates maximum flows for the next 3 days of at least 600 m³/s. For high waters characterized by maximum flow in the range (200–600 m³/s), the water level in the reservoir must be kept almost constant, and the output discharge must be equal to the input flow in the reservoir.

After receiving the flood warning the operating rules correspond to the 10% flood. Based on the continuous hydrological forecast, at 91.00 MASL (representing the first point of divergence in flood mitigation), water managers must decide whether to follow the 10% operating rules or switch to the 1% flood rules. The second point of divergence is at 95.50 MASL when the decision is to continue with 1% flood rules or to adopt 0.1% operating rules.

Operating rules for intermediate floods such as 5% and 2%, were also analysed. The goal is to derive clear operating rules to avoid inappropriate decisions in real-time operations. The number of outlet manoeuvres must be as low as possible.

The operating rules were obtained for the following cases: (1) the most likely scenario with two sub-cases: (a) maximum discharge flood $(Q_{P\%}^{max}; V_{P\%}^L)$ and (b) maximum volume flood $(Q_{P\%}^{\max L}; V_{P\%})$; (2) the worst-case scenario corresponding to a maximum possible flood under current hydrological conditions ($Q_{P\%}^{max};$ $V_{P\%}$); and (3) the flood under climate change conditions ($Q_{P\%}^{\max CC};$ $V_{P\%}^{CC}$).

Since $Q_{P\%}^{\max CC}>Q_{P\%}^{max}$ and $V_{P\%}^{CC}$ > $V_{P\%}$, the 3rd case leads to the most difficult operating conditions. If during real-time operation either the maximum discharge or the flood volume is lower than the climate change values, compliance with the CC rules provides a safety margin. As previously mentioned, the shape of the design flood is like that of the 2008 flood, characterized by high compactness, which was not encountered before or after 2008. Such a shape of the design flood leads to difficult mitigation cases, providing an additional degree of protection in real-time flood operation.

Operating Rules for the Flood 10% CC ($Q_{10\%}^{max CC}$ = 1575 m³/s; $V_{10\%}^{CC}$ = 770 mil m³)

If the hydrological forecast at Rădăuți–Prut gauge station anticipates flows of at least 600 m³/s, but less than 1500 m³/s, the following operating rules are adopted (Figure 19):

-

Hydropower galleries—permanently open

-

Central bottom outlets (no. 2 and 3)—open during the pre-flood and flood event; closed when the water level in the reservoir is at 91.00 MASL in the post-flood phase.

-

Backup intake—same operating rules as central bottom outlets.

-

All other outlets are closed, and the flap gates of the weirs are in the raised position.

The maximum water level in the reservoir is below the level of the spillway crest (95.50 MSL), while the maximum discharge is close to the carrying capacity of the riverbed (

Table 8. Flood 10% CC ($Q_{10\%}^{max CC}$ = 1575 m³/s; $V_{10\%}^{CC}$ = 770 mil. m³).

Max Water Level (MASL)	Max Downstream Discharge (m3/s)
94.17	581

).

Operating Rules for the Flood 5% CC ($Q_{10\%}^{maxCC}$ = 2000 m³/s; $V_{10\%}^{CC}$ = 1100 mil m³)

If the water level in the reservoir is at 91.00 MASL and the hydrological forecast for 3 days at Rădăuți–Prut gauge station indicates discharges in the range 1500–2000 m³/s the 10% flood rules remain unchanged, but the operating time of the outlets is longer, lasting until the normal operating level is reached (Figure 20).

The larger volume of the 5% CC flood (1100 mil m³ vs. 770 mil m³ for the 10% CC flood) will result in higher water levels in the reservoir (96.85 MASL vs. 94.17 MASL) but the maximum downstream discharge remains basically the same (

Table 9. Flood 5% CC ($Q_{5\%}^{max CC}$ = 2000 m³/s; $V_{5\%}^{CC}$ = 1100 mil. m³).

Max Water Level (MASL)	Max Downstream Discharge (m3/s)
96.85	600

).

The main finding related to frequent floods (up to 2000 m³/s) is that the operating rules are the same regardless of the flood volume. The hydropower galleries, central bottom outlets, and backup intake are opened when the flood warning is issued and remain open until the reservoir water level in the post-flood period reaches 91.00 MASL. The only difference is related to the operating time, which is longer the lower the exceedance probability of the flood.

Operating Rules for Medium Floods

A second category of operating rules is obtained for medium floods, whose maximum discharge is in the range (2000, 3600) m³/s. The maximum overflow above the crest of the flap gates is limited to 0.50 m. More than the peak flow, the flood volume dictates the operating rules, which are different for the following subcategories: (1000–1200); (1200–1500); (1500–1800) mil. m³. A 5-to-10-day forecast would be needed to estimate the flood volume based on coupling the meteorological forecast with rainfall–runoff modelling.

In this subsection, the focus will be on the 1% flood, although the findings are valid also for all floods in the 5–1% range. It is recalled that 91.00 MASL represents the first point of divergence in the outlet’s operating rules.

• The 2008 flood ($Q_{2008}^{max}$ = 3380 m³/s; $V_{2008}$ = 1135 mil m³)

The maximum flow of the 2008 flood is close to the 1% AEP, while its volume can be associated with a 5% AEP. The following optimal operating rules are recommended (Figure 21):

-

Hydropower galleries—permanently open.

-

Central bottom outlets (no. 2 and 3)—opened at flood warning and closed at 91.00 MASL water level in the reservoir.

-

The lateral bottom outlets (1 and 4) come into operation when the water level reaches 91.00 MASL and remain open until the water level reaches 91.00 again during post-flood.

-

Backup intake—continuous operation, until level 91.00 MASL is reached during reservoir emptying.

-

Flap gates of the weirs are in the raised position.

The maximum water level in the reservoir slightly exceeds the level of the flap crest (98.50 MASL), while the maximum discharge overflows the defence dikes downstream (

Table 10. Flood 2008 ($Q_{2008}^{max}$ = 3380 m³/s; $V_{2008}$ = 1135 mil. m³).

Max Water Level (MASL)	Max Downstream Discharge (m3/s)
98.52	890

). Based on the knowledge of the entire flood wave, the operating rules obtained through optimization (Figure 21) are simpler and easier to implement, using a smaller number of manoeuvres, than those used during the 2008 flood (Figure 7).

2.

The 1% flood ($Q_{1\%}^{max}$ = 3350 m³/s; $V_{1\%}^L$ = 1475 mil m³)

The optimal operating rules when the forecast volume is in the range (1200–1500) mil. m³ are as follows (Figure 22):

-

Hydropower galleries—permanently open.

-

Central bottom outlets (no. 2 and 3)—opened at flood warning and closed at 91.00 MASL water level during post-flood.

-

The lateral bottom outlets (1 and 4) come into operation when the water level reaches 91.00 MASL and remain open until the water level reaches 91.00 MASL during post-flood.

-

Backup intake—in operation, until level 91.00 is reached during the emptying phase.

-

Flap gates of the weirs are in the raised position.

The maximum water level in the reservoir (

Table 11. Flood 1% ($Q_{1\%}^{max}$ = 3350 m³/s; $V_{1\%}^L$ = 1475 mil. m³).

Max Water Level (MASL)	Max Downstream Discharge (m3/s)
98.70	1350

) exceeds 0.50 m the level of the flap crest (which is acceptable), while the maximum discharge downstream is much larger than in the case of the 2008 flood (1350 m³/s vs. 890 m³/s). The difference in volume between the 2008 flood (1135 million m³) and the lower limit of the 1% flood volume (1475 million m³) leads to the alteration of the optimal operating rule, as well as extensive downstream flooding.

3.

Operating rules for the flood 1% CC ($Q_{1\%}^{max CC}$ = 3600 m³/s; $V_{1\%}^{CC}$ = 1850 mil m³)

In the climate change scenario, the peak flow is about 10% higher than the $Q_{1\%}^{max}$, while the flood volume is about 25% larger than the $V_{1\%}^L$. This will result in higher levels in the reservoir as well as a much higher maximum downstream flow than in the previous case. To prevent excessive overflow above the flap gates (higher than 0.50 m), all weirs must be opened when the water level is at the crest of the spillway. For the CC scenario, or when the forecast volume is in the range (1500–1850) mil. m³, the optimal operating rules are as follows (Figure 23):

-

Hydropower galleries—permanent operation

-

Central bottom outlets (2 and 3)—opened at flood warning and closed at 91.00 MASL water level in the reservoir during the post-flood phase.

-

Lateral bottom outlets (1 and 4) come into operation when the water level reaches 91.00 MASL and remain open until the water level reaches 91.00 MASL during the post-flood phase.

-

Backup intake—in operation, until level 91.00 is reached during the emptying phase.

-

The flap gates of all weirs are lowered when 95.50 MASL water level is reached.

In the 1% CC scenario, the maximum water level in the reservoir (

Table 12. Flood 1% CC ($Q_{1\%}^{\max CC}$ = 3600 m³/s; $V_{1\%}^{CC}$ = 1850 mil. m³).

Max Water Level (MASL)	Max Downstream Discharge (m3/s)
98.74	2440

) exceeds the limit set for 1% flood level (98.20 MASL) in the design phase. Extensive flooding will occur downstream of the dam.

Operating Rules for Rare Floods

In this subsection, the mitigation of the 0.1% flood will be analysed. The operating rules derived for this flood are, however, valid for all floods in the 1–0.1% range. The second divergence point regarding the operating decision is at 95.50 MASL in the reservoir. If at this level the flow forecast at Rădăuți–Prut for the next 3 days indicates a downward trend (the flow falls below 3000 m³/s), mitigation continues according to the 1% flood rules. If, on the contrary, the flows at Rădăuți–Prut still have an increasing tendency, and the forecast flows exceed 4000–4500 m³/s, the operating rules according to the 0.1% flood are adopted.

The 0.1% Flood ($Q_{0.1\%}^{max}$ = 4900 m³/s; $V_{0.1\%}^L$ = 1830 mil m³)

The following operating rules have been obtained (Figure 24):

-

Hydropower galleries—permanent operation

-

Central bottom outlets (2 and 3)—opened at flood warning and closed at 91.00 MASL water level in the reservoir during the post-flood phase.

-

Lateral bottom outlets (1 and 4) come into operation when the water level reaches 91.00 MASL and remain open until the water level reaches 91.00 MASL during the post-flood phase.

-

Backup intake—opened at flood warning and remains open until level 91.00 MASL is reached during the emptying phase of the reservoir.

-

All weirs (1…6) come into operation at a water level of 95.50 MASL; however, it is recommended to lower the flap gates before the water reaches the crest of the spillways.

The maximum water level in the reservoir (

Table 13. Flood 0.1% ($Q_{0.1\%}^{max}$ = 4900 m³/s; $V_{0.1\%}^L$ = 1830 mil. m³).

Max Water Level (MASL)	Max Downstream Discharge (m3/s)
99.84	3095

) exceeds the limit of 99.50 MASL established in the design phase for the 0.1% flood.

Keeping the same operating rules, the situation is much worse in the CC scenario (

Table 14. Flood 0.1% CC ($Q_{0.1\%}^{\max CC}$ = 5300 m³/s; $V_{0.1\%}^{CC}$ = 2400 mil. m³).

Max Water Level (MASL)	Max Downstream Discharge (m3/s)
100.74	3650

). Less than 2 m of freeboard remains at the crest of the dam (102.50 MASL).

4. Discussion

1. The IPCC reports provide detailed projections for the 21st century. Global warming will continue, with estimates indicating that the Earth could warm as much as 3 °C by the year 2100 [91]. However, the target agreed by many countries in the Copenhagen Agreement in 2009 was 2 °C, which was later reassessed to 1.5 °C. It should be noted, however, that for Central and Southern Europe an increase of 1 °C above the global average is expected in both the +2 °C and +1.5 °C scenarios. Climate projections show that changes in average temperature and average precipitation amounts occur together with changes in the statistics of extreme phenomena, such as increased intensity and frequency of heat waves, increased precipitation intensity, etc. [92].

To assess the impact of climate change on the maximum flows in Romania [93] for the time horizon (2021–2050), the Romanian hydrological model CONSUL was used. The hydrological simulations were carried out considering two scenarios: Scenario 0, where the flow was calculated for the reference period 1971–2000, and Scenario 1, which simulates the flow for the period 2021–2050. The period 2000–2006 served for calibration. Within the CLAVIER Project [94] (REMO model, version 5.7) simulations were performed using as input data the precipitation and temperature time series resulting from two different climate change scenarios [95].

Long-term simulations show that on more than half of Romania’s territory, the maximum flow with an annual probability of exceedance of 1% will increase in the period 2021–2050 compared to the reference period. The National Institute of Hydrology and Water Management in Romania has for now recommended the use of the climate change scenario only for discharges corresponding to 1% AEP. The increase is between 10% and 20% compared to the estimated flow for the reference period. The design flood should be increased with caution, however, as it involves increased investment. Given that climate projections still have a high degree of uncertainty, further detailed analysis of the impact of climate change on design floods is needed. It is expected that in the period 2051–2100, the hydrological changes compared to the reference period 1971–2000 will be greater.

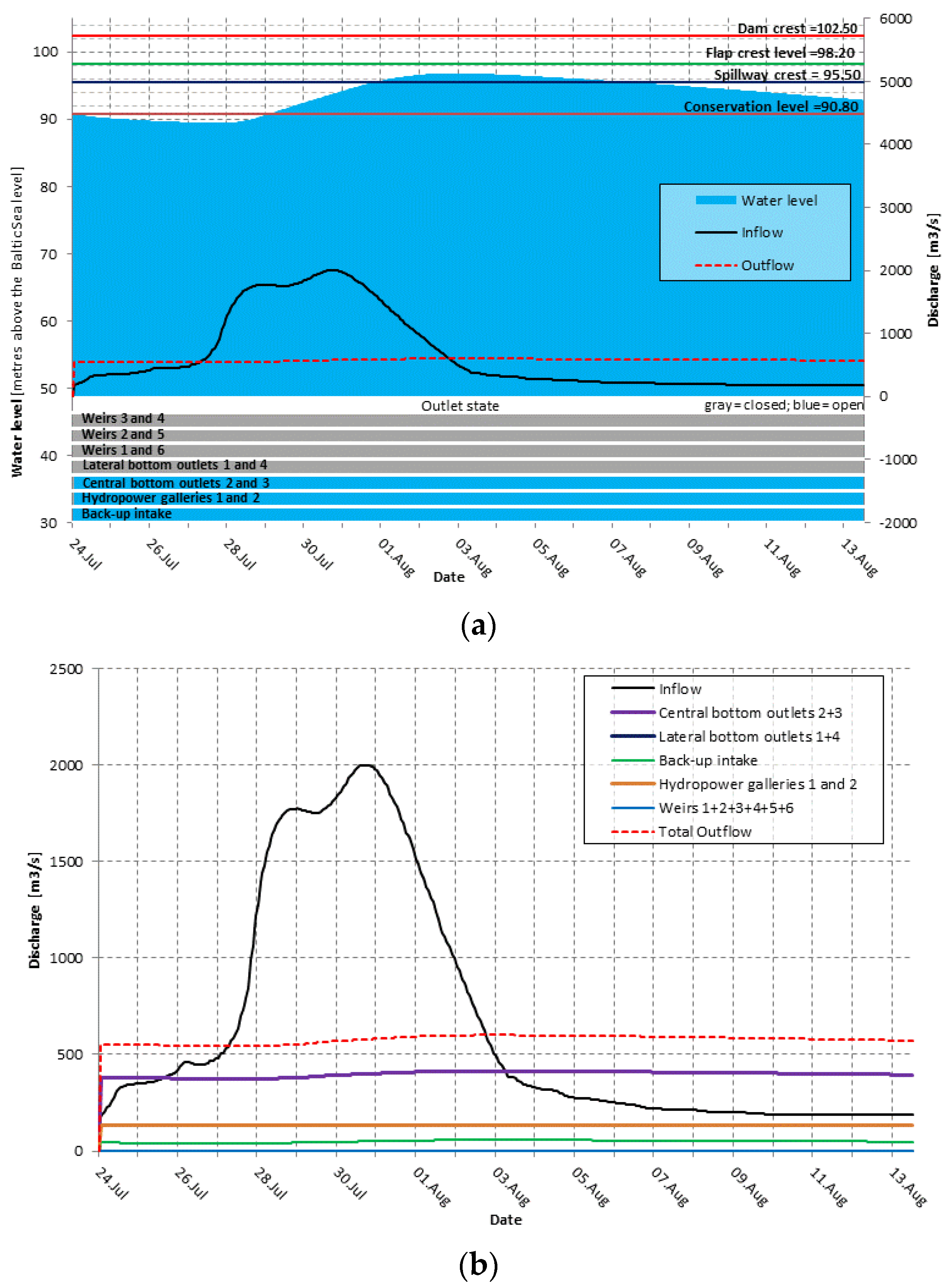
2. Reservoir operation during floods is dependent on forecast accuracy, which is never perfect. The flood protection volume of the Stânca–Costești reservoir is large enough to prevent downstream flooding and to guarantee the safety of the dam for current floods (5% or higher AEP of the design flood). For such floods, the maximum water level in the reservoir is lower than the crest of the spillway and the flap gates of the weirs can be kept in the raised position. At the same time, the maximum downstream flow is carried by the riverbed without overflowing its banks.

If the maximum inflow is less than 600 m³/s, the water level is kept almost constant by opening the hydropower galleries, the backup intake and if necessary, the central bottom outlets (no. 2 and 3). For higher discharges, corresponding to a 10–5% probability of exceedance of the design floods, all bottom outlets are opened and, except for the hydropower galleries, will be closed when the water level is at 91.00 MASL during the post-flood period.

3. Problems may occur with medium floods (in the range of 5–1% probability of exceedance of the design flood). For the most severe flood registered to date, in 2008, the optimal operating rules are obtained by closing the central bottom outlets at 91.00 MASL and opening the lateral bottom outlets at the same level, while the hydropower galleries and the backup intake work continuously. The maximum water level (98.52 MASL) is slightly above the crest of the flap gates in the raised position (98.50 MASL), while the downstream discharge (890 m³/s) exceeds the carrying capacity of the riverbed (700 m³/s).

The operating rules are slightly modified for the 1% design flood calculated for current hydrology. Thus, the central bottom outlets (no. 2 and 3) are maintained continuously open, while the operation of the other outlets is the same as in the case of the 2008 flood. In this case, the maximum output flow is 1350 m³/s, resulting in downstream floodings. The crest level of flap gates in the raised position is 98.20 MASL and the maximum allowable overflow above them is 0.50 m. The highest water level of 98.70 MSL reached during the simulation represents the maximum level of water that can still be accepted in the reservoir.

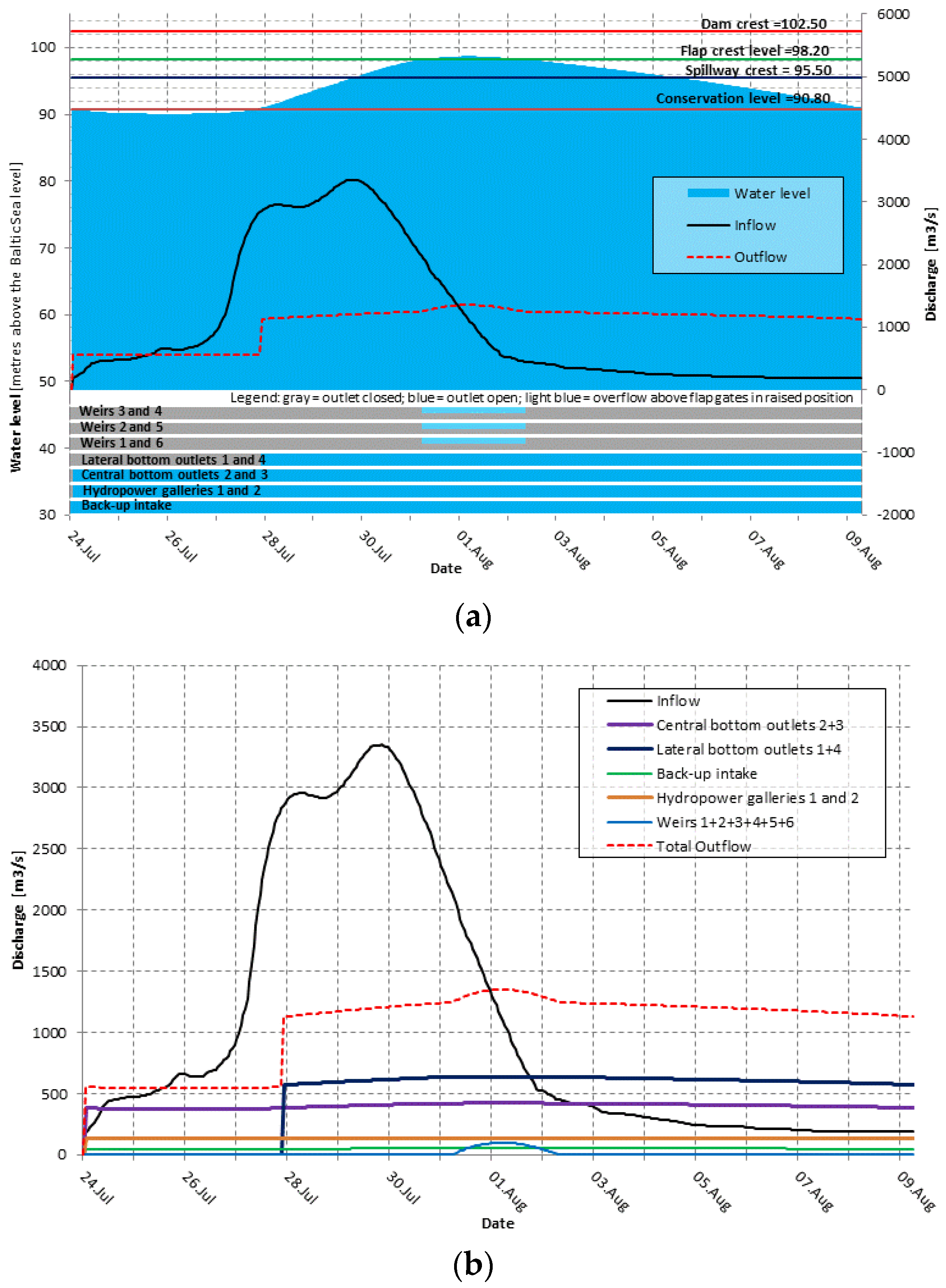
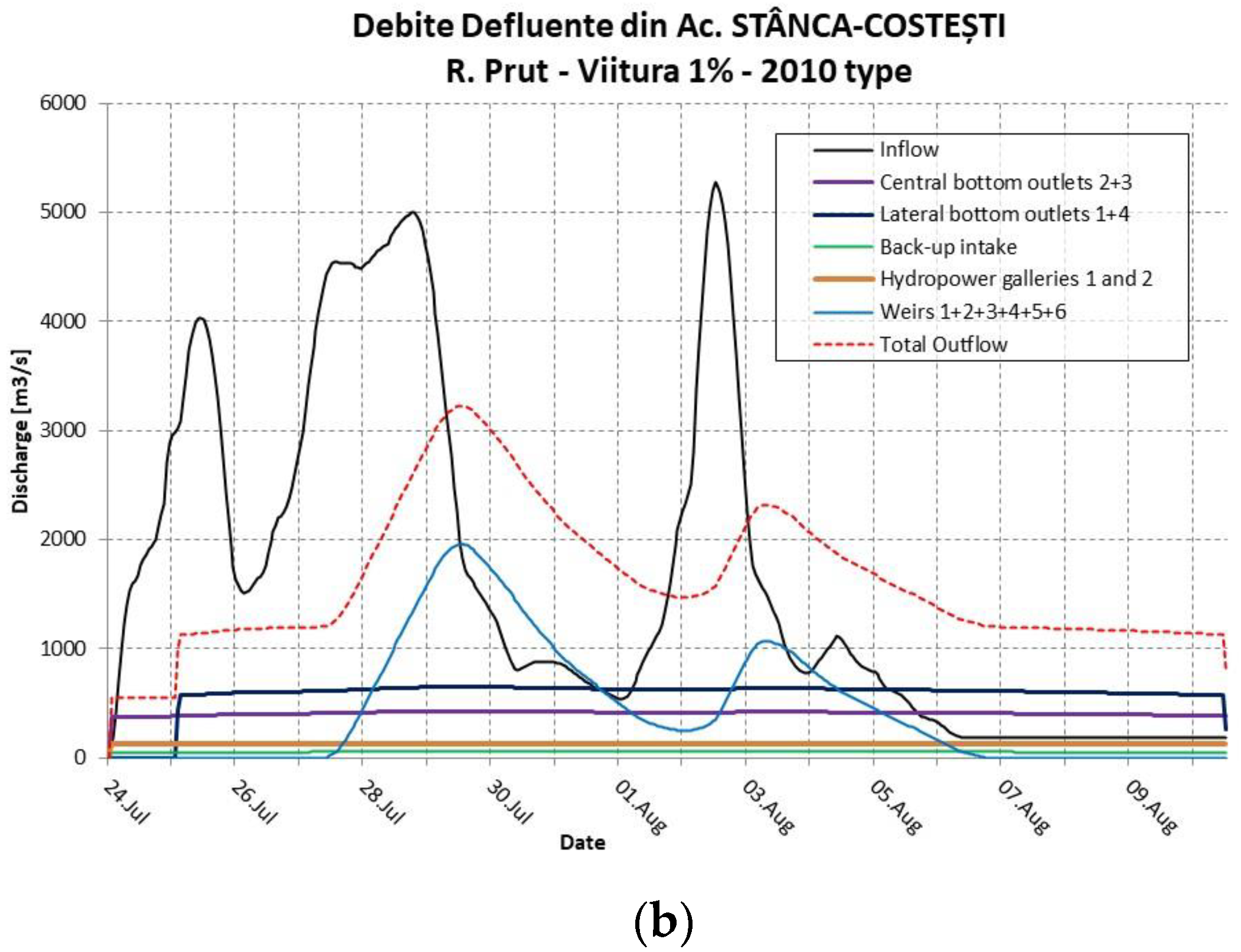
In the case of the 1% CC scenarios, the water level will be much higher, and the flap gates must be lowered when the 95.50 MASL is reached. At the same time, the operating rules are modified as follows: the central bottom outlets are kept open throughout the flood event; the lateral bottom outlets are opened when the water level is at 91.00 MASL and the hydrological forecast anticipates large volumes (over 1800 mil. m³, compared to 1100 mil. m³ during the 2008 flood); and the flap gates of all weirs are lowered when the water level of 95.50 MASL is reached. The maximum water level in the reservoir is at 98.74 MASL (close to 0.1% design level) and the maximum outflow is 2440 m³/s.

4. The same operating rules as for the 1% CC scenario will be kept for the 0.1% design flood and 0.1% CC scenarios, but the water levels and outflows are extremely high. Thus, depending on flood volume, the maximum values for water level and downstream discharge are in the range 99.84–100.74 MASL and 3095–3650 m³/s, respectively. It is recalled that the crest of the dam is at 102.50 MASL. The only solution to avoid potentially dangerous situations would be to extend the forecast period and open the lateral bottom outlets at the same time as the other bottom outlets.

In all scenarios, the bottom outlets, except for hydropower galleries, will be closed when the water level falls to 91.00 MASL during the post-flood period.

5. The operation decision depends not only on the water level in the reservoir, but also on the flood hydrograph forecast (including its volume) for the next few days.

When the water level in the reservoir at the beginning of the flood reaches 91.00 MASL, the operation decision is at the first divergence point. If the forecast at Rădăuți–Prut for the next 3 days indicates flows below 2000 m³/s and volumes lower than 1000 mil. m³ the operation will be carried out according to the 5–10% flood rules. Otherwise, if the forecast flows are in the range of 2000–3600 m³/s, the operating rules are according to the 1% flood but depend on the forecasted flood volume: (1000–1200), (1200–1500), (1500–1800) mil. m³. The second divergence point regarding the operation decision is at 95.50 MASL. If the flow forecast at Rădăuți–Prut for the next 3 days indicates an increasing trend, and the forecast flows exceed 4000–4500 m³/s, the flood operating rules of 0.1% flood are adopted. If, on the contrary, the flows at Rădăuți–Prut have a downward trend (the flow falls below 3000 m³/s), the operation continues according to the 1% flood rules.

6. As previously mentioned, the 2008 flood was the most compact on record. For any other shape of the flood (recorded or synthetic), by applying the operating rules established for the 2008 shape, flood attenuation occurs in more advantageous conditions regardless of the probability of exceedance. This statement also holds for multi-peak floods, characterized by higher flood volumes and longer durations than single-peak floods. An example of the 0.1% CC flood mitigation considering the 2010 shape of the flood is shown in Figure 25. The maximum water level (100.04 MASL—

Table 15. Flood 0.1% multipeak CC ($Q_{0.1\%}^{\max CC}$ = 5300 m³/s; $V_{0.1\%}^{CC}$ = 2400 mil. m³).

Max Water Level (MASL)	Max Downstream Discharge (m3/s)
100.04	3225

) is higher for one day than the design level for the 0.1% flood (99.50 MASL), but without raising serious problems related to the safety of the dam. On the other hand, the maximum downstream flow is lower than for a single-peak flood having the same volume and maximum discharge (Table 14).

As a finding, multi-peak floods are easier to manage than single-peak floods due to their long duration, which favours the release of large volumes during the flood event. Both maximum reservoir levels and maximum downstream flows are lower than in single-peak floods, which are more compact.

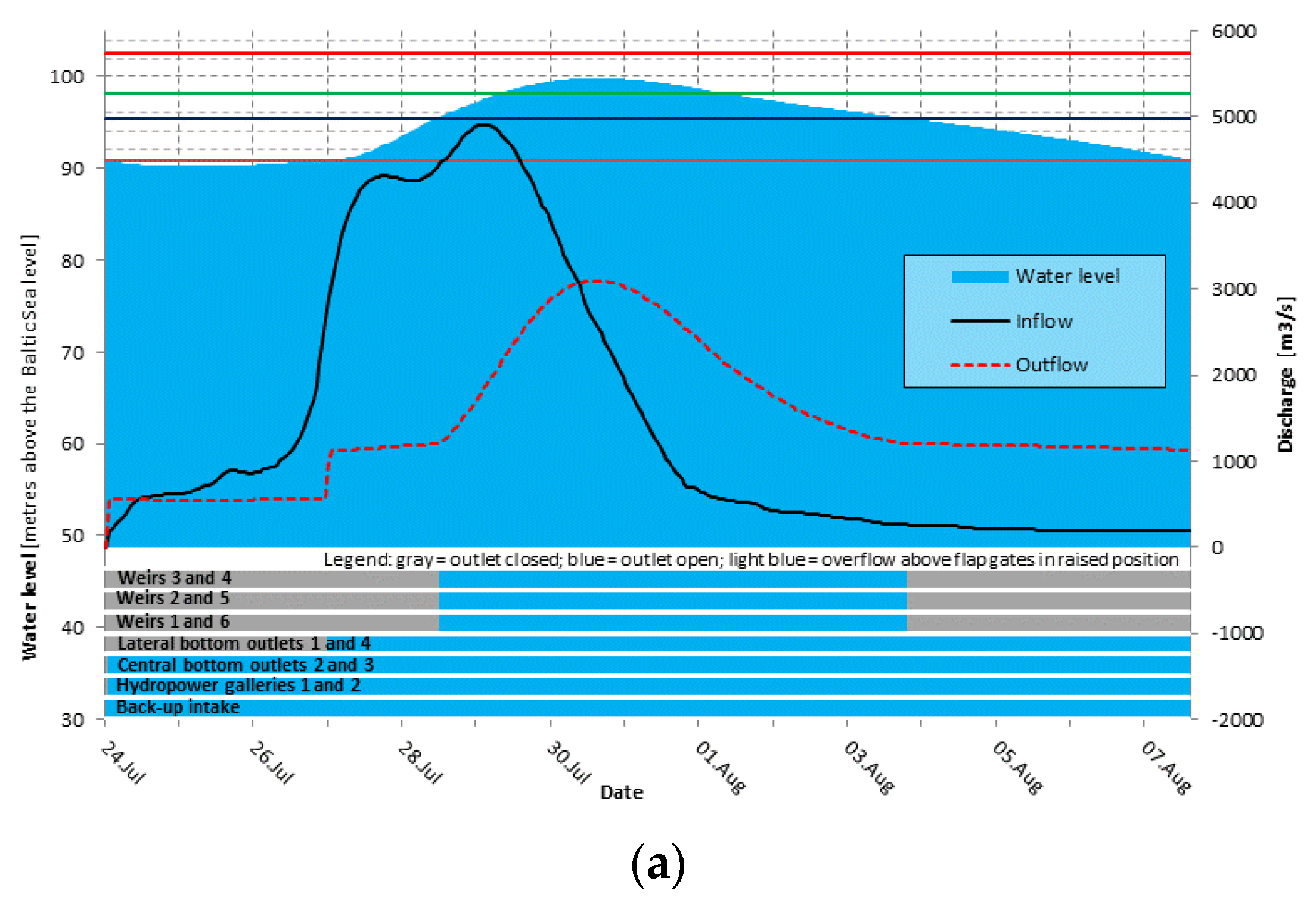
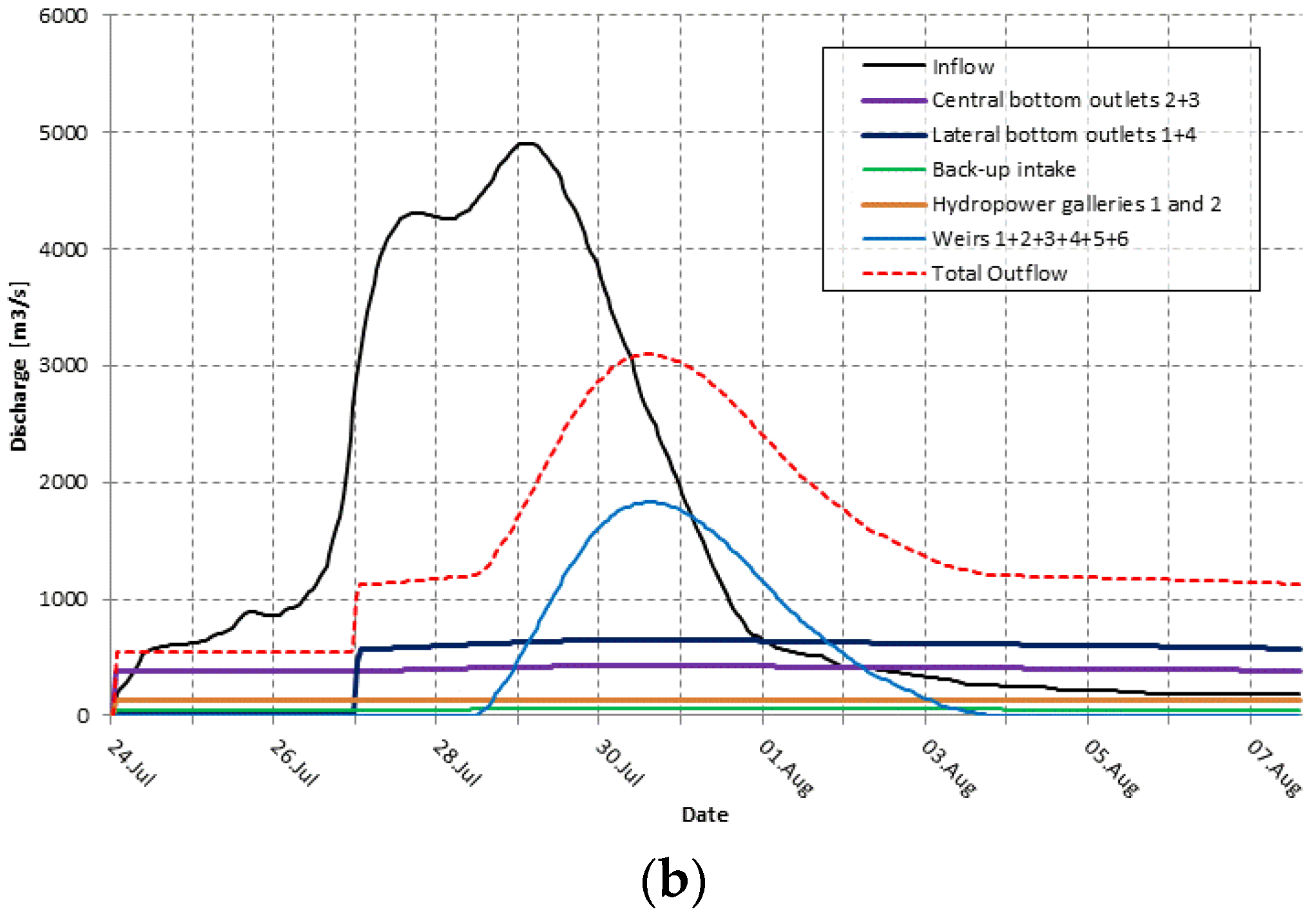
7. It was shown that based on the knowledge of the entire flood wave, the operating rules obtained through optimization (Figure 21) are simpler and easier to implement, using a smaller number of manoeuvres than those used during the 2008 flood (Figure 7). The number of manoeuvres is also reduced compared to the rules in place at the time of commissioning of the reservoir, while having greater efficiency in the mitigation process (Figure 18).

8. A valuable piece of information for adopting the best operating rules is the forecast of the cumulative average rainfall for the next 10 days on the entire basin upstream of the Rădăuți–Prut gauge station (

Table 16. Operation decision according to average areal rainfall.

Cumulative Average Rainfall in 10 Days (mm)	$\mathbf{Operating} \mathbf{Decision} \mathbf{According} \mathbf{to} \mathit{P}\mathit{\%}$ Design Flood
<100	5% (10%)
100–200	1%
>200	0.1%

). Based on the rainfall forecast, water managers can anticipate the operation decisions that will be made in the coming days. Three categories of operating rules should be considered: (a) if the cumulative average rainfall upstream of the Rădăuți–Prut gauge station for the next 10 days is less than 100 mm, the operating rules for the 5% design flood will most likely be adopted; (b) for 100–200 mm, the operating rules for the 1% design flood are expected; (c) for exceptional rainfall greater than 200 mm, the operating rules for the 0.1% design flood are mandatory.

5. Conclusions

1. The exceptional floods of 2008 ($Q_{2008}^{max}$ = 3300 m³/s; $V_{2008}$ = 1135 mil m³) and 2010 ($Q_{2010}^{max}$ = 1710 m³/s; $V_{2010}$ = 1695 mil m³) on the Prut River increased both peak flow and flood volume values for all AEPs. Thus, a new statistical analysis was necessary.

2. Registered floods at Rădăuți–Prut gauging station are of two types: single-peak floods lasting up to 10–15 days, and multi-peak floods exceeding 20 days. For the single-peak design flood, the 2008 flood shape is chosen due to its high compactness, while the typical multi-peak design flood reproduces the 2010 flood shape characterized by a long duration.

3. The simulations showed that, due to the limited flood control volume of the reservoir compared to the inflow volume for low values of AEPs, bottom outlets must be opened in the initial stages of flood generation. The operating decision depends not only on the water level in the reservoir, but also on the flood hydrograph forecast (and implicitly its volume) for the following days. Ignoring the hydrological forecast leads to higher levels in the reservoir, the structural safety of the dam being ensured by discharging increased flows downstream.

4. Based on the meteorological and hydrological forecast, the predicted floods are assimilated to one of the following categories of AEPs: (10–5%), (5–1%), and (1–0.1%). Floods with an AEP greater than 5% will be operated according to the 5% design flood rules, floods with an AEP between 1% and 5% will be managed according to the 1% design flood rules, and floods with an AEP less than 1% will be managed according to the 0.1% design flood rules.

There are two divergence points during flood mitigation, at 91.00 MASL and at 95.50 MASL, respectively, when framework decisions (10%, 1% or 0.1%) are adopted based on flood forecast. When in flood conditions, the water level in the reservoir reaches 91.00 MASL, and the forecast flow at Rădăuți–Prut for the next 3 days indicates discharges below 2000 m³/s, operations will be carried out according to the 5–10% flood rules. Otherwise, if the forecast discharges are in the range of 2000–3600 m³/s, the operating rules for 1% floods will be followed. In this case, the operation is different depending on the forecast flood volume: (1000–1200), (1200–1500), and (1500–1800) mil. m³. For higher flows and volumes, the rules corresponding to the 0.1% design flood are adopted.

Current floods with a maximum discharge of less than 2000 m³/s and a volume of less than 1100 million m³ (representing most recorded floods) can be mitigated without exceeding the carrying capacity of the river downstream of the dam. For larger floods, dam security prevails, and the outflows are increased resulting in significant flooding downstream of the dam.

5. Following the simulations, it was found that the flood volume is as important as the maximum flow, both conditioning the optimal flood mitigation rules. This finding is important because, in current management practice, design floods corresponding to different AEPs are usually characterized only by maximum discharge, the importance of the flood volume usually being underestimated. The shape of the flood, characterized by its compactness, is also important. Consequently, a well-defined design flood is characterized by the following parameters: maximum discharge, flood volume, time to peak, flood duration, and shape.

6. For effective operation, the operating rules must be detailed at the level of each outlet, instead of providing global rules that depend only on the water level in the reservoir. This approach is only possible for dams that have moving parts, such as bottom gates or controlled spillways.

7. The optimal operating rules obtained for the most-used AEPs (10%, 5%, 2%, 1%, 0.5%, 0.2%, and 0.1%) represent framework operating rules. At the flood warning, the operating rules corresponding to the 10% design flood rules are adopted. Depending on the flood forecast as well as the water levels in the reservoir, the operating rules are adapted successively from the rules corresponding to the 10% flood to the 1% flood rules and then, if necessary, to the 0.1% flood rules. Obviously, adaptive operation based on successive short-term forecasts during the real-time flood event leads to a slightly lower performance than in the case of perfect knowledge in advance of the flood hydrograph.

In the post-flood phase, the bottom outlets can be left open until the conservation level is reached, or they can be closed earlier to exploit the surplus resource. The decision depends on the hydrological forecast. If a future flood is expected, the flood protection volume should be emptied as soon as possible.

Future work. The flood mitigation analysis was completed assuming that the outlets can be closed or opened when needed without incident. However, this may not always be the case. Thus, during the 2008 flood, one of the floodgates could not be lowered due to a failure of the operating mechanism. A future research direction is to find alternative operating rules when some outlets are blocked for various reasons. A DSS will be developed to offer real-time operating alternatives in such situations. Construction of an additional spillway on the left bank will also be considered to avoid dangerous water levels in the reservoir during flood events, which would jeopardize the safety of the dam.