Comparing Methods for the Regionalization of Intensity−Duration−Frequency (IDF) Curve Parameters in Sparsely-Gauged and Ungauged Areas of Central Chile
Abstract
1. Introduction
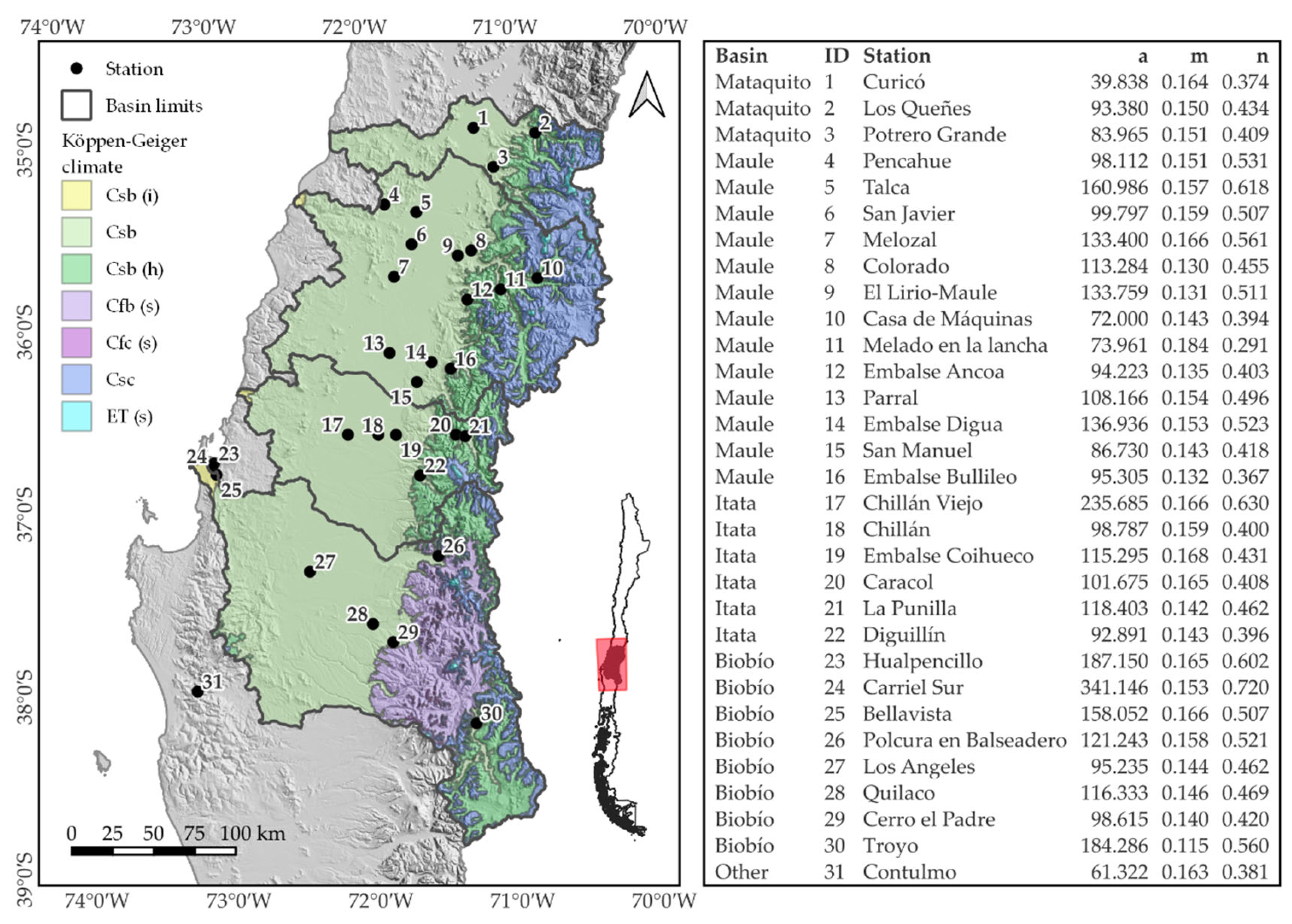
2. Materials and Methods
2.1. IDF Curves
2.2. Spatial Interpolation and Extrapolation of IDF Curves
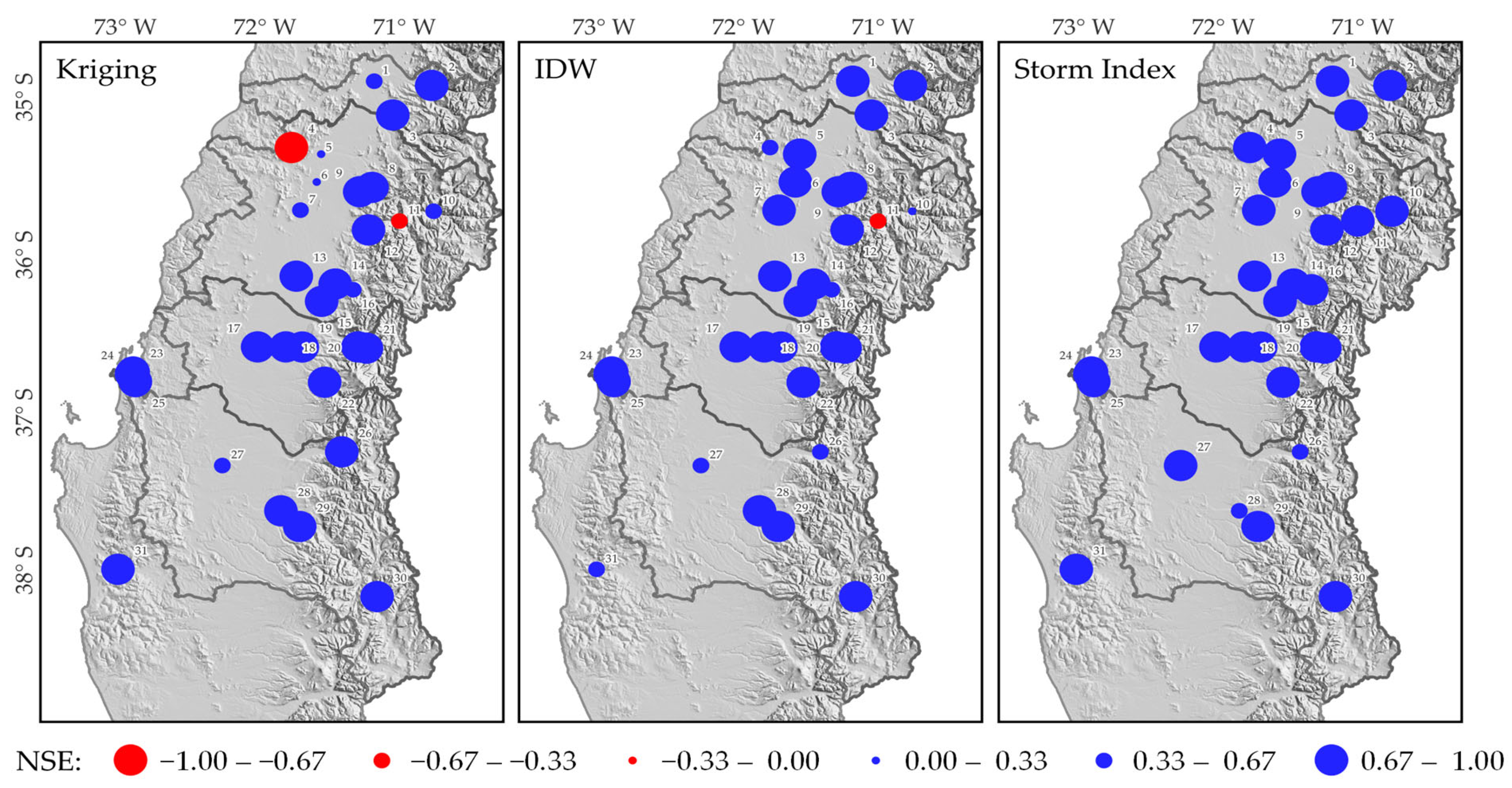
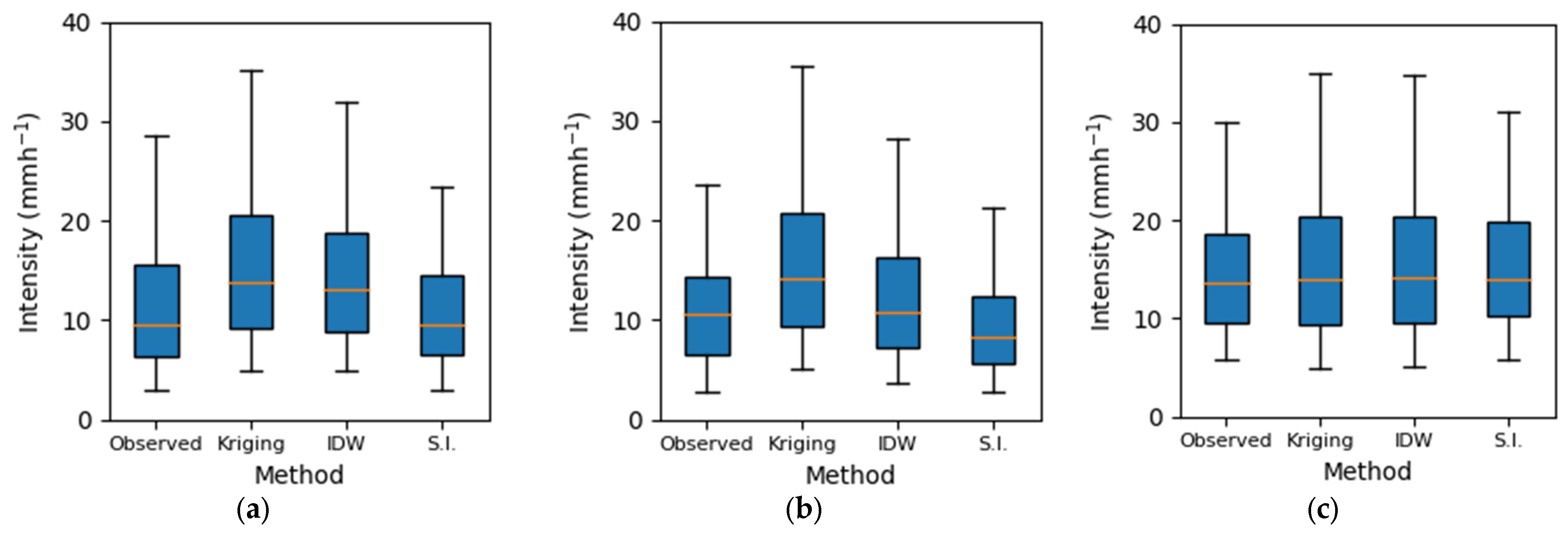
3. Results
Goodness-of-Fit of the Methods
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Link, G.A.; Watkins, D.W., Jr.; Johnson, D.L. Mapping Spatial Variation in Rainfall Intensity-Duration-Frequency Estimates Using a Geographical Information System. In Proceedings of the Joint Conference on Water Resource Engineering and Water Resources Planning and Management 2000, Minneapolis, MN, USA, 30 July–2 August 2000; pp. 1–10. [Google Scholar]
- Bara, M.; Gaál, L.; Szolgay, J.; Kohnová, S. Estimation of IDF Curves of Extreme Rainfall by Simple Scaling in Slovakia. Contributions to Geophysics and Geodesy. Contrib. Geophys. Geod. 2009, 39, 187–206. [Google Scholar]
- Asikoglu, O.L.; Benzeden, E. Simple Generalization Approach for Intensity-Duration-Frequency Relationships: Simple generalization approach for IDF relationships. Hydrol. Process. 2014, 28, 1114–1123. [Google Scholar] [CrossRef]
- Uboldi, F.; Sulis, A.N.; Lussana, C.; Cislaghi, M.; Russo, M. A Spatial Bootstrap Technique for Parameter Estimation of Rainfall Annual Maxima Distribution. Hydrol. Earth Syst. Sci. 2014, 18, 981–995. [Google Scholar] [CrossRef]
- Pizarro, R.; Valdés, R.; Abarza, A.; Garcia-Chevesich, P. A Simplified Storm Index Method to Extrapolate Intensity-Duration-Frequency (IDF) Curves for Ungauged Stations in Central Chile: Storm index method to extrapolate IDF curves. Hydrol. Process. 2015, 29, 641–652. [Google Scholar] [CrossRef]
- Langousis, A.; Veneziano, D. Intensity-Duration-Frequency Curves from Scaling Representations of Rainfall: Scaling representations of rainfall. Water Resour. Res. 2007, 43, W02422. [Google Scholar] [CrossRef]
- Elsebaie, I.H. Developing Rainfall Intensity–Duration–Frequency Relationship for Two Regions in Saudi Arabia. J. King Saud Univ.—Eng. Sci. 2012, 24, 131–140. [Google Scholar] [CrossRef]
- Sivapalan, M.; Blöschl, G. Time Scale Interactions and the Coevolution of Humans and Water: Time scale interactions and coevolution of humans and water. Water Resour. Res. 2015, 51, 6988–7022. [Google Scholar] [CrossRef]
- Chow, V.T.; Maidment, D.; Mays, L. Hidrología Aplicada; McGraw-Hill: Bogotá, Colombia, 2000; ISBN 978-958-600-171-7. [Google Scholar]
- Simonović, S.P. Floods in a Changing Climate. Risk Management; International Hydrology Series; Cambridge University Press: Cambridge, UK, 2012; ISBN 978-1-107-01874-7. [Google Scholar]
- Kundzewicz, Z.W.; Kanae, S.; Seneviratne, S.I.; Handmer, J.; Nicholls, N.; Peduzzi, P.; Mechler, R.; Bouwer, L.M.; Arnell, N.; Mach, K.; et al. Flood Risk and Climate Change: Global and Regional Perspectives. Hydrol. Sci. J. 2014, 59, 1–28. [Google Scholar] [CrossRef]
- Singh, V.P.; Zhang, L. IDF Curves Using the Frank Archimedean Copula. J. Hydrol. Eng. 2007, 12, 651–662. [Google Scholar] [CrossRef]
- Burn, D.H. A Framework for Regional Estimation of Intensity-Duration-Frequency (IDF) Curves: Regional estimation of intensity-duration-frequency (IDF) curves. Hydrol. Process. 2014, 28, 4209–4218. [Google Scholar] [CrossRef]
- Mirhosseini, G.; Srivastava, P.; Sharifi, A. Developing Probability-Based IDF Curves Using Kernel Density Estimator. J. Hydrol. Eng. 2015, 20, 04015002. [Google Scholar] [CrossRef]
- Sušin, N.; Peer, P. Open-Source Tool for Interactive Digitisation of Pluviograph Strip Charts. Weather 2018, 73, 222–226. [Google Scholar] [CrossRef]
- Casas-Castillo, M.C.; Rodríguez-Solà, R.; Navarro, X.; Russo, B.; Lastra, A.; González, P.; Redaño, A. On the Consideration of Scaling Properties of Extreme Rainfall in Madrid (Spain) for Developing a Generalized Intensity-Duration-Frequency Equation and Assessing Probable Maximum Precipitation Estimates. Theor. Appl. Climatol. 2018, 131, 573–580. [Google Scholar] [CrossRef]
- Espíldora, B. Estimación de Curvas Intensidad-Duración-Frecuencia Mediante Coeficientes Generalizados. In Proceedings of the I Coloquio Nacional Sociedad Chilena de Ingeniería, Sociedad Chilena de Ingeniería Hidráulica, Santiago, Chile, 30 June–2 July 1971. [Google Scholar]
- UNESCO. Curvas Intensidad–Duración–Frecuencia para la Zona Centro sur de Chile; Documento Técnico del PHI-LAC; PHI-LAC: Montevideo, Uruguay, 2007; ISBN 978-92-9089-100-0. [Google Scholar]
- UNESCO. Curvas Intensidad Duración Frecuencia para las Regiones Metropolitana, Maule y Biobío. Intensidades Desde 15 Minutos a 24 Horas; Documento Técnico del PHI-LAC; PHI-LAC: Montevideo, Uruguay, 2013; ISBN 978-92-9089-189-5. [Google Scholar]
- Soto, X.; Meier, C. Subestimación de Los Valores IDF En Concepción. 1: Derivación Completa y Comparación Con Otras Metodologías. In Proceedings of the XXI Congreso Chileno de Hidráulica, Concepción, Chile, 23–25 October 2013. [Google Scholar]
- Soto, C. Construcción de Curvas Intensidad-Duración-Frecuencia, de Alta Resolución Espacial Para La Zona Centro-Sur de Chile; Universidad de la Frontera: Temuco, Chile, 2019. [Google Scholar]
- Alila, Y. A Hierarchical Approach for the Regionalization of Precipitation Annual Maxima in Canada. J. Geophys. Res. Atmos. 1999, 104, 31645–31655. [Google Scholar] [CrossRef]
- Liew, S.; Raghavan, S.V.; Liong, S.-Y. Development of Intensity-Duration-Frequency Curves at Ungauged Sites: Risk Management under Changing Climate. Geosci. Lett. 2014, 1, 8. [Google Scholar] [CrossRef][Green Version]
- Mauriño, M.F. Generalized Rainfall-Duration-Frequency Relationships: Applicability in Different Climatic Regions of Argentina. J. Hydrol. Eng. 2004, 9, 269–274. [Google Scholar] [CrossRef]
- Mailhot, A.; Duchesne, S.; Caya, D.; Talbot, G. Assessment of Future Change in Intensity–Duration–Frequency (IDF) Curves for Southern Quebec Using the Canadian Regional Climate Model (CRCM). J. Hydrol. 2007, 347, 197–210. [Google Scholar] [CrossRef]
- Zhu, J.; Stone, M.C.; Forsee, W. Analysis of Potential Impacts of Climate Change on Intensity–Duration–Frequency (IDF) Relationships for Six Regions in the United States. J. Water Clim. Change 2012, 3, 185–196. [Google Scholar] [CrossRef]
- Liew, S.C.; Raghavan, S.V.; Liong, S.-Y. How to Construct Future IDF Curves, under Changing Climate, for Sites with Scarce Rainfall Records?: Derivation of IDF curves for ungauged sites. Hydrol. Process. 2014, 28, 3276–3287. [Google Scholar] [CrossRef]
- Madsen, H.; Mikkelsen, P.S.; Rosbjerg, D.; Harremoës, P. Regional Estimation of Rainfall Intensity-Duration-Frequency Curves Using Generalized Least Squares Regression of Partial Duration Series Statistics: Regional estimation of rainfall IDF curves. Water Resour. Res. 2002, 38, 21-1–21-11. [Google Scholar] [CrossRef]
- Salas, L.; Fernández, J. Nueva Metodología Para El Análisis de La Variable Intensidad Máxima Anual de Precipitación. Ecología 2006, 20, 435–440. [Google Scholar]
- Soltani, S.; Helfi, R.; Almasi, P.; Modarres, R. Regionalization of Rainfall Intensity-Duration-Frequency Using a Simple Scaling Model. Water Resour. Manag. 2017, 31, 4253–4273. [Google Scholar] [CrossRef]
- Svensson, C.; Clarke, R.T.; Jones, D.A. An Experimental Comparison of Methods for Estimating Rainfall Intensity-Duration-Frequency Relations from Fragmentary Records. J. Hydrol. 2007, 341, 79–89. [Google Scholar] [CrossRef]
- Jaklič, A.; Šajn, L.; Derganc, G.; Peer, P. Automatic Digitization of Pluviograph Strip Charts: Automatic Digitization of Pluviograph Strip Charts. Meteorol. Appl. 2016, 23, 57–64. [Google Scholar] [CrossRef]
- Yu, P.-S.; Chen, C.-J. Potential of extending the rainfall intensity–duration–frequency relationship to non-recording rain gauges. Hydrol. Process. 1997, 11, 377–390. [Google Scholar] [CrossRef]
- Willems, P.; Arnbjerg-Nielsen, K.; Olsson, J.; Nguyen, V.T.V. Climate Change Impact Assessment on Urban Rainfall Extremes and Urban Drainage: Methods and Shortcomings. Atmos. Res. 2012, 103, 106–118. [Google Scholar] [CrossRef]
- Jacques-Coper, M.; Garreaud, R.D. Characterization of the 1970s Climate Shift in South America: Characterization of the 1970 S climate shift in south America. Int. J. Climatol. 2015, 35, 2164–2179. [Google Scholar] [CrossRef]
- Jordan, T.; Riquelme, R.; González, G.; Herrera, C.; Godfrey, L.; Colucci, S.; Gironás, J.; Gamboa, C.; Urrutia, J.; Tapia, L.; et al. Hydrological and Geomorphological Consequences of the Extreme Precipitation Event of 24–26 March 2015, Chile. In Proceedings of the Los Aluviones de Atacama, Contexto, Causas y Efectos, La Serena, Chile, 1 October 2015; pp. 777–780. [Google Scholar]
- Pizarro, R.; Ingram, B.; Gonzalez-Leiva, F.; Valdés-Pineda, R.; Sangüesa, C.; Delgado, N.; García-Chevesich, P.; Valdés, J. WEBSEIDF: A Web-Based System for the Estimation of IDF Curves in Central Chile. Hydrology 2018, 5, 40. [Google Scholar] [CrossRef]
- Pizarro-Tapia, R.; González-Leiva, F.; Valdés-Pineda, R.; Ingram, B.; Sangüesa, C.; Vallejos, C. A Rainfall Intensity Data Rescue Initiative for Central Chile Utilizing a Pluviograph Strip Charts Reader (PSCR). Water 2020, 12, 1887. [Google Scholar] [CrossRef]
- Pizarro, R.; Valdés, R.; García-Chevesich, P.; Vallejos, C.; Sangüesa, C.; Morales, C.; Balocchi, F.; Abarza, A.; Fuentes, R. Latitudinal Analysis of Rainfall Intensity and Mean Annual Precipitation in Chile [Análisis Latitudinal de La Intensidad de Lluvias y Precipitación Media Anual En Chile]. Chil. J. Agric. Res. 2012, 72, 252–261. [Google Scholar] [CrossRef]
- Kono, M.; Fukao, Y.; Yamamoto, A. Mountain Building in the Central Andes. J. Geophys. Res. Solid Earth 1989, 94, 3891–3905. [Google Scholar] [CrossRef]
- Sarricolea, P.; Herrera-Ossandon, M.; Meseguer-Ruiz, Ó. Climatic Regionalisation of Continental Chile. J. Maps 2017, 13, 66–73. [Google Scholar] [CrossRef]
- Willems, P. Compound Intensity/Duration/Frequency-Relationships of Extreme Precipitation for Two Seasons and Two Storm Types. J. Hydrol. 2000, 233, 189–205. [Google Scholar] [CrossRef]
- Koutsoyiannis, D.; Kozonis, D.; Manetas, A. A Mathematical Framework for Studying Rainfall Intensity-Duration-Frequency Relationships. J. Hydrol. 1998, 206, 118–135. [Google Scholar] [CrossRef]
- Wayal, A.; Menon, K. Intensity-Duration-Frequency Curves and Regionalisation. International. International. J. Innov. Res. Adv. Eng. 2014, 1, 28–32. [Google Scholar]
- Pereyra-Díaz, D.; Pérez-Sesma, J.; Gómez-Romero, L. Ecuaciones Que Estiman Las Curvas Intensidad-Duración- Periodo de Retorno de La Lluvia. GEOS 2004, 24, 46–56. [Google Scholar]
- Gutiérrez-López, A.; Barragán-Regalado, R. Ajuste de Curvas IDF a Partir de Tormentas de Corta Duración. Tecnol. Cienc. Agua 2019, 10, 01–24. [Google Scholar] [CrossRef]
- Chandra, R.; Saha, U.; Mujumdar, P.P. Model and Parameter Uncertainty in IDF Relationships under Climate Change. Adv. Water Resour. 2015, 79, 127–139. [Google Scholar] [CrossRef]
- Paixao, E.; Mirza, M.M.Q.; Shephard, M.W.; Auld, H.; Klaassen, J.; Smith, G. An Integrated Approach for Identifying Homogeneous Regions of Extreme Rainfall Events and Estimating IDF Curves in Southern Ontario, Canada: Incorporating Radar Observations. J. Hydrol. 2015, 528, 734–750. [Google Scholar] [CrossRef]
- Cao, R.; Zee Ma, Y.; Gomez, E. Geostatistical Applications in Petroleum Reservoir Modelling. J. South. Afr. Inst. Min. Metall. 2014, 114, 625–631. [Google Scholar]
- Liu, W.; Du, P.; Zhao, Z.; Zhang, L. An Adaptive Weighting Algorithm for Interpolating the Soil Potassium Content. Sci. Rep. 2016, 6, 23889. [Google Scholar] [CrossRef] [PubMed]
- Goovaerts, P. Geostatistics for Natural Resources Evaluation; Applied Geostatistics Series; Oxford University Press: New York, NY, USA, 1997; ISBN 978-0-19-511538-3. [Google Scholar]
- Cressie, N.A.C. Statistics for Spatial Data; John Wiley & Sons, Inc.: New York, NY, USA, 1993. [Google Scholar]
- Chiles, J.-P.; Delfiner, P. Geostatistics: Modeling Spatial Uncertainty, 2nd ed.; Wiley Series in Probability and Statistics; Wiley: Hoboken, NJ, USA, 2012; ISBN 978-0-470-18315-1. [Google Scholar]
- Yang, X.; Xie, X.; Liu, D.L.; Ji, F.; Wang, L. Spatial Interpolation of Daily Rainfall Data for Local Climate Impact Assessment over Greater Sydney Region. Adv. Meteorol. 2015, 2015, 563629. [Google Scholar] [CrossRef]
- Chaplot, V.; Darboux, F.; Bourennane, H.; Leguédois, S.; Silvera, N.; Phachomphon, K. Accuracy of Interpolation Techniques for the Derivation of Digital Elevation Models in Relation to Landform Types and Data Density. Geomorphology 2006, 77, 126–141. [Google Scholar] [CrossRef]
- Azpurua, M.A.; Ramos, K.D. A Comparison of Spatial Interpolation Methods for Estimation of Average Electromagnetic Field Magnitude. Prog. Electromagn. Res. M 2010, 14, 135–145. [Google Scholar] [CrossRef]
- Yasrebi, J.; Fathi, H.; Karimian, N.; Moazallahi, M.; Gazni, R. Evaluation and Comparison of Ordinary Kriging and Inverse Distance Weighting Methods for Prediction of Spatial Variability of Some Soil Chemical Parameters. Res. J. Biol. Sci. 2009, 4, 93–102. [Google Scholar]
- Setianto, A.; Triandini, T. Comparison of Kriging and Inverse Distance Weighted (IDW) Interpolation Methods in Lineament Extraction and Analysis. J. Appl. Geol. 2013, 5. [Google Scholar] [CrossRef]
- Lark, R.M. Estimating Variograms of Soil Properties by the Method-of-Moments and Maximum Likelihood: Estimating Variograms. Eur. J. Soil Sci. 2000, 51, 717–728. [Google Scholar] [CrossRef]
- Lobato-Sanchez, R.; Aparicio-Mijares, F.; Sosa-Chinas, M.; Mendoza-Uribe, I. Caracterización Espacial de Redes Pluviográficas: Caso de La Cuenca de La Presa Peñitas. Tecnol. Cienc. Agua 2012, 3, 103–121. [Google Scholar]
- Moncho, R.; Belda, F.; Caselles, V. Climatic Study of the Exponent “n” in IDF Curves: Application for the Iberian Peninsula. Tethys J. Weather Clim. West. Mediterr. 2009, 6, 3–14. [Google Scholar] [CrossRef]
- Szolgay, J.; Parajka, J.; Kohnová, S.; Hlavčová, K. Comparison of Mapping Approaches of Design Annual Maximum Daily Precipitation. Atmos. Res. 2009, 92, 289–307. [Google Scholar] [CrossRef]
- Shehu, B.; Willems, W.; Stockel, H.; Thiele, L.; Haberlandt, U. Regionalisation of Rainfall Depth-Duration-Frequency Curves in Germany. Hydrometeorol./Model. Approaches 2022. [Google Scholar] [CrossRef]
- Teegavarapu, R.S.V. Floods in a Changing Climate: Hydrologic Modeling; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar]
- Pebesma, E.J. Multivariable Geostatistics in S: The Gstat Package. Comput. Geosci. 2004, 30, 683–691. [Google Scholar] [CrossRef]
- Papari, G.; Petkov, N. Reduced Inverse Distance Weighting Interpolation for Painterly Rendering. In Computer Analysis of Images and Patterns; Jiang, X., Petkov, N., Eds.; Lecture Notes in Computer Science; Springer Berlin Heidelberg: Berlin/Heidelberg, Germany, 2009; Volume 5702, pp. 509–516. ISBN 978-3-642-03766-5. [Google Scholar]
- Yao, X.; Fu, B.; Lü, Y.; Sun, F.; Wang, S.; Liu, M. Comparison of Four Spatial Interpolation Methods for Estimating Soil Moisture in a Complex Terrain Catchment. PLoS ONE 2013, 8, e54660. [Google Scholar] [CrossRef] [PubMed]
- Almasi, A.; Jalalian, A.; Toomanian, N. Using OK and IDW Methods for Prediction the Spatial Variability of A Horizon Depth and OM in Soils of Shahrekord, Iran. J. Environ. Earth Sci. 2014, 4, 17–27. [Google Scholar]
- Dodson, R.; Marks, D. Daily Air Temperature Interpolated at High Spatial Resolution over a Large Mountainous Region. Clim. Res. 1997, 8, 1–20. [Google Scholar] [CrossRef]
- Villatoro, M.; Henríquez, C.; Sancho, F. Comparación de Los Interpoladores IDW y Kriging En La Variación Espacial de Ph, ca, Cice y p Del Suelo. Agron. Costarric. 2008, 32, 95–105. [Google Scholar]
- Gong, G.; Mattevada, S.; O’Bryant, S.E. Comparison of the Accuracy of Kriging and IDW Interpolations in Estimating Groundwater Arsenic Concentrations in Texas. Environ. Res. 2014, 130, 59–69. [Google Scholar] [CrossRef]
- Becerra Oviedo, J.A.; Sánchez Mazorca, L.F.; Acosta Castellano, P.M.; Díaz Arévalo, J.L. Regionalización de Curvas IDF Para El Uso de Modelos Hidrometeorológicos En La Sabana Occidental Del Departamento de Cundinamarca. Ing. Región 2015, 14, 143. [Google Scholar] [CrossRef]
- Kumari, M.; Basistha, A.; Bakimchandra, O.; Singh, C.K. Comparison of Spatial Interpolation Methods for Mapping Rainfall in Indian Himalayas of Uttarakhand Region. In Geostatistical and Geospatial Approaches for the Characterization of Natural Resources in the Environment; Raju, N.J., Ed.; Springer International Publishing: Cham, Switzerland, 2016; pp. 159–168. ISBN 978-3-319-18662-7. [Google Scholar]
- Nash, J.E.; Sutcliffe, J.V. River Flow Forecasting through Conceptual Models Part I—A Discussion of Principles. J. Hydrol. 1970, 10, 282–290. [Google Scholar] [CrossRef]
- Esquivel, G.; Sánchez, I.; Velásquez, M.; López, A.; López, R.; Bueno, P. Modelación Del Escurrimiento En Una Subcuenca Del Trópico Húmedo de México y Su Análisis Mediante Indices de Eficiencia Predictiva. Agrofaz 2013, 13, 113–118. [Google Scholar]
- Moriasi, D.N.; Arnold, J.G.; van Liew, M.W.; Bingner, R.L.; Harmel, R.D.; Veith, T.L. Veith Model Evaluation Guidelines for Systematic Quantification of Accuracy in Watershed Simulations. Trans. ASABE 2007, 50, 885–900. [Google Scholar] [CrossRef]
- Beard, L. Statistical Methods in Hydrology; U.S. Army Engineer District Corps of Engineers: Sacramento, CA, USA, 1962. [Google Scholar]
- Torres, V.; Barbosa, I.; Meyer, R.; Noda, A.; Sarduy, L. Criterios de Bondad de Ajuste En La Selección de Modelos No Lineales En La Descripción de Comportamientos Biológicos. Rev. Cuba. Cienc. Agrícola 2012, 46, 345–350. [Google Scholar]
- Fagerland, M.W. T-Tests, Non-Parametric Tests, and Large Studies—A Paradox of Statistical Practice? BMC Med. Res. Methodol. 2012, 12, 78. [Google Scholar] [CrossRef]
- Nachar, N. The Mann-Whitney U: A Test for Assessing Whether Two Independent Samples Come from the Same Distribution. Tutor. Quant. Methods Psychol. 2008, 4, 13–20. [Google Scholar] [CrossRef]
- Yue, S.; Pilon, P.; Cavadias, G. Power of the Mann–Kendall and Spearman’s Rho Tests for Detecting Monotonic Trends in Hydrological Series. J. Hydrol. 2002, 259, 254–271. [Google Scholar] [CrossRef]
- Liu, G.; Yang, X. Spatial Variability Analysis of Soil Properties within a Field. In Computer and Computing Technologies in Agriculture, Volume II; Li, D., Ed.; The International Federation for Information Processing; Springer: Boston, MA, USA, 2008; Volume 259, pp. 1341–1344. ISBN 978-0-387-77252-3. [Google Scholar]
- Weber, D.; Englund, E. Evaluation and Comparison of Spatial Interpolators. Math. Geol. 1992, 24, 381–391. [Google Scholar] [CrossRef]
- Gotway, C.A.; Ferguson, R.B.; Hergert, G.W.; Peterson, T.A. Comparison of Kriging and Inverse-Distance Methods for Mapping Soil Parameters. Soil Sci. Soc. Am. J. 1996, 60, 1237–1247. [Google Scholar] [CrossRef]
- Li, J.; Heap, A.D. A Review of Comparative Studies of Spatial Interpolation Methods in Environmental Sciences: Performance and Impact Factors. Ecol. Inform. 2011, 6, 228–241. [Google Scholar] [CrossRef]
- Hershfield, D. Rainfall Frequency Atlas of the United States for Durations from 30 Minutes to 24 Hour and Return Periods from 1 to 100 Years; Technical Paper; Department of Commerce: Washington, DC, USA, 1961. [Google Scholar]
- Basumatary, V.; Sil, B. Generation of Rainfall Intensity-Duration-Frequency Curves for the Barak River Basin. Meteorol. Hydrol. Water Manag. 2017, 6, 47–57. [Google Scholar] [CrossRef]
- Alemaw, B.F.; Chaoka, R.T. Regionalization of Rainfall Intensity-Duration-Frequency (IDF) Curves in Botswana. J. Water Resour. Prot. 2016, 8, 1128–1144. [Google Scholar] [CrossRef][Green Version]
- Rodríguez-Solà, R.; Casas-Castillo, M.C.; Navarro, X.; Redaño, Á. A Study of the Scaling Properties of Rainfall in Spain and Its Appropriateness to Generate Intensity-Duration-Frequency Curves from Daily Records: A study of scaling rainfall properties in Spain to generate IDF curves. Int. J. Climatol. 2017, 37, 770–780. [Google Scholar] [CrossRef]
- Puricelli, M. Rainfall Extremes Modeling under Shortage of Data and Uncertainty in the Pampean Region (Argentina). Cuad. Investig. Geográfica 2018, 44, 719. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sangüesa, C.; Pizarro, R.; Ingram, B.; Ibáñez, A.; Rivera, D.; García-Chevesich, P.; Pino, J.; Pérez, F.; Balocchi, F.; Peña, F. Comparing Methods for the Regionalization of Intensity−Duration−Frequency (IDF) Curve Parameters in Sparsely-Gauged and Ungauged Areas of Central Chile. Hydrology 2023, 10, 179. https://doi.org/10.3390/hydrology10090179
Sangüesa C, Pizarro R, Ingram B, Ibáñez A, Rivera D, García-Chevesich P, Pino J, Pérez F, Balocchi F, Peña F. Comparing Methods for the Regionalization of Intensity−Duration−Frequency (IDF) Curve Parameters in Sparsely-Gauged and Ungauged Areas of Central Chile. Hydrology. 2023; 10(9):179. https://doi.org/10.3390/hydrology10090179
Chicago/Turabian StyleSangüesa, Claudia, Roberto Pizarro, Ben Ingram, Alfredo Ibáñez, Diego Rivera, Pablo García-Chevesich, Juan Pino, Felipe Pérez, Francisco Balocchi, and Francisco Peña. 2023. "Comparing Methods for the Regionalization of Intensity−Duration−Frequency (IDF) Curve Parameters in Sparsely-Gauged and Ungauged Areas of Central Chile" Hydrology 10, no. 9: 179. https://doi.org/10.3390/hydrology10090179
APA StyleSangüesa, C., Pizarro, R., Ingram, B., Ibáñez, A., Rivera, D., García-Chevesich, P., Pino, J., Pérez, F., Balocchi, F., & Peña, F. (2023). Comparing Methods for the Regionalization of Intensity−Duration−Frequency (IDF) Curve Parameters in Sparsely-Gauged and Ungauged Areas of Central Chile. Hydrology, 10(9), 179. https://doi.org/10.3390/hydrology10090179








