A Novel Approach to Optimize the Industrial Process of Membrane Concentration of Grape Musts
Abstract
1. Introduction
2. Material and Methods
2.1. Materials
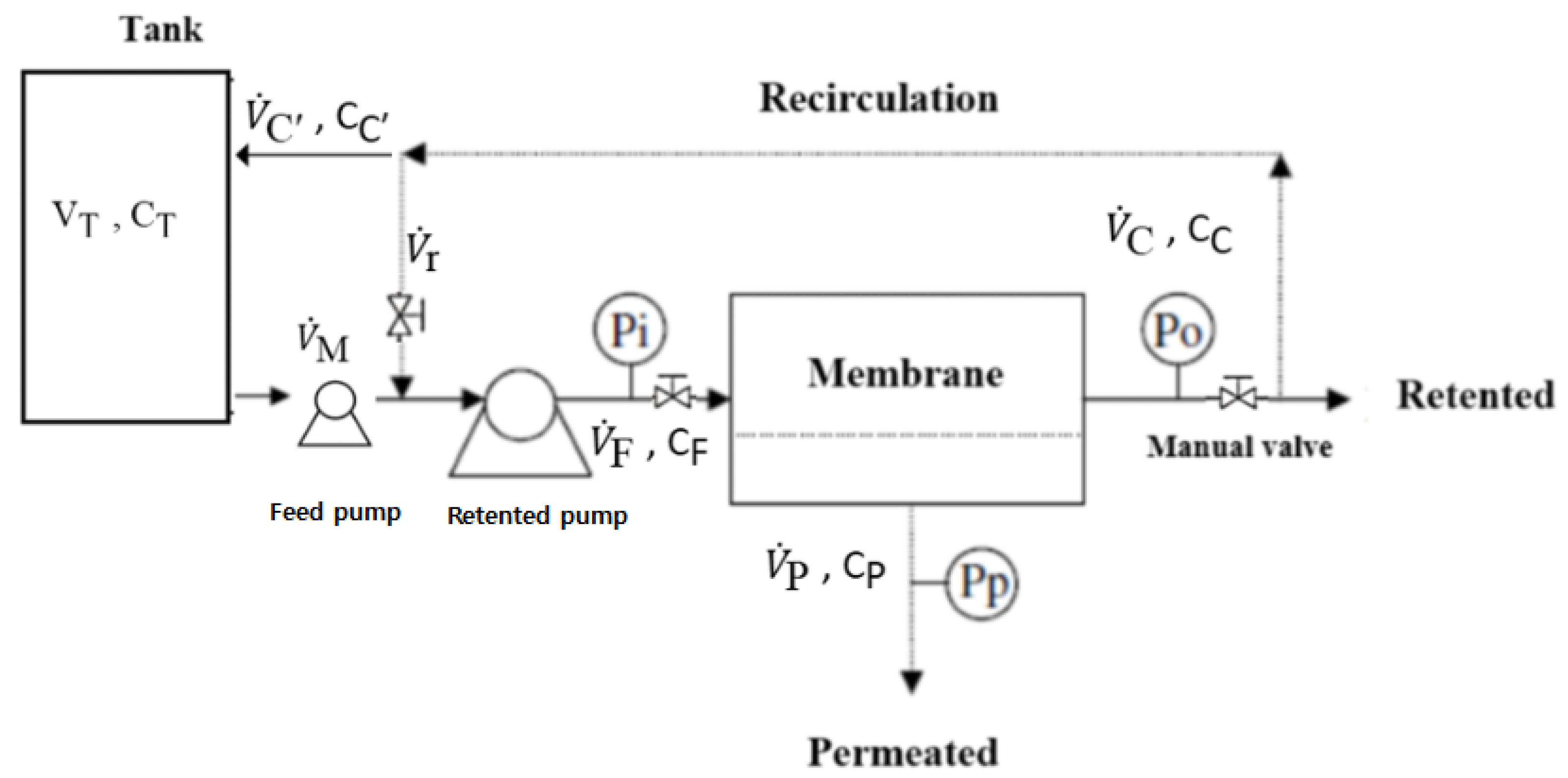
2.2. Membrane Lab-Scale Plants
- Pretretment
- Nanofiltration membranes
- Reverse osmosis membranes
- Industrial-scale plant fitted with NF or RO membranes
Industrial-Scale Plant Fitted with NF or RO Membranes
2.3. Fouling Mechanisms
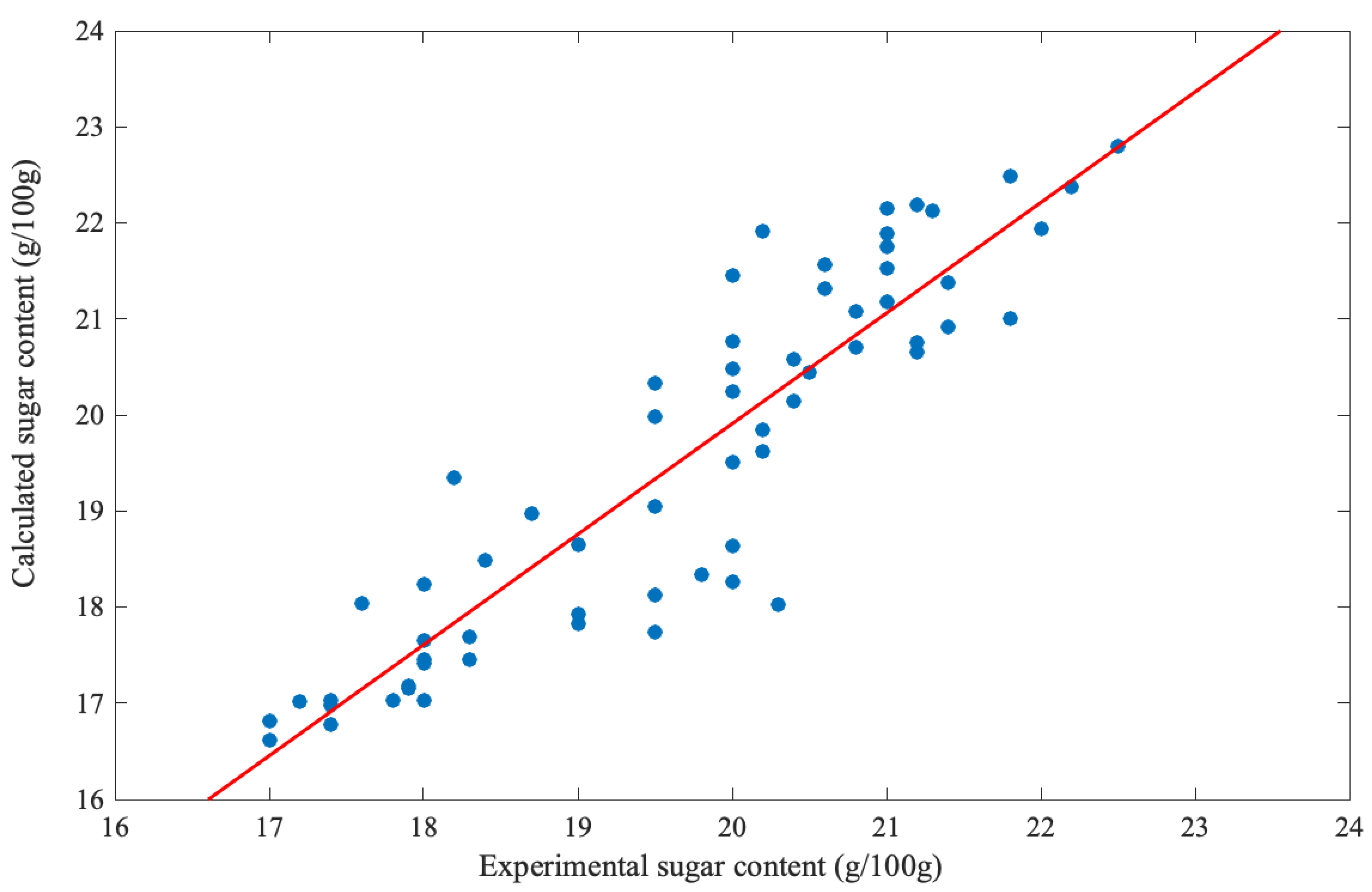
2.4. Industrial Plant Modeling
3. Results and Discussion
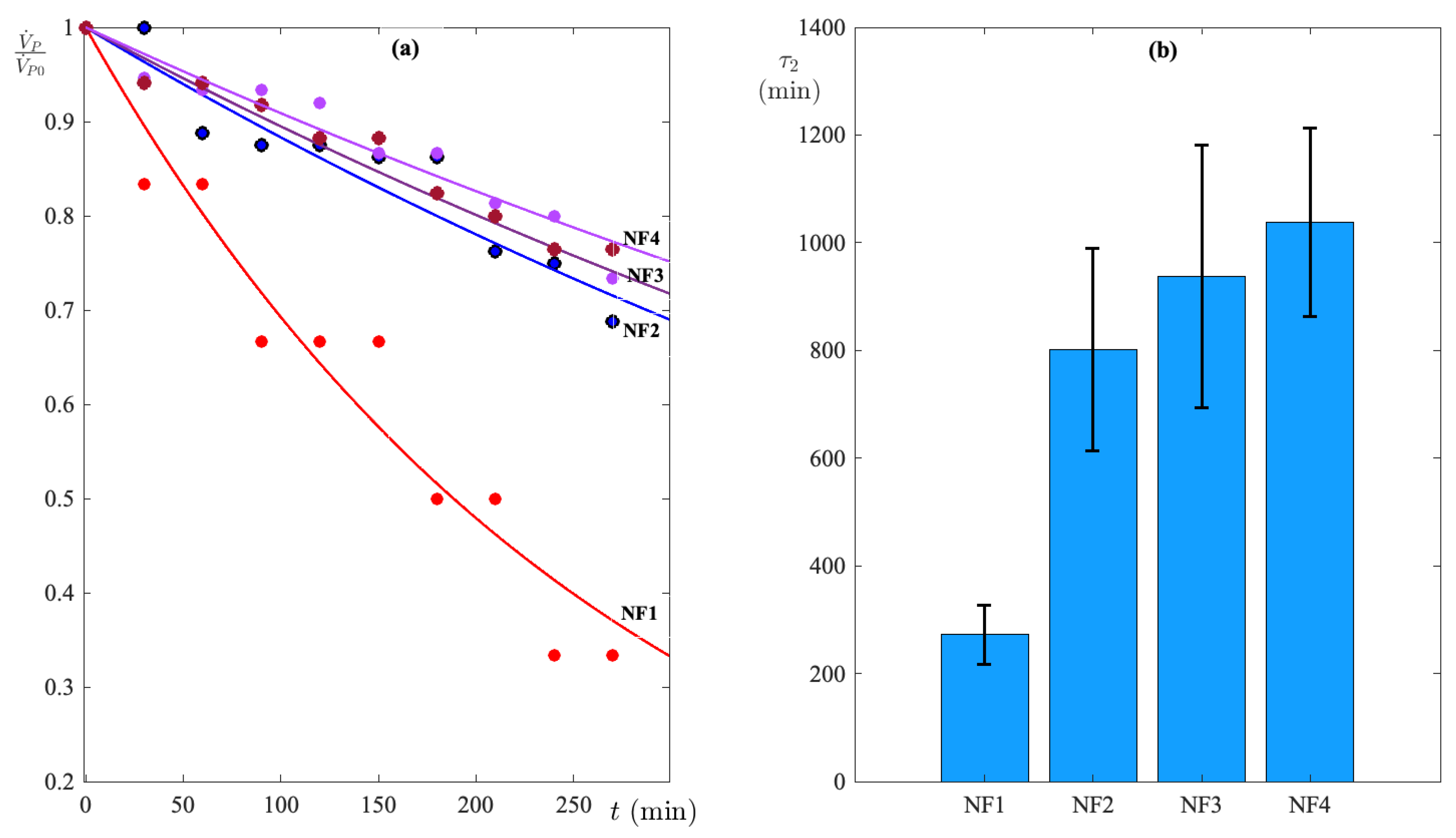
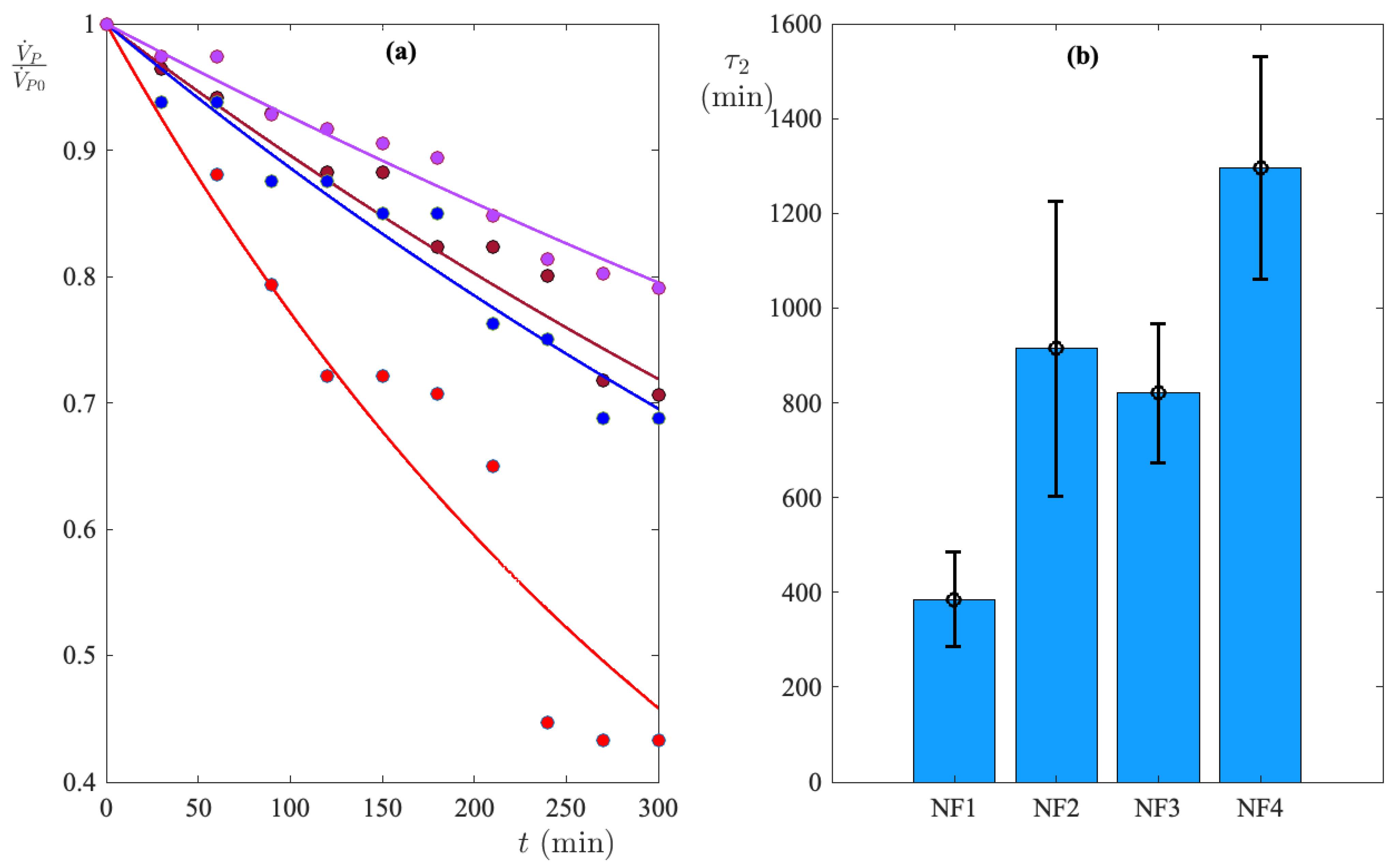
3.1. Test Selection of Membranes
3.1.1. Nanofiltration
3.1.2. Reverse Osmosis
3.2. Test Results on the Industrial Plants
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Jiao, B.; Cassano, A.; Drioli, E. Recent advances on membrane processes for the concentration of fruit juices: A review. J. Food Eng. 2004, 63, 303–324. [Google Scholar] [CrossRef]
- Mietton-Peuchot, M.; Milisic, V.; Noilet, P. Grape must concentration by using reverse osmosis. Comparison with chaptalization. Desalination 2002, 148, 125–129. [Google Scholar] [CrossRef]
- Drioli, E.; Fontanova, E. Membrane technology and sustainable growth. Chem. Eng. Res. Des. 2004, 82, 1557–1562. [Google Scholar] [CrossRef]
- Gurak, P.D.; Cabral, L.M.C.; Rocha-Leão, M.H.M.; Matta, V.M.; Freitas, S.P. Quality evaluation of grape juice concentrated by reverse osmosis. J. Food Eng. 2010, 96, 421–426. [Google Scholar] [CrossRef]
- Grace, H.P.; Fontanova, E. Structure and performance of filter media. II. Performance of filter media in liquid service. AIChE J. 1956, 2, 316–336. [Google Scholar] [CrossRef]
- Hermia, J. Constant pressure blocking filtration laws: Application to power-law non-Newtonian fluids. Inst. Chem. Eng. 1982, 60, 183–187. [Google Scholar]
- Ho, C.-C.; Zydney, A.L. A combined pore blockage and cake filtration model for protein fouling during microfiltration. J. Colloid Interface Sci. 2000, 232, 389–399. [Google Scholar] [CrossRef] [PubMed]
- Iritani, E.; Katagiri, N. Developments of blocking filtration model in membrane filtration. KONA Powder Part. J. 2016, 33, 179–202. [Google Scholar] [CrossRef]
- Di Biasio, A.; Ambrosone, L.; Cametti, C. Numerical simulation of dielectric spectra of aqueous suspensions of non-spheroidal differently shaped biological cells. J. Phys. D Appl. Phys. 2008, 42, 025401–025410. [Google Scholar] [CrossRef]
- Notarnicola, B.; Tassielli, G.; Renzulli, P.A. Environmental and technical improvement of a grape must concentration system via a life cycle approach. J. Clean. Prod. 2015, 89, 87–98. [Google Scholar] [CrossRef]
- Tomaszewska, M.; Orecki, A.; Karakulski, K. Treatment of bilge water using a combination of ultrafiltration and reverse osmosis. Desalination 2005, 185, 203–212. [Google Scholar] [CrossRef]
- Pati, S.; La Notte, D.; Clodoveo, M.L.; Cicco, G.; Esti, M. Reverse osmosis and nanofiltration membranes for the improvement of must quality. Eur. Food Res. Technol. 2014, 239, 595–602. [Google Scholar] [CrossRef]
- Laganà, F.; Barbieri, G.; Drioli, E. Direct contact membrane distillation: Modelling and concentration experiments. J. Membr. Sci. 2000, 166, 1–11. [Google Scholar] [CrossRef]
- Mirsaeedghazi, H.; Emam-Djomeh, Z.; Mousavi, S.M.; Enjileha, V.; Navidbakhsh, M.; Mirhashemi, S.M. Mathematical modelling of mass transfer in the concentration polarisation layer of flat-sheet membranes during clarification of pomegranate juice. Int. J. Food Sci. Technol. 2010, 45, 2096–2100. [Google Scholar] [CrossRef]
- Mulligan, K.; Jakubek, Z.J.; Johnston, L. Supported lipid bilayers on biocompatible polysaccharide multilayers. Langmuir 2011, 27, 14352–14359. [Google Scholar] [CrossRef] [PubMed]
- Mosca, M.; Murgia, S.; Ceglie, A.; Monduzzi, M.; Ambrosone, L. Biocompatible lipid-based liquid crystals and emulsions. J. Phys. Chem. B 2006, 110, 25994–26000. [Google Scholar] [CrossRef] [PubMed]
- Kofránek, J.; Kulhánek, T.; Mateják, M.; Ježek, F.; Šilar, J. Integrative physiology in Modelica. In Proceedings of the 12th International Modelica Conference, Prague, Czech Republic, 15–17 May 2017; pp. 589–603. [Google Scholar]
- Bianchi, B.; Catalano, F.; Oliveto, R.; Ricciardi, S. Dynamic simulation driven design and management of production facilities in agricultural/food industry. Acta Hortic. 2021, 1311, 241–248. [Google Scholar] [CrossRef]
- Venditti, F.; Bufalo, G.; Lopez, F.; Ambrosone, L. Pollutants adsorption from aqueous solutions: The role of the mean lifetime. Chem. Eng. Sci. 2011, 66, 5922–5929. [Google Scholar] [CrossRef]
- Minó, A.; Zeppa, L.; Ambrosone, L. Excess Absorbance as a Novel Approach for Studying the Self-Aggregation of Vital Dyes in Liquid Solution. Int. J. Mol. Sci. 2023, 24, 1645. [Google Scholar] [CrossRef] [PubMed]
- Matsuura, T. Synthetic Membranes and Membrane Separation Processes, 1st ed.; Taylor & Francis: London, UK, 1993. [Google Scholar]
- Jackson, R.S. Wine Science: Principles and Applications, 3rd ed.; Academic Press: Boston, MA, USA, 2008. [Google Scholar]
- Ambrosone, L.; D’Errico, G.; Sartorio, R.; Vitagliano, V. Analysis of velocity cross-correlation and preferential solvation for the system N-methylpyrrolidone–water at 20 °C. J. Chem. Soc. Faraday Trans. 1995, 91, 1339–1344. [Google Scholar] [CrossRef]

| Musts | Density kgm | Sugars °Bx | Dry Extract gL |
|---|---|---|---|
| Black Bombino (lab. and industrial tests) | 1088 | 18.9 ± 0.1 | 181 ± 3 |
| Verdeca (lab. and industrial tests) | 1079 | 19.7 ± 0.1 | 191 ± 2 |
| Trebbiano (industrial tests) | 1062 | 15.8 ± 0.1 | 159 ± 4 |
| Permeate Diameter Pipes Dp (cm) | Retentate Diameter Pipes Dc | Features Centrifugal Pump | Features Volumetric Pump | Total Membrane Area m2 |
|---|---|---|---|---|
| 1.74 | 3 | Flow: up to 520 Lmin Pressure: = 3.0 bar Power: 3.0 kW | Flow: up to 42 Lmin Pressure: bar Power: 11 kW | 55 |
| Bombino | Verdeca | |||
|---|---|---|---|---|
| Membrane | ||||
| RO1 | 42 ± 20 | 0.41 ± 0.06 | 97 ± 41 | 0.34 ± 0.08 |
| RO2 | 116 ± 93 | 0.30 ± 0.2 | 44 ± 30 | 0.46 ± 0.07 |
| RO3 | 109 ± 61 | 0.48 ± 0.09 | 194 ± 113 | 0.31 ± 0.2 |
| Black Bombino | Verdeca | ||||||
|---|---|---|---|---|---|---|---|
| Membrane | P (bar) | Density (kgL) | Sugars (g/100 g) | DryExtract (gL) | Density (kgL) | Sugars (g/100 g) | DryExtract (gL) |
| RO4 | 40 | 1.085 ± 0.001 | 19.5 ± 0.1 | 187 ± 3 | 1.089 ± 0.001 | 21.0 ± 0.2 | 208 ± 2 |
| 50 | 1.086 ± 0.001 | 20.0 ± 0.1 | 191 ± 1 | 1.089 ± 0.001 | 20.6 ± 0.1 | 208 ± 1 | |
| 60 | 1.087 ± 0.001 | 20.0 ± 0.2 | 195 ± 1 | 1.090 ± 0.001 | 21.0 ± 0.2 | 211 ± 3 | |
| 70 | 1.088 ± 0.001 | 20.2 ± 0.1 | 198 ± 2 | 1.091 ± 0.001 | 21.2 ± 0.2 | 215 ± 1 | |
| RO5 | 40 | 1.085 ± 0.001 | 19.5 ± 0.3 | 190 ± 2 | 1.088 ± 0.001 | 20.6 ± 0.2 | 205 ± 2 |
| 50 | 1.087 ± 0.001 | 20.0 ± 0.1 | 194 ± 1 | 1.090 ± 0.001 | 21.0 ± 0.2 | 210 ± 3 | |
| 60 | 1.089 ± 0.001 | 20.0 ± 0.3 | 200 ± 2 | 1.092 ± 0.001 | 21.3 ± 0.1 | 218 ± 4 | |
| 70 | 1.091 ± 0.001 | 20.8 ± 0.1 | 201 ± 2 | 1.093 ± 0.001 | 21.8 ± 0.2 | 220 ± 2 | |
| White Bombino | Trebbiano | ||||||
| RO4 | 40 | 1.078 ± 0.001 | 17.6 ± 0.2 | 179 ± 1 | 1.076 ± 0.001 | 17.4 ± 0.1 | 169 ± 2 |
| 50 | 1.080 ± 0.001 | 18.4 ± 0.3 | 181 ± 4 | 1.077 ± 0.001 | 17.9 ± 0.2 | 176 ± 4 | |
| 60 | 1.080 ± 0.001 | 18.7 ± 0.2 | 182 ± 4 | 1.079 ± 0.001 | 18.3 ± 0.3 | 176 ± 2 | |
| 70 | 1.086 ± 0.001 | 18.2 ± 0.1 | 183 ± 3 | 1.080 ± 0.001 | 18.3 ± 0.1 | 176 ± 6 | |
| RO5 | 40 | 1.079 ± 0.001 | 18.0 ± 0.2 | 178 ± 3 | 1.076 ± 0.001 | 17.9 ± 0.2 | 169 ± 2 |
| 50 | 1.083 ± 0.001 | 19.0 ± 0.2 | 189 ± 3 | 1.079 ± 0.001 | 18.0 ± 0.3 | 176 ± 4 | |
| 60 | 1.085 ± 0.001 | 19.5 ± 0.1 | 192 ± 3 | 1.082 ± 0.001 | 19.5 ± 0.3 | 176 ± 2 | |
| 70 | 1.086 ± 0.001 | 19.8 ± 0.3 | 194 ± 4 | 1.084 ± 0.001 | 20.3 ± 0.1 | 183 ± 1 | |
| Black Bombino | Verdeca | ||||||
|---|---|---|---|---|---|---|---|
| Membrane | P (bar) | Density (kgL) | Sugars (g/100 g) | DryExtract (gL) | Density (kgL) | Sugars (g/100 g) | DryExtract (gL) |
| NF2 | 25 | 1.088 ± 0.001 | 20.2 ± 0.1 | 194 ± 2 | 1.092 ± 0.001 | 21.2 ± 0.1 | 217 ± 5 |
| 30 | 1.089 ± 0.001 | 20.2 ± 0.3 | 196 ± 6 | 1.092 ± 0.001 | 21.2 ± 0.2 | 217 ± 3 | |
| 35 | 1.090 ± 0.001 | 20.4 ± 0.1 | 199 ± 5 | 1.092 ± 0.001 | 21.4 ± 0.2 | 217 ± 3 | |
| 40 | 1.091 ± 0.001 | 20.5 ± 0.2 | 200 ± 3 | 1.093 ± 0.001 | 21.8 ± 0.3 | 220 ± 2 | |
| NF4 | 25 | 1.089 ± 0.001 | 20.4 ± 0.2 | 198 ± 4 | 1.092 ± 0.001 | 21.4 ± 0.1 | 215 ± 4 |
| 30 | 1.090 ± 0.001 | 20.8 ± 0.1 | 199 ± 3 | 1.095 ± 0.001 | 22.0 ± 0.1 | 219 ± 3 | |
| 35 | 1.092 ± 0.001 | 21.0 ± 0.2 | 205 ± 2 | 1.097 ± 0.001 | 22.2 ± 0.1 | 222 ± 6 | |
| 40 | 1.092 ± 0.001 | 21.0 ± 0.1 | 205 ± 2 | 1.099 ± 0.001 | 22.5 ± 0.1 | 229 ± 2 | |
| White Bombino | Trebbiano | ||||||
| NF2 | 25 | 1.074 ± 0.001 | 17.2 ± 0.2 | 163 ± 1 | 1.073 ± 0.001 | 17.0 ± 0.2 | 157 ± 4 |
| 30 | 1.075 ± 0.001 | 17.4 ± 0.2 | 166 ± 2 | 1.074 ± 0.001 | 17.0 ± 0.3 | 160 ± 2 | |
| 35 | 1.076 ± 0.001 | 17.8 ± 0.1 | 169 ± 3 | 1.076 ± 0.001 | 17.4 ± 0.2 | 166 ± 3 | |
| 40 | 1.077 ± 0.001 | 18.0 ± 0.2 | 172 ± 4 | 1.077 ± 0.001 | 18.0 ± 0.1 | 166 ± 4 | |
| NF4 | 25 | 1.079 ± 0.001 | 18.0 ± 0.2 | 178 ± 3 | 1.078 ± 0.001 | 18.0 ± 0.3 | 173 ± 1 |
| 30 | 1.082 ± 0.001 | 19.0 ± 0.3 | 191 ± 6 | 1.084 ± 0.001 | 19.0 ± 0.2 | 188 ± 4 | |
| 35 | 1.085 ± 0.001 | 19.5 ± 0.2 | 195 ± 2 | 1.087 ± 0.001 | 20.0 ± 0.3 | 197 ± 3 | |
| 40 | 1.088 ± 0.001 | 20.0 ± 0.2 | 203 ± 2 | 1.087 ± 0.001 | 20.0 ± 0.3 | 197 ± 4 | |
| Membrane | P (bar) | Black Bombino | Verdeca | White Bombino | Trebbiano |
|---|---|---|---|---|---|
| NF2 | 25 | 15.8 ± 0.4 | 16.8 ± 0.2 | 12.7 ± 0.2 | 13.2 ± 0.3 |
| 30 | 15.0 ± 0.3 | 16.8 ± 0.1 | 12.4 ± 0.1 | 12.6 ± 0.2 | |
| 35 | 14.0 ± 0.2 | 16.5 ± 0.2 | 12.0 ± 0.2 | 12.4 ± 0.3 | |
| 40 | 13.4 ± 0.2 | 16.6 ± 0.1 | 13.0 ± 0.1 | 11.4 ± 0.2 | |
| NF4 | 25 | 9.4 ± 0.2 | 12.4 ± 0.3 | 8.2 ± 0.3 | 8.2 ± 0.3 |
| 30 | 8.4 ± 0.3 | 10.8 ± 0.2 | 7.5 ± 0.2 | 7.8 ± 0.2 | |
| 35 | 8.0 ± 0.2 | 10.4 ± 0.1 | 7.2 ± 0.3 | 7.4 ± 0.1 | |
| 40 | 7.2 ± 0.2 | 10.2 ± 0.1 | 6.8 ± 0.1 | 7.4 ± 0.2 | |
| RO4 | 40 | 5.0 ± 0.2 | 17.2 ± 0.2 | 163 ± 1 | 5.6 ± 0.3 |
| 50 | 3.8 ± 0.2 | 17.4 ± 0.2 | 166 ± 2 | 2.4 ± 0.2 | |
| 60 | 1.2 ± 0.1 | 17.8 ± 0.1 | 169 ± 3 | 1.4 ± 0.2 | |
| 70 | 1.0 ± 0.1 | 18.0 ± 0.2 | 172 ± 4 | 1.2 ± 0.1 | |
| RO5 | 40 | 1.079 ± 0.001 | 18.0 ± 0.2 | 178 ± 3 | 0.4 ± 0.1 |
| 50 | 1.082 ± 0.001 | 19.0 ± 0.3 | 191 ± 6 | 04 ± 0.1 | |
| 60 | 1.085 ± 0.001 | 19.5 ± 0.2 | 195 ± 2 | 0.2 ± 0.1 | |
| 70 | 1.088 ± 0.001 | 20.0 ± 0.2 | 203 ± 2 | 0.2 ± 0.1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bianchi, B.; Molino, B.; Catalano, F.; Giametta, F.; Molino, A.J.; Ambrosone, L. A Novel Approach to Optimize the Industrial Process of Membrane Concentration of Grape Musts. ChemEngineering 2023, 7, 48. https://doi.org/10.3390/chemengineering7030048
Bianchi B, Molino B, Catalano F, Giametta F, Molino AJ, Ambrosone L. A Novel Approach to Optimize the Industrial Process of Membrane Concentration of Grape Musts. ChemEngineering. 2023; 7(3):48. https://doi.org/10.3390/chemengineering7030048
Chicago/Turabian StyleBianchi, Biagio, Bruno Molino, Filippo Catalano, Ferruccio Giametta, Antonio Jacopo Molino, and Luigi Ambrosone. 2023. "A Novel Approach to Optimize the Industrial Process of Membrane Concentration of Grape Musts" ChemEngineering 7, no. 3: 48. https://doi.org/10.3390/chemengineering7030048
APA StyleBianchi, B., Molino, B., Catalano, F., Giametta, F., Molino, A. J., & Ambrosone, L. (2023). A Novel Approach to Optimize the Industrial Process of Membrane Concentration of Grape Musts. ChemEngineering, 7(3), 48. https://doi.org/10.3390/chemengineering7030048







