A Newly Developed Empirical Predictive Model for the Dispersed Phase (DP) Holdup in Rotating Disc Contactors
Abstract
:1. Introduction
2. Experimental Method
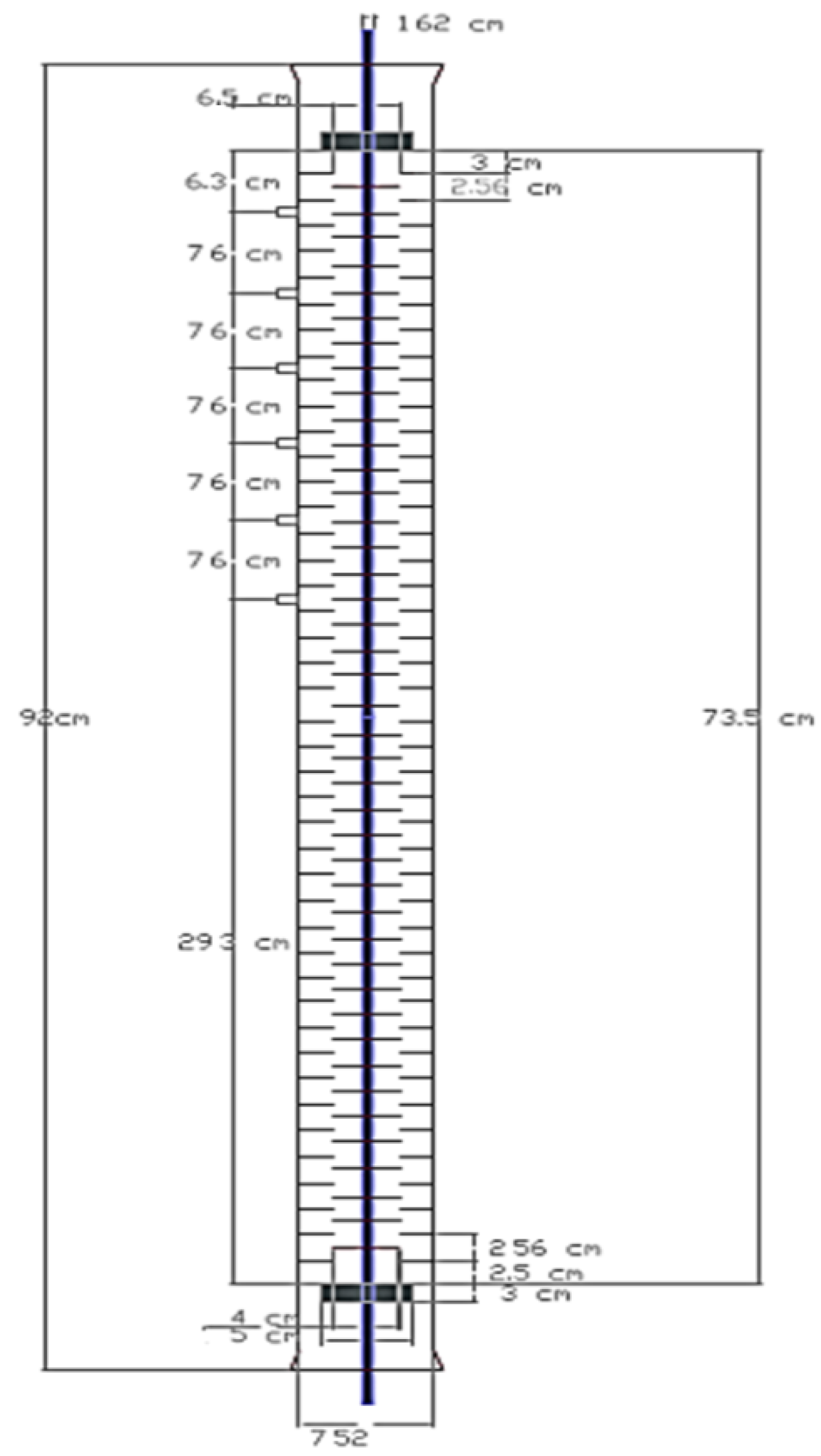
2.1. Experimental Setup
2.2. Experimental Procedure
3. Results and Discussion
3.1. Correlations Proposed
3.2. Applicability of the Proposed Correlations
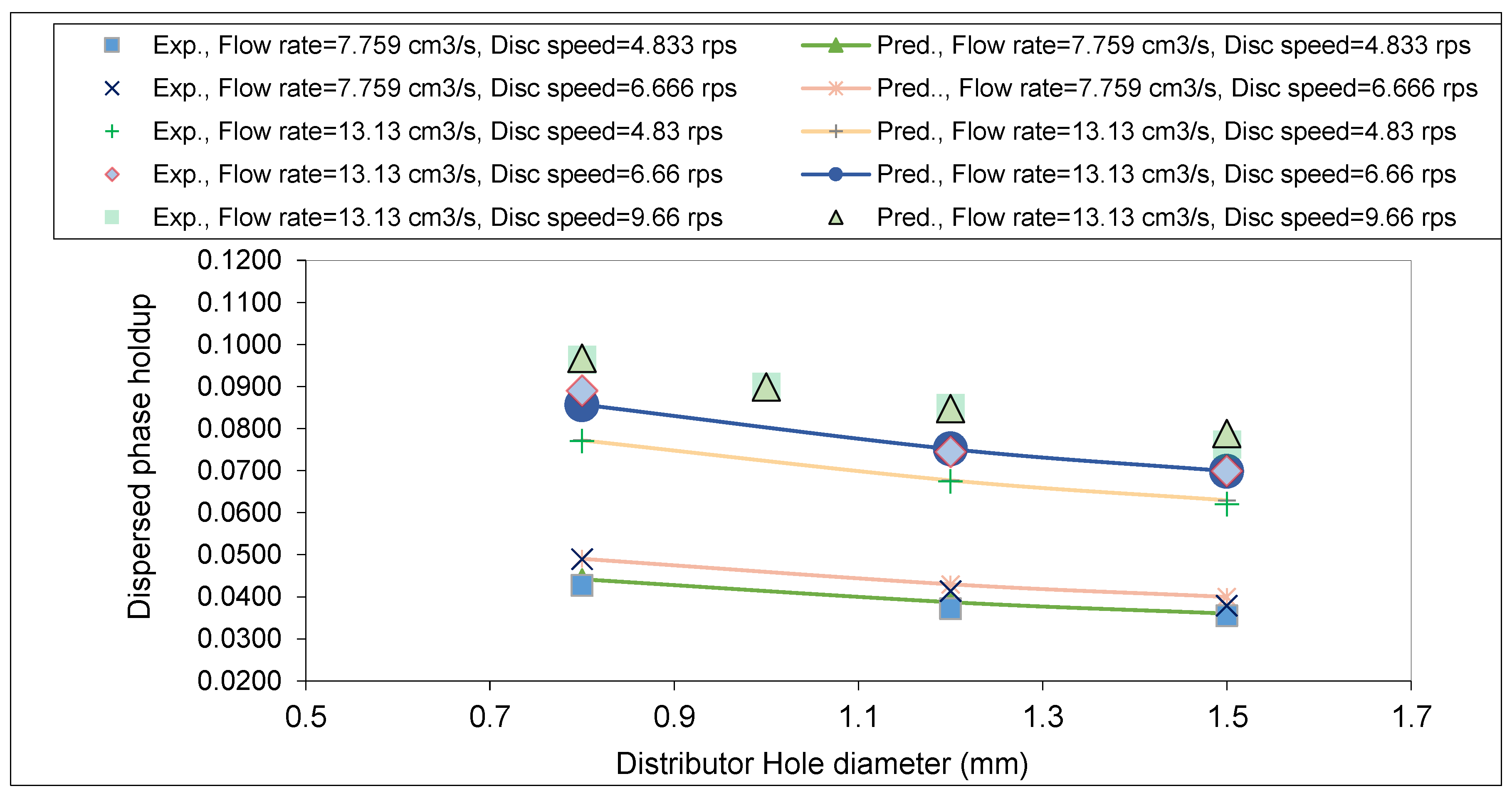
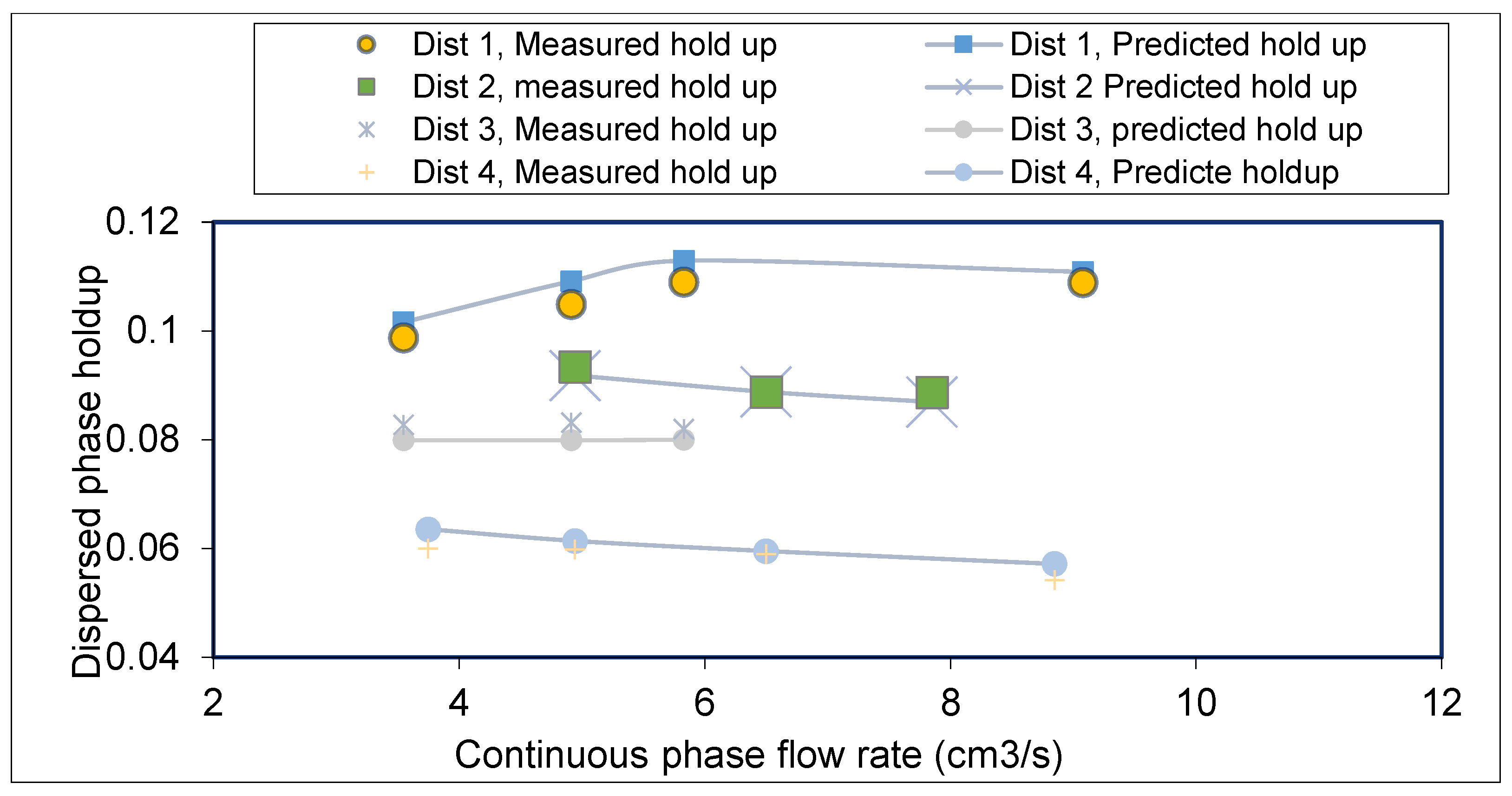
3.2.1. Effect of Dispersed Phase Distributor
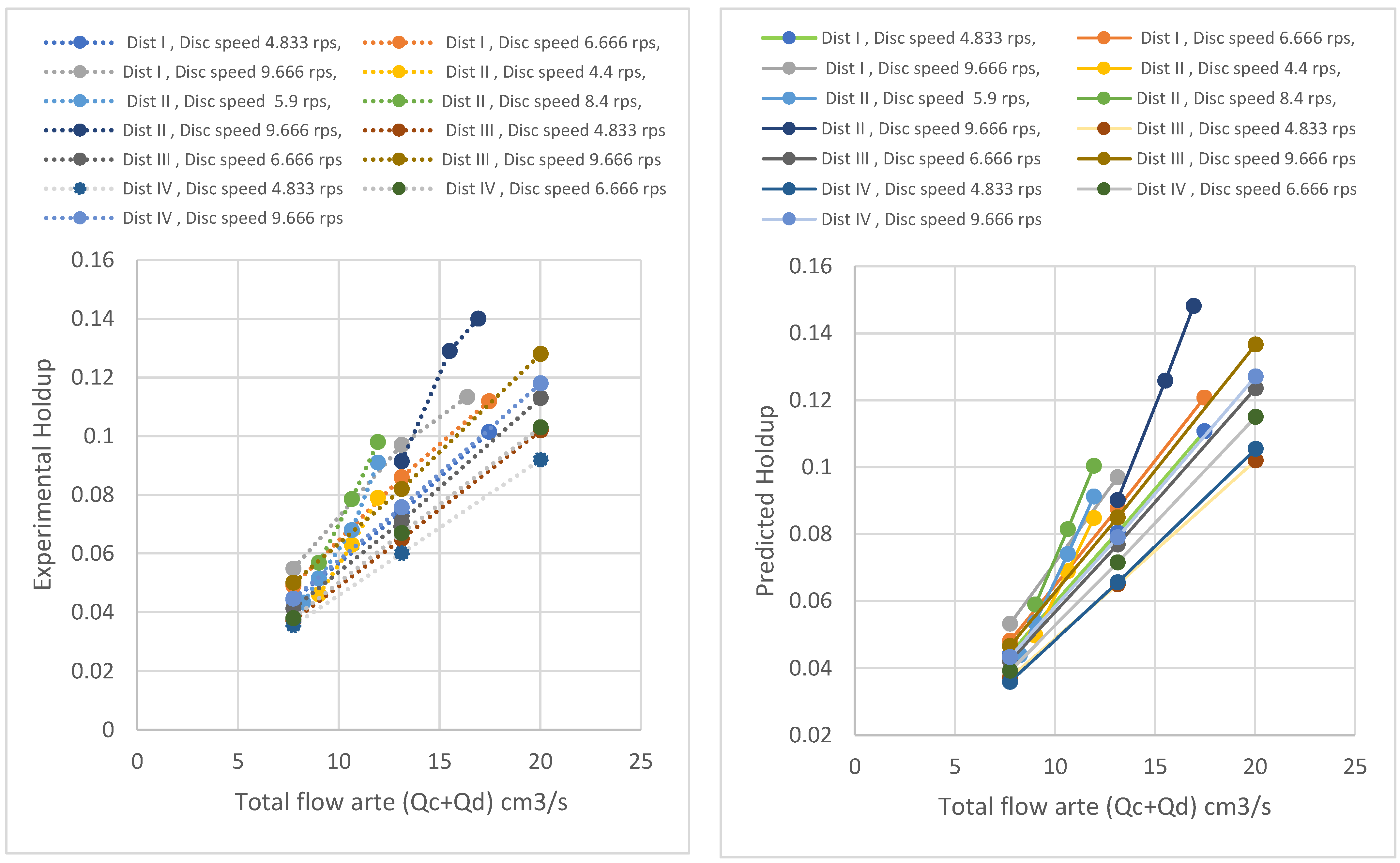
3.2.2. Effect of Flow Rate and Disc Speed
3.2.3. Effect of Column Diameter
3.2.4. Effect of Chemical System
3.2.5. Validating the Holdup Correlation with the Reported Data
3.2.6. Effect of the Geometry Term
3.3. Comparing the Predictions of the New Correlation with Those Reported in the Literature
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| Symbols Used | ||
| Ch | [cm] | Stage height, or compartment height |
| d32 | [mm] | Average mean drop diameter |
| Dc | [cm] | Column diameter |
| D, DR | [cm] | Rotating disc diameter |
| Ds | [cm] | stator opening diameter |
| hd | [cm] | Dispersed phase distributor hole diameter |
| N | [s−1] | Disc speed (revolution per second) |
| Qd | [cm3 s−1] | Dispersed phase flow rate |
| Qc | [cm3 s−1] | Continuous phase flow rate |
| Vd | [cm/s] | dispersed phase superficial velocity |
| Vc | [cm/s] | continuous phase superficial velocity |
| Greek Letters | ||
| [g cm−3] | Continuous phase density | |
| [g cm−3] | Dispersed phase density | |
| [g cm−3] | Density difference () | |
| [g s cm−1] | Continuous phase viscosity | |
| [g s cm−1] | Dispersed phase viscosity | |
| [g s−2] | Interfacial surface tension | |
| g | [cm s−2] | Gravitational acceleration |
Appendix A
| Run No | Disc Speed (rps) | Distributor Hole Diameter (cm) | Continuous Phase Flow Rate (cm3/s) | Dispersed Phase Flow Rate (cm3/s) | Measured Holdup |
|---|---|---|---|---|---|
| 1 | 4.833 | 0.08 | 3.459 | 4.3 | 0.0443 |
| 2 | 6.666 | 0.08 | 3.459 | 4.3 | 0.049 |
| 3 | 9.666 | 0.08 | 3.459 | 4.3 | 0.0548 |
| 4 | 4.833 | 0.08 | 5.83 | 7.3 | 0.0704 |
| 5 | 6.666 | 0.08 | 5.83 | 7.3 | 0.0896 |
| 6 | 9.666 | 0.08 | 5.83 | 7.3 | 0.1116 |
| 7 | 4.833 | 0.08 | 7.787 | 9.68 | 0.1049 |
| 8 | 6.666 | 0.08 | 7.787 | 9.68 | 0.1184 |
| 9 | 5.9 | 0.08 | 3.87 | 4.3 | 0.0473 |
| 10 | 5.9 | 0.08 | 3.87 | 6.8 | 0.0693 |
| 11 | 5.9 | 0.08 | 3.87 | 8.1 | 0.0846 |
| 12 | 9.666 | 0.08 | 3.55 | 7.3 | 0.09983 |
| 13 | 9.666 | 0.08 | 4.915 | 7.3 | 0.1066 |
| 14 | 9.666 | 0.08 | 5.83 | 7.3 | 0.1116 |
| 15 | 9.666 | 0.08 | 9.08 | 7.3 | 0.11336 |
| 16 | 5.9 | 0.1 | 3.87 | 4.37 | 0.0433 |
| 17 | 9.666 | 0.1 | 3.87 | 4.37 | 0.054 |
| 18 | 11.833 | 0.1 | 3.87 | 4.37 | 0.0653 |
| 19 | 4.5 | 0.1 | 3.85 | 5.17 | 0.0443 |
| 20 | 5.9 | 0.1 | 3.85 | 5.17 | 0.047 |
| 21 | 8.4 | 0.1 | 3.85 | 5.17 | 0.0558 |
| 22 | 4.5 | 0.1 | 3.85 | 6.8 | 0.0598 |
| 23 | 5.9 | 0.1 | 3.85 | 6.8 | 0.0609 |
| 24 | 8.4 | 0.1 | 3.85 | 6.8 | 0.071 |
| 25 | 4.5 | 0.1 | 3.85 | 8.1 | 0.0744 |
| 26 | 5.9 | 0.1 | 3.85 | 8.1 | 0.0761 |
| 27 | 8.4 | 0.1 | 3.85 | 8.1 | 0.0863 |
| 28 | 9.666 | 0.1 | 3.87 | 6.11 | 0.0795 |
| 29 | 10.833 | 0.1 | 3.87 | 6.11 | 0.0856 |
| 30 | 11.833 | 0.1 | 3.87 | 6.11 | 0.0947 |
| 31 | 8.833 | 0.1 | 5.83 | 7.3 | 0.0829 |
| 32 | 9.666 | 0.1 | 5.83 | 7.3 | 0.0914 |
| 33 | 10.833 | 0.1 | 5.83 | 7.3 | 0.0947 |
| 34 | 11.833 | 0.1 | 5.83 | 7.3 | 0.0998 |
| 35 | 9.666 | 0.1 | 5.83 | 9.68 | 0.105 |
| 36 | 10.166 | 0.1 | 5.83 | 9.68 | 0.1245 |
| 37 | 8.833 | 0.1 | 5.83 | 11.1 | 0.1076 |
| 38 | 9.666 | 0.1 | 5.83 | 11.1 | 0.1201 |
| 39 | 8.4 | 0.1 | 3.85 | 5.17 | 0.0558 |
| 40 | 8.4 | 0.1 | 3.85 | 6.8 | 0.071 |
| 41 | 8.4 | 0.1 | 3.85 | 8.1 | 0.0863 |
| 42 | 9.666 | 0.1 | 5.83 | 7.3 | 0.09137 |
| 43 | 9.666 | 0.1 | 5.83 | 9.68 | 0.1049 |
| 44 | 9.666 | 0.1 | 5.83 | 11.1 | 0.1201 |
| 45 | 9.666 | 0.12 | 3.55 | 7.3 | 0.0765 |
| 46 | 9.666 | 0.12 | 4.915 | 7.3 | 0.0772 |
| 47 | 9.666 | 0.12 | 5.83 | 7.3 | 0.0778 |
| 48 | 4.833 | 0.12 | 3.459 | 4.3 | 0.0372 |
| 49 | 6.666 | 0.12 | 3.459 | 4.3 | 0.0413 |
| 50 | 9.666 | 0.12 | 3.459 | 4.3 | 0.0512 |
| 51 | 4.833 | 0.12 | 5.83 | 7.3 | 0.0609 |
| 52 | 6.666 | 0.12 | 5.83 | 7.3 | 0.069 |
| 53 | 9.666 | 0.12 | 5.83 | 7.3 | 0.0778 |
| 54 | 11.833 | 0.12 | 5.83 | 7.3 | 0.112 |
| 55 | 4.833 | 0.12 | 8.93 | 11.1 | 0.0913 |
| 56 | 6.666 | 0.12 | 8.93 | 11.1 | 0.1066 |
| 57 | 9.666 | 0.12 | 8.93 | 11.1 | 0.1218 |
| 58 | 5.9 | 0.15 | 3.87 | 4.3 | 0.0364 |
| 59 | 5.9 | 0.15 | 3.87 | 6.8 | 0.048 |
| 60 | 5.9 | 0.15 | 3.87 | 8.1 | 0.0534 |
| 61 | 4.833 | 0.15 | 3.459 | 4.3 | 0.0355 |
| 62 | 6.666 | 0.15 | 3.459 | 4.3 | 0.0379 |
| 63 | 9.666 | 0.15 | 3.459 | 4.3 | 0.0446 |
| 64 | 4.833 | 0.15 | 5.83 | 7.3 | 0.0602 |
| 65 | 6.666 | 0.15 | 5.83 | 7.3 | 0.0626 |
| 66 | 9.666 | 0.15 | 5.83 | 7.3 | 0.0727 |
| 67 | 4.833 | 0.15 | 8.93 | 11.1 | 0.0879 |
| 68 | 6.666 | 0.15 | 8.93 | 11.1 | 0.0896 |
| 69 | 9.666 | 0.15 | 8.93 | 11.1 | 0.0964 |
References
- Ayham, M.; Al-Rahawi, I.; Salam, K. Influence of inlet distributor and column diameter on hydrodynamic characteristics in RDC liquid extraction column. J. Res. Eng. Technol. 2016, 5, 359–371. [Google Scholar]
- Al-Aswad, K.K.; Mumford, C.J.; Jeffreys, G.V. The Application of Drop Size distribution and Discrete Drop Mass Transfer Distribution and Models to Assess the Performance of a Rotating Disc Contactor. AIChE J. 1985, 31, 1488–1497. [Google Scholar] [CrossRef]
- Kirou, V.I.; Tavlarides, L.L.; Bonnet, J.C.; Tsouris, C. Flooding, hold-up, and drop size measurment in multistage column extractor. AIChE J. 2004, 34, 283–292. [Google Scholar] [CrossRef]
- Slater, M.J. Liquid–Liquid extraction column design. Can. J. Chem. Eng. 1985, 63, 1004–1005. [Google Scholar] [CrossRef]
- Kalaichelvi, P.; Murugesan, T. Dispersed phase hold-up in rotary disc contactor. Bioprocess Biosyst. Eng. 1988, 18, 105–111. [Google Scholar] [CrossRef]
- Korchinsky, W.J.; Ismail, A.M. Mass Transfer Parameters in Rotating Disc Contactors: Influence of Column Diameter. J. Chem. Technol. Biotechnol. 1988, 43, 147–158. [Google Scholar] [CrossRef]
- Johnson, A.I.; Lavergne, E.A.L. Hold-up in liquid-liquid extraction columns. Can. J. Chem. Eng. 2010, 1, 37–41. [Google Scholar]
- Cruz-Pinto, J.J.C.; Korchinsky, W.J.; Al-Husseini, R. I.S.E.C.: Mass Transfer to Non-uniform Dispersions in Countercurrent Flow Liquid-Liquid Extraction Columns. In Proceedings of the ISEC 83, American Institute of Chemical Engineering, Denver, CO, USA, 4 May 1983. [Google Scholar]
- Kumar, A.; Hartland, S. Prediction of dispersed phase hold-up, in rotating disc extractors. Chem. Eng. Comm. 1987, 56, 87–106. [Google Scholar] [CrossRef]
- Kumar, A.; Hartland, S. Independent Prediction of slip velocity and dispersed phase hold-up, in liquid-liquid extraction columns. Can. J. Chem. Eng. 1989, 67, 17–25. [Google Scholar] [CrossRef]
- Kumar, A.; Hartland, S. A Unified Correlation for the Prediction of Dispersed-Phase Hold-Up in Liquid-Liquid Extraction Columns. Ind. Chem. Eng. Res. 1995, 34, 3925–3940. [Google Scholar] [CrossRef]
- Korchinisky, W.J. Liquid-Liquid Extraction column modeling: Is the forward mixing influence is necessary? Chem. Eng. Res. Des. 1992, 70, 333–345. [Google Scholar]
- Murakami, A.; Misonou, A.; Inoue, K. Dispersed Phase Hold-up in a Rotating Disc Extraction Column. Int. Chem. Eng. 1978, 18, 16–22. [Google Scholar]
- Hemmati, A.R.; Shirvani, M.; Torab-Mostaedi, M.; Ghaem, A. Hold-up and Flooding characteristics in a perforated rotating disc contactor (PRDC). RSC Adv. 2015, 5, 63025–63033. [Google Scholar] [CrossRef]
- Al-Rahawi, I.A.M. New predictive correlations for the drop size in RDC liquid–liquid extraction column. Chem. Eng. Technol. 2007, 30, 1–10. [Google Scholar]
- Sá, R.M.; Góis, L.M.N.; Cavalcanti, C.F. Dispersed phase hold-up in a liquid-liquid extraction column. Lat. Am. Appl. Res. 2010, 40, 373–376. [Google Scholar]
- Shahrokh, H. Meisam Torab-Mostaedi2 and Seyed Hamed Mousavi, Prediction of Dispersed Phase Hold-up in Scheibel Extraction Columns by a New Correlation. J. Chem. Pet. Eng. 2017, 51, 105–111. [Google Scholar]
- Leila, S.M.; Hassan, M.M.; Rezvan, T.; Jaber, S. Prediction of the mean drop diameter, dispersed phase hold-up and slip velocity in a horizontal pulsed sieve plate column for uranium stripping from Loaded Alamine 336 by ammonium carbonate. Prog. Nucl. Energy 2019, 117, 103082. [Google Scholar]
- Khanramaki, F.; Safdari, J.; Shirani, A.S.; Torkaman, R. Experimental study on hold-up, characteristic and slip velocities in a horizontal pulsed sieve-plate column and uranium reactive extraction condition in aqueous phase. Prog. Nucl. Energy 2018, 109, 159–170. [Google Scholar] [CrossRef]
- Walter, J.K.; Daryoush, B. Application of ‘Forward Mixing’ Model to the Low Interfacial Tension System n-Butanol-Succinic Acid-Water in Rotating Disc-Contactor Liquid Extraction Columns. J. Chem. Technol. Biotechnol. 1993, 58, 113–122. [Google Scholar]
- Korchinsky, W.J.; Al-Husseini, R. Liquid-liquid extraction column (rotating disc contactor)-model parameters from drop size distribution and solute concentration measurements. J. Chem. Technol. Biotechnol. 1986, 36, 395–409. [Google Scholar] [CrossRef]
- Rinco-Rubio, L.M.; Kumar, A.; Hartland, S. Continuous phase axial dispersion in Wirz extraction column. Chem. Eng. Res. Des. 1995, 73, 33–39. [Google Scholar]

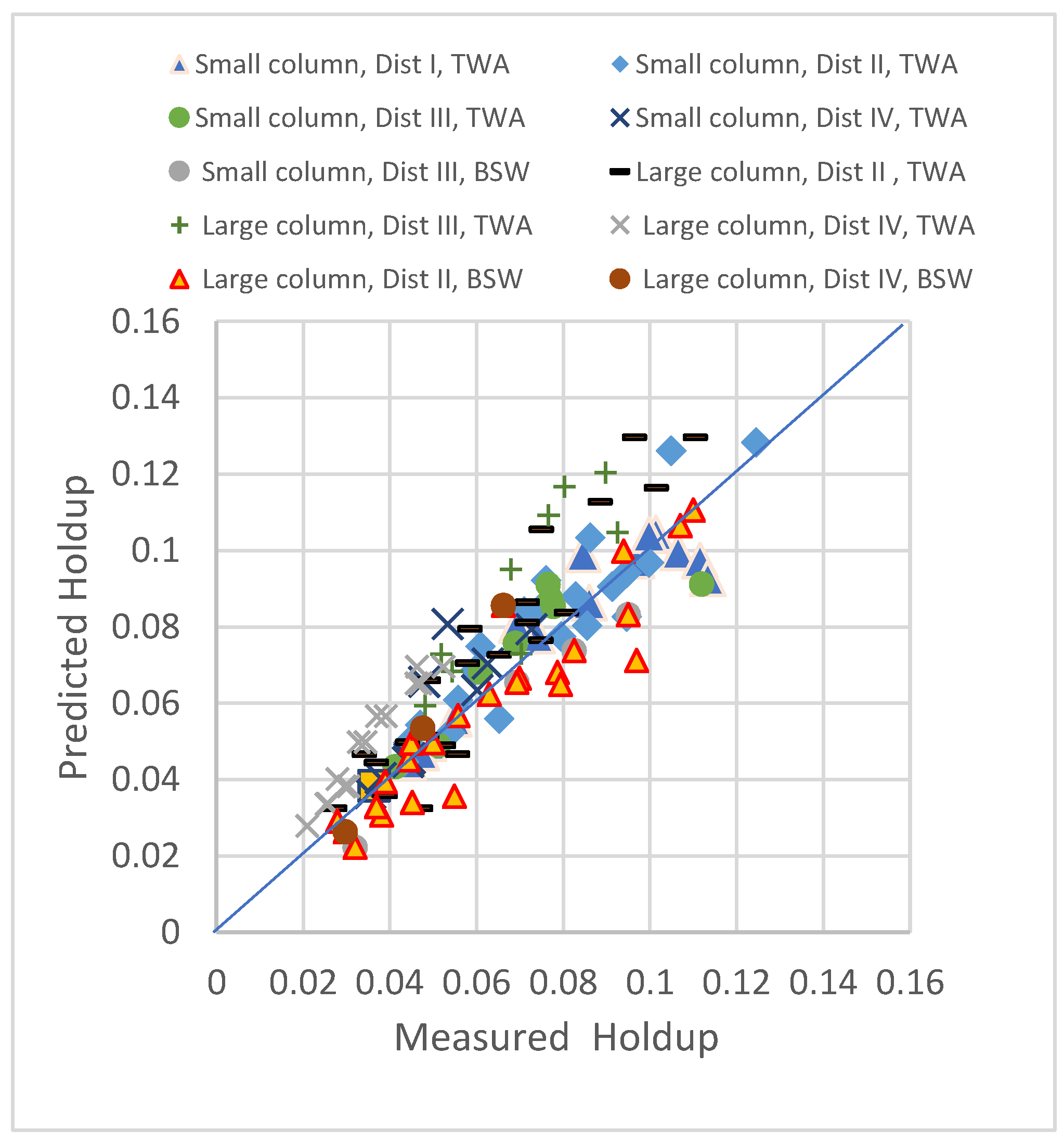


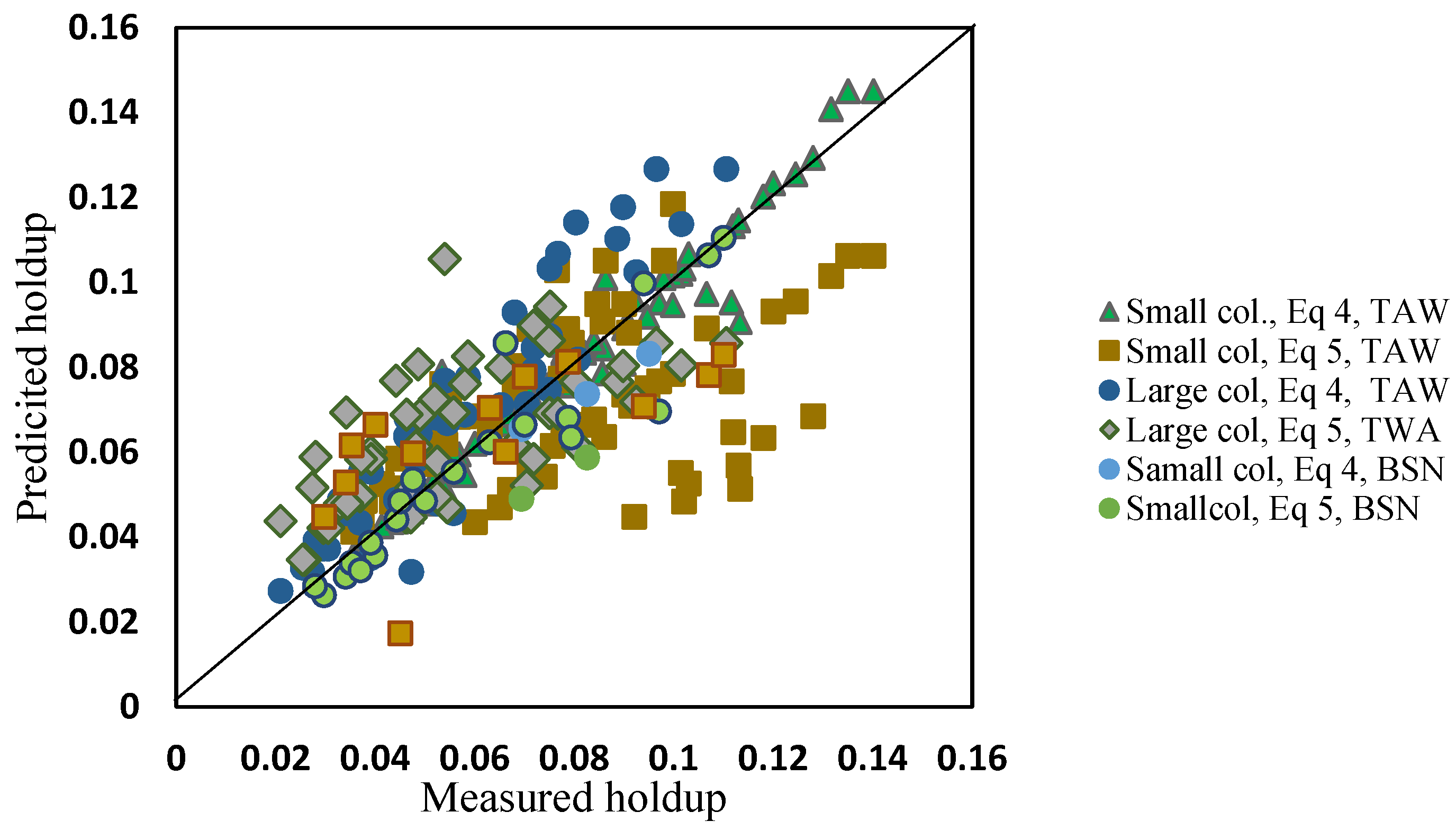

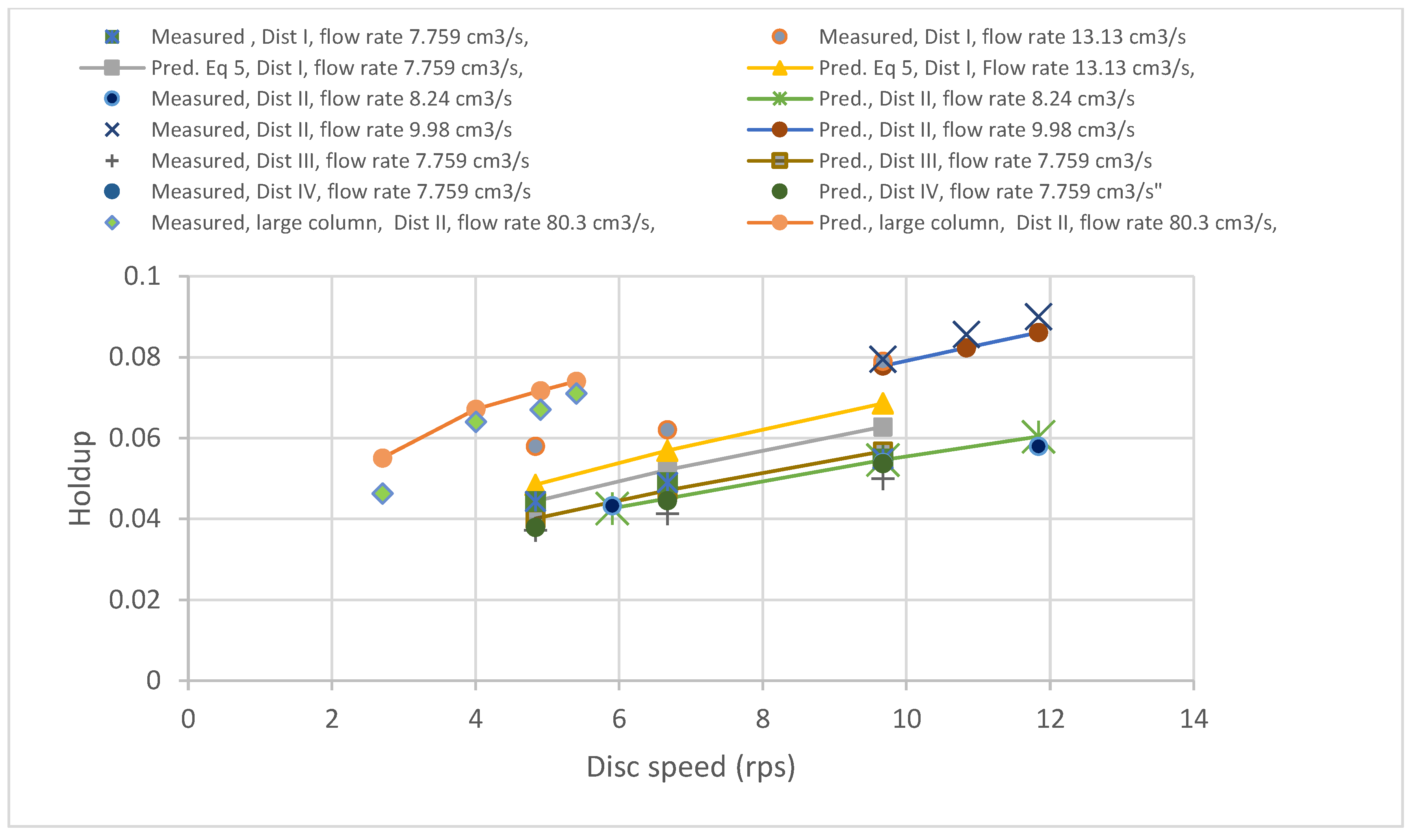

| Mass Transfer Direction | k1 | k2 | n1 | n2 | n3 | n4 |
|---|---|---|---|---|---|---|
| (case 1) No solute transfer | 570.53 | 747.78 | 1.28 | −0.45 | −0.58 | 0.85 |
| (case 2) c→d transfer | 6.4 | 14.08 | 1.28 | −0.16 | −0.68 | 0.29 |
| (case 3) d→c transfer | 19.68 | 60.00 | 0.68 | −0.17 | −0.78 | 0.64 |
| (case 4) General for above three cases | 65.73 | 74.2 | 1.24 | −0.34 | −0.49 | 0.53 |
| Continuous Phase Flow (cm3/s) | Dispersed Phase Flow (cm3/s) | Disc Speed (r.p.s) | Ref. |
|---|---|---|---|
| 3.45–9.45 | 4.15–10.59 | 3.5–11.86 | [1] |
| 19.5–52.3 | 23.456–95.7 | 2.9–6.8 | [8] |
| 11.6–231 | 5.8–126.7 | 3–32 | [9] |
| 32–45 | 25.9–43.27 | 3.67–13.33 | [13] |
| 38.29–95.82 | 72.07–92.54 | 5–7 | [14] |
| 2.57–57.2 | 3.38–80.0 | 1.3–9.7 | [20] |
| 20.2–48.2 | 26.7–90.0 | 4.0–6.3 | [21] |
| 2.76–9.08 | 4.3–11.1 | 4.8–11.83 | This work |
| Distributor No. | A | B | C | D |
|---|---|---|---|---|
| Average hole diameter Dh (mm) | 0.80 | 1.00 | 1.22 | 1.50 |
| Holes number | 19 | 12 | 8 | 5 |
| Hole Area (mm2) | 0.503 | 0.786 | 1.17 | 1.77 |
| Total holes area (mm2) | 9.55 | 9.43 | 9.36 | 8.84 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Al-Rahawi, A.M.I.; Noori, W.A.; Abdulrahman, A.A.; Majdi, H.S.; Salih, I.K.; Alsalhy, Q.F.; Mjalli, F.S. A Newly Developed Empirical Predictive Model for the Dispersed Phase (DP) Holdup in Rotating Disc Contactors. ChemEngineering 2021, 5, 79. https://doi.org/10.3390/chemengineering5040079
Al-Rahawi AMI, Noori WA, Abdulrahman AA, Majdi HS, Salih IK, Alsalhy QF, Mjalli FS. A Newly Developed Empirical Predictive Model for the Dispersed Phase (DP) Holdup in Rotating Disc Contactors. ChemEngineering. 2021; 5(4):79. https://doi.org/10.3390/chemengineering5040079
Chicago/Turabian StyleAl-Rahawi, Ayham M. I., Wallaa A. Noori, Amer A. Abdulrahman, Hasan Sh. Majdi, Issam K. Salih, Qusay F. Alsalhy, and Farouq S. Mjalli. 2021. "A Newly Developed Empirical Predictive Model for the Dispersed Phase (DP) Holdup in Rotating Disc Contactors" ChemEngineering 5, no. 4: 79. https://doi.org/10.3390/chemengineering5040079
APA StyleAl-Rahawi, A. M. I., Noori, W. A., Abdulrahman, A. A., Majdi, H. S., Salih, I. K., Alsalhy, Q. F., & Mjalli, F. S. (2021). A Newly Developed Empirical Predictive Model for the Dispersed Phase (DP) Holdup in Rotating Disc Contactors. ChemEngineering, 5(4), 79. https://doi.org/10.3390/chemengineering5040079








