Design and Investigation of a 3D-Printed Micro-Fluidized Bed
Abstract
1. Introduction
2. Materials and Methods
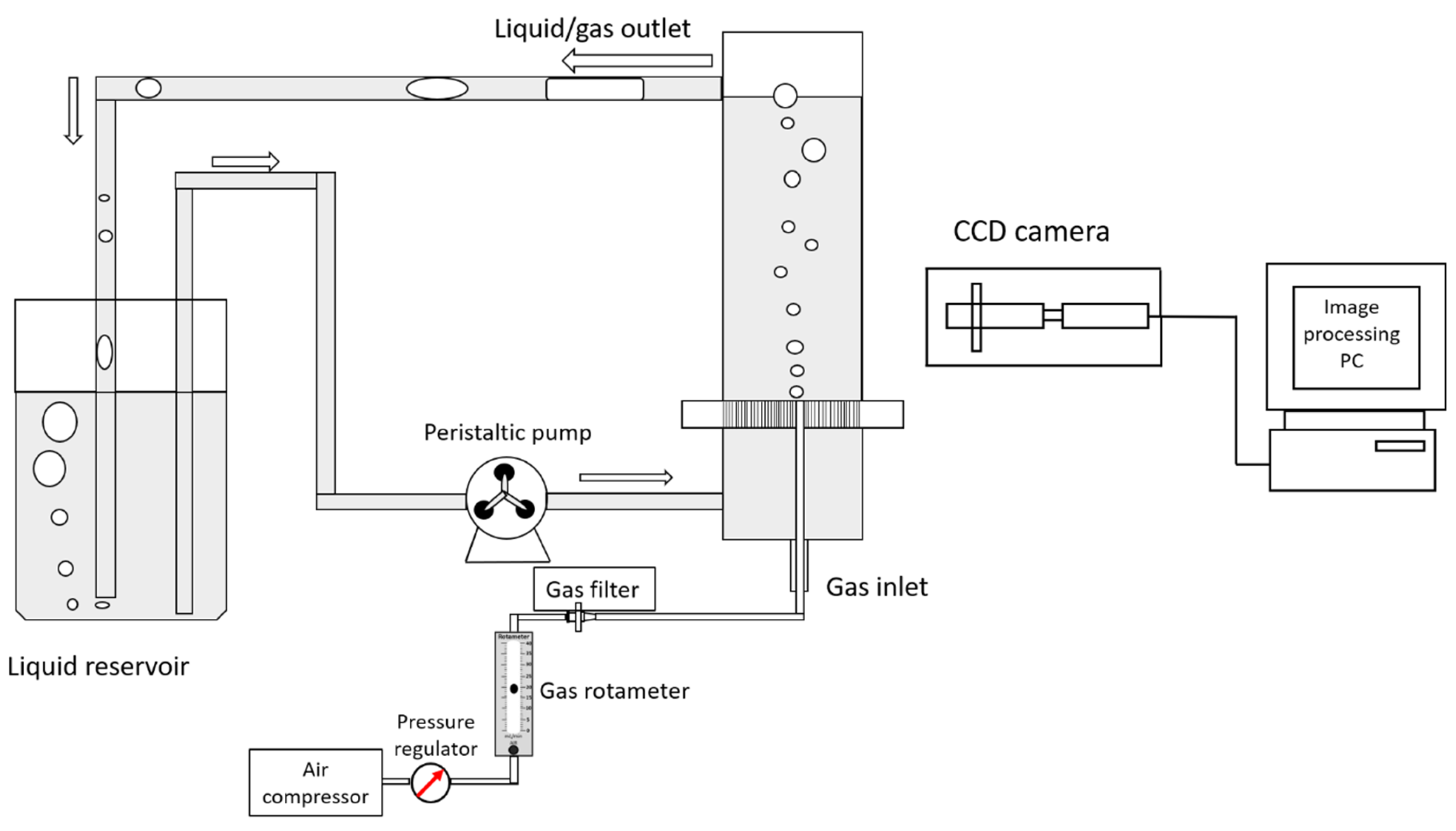
2.1. Fluidized Bed Design and Fabrication
2.2. Materials and Procedures
2.3. Bubbles’ Characterization
2.3.1. Bubble Size
2.3.2. Bubbles’ Velocity
2.3.3. Gas Volume Fraction
2.4. Bed Voidage Characterization
3. Results and Discussion
3.1. Gas–Liquid System
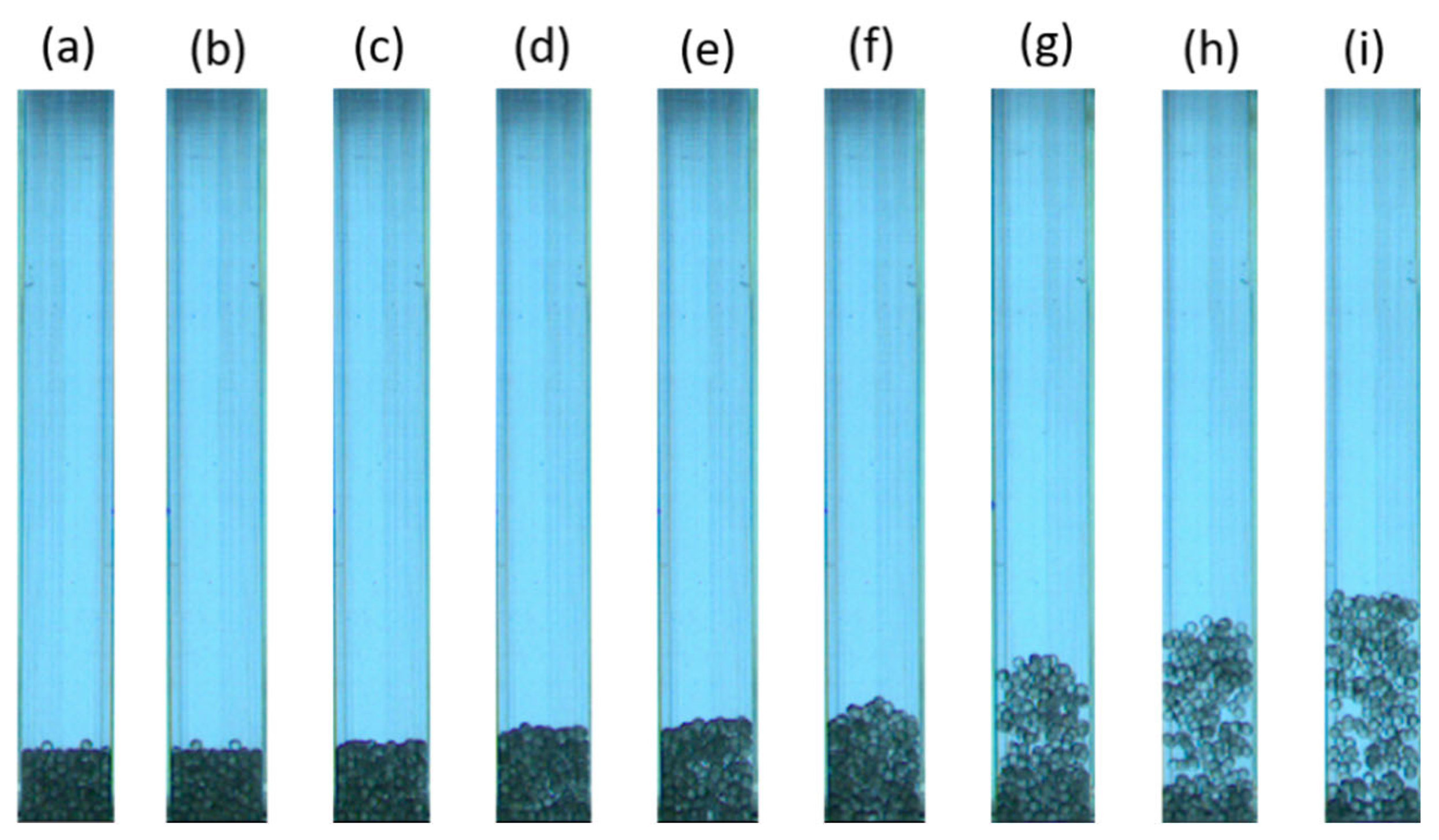
3.1.1. Bubble Flow Regimes
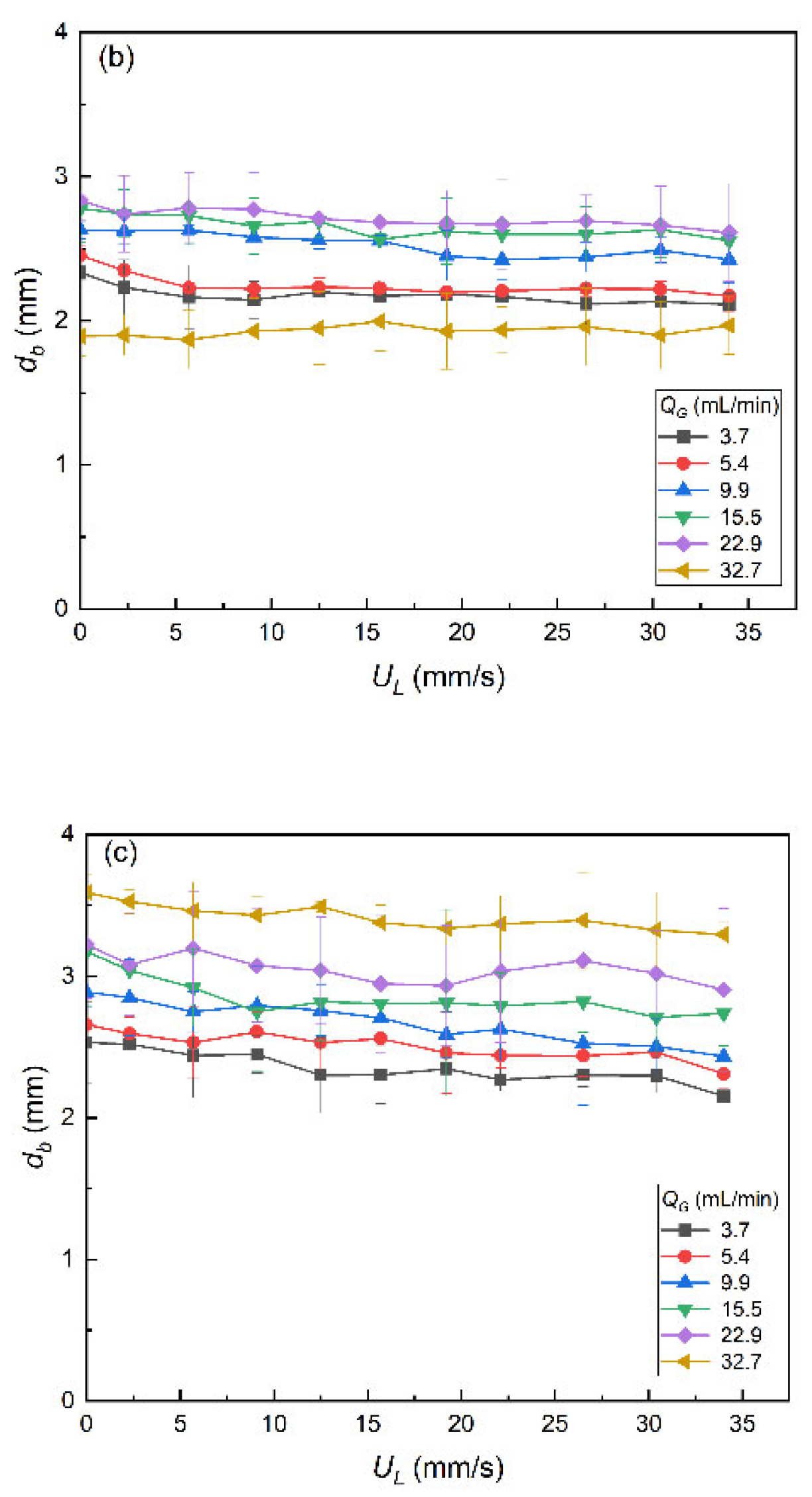
3.1.2. Bubbles’ Size
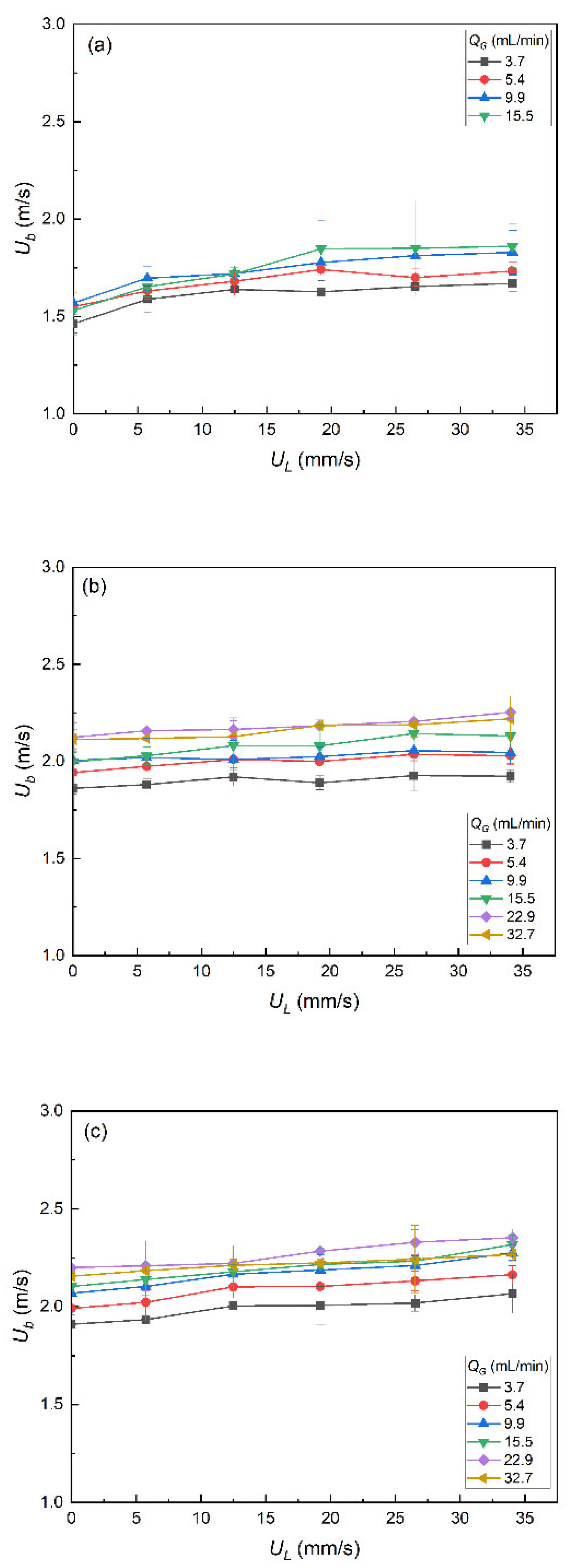
3.1.3. Bubbles’ Velocity
- (1)
- The proximity and size of neighboring bubbles influenced the individual rising bubbles [59].
- (2)
- During the flow regime transition from homogenous bubble regime to heterogeneous bubble regime, bubbles tended to coalesce which impacted each other’s rise velocity [60].
- (3)
- The variations of bubbles’ velocity were observed even for single bubbles of the one size rising in an infinite medium [61].
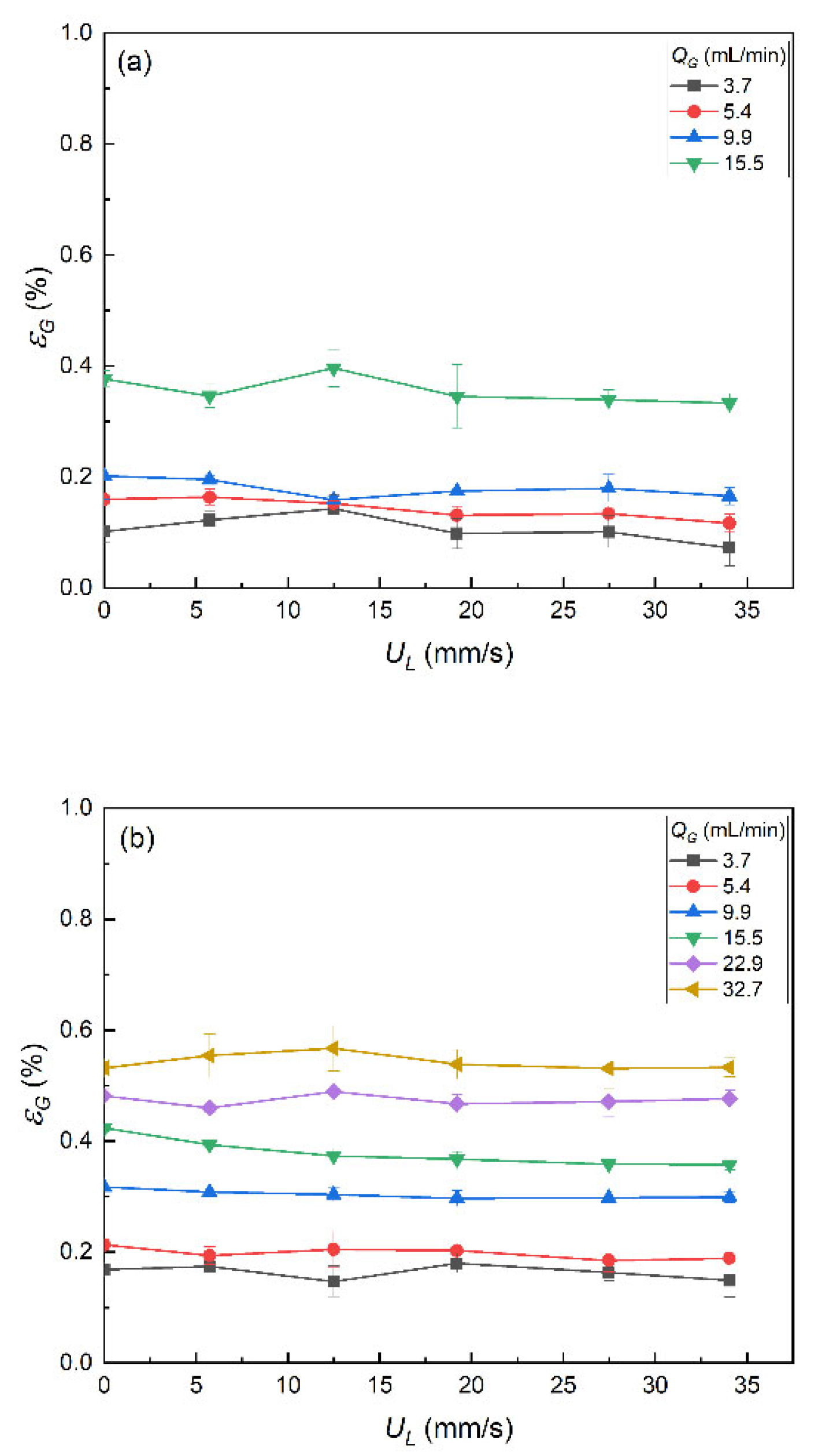
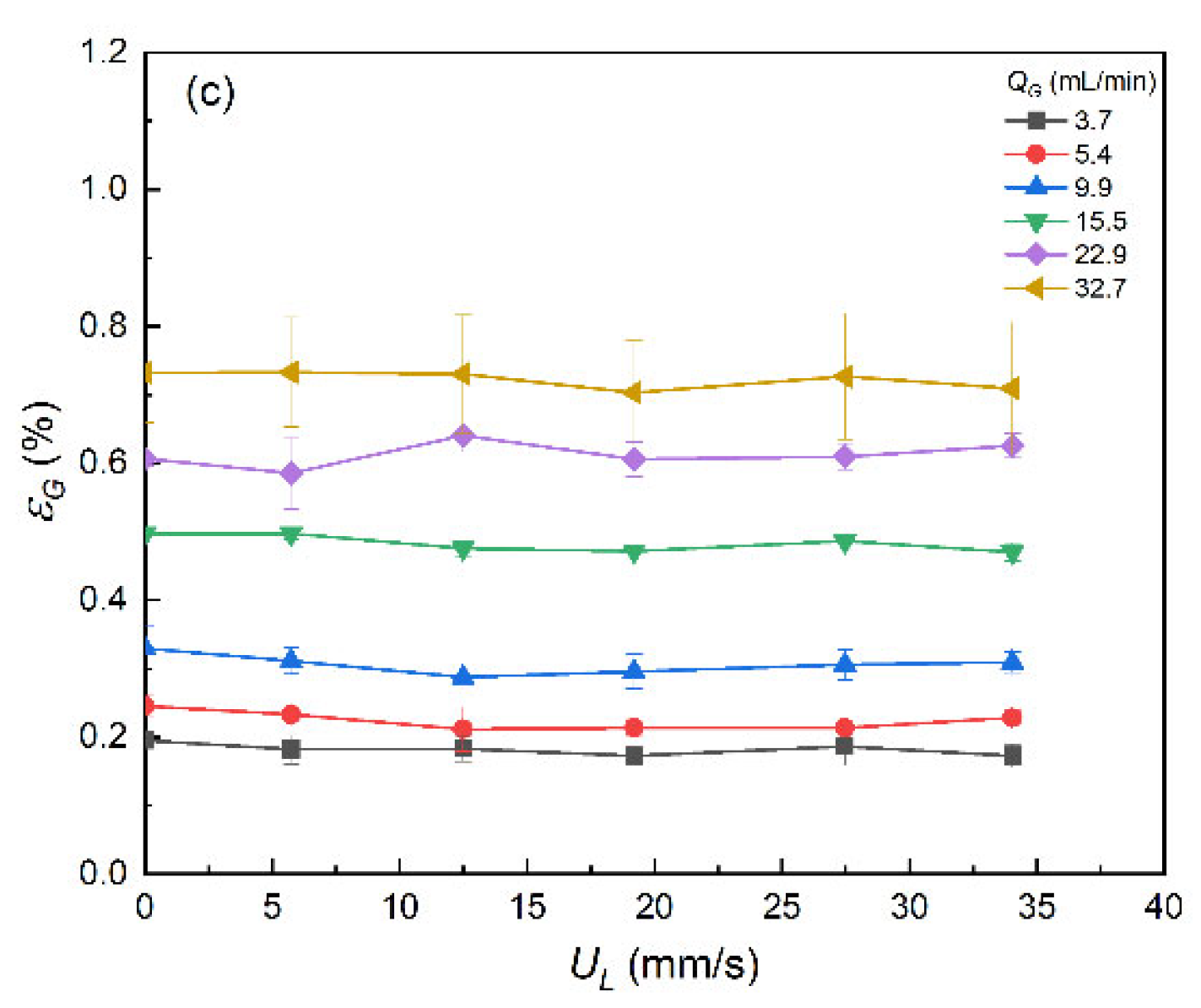
3.1.4. Gas Volume Fraction
3.2. Liquid–Solid Fluidizations
3.2.1. Flow Regimes
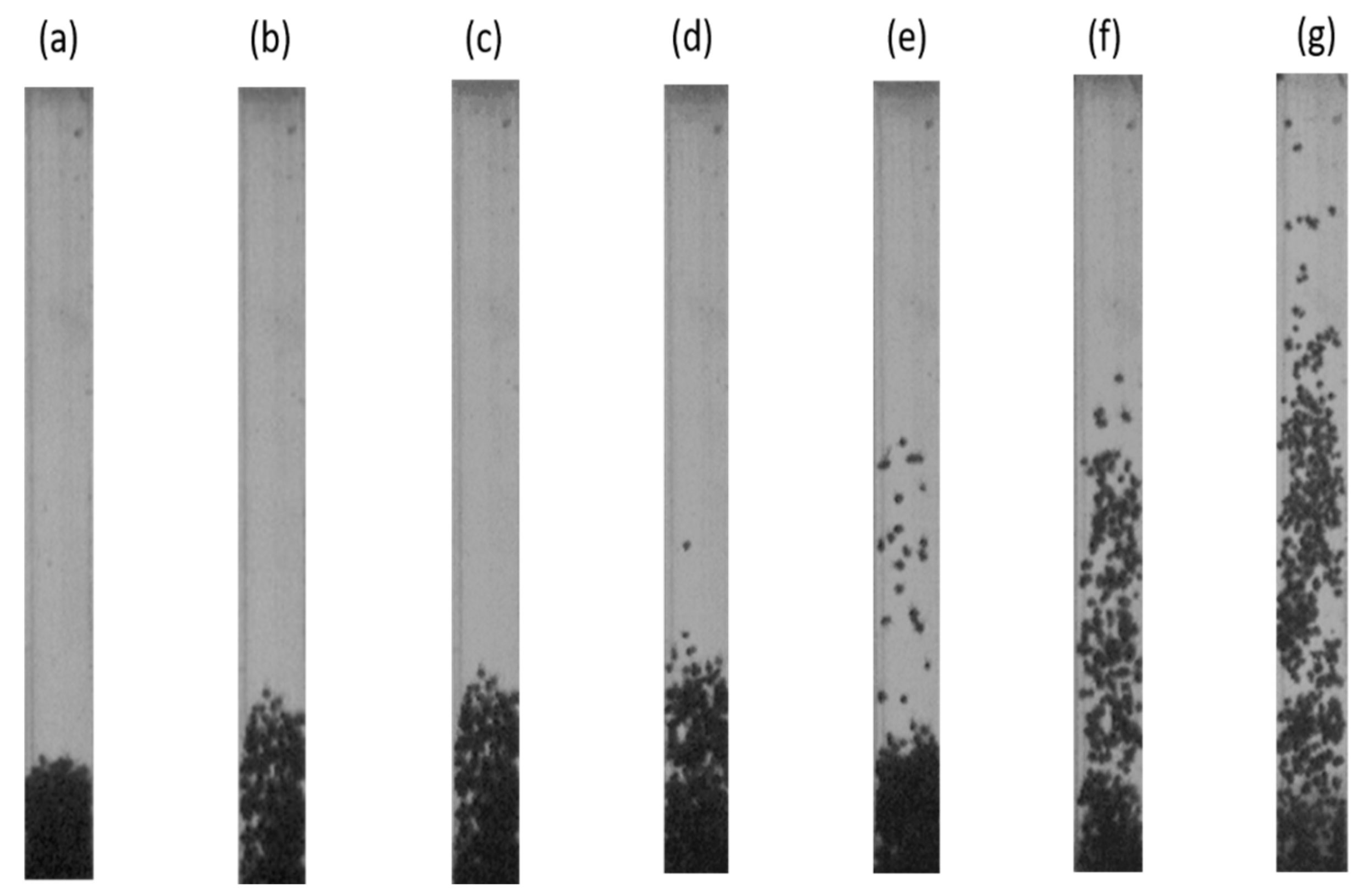
- Packed bed. Fixed particle bed structure with no bed height changes at low superficial liquid velocity (Figure 8a,b).
- Creepingly fluidization. The packed bed became loose, and the bed height started to expand creepingly when the superficial liquid velocity reached the minimum fluidization velocity (Figure 8c,d).
- Particulate fluidization. Followed by minimum fluidization, there was little motion of the particles across the bulk of the cross-section, although some porous gaps in the particles’ bed were observed along the walls (Figure 8e,f).
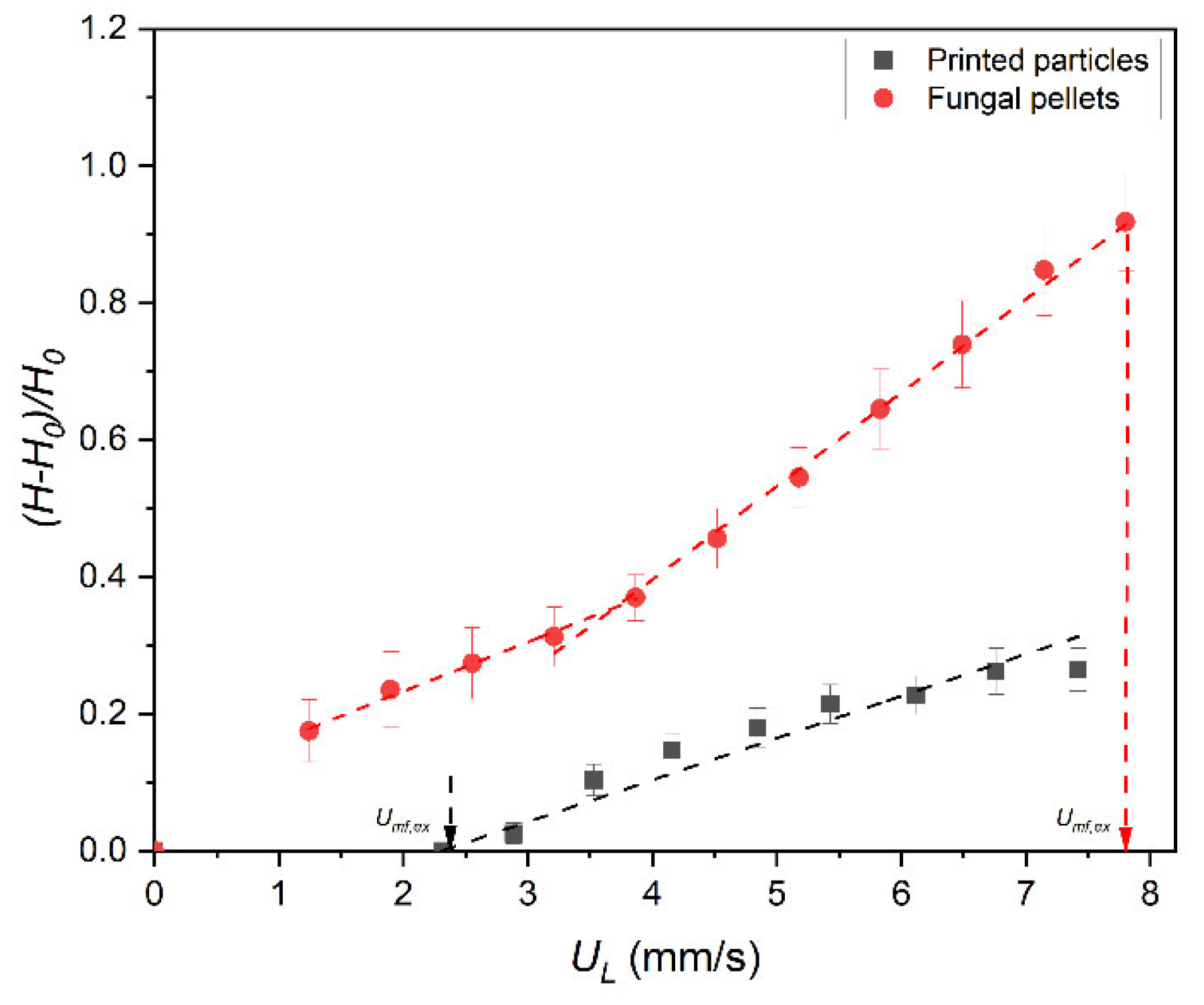
3.2.2. Bed Expansion Study
3.2.3. Richardson–Zaki Expansion Curves Fitting
3.3. Fluidizations by Bubbles in Gas–Liquid–Solid System
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| A | Cross-sectional area of bed column |
| db | Bubbles’ Sauter mean diameter |
| dB | Diameter of internal bed |
| dG | Gas sparger size |
| di | Individual bubble diameter |
| dS | Solid diameter |
| H0 | Static bed height |
| H | Bed height |
| ID | Inner diameter |
| MFB | Micro-fluidized bed |
| m | Solid mass |
| n | Exponent of Richardson–Zaki equation |
| ni | Number of bubbles |
| QG | Volumetric gas flowrate |
| R2 | Coefficient of determination |
| Ub | Bubble rising velocity |
| UL | Liquid velocity |
| Umf | Minimum fluidization velocity |
| Ut | Extrapolated terminal velocity |
| Vb | Individual bubble volume |
| VB | Volume of bubble column |
| Greek Letters | |
| Ɛ | Bed voidage |
| ƐG | Gas volume fraction |
| Ɛ0 | Initial/static bed voidage |
| ρS | Solid density |
References
- Anotai, J.; Wasukran, N.; Boonrattanakij, N. Heterogeneous fluidized-bed Fenton process: Factors affecting iron removal and tertiary treatment application. Chem. Eng. J. 2018, 352, 247–254. [Google Scholar] [CrossRef]
- Lundberg, L.; Soria-Verdugo, A.; Pallarès, D.; Johansson, R.; Thunman, H. The role of fuel mixing on char conversion in a fluidized bed. Powder Technol. 2017, 316, 677–686. [Google Scholar] [CrossRef]
- Wang, C.; Zhu, J. Developments in the understanding of gas–solid contact efficiency in the circulating fluidized bed riser reactor: A review. Chin. J. Chem. Eng. 2016, 24, 53–62. [Google Scholar] [CrossRef]
- Zhang, Y.; Goh, K.-L.; Ng, Y.L.; Chow, Y.; Wang, S.; Zivkovic, V. Process intensification in micro-fluidized bed systems: A review. Chem. Eng. Process. Process Intensif. 2021, 164, 108397. [Google Scholar] [CrossRef]
- Han, Z.; Yue, J.; Zeng, X.; Yu, J.; Wang, F.; Sun, S.; Yao, H.; Luo, G.; Liu, X.; Sun, Y.; et al. Characteristics of gas-solid micro fluidized beds for thermochemical reaction analysis. Carbon Resour. Convers. 2020, 3, 203–218. [Google Scholar] [CrossRef]
- Li, J.; Liu, X.; Zhou, L.; Zhu, Q.; Li, H. A two-stage reduction process for the production of high-purity ultrafine Ni particles in a micro-fluidized bed reactor. Particuology 2015, 19, 27–34. [Google Scholar] [CrossRef]
- Tsutsumi, A.; Nakamoto, S.; Mineo, T.; Yoshida, K. A novel fluidized-bed coating of fine particles by rapid expansion of supercritical fluid solutions. Powder Technol. 1995, 85, 275–278. [Google Scholar] [CrossRef]
- Wang, F.; Zeng, X.; Shao, R.; Wang, Y.; Yu, J.; Xu, G. Isothermal gasification of in situ/ex situ coal char with CO2 in a micro fluidized bed reaction analyzer. Energy Fuels 2015, 29, 4795–4802. [Google Scholar] [CrossRef]
- Yu, J.; Yao, C.; Zeng, X.; Geng, S.; Dong, L.; Wang, Y.; Gao, S.; Xu, G. Biomass pyrolysis in a micro-fluidized bed reactor: Characterization and kinetics. Chem. Eng. J. 2011, 168, 839–847. [Google Scholar] [CrossRef]
- Zeng, X.; Wang, F.; Wang, Y.; Li, A.; Yu, J.; Xu, G. Characterization of char gasification in a micro fluidized bed reaction analyzer. Energy Fuels 2014, 28, 1838–1845. [Google Scholar] [CrossRef]
- Shen, T.; Zhu, X.; Yan, J.; Shen, L. Design of micro interconnected fluidized bed for oxygen carrier evaluation. Int. J. Greenh. Gas Control 2019, 90, 102806. [Google Scholar] [CrossRef]
- Kuyukina, M.S.; Ivshina, I.B.; Serebrennikova, M.K.; Krivoruchko, A.V.; Korshunova, I.O.; Peshkur, T.A.; Cunningham, C.J. Oilfield wastewater biotreatment in a fluidized-bed bioreactor using co-immobilized Rhodococcus cultures. J. Environ. Chem. Eng. 2017, 5, 1252–1260. [Google Scholar] [CrossRef]
- Qiu, L.; Chen, W.; Zhong, L.; Wu, W.; Wu, S.; Chen, J.; Zhang, F.; Zhong, W. Formaldehyde biodegradation by immobilized Methylobacterium sp. XJLW cells in a three-phase fluidized bed reactor. Bioprocess Biosyst. Eng. 2014, 37, 1377–1384. [Google Scholar] [CrossRef]
- Dussán, K.J.; Justo, O.R.; Perez, V.H.; David, G.F.; Junior, E.G.S.; da Silva, S.S. Bioethanol production from sugarcane bagasse hemicellulose hydrolysate by immobilized s. shehatae in a fluidized bed fermenter under magnetic field. BioEnergy Res. 2019, 12, 338–346. [Google Scholar] [CrossRef]
- Da Silva, A.N.; Macêdo, W.V.; Sakamoto, I.K.; Pereyra, D.D.L.A.D.; Mendes, C.O.; Maintinguer, S.I.; Caffaro Filho, R.A.; Damianovic, M.H.Z.; Varesche, M.B.A.; de Amorim, E.L.C. Biohydrogen production from dairy industry wastewater in an anaerobic fluidized-bed reactor. Biomass Bioenergy 2019, 120, 257–264. [Google Scholar] [CrossRef]
- Potic, B.; Kersten, S.R.A.; Ye, M.; van der Hoef, M.A.; Kuipers, J.A.M.; van Swaaij, W.P.M. Fluidization with hot compressed water in micro-reactors. Chem. Eng. Sci. 2005, 60, 5982–5990. [Google Scholar] [CrossRef]
- Zivkovic, V.; Biggs, M.J. On importance of surface forces in a microfluidic fluidized bed. Chem. Eng. Sci. 2015, 126, 143–149. [Google Scholar] [CrossRef][Green Version]
- Li, X.; Liu, M.; Li, Y. Bed expansion and multi-bubble behavior of gas-liquid-solid micro-fluidized beds in sub-millimeter capillary. Chem. Eng. J. 2017, 328, 1122–1138. [Google Scholar] [CrossRef]
- do Nascimento, O.L.; Reay, D.A.; Zivkovic, V. Solid circulating velocity measurement in a liquid–solid micro-circulating fluidised bed. Processes 2020, 8, 1159. [Google Scholar] [CrossRef]
- do Nascimento, O.L.; Reay, D.A.; Zivkovic, V. Influence of surface forces and wall effects on the minimum fluidization velocity of liquid-solid micro-fluidized beds. Powder Technol. 2016, 304, 55–62. [Google Scholar] [CrossRef]
- Rao, A.; Curtis, J.S.; Hancock, B.C.; Wassgren, C. The effect of column diameter and bed height on minimum fluidization velocity. AIChE J. 2010, 56, 2304–2311. [Google Scholar] [CrossRef]
- Zeng, X.; Wang, F.; Adamu, M.H.; Zhang, L.; Han, Z.; Xu, G. High-temperature drying behavior and kinetics of lignite tested by the micro fluidization analytical method. Fuel 2019, 253, 180–188. [Google Scholar] [CrossRef]
- Zeng, X.; Zhang, J.; Adamu, M.H.; Wang, F.; Han, Z.; Zheng, Q.; Zhang, L.; Xu, G. Behavior and kinetics of drying, pyrolysis, gasification, and combustion tested by a microfluidized bed reaction analyzer for the staged-gasification process. Energy Fuels 2020, 34, 2553–2565. [Google Scholar] [CrossRef]
- Gao, W.; Farahani, M.R.; Jamil, M.K.; Siddiqui, M.K.; Siddiqui, H.M.A.; Imran, M.; Rezaee-Manesh, R. Kinetic modeling of pyrolysis of three Iranian waste oils in a micro-fluidized bed. Pet. Sci. Technol. 2017, 35, 183–189. [Google Scholar] [CrossRef]
- Liu, X.; Xu, G.; Gao, S. Micro fluidized beds: Wall effect and operability. Chem. Eng. J. 2008, 137, 302–307. [Google Scholar] [CrossRef]
- Tang, C.; Liu, M.; Li, Y. Experimental investigation of hydrodynamics of liquid–solid mini-fluidized beds. Particuology 2016, 27, 102–109. [Google Scholar] [CrossRef]
- Doroodchi, E.; Peng, Z.; Sathe, M.; Abbasi-Shavazi, E.; Evans, G.M. Fluidisation and packed bed behaviour in capillary tubes. Powder Technol. 2012, 223, 131–136. [Google Scholar] [CrossRef]
- Boffito, D.C.; Neagoe, C.; Edake, M.; Pastor-Ramirez, B.; Patience, G.S. Biofuel synthesis in a capillary fluidized bed. Catal. Today 2014, 237, 13–17. [Google Scholar] [CrossRef]
- Do Nascimento, O.L.; Reay, D.; Zivkovic, V. Study of transitional velocities of solid–liquid micro-circulating fluidized beds by visual observation. J. Chem. Eng. Jpn. 2018, 51, 349–355. [Google Scholar] [CrossRef]
- Zivkovic, V.; Kashani, M.N.; Biggs, M. Experimental and theoretical study of a micro-fluidized bed. AIP Conf. Proc. 2013, 1542, 93–96. [Google Scholar] [CrossRef]
- Lu, Y.; Zhao, L.; Han, Q.; Wei, L.; Zhang, X.; Guo, L.; Wei, J. Minimum fluidization velocities for supercritical water fluidized bed within the range of 633–693K and 23–27MPa. Int. J. Multiph. Flow 2013, 49, 78–82. [Google Scholar] [CrossRef]
- McDonough, J.R. A perspective on the current and future roles of additive manufacturing in process engineering, with an emphasis on heat transfer. Therm. Sci. Eng. Prog. 2020, 19, 100594. [Google Scholar] [CrossRef]
- McDonough, J.R.; Law, R.; Reay, D.A.; Groszek, D.; Zivkovic, V. Miniaturisation of the toroidal fluidization concept using 3D printing. Chem. Eng. Res. Des. 2020, 160, 129–140. [Google Scholar] [CrossRef]
- Qie, Z.; Alhassawi, H.; Sun, F.; Gao, J.; Zhao, G.; Fan, X. Characteristics and applications of micro fluidized beds (MFBs). Chem. Eng. J. 2021, 428, 131330. [Google Scholar] [CrossRef]
- Pjontek, D.; Donaldson, A.; Macchi, A. Three-phase (gas–liquid–solid) fluidization. In Essentials of Fluidization Technology; Wiley-VCH Verlag: Weinheim, Germany, 2020; pp. 553–589. [Google Scholar]
- Chowdhury, N.; Zhu, J.; Nakhla, G.; Patel, A.; Islam, M.J.C.E. A novel liquid-solid circulating fluidized-bed bioreactor for biological nutrient removal from municipal wastewater. Chem. Eng. Technol. 2009, 32, 364–372. [Google Scholar] [CrossRef]
- Kuyukina, M.S.; Ivshina, I.B.; Serebrennikova, M.K.; Krivorutchko, A.B.; Podorozhko, E.A.; Ivanov, R.V.; Lozinsky, V.I. Petroleum-contaminated water treatment in a fluidized-bed bioreactor with immobilized Rhodococcus cells. Int. Biodeterior. Biodegrad. 2009, 63, 427–432. [Google Scholar] [CrossRef]
- Wu, K.-J.; Chang, C.-F.; Chang, J.-S. Simultaneous production of biohydrogen and bioethanol with fluidized-bed and packed-bed bioreactors containing immobilized anaerobic sludge. Process Biochem. 2007, 42, 1165–1171. [Google Scholar] [CrossRef]
- Cavalcantedeamorim, E.; Barros, A.; Rissatozamariollidamianovic, M.; Silva, E. Anaerobic fluidized bed reactor with expanded clay as support for hydrogen production through dark fermentation of glucose. Int. J. Hydrog. Energy 2009, 34, 783–790. [Google Scholar] [CrossRef]
- Zhang, Y.; Ng, Y.L.; Goh, K.-L.; Chow, Y.; Wang, S.; Zivkovic, V. Fluidization of fungal pellets in a 3D-printed micro-fluidized bed. Chem. Eng. Sci. 2021, 236, 116466. [Google Scholar] [CrossRef]
- McDonough, J.R.; Law, R.; Reay, D.A.; Zivkovic, V. Fluidization in small-scale gas-solid 3D-printed fluidized beds. Chem. Eng. Sci. 2019, 200, 294–309. [Google Scholar] [CrossRef]
- Kowalczuk, P.B.; Drzymala, J. Physical meaning of the Sauter mean diameter of spherical particulate matter. Part. Sci. Technol. 2015, 34, 645–647. [Google Scholar] [CrossRef]
- Boubendir, L.; Chikh, S.; Tadrist, L. On the surface tension role in bubble growth and detachment in a micro-tube. Int. J. Multiph. Flow 2020, 124, 103196. [Google Scholar] [CrossRef]
- Kováts, P.; Thévenin, D.; Zähringer, K. Influence of viscosity and surface tension on bubble dynamics and mass transfer in a model bubble column. Int. J. Multiph. Flow 2020, 123, 103174. [Google Scholar] [CrossRef]
- Andrews, S.; Gilley, J.; Coleman, M.P. Difference Tracker: ImageJ plugins for fully automated analysis of multiple axonal transport parameters. J. Neurosci. Methods 2010, 193, 281–287. [Google Scholar] [CrossRef] [PubMed]
- Sardeshpande, M.V.; Harinarayan, S.; Ranade, V.V. Void fraction measurement using electrical capacitance tomography and high speed photography. Chem. Eng. Res. Des. 2015, 94, 1–11. [Google Scholar] [CrossRef]
- Besagni, G.; Inzoli, F.; De Guido, G.; Pellegrini, L.A. Experimental investigation on the influence of ethanol on bubble column hydrodynamics. Chem. Eng. Res. Des. 2016, 112, 1–15. [Google Scholar] [CrossRef]
- Kaji, M.; Sawai, T.; Mori, K.; Iguchi, M. Behaviours of bubble formation from a bottom porous nozzle bath. In Proceedings of the 5th World Conference on Experimental Heat Transfer, Fluid Mechanics and Thermodynamics (ExHFT-5), Thessaloniki, Greece, 24–28 September 2001; pp. 1503–1508. [Google Scholar]
- Zhang, J.P.; Grace, J.R.; Epstein, N.; Lim, K.S. Flow regime identification in gas-liquid flow and three-phase fluidized beds. Chem. Eng. Sci. 1997, 52, 3979–3992. [Google Scholar] [CrossRef]
- Sharaf, S.; Zednikova, M.; Ruzicka, M.C.; Azzopardi, B.J. Global and local hydrodynamics of bubble columns—Effect of gas distributor. Chem. Eng. J. 2016, 288, 489–504. [Google Scholar] [CrossRef]
- Werther, J.; Molerus, O. The local structure of gas fluidized beds—I. A statistically based measuring system. Int. J. Multiph. Flow 1973, 1, 103–122. [Google Scholar] [CrossRef]
- Xue, J.; Al-Dahhan, M.; Dudukovic, M.P.; Mudde, R.F. Four-point optical probe for measurement of bubble dynamics: Validation of the technique. Flow Meas. Instrum. 2008, 19, 293–300. [Google Scholar] [CrossRef]
- Ramakrishnan, S.; Kumar, R.; Kuloor, N.R. Studies in bubble formation—I bubble formation under constant flow conditions. Chem. Eng. Sci. 1969, 24, 731–747. [Google Scholar] [CrossRef]
- Akita, K.; Yoshida, F. Bubble size, interfacial area, and liquid-phase mass transfer coefficient in bubble columns. Ind. Eng. Chem. Process Des. Dev. 1974, 13, 84–91. [Google Scholar] [CrossRef]
- Kulkarni, A.A.; Joshi, J.B. Bubble formation and bubble rise velocity in gas–Liquid systems: A review. Ind. Eng. Chem. Res. 2005, 44, 5873–5931. [Google Scholar] [CrossRef]
- Zivkovic, V.; Zerna, P.; Alwahabi, Z.T.; Biggs, M.J. A pressure drop correlation for low Reynolds number Newtonian flows through a rectangular orifice in a similarly shaped micro-channel. Chem. Eng. Res. Des. 2013, 91, 1–6. [Google Scholar] [CrossRef]
- Whitehead, A.B.; Dent, D.C.; Bhat, G.N. Fluidisation studies in large gas-solid systems Part I: Bubble rise rates. Powder Technol. 1967, 1, 143–148. [Google Scholar] [CrossRef]
- Whitehead, A.B.; Dent, D.C.; Young, A.D. Fluidisation studies in large gas-solid systems Part II: The effect of distributor design and solid properties on fluidisation quality. Powder Technol. 1967, 1, 149–156. [Google Scholar] [CrossRef]
- Godard, K.; Richardson, J. Bubble velocities and bed expansions in freely bubbling fluidised beds. Chem. Eng. Sci. 1969, 24, 663–670. [Google Scholar] [CrossRef]
- Clift, R.; Grace, J. Bubble Interaction in Fluidised Beds. Ph.D. Thesis, McGill University, Montréal, QC, Canada, 1970. [Google Scholar]
- Rowe, P.; Matsuno, R. Single bubbles injected into a gas fluidised bed and observed by X-rays. Chem. Eng. Sci. 1971, 26, 923–935. [Google Scholar] [CrossRef]
- Kiambi, S.L.; Duquenne, A.-M.; Dupont, J.-B.; Colin, C.; Risso, F.; Delmas, H. Measurements of bubble characteristics: Comparison between double optical probe and imaging. Can. J. Chem. Eng. 2003, 81, 764–770. [Google Scholar] [CrossRef]
- Rüdisüli, M.; Schildhauer, T.J.; Biollaz, S.M.A.; Ruud van Ommen, J. Bubble characterization in a fluidized bed by means of optical probes. Int. J. Multiph. Flow 2012, 41, 56–67. [Google Scholar] [CrossRef]
- Werther, J.; Molerus, O. The local structure of gas fluidized beds—II. The spatial distribution of bubbles. Int. J. Multiph. Flow 1973, 1, 123–138. [Google Scholar] [CrossRef]
- Zenit, R.; Koch, D.L.; Sangani, A.S. Impedance probe to measure local gas volume fraction and bubble velocity in a bubbly liquid. Rev. Sci. Instrum. 2003, 74, 2817–2827. [Google Scholar] [CrossRef]
- Di Felice, R. Hydrodynamics of liquid fluidisation. Chem. Eng. Sci. 1995, 50, 1213–1245. [Google Scholar] [CrossRef]
- Zivkovic, V.; Biggs, M.J.; Alwahabi, Z.T. Experimental study of a liquid fluidization in a microfluidic channel. AIChE J. 2013, 59, 361–364. [Google Scholar] [CrossRef]
- Smith, E.; James, A.; Fidgett, M. Fluidization of microbial aggregates in tower fermenters. In Proceedings of the 2nd Engineering Foundation Conference, Cambridge, UK, 2–6 April 1978; pp. 196–201. [Google Scholar]
- Richardson, J.F.; Zaki, W.N. The sedimentation of a suspension of uniform spheres under conditions of viscous flow. Chem. Eng. Sci. 1954, 3, 65–73. [Google Scholar] [CrossRef]
- Espinosa-Ortiz, E.J.; Rene, E.R.; Pakshirajan, K.; van Hullebusch, E.D.; Lens, P.N.L. Fungal pelleted reactors in wastewater treatment: Applications and perspectives. Chem. Eng. J. 2016, 283, 553–571. [Google Scholar] [CrossRef]

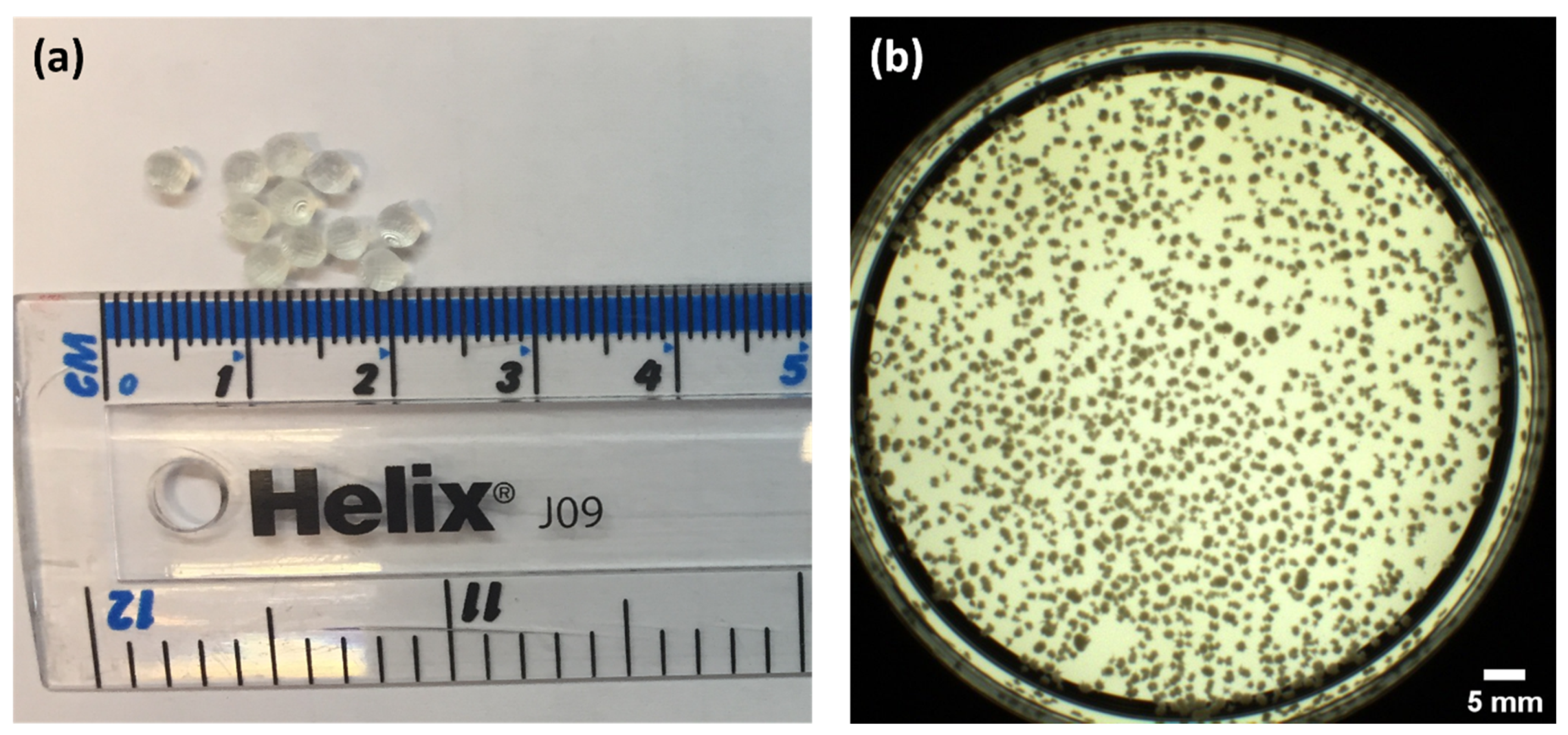




| Materials | Density (g/cm3) | Diameter (mm) | Sphericity |
|---|---|---|---|
| Printed particles | 1.20 ± 0.02 | 2.0 | 0.99 |
| Fungal pellets | 1.09 ± 0.09 | 1.80 ± 0.55 | 0.82 ± 0.12 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Goh, K.-L.; Ng, Y.-L.; Chow, Y.; Zivkovic, V. Design and Investigation of a 3D-Printed Micro-Fluidized Bed. ChemEngineering 2021, 5, 62. https://doi.org/10.3390/chemengineering5030062
Zhang Y, Goh K-L, Ng Y-L, Chow Y, Zivkovic V. Design and Investigation of a 3D-Printed Micro-Fluidized Bed. ChemEngineering. 2021; 5(3):62. https://doi.org/10.3390/chemengineering5030062
Chicago/Turabian StyleZhang, Yi, Kheng-Lim Goh, Yuen-Ling Ng, Yvonne Chow, and Vladimir Zivkovic. 2021. "Design and Investigation of a 3D-Printed Micro-Fluidized Bed" ChemEngineering 5, no. 3: 62. https://doi.org/10.3390/chemengineering5030062
APA StyleZhang, Y., Goh, K.-L., Ng, Y.-L., Chow, Y., & Zivkovic, V. (2021). Design and Investigation of a 3D-Printed Micro-Fluidized Bed. ChemEngineering, 5(3), 62. https://doi.org/10.3390/chemengineering5030062








