On the Impact of the Dynamics of Heat Transfer of the Thermal Machine Working Fluid and Heat Sources on the Shape of the Boundary of the Set of Realizable Regimes

Abstract

From the point of view of finite time thermodynamics, the performance boundaries of thermal machines are considered, taking into account the irreversibility of the heat exchange processes of the working fluid with hot and cold sources. We show how the dynamics of heat exchange affects the shape of the optimal cycle of a heat engine and its performance, in particular, energy conversion efficiency in the maximum power mode. This energy conversion efficiency can depend only on the ratio of the heat transfer coefficients to the sources, or not depend on them at all. A class of dynamic functions corresponding to “natural” requirements is introduced and it is shown that, for any dynamics from this class, the optimal cycle consists of two isotherms and two adiabats, not only for the maximum power problem, but also for the problem of maximum energy conversion efficiency at a given power. Examples are given for calculating the parameters of the optimal cycle for the cases when the heat transfer coefficient to the cold source is arbitrarily large, and for dynamics in the form of a linear phenomenological (Fourier heat transfer) law.

1. Introduction

Systems converting heat into work have been the main object of thermodynamic research since the time of S. Carnot, who gave an upper boundary for the efficiency of such a transformation. He considered a heat engine as a system consisting of a working fluid which is in contact with two heat sources, a hot and a cold one. Carnot efficiency (the ratio of the work produced to the heat received from the hot source) was achieved in the limiting reversible process when both the power generated by the machine and the heat flows tend to zero, or when the heat transfer coefficients between the working fluid and the heat sources tend to very large numbers. The friction effects and inhomogeneities of the temperature field inside the working fluid leading to irreversibilities were negligible. In real heat engines, the power is fixed, the coefficients of heat exchange is limited and their efficiency is significantly less than the efficiency of Carnot. We, as is customary in finite time thermodynamics, consider each element of the system under consideration of internal equilibrium, and we consider the associaton with the entropy production processes, concentrated on the boundaries of the subsystems during their contacts with each other.

With the development of nuclear power, when the part of fuel cost in generated energy cost per kilowatt is much less, and the part of capital costs is much more than for non-nuclear thermal power plants (see [1]), the problem of the maximum power of thermal machines with limited coefficients of heat exchange with heat sources arose. I.I. Novikov assumed in his publication [2] that the cycle of maximum power, like the Carnot cycle, consists of two isotherms and two adiabats. He showed that the maximum power is limited, found the corresponding value, depending on the coefficient of heat transfer with sources and the corresponding efficiency, that turned out to be related to the Carnot efficiency ${\eta }_c$ by the expression

$${\eta }_N=1-\sqrt{1-{\eta }_C}.$$

(1)

Novikov assumed that the dynamics of heat exchange (the dependence of heat flow on the temperature of the contacting bodies) is linear with fixed heat transfer coefficients. The heat flux $q_i$ from the i-th source is proportional to the temperature difference between the source $T_i$ and the working fluid T:

$$q_i={\alpha }_i(T_i-T),$$

(2)

where i denotes the index of an arbitrary source and ${\alpha }_i$ is the transfer coefficient. This kind of dynamics is often called Newtonian. With a limited coefficient ${\alpha }_i$, the heat exchange is irreversible. Note that ${\eta }_N$ as the Carnot efficiency does not depend on the heat transfer coefficients with the sources, and that is not obvious.

Novikov’s conclusions was later independently confirmed by Curzon and Ahlborn [3], assuming that the heat transport to the cold heat bath is irreversible, too.

Later, the problems of the maximum power of the thermal machine and its maximum energy conversion efficiency at a given power were considered in [4,5,6] and it was shown that for any heat transfer dynamics in the maximum power case, the optimal cycle consists of two isotherms and two adiabats. In the case of maximum energy conversion efficiency at a fixed power, or for the maximum power at a fixed heat consumption, the optimal cycle can consist of no more than three isotherms and three adiabats. An example of such a cycle was given in [7] for a somewhat exotic form of dynamic dependencies. These results were based on the methods of averaged optimization [8,9]. The problems of thermodynamic analysis of heat conversion to the work of separation are also considered in [10,11,12,13,14,15,16,17] as well. There are a number of papers in which similar problems are considered, for example, [18], where the relationship between efficiency at maximum power and Carnot efficiency for a particular type of kinetic equations is considered. Another problem is given in [19], where reversible cycles with a finite heat capacity of the working fluid are considered. The duration of the cycle in this task does not appear.

There are the following problems in connection with these studies:

• Find the dynamic laws of heat transfer with sources for which the efficiency of the machine in the maximum power mode does not depend on the dynamic coefficients
• Determine the laws of dynamics in which the cycle corresponding to the maximum efficiency at a given heat consumption consists of two and three isotherms.
• If the optimal cycle consists of three isotherms, then sources two of them are realized?

This work is devoted to solving these problems.

2. Averaged Optimization and Convex Hulls of a Function

The working fluid of a heat engine changes its parameters cyclically in time or in space. In the latter case, it circulates in a closed loop, receiving and giving heat to sources and doing work, therefore, all parameters of flows (power, heat) are necessary to average per cycle. This leads to the fact that it is convenient to use the methods of average optimization for the cycle analyzing. Now we will give certain information about these methods.

2.1. Convex Hulls of a Function

The convex hull a function $f\left(x\right)$ on the set $x\ni V$ is the maximum of the mean value of the function with a given mean value of its argument. The formal description of this problem:

$$\langle f\rangle ={\int }_{V}P\left(x\right)f\left(x\right)dx\to max/{\int }_{V}P\left(x\right)xdx=\langle x\rangle .$$

(3)

Here, the angle brackets correspond to averaging, $P\left(x\right)$, the function of measure. This function is non-negative and has an area equal to one. Exactly the measure function is the required solution to problem (3). The Caratheodory theorem [20] reduces this problem to the common task of nonlinear programming. It approves that the measure functions have a view

$$P\left(x\right)=\sum _{\nu =0}^n{\gamma }_{\nu }\delta (x-x^{\nu }),{\gamma }_{\nu }\ge 0,\sum _{\nu =0}^n{\gamma }_{\nu }=1.$$

(4)

at the optimal solution. The n is the dimension of vector x. The values of $x^{\nu }$ of the vector x are called basic. Thus, the optimal measure is concentrated no more than at the $(n+1)$-th base point on V, and ${\gamma }_{\nu }$ are the weight coefficients of the corresponding values of x and $f\left(x\right)$.

After the substitution of the measure in the form (4) into the problem (3) it takes the form:

$$\langle f\rangle =\sum _{\nu }{\gamma }_{\nu }f\left(x^{\nu }\right)\to max/\sum _{\nu }{\gamma }_{\nu }{x}^{\nu }=\langle x\rangle {\gamma }_{\nu }\ge 0,\sum _{\nu =0}^n{\gamma }_{\nu }=1.$$

(5)

This is a finite-dimensional problem of nonlinear programming. For functions of one variable, there are no more than two base values. The convex hull either coincides with the graph of the function $f\left(x\right)$ (for one base value) or passes above the graph and represents a segment of a straight line tangent to the function $f\left(x\right)$ in two base points. Similarly, for functions of two variables, a convex hull, if it does not coincide with $f\left(x\right)$, it may either be a line segment (for two points), or a region of the plane (for three base points).

2.2. Averaged Problem of Conditional Optimization

In [8,9] and very briefly in Appendix A, the averaged problem of conditional optimization was considered:

$$\langle f_0\rangle ={\int }_{V}P\left(x\right)f_0\left(x\right)dx\to max/{\int }_{V}P\left(x\right)f_k\left(x\right)dx=\langle f_k\left(x\right)\rangle ,k=1,\dots m.$$

(6)

As proven for this problem, from the theorem Caratheodory it follows that the measure function on the optimal solution have a form

$$P\left(x\right)=\sum _{\nu =0}^m{\gamma }_{\nu }\delta (x-x^{\nu }),{\gamma }_{\nu }\ge 0,\sum _{\nu =0}^m{\gamma }_{\nu }=1.$$

(7)

where the m is the dimension of the vector function $f\left(x\right)$ (the number of conditions). The values of $x^{\nu }$ of the vector x are also called base values here. Their number, in contrast to the problem of a convex hull of function, does not depend on the dimension of x and no more than one more than the dimension of $f\left(x\right)$. Thus, in the problem with a single average condition, the number of base values is not more than two, and in a problem with two conditions, not more than three values. As will be shown below, the problem of the maximum power of the heat machine has one, and the problem of maximum efficiency for a given power has two averaged conditions.

3. “Natural” Dynamic Functions and Optimal Cycles Structure

We will call natural dynamic dependencies relating the heat flow q with temperatures $T_i,T$ source and the working fluid, if they satisfy the following conditions:

• $q_i(T_i,T)$ are continuous and differentiable with respect to their arguments everywhere except for $T_i=T$. $\frac{\partial q_i}{\partial T_i}>0$ and $\frac{\partial q_i}{\partial T}<0$ hold true (these are the monotonicity conditions). The same applies to the signs of the derivatives $T_i,T$ on $q_i$.
• The sign of the heat flux coincides with the sign of the temperature difference $T_i-T$ (the second law of thermodynamics). $q_i(T_i,T)=0$ at $T_i=T$ holds true.
• The dynamic dependence can be written in the form $q_i(T_i,T)={\alpha }_i{f}_i(T_i,T)$, where the heat transfer coefficient ${\alpha }_i>0$ is proportional to the contact surface of the working fluid with the source. For example, the dependency of type $q_i(T_i,T)={\alpha }_i{f}_i(T_i,T)+{\beta }_i{r}_i(T_i,T)$ does not match this requirement. This type of dynamic was investigated in [7]. The optimal cycle consisted of three isotherms and three adiabats in this work.

Cyclicity in the state of the working fluid causes what can distinguish two half-cycles, heating and cooling; the first of which, the working fluid, is contacted with hot and secondly with a cold source.

Statement of the Problem and Solutions Structure Corresponding to the Boundary of Realizability Region

The area of realizability of a thermal machine is a region in a plane whose axis of ordinates is the machine’s power, and whose abscissa is the heat flux taken away from the hot source. This region is for specific forms of dynamic dependence and has been built in many papers, see [21] and others. We shall consider it peculiar properties, not specifying the form of dynamic functions $q_{+},q_{-}$, which determine the heat transfer from hot and cold sources to the working fluid.

We assume a working fluid heat engine uniform temperature at each time or, in each section. Its extensive variables, such as internal energy and entropy, vary cyclically.

Let $q\left(\tilde{T}\right)=\alpha f\left(\tilde{T}\right)$ be the heat flux of exchange of working fluid with sources, which changes the sign and shape of the dynamic function depending on the sign ${\mathrm{\Delta }}_i=T_i-T$.

Each point of the boundary of the realizability region in the working load range is the solution of the following problem: Given a power p of the heat machine, find such a temperature vector $\tilde{T}=\left\{T_i,T\right\}$ of sources and the working fluid so that the energy conversion efficiency of the machine $\eta =\frac{p}{〈q_{+}〉}$ is maximal.

This problem is equivalent to problems about the maximum power of the machine at a given value of the average heat flux $q_{+}$, taken from the hot source.

The formal optimization takes the form

$$p=〈q〉\Rightarrow max,$$

(8)

under conditions

$$〈\frac{q}{T}〉=0;$$

(9)

$$\langle q_{+}\rangle =fix.$$

(10)

Here, $q_{+}>0$ is the heat supplied to the working fluid from the hot source, and $q_{-}<0$ is the heat drawn from it to the cold source. The union of these two heat fluxes is the heat flux q between the working fluid and the sources. So, $q_{+}$ is equal to q, when q is positive, which can mathematically be expressed as $q_{+}=0.5(q+|q\left|\right)$. Analogously, $q_{-}$ is equal to q, when q is negative, which can be expressed as $q_{-}=0.5(q-|q\left|\right)$.

The condition (9) follows from the entropy balance equation (the entropy change rate of the working fluid is ${\displaystyle \frac{q}{T}}$, and its entropy does not change at a cycle).

The set of possible values of the vector $\tilde{T}$ is defined by the fact that the range of variation of working fluid temperature T lies inside the range of variation of sources temperature $T_i$. The range of variation of $T_i$ takes the values $T_{+}$ and $T_{-}$.

The problem (8)–(10) contains the averaged constraint (9) and the restriction on the average difference value between the input and output heat fluxes (machine power). From the results of the average optimization in [8,9] it follows that:

• The optimal solution of this problem can take no more than three (base) values of the vector $\tilde{T}={\tilde{T}}^{\nu }$; i.e., no more than three isothermal sections with adiabatic transitions from one isotherm to another, where $\nu$ is the base solution index.
• The duration of each isothermal section is a certain part ${\gamma }_{\nu }$ of the cycle time, and the adiabatic transitions occurs instantaneously.

In the problem of limiting power, the condition (10) is absent, and the average problem has no more than two base points. This means that for any form of dynamic, the optimal cycle of change in the state of the working fluid may consist of only two isotherms and two adiabatic (the decision having one base point does not satisfy the condition (9)).

We show that for any heat transfer dynamic that satisfies the “natural” requirements listed in the introduction and the mentioned additional inequality, the problem (8)–(10) has two base solutions, i.e., the corresponding optimal cycle, like the Carnot and Novikov cycles, consists of two isotherms and two adiabats.

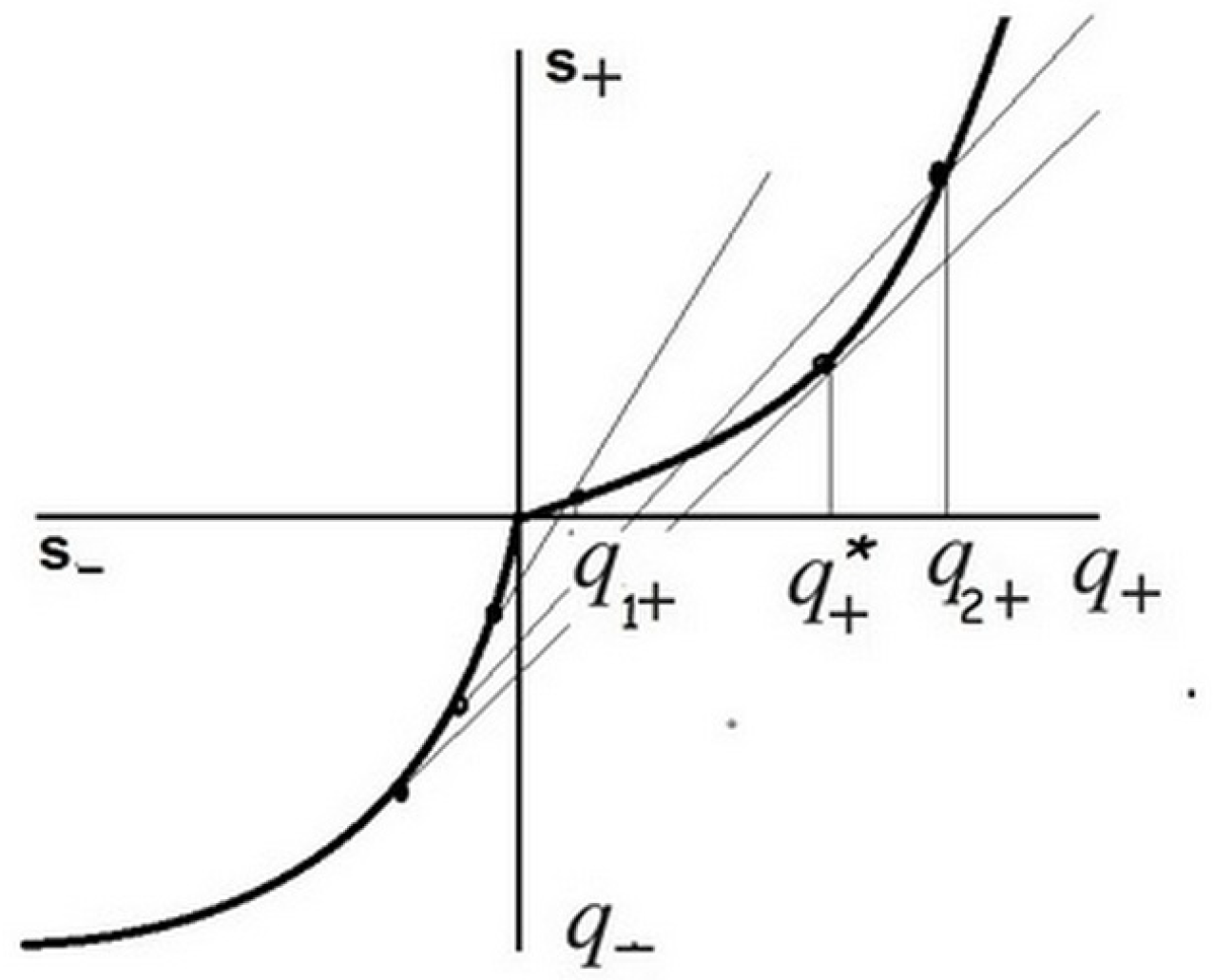
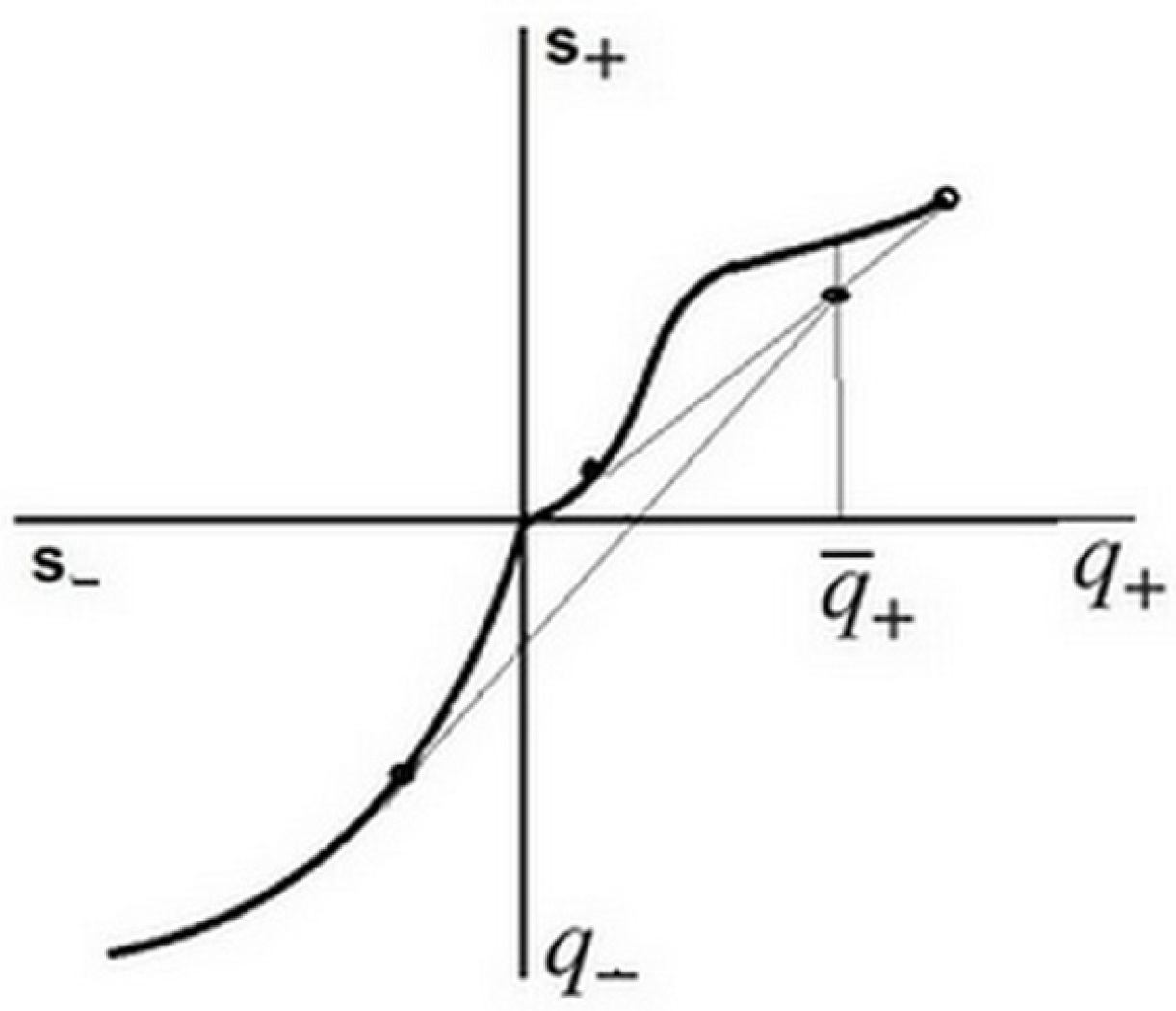
4. Graphic Interpretation of the Solution

Below we give a graphic interpretation of the solution of the provided problems and show under what condition is the optimal solution of the problem of the boundary of the set of realizable regimes, and hence of the maximum efficiency at a given power, consists of two isotherms and two adiabats. If the additional inequality (11) not met then the optimal cycle may contains three isothermal sections, the two of them can be only in the half-cycle of working fluid heating.

We use the index “+”, when something is related to the source with a high temperature and an index “−”, when something is related to the source with a low temperature.

First, we deduce the system of equations and inequalities that define the boundary of the working region for an arbitrary form of dynamic dependencies.

We divide the set of admissible values of the vector $\tilde{T}$ into two disjoint subsets. The first of which $T_i=T_{+}>T$, and the second is $T_i=T_{-}<T$. The line $T_i=T$ does not contain the desired solution, since $q=0$ on it. The optimal solution can not be unique, since in this case, for $T_i\ne T$ the heat flux retains its sign and the condition (9) can not be satisfied.

We introduce alongside heat fluxes two functions

$$s_{+}\left(q_{+}\right)=\frac{q_{+}}{T_1\left(q_{+}\right)},s_{-}\left(q_{-}\right)=\frac{q_{-}}{T_2\left(q_{-}\right)},$$

characterizing fluxes of entropy supplied and removed from the working fluid. Monotonicity conditions ensure that the dependencies $T_1\left(q_{+}\right)$ and $T_2\left(q_{-}\right)$ are unambiguous and monotonic; they are zero when $T_1=T_{+},T_2=T_{-}$. Having calculated the derivatives of curves $s_{+}\left(q_{+}\right)$ and $s_{-}\left(q_{-}\right)$, we obtain that for any heat transfer laws their slopes at the origin are $1/T_{+}$ and $1/T_{-}$, respectively (see Figure 1).

This means that in the neighborhood of the origin condition $s_{+}\left(q_{+}\right)+s_{-}\left(q_{-}\right)=0$ corresponds to the equality

$$q_{+}/T_{+}+q_{-}/T_{-}=0\Rightarrow \frac{q_{-}}{q_{+}}=\frac{T_{-}}{T_{+}},$$

and the efficiency of a machine having arbitrarily low power for any dynamics is

$$\eta =\frac{p}{q_{+}}=\frac{q_{+}+q_{-}}{q_{+}}=1-\frac{T_{-}}{T_{+}}={\eta }_c.$$

The problem of the maximum power in the absence of a restriction (10) on the heat flux is reduced to the construction of a convex hull of the set bounded below by the curves $s_{+}\left(q_{+}\right)$ and $s_{-}\left(q_{-}\right)$. The boundary of the convex hull has only two common points with these curves, in which it is a, tangent. At the point of intersection of this boundary with the abscissa axis, the average entropy flux is zero, and the power (average heat flux value) is maximum. The corresponding value of $\langle q\rangle =p^{*}.$ Values of weight coefficients easily find so as their sum of equal is one, and the attitude ${\gamma }_1/{\gamma }_2$ is equal to the ratio of the segments of the convex shell lying above and below the axis of the abscissa.

The average value of the flow is $q_{+}$ fixed the problem of maximum efficiency.

If the dependence $s_{+}\left(q_{+}\right)$ convex downward, its convex hull throughout it coincides with the average value of the heat flow corresponding to the point on this curve with ordinate $s_{+}\left(\langle q_{+}\rangle \right)$. If from this point we were to conduct tangent to the curve $s_{-}\left(q_{-}\right)$, then the intersection of this tangent with the abscissa corresponds to the maximum average value of heat flow. The point of this intersection is at the maximum distance from the origin if only the drawn line is a tangent.

Note that the tangent can be drawn in the case when the curve $s_{-}\left(q_{-}\right)$ is not smooth and not convex. In any case, the temperature $T_2$ corresponding to the touch point is the only one and, consequently, the optimal half-cycle cooling the working fluid is always composed of a single isotherm.

If the average heat flux $\langle q_{+}\rangle$ is given and the curve $s_{+}\left(q_{+}\right)$ is not convex, then the point corresponding to the average heat flux can not be on the curve $s_{+}\left(q_{+}\right)$, but on its convex hull. This means that the corresponding flow can be realized by alternately switching the temperatures corresponding to the tangency points of the convex hull with the curve $s_{+}\left(q_{+}\right)$.

Figure 2 shows the case when this dependence is not convex and the given average value of the input heat flux $q_{+}^0$ corresponds to a point on its convex hull, such that the tangent drawn from it to the curve $s_{-}\left(q_{-}\right)$ intersects with the abscissa axis more to the right than the tangent drawn from the point $s_{+}\left(q_{+}^0\right)$. The optimal heating half-cycle in this case contains two isotherms, and the cooling half-cycle always contains one. Note that when changing the set average value of $\langle q_{+}\rangle$, the temperature of these isotherms is not changed, but only a fraction of the cycle length that corresponds to them is changed.

Under the conditions of the monotonicity, the slope of the curve $s_{+}\left(q_{+}\right)=\frac{q_{+}}{T_1\left(q_{+}\right)}$ almost always increases with increasing $q_{+}$ (the numerator increases linearly, and the denominator decreases). The condition of nonnegativity of the second derivative leads to inequality:

$$q_{+}\frac{{\partial }^2{T}_1}{\partial q_{+}^2}\le 2\frac{\partial T_1}{\partial q_{+}}\left(\frac{q_{+}}{T_1}\frac{\partial T_1}{\partial q_{+}}-1\right),$$

(11)

that guarantees the convexity of this dependence.

5. The Optimal Solution

The fact that the averaged problem (8)–(10) has an optimal solution in the form of a cycle containing two isotherms and two adiobates reduces its solution to a finite-dimensional problem with three variables: $T_1,T_2$ and $\gamma$, where $\gamma$ is the fraction of the cycle during which the working fluid contacts the hot source. The fraction of contact time with a cold source is $1-\gamma$.

The value of $\gamma$ can be expressed through the coordinates of the points on the curves $s_{-}\left(q_{-}\right)$ and $s_{+}\left(q_{+}\right)$ through which the tangent to the function $s_{-}\left(q_{-}\right)$ passes. You can also find the optimal temperature of the working fluid when it is heated and cooled $T_1$ and $T_2$.

The point on the heating curve $s_{+}^0=s_{+}\left(q_{+}^0\right),q_{+}^0$ is given and the dependence $T_1\left(q_{+}\right)$ determines the value $T_1^0=T_1\left(q_{+}^0\right)$. The tangent equation has the form:

$$s=s_{+}^0+{\left(\frac{d{s}_{-}}{d{q}_{-}}\right)}_0(q-q_{+}^0).$$

(12)

Here and further the subscript “0” shows that the derivative is calculated in point $q=q_{-}^0$. To determine the coordinates of this point, we have a tangent condition:

$$s_{+}^0-s_{-}\left(q_{-}^0\right)={\left(\frac{d{s}_{-}}{d{q}_{-}}\right)}_0(q_{+}^0-q_{-}^0).$$

(13)

From this equation find $q_{-}^0$, and then $s_{-}^0$ and $T_2$.

The point of intersection of the tangent with the abscissa axis determines the maximum power achievable at a spending of heat $q_{+}^0$:

$$p^{*}\left(q_{+}^0\right)=q_{+}^0-\frac{s_{+}^0}{{\left(\frac{d{s}_{-}}{d{q}_{-}}\right)}_0}.$$

The condition of constancy of working fluid entropy $\gamma s_{+}^0+(1-\gamma )s_{-}^0=0$ defines time share his contact with the sources:

$$\gamma =-\frac{s_{-}^0}{s_{+}^0-s_{-}^0},1-\gamma =\frac{s_{+}^0}{s_{+}^0-s_{-}^0}.$$

(14)

The Relation between the Power and the Entropy Production in the System

The production of entropy in the system is caused by the irreversible process of heat exchange between the sources and the working fluid. It is equal to:

$$\sigma =q_{-}\left(\frac{1}{T_{-}}-\frac{1}{T_2}\right)+q_{+}\left(\frac{1}{T_1}-\frac{1}{T_{+}}\right).$$

(15)

With increasing flux intensity, the driving forces enclosed in the round parentheses, grow, so the production of entropy with increasing intensity of fluxes grows faster than linear dependence. So, for Newtonian dynamics:

$$\sigma =\left(\frac{q_{-}^2}{{\alpha }_{-}{T}_2{T}_{-}}\right)+\left(\frac{q_{+}^2}{{\alpha }_{+}{T}_1{T}_{+}}\right).$$

Eliminating $q_{-}$ which is $q_{+}-p$ we obtain:

$$p=q_{+}\left(1-\frac{T_{-}}{T_{+}}\right)-\sigma T_{-}=q_{+}{\eta }_C-T_{-}\sigma .$$

(16)

from the condition (16).

This expression is an analogue of the well-known Stodola formula (see [22]), written down for fluxes of heat and work. Maximum power at the fixed value of $q_{+}$ corresponds to the minimum production entropy for any form of dynamic dependencies.

The maximum power for a fixed value of $q_{+}$ corresponds to minimum entropy production for any form of dynamic dependencies. Since the entropy production grows faster than the first term with increasing $q_{+}$, then the power at $q_{+}=0$ is zero, then increases to certain maximum, and then decreases to zero.

The machine’s efficiency is associated with the production of entropy as:

$$\eta ={\eta }_C-\frac{T_{-}\sigma }{q_{+}}.$$

(17)

We note that for any heat transfer laws, the production of entropy decreases monotonically with increasing heat transfer coefficients ${\alpha }_i$. The Lagrange function in the problem of maximum power (minimum dissipation) for a given sum of heat transfer coefficients has the form:

$$L=\sigma ({\alpha }_{+},{\alpha }_{-})-\lambda ({\alpha }_{+}+{\alpha }_{-}).$$

The conditions of its stationarity for the sought-for variables leads to the requirement of equality:

$$\frac{\partial \sigma }{\partial {\alpha }_{+}}=\frac{\partial \sigma }{\partial {\alpha }_{-}}.$$

(18)

Solving the problem for the case when the half-cycle of heating can consist of two isotherms, does in two stages. At first solves the problem of constructing convex hull of $s_{+}\left(q_{+}\right)$. Its solution determines the temperature of two isotherms and the proportion of the duration of heating half cycles for each of the dependent given average heat flow $q_{+}$. The second stage solves the problem of determination cooling isotherm and corresponds to the tangency line drawn from the calculated at the first stage point of intersection of the vertical line with abscissa of $\langle q_{+}\rangle$ with the convex hull $s_{+}\left(q_{+}\right)$, the dependence of $s_{-}\left(q_{-}\right)$. At this stage, we determine the shares of the cooling cycle and heating from the overall cycle time.

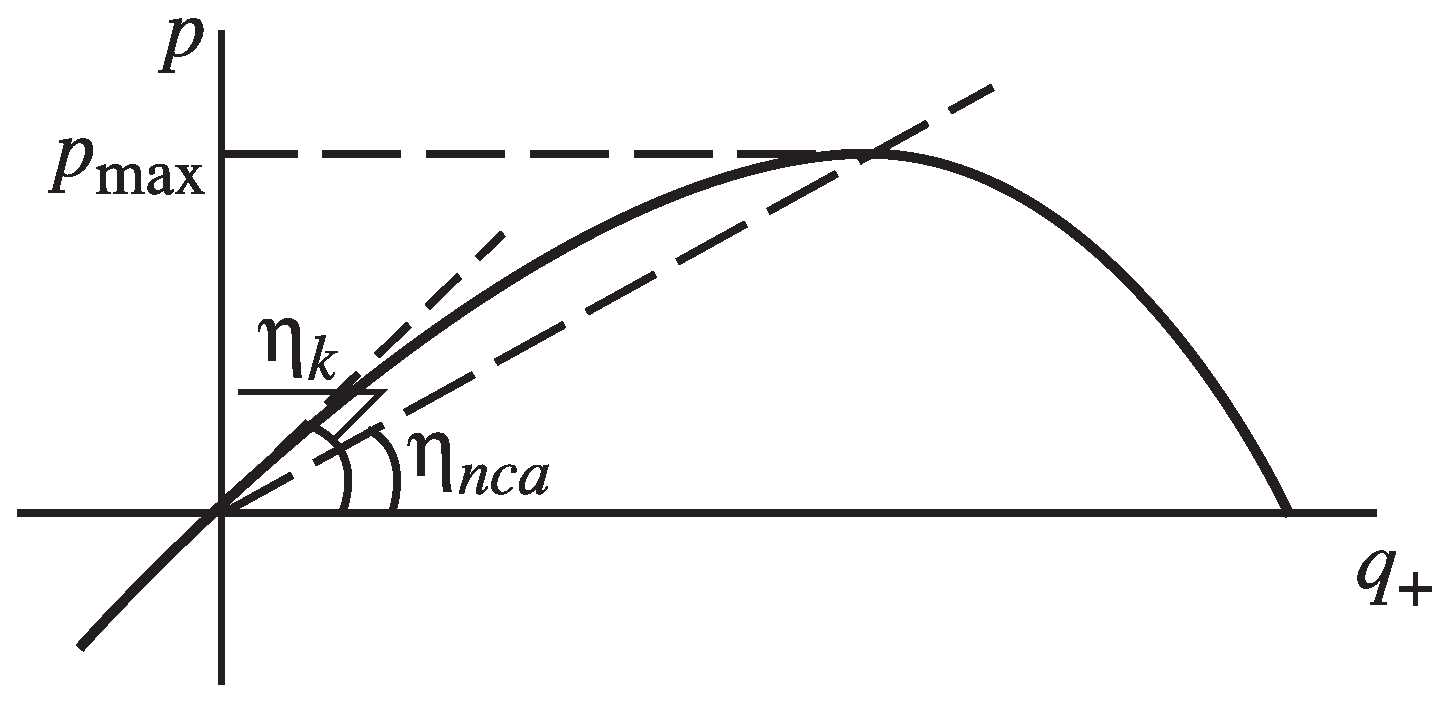
6. The Realizability Region Boundary

6.1. Characteristic Points of the Boundary

The boundary of the realizability region has three characteristic points (see Figure 3):

• The origin of coordinates where $q_{+}$ and p tend to zero. The energy and conversion efficiency for any heat exchange law at this point is ${\eta }_C=1-\frac{T_{-}}{T_{+}}$.
• The point at which the power of the machine is maximal. This point is denoted by $p^{*}$. The corresponding energy conversion efficiency and heat flux will be denoted by ${\eta }^{*}$ and $q_{+}^{*}$.
• The point at which the irreversibility of the processes is so great that the power of the machine turns out to be zero. The value of the heat flux corresponding to this point is denoted by $q_{+}^{max}$.

We define the energy conversion efficiency of converter as

$$\eta =\frac{p}{q_{+}(T_{+},T_1)}$$

(19)

and find the relationship between the power p, the energy conversion efficiency and the heat consumption $q_{+}$.

From the condition of entropy invariance of the working fluid,

$$q_{-}=-q_{+}\frac{T_2}{T_1},$$

(20)

it follows that

$$q_{+}\left(1-\frac{T_2}{T_1}\right)=p\to \eta =1-\frac{T_2}{T_1}.$$

(21)

6.2. Boundary Construction

The boundary of the realizability region is determined by the conditions:

$$q_{+}(T_{+},T_1)=-q_{-}(T_2,T_{-})+p,$$

(22)

$$\frac{q_{+}(T_{+},T_1)}{T_1}=\frac{-q_{-}(T_2,T_{-})}{T_2}.$$

(23)

which at fixed heat exchange coefficients ${\alpha }_{+}$, ${\alpha }_{-}$ determine the values of $T_1\left(p\right)$ and $T_2\left(p\right)$. The latter allows us to find the machine’s energy conversion efficiency as

$$\eta \left(p\right)=1-\frac{T_2\left(p\right)}{T_1\left(p\right)}.$$

(24)

For $0<p<p^{*}$ the solution of the Equations (22) and (23), and hence, the value of $\eta \left(p\right)$, is not unique, see Figure 3. The area lying to the left of $q_{+}^{*}$ corresponds to a higher energy conversion efficiency value. For $p=p^{*}$ the energy conversion efficiency value is unique and equal to ${\eta }^{*}$. The uniqueness condition for the real root of $p=p^{*}$ allows us to find $p^{*}$ and ${\eta }^{*}$. For $p>p^{*}$, the Equations (22) and (23) have no real roots.

These values can also be found from the solution of the problem

$$q_{+}(T_{+},T_1)+q_{-}(T_{-},T_2)\Rightarrow max$$

(25)

under the condition

$$\frac{q_{+}(T_{+},T_1)}{T_1}+\frac{q_{-}(T_{-},T_2)}{T_2}=0,$$

(26)

which determines the temperatures of the working fluid for the machine at maximum power.

At the root $q_{+}^{max}$ the energy conversion efficiency is zero, therefor $T_1=T_2=T_0$, see (21). The temperature $T_0$ is determined from the equation

$$q_{+}(T_{+},T_0)=-q_{-}(T_{-},T_0).$$

(27)

The left side of this equation decreases monotonically, while the right increases monotonically with increasing $T_0$, so it has a unique solution, which lies in the range $(T_{-},T_{+})$. For “natural” dynamic dependencies the value of $T_0$ depends only on the ratio of heat transfer coefficients.

6.3. Conditions of Independence Efficiency of the Machine in the Mode of Maximum Power from Coefficients of Heat Exchange with Sources

Let us show in which case the ratio of the working fluid temperatures $\frac{T_2}{T_1}$ in the solution of the problem (25) and (26), and hence the efficiency of the heat engine does not depend on the heat transfer coefficients. The Lagrange function for this the problem, has the form

$$L=q_{+}(T_{+},T_1)+q_{-}(T_{-},T_2)+\lambda \left(\frac{q_{+}(T_{+},T_1)}{T_1}+\frac{q_{-}(T_{-},T_2)}{T_2}\right).$$

The conditions for its stationarity on $T_1$ and $T_2$ lead after excluding $\lambda$ to the equation:

$${\left(\frac{T_2}{T_1}\right)}^2=\frac{(q_{-}^{\prime }{T}_2-q_{-})q_{+}^{\prime }}{(q_{+}^{\prime }{T}_1-q_{+})q_{-}^{\prime }}.$$

(28)

Here the sign “ ${}^{\prime }$ ” marks the partial derivatives of the dynamic functions with respect to $T_1$ and $T_2$, respectively. After substituting each of the functions in the equality (28) as

$$q_{+}(T_{+},T_1)={\alpha }_{+}{f}_{+}(T_{+},T_1),q_{-}(T_2,T_{-})={\alpha }_{-}{f}_{-}(T_2,T_{-})$$

(29)

we get

$${\left(\frac{T_2}{T_1}\right)}^2=\frac{(f_{-}^{\prime }{T}_2-f_{-})f_{+}^{\prime }}{(f_{+}^{\prime }{T}_1-f_{+})f_{-}^{\prime }}.$$

(30)

Thus, the temperature ratio for $p=p^{*}$, and hence the energy conversion efficiency (21), depends on the heat transfer coefficients only through $T_1,T_2$, which can be found by the solution of the equation system (30) and (26). In (26), as follows from (29), only the ratio of heat transfer coefficients appears.

If the dynamic dependences are such that the right-hand side of the optimality condition (30) does not depend on the temperatures of the working fluid (for Newtonian dynamics it is equal to $T_{-}/T_{+}$), then the energy conversion efficiency in the maximum power mode does not depend on the value of the heat transfer coefficients. In the general case, it can depend only on their ratio. So, if the heat transfer areas of the machine change proportionally, then its energy conversion efficiency ${\eta }^{*}$ remains unchanged.

6.4. Novikov Case

In a number of cases, the problem of calculating the boundaries of the realizability region is facilitated. This applies to the situation when the coefficients fulfill the relation ${\alpha }_{-}>>{\alpha }_{+}$. For thermal or solar power plants, for example, the heat transfer coefficient of condensing steam with the cold source (cooling water) is usually much greater than the heat transfer coefficient of evaporating water with the fuel gases.

In this case, we can assume that the temperature $T_2=T_{-}$. The relationship between power and heat consumption then takes the form:

$$p=q_{+}\left(1-\frac{T_{-}}{T_1(q_{+},T_{+})}\right),$$

(31)

and the efficiency is

$$\eta =1-\frac{T_{-}}{T_1}.$$

The maximum power condition $\frac{\partial p}{\partial q_{+}}=0$ in this case leads to the equation for $T_1$:

$$T_1^2-T_1{T}_{-}+T_{-}\frac{q_{+}(T_{+},T_1)}{q_{+}^{\prime }(T_{+},T_1)}=0.$$

(32)

The positive root of this equation, lying between $T_{-}$ and $T_{+}$, determines the energy conversion efficiency in the maximum power mode. Since the ratio of the heat flux and its derivative with respect to the temperature $T_1$ does not depend on the heat transfer coefficient, the solution of the Equation (32), and therefore, ${\eta }^{*}$ does not depend on the heat transfer coefficients for any kind of “natural” dynamic function.

7. The Boundary of the Realizability Region for Two Types of Heat Transfer Dynamics

In the following we will consider the realizability region for two well-known engines: the Curzon– Ahlborn engine with 1. Newtonian heat transfer and 2. Fourier heat transfer.

The temperatures: $T_2=T_2(q_{-},T_{-}),T_1=T_1(q_{+},T_{+})$. Given these dependencies and conditions (20) you can find the relationship of p with $\eta$ and $q_{+}$ for specific laws of heat transfer.

7.1. Newtonian Heat Transfer Laws

In this case we have

$$q_{+}={\alpha }_{+}(T_{+}-T_1),q_{-}={\alpha }_{-}(T_{-}-T_2).$$

(33)

Hence, it follows

$$T_1=T_{+}-\frac{q_{+}}{{\alpha }_{+}},T_2=T_{-}-\frac{q_{-}}{{\alpha }_{-}}=T_{-}+\frac{q_{+}}{{\alpha }_{-}}\frac{T_2}{T_1}.$$

We can conclude

$$T_2=\frac{T_{-}}{1-\frac{q_{+}}{{\alpha }_{-}{T}_1}}=\frac{T_{-}}{1-\frac{p}{\eta {\alpha }_{-}{T}_1}}$$

and

$$\frac{T_2}{T_1}=1-\eta =\frac{T_{-}}{T_1-\frac{p}{{\alpha }_{-}\eta }}=\frac{T_{-}}{T_{+}-\frac{p}{\eta {\alpha }_{+}}-\frac{p}{\eta {\alpha }_{-}}}$$

Thus, the power and energy conversion efficiency for Newtonian laws of heat transfer are related by equality:

$$\frac{1-\eta }{\eta }=\frac{T_{-}}{T_{+}\eta -\frac{p}{\overline{\alpha }}}$$

(34)

where $\overline{\alpha }=\frac{{\alpha }_{+}{\alpha }_{-}}{{\alpha }_{+}+{\alpha }_{-}}$. So we have

$$p\left(\eta \right)=\overline{\alpha }\eta \left(T_{+}-T_{-}\frac{1}{1-\eta }\right)$$

(35)

Obviously, for the case when $\eta$ is equal to the Carnot energy conversion efficiency of an reversible heat engine

$$\eta ={\eta }_C=1-\frac{T_{-}}{T_{+}},$$

the power $p\left({\eta }_k\right)=0$, as for $\eta =0$.

Let us find the value of $\eta$, for which the power of the heat engine is maximal, and the value $p^{*}$. It is not difficult to show that the function $p\left(\eta \right)$ is convex up, so the condition for its stationarity determines the maximum

$$\frac{dp}{d\eta }=0\to T_{+}-T_{-}\frac{1}{1-\eta }-\eta T_{-}\frac{1}{{(1-\eta )}^2}=0$$

whence taking into account the fact that $p>0$, we obtain

$${\eta }^{*}=1-\sqrt{T_{-}/T_{+}},$$

(36)

which is the well-known Curzon–Ahlborn efficiency.

Consequently, the maximum power is

$$p^{*}=q_{+}^{*}{\eta }^{*}=\overline{\alpha }\left(T_{+}-\sqrt{T_{+}{T}_{-}}\right)\left(1-\sqrt{\frac{T_{-}}{T_{+}}}\right)=\overline{\alpha }{\left(\sqrt{T_{+}}-\sqrt{T_{-}}\right)}^2.$$

(37)

As follows from the expression (27), the maximum heat flux is

$$q_{+}^{max}=\overline{\alpha }(T_{+}-T_{-}),$$

at which the power of the machine becomes zero.

7.2. The Linear Phenomenological Law

When we have The linear phenomenological law (Fourier heat transfer),

$$q_{+}={\alpha }_{+}\left(\frac{1}{T_1}-\frac{1}{T_{+}}\right),q_{-}={\alpha }_{-}\left(\frac{1}{T_2}-\frac{1}{T_{-}}\right),$$

we conclude

$$\frac{1}{T_1}=\frac{1}{T_{+}}+\frac{q_{+}}{{\alpha }_{+}},\frac{1}{T_2}=\frac{1}{T_{-}}+\frac{q_{-}}{{\alpha }_{-}},$$

and so

$$\frac{T_2}{T_1}=1-\eta =\frac{\frac{1}{T_{+}}+\frac{q_{+}}{{\alpha }_{+}}}{\frac{1}{T_{-}}-\frac{q_{+}}{{\alpha }_{-}}(1-\eta )}.$$

Thus

$$1-\eta =\frac{\frac{1}{T_{+}}+\frac{p}{{\alpha }_{+}\eta }}{\frac{1}{T_{-}}-\frac{p}{{\alpha }_{-}\eta }(1-\eta )},$$

and

$$p\left(\eta \right)=\frac{\eta \left(\frac{1-\eta }{T_{-}}-\frac{1}{T_{+}}\right){\alpha }_{-}{\alpha }_{+}}{{\alpha }_{+}{(1-\eta )}^2+{\alpha }_{-}}.$$

(38)

The maximum possible value of the heat flux is reached at $\eta =0$ and is equal to

$$q_{+}^{max}=\overline{\alpha }\left(\frac{1}{T_{-}}-\frac{1}{T_{+}}\right),$$

(39)

where $\overline{\alpha }$ is again defined as in the Newtonian case.

The power vanishes again at $\eta =0$ and at $\eta ={\eta }_C$, for some $\eta ={\eta }^{*}$ it reaches its maximum. Let us find this value by the condition of stationarity of the right-hand side of the Equation (38). We obtain a quadratic equation with respect to $\eta$:

$$\left(\frac{{\alpha }_{+}}{T_{-}}+\frac{{\alpha }_{+}}{T_{+}}\right){\eta }^{*2}-2\left(\frac{{\alpha }_{+}+{\alpha }_{-}}{T_{-}}\right){\eta }^{*}+({\alpha }_{+}+{\alpha }_{-})\left(\frac{1}{T_{-}}-\frac{1}{T_{+}}\right)=0.$$

(40)

After dividing the left side of the equation by $\frac{{\alpha }_{-}}{T_{-}}$ and denoting

$$\gamma =\frac{{\alpha }_{+}}{{\alpha }_{-}},r=\frac{T_{-}}{T_{+}}=1-{\eta }_C,$$

we get

$$\gamma (1+r){\eta }^{*2}-2(\gamma +1){\eta }^{*}+(1-r)(\gamma +1)=0.$$

(41)

In the case when the heat transfer coefficient at contact with the cold source is much greater than with the hot one, $\gamma$ goes to 0, and the root of the Equation (41) is equal to:

$${\eta }^{*}=0.5(1-r)=0.5{\eta }_C.$$

(42)

In the general case, the machine’s energy conversion efficiency in the maximum power mode is equal to the positive real root of Equation (41):

$${\eta }^{*}=\frac{(1+\gamma )-\sqrt{(1+\gamma )(1+\gamma r^2)}}{\gamma (1+r)}.$$

(43)

In deriving the dependencies between the power and the incoming heat, and between the power and energy conversion efficiency, we used the hypothesis of internal equilibrium of the working fluid, considering the production of entropy in it is equal to zero. If there is entropy production in the system that is not related with heat exchange between the working fluid and the sources, the value of $\sigma$ will be large, and therefore, for the same heat flux $q_{+}$ values $\eta$ and the power p decrease. So the dependence $p\left(q_{+}\right)$, constructed in Figure 3, is the boundary of the realizability region for the irreversible conversion of thermal energy into work in a system with two reservoirs. No real heat engine with non-zero power can work in the region lying above the curve $p\left(q_{+}\right)$, and have an energy conversion efficiency greater than that corresponding to the boundary points of this region realizability. The reasonable boundary of real engines is the left branch of this dependence corresponding to the growth of p with the growth of $q_{+}$.

We should note one important fact: the obtained dependencies do not include equations of the state of the working fluid. They require only knowledge of the heat transfer dynamics.

8. Conclusions

In this article, we show that the cycle heat engine in the maximum power regime for any heat dynamic consists of two isotherms and two adiabatic; we also demostrate the conditions under which its efficiency does not depend on heat transfer coefficient with the source.

Irreversible cycle of a machine with a maximum efficiency can have three isotherms and three adiabats, and two of them can only be in half-cycle heating.

Conditions under which the cycle of a machine with a maximum efficiency has two isothermal parts are obtained and we show that the efficiency in this case can only depend on the ratio of the coefficient of heat exchange.

If the total value of the heat transfer coefficient is given, and the dynamics of interaction with sources are of the same type, then the total contact surface needs to be distributed between the cold and hot sources so that the heat exchange coefficients are equal.