Truck–Shovel vs. In-Pit Crushing and Conveying Systems in Open Pit Mines: A Technical Evaluation for Selecting the Most Effective Transportation System by System Dynamics Modeling
Abstract
:1. Introduction
2. Materials and Methods
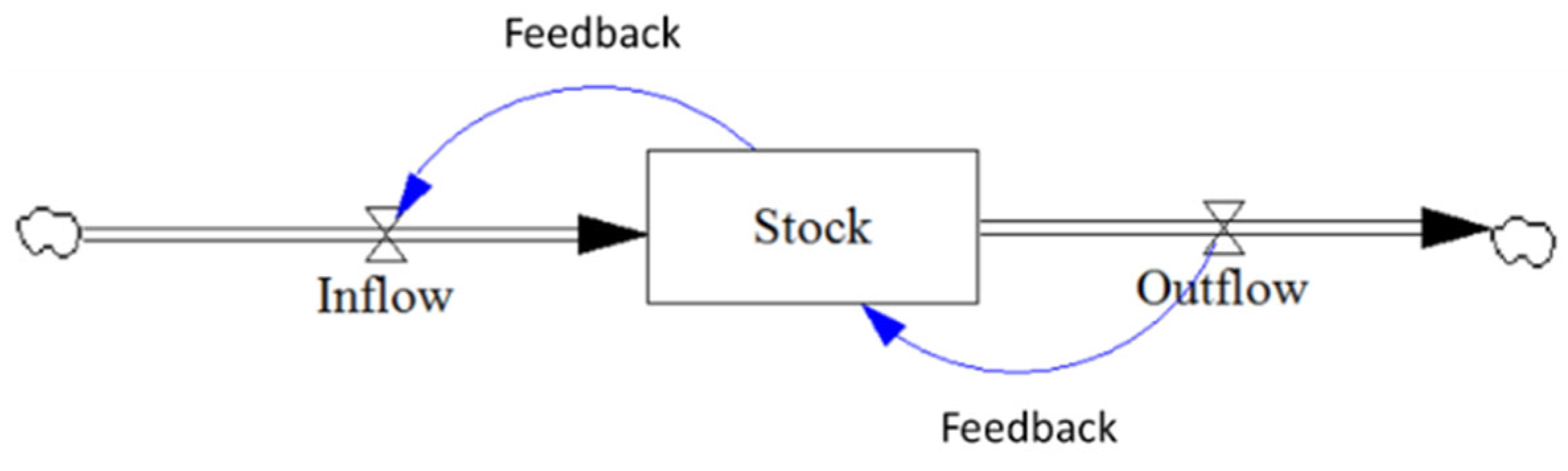
2.1. System Dynamics Modeling
2.2. The Concept of In-Pit Crushing and Conveying (IPCC) Systems
2.3. System Dynamics Modeling for Introducing Technical Index
2.3.1. System Availability
2.3.2. System Utilization
2.3.3. Power Consumption
2.3.4. Technical Index Equation
2.4. Case Study
3. Result and Discussion
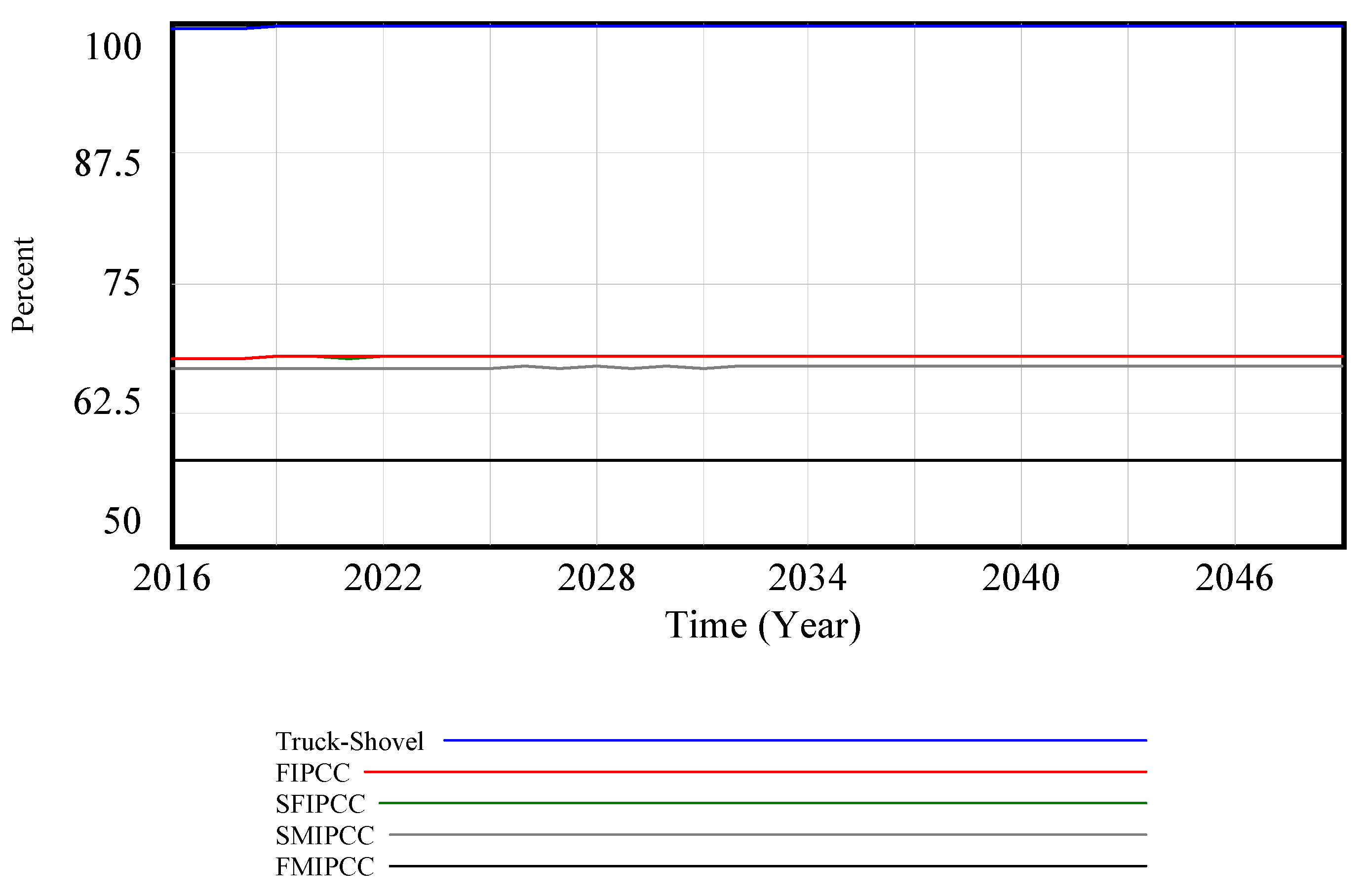
3.1. System Availability
3.2. System Utilization
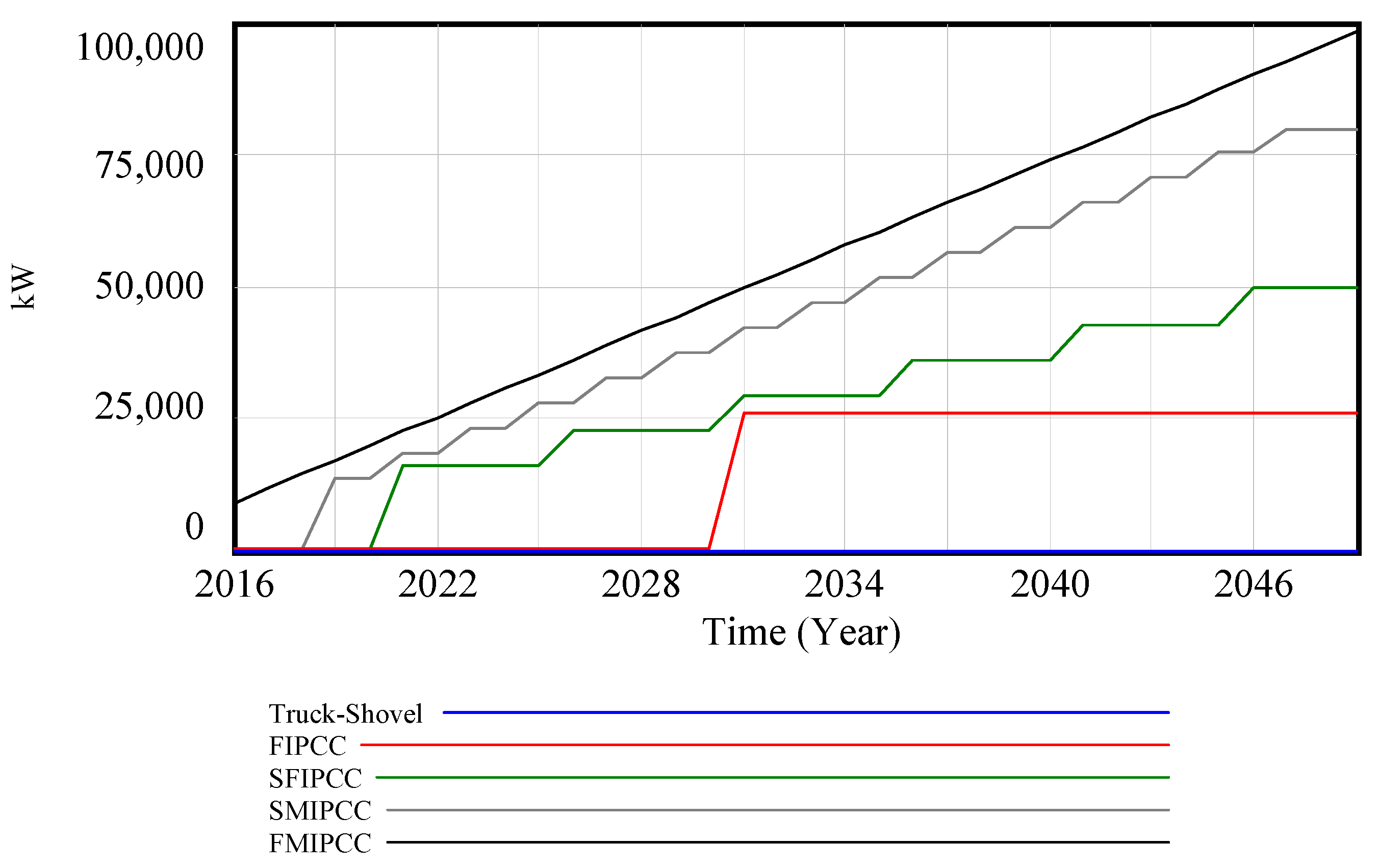
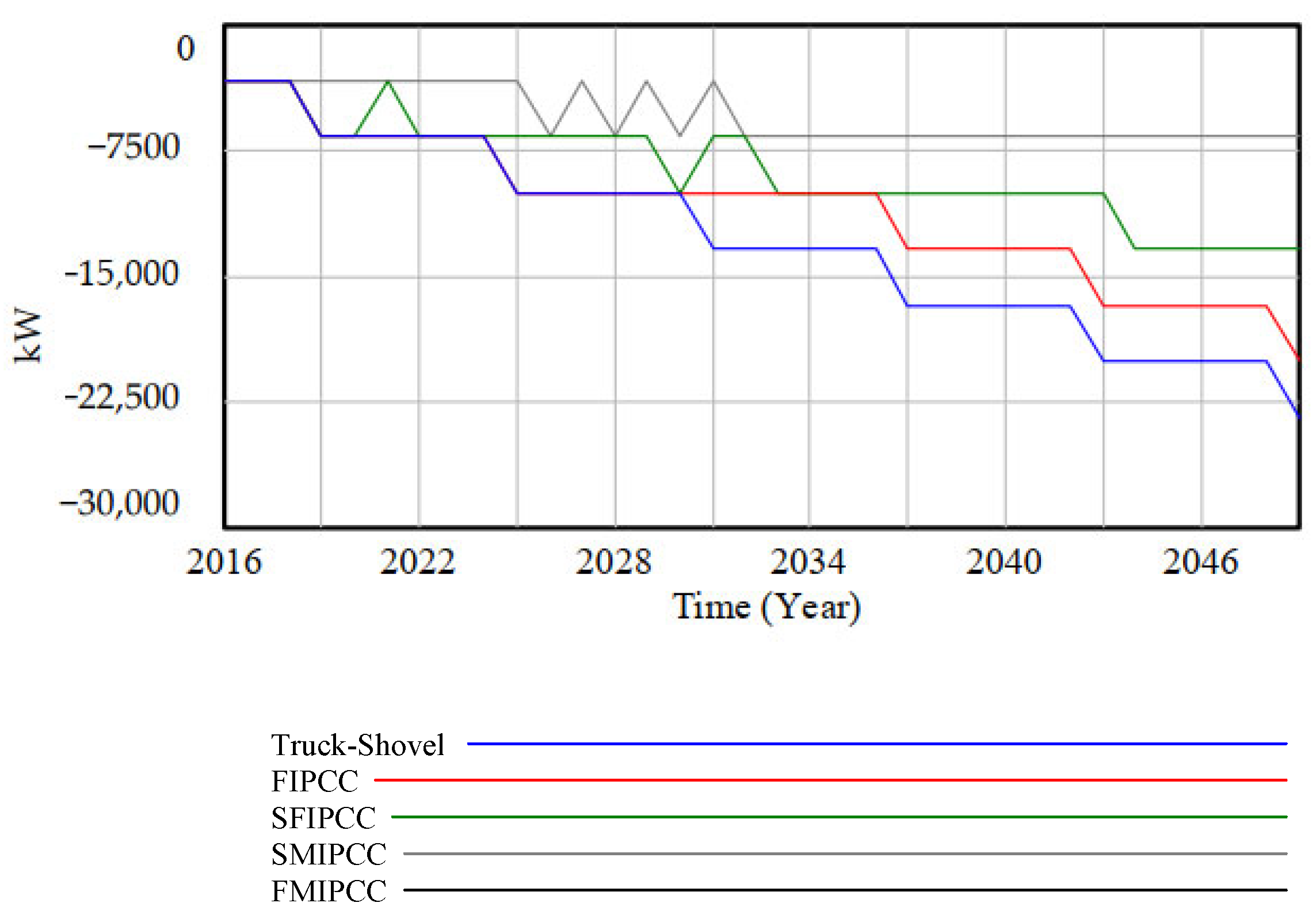
3.3. Power Consumption
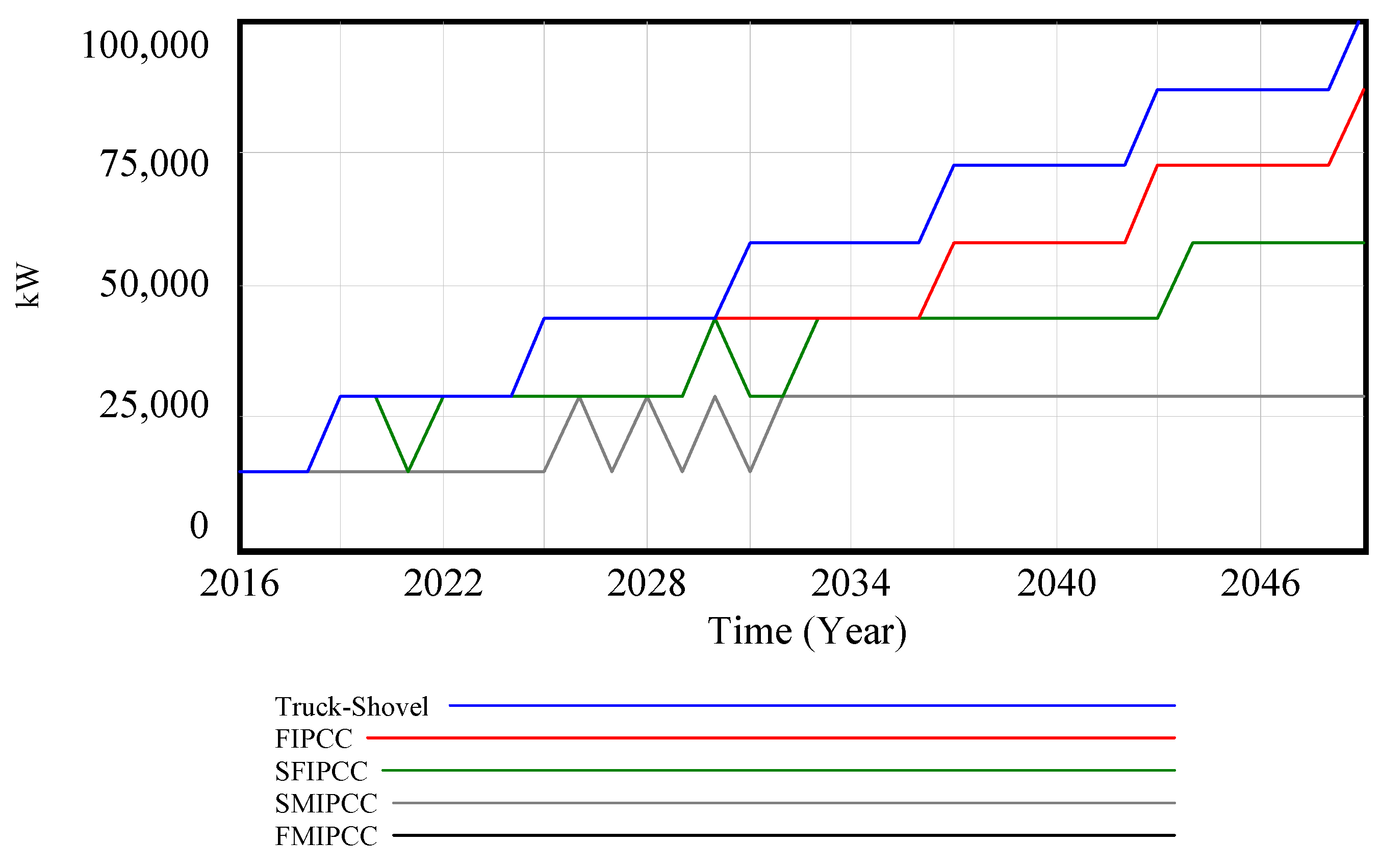
3.4. Technical Index
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Shah, K.; Rehman, S. Modeling and optimization of truck-shovel allocation to mining faces in cement quarry. J. Min. Environ. 2019, 11, 21–30. [Google Scholar]
- Upadhyay, S.P.; Askari-Nasab, H. Dynamic shovel allocation approach to short-term production planning in open-pit mines. Int. J. Min. Reclam. Environ. 2019, 33, 1–20. [Google Scholar] [CrossRef]
- Atchison, T.; Morrison, D. In-pit crushing and conveying bench operations. In Proceedings of the Iron Ore Conference, Pert, WA, Australia, 9–10 September 2011. [Google Scholar]
- Nehring, N.; Knights, P.; Kizil, M.; Hay, E. A comparison of strategic mine planning approaches for in-pit crushing and conveying, and truck/shovel systems. Int. J. Min. Sci. Technol. 2018, 28, 205–214. [Google Scholar] [CrossRef]
- Samavati, M. New Long-Term Production Scheduling Methodologies for Open-Pit Mines; The University of New South Wales: Canberra, NSW, Australia, 2017. [Google Scholar]
- Samavati, M.; Essam, D.; Nehring, N.; Sarker, R. Open-Pit Mine Production Planning and Scheduling: A Research Agenda. In Data and Decision Sciences in Action; Springer International Publishing AG: Cham, Switzerland, 2018; pp. 221–226. [Google Scholar]
- Johnson, M. Impact of In-Pit Crushing and Conveying on Pit Shell Optimization; Deswik Mining Consultants: Brisbane, Australia, 2014. [Google Scholar]
- Al Hasib, N.; Ben-Awuah, E.; Askari-Nasab, H. Review of recent developments in short-term mine planning and IPCC with a research agenda. Min. Technol. 2023, 132, 179–201. [Google Scholar] [CrossRef]
- Shamsi, M.; Pourrahimian, Y.; Rahmanpour, M. Optimisation of open-pit mine production scheduling considering optimum transportation system between truck haulage and semi-mobile in-pit crushing and conveying. Int. J. Min. Reclam. Environ. 2022, 36, 142–158. [Google Scholar] [CrossRef]
- Samavati, M.; Essam, D.; Nehring, M.; Sarker, R. Production planning and scheduling in mining scenarios under IPCC mining systems. Comput. Oper. Res. 2020, 115, 104714. [Google Scholar] [CrossRef]
- Dean, M.; Knights, P.; Kizil, M.S.; Nehring, M. Selection and planning of fully mobile in-pit crusher and conveyor systems for deep open pit metalliferous applications. In Proceedings of the Third international Future Mining Conference, Sydney, NSW, Australia, 4–6 November 2015. [Google Scholar]
- Sturgul, J. How to determine the optimum location of in-pit movable crusher. Int. J. Min. Geol. Eng. 1987, 5, 143–148. [Google Scholar] [CrossRef]
- Rahmanpour, M.; Osanloo, M.; Adibee, N.; AkbarpourShirazi, M. An approach to locate an in-pit crusher in open pit mines. Int. J. Eng. 2014, 9, 1475–1484. [Google Scholar]
- Paricheh, M.; Osanloo, M.; Rahmanpour, M. A heuristic approach for in-pit crusher and conveyor system’s time and location problem in large open-pit mining. Int. J. Min. Reclam. Environ. 2018, 32, 35–55. [Google Scholar] [CrossRef]
- Abbaspour, H.; Drebenstedt, C.; Paricheh, M.; Ritter, R. Optimum location and relocation plan of semi-mobile in-pit crushing and conveying systems in open-pit mines by transportation problem. Int. J. Min. Reclam. Environ. 2019, 33, 297–317. [Google Scholar] [CrossRef]
- Shamsi, M.; Nehring, M. Determination of the optimal transition point between a truck and shovel system and a semi-mobile in-pit crushing and conveying system. J. S. Afr. Inst. Min. Metall. 2021, 121, 497–504. [Google Scholar] [CrossRef]
- Changzhi, Y. In-pit crushing and conveying system in Dexin pit copper haulage optimization for ore transport. In Proceedings of the Fifth Large Open Pit Mining Conference, Kalgoorlie, WA, Australia, 3–5 November 2003. [Google Scholar]
- Konak, G. Selection of the optimum in-pit crusher location for an aggregate producer. J. S. Afr. 2007, 107, 161–166. [Google Scholar]
- Morriss, P. Key Production Drivers in In-Pit Crushing and Conveying (IPCC) Studies. In Proceedings of the The International Conference on Surface Mining 2008 ‘Challenges, Technology, Systems and Solutions’, Johannesburg, South Africa, 5–8 August 2008; The Southern African Institute of Mining and Metallurgy: Johannesburg, South Africa, 2008; pp. 23–34. [Google Scholar]
- McCarthy, B. Evaluating the lack of flexibility against the benefits of in-pit crushing and conveying. In Proceedings of the Optimizing Mine Operations Conference, Toronto, ON, Canada, 10–13 September 2013. [Google Scholar]
- Dzakpata, I.; Knights, P.; Kizil, M.S.; Nehring, M.; Aminossadati, S.M. Truck and Shovel Versus In-Pit Conveyor Systems: A Comparison Of The Valuable Operating Time. In Proceedings of the Coal Operators’ Conference, University of Wollongong, Wollongong NSW, Australia, 10–12 February 2016. [Google Scholar]
- Alpay, S.; Yavuz, M. A Decision Support System for Underground Mining Method Selection. In New Trends in Applied Artificial Intelligence, Proceedings of the International Conference on Industrial, Engineering and Other Applications of Applied Intelligent Systems, Kyoto, Japan, 26–29 June 2007; Okuno, H.G., Ali, M., Eds.; Springer: Berlin/Heidelberg, Germany, 2007; pp. 334–343. [Google Scholar]
- Sontamino, P. Decision Support System of Coal Mine Planning Using System Dynamics Model; Qucosa: Freiberg, Germany, 2014. [Google Scholar]
- Zuo, X.; Zhang, X.; Wang, Y.; Xu, Y.; Tao, W. Decision support system of coal mine exploitation disposition. In Computer Applications in the Mineral Industries; CRC Press: Boca Raton, FL, USA, 2001. [Google Scholar]
- Pruyt, E. Small System Dynamics Models for Big Issues; TU Delft Library: Delft, The Netherlands, 2013. [Google Scholar]
- Radzicki, M.J.; Taylor, R.A. Introduction to System Dynamics, a Systems Approach to Understanding Complex Policy Issues; Sustainable Solutions, Inc.: Washington DC, USA, 1997. [Google Scholar]
- Barlas, Y. System Dynamics; Encyclopedia of Life Support System: Oxford, UK, 2009. [Google Scholar]
- Ritter, R.; Herzog, A.; Drebenstedt, C. Automated Dozer Concept Aims to Cut IPCC Downtime. E&MJ 2014, 215, 52–55. [Google Scholar]
- Radlowski, J.K. In-Pit Crushing and Conveying as an Alternative to an All Truck System in Open Pit Mines; The University of British Columbia: Cracow, Poland, 1988. [Google Scholar]
- Terezopoulos, N. Continuous haulage and in-pit crushing in surface mining. Min. Sci. Technol. 1988, 7, 253–263. [Google Scholar] [CrossRef]
- Hill, J. An assessment of the effectiveness of safety interventions in the field of bulk material handling. In Proceedings of the International Materials Handling Conference, Pretoria, South Africa, 6–8 June 2011. [Google Scholar]
- Ritter, R. Contribution to the Capacity Determination of Semi-Mobile In-Pit Crushing and Conveying Systems. Ph.D. Thesis, Quality Content of Saxony (qucosa), TU Bergakademie Freiberg, Freiberg, Germany, 2016. [Google Scholar]
- Elevli, S.; Elevli, B. Performance measurement of mining equipments by utilizing OEE. Acta Montan. Slovaca 2010, 15, 95–101. [Google Scholar]
- Odeyar, P.; Apel, D.B.; Hall, R.; Zon, B.; Skrzypkowski, K. A Review of Reliability and Fault Analysis Methods for Heavy Equipment and Their Components Used in Mining. Energies 2022, 15, 6263. [Google Scholar] [CrossRef]
- Liu, S.Q.; Kozan, E.; Corry, P.; Masoud, M.; Luo, K. A real-world mine excavators timetabling methodology in open-pit mining. Optim. Eng. 2022, 24, 1493–1535. [Google Scholar] [CrossRef]
- Liu, S.Q.; Lin, Z.; Li, D.; Li, X.; Kozan, E.; Masoud, M. Recent Research Agendas in Mining Equipment Management: A Review. Mining 2022, 2, 769–790. [Google Scholar] [CrossRef]
- Rohani, H.; Roosta, A.K. Calculating Total System Availability. Inf. Serv. Organ. KLM-Air Fr. Amst. 2014. Available online: https://d1.awsstatic.com/whitepapers/architecture/CalculatingTotalSystemAvailability.pdf (accessed on 27 November 2023).
- Shu-zhao, C.; Qing-xiang, C.; Wei, Z.; Lei, Z. Study on new pattern of semi-continuous mining system used in surface mines. Procedia Earth Planet. Sci. 2009, 1, 243–249. [Google Scholar]
- Soofastaei, A.; Aminossadati, S.M.; Kizil, M.S.; Knights, P. Reducing fuel consumption of haul trucks in surface mines using artificial intelligence models. In Proceedings of the Coal Operators’ Conference, Wollongong NSW, Australia, 10–12 February 2016. [Google Scholar]
- Leiva, O. Haulage Profile Advances in Vulcan 9; MAPTEK: Sydney, Australia, 2013. [Google Scholar]
- Assakkaf, I. Machine Power. In Construction Equipment and Methods; University of Maryland: College Park, MD, USA, 2003. [Google Scholar]
- CEMA. Belt Conveyors for Bulk Materials. In Belt Tension, Power, and Drive Engineering; 6 Hrsg.; Conveyor Equipment Manufacturers Association: Bonita Springs, FL, USA, 2007; pp. 85–196. [Google Scholar]
- Catterpillar. 777D Off-Highway Truck; Caterpillar: USA, 2007; Available online: https://s7d2.scene7.com/is/content/Caterpillar/C229910 (accessed on 27 November 2023).
- Kecojevic, V.; Komljenovic, D. Haul truck fuel consumption and CO2 emission under various engine load conditions. Mininig Eng. 2010, 62, 44–48. [Google Scholar]



| State | NS | NT | System Configuration | System Availability |
|---|---|---|---|---|
| I | 1 | 1 | Serial | |
| II | 1 | >1 | Hybrid | |
| III | >1 | 1 | Hybrid | |
| IV | >1 | >1 | Hybrid |
| State | NS | NT | System Configuration | System Availability |
|---|---|---|---|---|
| I | 1 | 1 | Serial | |
| II | 1 | >1 | Hybrid | |
| III | >1 | 1 | Hybrid | |
| IV | >1 | >1 | Hybrid |
| State | NS | System Configuration | System Availability |
|---|---|---|---|
| I | 1 | Serial | |
| II | >1 | Hybrid |
| Parameter | Quantity | Unit | Reference |
|---|---|---|---|
| Mine and mill specification | |||
| Reserve of ore | 700,000,000 | t | |
| Commencement year for ore extraction | 2016 | ||
| Quantity of annual holidays | 15 | days | |
| Duration of daily maintenance hours | 2 | h | |
| Quantity of work shifts | 3 | ||
| Working hours per shift | 8 | h | |
| Rock density | 2.2 | t/m3 | |
| Average ore grade | 0.3 | % | |
| Road gradient | 10 | % | |
| Width of the road | 30 | m | |
| Quantity of faces | 3 | ||
| Quantity of faces in FMIPCC | 1 | ||
| Elevation of the surface | 2100 | m | |
| Elevation of the pit’s bottom | 780 | m | |
| Recovery of the mill | 80 | % | |
| Overall recovery rate for the smelter and refinery | 95 | % | |
| Truck specification | |||
| Capacity of the trucks | 60 | m3 | [43] |
| Speed (loaded truck) | 40 | km/h | |
| Speed (unloaded truck) | 50 | km/h | |
| Loading time | 30 | sec | |
| Availability | 86 | % | [19] |
| Utilization | 86.4 | % | [19] |
| Maneuver on the face | 15 | sec | |
| Maneuvering and unloading | 20 | sec | |
| Delay | 10 | sec | |
| Load factor (loaded truck) | 35 | % | [44] |
| Load factor (unloaded truck) | 25 | % | [44] |
| Truck mass (unloaded) | 165 | t | [43] |
| Rolling resistance | 5 | % | |
| Shovel specification | |||
| Quantity of shovels per face | 1 | ||
| Swell factor | 90 | % | |
| Load cycle time | 20 | sec | |
| Propel time factor | 90 | % | |
| Availability | 86 | % | [19] |
| Utilization | 81 | % | [19] |
| IPCC and spreader specification | |||
| FIPCC availability | 85 | % | [19] |
| FIPCC utilization | 85 | % | [19] |
| SFIPCC availability | 85 | % | [19] |
| SFIPCC utilization | 85 | % | [19] |
| SMIPCC availability | 83.7 | % | [19] |
| SMIPCC utilization | 87.8 | % | [19] |
| FMIPCC availability | 84 | % | [19] |
| FMIPCC utilization | 83.8 | % | [19] |
| Spreader availability | 87 | % | [19] |
| Spreader utilization | 91.7 | % | [19] |
| IPCCs relocation | |||
| The year of relocating the FIPCC | 2030 | ||
| The initial year of relocating SFIPCC | 2020 | ||
| The final year of relocating SFIPCC | 2046 | ||
| The initial year of relocating SMIPCC | 2018 | ||
| The final year of relocating SMIPCC | 2046 | ||
| The interval years of relocation for SFIPCC | 5 | ||
| The interval years of relocation for SMIPCC | 2 | ||
| Depth of relocation for FIPCC | 250 | m | |
| Depth of relocation for SFIPCC | 100 | m | |
| Depth of relocation for SMPCC | 70 | m | |
| Conveyor belt specification | |||
| Initial speed of the conveyor belt | 0 | ft/min | |
| Surcharge angle | 20 | ° | |
| Inclination of the conveyor belt | 16 | ° | |
| Quantity of tight pulleys | 0 | ||
| Quantity of slack pulleys | 0 | ||
| Ambient temperature correction factor | 3 | [42] | |
| Carrying run factor | 0.018 | [42] | |
| Fractional resistance of plows | 0 | lbs | |
| Resistance of trippers and stackers | 0 | lbs | |
| Resistance of belt-cleaning devices | 0 | lbs | |
| Skirtboard length | 10 | ft | |
| Skirtboard friction factor | 0.276 | [42] | |
| Depth of material touching skirtboard | 10 | ft | |
| Idler spacing | 3 | in | |
| Idler diameter | 6 | in | |
| Length of each conveyor set | 150 | m | |
| Availability | 92.2 | % | [19] |
| Utilization | 89.9 | % | [19] |
| Transportation Systems | Year | |||||
|---|---|---|---|---|---|---|
| 2016–2018 (2 Year) | 2018–2020 (2 Year) | 2020–2022 (2 Years) | 2022–2024 (2 Year) | 2024–2025 (1 Year) | 2025–2049 (24 Years) | |
| Rank | ||||||
| Truck–Shovel | 2 | 1 | 2 | 1 | 1 | 1 |
| FIPCC | 3 | 4 | 4 | 4 | 3 | 3 |
| SFIPCC | 3 | 4 | 5 | 5 | 5 | 5 |
| SMIPCC | 4 | 3 | 3 | 3 | 4 | 4 |
| FMIPCC | 1 | 2 | 1 | 2 | 2 | 2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abbaspour, H.; Drebenstedt, C. Truck–Shovel vs. In-Pit Crushing and Conveying Systems in Open Pit Mines: A Technical Evaluation for Selecting the Most Effective Transportation System by System Dynamics Modeling. Logistics 2023, 7, 92. https://doi.org/10.3390/logistics7040092
Abbaspour H, Drebenstedt C. Truck–Shovel vs. In-Pit Crushing and Conveying Systems in Open Pit Mines: A Technical Evaluation for Selecting the Most Effective Transportation System by System Dynamics Modeling. Logistics. 2023; 7(4):92. https://doi.org/10.3390/logistics7040092
Chicago/Turabian StyleAbbaspour, Hossein, and Carsten Drebenstedt. 2023. "Truck–Shovel vs. In-Pit Crushing and Conveying Systems in Open Pit Mines: A Technical Evaluation for Selecting the Most Effective Transportation System by System Dynamics Modeling" Logistics 7, no. 4: 92. https://doi.org/10.3390/logistics7040092
APA StyleAbbaspour, H., & Drebenstedt, C. (2023). Truck–Shovel vs. In-Pit Crushing and Conveying Systems in Open Pit Mines: A Technical Evaluation for Selecting the Most Effective Transportation System by System Dynamics Modeling. Logistics, 7(4), 92. https://doi.org/10.3390/logistics7040092





