Bone Density and Implant Primary Stability. A Study on Equine Bone Blocks
Abstract
1. Introduction
2. Materials and Methods
2.1. Equine Cancellous Bone Blocks
2.2. Implants
2.3. Instantaneous Torque Measuring Micromotor
2.4. Experimental Procedure
2.5. Data Analysis
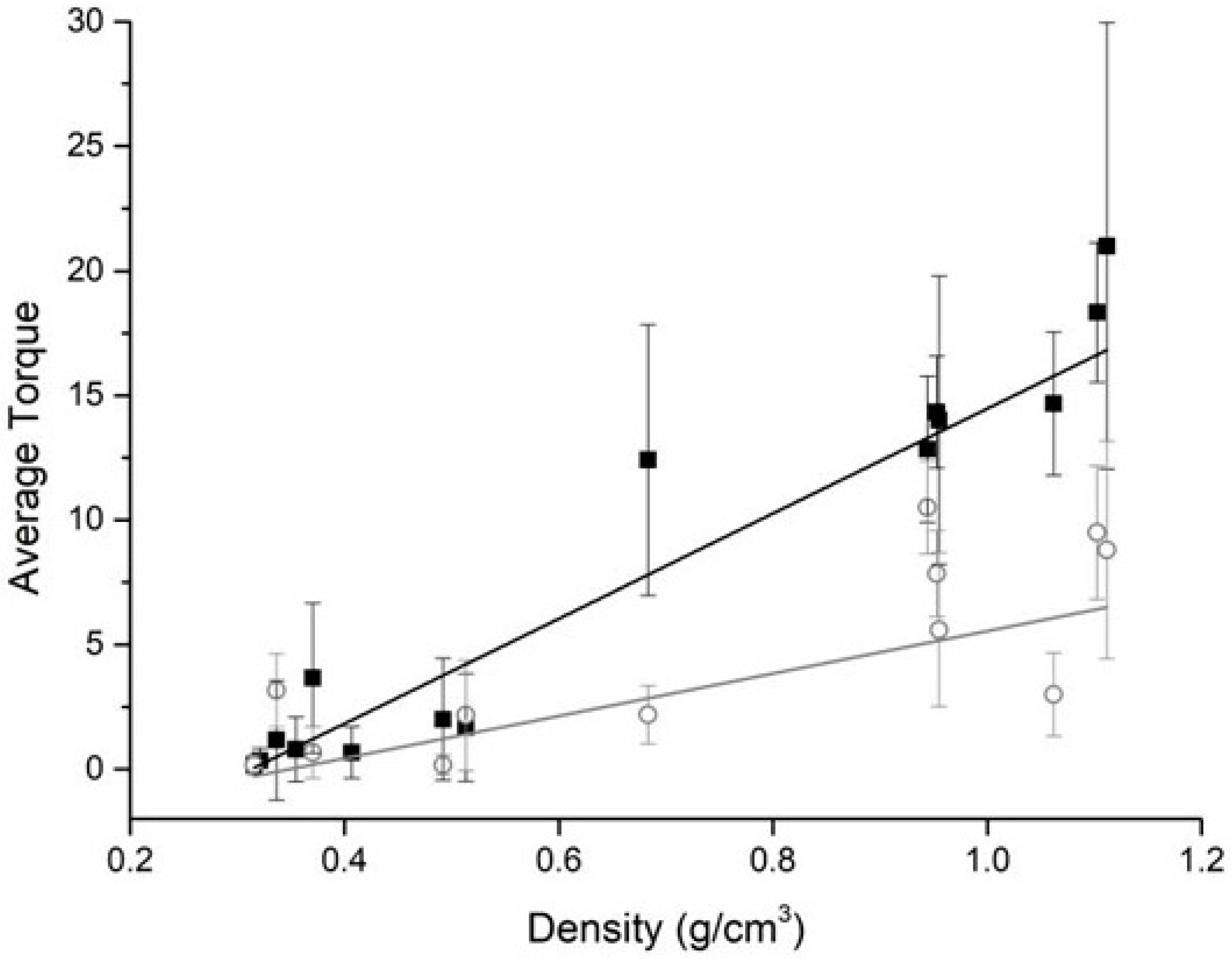
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Marquezan, M.; Osorio, A.; Sant’Anna, E.; Souza, M.M.; Maia, L. Does bone mineral density influence the primary stability of dental implants? A systematic review. Clin. Oral Implants Res. 2012, 23, 767–774. [Google Scholar] [CrossRef] [PubMed]
- Molly, L. Bone density and primary stability in implant therapy. Clin. Oral Implants Res. 2006, 17 (Suppl. 2), 124–135. [Google Scholar] [CrossRef]
- Javed, F.; Ahmed, H.B.; Crespi, R.; Romanos, G.E. Role of primary stability for successful osseointegration of dental implants: Factors of influence and evaluation. Interv. Med. Appl. Sci. 2013, 5, 162–167. [Google Scholar] [CrossRef] [PubMed]
- Javed, F.; Romanos, G.E. The role of primary stability for successful immediate loading of dental implants. A literature review. J. Dent. 2010, 38, 612–620. [Google Scholar] [CrossRef] [PubMed]
- Howashi, M.; Tsukiyama, Y.; Ayukawa, Y.; Isoda-Akizuki, K.; Kihara, M.; Imai, Y.; Sogo, M.; Koyano, K. Relationship between the CT Value and Cortical Bone Thickness at Implant Recipient Sites and Primary Implant Stability with Comparison of Different Implant Types. Clin. Implant Dent. Relat. Res. 2016, 18, 107–116. [Google Scholar] [CrossRef]
- Hong, J.; Lim, Y.J.; Park, S.O. Quantitative biomechanical analysis of the influence of the cortical bone and implant length on primary stability. Clin. Oral. Implants Res. 2012, 23, 1193–1197. [Google Scholar] [CrossRef]
- Morgan, E.F.; Bouxsein, M.L. Bone Biomechanics. In Principles of Bone Biology, 3rd ed.; Bilezikian, P.J., Raisz, L.G., Martin, J.T., Eds.; Academic Press: Cambridge, MA, USA, 2008; Volume 1, pp. 29–45. [Google Scholar]
- Wallace, M.J. Skeletal Hard Tissue Biomechanics. In Basic and Applied Bone Biology, 2nd ed.; Burr, D.B., Allen, M.R., Eds.; Academic Press: Cambridge, MA, USA, 2019; Volume 1, pp. 125–140. [Google Scholar]
- Robertson, D.M.; Smith, D.C. Compressive strength of mandibular bone as a function of microstructure and strain rate. J. Biomech. 1978, 11, 455–471. [Google Scholar] [CrossRef]
- Schaffler, M.B.; Burr, D.B. Stiffness of compact bone: Effects of porosity and density. J. Biomech. 1988, 21, 13–16. [Google Scholar] [CrossRef]
- Rho, J.Y.; Kuhn-Spearing, L.; Zioupos, P. Mechanical properties and the hierarchical structure of bone. Med. Eng. Phys. 1998, 20, 92–102. [Google Scholar] [CrossRef]
- Ko, Y.C.; Huang, H.L.; Shen, Y.W.; Cai, J.Y.; Fuh, L.J.; Hsu, J.T. Variations in crestal cortical bone thickness at dental implant sites in different regions of the jawbone. Clin. Implant Dent. Relat. Res. 2017, 19, 440–446. [Google Scholar] [CrossRef]
- Wang, R.; Eppell, S.J.; Nguyen, C.; Morris, N. Relative Contribution of Trabecular and Cortical Bone to Primary Implant Stability: An In Vitro Model Study. J. Oral Implantol. 2016, 42, 145–152. [Google Scholar] [CrossRef] [PubMed]
- De Oliveira Nicolau Mantovani, A.K.; de Mattias Sartori, I.A.; Azevedo-Alanis, L.R.; Tiossi, R.; Fontão, F.N.G.K. Influence of cortical bone anchorage on the primary stability of dental implants. Oral Maxillofac Surg. 2018, 22, 297–301. [Google Scholar] [CrossRef] [PubMed]
- Degidi, M.; Daprile, G.; Piattelli, A. Influence of underpreparation on primary stability of implants inserted in poor quality bone sites: An in vitro study. J. Oral Maxillofac. Surg. 2015, 73, 1084–1088. [Google Scholar] [CrossRef] [PubMed]
- Iezzi, G.; Scarano, A.; Di Stefano, D.A.; Arosio, P.; Doi, K.; Ricci, L.; Piattelli, A.; Perrotti, V. Correlation between the bone density recorded by a computerized implant motor and by a histomorphometric analysis: A preliminary in vitro study on bovine ribs. Clin. Implant Dent. Relat. Res. 2015, 17 (Suppl. 1), e35–e44. [Google Scholar] [CrossRef] [PubMed]
- Di Stefano, D.A.; Arosio, P. Correlation between Bone Density and Instantaneous Torque at Implant Site Preparation: A Validation on Polyurethane Foam Blocks of a Device Assessing Density of Jawbones. Int. J. Oral Maxillofac. Implants 2016, 31, e128–e135. [Google Scholar] [CrossRef] [PubMed]
- Degidi, M.; Daprile, G.; Piattelli, A.; Iezzi, G. Development of a new implant primary stability parameter: Insertion torque revisited. Clin. Implant Dent. Relat. Res. 2013, 15, 637–644. [Google Scholar] [CrossRef] [PubMed]
- Kim, S.H.; Lee, S.J.; Cho, I.S.; Kim, S.K.; Kim, T.W. Rotational resistance of surface-treated mini-implants. Angle Orthod. 2009, 79, 899–907. [Google Scholar] [CrossRef]
- Park, K.J.; Kwon, J.Y.; Kim, S.K.; Heo, S.J.; Koak, J.Y.; Lee, J.H.; Lee, S.J.; Kim, T.H.; Kim, M.J. The relationship between implant stability quotient values and implant insertion variables: A clinical study. J. Oral Rehabil. 2012, 39, 151–159. [Google Scholar] [CrossRef]
- Iezzi, G.; Filippone, A.; Stefano, D.A.; Arosio, P.; Piattelli, A.; Scarano, A.; Perrotti, V. A site-specific intraoperative measurement of bone-to-implant contact during implant insertion: A study on bovine ribs using a computerized implant motor. J. Dent. Sci. 2015, 10, 21–27. [Google Scholar] [CrossRef]
- Cappare, P.; Vinci, R.; Di Stefano, D.A.; Traini, T.; Pantaleo, G.; Gherlone, E.F.; Gastaldi, G. Correlation between Initial BIC and the Insertion Torque/Depth Integral Recorded with an Instantaneous Torque-Measuring Implant Motor: An in vivo Study. Clin. Implant Dent. Relat. Res. 2015, 17 (Suppl. 2), e613–e620. [Google Scholar] [CrossRef]
- Arosio, P.; Greco, G.B.; Zaniol, T.; Iezzi, G.; Perrotti, V.; Di Stefano, D.A. Sinus augmentation and concomitant implant placement in low bone-density sites. A retrospective study on an undersized drilling protocol and primary stability. Clin. Implant Dent. Relat. Res. 2018, 20, 151–159. [Google Scholar] [CrossRef] [PubMed]
- Degidi, M.; Daprile, G.; Piattelli, A. Influence of Stepped Osteotomy on Primary Stability of Implants Inserted in Low-Density Bone Sites: An In Vitro Study. Int. J. Oral Maxillofac. Implant 2017, 32, 37–41. [Google Scholar] [CrossRef] [PubMed]
- Di Stefano, D.A.; Arosio, P.; Gastaldi, G.; Gherlone, E. The insertion torque-depth curve integral as a measure of implant primary stability: An in vitro study on polyurethane foam blocks. J. Prosthet. Dent. 2018, 120, 706–714. [Google Scholar] [CrossRef] [PubMed]
- ASTM F1839-08(2016). Standard Specification for Rigid Polyurethane Foam for Use as a Standard Material for Testing Orthopaedic Devices and Instruments; ASTM International: West Conshohocken, PA, USA, January 2016; Available online: www.astm.org (accessed on 15 March 2019).
- Grant, J.A.; Bishop, N.E.; Gotzen, N.; Sprecher, C.; Honl, M.; Morlock, M.M. Artificial composite bone as a model of human trabecular bone: The implant-bone interface. J. Biomech. 2007, 40, 1158–1164. [Google Scholar] [CrossRef] [PubMed]
- De Angelis, N.; Scivetti, M. Lateral ridge augmentation using an equine flex bone block infused with recombinant human platelet-derived growth factor BB: A clinical and histologic study. Int. J. Periodontics Restor. Dent. 2011, 31, 383–388. [Google Scholar]
- Di Stefano, D.A.; Gastaldi, G.; Vinci, R.; Cinci, L.; Pieri, L.; Gherlone, E. Histomorphometric Comparison of Enzyme-Deantigenic Equine Bone and Anorganic Bovine Bone in Sinus Augmentation: A Randomized Clinical Trial with 3-Year Follow-Up. Int. J. Oral Maxillofac. Implants 2015, 30, 1161–1167. [Google Scholar] [CrossRef]
- Di Stefano, D.A.; Gastaldi, G.; Vinci, R.; Polizzi, E.M.; Cinci, L.; Pieri, L.; Gherlone, E. Bone Formation Following Sinus Augmentation with an Equine-Derived Bone Graft: A Retrospective Histologic and Histomorphometric Study with 36-Month Follow-up. Int. J. Oral Maxillofac. Implants 2016, 31, 406–412. [Google Scholar] [CrossRef]
- Eser, C.; Gencel, E.; Gokdogan, M.; Kesiktas, E.; Yavuz, M. Comparison of autologous and heterologous bone graft stability effects for filling maxillary bone gap after Le Fort I osteotomy. Adv. Clin. Exp. Med. 2015, 24, 341–348. [Google Scholar] [CrossRef]
- Iorio, R.; Pagnottelli, M.; Vadala, A.; Giannetti, S.; Di Sette, P.; Papandrea, P.; Conteduca, F.; Ferretti, A. Open-wedge high tibial osteotomy: Comparison between manual and computer-assisted techniques. Knee Surg. Sports Traumatol. Arthrosc. 2013, 21, 113–119. [Google Scholar] [CrossRef]
- Mattioli, B.; Iacoviello, P.; Aldiano, C.; Verrina, G. Subcranial Le Fort III Advancement with Equine-Derived Bone Grafts to Correct Syndromic Midfacial Hypoplasia: A Case Report. J. Maxillofac. Oral Surg. 2018, 17, 296–300. [Google Scholar] [CrossRef]
- Pistilli, R.; Signorini, L.; Pisacane, A.; Lizio, G.; Felice, P. Case of severe bone atrophy of the posterior maxilla rehabilitated with blocks of equine origin bone: Histological results. Implant Dent. 2013, 22. [Google Scholar] [CrossRef] [PubMed]
- Santini, S.; Barbera, P.; Modena, M.; Schiavon, R.; Bonato, M. Equine-derived bone substitutes in orthopedics and traumatology: authors’ experience. Minerva Chir. 2011, 66, 63–72. [Google Scholar] [PubMed]
- Sonmez, M.M.; Armagan, R.; Ugurlar, M.; Eren, T. Allografts versus Equine Xenografts in Calcaneal Fracture Repair. J. Foot Ankle Surg. 2017, 56, 510–513. [Google Scholar] [CrossRef] [PubMed]
- Friberg, B.; Sennerby, L.; Roos, J.; Johansson, P.; Strid, C.G.; Lekholm, U. Evaluation of bone density using cutting resistance measurements and microradiography: An in vitro study in pig ribs. Clin. Oral Implants Res. 1995, 6, 164–171. [Google Scholar] [CrossRef] [PubMed]
- Friberg, B.; Sennerby, L.; Roos, J.; Lekholm, U. Identification of bone quality in conjunction with insertion of titanium implants. A pilot study in jaw autopsy specimens. Clin. Oral Implants Res. 1995, 6, 213–219. [Google Scholar] [CrossRef] [PubMed]
- Sennerby, L.; Meredith, N. Implant stability measurements using resonance frequency analysis: Biological and biomechanical aspects and clinical implications. Periodontol 2000 2008, 47, 51–66. [Google Scholar] [CrossRef]




| Block | Density (g/cm3) | Body Preparation (∅, mm) | Head Preparation (∅, mm) |
|---|---|---|---|
| 1 | 0.316 ± 0.003 | 2.8 | 3.7 |
| 2 | 0.321 ± 0.005 | - | - |
| 3 | 0.337 ± 0.003 | - | - |
| 4 | 0.355 ± 0.003 | 2.8 | 3.7 |
| 5 | 0.371 ± 0.006 | - | - |
| 6 | 0.407 ± 0.006 | 2.8 | 3.7 |
| 7 | 0.492 ± 0.008 | - | - |
| 8 | 0.513 ± 0.006 | - | - |
| 9 | 0.683 ± 0.203 | 3.2 | 3.7 |
| 10 | 0.944 ± 0.114 | - | - |
| 11 | 0.953 ± 0.068 | - | - |
| 12 | 0.955 ± 0.072 | 3.4 | 3.7 |
| 13 | 1.062 ± 0.062 | - | - |
| 14 | 1.102 ± 0.115 | - | - |
| 15 | 1.112 ± 0.044 | 3.4 | 4.0 |
| I | IT | RT | ISQ | |
| I | 1 | 0.9276 * | 0.9429 * | 0.8857 * |
| - | 0.008 | 0.005 | 0.019 | |
| IT | 0.9861 * | 1 | 0.9856 * | 0.8986 * |
| <0.001 | - | <0.001 | 0.014 | |
| RT | 0.9973 * | 0.9856 * | 1 | 0.9429 * |
| <0.001 | <0.001 | - | 0.005 | |
| ISQ | 0.7714 | 0.8117 * | 0.77143 | 1 |
| 0.072 | 0.049 | 0.072 | - |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Orlando, F.; Arosio, F.; Arosio, P.; Di Stefano, D.A. Bone Density and Implant Primary Stability. A Study on Equine Bone Blocks. Dent. J. 2019, 7, 73. https://doi.org/10.3390/dj7030073
Orlando F, Arosio F, Arosio P, Di Stefano DA. Bone Density and Implant Primary Stability. A Study on Equine Bone Blocks. Dentistry Journal. 2019; 7(3):73. https://doi.org/10.3390/dj7030073
Chicago/Turabian StyleOrlando, Francesco, Federico Arosio, Paolo Arosio, and Danilo Alessio Di Stefano. 2019. "Bone Density and Implant Primary Stability. A Study on Equine Bone Blocks" Dentistry Journal 7, no. 3: 73. https://doi.org/10.3390/dj7030073
APA StyleOrlando, F., Arosio, F., Arosio, P., & Di Stefano, D. A. (2019). Bone Density and Implant Primary Stability. A Study on Equine Bone Blocks. Dentistry Journal, 7(3), 73. https://doi.org/10.3390/dj7030073




