Magnetic Transitions in the Co-Modified Mn2Sb System
Abstract
:1. Introduction
2. Results and Discussion
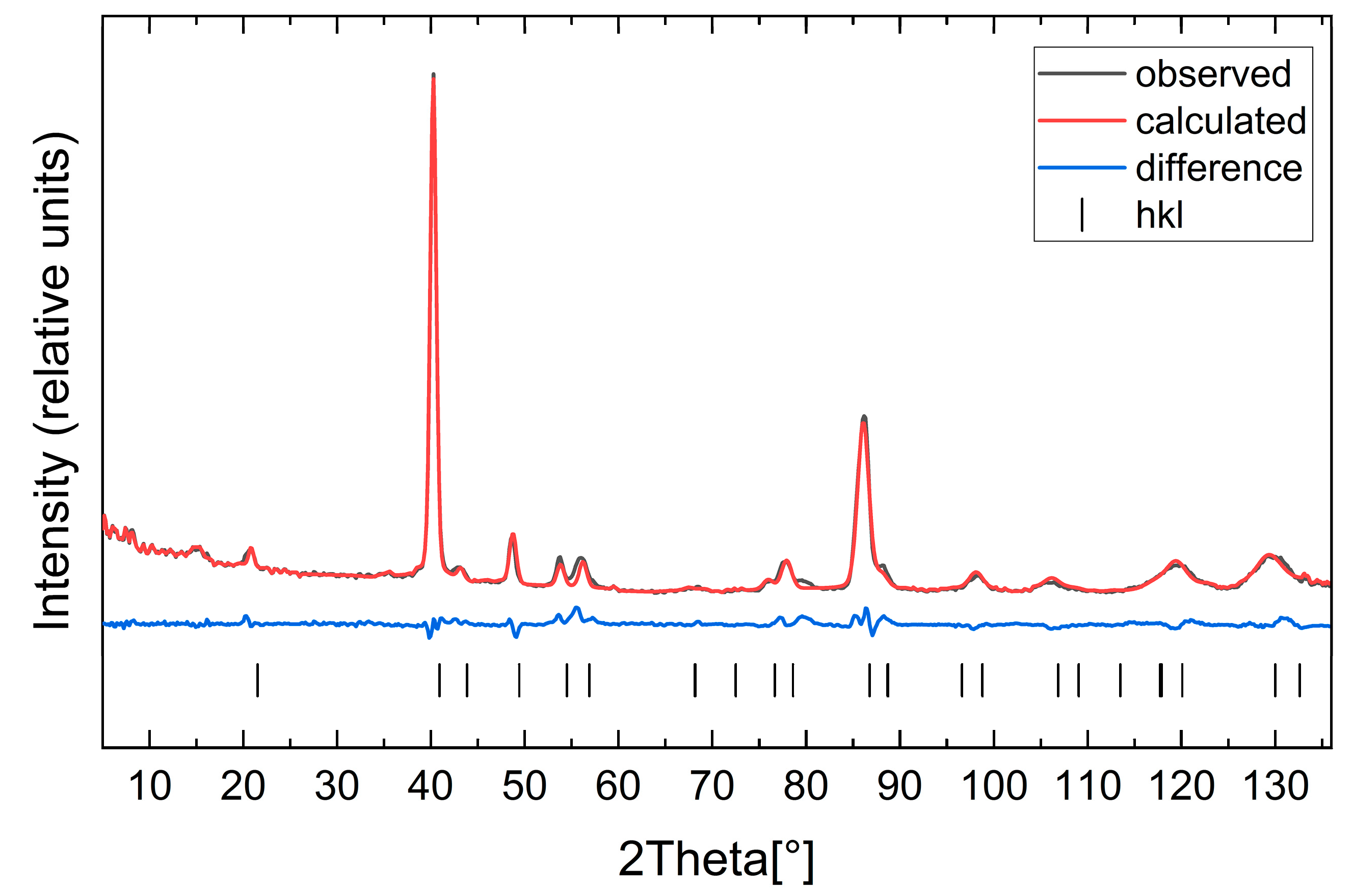
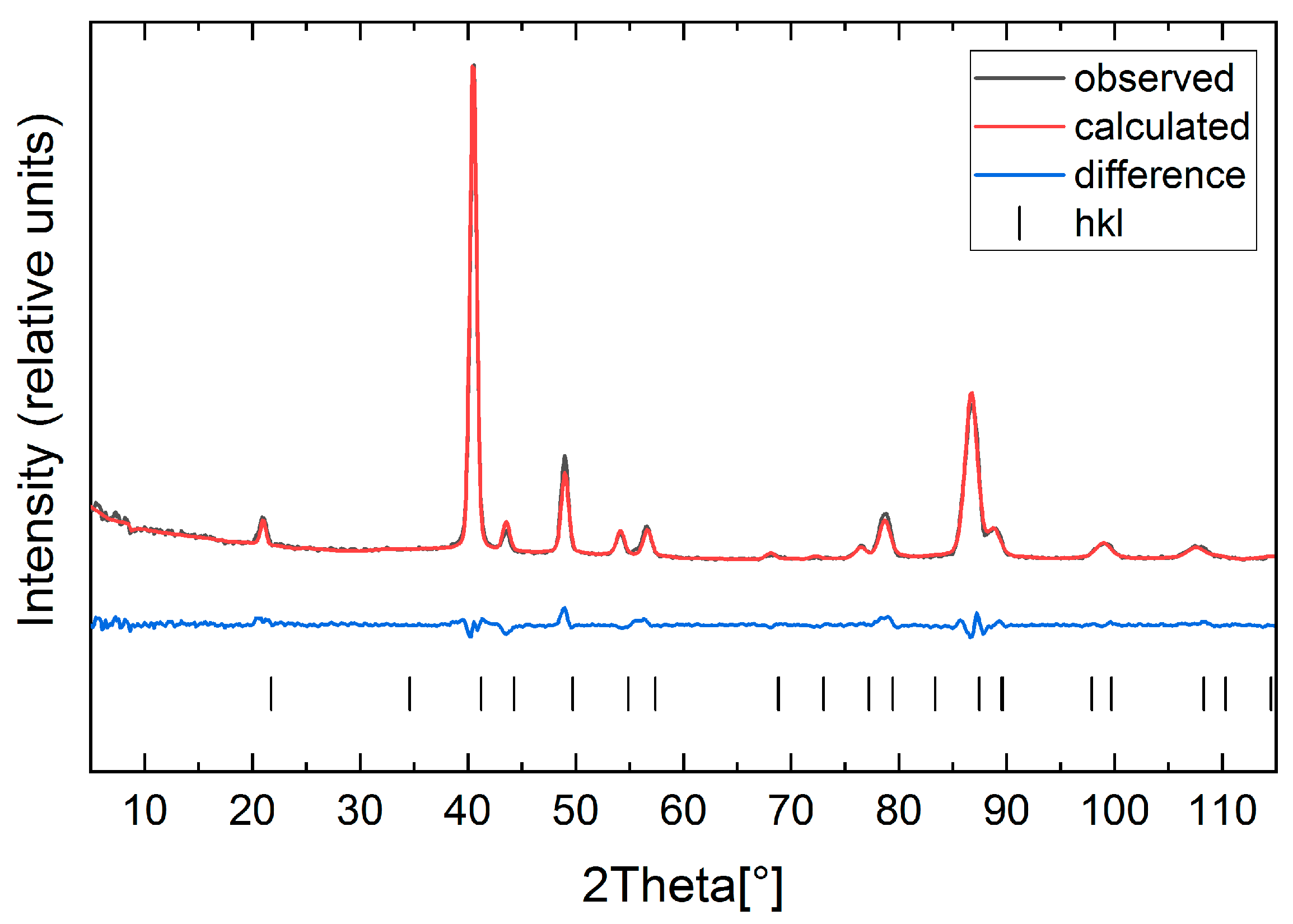
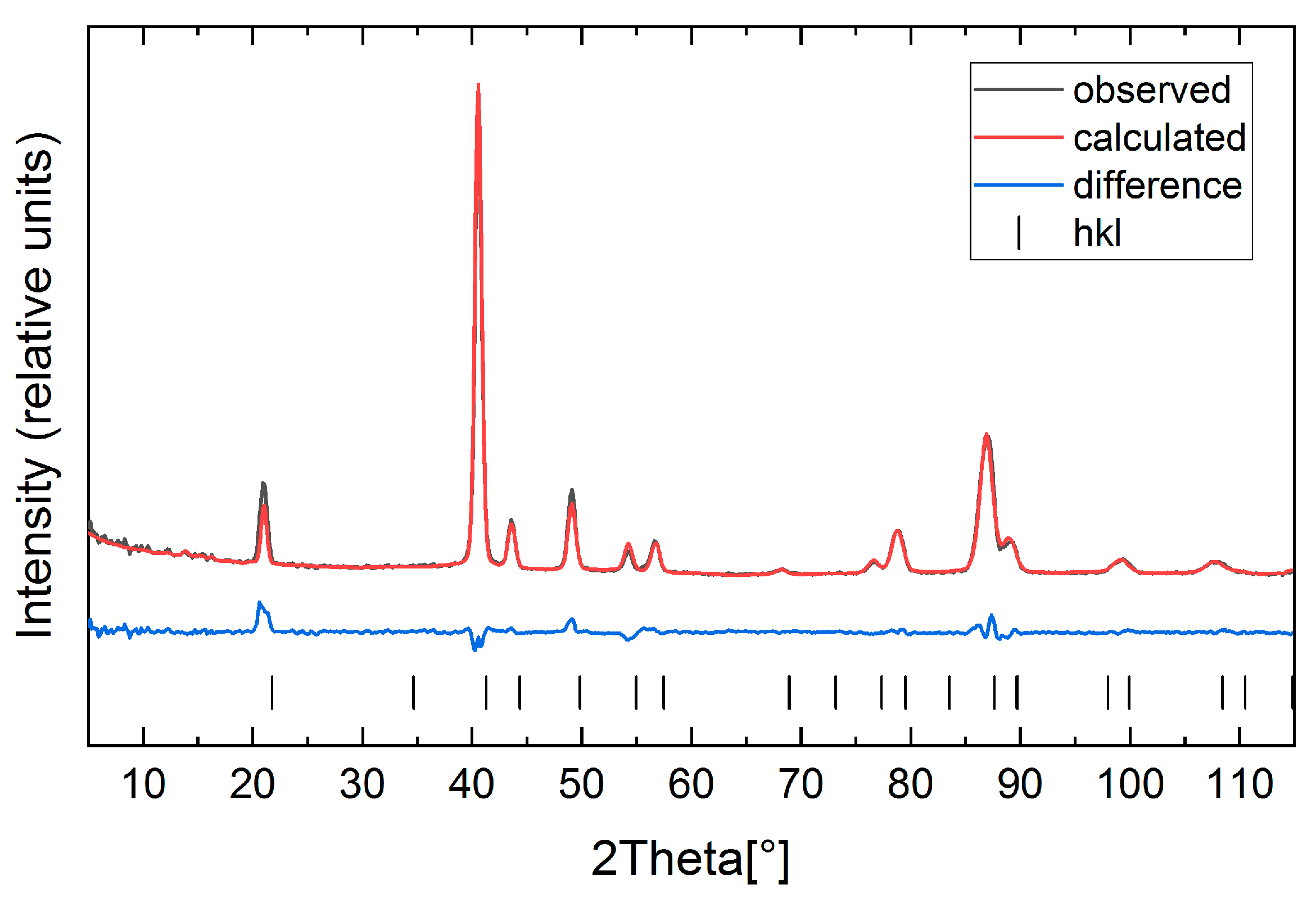
2.1. Chemical Analysis and Crystal Structure
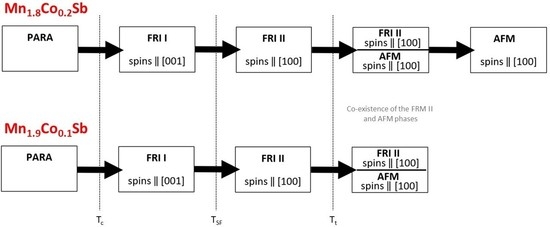
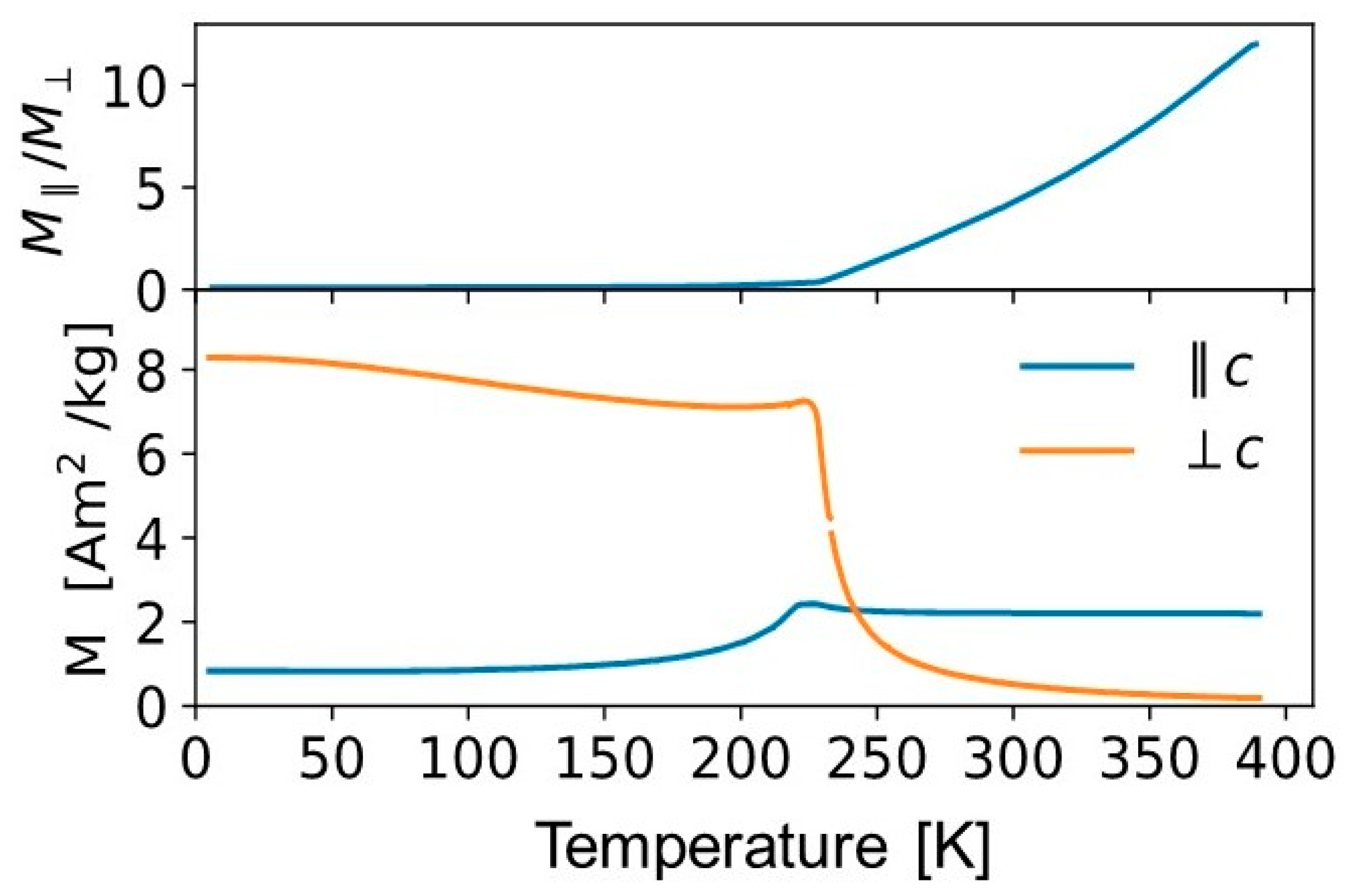
2.2. Magnetic Properties
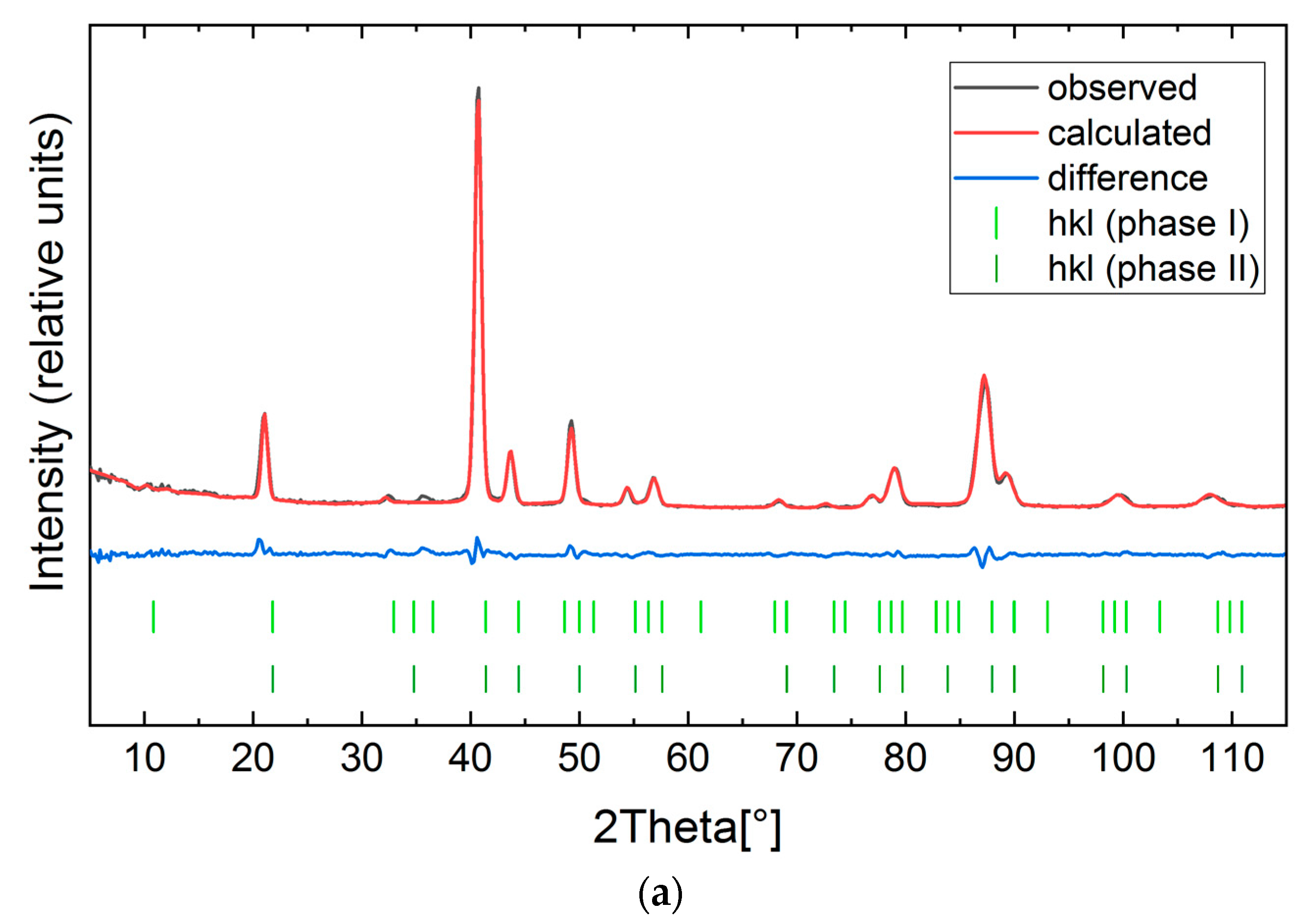
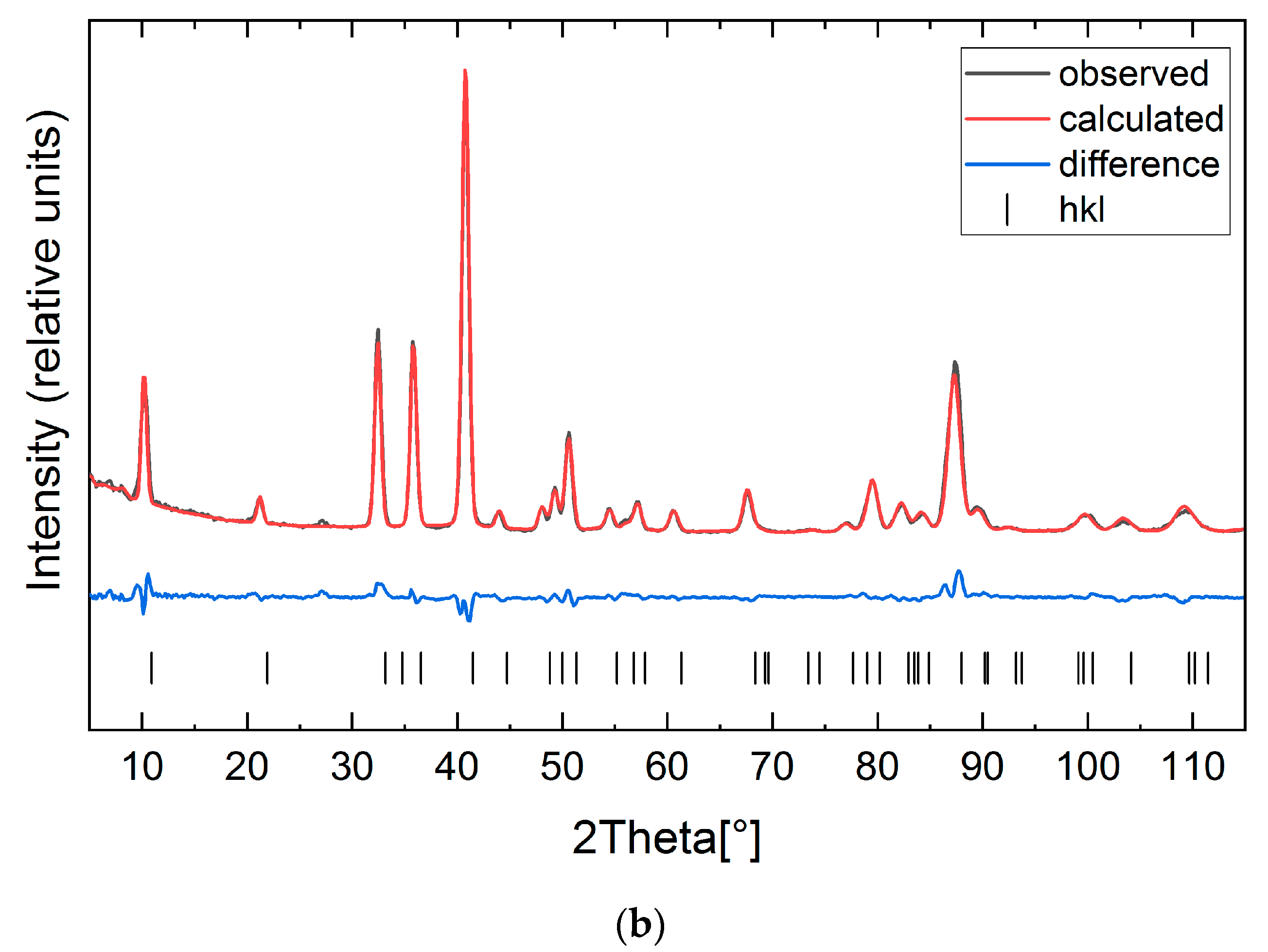
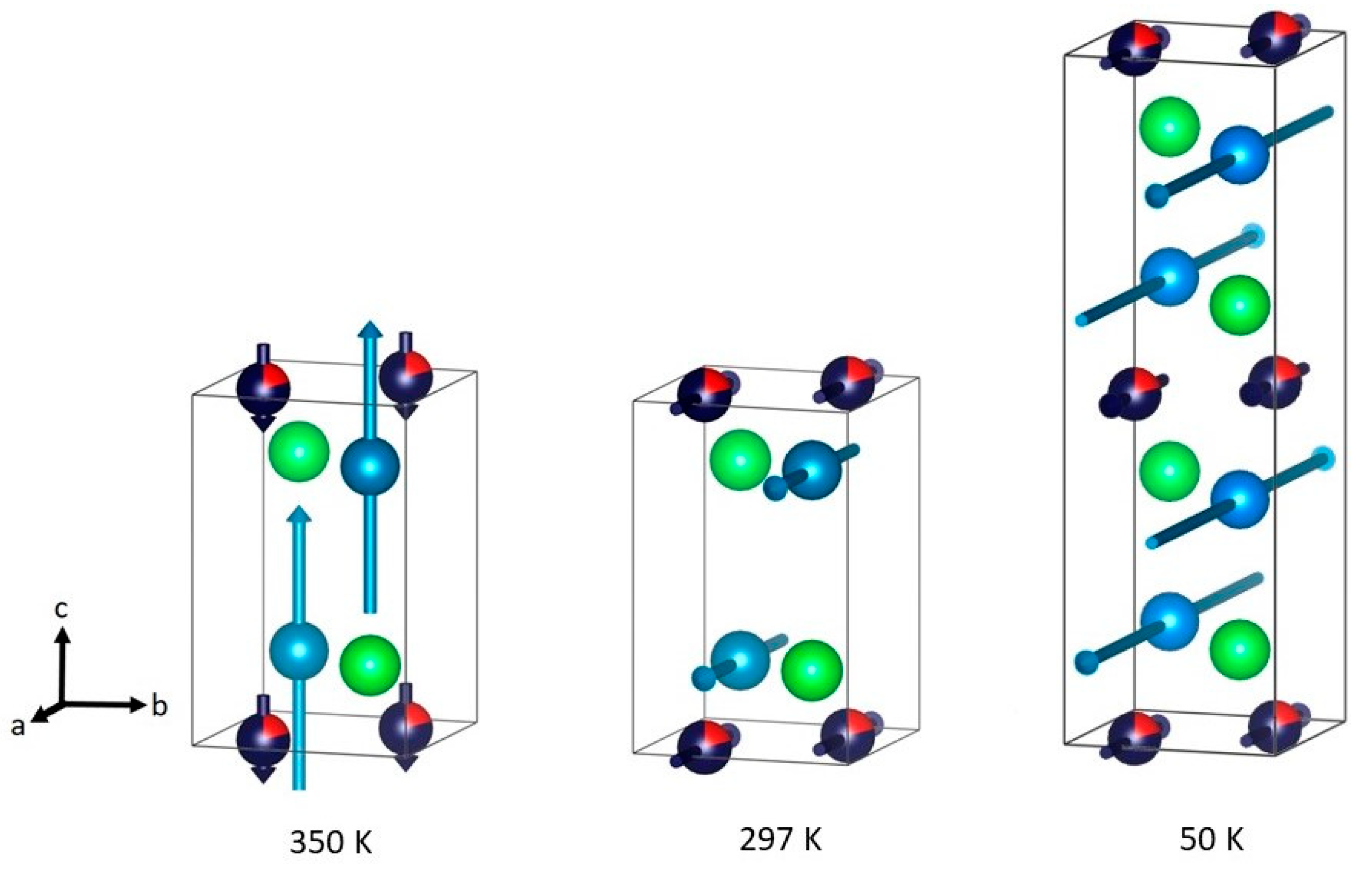
2.3. Neutron Powder Diffraction and Magnetic Structure
3. Materials and Methods
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Suzuki, T.; Kanomata, T.; Yoshida, H.; Kaneko, T. Thermal expansion and pressure effect on magnetic transition of Mn2−xCoxSb. J. Appl. Phys. 1990, 67, 4816–4817. [Google Scholar] [CrossRef]
- Kanomata, T.; Hasebe, Y.; Ito, T.; Yoshida, H.; Kaneko, T. Pressure effect on magnetic transition temperature and magnetic phase diagram of Mn2−xCoxSb. J. Appl. Phys. 1991, 69, 4642–4644. [Google Scholar] [CrossRef]
- Bartashevich, M.I.; Goto, T.; Tomita, T.; Baranov, N.V.; Zemlyanski, S.V.; Hilscher, G.; Michor, H. AF–FRI metamagnetic transition in itinerant Mn2−xCoxSb system: High-field and high-pressure effects. Phys. B Condens. Matter 2002, 318, 198–210. [Google Scholar] [CrossRef]
- Bartashevich, M.I.; Goto, T.; Baranov, N.V.; Gaviko, V.S. Volume magnetostriction at the AF–FRI metamagnetic transition in the itinerant-electron system Mn2−xTxSb (T = Co, Cr). Phys. B Condens. Matter 2004, 351, 71–76. [Google Scholar] [CrossRef]
- Caron, L.; Miao, X.F.; Klaasse, J.P.; Gama, S.; Brück, E. Tuning the giant inverse magnetocaloric effect in Mn2−xCrxSb compounds. Appl. Phys. Lett. 2013, 103, 112404. [Google Scholar] [CrossRef]
- Ma, S.C.; Hou, D.; Gong, Y.Y.; Wang, L.Y.; Huang, Y.L.; Zhong, Z.C.; Wang, D.H.; Du, Y.W. Giant magnetocaloric and magnetoresistance effects in ferrimagnetic Mn1.9Co0.1Sb alloy. Appl. Phys. Lett. 2014, 104, 022410. [Google Scholar] [CrossRef]
- Ma, S.; Su, Y.; Huang, Y.; Wu, Y.; Zhong, Z. Magnetic and Magnetocaloric Properties in Ferrimagnetic Mn2−xCoxSb (x = 0.15 and 0.20) Alloys. IEEE Trans. Magn. 2015, 51, 1–6. [Google Scholar] [CrossRef]
- Tekgül, A.; Acet, M.; Farle, M.; Ünal, N. Kinetic arrest of the ferrimagnetic state in indium-doped Mn1.82Co0.18Sb. J. Alloys Compd. 2017, 695, 418–425. [Google Scholar] [CrossRef]
- Brown, P.J.; Gandy, A.P.; Kanomata, T.; Ziebeck, K.R.A. Stability of Mn moments and exchange interactions in cobalt substituted Mn2Sb. J. Phys. Condens. Matter 2008, 20, 015220. [Google Scholar] [CrossRef]
- Wilkinson, M.K.; Gingrich, N.S.; Shull, C.G. The magnetic structure of Mn2Sb. J. Phys. Chem. Solids 1957, 2, 289–300. [Google Scholar] [CrossRef]
- Kimura, A.; Suga, S.; Matsushita, T.; Daimon, H.; Kaneko, T.; Kanomata, T. Photoemission and Absorption Spectroscopy of Mn2Sb, MnAlGe, Mn2As, Cr2As and Fe2As. J. Phys. Soc. Jpn 1993, 62, 1624–1633. [Google Scholar] [CrossRef]
- Ohashi, M.; Yamaguchi, Y.; Kanomata, T. Neutron diffraction studies of the ferrimagnetic-antiferromagnetic phase transition in cobalt modified Mn2Sb. J. Magn. Magn. Mater. 1992, 104, 925–926. [Google Scholar] [CrossRef]
- Kushwaha, P.; Rawat, R.; Chaddah, P. Metastability in the ferrimagnetic–antiferromagnetic phase transition in Co substituted Mn2Sb. J. Phys. Condens. Matter 2008, 20, 022204. [Google Scholar] [CrossRef]
- Kanomata, T.; Ido, H. Magnetic transitions in Mn2−xMxSb (M = 3 d metals). J. Appl. Phys. 1984, 55, 2039–2041. [Google Scholar] [CrossRef]
- Lawson, A.C.; Larson, A.C.; Aronson, M.C.; Johnson, S.; Fisk, Z.; Canfield, P.C.; Thompson, J.D.; Von Dreele, R.B. Magnetic and crystallographic order in α-manganese. J. Appl. Phys. 1994, 76, 7049–7051. [Google Scholar] [CrossRef]
- Petříček, V.; Fuksa, J.; Dušek, M. Magnetic space and superspace groups, representation analysis: Competing or friendly concepts? Acta Crystallogr. Sect. A 2010, 66, 649–655. [Google Scholar] [CrossRef] [PubMed]
- Petříček, V.; Dušek, M.; Palatinus, L. Crystallographic computing system JANA2006: General features. Z. Kristallogr. Cryst. Mater. 2014, 229, 345–352. [Google Scholar] [CrossRef]
- Gier, H. Kalt-Schmelz-Tiegel. European Patent EP 03,45,542 B1, 1993. [Google Scholar]
- Olesik, J.W. Elemental analysis using ICP-OES and ICP/MS. Anal. Chem. 1991, 63, 12A–21A. [Google Scholar] [CrossRef]
- Dollase, W.A. Correction of intensities for preferred orientation in powder diffractometry: Application of the March model. J. Appl. Crystallogr. 1986, 19, 267–272. [Google Scholar] [CrossRef]

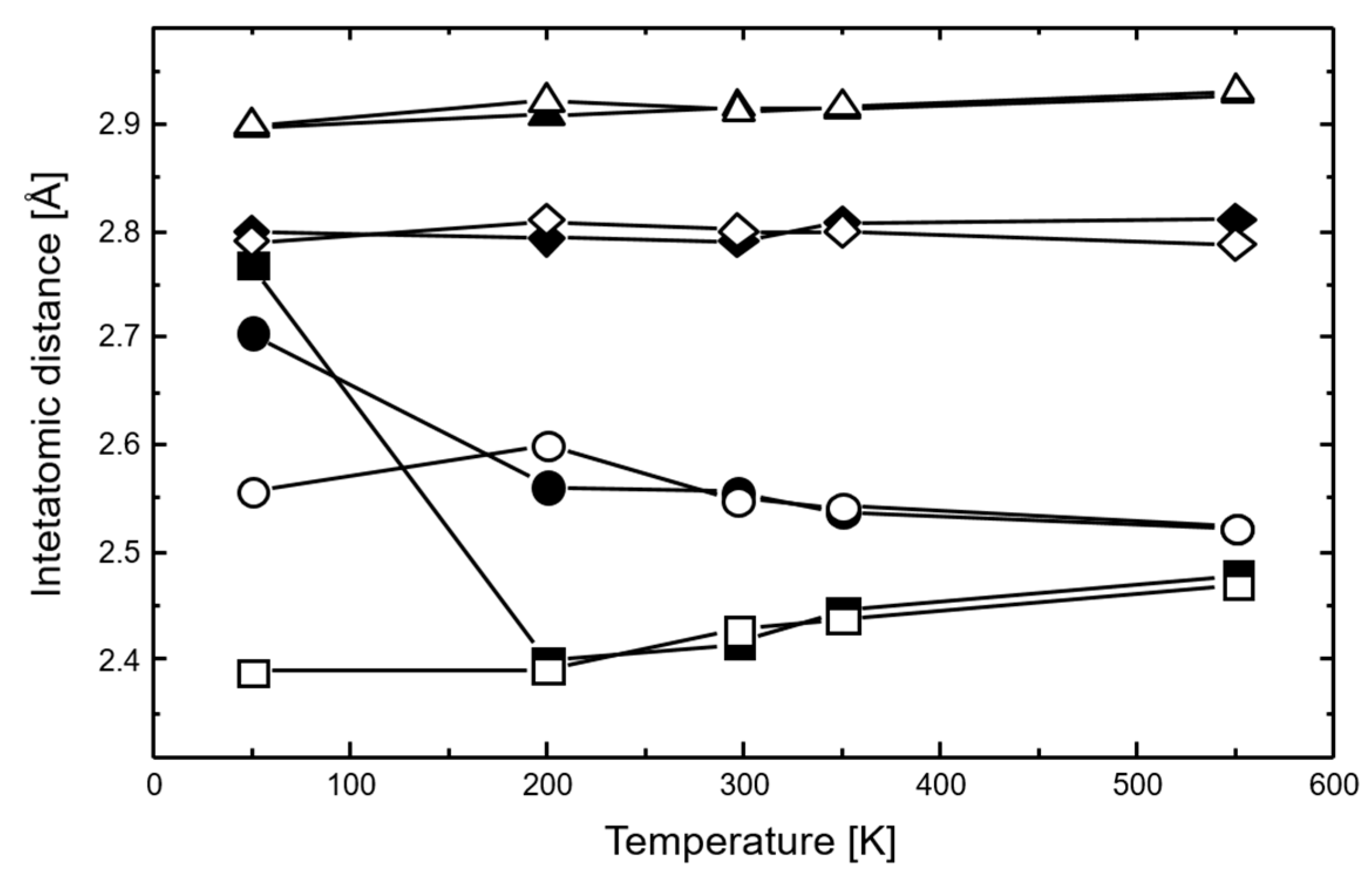
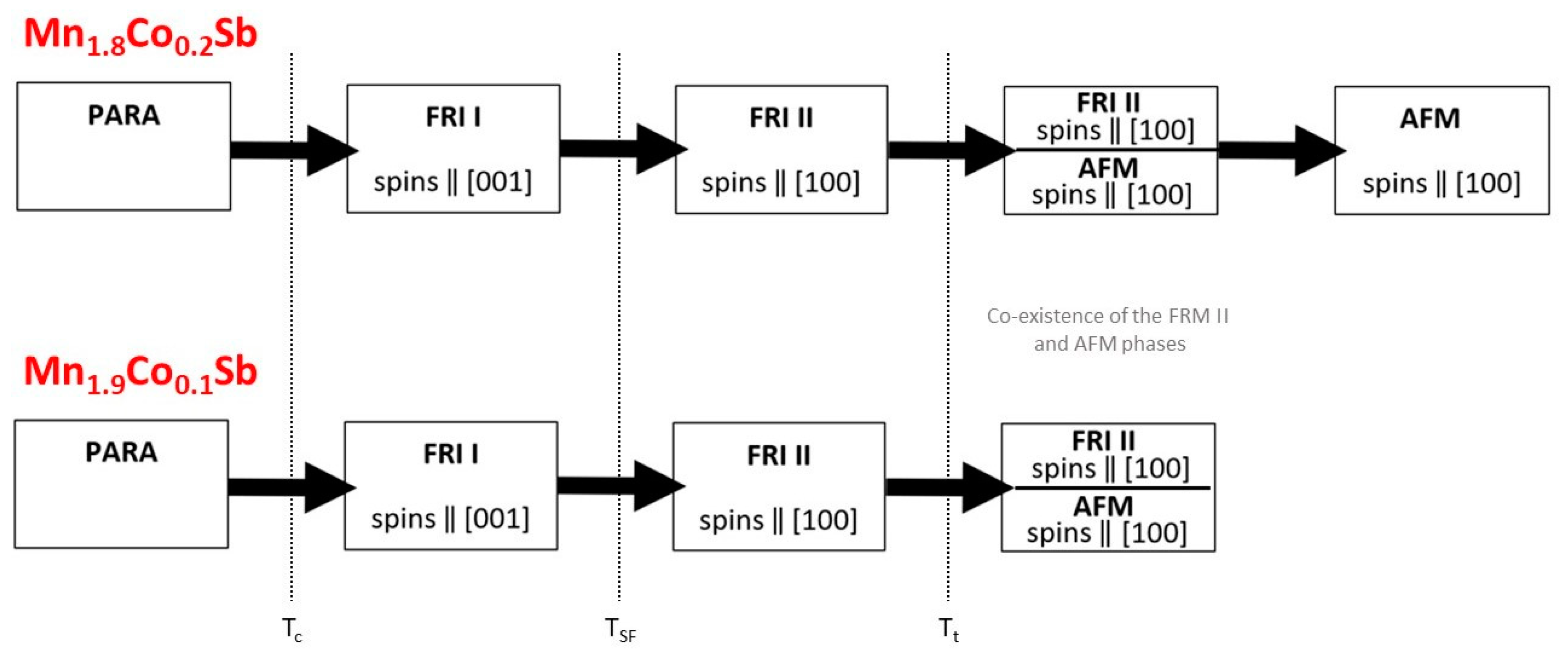
| Transition Temperatures | Cycle | x = 0.1 | x = 0.15 | x = 0.2 |
|---|---|---|---|---|
| TSF | cooling | 275 | 315 | - |
| Tt | 105 | 93 | 133 | |
| TSF | heating | 280 | 316 | 346 |
| Tt | 122 | 116 | 136 | |
| Thys | - | 17 | 23 | 3 |
| 350 K | Robs (%) | Rwp (%) | Rp (%) | |
| P4/nm’m’ | 4.22 | 4.40 | 3.35 | |
| Cm’me’ | 6.41 | 5.37 | 3.80 | |
| Pmm’n’ | 6.08 | 5.37 | 3.80 | |
| RT | Robs (%) | Rwp (%) | Rp (%) | |
| P4/nm’m’ | 8.33 | 6.76 | 4.98 | |
| Cm’me’ | 4.43 | 5.81 | 4.52 | |
| Pmm’n’ | 3.29 | 5.82 | 4.52 | |
| 200 K | Robs (%) Phase I/II | Rwp (%) | Rp (%) | Volume fractions phase I/II |
| C[c]mce | 3.30/3.03 | 5.54 | 4.40 | 0.18(4)/0.82(4) |
| P[c]mcn | 3.00/3.19 | 5.59 | 4.46 | 0.18(4)/0.82(4) |
| R factors from magnetic reflections | Robs (%) | wRobs | Rall (%) | wRall |
| C[c]mce | 12.12 | 12.86 | 33.69 | 14.17 |
| P[c]mcn | 9.31 | 9.12 | 36.26 | 10.53 |
| 50 K | Robs (%) | Rwp (%) | Rp (%) | Robs (%) Magnetic |
| C[c]mce | 4.80 | 6.18 | 4.61 | 5.94 |
| P[c]mcn | 4.56 | 6.18 | 4.61 | 5.35 |
| x | Temperature [K] | Magnetic State | Shubnikov Group | Magnetic Moment µMn I | Magnetic Moment µMn II |
|---|---|---|---|---|---|
| 0.1 | 350 | FRI-I | P4/nm’m’ | along c: −0.76(11) | along c: 2.24(23) |
| 297 | FRI-I | P4/nm’m’ | along c: −0.79(9) | along c: 2.47(18) | |
| 200 | FRI-II | Pmm’n’ | along a: 1.34(15) | along a: −2.01(30) | |
| 50 | AFM + FRI-II | P[c]mcn | along a: −0.53(10) | along a: −0.42(12) | |
| Pmm’n’ | along a: 0.73(13) | along a: −3.05(24) | |||
| 0.2 | 350 | FRI-I | P4/nm’m’ | along c: −0.55(6) | along c: 1.81(11) |
| 297 | FRI-II | Pmm’n’ | along a: −1.10(11) | along a: 1.61(21) | |
| 200 | AFM + FRI-II | P[c]mcn | along a: −0.51(16) | along a: 0.50(25) | |
| Pmm’n’ | along a: 0.63(11) | along a: −2.75(17) | |||
| 50 | AFM | P[c]mcn | along a: −1.12(3) | along a: 3.44(5) |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wilden, J.S.; Hoser, A.; Chikovani, M.; Perßon, J.; Voigt, J.; Friese, K.; Grzechnik, A. Magnetic Transitions in the Co-Modified Mn2Sb System. Inorganics 2018, 6, 113. https://doi.org/10.3390/inorganics6040113
Wilden JS, Hoser A, Chikovani M, Perßon J, Voigt J, Friese K, Grzechnik A. Magnetic Transitions in the Co-Modified Mn2Sb System. Inorganics. 2018; 6(4):113. https://doi.org/10.3390/inorganics6040113
Chicago/Turabian StyleWilden, Johanna S., Andreas Hoser, Mamuka Chikovani, Jörg Perßon, Jörg Voigt, Karen Friese, and Andrzej Grzechnik. 2018. "Magnetic Transitions in the Co-Modified Mn2Sb System" Inorganics 6, no. 4: 113. https://doi.org/10.3390/inorganics6040113
APA StyleWilden, J. S., Hoser, A., Chikovani, M., Perßon, J., Voigt, J., Friese, K., & Grzechnik, A. (2018). Magnetic Transitions in the Co-Modified Mn2Sb System. Inorganics, 6(4), 113. https://doi.org/10.3390/inorganics6040113