Raman Spectroscopy and Microstructural Characterization of Hot-Rolled Copper/Graphene Composite Materials
Abstract
1. Introduction
2. Experimental Section
2.1. Type of Cu Powder and Graphene
2.2. Fabrication of the Composite Material and Post-Treatment
2.2.1. Fabrication of the Composite Materials
2.2.2. HR Post-Treatment
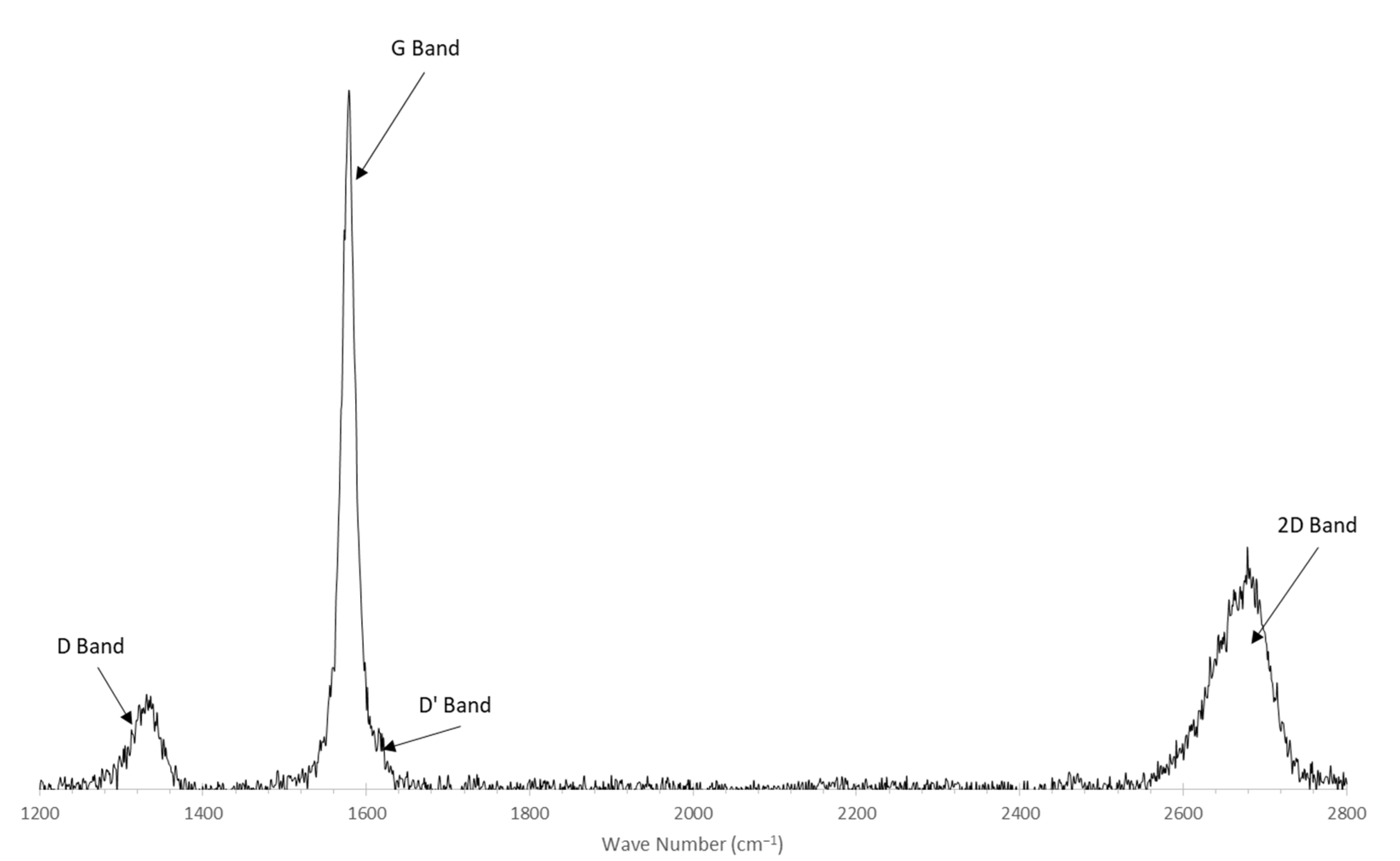
2.2.3. Polarized Raman Spectroscopy
2.3. Hardness and Microstructural Characterization
3. Results and Discussion
3.1. Cu/Gr Composite Materials and HR Post-Treatment
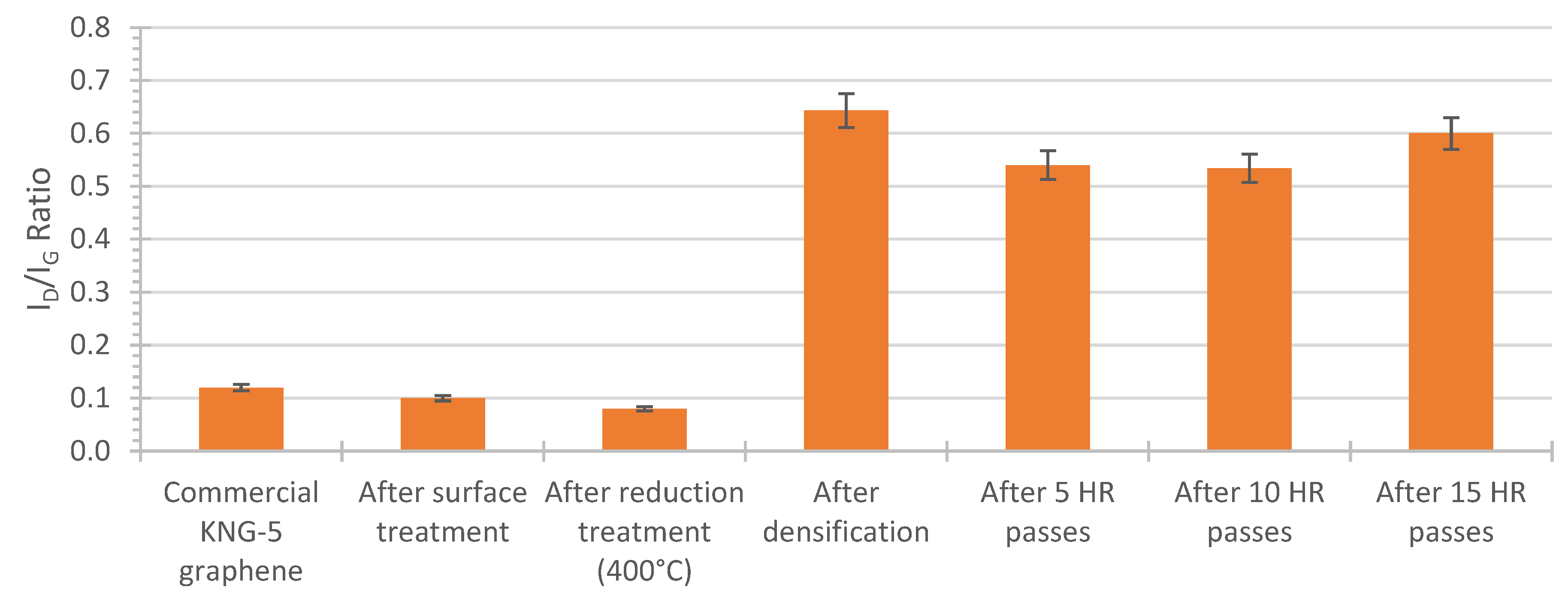
3.2. Polarized Raman Spectroscopy: Effect on HR of Gr Alignment
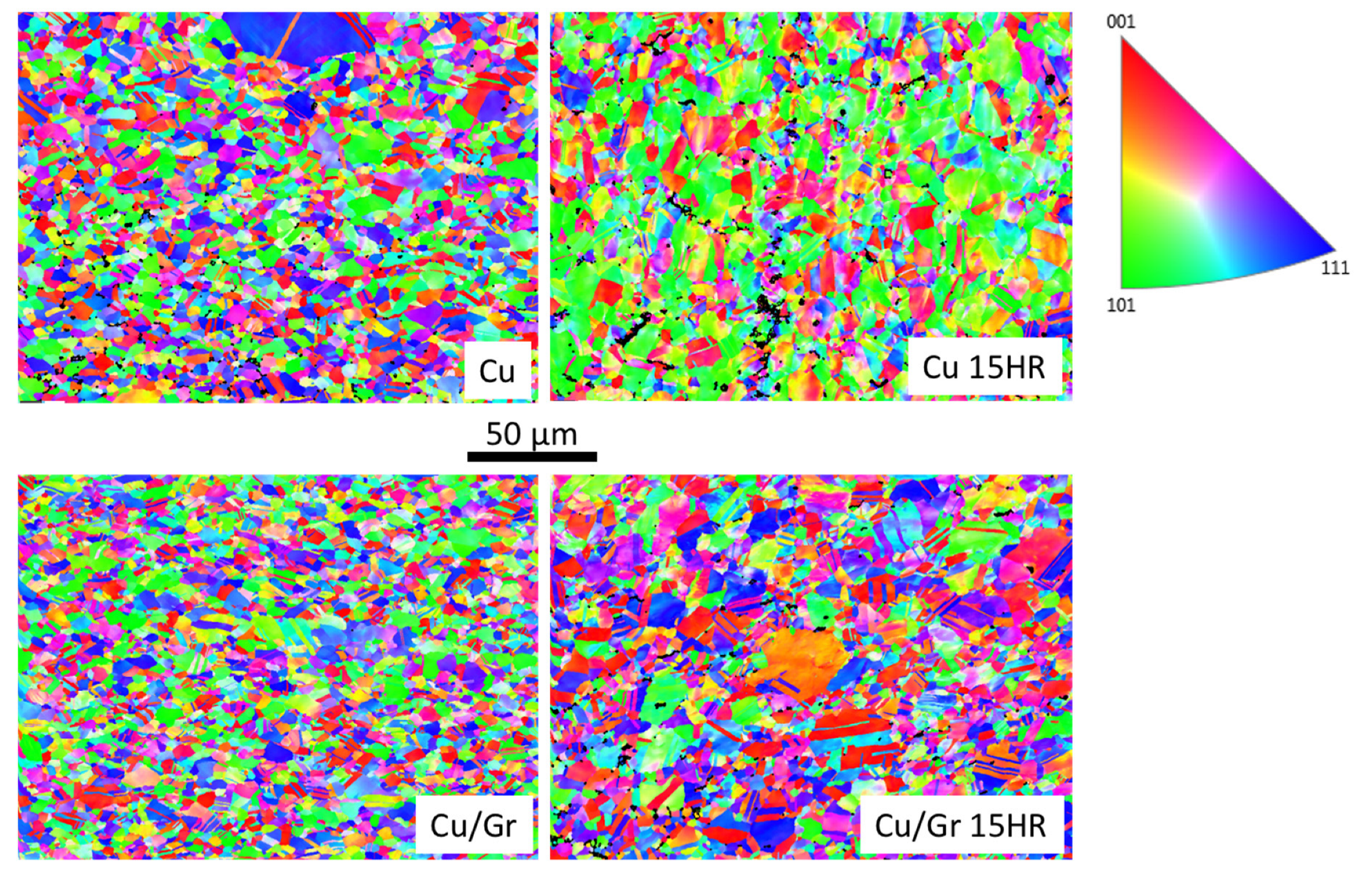
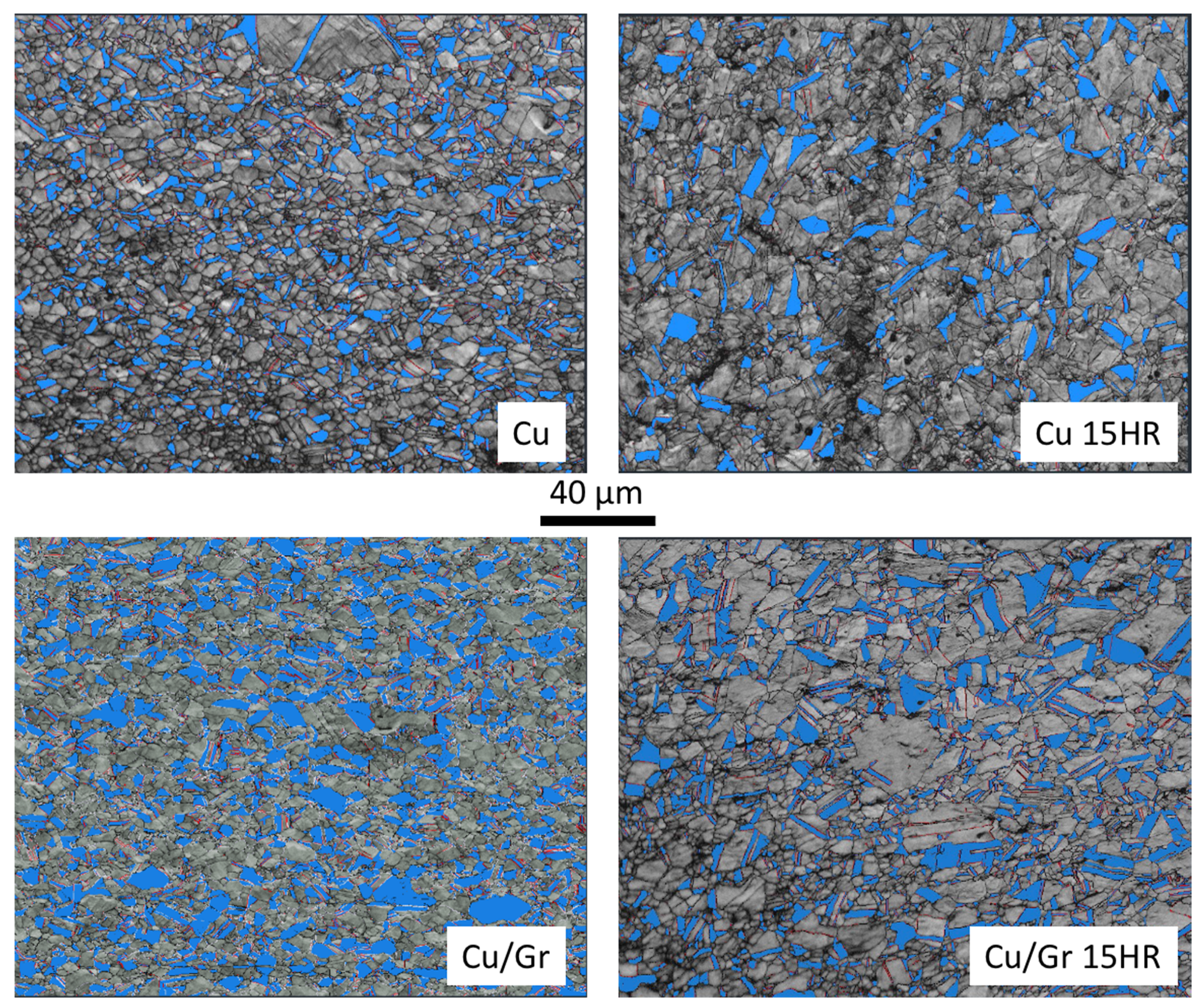
3.3. Microstructural Analysis and Hardness Measurements
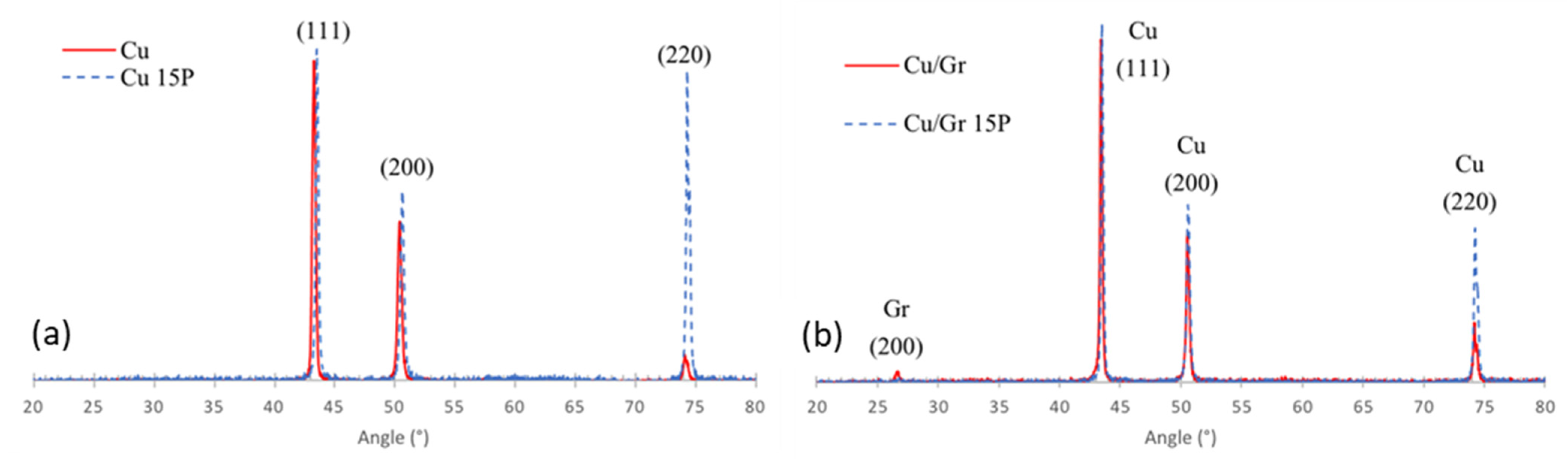
3.3.1. XRD Analysis
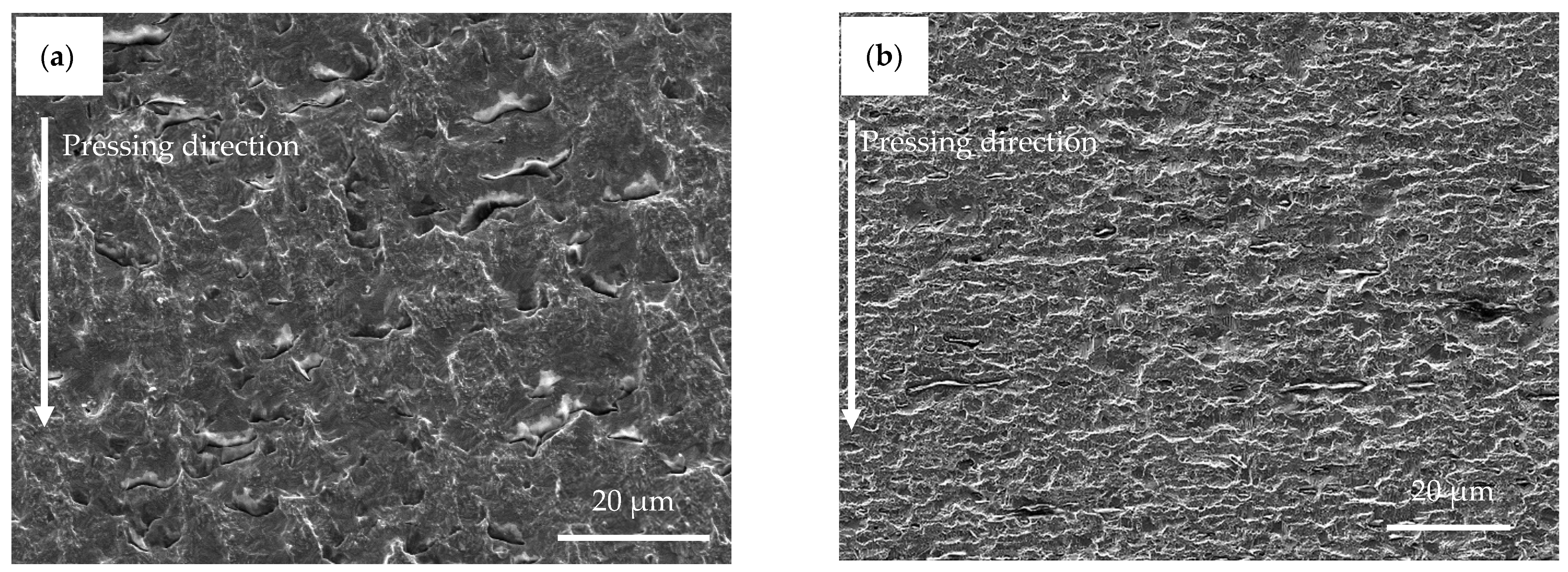
3.3.2. EBSD Analysis
3.3.3. Hardness Measurements
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wallace, P.R. The Band Theory of Graphite. Phys. Rev. 1947, 71, 622. [Google Scholar] [CrossRef]
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Zhang, Y.; Dubonos, S.V.; Grigorieva, I.V.; Firsov, A.A. Electric Field Effect in Atomically Thin Carbon Films. Science 2004, 306, 660–669. [Google Scholar] [CrossRef] [PubMed]
- Geim, A.K.; Novoselov, K.S. The rise of Graphene. Nat. Mater. 2007, 6, 183–191. [Google Scholar] [CrossRef]
- Dean, C.R.; Yong, A.F.; Meric, I.; Wang, L.; Sorgenfrei, S.; Watanabe, K.; Taniguchi, T.; Kim, P.; Shepard, K.L.; Hone, J. Boron nitride substrates for high-quality graphene electronics. Nat. Nanotechnol. 2010, 5, 722–726. [Google Scholar] [CrossRef] [PubMed]
- Hirai, H.; Tsuchiya, H.; Kamakura, Y.; Mori, N.; Ogawa, M. Electron mobility calculation for graphene on substrates. J. Appl. Phys. 2014, 116, 08703. [Google Scholar] [CrossRef]
- Loh, G.C.; Teo EH, T.; Tay, B.K. Thermal transport around tears in graphene. J. Appl. Phys. 2011, 109, 043508. [Google Scholar] [CrossRef]
- Du, X.M.; Zheng, K.F.; Chen, R.Q.; Liu, F.G. First-principle study of the interaction between graphene and metals. Dig. J. Nanomater. Biostructures 2017, 12, 463–471. [Google Scholar]
- Nair, R.R.; Blake, P.; Grigorenko, N.; Novoselov, K.S.; Booth, T.J.; Stauber, T.; Peres NM, R.; Giem, K. Fine Structure Constant Defines Visual Transparency of Graphene. Science 2008, 320, 1308. [Google Scholar] [CrossRef]
- Du, X.; Skachko, I.; Barker, A.; Andrei, E.Y. Approaching ballistic transport in suspended graphene. Nat. Nanotechnol. 2008, 3, 491–495. [Google Scholar] [CrossRef]
- Wu, Q.; Xu, Y.; Yao, Z.; Liu, A.; Shi, G. Supercapacitors based on flexible graphene/polyaniline nanofiber composite films. ACS Nano 2010, 4, 1963–1970. [Google Scholar] [CrossRef]
- Stankovich, S.; Dikin, D.A.; Dommett GH, B.; Kohlhaas, K.M.; Zimney, E.J.; Stach, E.A.; Piner, R.D.; Nguyen, S.T.; Ruoff, R.S. Graphene-based composite materials. Nature 2006, 442, 282–286. [Google Scholar] [CrossRef]
- Nirmalraj, P.N.; Lutz, T.; Kumar, S.; Duesberg, G.S.; Boland, J.J. Nanoscale Mapping of Electrical Resistivity and Connectivity in Graphene Strips and Networks. Nano Lett. 2011, 11, 16–22. [Google Scholar] [CrossRef]
- Wei, C.-K.; Peng, H.-Y.; Tsai, Y.-C.; Chen, T.-C.; Yang, C.-M. Fluorographene sensing membrane in a light-addressable potentiometric sensor. Ceram. Int. 2019, 45, 9074–9081. [Google Scholar] [CrossRef]
- Wang, J.-Z.; Lu, L.; Choucair, M.; Stride, J.A.; Xun, X.; Liu, H.-K. Sulfur-graphene composite for rechargeable lithium batteries. J. Power Sources 2011, 196, 7030–7034. [Google Scholar] [CrossRef]
- Jagannadham, K. Orientation dependence of thermal conductivity in copper-graphene composites. J. Appl. Phys. 2011, 110, 074901. [Google Scholar] [CrossRef]
- Boden, A.; Boerner, B.; Kusch, P.; Firkowska, I.; Reich, S. Nanoplatelet Size to Control the Alignment and Thermal Conductivity in Copper−Graphite Composites. Nano Lett. 2014, 14, 3640–3644. [Google Scholar] [CrossRef]
- Wu, S.; Ladani, R.B.; Zhang, J.; Bafekrpour, E.; Ghorbani, K.; Mouritz, A.P.; Kinloch, A.J.; Wang, C.H. Aligning multilayer graphene flakes with an external electric field to improve multifunctional properties of epoxy nanocomposites. Carbon 2015, 94, 607–618. [Google Scholar] [CrossRef]
- Cao, M.; Xiong, D.-B.; Tan, Z.; Ji, G. Aligning graphene in bulk copper: Nacre-inspired nanolaminated architecture coupled with in-situ processing for enhanced mechanical properties and high electrical conductivity. Carbon 2017, 117, 65–74. [Google Scholar] [CrossRef]
- Embrey, L. Three-Dimensional Graphene Foam Reinforced Epoxy Composites. Master’s Thesis, Florida International University, Miami, FL, USA, 2017. [Google Scholar] [CrossRef]
- Saheb, N.; Qadir, N.U.I.; Siddiqui, M.U.; Arif, A.F.M. Characterization of Nanoreinforcement Dispersion in Inorganic Nanocomposites: A Review. Materials 2014, 7, 4148–4181. [Google Scholar] [CrossRef]
- Li, W.; Liu, Y.; Wu, G. Preparation of graphite flakes/Al with preferred orientation and high thermal conductivity by squeeze casting. Carbon 2015, 95, 945–951. [Google Scholar] [CrossRef]
- Montmitonnet, P. Laminage à chaud-Théorie du laminage, Technique de l’Ingénieur M7840 v1. 1991. Available online: https://www.techniques-ingenieur.fr/base-documentaire/materiaux-th11/elaboration-des-metaux-ferreux-42367210/laminage-a-chaud-m7840/ (accessed on 27 December 2023).
- Wang, M.; Sheng, J.; Wang, L.-D.; Yang, Z.-Y.; Shi, Z.-D.; Wang, X.-J.; Fei, W.-D. Hot rolling behavior of graphene/Cu composites. J. Alloys Compd. 2020, 816, 153204. [Google Scholar] [CrossRef]
- Cassinese, A. Conductive Composites, Wiley Online Library 2011. Available online: https://onlinelibrary.wiley.com/doi/abs/10.1002/9781118097298.weoc054 (accessed on 27 December 2023).
- Lin, J.; Balint, D.; Pietrzyk, M. (Eds.) Microstructure Evolution in Metal Forming Processes; A volume in Woodhead Publishing Series in Metals and Surface Engineering; Woodhead Publishing: Cambridge, UK, 2012. [Google Scholar]
- Li, Z.; Young, R.J.; Kinloch, I.A.; Wilson, N.R.; Marsden, A.J.; Raju, A.P.A. Quantitative determination of the spatial orientation of graphene by polarized Raman spectroscopy. Carbon 2015, 88, 215–224. [Google Scholar] [CrossRef]
- Casiraghi, C.; Hartschuh, A.; Qian, H.; Piscanec, S.; Georgi, C.; Fasoli, A.; Novoselov, K.S.; Basko, D.M.; Ferrari, A.C. Raman Spectroscopy of Graphene Edges. Nano Lett. 2009, 9, 1433–1441. [Google Scholar] [CrossRef] [PubMed]
- Sahoo, S.; Palai, R.; Katiyar, R.S. Polarized Raman scattering in monolayer, bilayer, and suspended bilayer graphene. J. Appl. Phys. 2011, 110, 044320. [Google Scholar] [CrossRef]
- Cong, C.; Yu, T.; Wang, H. Raman Study on the G Mode of Graphene for Determination of Edge Orientation. ACS Nano 2010, 4, 3175–3180. [Google Scholar] [CrossRef] [PubMed]
- Chu, K.; Wang, F.; Huang, D.-J. Anisotropic mechanical properties of graphene/copper composites with aligned graphene. Mater. Sci. Eng. A 2018, 713, 269–277. [Google Scholar] [CrossRef]
- Eckmann, A.; Felten, A.; Mishchenko, A.; Britnell, L.; Krupke, R.; Noveselov, K.S.; Casiraghi, C. Probing the nature in graphene by Raman spectroscopy. Nano Lett. 2012, 12, 3925–3930. [Google Scholar] [CrossRef]
- Cançado, L.G.; Takai, K.; Enoki, T. General equation of the determination of the crystallite size of nanographite by Raman Spectroscopy. Appl. Phys. Lett. 2006, 88, 163106. [Google Scholar] [CrossRef]
- Bident, A. Elaboration de Matériaux Composites Cuivre/Graphene à Propriétés Physiques Améliorées par Métallurgie des Poudres. Ph.D. Thesis, University of Bordeaux, Bordeaux, France, 2022. [Google Scholar]
- Bident, A.; Delange, F.; Labrugere, C.; Debiemme-Chouvy, C.; Lu, Y.; Silvain, J.-F. Fabrication and characterization of copper and copper alloy reinforced by graphene. J. Compos. Mater. 2024, 58, 109–117. [Google Scholar] [CrossRef]
- Silvain, J.-F.; Heintz, J.-M.; Veillere, A.; Constantin, L.; Lu, Y.F. A review of processing of Cu/C base plate composites for interfacial control and improved properties. Int. J. Extrem. Manuf. 2020, 2, 012002. [Google Scholar] [CrossRef]
- Alemour, B.; Yaacob, M.H.; Hassan, M.R. Review of Electrical Properties of Graphene Conductive Composites. Int. J. Nanoelectron. Mater. 2018, 11, 371–398. [Google Scholar]
- Banhart, F.; Kotakoski, J.; Krasheninnikov, A.V. Structural defects in graphene. ACS Nano 2011, 5, 26–41. [Google Scholar] [CrossRef] [PubMed]
- Leffers, T.; Ray, R.K. The brass-type texture and its deviation from the copper-type texture. Prog. Mater. Sci. 2009, 54, 351–396. [Google Scholar] [CrossRef]
- Prasad Reddy, A.; Vamsi Krishna, P.; Narasimha Rao, R.; Murthy, N.V. Silicon carbide reinforced aluminium metal matrix nano composite—A review. Mater. Today Proc. 2017, 4, 3959–3971. [Google Scholar] [CrossRef]
- Carreño-Gallardo, C.; Estrada-Guel, I.; López-Meléndez, C.; Martínez-Sánchez, R. Dispersion of silicon carbide nanoparticles in a AA2024 aluminium alloy by a high-energy ball mill. J. Alloys Comp. 2014, 586, S68–S72. [Google Scholar] [CrossRef]
- Tayeh, T.; Douin, J.; Jouannigot, S.; Zakhour, M.; Nakhl, M.; Silvain, J.-F.; Bobet, J.-L. Hardness and Young’s modulus behavior of Al composites reinforced by nanometric TiB2 elaborated by mechanosynthesis. Mater. Sci. Eng. A 2014, 591, 1–8. [Google Scholar] [CrossRef]
- Orowan, E. Symposium on Internal Stresses in Metals; Institute of Metals: London, UK, 1948; p. 451. [Google Scholar]
- Kumar, N.; Gautam, G.; Gautam, R.K.; Mohan, A.; Mohan, S. Synthesis and characterization of TiB2 reinforced aluminum matrix composites: A review. J. Inst. Eng. India Ser. D 2015, 97, 233–253. [Google Scholar] [CrossRef]
- Gates, J.; Atrens, A.; Smith, I. Microstructure of as-quenched 3.5 NiCrMoV rotor steel; Part I, General structure and retain ausenite. Mater. Werkst 1987, 18, 165–170. [Google Scholar] [CrossRef]



| Number of Passes | 1 | 2 | 3 | 4 | 5 | 10 | 15 |
|---|---|---|---|---|---|---|---|
| Thickness reduction (%) | 10 | 19 | 27 | 34 | 41 | 65 | 79 |
| (111)/(200) | (111)/(220) | |
|---|---|---|
| Cu | 2.1 | 17.0 |
| Cu 15 HR | 1.8 | 1.1 |
| Cu/Gr | 2.3 | 6.0 |
| Cu/Gr 15 HR | 2.1 | 2.3 |
| Cu | Cu 15 | |
|---|---|---|
| Grain size (μm2) | 13 | 9 |
| Surface fraction of twins | 13 | 31 |
| Cu/Gr | Cu/Gr 15 | |
| Grain size (μm2) | 15 | 19 |
| Surface fraction of twins | 15 | 21 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bident, A.; Grosseau-Poussard, J.-L.; Delange, F.; Addad, A.; Ji, G.; Lu, Y.; Bobet, J.-L.; Veillere, A.; Silvain, J.-F. Raman Spectroscopy and Microstructural Characterization of Hot-Rolled Copper/Graphene Composite Materials. Inorganics 2024, 12, 227. https://doi.org/10.3390/inorganics12080227
Bident A, Grosseau-Poussard J-L, Delange F, Addad A, Ji G, Lu Y, Bobet J-L, Veillere A, Silvain J-F. Raman Spectroscopy and Microstructural Characterization of Hot-Rolled Copper/Graphene Composite Materials. Inorganics. 2024; 12(8):227. https://doi.org/10.3390/inorganics12080227
Chicago/Turabian StyleBident, Antoine, Jean-Luc Grosseau-Poussard, Florence Delange, Ahmed Addad, Gang Ji, Yongfeng Lu, Jean-Louis Bobet, Amélie Veillere, and Jean-François Silvain. 2024. "Raman Spectroscopy and Microstructural Characterization of Hot-Rolled Copper/Graphene Composite Materials" Inorganics 12, no. 8: 227. https://doi.org/10.3390/inorganics12080227
APA StyleBident, A., Grosseau-Poussard, J.-L., Delange, F., Addad, A., Ji, G., Lu, Y., Bobet, J.-L., Veillere, A., & Silvain, J.-F. (2024). Raman Spectroscopy and Microstructural Characterization of Hot-Rolled Copper/Graphene Composite Materials. Inorganics, 12(8), 227. https://doi.org/10.3390/inorganics12080227







