First-Principles Study of Strain Effects on the Perpendicular Magnetic Anisotropy of Fe/MgO Heterostructures
Abstract
1. Introduction
2. Results and Discussion
2.1. Structural Models
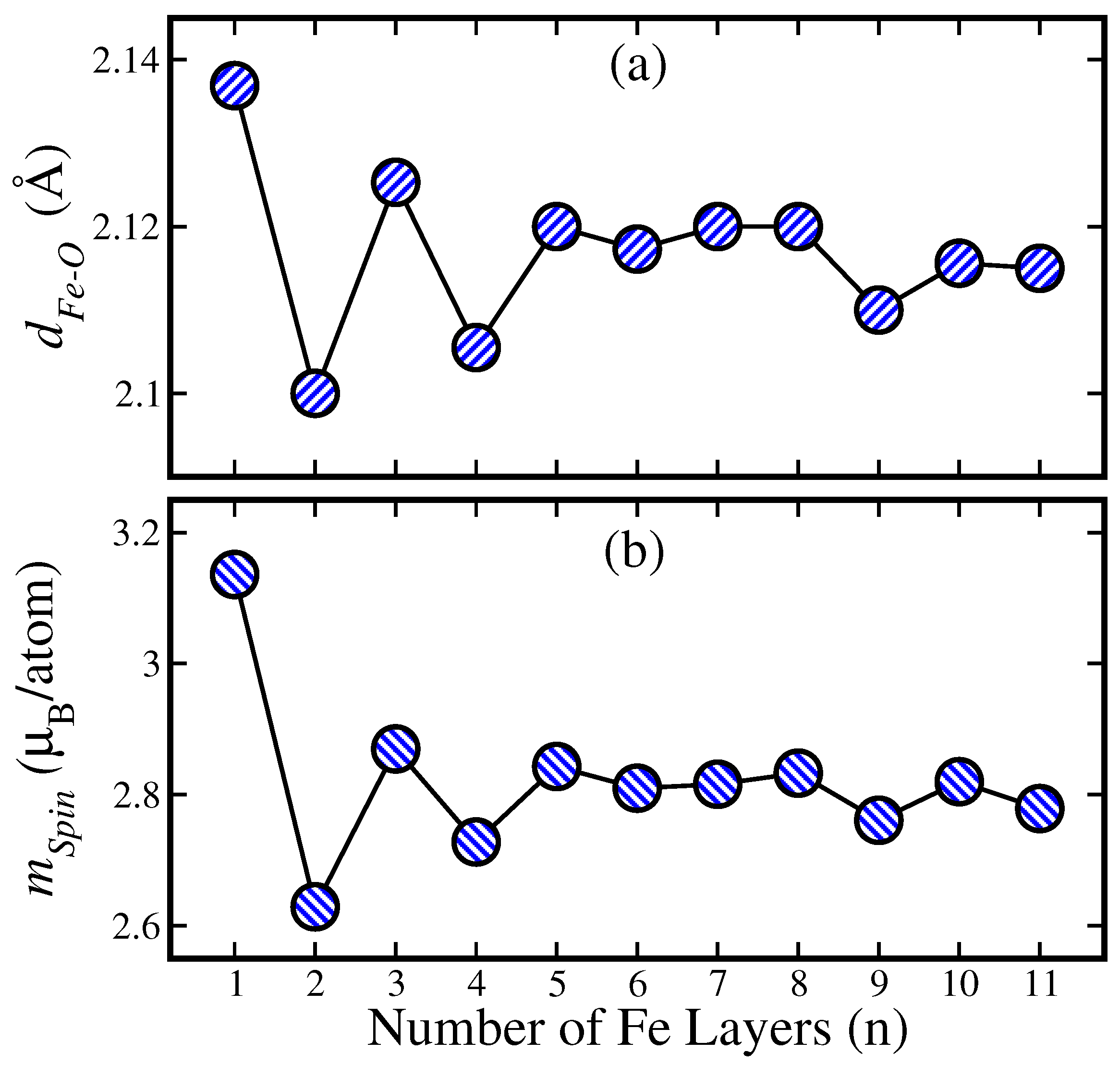
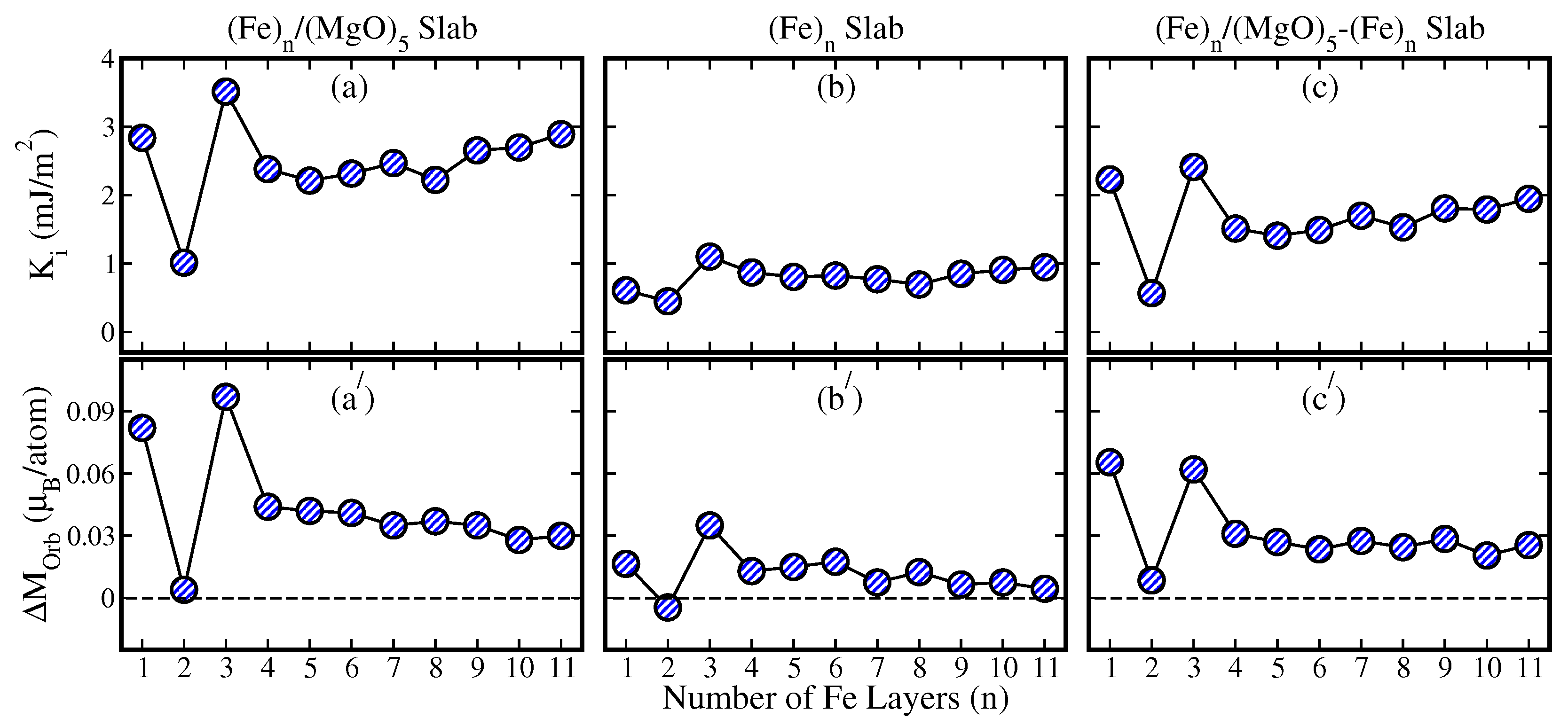
2.2. Unstrained Fe/MgO Interface
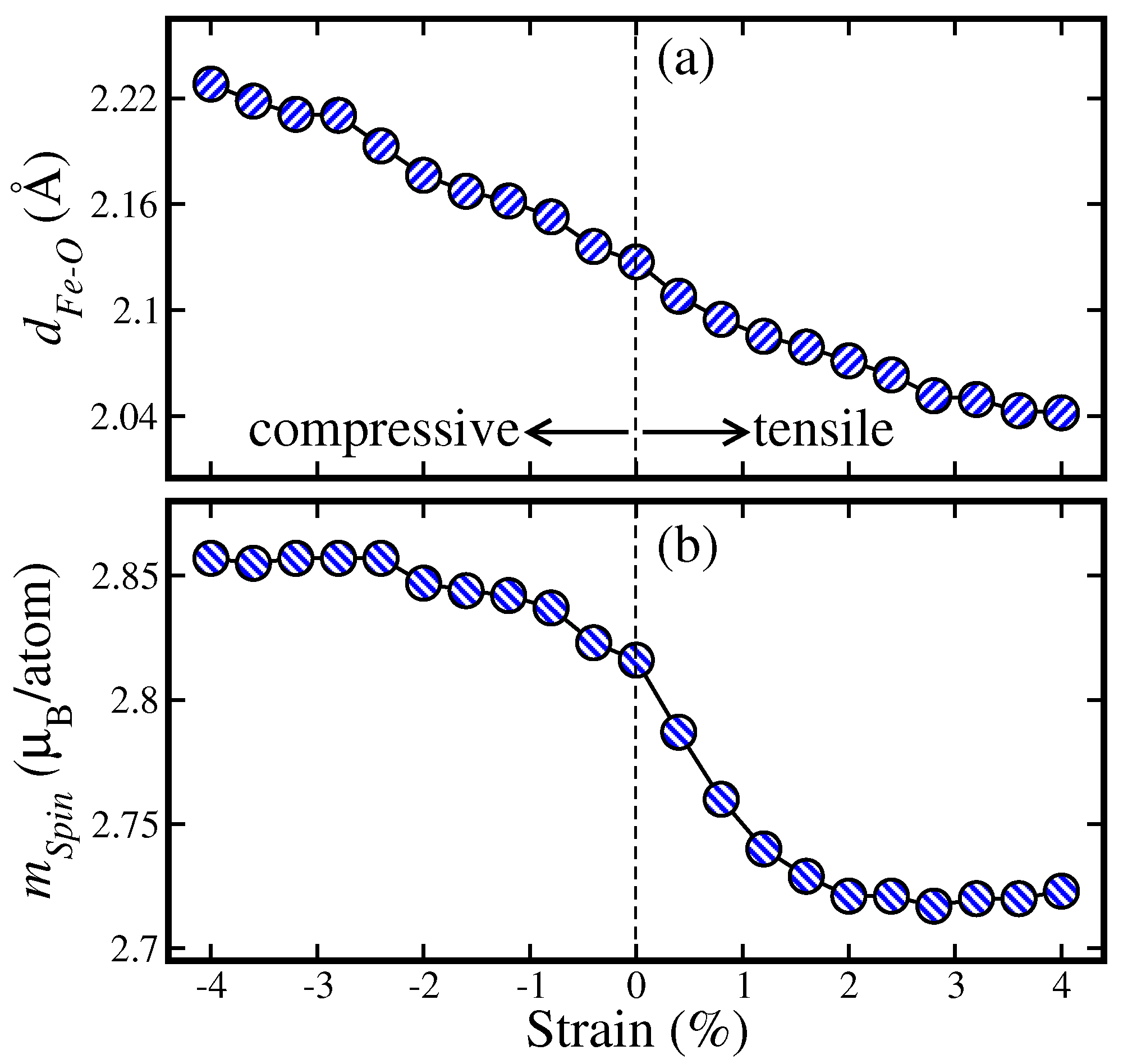
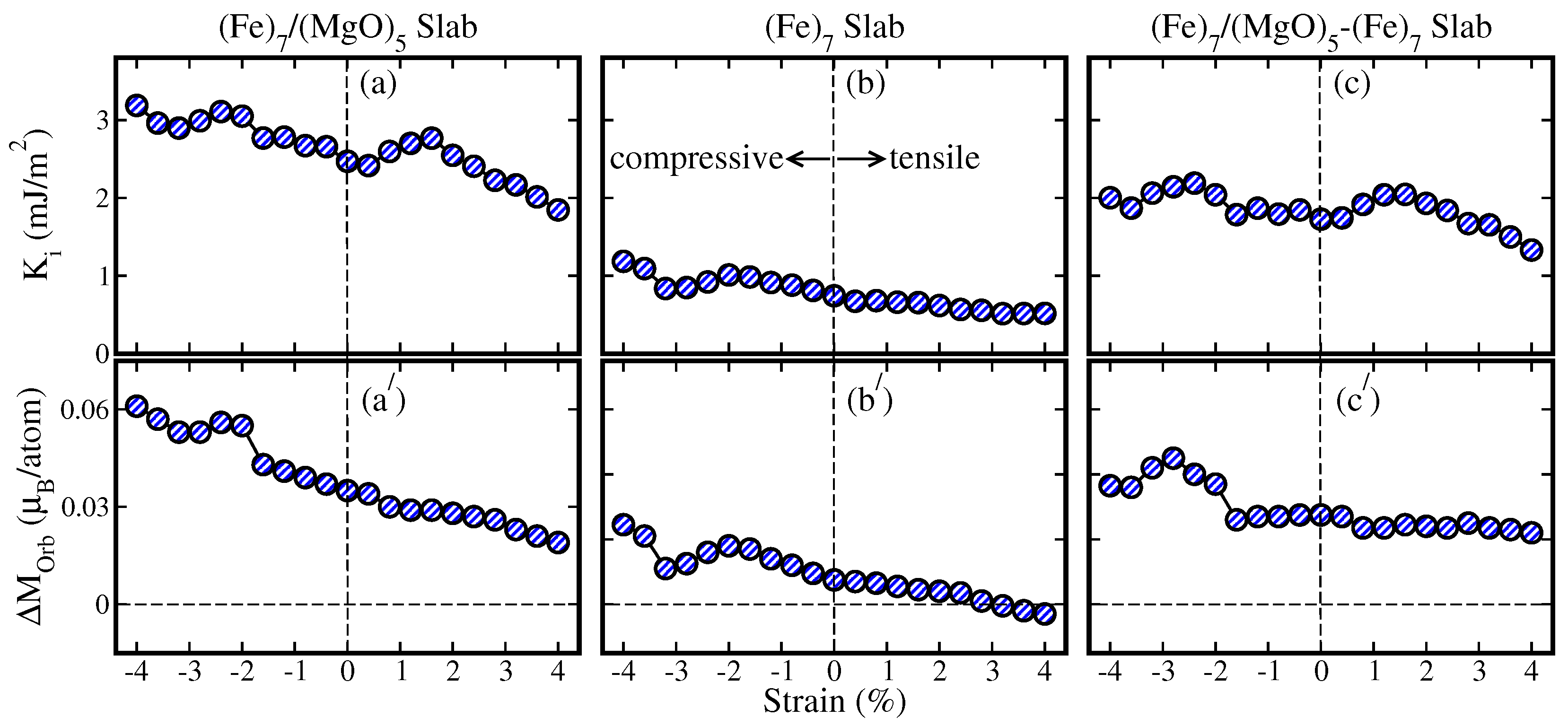
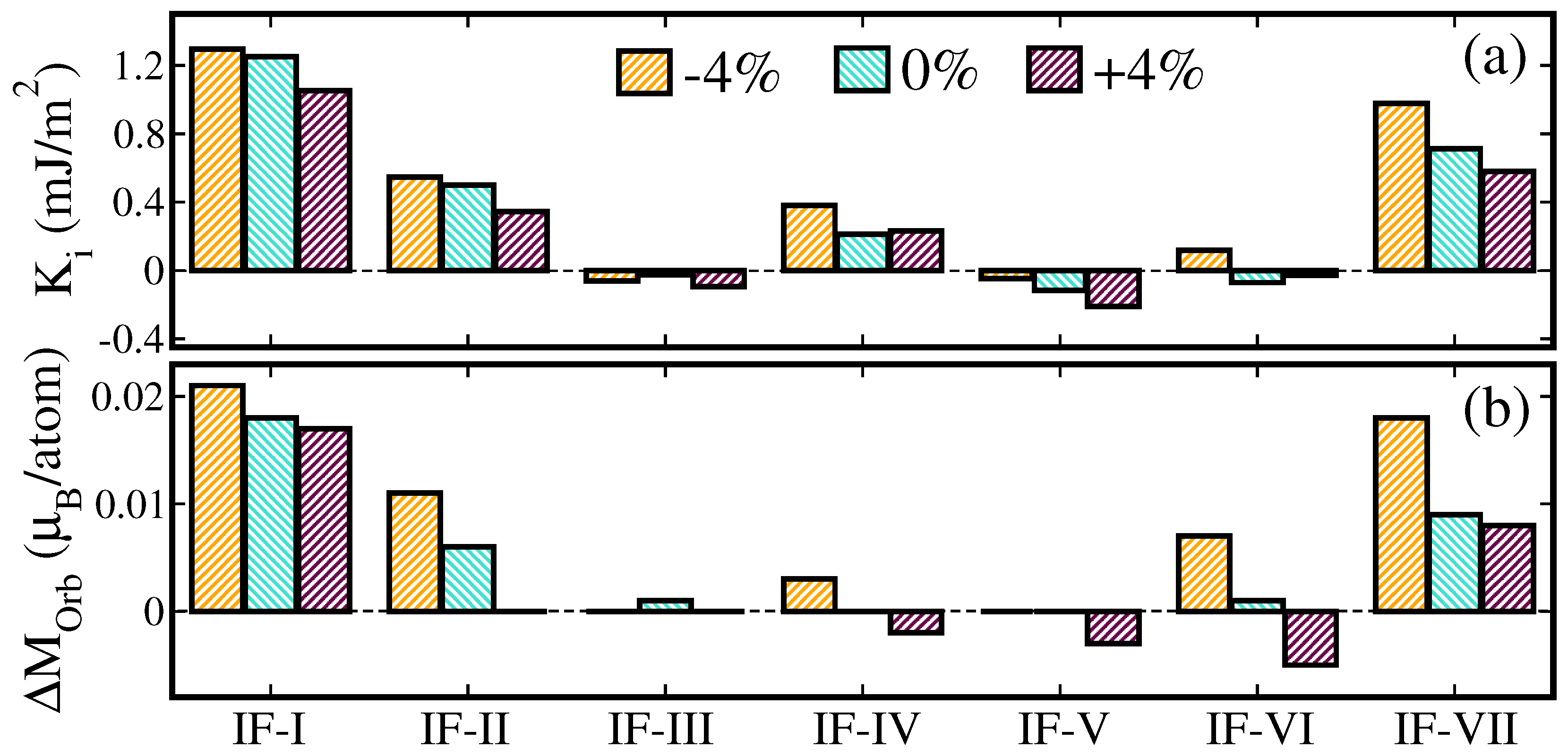
2.3. Strained Fe/MgO Interface
3. Materials and Methods
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| PMA | Perpendicular magnetic anisotropy |
| MTJ | Magnetic tunnel junction |
| VASP | Vienna Ab Initio Simulation Package |
| PAW | Projector augmented wave |
| GGA | Generalized gradient approximation |
| PBE | Perdew–Burke–Ernzerhof |
References
- Nakayama, M.; Kai, T.; Shimomura, N.; Amano, M.; Kitagawa, E.; Nagase, T.; Yoshikawa, M.; Kishi, T.; Ikegawa, S.; Yoda, H. Spin Transfer Switching in TbCoFe/CoFeB/MgO/CoFeB/TbCoFe Magnetic Tunnel Junctions with Perpendicular Magnetic Anisotropy. J. Appl. Phys. 2008, 103, 07A710. [Google Scholar] [CrossRef]
- Ikeda, S.; Miura, K.; Yamamoto, H.; Mizunuma, K.; Gan, H.D.; Endo, M.; Kanai, S.; Hayakawa, J.; Matsukura, F.; Ohno, H. A Perpendicular-Anisotropy CoFeB-MgO Magnetic Tunnel Junction. Nat. Mater. 2010, 9, 721–724. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Yang, K. Engineering Mn3Ga/GaAs Interfaces: A First-Principles Study on Energetic Stability and Magnetic Anisotropy. J. Phys. D Appl. Phys. 2024, 57, 255006. [Google Scholar] [CrossRef]
- Jiang, S.; Yang, K. High-Throughput Design of Perpendicular Magnetic Anisotropy at Quaternary Heusler and MgO Interfaces. Npj Comput. Mater. 2023, 9, 123. [Google Scholar] [CrossRef]
- Jiang, S.; Nazir, S.; Yang, K. High-Throughput Design of Interfacial Perpendicular Magnetic Anisotropy at Heusler/MgO Heterostructures. ACS Appl. Mater. Interfaces 2022, 14, 9734–9743. [Google Scholar] [CrossRef] [PubMed]
- Jiang, S.; Nazir, S.; Yang, K. Origin of the Large Interfacial Perpendicular Magnetic Anisotropy in MgO/Co2FeAl. Phys. Rev. B 2020, 101, 134405. [Google Scholar] [CrossRef]
- Nazir, S.; Jiang, S.; Cheng, J.; Yang, K. Enhanced Interfacial Perpendicular Magnetic Anisotropy in Fe/MgO Heterostructure Via Interfacial Engineering. Appl. Phys. Lett. 2019, 114, 072407. [Google Scholar] [CrossRef]
- Dieny, B.; Chshiev, M. Perpendicular Magnetic Anisotropy at Transition Metal/Oxide Interfaces and Applications. Rev. Mod. Phys. 2017, 89, 025008. [Google Scholar] [CrossRef]
- Tsunegi, S.; Kubota, H.; Tamaru, S.; Yakushiji, K.; Konoto, M.; Fukushima, A.; Taniguchi, T.; Arai, H.; Imamura, H.; Yuasa, S. Damping Parameter and Interfacial Perpendicular Magnetic Anisotropy of FeB Nanopillar Sandwiched Between MgO Barrier and Cap Layers in Magnetic Tunnel Junctions. Appl. Phys. Express 2014, 7, 033004. [Google Scholar] [CrossRef]
- Xiang, Q.; Mandal, R.; Sukegawa, H.; Takahashi, Y.K.; Mitani, S. Large Perpendicular Magnetic Anisotropy in Epitaxial Fe/MgAl2O4 (001) Heterostructures. Appl. Phys. Express 2018, 11, 063008. [Google Scholar] [CrossRef]
- Kent, A.D. Perpendicular All the Way. Nat. Mater. 2010, 9, 699. [Google Scholar] [CrossRef] [PubMed]
- Okabayashi, J.; Koo, J.W.; Sukegawa, H.; Mitani, S.; Takagi, Y.; Yokoyama, T. Perpendicular Magnetic Anisotropy at the Interface Between Ultrathin Fe Film and MgO Studied by Angular-Dependent X-Ray Magnetic Circular Dichroism. Appl. Phys. Lett. 2014, 105, 122408. [Google Scholar] [CrossRef]
- Yang, H.X.; Chshiev, M.; Dieny, B.; Lee, J.H.; Manchon, A.; Shin, K.H. First-Principles Investigation of the Very Large Perpendicular Magnetic Anisotropy at Fe/MgO and Co/MgO Interfaces. Phys. Rev. B 2011, 84, 054401. [Google Scholar] [CrossRef]
- Hallal, A.; Yang, H.X.; Dieny, B.; Chshiev, M. Anatomy of Perpendicular Magnetic Anisotropy in Fe/MgO Magnetic Tunnel Junctions: First-Principles Insight. Phys. Rev. B 2013, 88, 184423. [Google Scholar] [CrossRef]
- Lambert, C.H.; Rajanikanth, A.; Hauet, T.; Mangin, S.; Fullerton, E.E.; Andrieu, S. Quantifying Perpendicular Magnetic Anisotropy at the Fe-MgO(001) Interface. Appl. Phys. Lett. 2013, 102, 122410. [Google Scholar] [CrossRef]
- Kozioł-Rachwat, A.; Skowronski, W.; Slezak, T.; Wilgocka-Slezak, D.; Przewoznik, J.; Stobiecki, T.; Qin, Q.H.; van Dijken, S.; Korecki, J. Room-Temperature Perpendicular Magnetic Anisotropy of MgO/Fe/MgO Ultrathin Films. J. Appl. Phys. 2013, 114, 224307. [Google Scholar] [CrossRef]
- Koo, J.W.; Mitani, S.; Sasaki, T.T.; Sukegawa, H.; Wen, Z.C.; Ohkubo, T.; Niizeki, T.; Inomata, K.; Hono, K. Large Perpendicular Magnetic Anisotropy at Fe/MgO Interface. Appl. Phys. Lett. 2013, 103, 192401. [Google Scholar] [CrossRef]
- Nazir, S.; Yang, K. Elucidate Interfacial Disorder Effects on the Perpendicular Magnetic Anisotropy at Fe/MgO Heterostructure from First-Principles Calculations. J. Phys. Condens. Matter 2022, 34, 214009. [Google Scholar] [CrossRef]
- Hu, Y.; Yan, S.; Jin, D.; Qiao, W.; Bai, R.; Zhou, T. Giant and Strain-Tunable Interfacial Magnetic Anisotropy in MgO-Based Magnetic Heterostructures with Heavy Atoms Insertion. Phys. Scr. 2022, 98, 015022. [Google Scholar] [CrossRef]
- Niranjan, M.K.; Duan, C.G.; Jaswal, S.S.; Tsymbal, E.Y. Electric Field Effect on Magnetization at the Fe/MgO(001) Interface. Appl. Phys. Lett. 2010, 96, 222504. [Google Scholar] [CrossRef]
- Nakamura, K.; Akiyama, T.; Ito, T.; Weinert, M.; Freeman, A.J. Role of an Interfacial FeO Layer in the Electric-Field-Driven Switching of Magnetocrystalline Anisotropy at the Fe/MgO Interface. Phys. Rev. B 2010, 81, 220409. [Google Scholar] [CrossRef]
- Li, Z.; Zhang, Y.; Wen, H.; Dong, C. Strain and Ferroelectric Polarization Influence on Perpendicular Magnetic Anisotropy of CoFe3N/BaTiO3 Heterostructure. Results Phys. 2024, 57, 107388. [Google Scholar] [CrossRef]
- Rodewald, J.; Thien, J.; Ruwisch, K.; Pohlmann, T.; Hoppe, M.; Schmalhorst, J.; Küpper, K.; Wollschläger, J. Structure-Related Electronic and Magnetic Properties in Ultrathin Epitaxial NixFe3−xO4 Films on MgO(001). Nanomaterials 2024, 14, 694. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Behtash, M.; Wong, J.; Yang, K. Enhancing Ferroelectric Dipole Ordering in Organic–Inorganic Hybrid Perovskite CH3NH3PbI3: Strain and Doping Engineering. J. Phys. Chem. C 2018, 122, 177–184. [Google Scholar] [CrossRef]
- Chen, Y.; Lei, Y.; Li, Y.; Yu, Y.; Cai, J.; Chiu, M.H.; Rao, R.; Gu, Y.; Wang, C.; Choi, W.; et al. Strain Engineering and Epitaxial Stabilization of Halide Perovskites. Nature 2020, 577, 209. [Google Scholar] [CrossRef] [PubMed]
- Nazir, S.; Behtash, M.; Yang, K. Enhancing Interfacial Conductivity and Spatial Charge Confinement of LaAlO3/SrTiO3 Heterostructures Via Strain Engineering. Appl. Phys. Lett. 2014, 105, 141602. [Google Scholar] [CrossRef]
- Nazir, S.; Behtash, M.; Yang, K. The Role of Uniaxial Strain in Tailoring the Interfacial Properties of LaAlO3/SrTiO3(001) Heterostructure. RSC Adv. 2015, 5, 15682–15689. [Google Scholar] [CrossRef]
- Nazir, S.; Yang, K. First-Principles Characterization of the Critical Thickness for Forming Metallic States in Strained LaAlO3/SrTiO3(001) Heterostructure. ACS Appl. Mater. Interfaces 2014, 6, 22351–22358. [Google Scholar] [CrossRef]
- Ong, P.V.; Kioussis, N.; Amiri, P.K.; Wang, K.L.; Carman, G.P. Strain Control Magnetocrystalline Anisotropy of Ta/FeCo/MgO Heterostructures. J. Appl. Phys. 2015, 117, 17B518. [Google Scholar] [CrossRef]
- Huang, W.; Zhu, J.; Zeng, H.; Wei, X.; Zhang, Y.; Li, Y. Strain Induced Magnetic Anisotropy in Highly Epitaxial CoFe2O4 Thin Films. Appl. Phys. Lett. 2006, 89, 262506. [Google Scholar] [CrossRef]
- Magnifouet, G.; Vallet, M.; Meslin, E.; Walls, M.; Bouillet, C.; Arabski, J.; Pierron-Bohnes, V. Strains in Fe/Cr/Fe Trilayers and (Fe/Cr)5/Fe Multilayers Epitaxied on MgO and MgO/SrTiO3. Thin Solid Films 2023, 780, 139949. [Google Scholar] [CrossRef]
- Kim, K.h.; Kim, H.j.; Kim, G.H.; Chang, J.; Han, S.h. Strain-Induced Microstructural Evolution in Epitaxial Fe/MgO Layers Grown on InxGa1−xAs(001) Substrates. Appl. Phys. Lett. 2009, 95, 164103. [Google Scholar] [CrossRef]
- Park, J.; Yu, B.D. Epitaxial Strain and Interfacial Electronic Topological Transition in O-rich MgO/FeO/Fe(001) Interfaces. Phys. Rev. B 2011, 83, 144431. [Google Scholar] [CrossRef]
- Han, X.; Cui, H.; Liu, B.; Tian, C.; Wang, J.; Chen, H.; Yuan, H. Effects of Overlayer Capping and Lattice Strain on Perpendicular Magnetic Anisotropy of TM/FePt/MgO Heterostructures. Sci. Rep. 2018, 8, 9429. [Google Scholar] [CrossRef] [PubMed]
- Shiga, M.; Sakamoto, S.; Tsujikawa, T.; Ando, R.; Amemiya, K.; Miwa, S. Influence of Epitaxial Strain on the Perpendicular Magnetic Anisotropy of Fe/MgO Systems. Phys. Rev. B 2021, 104, L140406. [Google Scholar] [CrossRef]
- Subagyo, A.; Oka, H.; Eilers, G.; Sueoka, K.; Mukasa, K. Scanning Tunneling Microscopy Observation of Epitaxial Bcc-Fe(001) Surface. Jpn. J. Appl. Phys. 2000, 39, 3777. [Google Scholar] [CrossRef]
- Dugerjav, O.; Kim, H.; Seo, J.M. Growth of a Crystalline and Ultrathin MgO Film on Fe(001). AIP Adv. 2011, 1, 032156. [Google Scholar] [CrossRef]
- Baumann, S.; Donati, F.; Stepanow, S.; Rusponi, S.; Paul, W.; Gangopadhyay, S.; Rau, I.G.; Pacchioni, G.E.; Gragnaniello, L.; Pivetta, M.; et al. Origin of Perpendicular Magnetic Anisotropy and Large Orbital Moment in Fe Atoms on MgO. Phys. Rev. Lett. 2015, 115, 237202. [Google Scholar] [CrossRef] [PubMed]
- Yang, K.; Wu, R.; Shen, L.; Feng, Y.P.; Dai, Y.; Huang, B. Origin of d0 Magnetism in II-VI and III-V Semiconductors by Substitutional Doping at Anion Site. Phys. Rev. B 2010, 81, 125211. [Google Scholar] [CrossRef]
- Bruno, P. Tight-Binding Approach to the Orbital Magnetic Moment and Magnetocrystalline Anisotropy of Transition-Metal Monolayers. Phys. Rev. B 1989, 39, 865–868. [Google Scholar] [CrossRef]
- Masuda, K.; Kasai, S.; Miura, Y.; Hono, K. Giant Interfacial Perpendicular Magnetic Anisotropy in Fe/CuIn1−xGaxSe2 Beyond Fe/MgO. Phys. Rev. B 2017, 96, 174401. [Google Scholar] [CrossRef]
- Odkhuu, D. Giant Strain Control of Magnetoelectric Effect in Ta/Fe/MgO. Sci. Rep. 2016, 6, 32742–32750. [Google Scholar] [CrossRef] [PubMed]
- Kresse, G.; Furthmuller, J. Efficiency of Ab-Initio Total Energy Calculations for Metals and Semiconductors Using a Plane-Wave Basis Set. Comput. Mater. Sci. 1996, 6, 15–50. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmuller, J. Efficient Iterative Schemes for Ab-Initio Total-Energy Calculations Using a Plane-Wave Basis Set. Phys. Rev. B 1996, 54, 11169–11186. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nazir, S.; Jiang, S.; Yang, K. First-Principles Study of Strain Effects on the Perpendicular Magnetic Anisotropy of Fe/MgO Heterostructures. Inorganics 2024, 12, 211. https://doi.org/10.3390/inorganics12080211
Nazir S, Jiang S, Yang K. First-Principles Study of Strain Effects on the Perpendicular Magnetic Anisotropy of Fe/MgO Heterostructures. Inorganics. 2024; 12(8):211. https://doi.org/10.3390/inorganics12080211
Chicago/Turabian StyleNazir, Safdar, Sicong Jiang, and Kesong Yang. 2024. "First-Principles Study of Strain Effects on the Perpendicular Magnetic Anisotropy of Fe/MgO Heterostructures" Inorganics 12, no. 8: 211. https://doi.org/10.3390/inorganics12080211
APA StyleNazir, S., Jiang, S., & Yang, K. (2024). First-Principles Study of Strain Effects on the Perpendicular Magnetic Anisotropy of Fe/MgO Heterostructures. Inorganics, 12(8), 211. https://doi.org/10.3390/inorganics12080211





