Heavy-Fermion Properties of Yb2Pd2SnH≈2
Abstract
:1. Introduction
2. Results
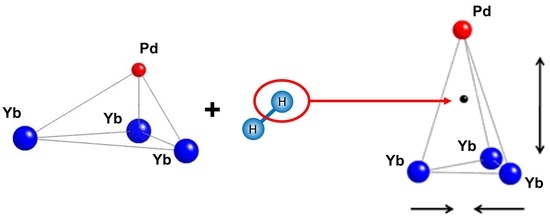
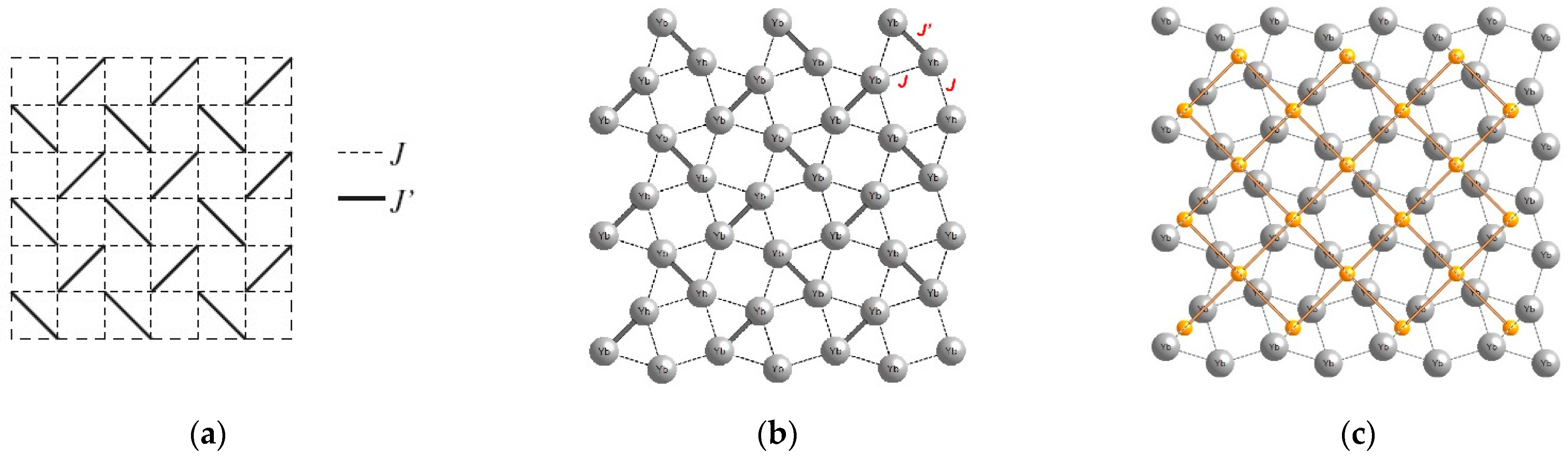
2.1. Crystal Structure
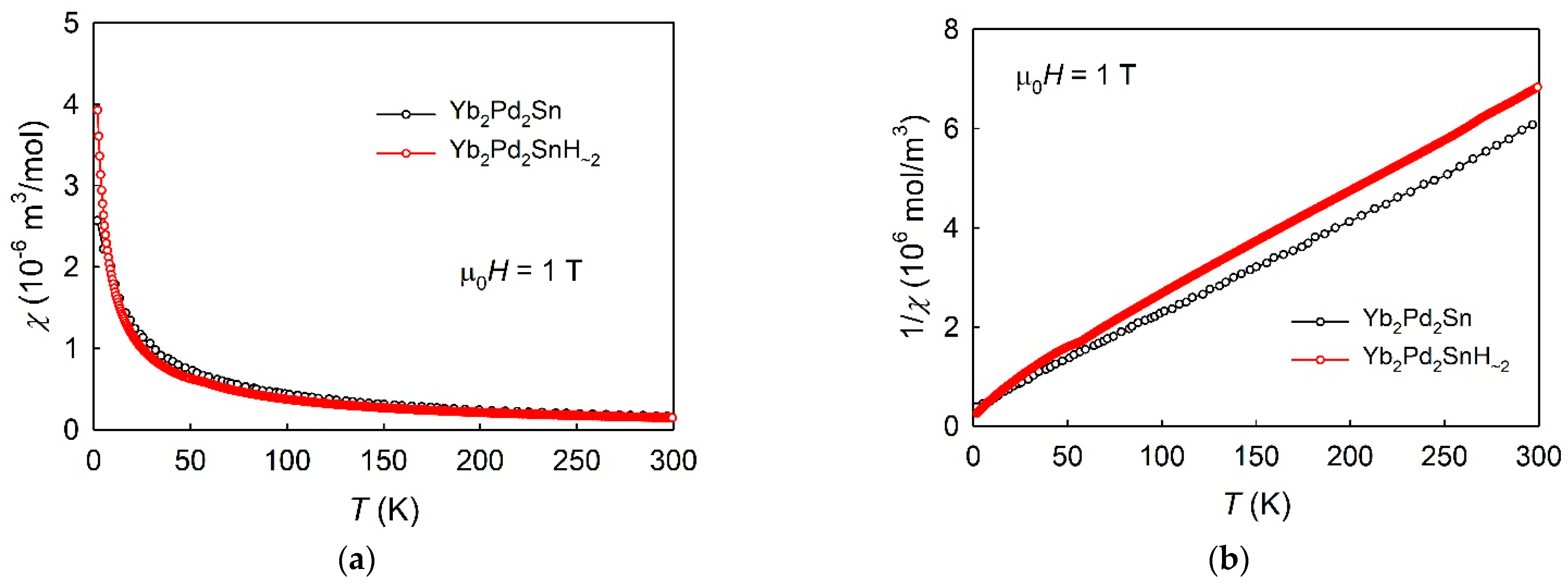
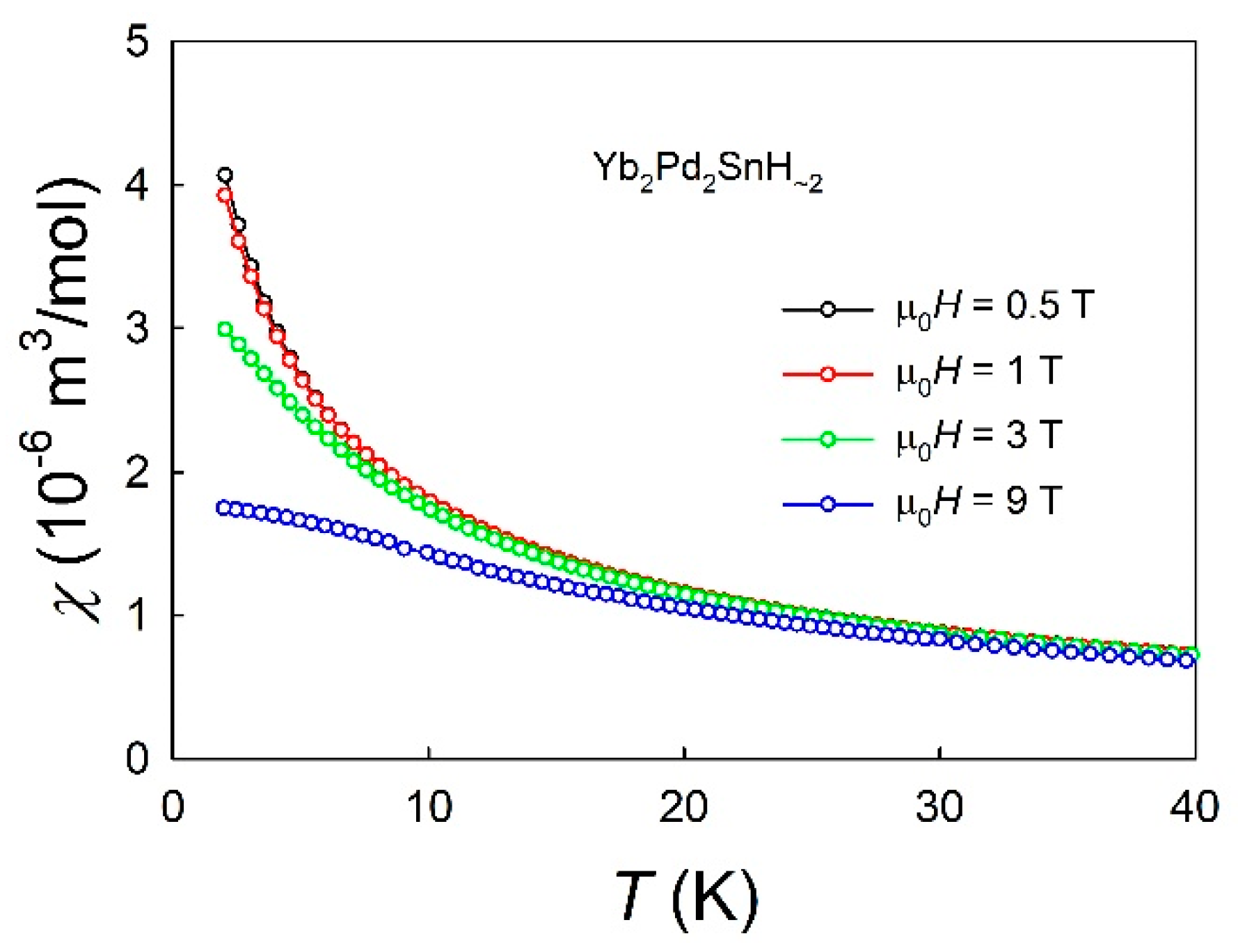
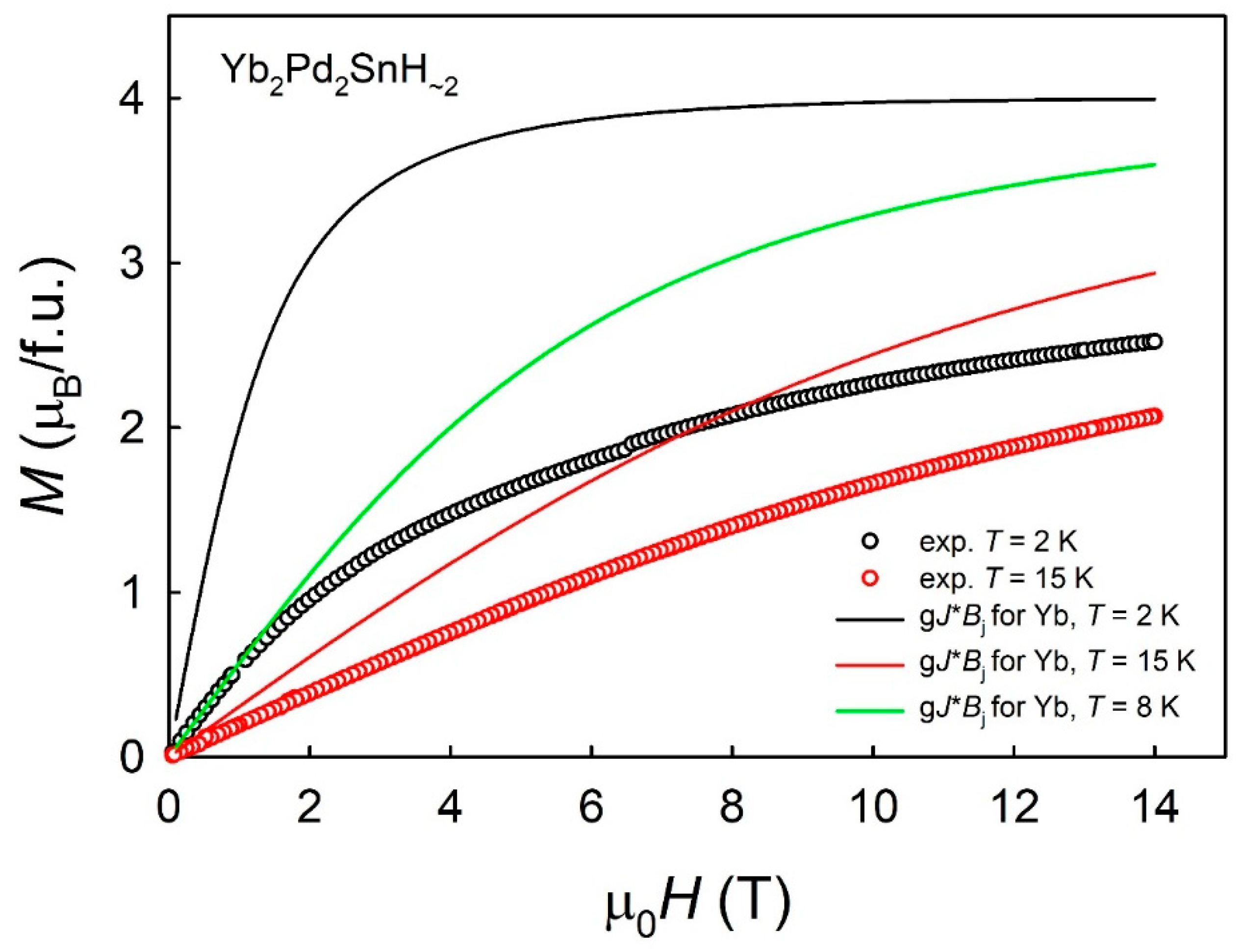
2.2. Magnetic Properties
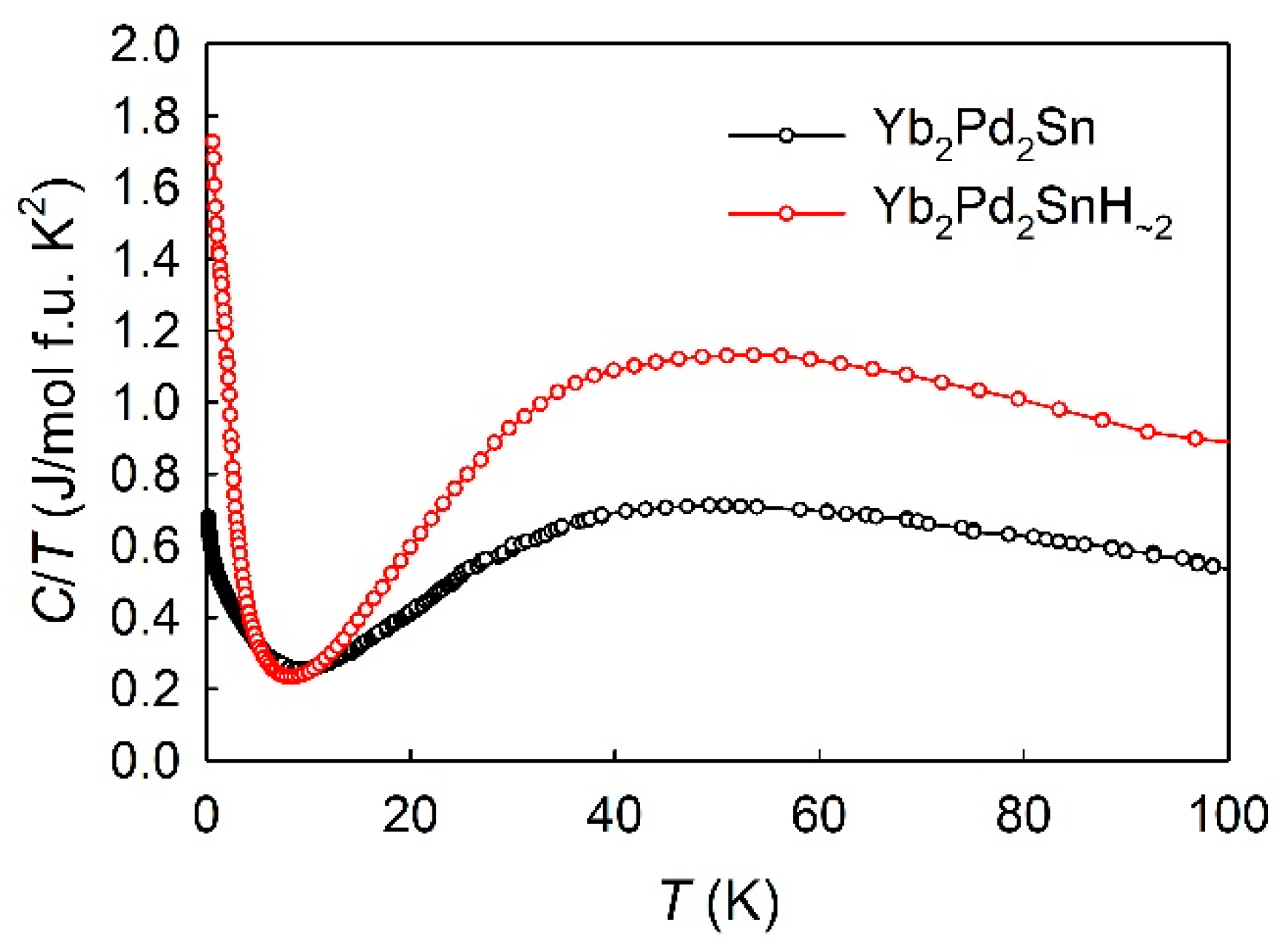
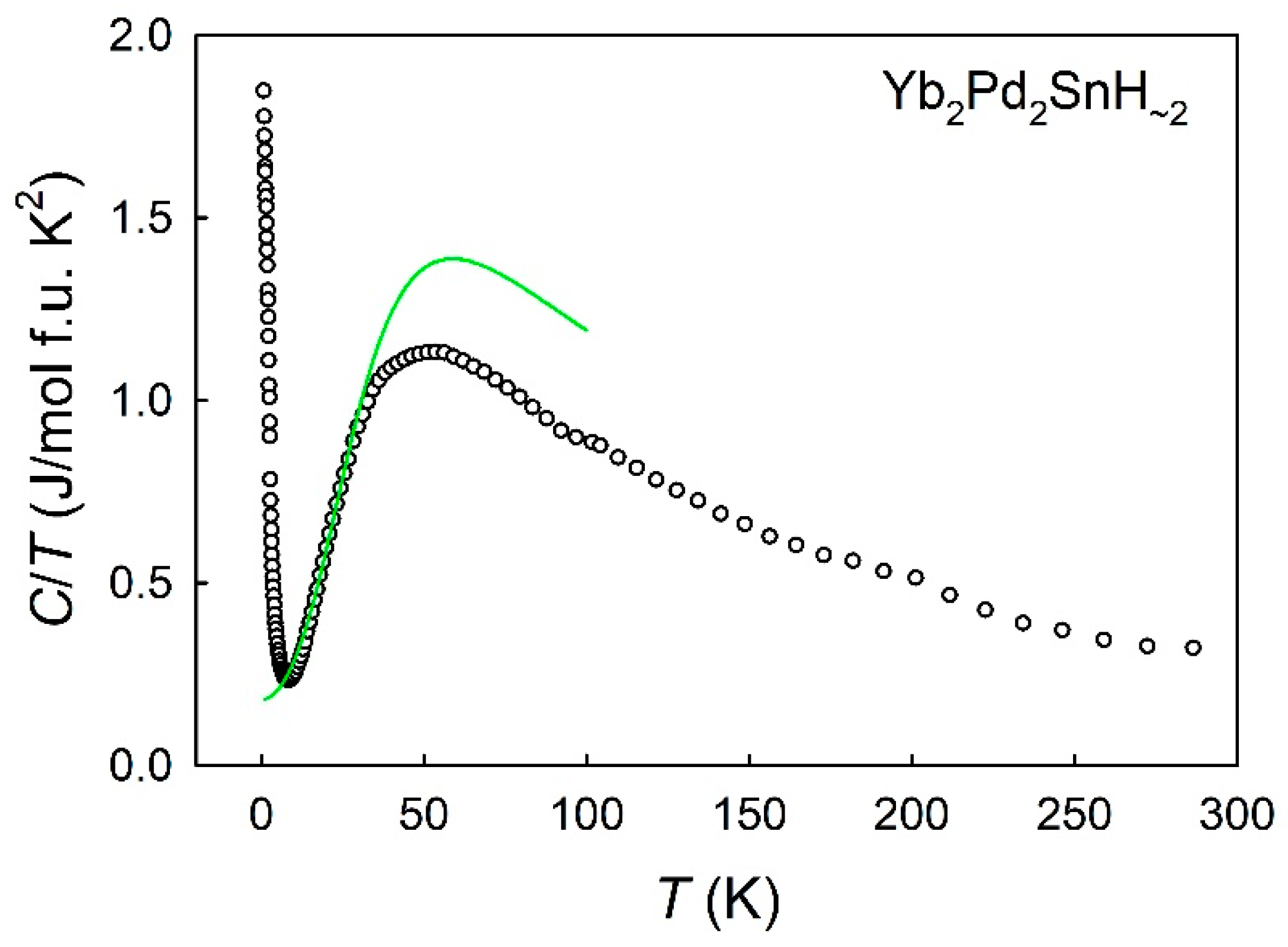
2.3. Specific Heat
3. Discussion
4. Materials and Methods
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Maiwald, J.; Mazin, I.; Gurevich, A.; Aronson, M. Superconductivity in La2Ni2In. Phys. Rev. B 2020, 102, 165125. [Google Scholar] [CrossRef]
- Dudka, A.; Nesterenko, S.; Tursina, A. Multi-temperature X-ray diffraction study of a reversible structural phase transition in the high-temperature polymorph of Ce2Rh2Ga compound. J. Alloys Compd. 2021, 890, 161759. [Google Scholar] [CrossRef]
- Sahu, B. Structural, magnetic, thermodynamic and electrical transport properties of a new compound Pr2Rh2Ga. arXiv 2021, arXiv:2102.04106. [Google Scholar]
- Menouer, S.; Abid, O.; Benzair, A.; Yakoubi, A.; Khachai, H.; Schwingenschlögl, U. First principles calculations of the structural, electronic, magnetic, and thermodynamic properties of the Nd2MgGe2 and Gd2MgGe2 intermetallic compounds. Sci. Rep. 2021, 11, 10870. [Google Scholar] [CrossRef]
- Zhang, Y. Review of the structural, magnetic and magnetocaloric properties in ternary rare earth RE2T2X type intermetallic compounds. J. Alloys Compd. 2019, 787, 1173. [Google Scholar] [CrossRef]
- Li, L.; Mi, Y. Recent progresses in exploring the rare earth based intermetallic compounds for cryogenic magnetic refrigeration. J. Alloys Compd. 2020, 823, 153810. [Google Scholar] [CrossRef]
- Nesterenko, S.N.; Tursina, A.I.; Kuznetsov, A.N. Extending interstitial tetrelides into carbides: Synthesis and crystal and electronic structures- of RE2Co2AlC (RE = La, Pr, Nd). Dalton Trans. 2023, 52, 11611. [Google Scholar] [CrossRef]
- Reimann, M.K.; Matar, S.F.; Pöttgen, R. Intermetallic compounds RE2Ga2Mg (RE = Tb-Tm, Lu) with Mo2B2Fe-type structure. Z. Für Naturforschung B 2022, 77, 693–702. [Google Scholar] [CrossRef]
- Lukachuk, M.; Pöttgen, R. Intermetallic compounds with ordered U3Si2 or Zr3Si2 type structure—Crystal chemistry, chemical bonding and physical properties. Z. Kristallogr. 2003, 218, 767–787. [Google Scholar] [CrossRef]
- Shastry, B.; Sutherland, B. Exact ground state of a quantum mechanical antiferromagnet. Physica B+C 1981, 108, 1069–1070. [Google Scholar] [CrossRef]
- Shimura, Y.; Sakakibara, T.; Iwakawa, K.; Sugiyama, K.; Onuki, Y. Low temperature magnetization of Yb2Pt2Pb with the Shastry-Sutherland type lattice and a high-rank multipole interaction. J. Phys. Soc. Jpn. 2012, 81, 103601. [Google Scholar] [CrossRef]
- Wu, L.S.; Gannon, W.J.; Zaliznyak, A.I.; Tsvelik, A.M.; Brockmann, M.; Caux, J.S.; Kim, M.S.; Qiu, Y.; Copley, J.R.D.; Ehlers, G.; et al. Orbital-exchange and fractional quantum number excitations in an f-electron metal, Yb2Pt2Pb. Science 2016, 352, 1206–1210. [Google Scholar] [CrossRef]
- Sereni, J.G.; Gómez Berisso, M.; Braghta, A.; Schmerber, G.; Kappler, J.P. Unstable Shastry-Sutherland phase in Ce2Pd2Sn. Phys. Rev. B 2009, 80, 024428. [Google Scholar] [CrossRef]
- Kim, M.S.; Aronson, M.C. Spin Liquids and Antiferromagnetic Order in the Shastry-Sutherland-Lattice Compound Yb2Pt2Pb. Phys. Rev. Lett. 2013, 110, 017201. [Google Scholar] [CrossRef]
- Kim, M.S.; Bennett, M.C.; Aronson, M.C. Yb2Pt2Pb: Magnetic frustration in the Shastry-Sutherland lattice. Phys. Rev. B 2008, 77, 144425. [Google Scholar] [CrossRef]
- Sahu, B.; Djoumessi, R.; Strydom, A. Spin-glass behavior in Shastry-Sutherland lattice of Tm2Cu2In. J. Magn. Magn. Mat. 2021, 543, 168599. [Google Scholar] [CrossRef]
- Sachdev, S. Quantum Phase Transitions; Cambridge University Press: Cambridge, UK, 2000. [Google Scholar] [CrossRef]
- Mašková, S.; Havela, L.; Kolomiets, A.; Miliyanchuk, K.; Andreev, A.V.; Nakotte, H.; Peterson, J.; Skourski, Y.; Yasin, S.; Zherlitsyn, S.; et al. Onset of magnetic order in U2(Ni1-xFex)2Sn-H. J. Korean Phys. Soc. 2013, 62, 1542–1546. [Google Scholar] [CrossRef]
- Maskova, S.; Chotard, J.-N.; Denys, R.V.; Miliyanchuk, K.; Yartys, V.; Giovannini, M.; Akselrud, L.; Halevy, I.; Prokleska, J.; Havela, L. Nd2Ni2MgH8 hydride: Synthesis, structure and magnetic properties. Intermetallics 2017, 87, 13–20. [Google Scholar] [CrossRef]
- Muramatsu, T.; Kanemasa, T.; Kagayama, T.; Shimizu, K.; Aoki, Y.; Sato, H.; Giovannini, M.; Bonville, P.; Zlatic, V.; Aviani, I.; et al. Reentrant quantum criticality in Yb2Pd2Sn. Phys. Rev. B 2011, 83, 180404. [Google Scholar] [CrossRef]
- Bauer, E.; Khan, R.T.; Giovannini, M.; Ritter, C. Appearance of long range magnetic order in a nonmagnetic periphery: Yb2Pd2(In,Sn). Phys. Status Solidi B 2010, 247, 717–719. [Google Scholar] [CrossRef]
- Ruderman, M.A.; Kittel, C. Indirect exchange coupling of nuclear magnetic moments by conduction electrons. Phys. Rev. 1954, 96, 99–102. [Google Scholar] [CrossRef]
- Kong, B.; Zhang, L.; Chen, X.-R.; Deng, M.-S.; Cai, L.-C.; Ling-Hu, R.-F. Magnetic, electronic and optical properties of lanthanide hydrides, GdH2 and GdH3. J. Phys. Chem. Solids 2013, 74, 1322–1328. [Google Scholar] [CrossRef]
- Carlin, R.L.; Chirico, R.D.; Joung, K.O.; Shenoy, G.K.; Westlake, D.G. Magnetic ordering in GdH3. Phys. Lett. A 1980, 75, 413–414. [Google Scholar] [CrossRef]
- Huiberts, J.N.; Griessen, R.; Rector, J.H.; Wijngaarden, R.J.; Dekker, J.P.; De Groot, D.G.; Koeman, N.J. Yttrium and lanthanum hydride films with switchable optical properties. Nature 1996, 380, 231–234. [Google Scholar] [CrossRef]
- Fuggle, J.C.; Hillebrecht, F.U.; Zeller, R.; Zołnierek, Z.; Bennett, P.A.; Freiburg, C. Electronic structure of Ni and Pd alloys. I. X-ray photoelectron spectroscopy of the valence bands. Phys. Rev. B 1983, 27, 2145–2178. [Google Scholar] [CrossRef]
- Havela, L. Hydrogen impact on magnetic properties of metallic systems. J. Alloys Compd. 2022, 895, 162721. [Google Scholar] [CrossRef]
- Javorsky, P.; Tuan, N.C.; Divis, M.; Havela, L.; Svoboda, P.; Sechovsky, V.; Hilscher, G. Magnetic properties of RCuAl and RNiAl compounds. J. Magn. Magn. Mat. 1995, 140–144, 1139–1140. [Google Scholar] [CrossRef]
- Gaudin, E.; Matar, S.F.; Pottgen, R.; Eul, M.; Chevalier, B. Drastic Change of the Ferromagnetic Properties of the Ternary Germanide GdTiGe through Hydrogen Insertion. Inorg. Chem. 2011, 50, 11046–11054. [Google Scholar] [CrossRef]
- Mašková, S.; Kolomiets, A.; Havela, L.; Andreev, A.V.; Svoboda, P. Impact of hydrogen absorption on crystal structure and magnetic properties of RE2T2X compounds. J. Alloys Compd. 2015, 645, S76–S79. [Google Scholar] [CrossRef]
- Chevalier, B.; Gaudin, E.; Tencé, S.; Malaman, B.; Fernandez, J.R.; André, G.; Coqblin, B. Hydrogenation Inducing Antiferromagnetism in the Heavy-Fermion Ternary Silicide CeRuSi. Phys. Rev. B 2008, 77, 014414. [Google Scholar] [CrossRef]
- Iwasieczko, W.; Kazorowski, D. Influence of hydrogen absorption on structural and magnetic properties of Ce2Ni2In. J. Alloys Compd. 2010, 507, 376–379. [Google Scholar] [CrossRef]
- Chevalier, B.; Matar, S.F.; Ménétrier, M.; Sanchez Marcos, J.; Rodriguez Fernandez, J. Influence of Ce-H bonding on the physical properties of the hydrides CeCoSiH(1.0) and CeCoGeH(1.0). J. Phys. Condens. Matter 2006, 18, 6045–6056. [Google Scholar] [CrossRef] [PubMed]
- Iwasieczko, W.; Kazorowski, D. Hydrogen insertion effect on the magnetic properties of Ce2Pd2In. J. Alloys Compd. 2011, 509, 1384–1388. [Google Scholar] [CrossRef]
- Iwasieczko, W.; Kazorowski, D. Hydrogenation studies on structural and magnetic properties of antiferromagnetic Kondo lattice Ce2Cu2In. J. Alloys Compd. 2013, 553, 364–366. [Google Scholar] [CrossRef]
- Drulis, M.K.; Drulis, H. Double Kondo-lattice-like system in the ytterbium deuterides. J. Alloys Compd. 2004, 366, 9–14. [Google Scholar] [CrossRef]
- Warf, J.C.; Hardcastle, K.J. Rare Earth-Hydrogen Systems. IV. The Higher Hydride of Ytterbium, a New Type of Hydride. Inorg. Chem. 1966, 5, 1736–1740. [Google Scholar] [CrossRef]
- Iwasieczko, W.; Drulis, M.; Drulis, H. Magnetic properties of the ytterbium non-stoichiometric trihydride phase. J. Alloys Compd. 1997, 259, 62–64. [Google Scholar] [CrossRef]
- Aoki, Y.; Sato, H.R.; Sugawara, H.; Sato, H. Anomalous magnetic properties of Heusler superconductor YbPd2Sn. Phys. C Supercond. 2000, 333, 187–194. [Google Scholar] [CrossRef]
- Dönni, A.; Fischer, P.; Fauth, F.; Convert, P.; Aoki, Y.; Sugawara, H.; Sato, H. Antiferromagnetic ordering in the cubic superconductor YbPd2Sn. Phys. B 1999, 259–261, 705–706. [Google Scholar] [CrossRef]
- Shoemaker, D.P.; Shoemaker, C.B. Concerning atomic sites and capacities for hydrogen absorption in the AB2 Friauf-Laves phases. J. Less-Common Met. 1979, 68, 43–58. [Google Scholar] [CrossRef]
- Maskova-Cerna, S.; Kolomiets, A.; Prchal, J.; Halevy, I.; Buturlim, V.; Nikolaevsky, M.; Koloskova, O.; Kozelj, P.; König, M.; Divis, M.; et al. Insight into the physics of the 5f -band antiferromagnet U2Ni2Sn from the pressure dependence of crystal structure and electrical resistivity. Phys. Rev. B 2021, 103, 035104. [Google Scholar] [CrossRef]
- van den Berg, G.J. Magnetism—Selected Topic; Foner, S., Ed.; Gordon and Breach Science Publishers: New York, NY, USA, 1976; pp. 117–172. [Google Scholar]
- Steppke, A.; Brando, M.; Oeschler, N.; Krellner, C.; Geibel, C.; Steglich, F. Nuclear contribution to the specific heat of Yb(Rh0.93Co0.07)2Si2. Phys. Status Solidi B 2010, 247, 737–739. [Google Scholar] [CrossRef]
- Havela, L.; Miliyanchuk, K.; Kolomiets, A. f-Element hydrides: Structure and magnetism. Int. J. Mat. Res. 2009, 100, 1182–1186. [Google Scholar] [CrossRef]
- Kim, J.S.; Alwood, J.; Getty, S.A.; Sharifi, F.; Stewart, G.R. U2Co2Sn: An undoped non-Fermi-liquid system with Ce ≈ γ − A√T. Phys. Rev. B 2000, 62, 6986–6990. [Google Scholar] [CrossRef]
- Rojas, D.P.; Espeso, J.I.; Rodríguez Fernández, L.; Fernández Barquín, L. Heat capacity of nanocrystalline Yb2O3. Ceram. Int. 2022, 48, 879–886. [Google Scholar] [CrossRef]
- Bickers, N.E.; Cox, D.L.; Wilkins, J.W. Self-consistent large-N expansion for normal-state properties of dilute magnetic alloys. Phys. Rev. B 1987, 36, 2036–2079. [Google Scholar] [CrossRef]
- Brodale, G.E.; Fisher, R.A.; Philips, N.E.; Fouquet, J. Pressure dependence of the low-temperature specific heat of the heavy-fermion compound CeAl3. Phys. Rev. Lett. 1986, 56, 390–393. [Google Scholar] [CrossRef] [PubMed]
- Sudhindra, R.; Poettgen, R. Antiferromagnetic ordering in the heavy-fermion system Ce2Au2Cd. Phys. Rev. B 2005, 72, 214435. [Google Scholar]
- Sandratskii, L.M.; Havela, L. Microscopic nature of drastic influence of hydrogen on the magnetic anisotropy of 5f-electron system. Phys. Rev. B 2020, 101, 100409. [Google Scholar] [CrossRef]
- Rodriguez-Carvajal, J. Recent developments of the program FULLPROF. Comm. Powder Diffr. IUCr Newsl. 2001, 26, 12–19. [Google Scholar]

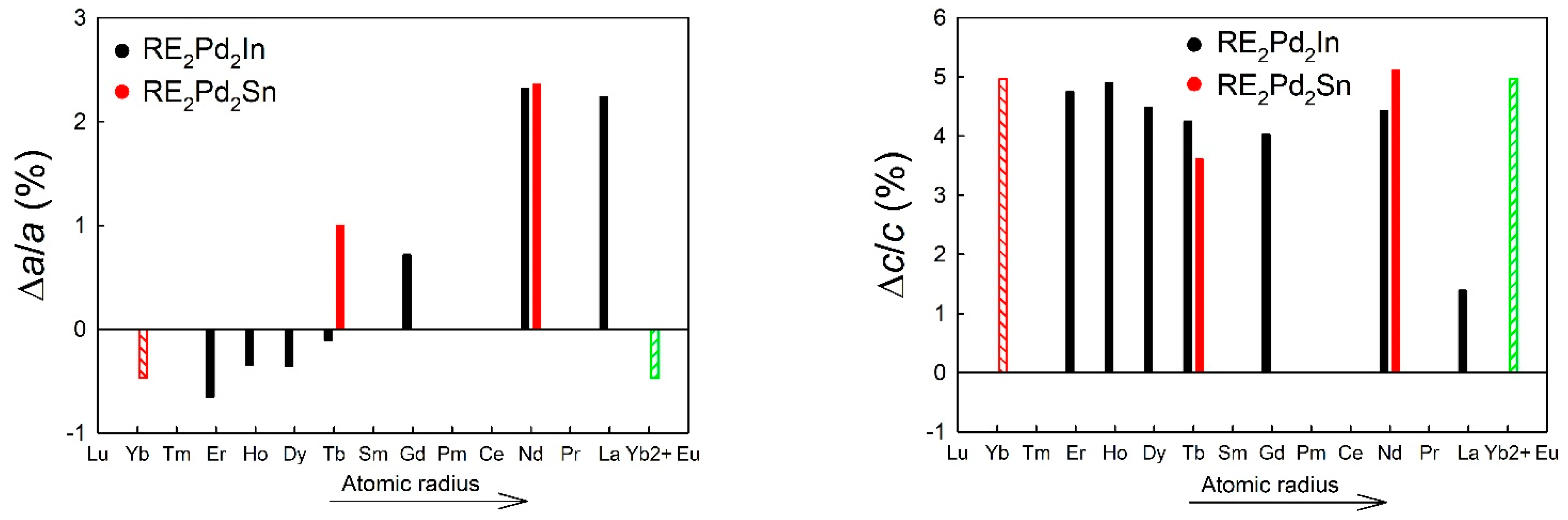

| Yb2Pd2Sn | Yb2Pd2SnH≈2 | ||
|---|---|---|---|
| a (Å) | 7.580 | 7.544 (2) | |
| c (Å) | 3.639 | 3.820 (1) | |
| V (Å3) | 209.1 | 217.4 (1) | |
| ∆a/a (%) | - | −0.47 | |
| ∆c/c (%) | - | 4.97 | |
| ∆V/V (%) | - | 3.97 | |
| Atomic positions | Yb | (0.1724, 0.6724, 0.5) | (0.1712 (4), 0.6712 (4), 0.5) |
| Pd | (0.3716, 0.8716, 0) | (0.3699 (5), 0.8699 (5), 0) | |
| Sn | (0, 0, 0) | (0, 0, 0) | |
| Shortest basal-plane dYb-Yb (Å) | 3.696 | 3.653 | |
| c-axis dYb-Yb (Å) | 3.639 | 3.8197 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Maskova-Cerna, S.; Bauer, E.; Giovannini, M.; Havela, L. Heavy-Fermion Properties of Yb2Pd2SnH≈2. Inorganics 2023, 11, 414. https://doi.org/10.3390/inorganics11100414
Maskova-Cerna S, Bauer E, Giovannini M, Havela L. Heavy-Fermion Properties of Yb2Pd2SnH≈2. Inorganics. 2023; 11(10):414. https://doi.org/10.3390/inorganics11100414
Chicago/Turabian StyleMaskova-Cerna, Silvie, Ernst Bauer, Mauro Giovannini, and Ladislav Havela. 2023. "Heavy-Fermion Properties of Yb2Pd2SnH≈2" Inorganics 11, no. 10: 414. https://doi.org/10.3390/inorganics11100414
APA StyleMaskova-Cerna, S., Bauer, E., Giovannini, M., & Havela, L. (2023). Heavy-Fermion Properties of Yb2Pd2SnH≈2. Inorganics, 11(10), 414. https://doi.org/10.3390/inorganics11100414