Bulk Physical Properties of a Magnetic Weyl Semimetal Candidate NdAlGe Grown by a Laser Floating-Zone Method
Abstract
:1. Introduction
2. Materials and Methods
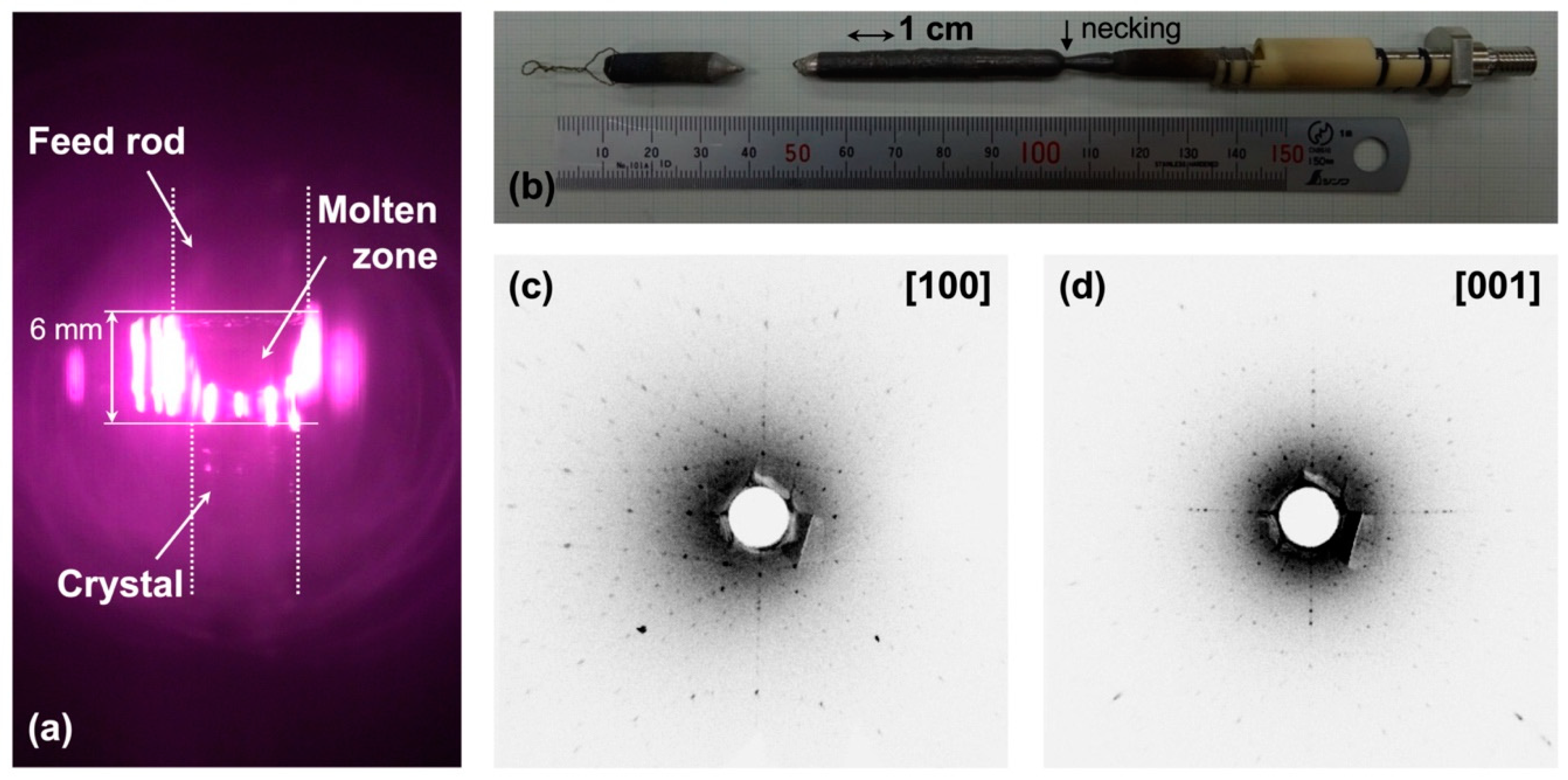
2.1. Crystal Growth by the Floating-Zone Method
2.2. Laser Floating-Zone Technique
2.3. Preparation of Feed and Seed Rods
2.4. Crystal Growth
2.5. Characterization
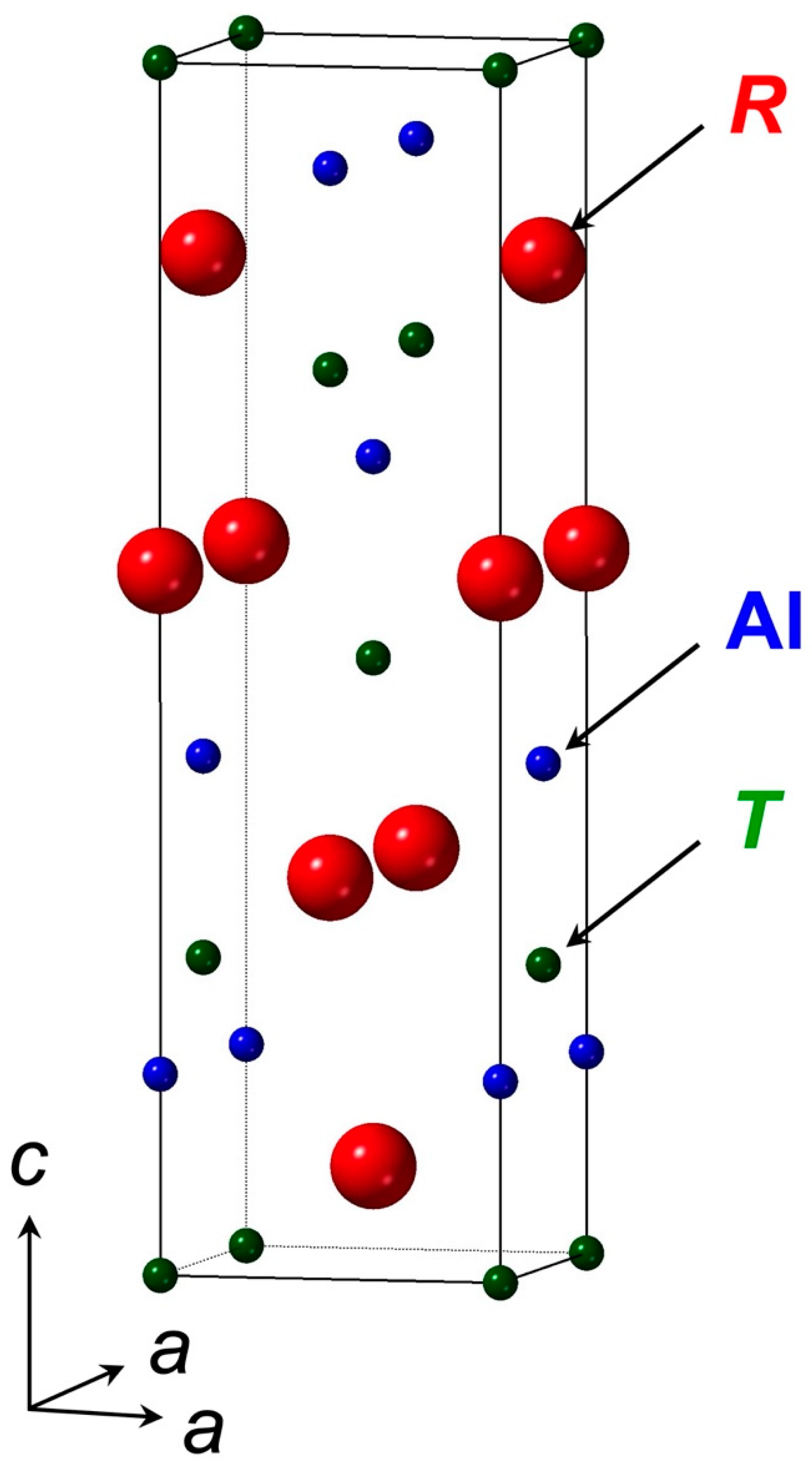
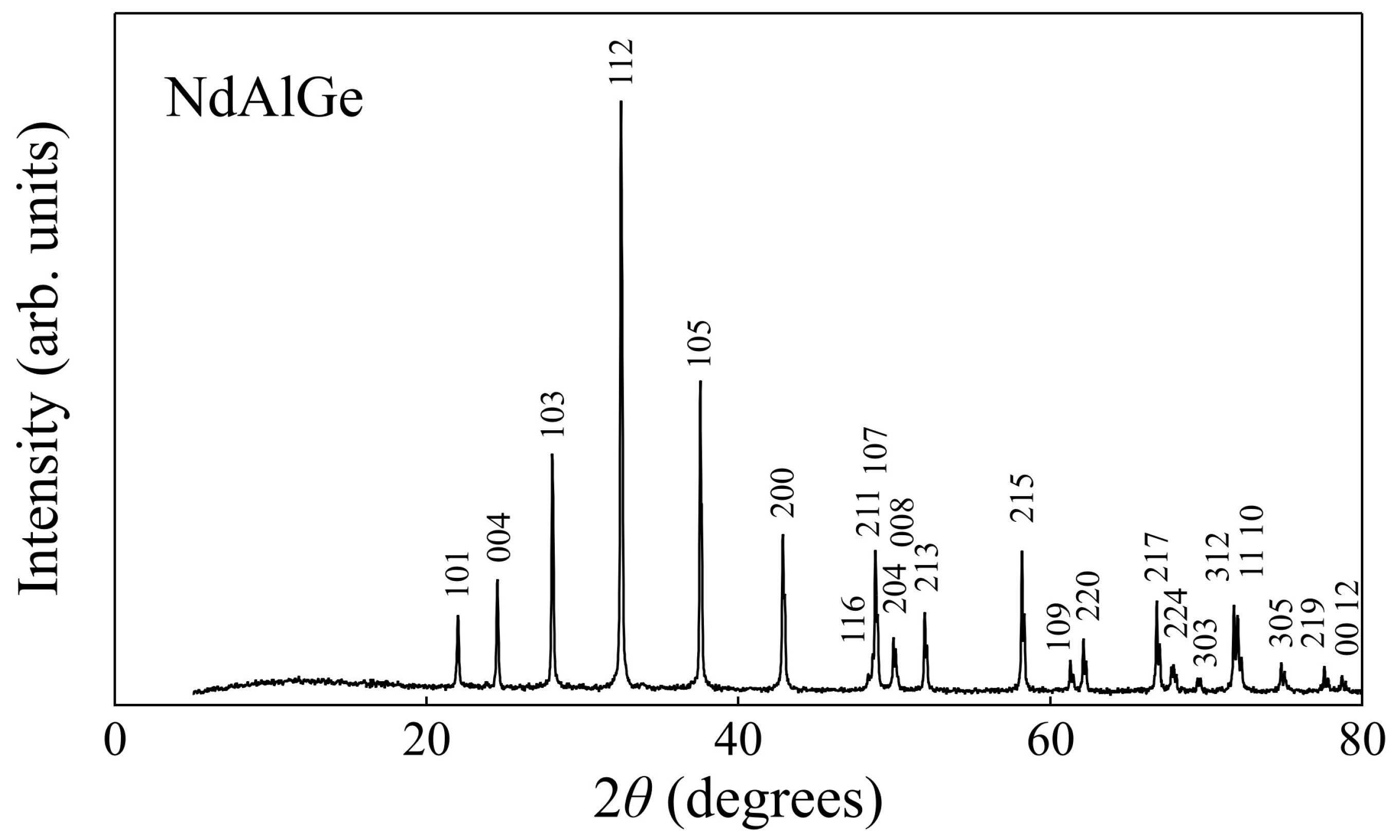
3. Results and Discussion
3.1. Crystal Growth
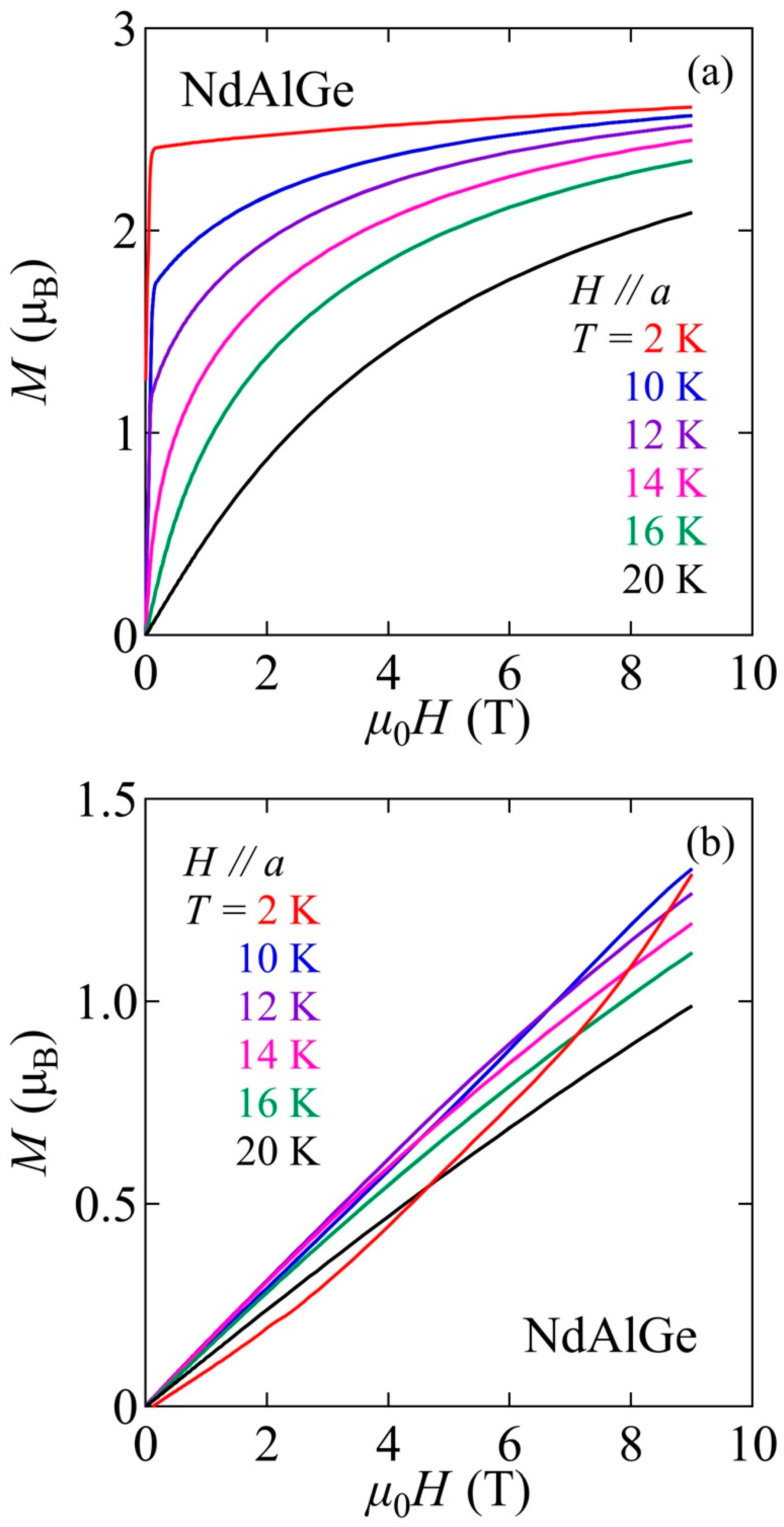
3.2. Bulk Properties of the Grown Crystal
4. Summary
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Wang, Z.; Zhang, S.-C. Chiral Anomaly, Charge Density Waves, and Axion Strings from Weyl Semimetals. Phys. Rev. B 2013, 87, 161107. [Google Scholar] [CrossRef] [Green Version]
- Burkov, A.A. Topological Semimetals. Nat. Mater. 2016, 15, 1145. [Google Scholar] [CrossRef] [PubMed]
- Yan, B.; Felser, C. Topological Materials: Weyl Semimetals. Annu. Rev. Condens. Matter Phys. 2017, 8, 337. [Google Scholar] [CrossRef] [Green Version]
- Armitage, N.P.; Mele, E.J.; Vishwanath, A. Weyl and Dirac Semimetals in Three-Dimensional Solids. Rev. Mod. Phys. 2018, 90, 015001. [Google Scholar] [CrossRef] [Green Version]
- Nagaosa, N.; Morimoto, T.; Tokura, Y. Transport, Magnetic and Optical Properties of Weyl Materials. Nat. Rev. Mater. 2020, 5, 621. [Google Scholar] [CrossRef]
- Otrokov, M.M.; Klimovskikh, I.I.; Bentmann, H.; Estyunin, D.; Zeugner, A.; Aliev, Z.S.; Gaß, S.; Wolter, A.U.B.; Koroleva, A.V.; Shikin, A.M.; et al. Prediction and Observation of an Antiferromagnetic Topological Insulator. Nature 2019, 576, 416. [Google Scholar] [CrossRef] [Green Version]
- Rienks, E.D.L.; Wimmer, S.; Sánchez-Barriga, J.; Caha, O.; Mandal, P.S.; Růžička, J.; Ney, A.; Steiner, H.; Volobuev, V.V.; Groiss, H.; et al. Large Magnetic Gap at the Dirac Point in Bi2Te3/MnBi2Te4 Heterostructures. Nature 2019, 576, 423. [Google Scholar] [CrossRef] [Green Version]
- Bernevig, B.A.; Felser, C.; Beidenkopf, H. Progress and Prospects in Magnetic Topological Materials. Nature 2022, 603, 41. [Google Scholar] [CrossRef]
- He, Q.L.; Hughes, T.L.; Armitage, N.P.; Tokura, Y.; Wang, K.L. Topological Spintronics and Magnetoelectronics. Nat. Mater. 2022, 21, 15. [Google Scholar] [CrossRef]
- Giustino, F.; Lee, J.H.; Trier, F.; Bibes, M.; Winter, S.M.; Valentí, R.; Son, Y.-W.; Taillefer, L.; Heil, C.; Figueroa, A.I.; et al. The 2021 Quantum Materials Roadmap. J. Phys. Mater. 2020, 3, 042006. [Google Scholar] [CrossRef]
- Puphal, P.; Mielke, C.; Kumar, N.; Soh, Y.; Shang, T.; Medarde, M.; White, J.S.; Pomjakushina, E. Bulk Single-Crystal Growth of the Theoretically Predicted Magnetic Weyl Semimetals RAlGe (R = Pr, Ce). Phys. Rev. Mater. 2019, 3, 024204. [Google Scholar] [CrossRef] [Green Version]
- Chang, G.; Singh, B.; Xu, S.-Y.; Bian, G.; Huang, S.-M.; Hsu, C.-H.; Belopolski, I.; Alidoust, N.; Sanchez, D.S.; Zheng, H.; et al. Magnetic and Noncentrosymmetric Weyl Fermion Semimetals in the RAlGe Family of Compounds (R = Rare Earth). Phys. Rev. B 2018, 97, 041104. [Google Scholar] [CrossRef] [Green Version]
- Yao, X.; Verma, R.; Graf, D.E.; Yang, H.Y.; Bahrami, F.; Zhang, R.; Aczel, A.A.; Subedi, S.; Torchinsky, D.H.; Sun, J.; et al. Topological Spiral Magnetism in the Weyl Semimetal SmAlSi. arXiv 2022, arXiv:2206.05121. [Google Scholar]
- Gaudet, J.; Yang, H.-Y.; Baidya, S.; Lu, B.; Xu, G.; Zhao, Y.; Rodriguez-Rivera, J.A.; Hoffmann, C.M.; Graf, D.E.; Torchinsky, D.H.; et al. Weyl-Mediated Helical Magnetism in NdAlSi. Nat. Mater. 2021, 20, 1650. [Google Scholar] [CrossRef]
- Puphal, P.; Pomjakushin, V.; Kanazawa, N.; Ukleev, V.; Gawryluk, D.J.; Ma, J.; Naamneh, M.; Plumb, N.C.; Keller, L.; Cubitt, R.; et al. Topological Magnetic Phase in the Candidate Weyl Semimetal CeAlGe. Phys. Rev. Lett. 2020, 124, 017202. [Google Scholar] [CrossRef] [Green Version]
- Meng, B.; Wu, H.; Qiu, Y.; Wang, C.; Liu, Y.; Xia, Z.; Yuan, S.; Chang, H.; Tian, Z. Large Anomalous Hall Effect in Ferromagnetic Weyl Semimetal Candidate PrAlGe. APL Mater. 2019, 7, 051110. [Google Scholar] [CrossRef] [Green Version]
- Destraz, D.; Das, L.; Tsirkin, S.S.; Xu, Y.; Neupert, T.; Chang, J.; Schilling, A.; Grushin, A.G.; Kohlbrecher, J.; Keller, L.; et al. Magnetism and Anomalous Transport in the Weyl Semimetal PrAlGe: Possible Route to Axial Gauge Fields. NPJ Quantum Mater. 2020, 5, 5. [Google Scholar] [CrossRef] [Green Version]
- Yang, H.-Y.; Singh, B.; Lu, B.; Huang, C.-Y.; Bahrami, F.; Chiu, W.-C.; Graf, D.; Huang, S.-M.; Wang, B.; Lin, H.; et al. Transition from Intrinsic to Extrinsic Anomalous Hall Effect in the Ferromagnetic Weyl Semimetal PrAlGe1-xSix. APL Mater. 2020, 8, 011111. [Google Scholar] [CrossRef] [Green Version]
- Wang, J.-F.; Dong, Q.X.; Huang, Y.F.; Wang, Z.S.; Guo, Z.P.; Wang, Z.J.; Ren, Z.A.; Li, G.; Sun, P.J.; Dai, X.; et al. Temperature Dependent Quantum Oscillations at a Constant Magnetic Field in a Magnetic Weyl Semimetal. arXiv 2022, arXiv:2201.06412, 06412. [Google Scholar]
- Piva, M.M.; Souza, J.C.; Brousseau-Couture, V.; Pakuszewski, K.R.; John, J.K.; Adriano, C.; Côté, M.; Pagliuso, P.G.; Nicklas, M. Tuning the Nontrivial Topological Properties of the Weyl Semimetal CeAlSi. arXiv 2021, arXiv:2111.05742, 05742. [Google Scholar]
- He, X.; Li, Y.; Zeng, H.; Zhu, Z.; Cao, C.; Luo, Y. Pressure Tuning Domain-Wall Chirality in Noncentrosymmetric Magnetic Weyl Semimetal CeAlGe. arXiv 2022, arXiv:2207.08442. [Google Scholar]
- Sanchez, D.S.; Chang, G.; Belopolski, I.; Lu, H.; Yin, J.-X.; Alidoust, N.; Xu, X.; Cochran, T.A.; Zhang, X.; Bian, Y.; et al. Observation of Weyl Fermions in a Magnetic Non-centrosymmetric Crystal. Nat. Commun. 2020, 11, 3356. [Google Scholar] [CrossRef] [PubMed]
- Zhao, J.; Liu, W.; Rahman, A.U.; Meng, F.; Ling, L.; Xi, C.; Tong, W.; Bai, Y.; Tian, Z.; Zhong, Y.; et al. Field-Induced Tricritical Phenomenon and Magnetic Structures in Magnetic Weyl Semimetal Candidate NdAlGe. New J. Phys. 2022, 24, 013010. [Google Scholar] [CrossRef]
- Schmehr, J.L.; Wilson, S.D. Active Crystal Growth Techniques for Quantum Materials. Annu. Rev. Mater. Res. 2017, 47, 153. [Google Scholar] [CrossRef]
- Basov, D.N.; Averitt, R.D.; Hsieh, D. Towards Properties on Demand in Quantum Materials. Nat. Mater. 2017, 16, 1077. [Google Scholar] [CrossRef]
- Samarth, N. Quantum Materials Discovery from a Synthesis Perspective. Nat. Mater. 2017, 16, 1068. [Google Scholar] [CrossRef]
- Bobowski, J.S.; Kikugawa, N.; Miyoshi, T.; Suwa, H.; Xu, H.-S.; Yonezawa, S.; Sokolov, D.A.; Mackenzie, A.P.; Maeno, Y. Improved Single-Crystal Growth of Sr2RuO4. Condens. Matter 2019, 4, 6. [Google Scholar] [CrossRef] [Green Version]
- Ito, T.; Ushiyama, T.; Yanagisawa, Y.; Tomioka, Y.; Shindo, I.; Yanase, A. Laser-Diode-Heated Floating Zone (LDFZ) Method Appropriate to Crystal Growth of Incongruently Melting Materials. J. Cryst. Growth 2013, 363, 264. [Google Scholar] [CrossRef] [Green Version]
- Rey-García, F.; Ibáñez, R.; Angurel, L.A.; Costa, F.M.; de la Fuente, G.F. Laser Floating Zone Growth: Overview, Singular Materials, Broad Applications, and Future Perspectives. Crystals 2020, 11, 38. [Google Scholar] [CrossRef]
- Kaneko, Y.; Tokura, Y. Floating Zone Furnace Equipped with a High Power Laser of 1 kW Composed of Five Smart Beams. J. Cryst. Growth 2020, 533, 125435. [Google Scholar] [CrossRef] [Green Version]
- Admasu, A.S.; Vavilapalli, D.S. The Laser-Diode Heated Floating Zone Method for Automated Optimal Synthesis of Refractory Oxides and Alloys. arXiv 2021, arXiv:2103.05587. [Google Scholar]
- Neubauer, A.; Jonietz, F.; Meven, M.; Georgii, R.; Brandl, G.; Behr, G.; Böni, P.; Pfleiderer, C. Optical Floating Zone Growth of High-Quality Cu2MnAl Single Crystals. Nucl. Instrum. Methods Phys. Res. A 2012, 688, 66. [Google Scholar] [CrossRef] [Green Version]
- Brunt, D.; Hatnean, M.C.; Petrenko, O.A.; Lees, M.R.; Balakrishnan, G. Single-Crystal Growth of Metallic Rare-Earth Tetraborides by the Floating-Zone Technique. Crystals 2019, 9, 211. [Google Scholar] [CrossRef] [Green Version]
- Amigó, M.L.; Maljuk, A.; Manna, K.; Stahl, Q.; Felser, C.; Hess, C.; Wolter, A.U.B.; Geck, J.; Seiro, S.; Büchner, B. Laser-Assisted Floating Zone Growth of BaFe2S3 Large-Sized Ferromagnetic-Impurity-Free Single Crystals. Crystals 2021, 11, 758. [Google Scholar] [CrossRef]
- Sinha, M.; Vivanco, H.K.; Wan, C.; Siegler, M.A.; Stewart, V.J.; Pogue, E.A.; Pressley, L.A.; Berry, T.; Wang, Z.; Johnson, I.; et al. Twisting of 2D Kagomé Sheets in Layered Intermetallics. ACS Cent. Sci. 2021, 7, 1381. [Google Scholar] [CrossRef] [PubMed]
- Bauer, A.; Neubauer, A.; Münzer, W.; Regnat, A.; Benka, G.; Meven, M.; Pedersen, B.; Pfleiderer, C. Ultra-High Vacuum Compatible Induction-Heated Rod Casting Furnace. Rev. Sci. Instrum. 2016, 87, 063909. [Google Scholar] [CrossRef] [Green Version]
- Nagai, I.; Shirakawa, N.; Ikeda, S.-I.; Iwasaki, R.; Nishimura, H.; Kosaka, M. Highest Conductivity Oxide SrMoO3 Grown by a Floating-Zone Method under Ultralow Oxygen Partial Pressure. Appl. Phys. Lett. 2005, 87, 024105. [Google Scholar] [CrossRef]
- Mao, Z.Q.; Maeno, Y.; Fukazawa, H. Crystal Growth of Sr2RuO4. Mater. Res. Bull. 2000, 35, 1813. [Google Scholar] [CrossRef]
- Perry, R.S.; Maeno, Y. Systematic Approach to the Growth of High-Quality Single Crystals of Sr3Ru2O7. J. Cryst. Growth 2004, 271, 134. [Google Scholar] [CrossRef] [Green Version]
- Zhou, M.; Hooper, J.; Fobes, D.; Mao, Z.Q.; Golub, V.; O’Connor, C.J. Electronic and Magnetic Properties of Triple-Layered Ruthenate Sr4Ru3O10 Single Crystals Grown by a Floating-Zone Method. Mater. Res. Bull. 2005, 40, 942. [Google Scholar] [CrossRef]
- Kikugawa, N.; Balicas, L.; Mackenzie, A.P. Physical Properties of Single-Crystalline CaRuO3 Grown by a Floating-Zone Method. J. Phys. Soc. Jpn. 2009, 78, 014701. [Google Scholar] [CrossRef]
- Kikugawa, N.; Baumbach, R.; Brooks, J.S.; Terashima, T.; Uji, S.; Maeno, Y. Single-Crystal Growth of a Perovskite Ruthenate SrRuO3 by the Floating-Zone Method. Cryst. Growth Des. 2015, 15, 5573. [Google Scholar] [CrossRef]
- Kikugawa, N.; Sokolov, D.A.; Nagasawa, T.; Mackenzie, A.P. Single-Crystal Growth of Sr2RuO4 by the Floating-Zone Method Using an Infrared Image Furnace with Improved Halogen Lamps. Crystals 2021, 11, 392. [Google Scholar] [CrossRef]
- Martien, D.; Williamsen, M.; Spagna, S.; Black, R.; DaPron, T.; Hogan, T.; Snow, D. An Ultrasensitive Differential Capacitive Dilatometer. IEEE Trans. Magn. 2019, 55, 1. [Google Scholar] [CrossRef]
- Gschneidner, K.A.; Pecharsky, V.K. Magnetocaloric Materials. Annu. Rev. Mater. Sci. 2000, 30, 387. [Google Scholar] [CrossRef]
- Wang, T.; Guo, Y.; Wang, C.; Yang, S. Correlation between Non-centrosymmetic Structure and Magnetic Properties in Weyl Semimetal NdAlGe. Solid State Commun. 2020, 321, 114041. [Google Scholar] [CrossRef]
- Barron, T.H.K.; White, G.K. Heat Capacity and Thermal Expansion at Low Temperatures; Kluwer Academic/Plenum: New York, NY, USA, 1999. [Google Scholar]
- Tishin, A.M. Magnetocaloric Effect: From Theory to Practice, in Encyclopedia of Materials: Science and Technology; Buschow, K.H.J., Cahn, R.W., Flemings, M.C., Ilschner, B., Kramer, E.J., Mahajan, S., Veyssière, P., Eds.; Elsevier: Oxford, UK, 2001; pp. 5035–5041. [Google Scholar]
- Patra, M.; Majumdar, S.; Giri, S.; Xiao, Y.; Chatterji, T. Magnetic, Magnetocaloric and Magnetoresistive Properties of Cubic Laves Phase HoAl2 Single Crystal. J. Phys. Condens. Matter 2014, 26, 046004. [Google Scholar] [CrossRef]
- Pecharsky, V.K.; Gschneidner, K.A., Jr. Giant Magnetocaloric Effect in Gd5(Si2Ge2). Phys. Rev. Lett. 1997, 78, 4494. [Google Scholar] [CrossRef]
- Fujieda, S.; Fujita, A.; Fukamichi, K. Large Magnetocaloric Effect in La(FexSi1-x)13 Itinerant-Electron Metamagnetic Compounds. Appl. Phys. Lett. 2002, 81, 1276. [Google Scholar] [CrossRef]
- Gupta, S.; Suresh, K.G. Review on Magnetic and Related Properties of RTX Compounds. J. Alloys Compd. 2015, 618, 562. [Google Scholar] [CrossRef] [Green Version]
- Krenke, T.; Duman, E.; Acet, M.; Wassermann, E.F.; Moya, X.; Mañosa, L.; Planes, A. Inverse Magnetocaloric Effect in Ferromagnetic Ni-Mn-Sn Alloys. Nat. Mater. 2005, 4, 450. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Van Peski-Tinbergen, T.; Dekker, A.J. Spin-Dependent Scattering and Resistivity of Magnetic Metals and Alloys. Physica 1963, 29, 917. [Google Scholar] [CrossRef]
- Maki, S.; Adachi, K. Antiferromagnetism and Weak Ferromagnetism of Disordered Bcc Cr-Mn Alloys. J. Phys. Soc. Jpn. 1979, 46, 1131. [Google Scholar] [CrossRef]
- Terashima, T.; Kikugawa, N.; Kasahara, S.; Watashige, T.; Shibauchi, T.; Matsuda, Y.; Wolf, T.; Böhmer, A.E.; Hardy, F.; Meingast, C.; et al. Pressure-Induced Antiferromagnetic Transition and Phase Diagram in FeSe. J. Phys. Soc. Jpn. 2015, 84, 063701. [Google Scholar] [CrossRef]


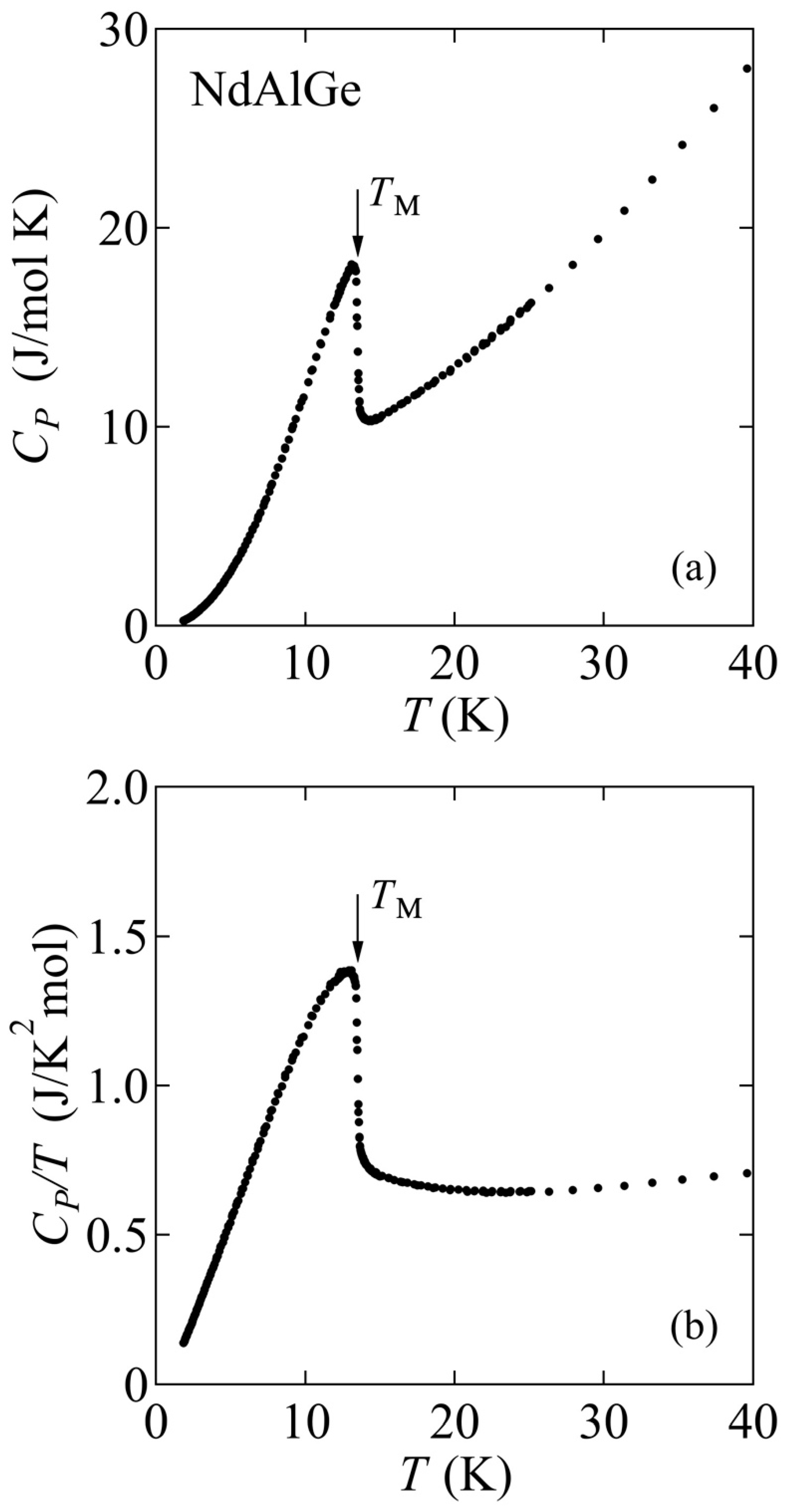
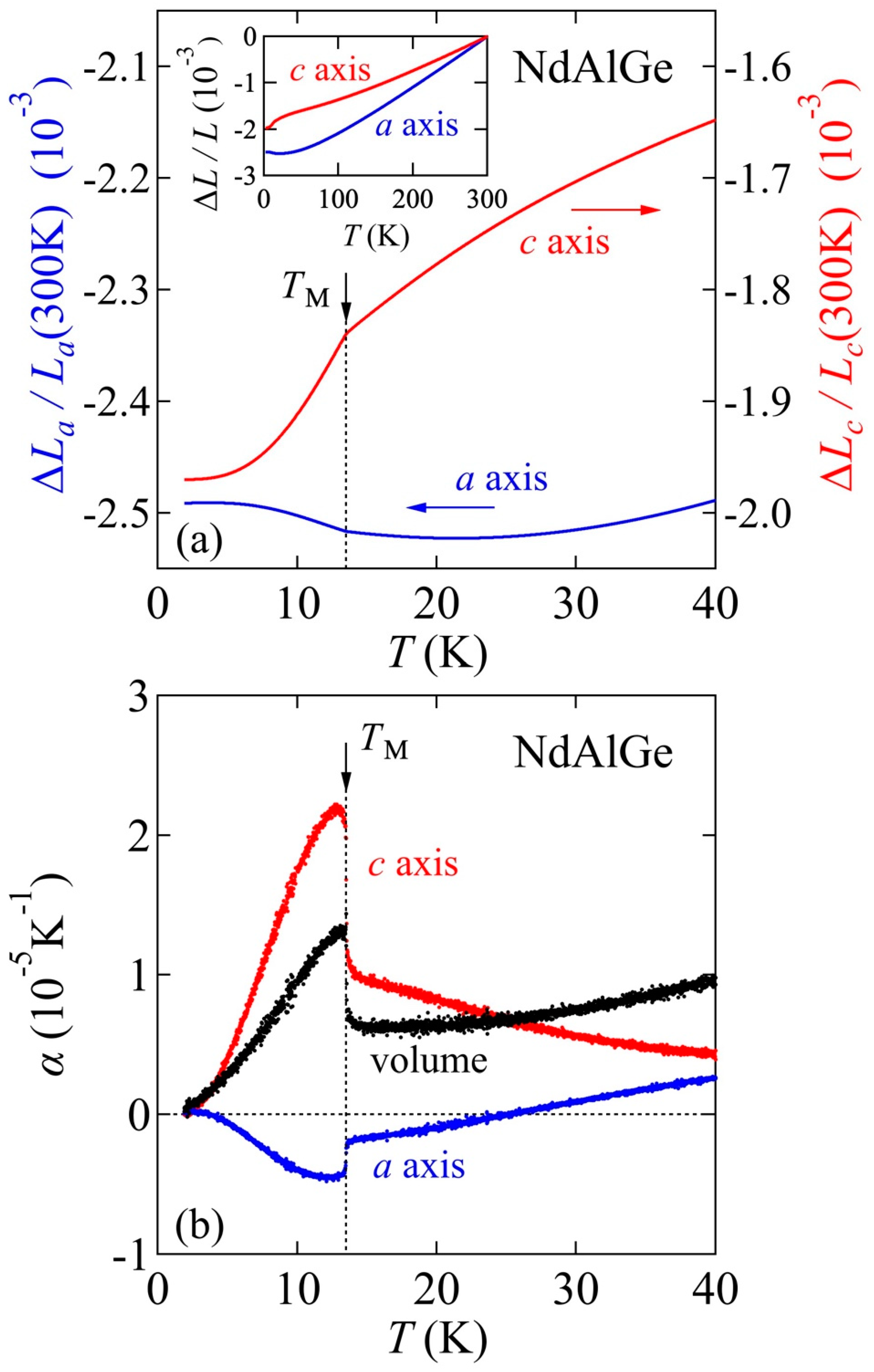
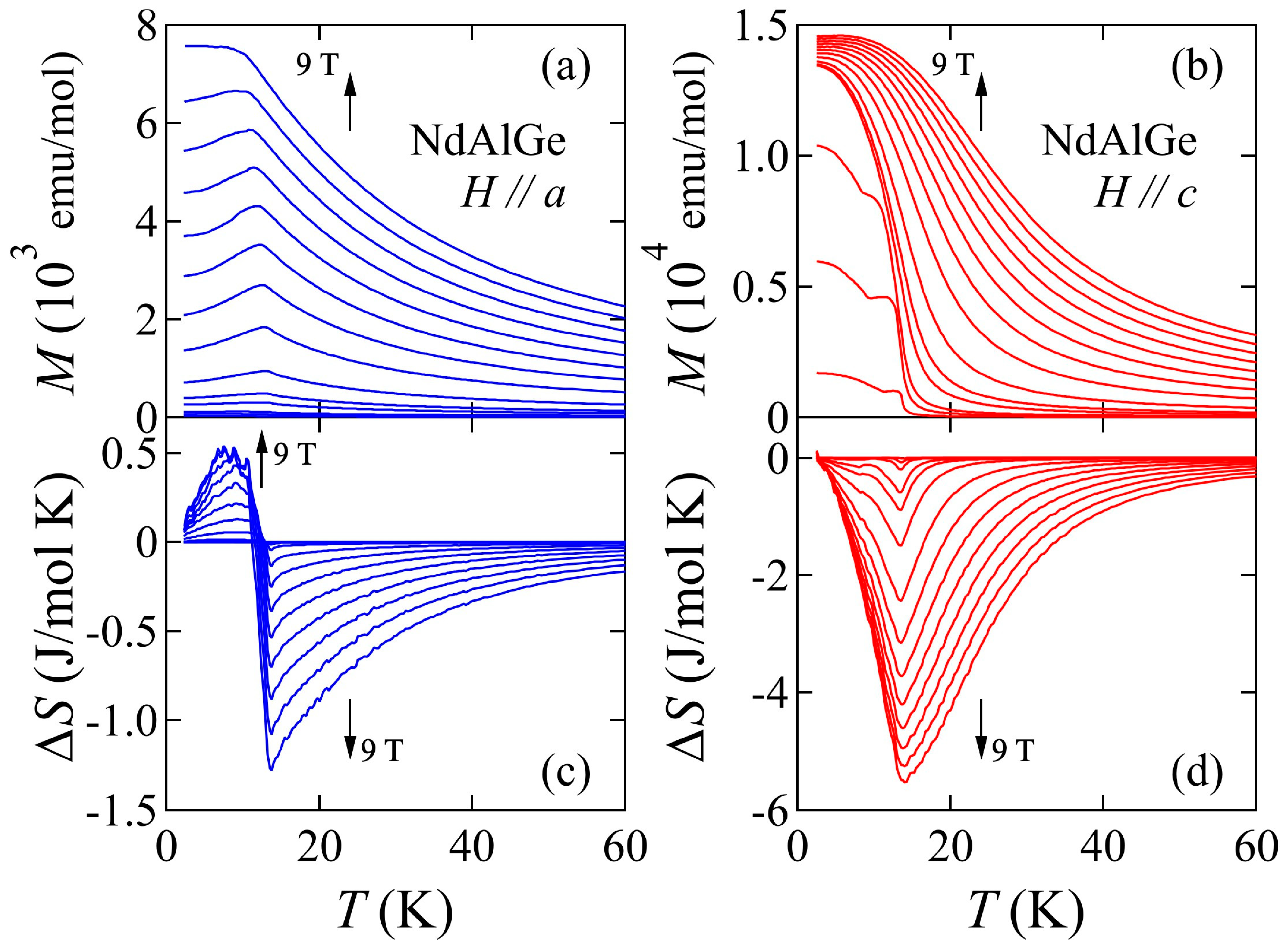

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kikugawa, N.; Terashima, T.; Kato, T.; Hayashi, M.; Yamaguchi, H.; Uji, S. Bulk Physical Properties of a Magnetic Weyl Semimetal Candidate NdAlGe Grown by a Laser Floating-Zone Method. Inorganics 2023, 11, 20. https://doi.org/10.3390/inorganics11010020
Kikugawa N, Terashima T, Kato T, Hayashi M, Yamaguchi H, Uji S. Bulk Physical Properties of a Magnetic Weyl Semimetal Candidate NdAlGe Grown by a Laser Floating-Zone Method. Inorganics. 2023; 11(1):20. https://doi.org/10.3390/inorganics11010020
Chicago/Turabian StyleKikugawa, Naoki, Taichi Terashima, Takashi Kato, Momoko Hayashi, Hitoshi Yamaguchi, and Shinya Uji. 2023. "Bulk Physical Properties of a Magnetic Weyl Semimetal Candidate NdAlGe Grown by a Laser Floating-Zone Method" Inorganics 11, no. 1: 20. https://doi.org/10.3390/inorganics11010020
APA StyleKikugawa, N., Terashima, T., Kato, T., Hayashi, M., Yamaguchi, H., & Uji, S. (2023). Bulk Physical Properties of a Magnetic Weyl Semimetal Candidate NdAlGe Grown by a Laser Floating-Zone Method. Inorganics, 11(1), 20. https://doi.org/10.3390/inorganics11010020





