Prisoners’ Dilemma in a Spatially Separated System Based on Spin–Photon Interactions
Abstract
1. Introduction
2. Materials and Methods
2.1. Playing the Prisoners’ Dilemma Game
2.2. Decomposition of SWAP and Gates via Spin–Photon Interactions
2.3. Implementing the Gate

3. Results
3.1. Distributed Quantum Circuit for Spatially Separated Players
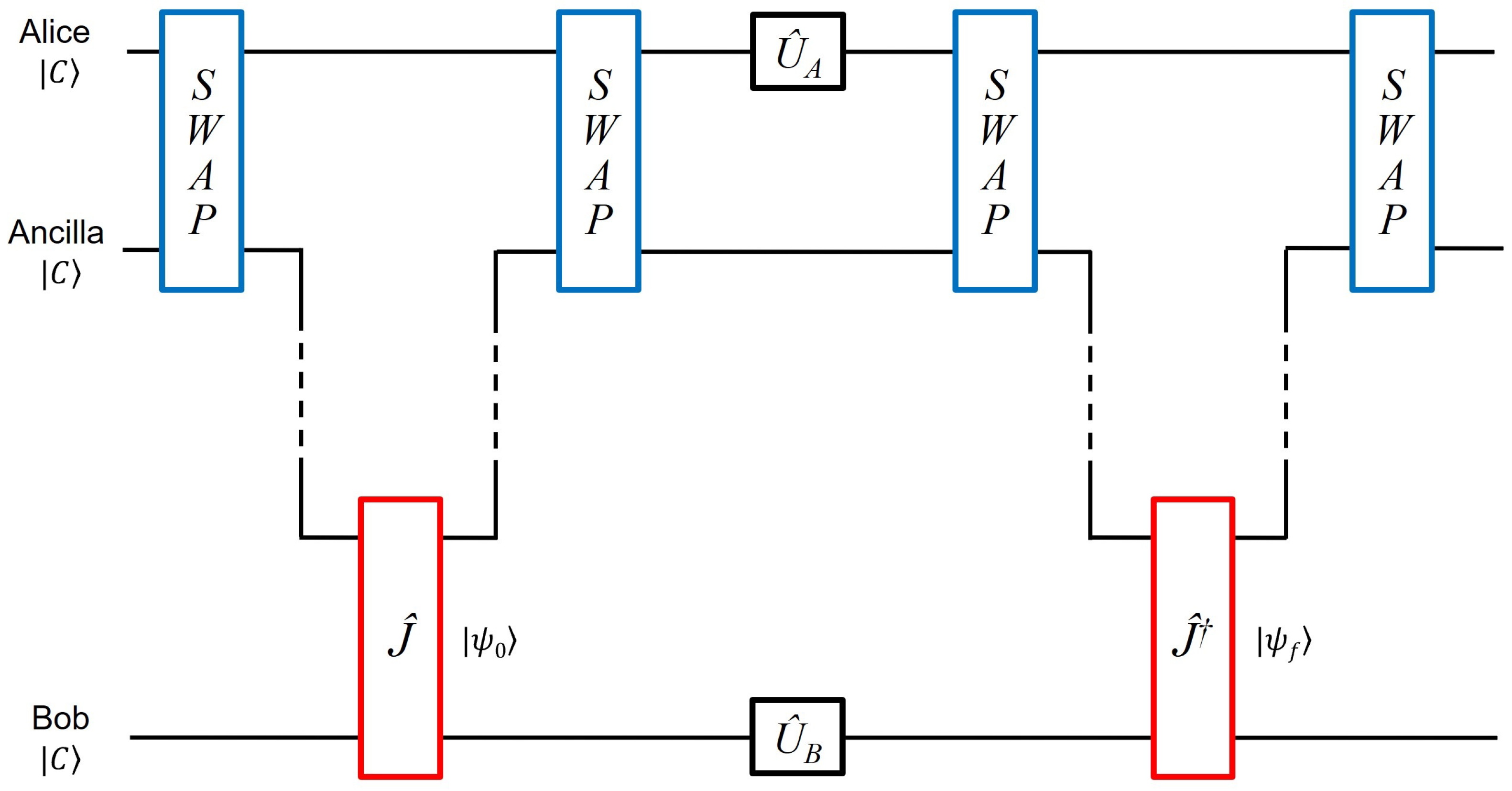
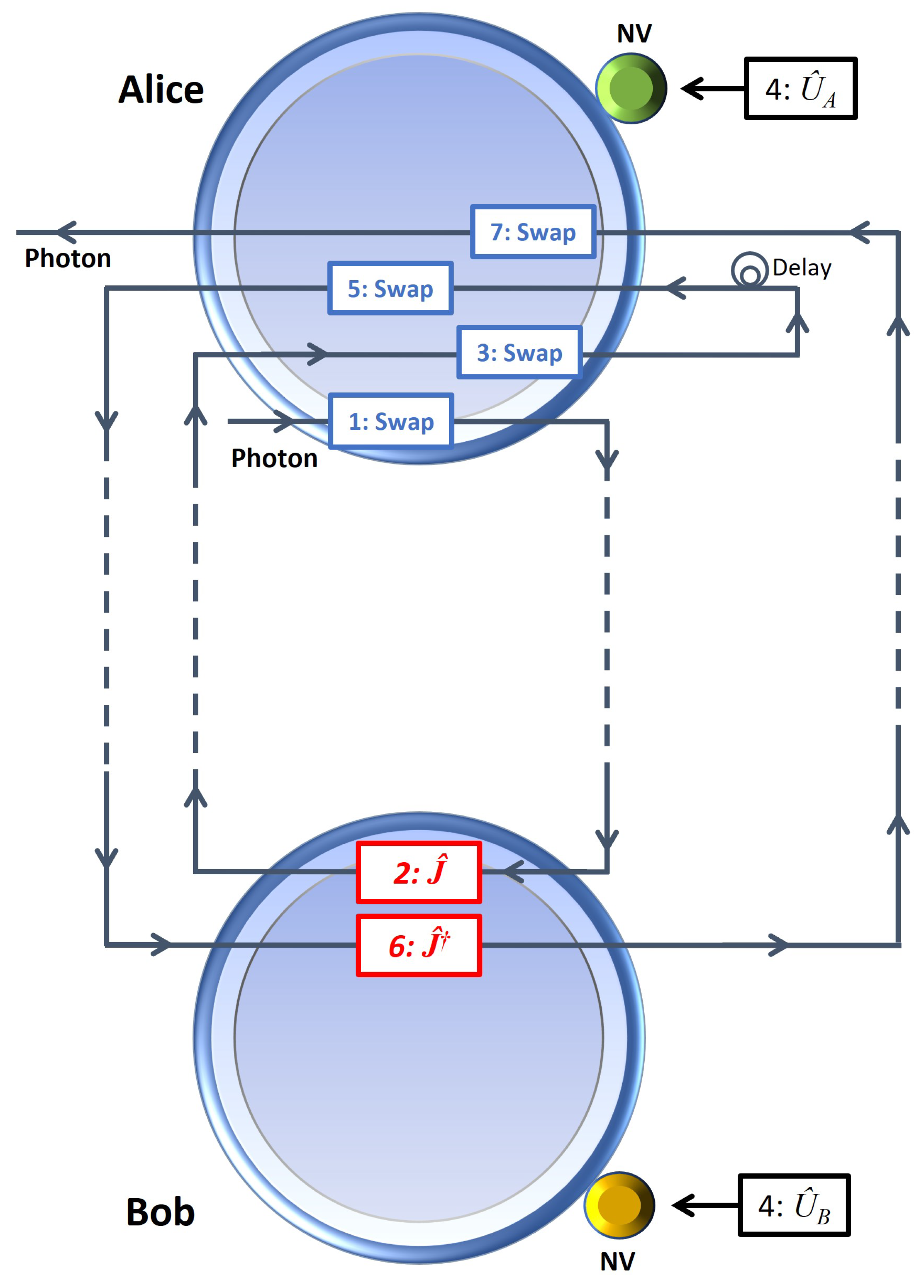
3.2. Physical Setup Based on Spin–Photon Interactions
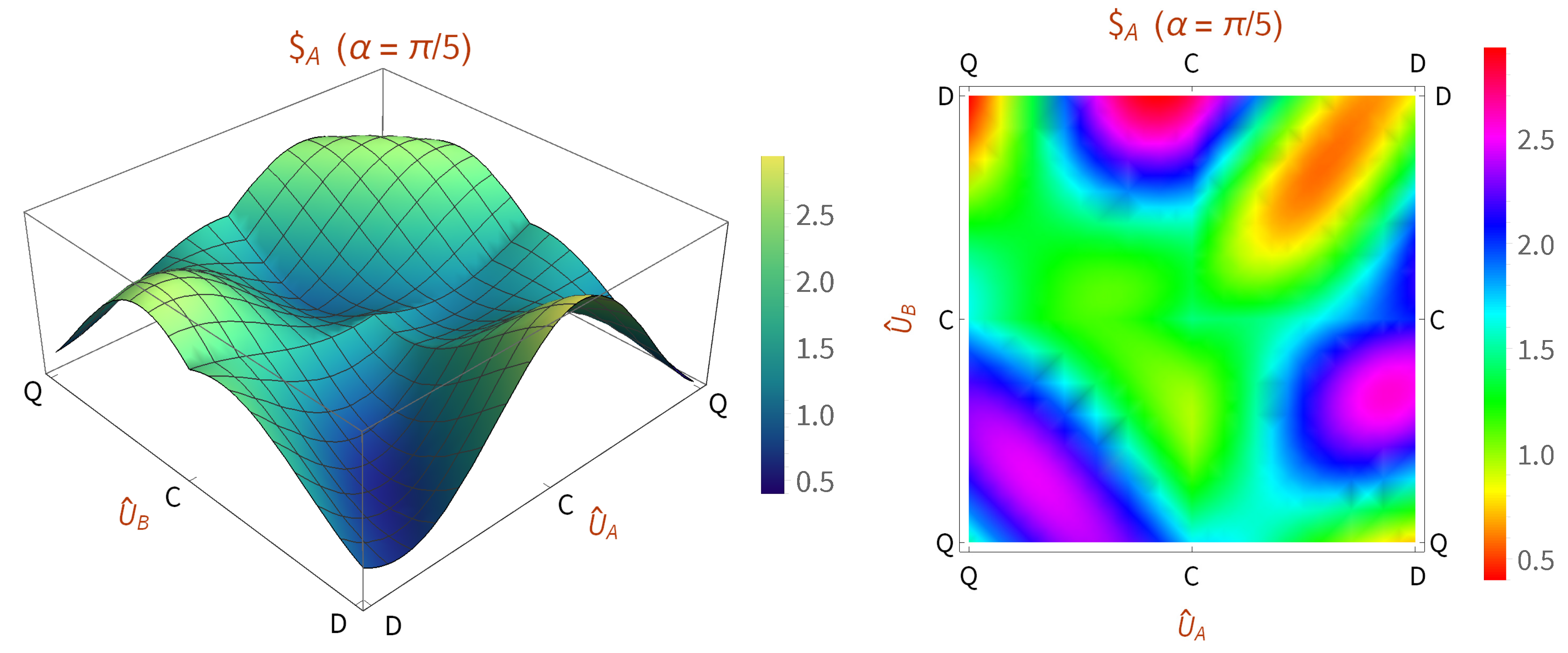
3.3. Imperfect Realization and the Revival of the Dilemma
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| CNOT | Controlled-NOT gate |
| CP | Controlled-phase gate |
| CZ | Controlled-Z gate |
| Strategy to cooperate | |
| Strategy to defect | |
| Quantum strategy | |
| ESD | Entanglement sudden death |
| MSG | Magic square game |
| NMR | Nuclear magnetic resonance |
| NV | Nitrogen vacancy |
| PD | Prisoners’ dilemma |
References
- Eisert, J.; Wilkens, M.; Lewenstein, M. Quantum Games and Quantum Strategies. Phys. Rev. Lett. 1999, 83, 3077–3080. [Google Scholar] [CrossRef]
- Brassard, G.; Broadbent, A.; Tapp, A. Quantum pseudo-telepathy. Found. Phys. 2005, 35, 1877–1907. [Google Scholar] [CrossRef]
- Özdemir, S.K.; Shimamura, J.; Morikoshi, F.; Imoto, N. Dynamics of a discoordination game with classical and quantum correlations. Phys. Lett. A 2004, 333, 218–231. [Google Scholar] [CrossRef]
- Bennett, C.H.; Wiesner, S.J. Communication via one- and two-particle operators on Einstein-Podolsky-Rosen states. Phys. Rev. Lett. 1992, 69, 2881–2884. [Google Scholar] [CrossRef]
- Mattle, K.; Weinfurter, H.; Kwiat, P.G.; Zeilinger, A. Dense Coding in Experimental Quantum Communication. Phys. Rev. Lett. 1996, 76, 4656–4659. [Google Scholar] [CrossRef]
- Bouwmeester, D.; Pan, J.W.; Mattle, K.; Eibl, M.; Weinfurter, H.; Zeilinger, A. Experimental quantum teleportation. Nature 1997, 390, 575–579. [Google Scholar] [CrossRef]
- Pirandola, S.; Eisert, J.; Weedbrook, C.; Furusawa, A.; Braunstein, S.L. Advances in quantum teleportation. Nat. Photonics 2015, 9, 641–652. [Google Scholar] [CrossRef]
- Li, K.; Kong, F.Z.; Yang, M.; Ozaydin, F.; Yang, Q.; Cao, Z.L. Generating Multi-Photon W-like States for Perfect Quantum Teleportation and Superdense Coding. Quant. Inf. Process. 2016, 15, 3137. [Google Scholar] [CrossRef]
- Im, D.G.; Lee, C.H.; Kim, Y.; Nha, H.; Kim, M.S.; Lee, S.W.; Kim, Y.H. Optimal teleportation via noisy quantum channels without additional qubit resources. NPJ Quantum Inf. 2021, 7, 86. [Google Scholar] [CrossRef]
- Woerner, S.; Egger, D.J. Quantum risk analysis. NPJ Quantum Inf. 2019, 5, 15. [Google Scholar] [CrossRef]
- Arute, F.; Arya, K.; Babbush, R.; Bacon, D.; Bardin, J.C.; Barends, R.; Biswas, R.; Boixo, S.; Brandao, F.G.; Buell, D.A.; et al. Quantum supremacy using a programmable superconducting processor. Nature 2019, 574, 505–510. [Google Scholar] [CrossRef] [PubMed]
- Smith, G.; Yard, J. Quantum Communication with Zero-Capacity Channels. Science 2008, 321, 1812–1815. [Google Scholar] [CrossRef] [PubMed]
- Hastings, M.B. Superadditivity of communication capacity using entangled inputs. Nat. Phys. 2009, 5, 255–257. [Google Scholar] [CrossRef]
- Bennett, C.H.; Brassard, G. Quantum cryptography: Public key distribution and coin tossing. Theor. Comput. Sci. 2014, 560, 7–11. [Google Scholar] [CrossRef]
- Scarani, V.; Bechmann-Pasquinucci, H.; Cerf, N.J.; Dušek, M.; Lütkenhaus, N.; Peev, M. The security of practical quantum key distribution. Rev. Mod. Phys. 2009, 81, 1301–1350. [Google Scholar] [CrossRef]
- Acín, A.; Cirac, J.I.; Lewenstein, M. Entanglement percolation in quantum networks. Nat. Phys. 2007, 3, 256–259. [Google Scholar] [CrossRef]
- Bugu, S.; Yesilyurt, C.; Ozaydin, F. Enhancing the W-State Quantum-Network-Fusion Process with a Single Fredkin Gate. Phys. Rev. A 2013, 87, 032331. [Google Scholar] [CrossRef]
- Yesilyurt, C.; Bugu, S.; Ozaydin, F.; Altintas, A.A.; Tame, M.; Yang, L.; Ozdemir, S.K. Deterministic Local Doubling of W states. J. Opt. Soc. Am. B 2016, 33, 2313. [Google Scholar] [CrossRef]
- Pezzé, L.; Smerzi, A. Entanglement, nonlinear dynamics, and the Heisenberg limit. Phys. Rev. Lett. 2009, 102, 100401. [Google Scholar] [CrossRef]
- Ozaydin, F.; Altintas, A.A. Quantum Metrology: Surpassing the shot-noise limit with Dzyaloshinskii-Moriya interaction. Sci. Rep. 2015, 5, 16360. [Google Scholar] [CrossRef]
- Ozaydin, F.; Altintas, A.A. Parameter estimation with Dzyaloshinskii–Moriya interaction under external magnetic fields. Opt. Quantum Electron. 2020, 52, 70. [Google Scholar] [CrossRef]
- Kim, Y.; Yoo, S.Y.; Kim, Y.H. Heisenberg-Limited Metrology via Weak-Value Amplification without Using Entangled Resources. Phys. Rev. Lett. 2022, 128, 040503. [Google Scholar] [CrossRef] [PubMed]
- Scully, M.O.; Zubairy, M.S.; Agarwal, G.S.; Walther, H. Extracting work from a single heat bath via vanishing quantum coherence. Science 2003, 299, 862–864. [Google Scholar] [CrossRef] [PubMed]
- Dag, C.B.; Niedenzu, W.; Ozaydin, F.; Mustecaplıoglu, O.E.; Kurizki, G. Temperature control in dissipative cavities by entangled dimers. J. Phys. Chem. C 2019, 123, 4035–4043. [Google Scholar] [CrossRef]
- Tuncer, A.; Izadyari, M.; Dağ, C.B.; Ozaydin, F.; Müstecaplıoğlu, Ö.E. Work and heat value of bound entanglement. Quantum Inf. Process. 2019, 18, 373. [Google Scholar] [CrossRef]
- Dawkins, R.; Davis, N. The Selfish Gene; Macat Library: London, UK, 2017. [Google Scholar] [CrossRef]
- Kastampolidou, K.; Andronikos, T. A survey of evolutionary games in biology. GeNeDis 2020, 2018, 253–261. [Google Scholar] [CrossRef]
- McFadden, J.; Al-Khalili, J. The origins of quantum biology. Proc. R. Soc. A 2018, 474, 20180674. [Google Scholar] [CrossRef]
- Marais, A.; Adams, B.; Ringsmuth, A.K.; Ferretti, M.; Gruber, J.M.; Hendrikx, R.; Schuld, M.; Smith, S.L.; Sinayskiy, I.; Krüger, T.P.; et al. The future of quantum biology. J. R. Soc. Interface 2018, 15, 20180640. [Google Scholar] [CrossRef]
- Tuszynski, J.A. From quantum chemistry to quantum biology: A path toward consciousness. J. Integr. Neurosci. 2020, 19, 687–700. [Google Scholar] [CrossRef]
- Zurek, W.H. Decoherence and the transition from quantum to classical—Revisited. In Quantum Decoherence; Springer: Berlin/Heidelberg, Germany, 2006; pp. 1–31. [Google Scholar] [CrossRef]
- Im, D.G.; Kim, Y.H. Decoherence-Induced Sudden Death of Entanglement and Bell Nonlocality. Photonics 2022, 9, 58. [Google Scholar] [CrossRef]
- Shimamura, J.; Özdemir, S.K.; Morikoshi, F.; Imoto, N. Entangled states that cannot reproduce original classical games in their quantum version. Phys. Lett. A 2004, 328, 20–25. [Google Scholar] [CrossRef][Green Version]
- Özdemir, S.K.; Shimamura, J.; Imoto, N. Quantum advantage does not survive in the presence of a corrupt source: Optimal strategies in simultaneous move games. Phys. Lett. A 2004, 325, 104–111. [Google Scholar] [CrossRef]
- Özdemir, S.K.; Shimamura, J.; Imoto, N. A necessary and sufficient condition to play games in quantum mechanical settings. New J. Phys. 2007, 9, 43. [Google Scholar] [CrossRef]
- Gawron, P.; Miszczak, J.; Sładkowski, J. Noise effects in quantum magic squares game. Int. J. Quantum Inf. 2008, 6, 667–673. [Google Scholar] [CrossRef]
- Fialík, I. Noise and the magic square game. Quantum Inf. Process. 2012, 11, 411–429. [Google Scholar] [CrossRef]
- Ramzan, M.; Khan, M. Distinguishing quantum channels via magic squares game. Quantum Inf. Process. 2010, 9, 667–679. [Google Scholar] [CrossRef][Green Version]
- Bugu, S.; Ozaydin, F.; Kodera, T. Surpassing the classical limit in magic square game with distant quantum dots coupled to optical cavities. Sci. Rep. 2020, 10, 22202. [Google Scholar] [CrossRef]
- Ozaydin, F. Quantum pseudo-telepathy in spin systems: The magic square game under magnetic fields and the Dzyaloshinskii–Moriya interaction. Laser Phys. 2020, 30, 025203. [Google Scholar] [CrossRef]
- Du, J.; Li, H.; Xu, X.; Shi, M.; Wu, J.; Zhou, X.; Han, R. Experimental Realization of Quantum Games on a Quantum Computer. Phys. Rev. Lett. 2002, 88, 137902. [Google Scholar] [CrossRef]
- Paternostro, M.; Tame, M.; Kim, M. Hybrid cluster state proposal for a quantum game. New J. Phys. 2005, 7, 226. [Google Scholar] [CrossRef]
- Shuai, C.; Mao-Fa, F. Linear optics implementation for quantum game under quantum noise. Chin. Phys. 2006, 15, 276–280. [Google Scholar] [CrossRef]
- Shuai, C.; Mao-Fa, F.; Xiao-Juan, Z. The effect of quantum noise on multiplayer quantum game. Chin. Phys. 2007, 16, 915–918. [Google Scholar] [CrossRef]
- Ramzan, M.; Khan, M.K. Noise effects in a three-player prisoner’s dilemma quantum game. J. Phys. A Math. Theor. 2008, 41, 435302. [Google Scholar] [CrossRef]
- Nielsen, M.A.; Chuang, I.L. Quantum Computation and Quantum Information: 10th Anniversary Edition; Cambridge University Press: Cambridge, UK, 2011. [Google Scholar]
- Chen, Q.; Yang, W.; Feng, M.; Du, J. Entangling separate nitrogen-vacancy centers in a scalable fashion via coupling to microtoroidal resonators. Phys. Rev. A 2011, 83, 054305. [Google Scholar] [CrossRef]
- Cheng, L.Y.; Wang, H.F.; Zhang, S. Simple schemes for universal quantum gates with nitrogen-vacancy centers in diamond. J. Opt. Soc. Am. B 2013, 30, 1821–1826. [Google Scholar] [CrossRef]
- Cheng, L.Y.; Wang, H.F.; Zhang, S.; Yeon, K.H. Quantum state engineering with nitrogen-vacancy centers coupled to low-Q microresonator. Opt. Express 2013, 21, 5988–5997. [Google Scholar] [CrossRef]
- Zang, X.P.; Yang, M.; Ozaydin, F.; Song, W.; Cao, Z.L. Generating Multi-Atom Entangled W States via Light-Matter Interface Based Fusion Mechanism. Sci. Rep. 2015, 5, 16245. [Google Scholar] [CrossRef]
- Zang, X.P.; Yang, M.; Ozaydin, F.; Song, W.; Cao, Z.L. Deterministic Generation of Large Scale Atomic W States. Opt. Exp. 2015, 24, 12293. [Google Scholar] [CrossRef]
- Shen, L.T.; Shi, Z.C.; Wu, H.Z.; Yang, Z.B. Dynamics of Entanglement in Jaynes–Cummings Nodes with Nonidentical Qubit-Field Coupling Strengths. Entropy 2017, 19, 331. [Google Scholar] [CrossRef]
- Shen, L.T.; Chen, R.X.; Wu, H.Z.; Yang, Z.B. Distributed manipulation of two-qubit entanglement with coupled continuous variables. JOSA B 2015, 32, 297–302. [Google Scholar] [CrossRef]
- Chan, M.L.; Tiranov, A.; Appel, M.H.; Wang, Y.; Midolo, L.; Scholz, S.; Wieck, A.D.; Ludwig, A.; Sørensen, A.S.; Lodahl, P. On-chip spin-photon entanglement based on single-photon scattering. arXiv 2022, arXiv:2205.12844. [Google Scholar] [CrossRef]
- Ozaydin, F.; Yesilyurt, C.; Bugu, S.; Koashi, M. Deterministic preparation of W states via spin-photon interactions. Phys. Rev. A 2021, 103, 052421. [Google Scholar] [CrossRef]
- Oh, E.; Lai, X.; Wen, J.; Du, S. Distributed quantum computing with photons and atomic memories. arXiv 2022, arXiv:2207.02350. [Google Scholar] [CrossRef]
- Shuai, C.; Mao-Fa, F.; Jian-Bin, L.; Xin-Wen, W.; Xiao-Juan, Z. A scheme for implementing quantum game in cavity QED. Chin. Phys. B 2009, 18, 894–897. [Google Scholar] [CrossRef]
- Walls, D.F.; Milburn, G.J. Quantum Optics; Springer: Berlin/Heidelberg, Germany; New York, NY, USA, 1995; p. xii, 351p. [Google Scholar]
- Hu, C.Y.; Young, A.; O’Brien, J.L.; Munro, W.J.; Rarity, J.G. Giant optical Faraday rotation induced by a single-electron spin in a quantum dot: Applications to entangling remote spins via a single photon. Phys. Rev. B 2008, 78, 085307. [Google Scholar] [CrossRef]
- Hu, C.Y.; Munro, W.J.; Rarity, J.G. Deterministic photon entangler using a charged quantum dot inside a microcavity. Phys. Rev. B 2008, 78, 125318. [Google Scholar] [CrossRef]
- Bartkowiak, M.; Miranowicz, A. Linear-optical implementations of the iSWAP and controlled NOT gates based on conventional detectors. J. Opt. Soc. Am. B 2010, 27, 2369–2377. [Google Scholar] [CrossRef]
- Nemoto, K.; Trupke, M.; Devitt, S.J.; Stephens, A.M.; Scharfenberger, B.; Buczak, K.; Nöbauer, T.; Everitt, M.S.; Schmiedmayer, J.; Munro, W.J. Photonic Architecture for Scalable Quantum Information Processing in Diamond. Phys. Rev. X 2014, 4, 031022. [Google Scholar] [CrossRef]
- Bodey, J.; Stockill, R.; Denning, E.; Gangloff, D.; Éthier-Majcher, G.; Jackson, D.; Clarke, E.; Hugues, M.; Gall, C.L.; Atatüre, M. Optical spin locking of a solid-state qubit. NPJ Quantum Inf. 2019, 5, 95. [Google Scholar] [CrossRef]
- Rong, X.; Geng, J.; Shi, F.; Liu, Y.; Xu, K.; Ma, W.; Kong, F.; Jiang, Z.; Wu, Y.; Du, J. Experimental fault-tolerant universal quantum gates with solid-state spins under ambient conditions. Nat. Commun. 2015, 6, 8748. [Google Scholar] [CrossRef]



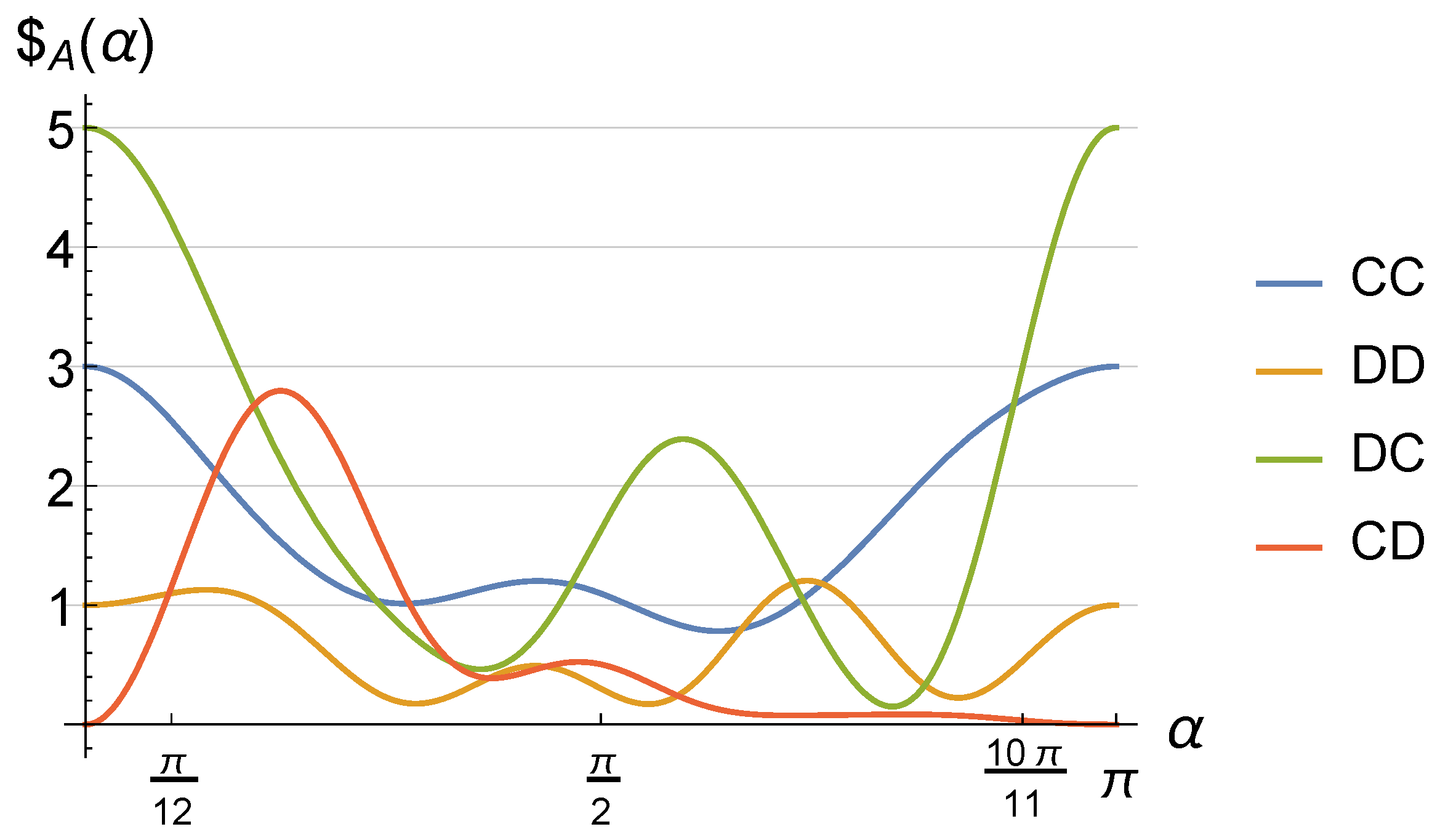
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Altintas, A.A.; Ozaydin, F.; Bayindir, C.; Bayrakci, V. Prisoners’ Dilemma in a Spatially Separated System Based on Spin–Photon Interactions. Photonics 2022, 9, 617. https://doi.org/10.3390/photonics9090617
Altintas AA, Ozaydin F, Bayindir C, Bayrakci V. Prisoners’ Dilemma in a Spatially Separated System Based on Spin–Photon Interactions. Photonics. 2022; 9(9):617. https://doi.org/10.3390/photonics9090617
Chicago/Turabian StyleAltintas, Azmi Ali, Fatih Ozaydin, Cihan Bayindir, and Veysel Bayrakci. 2022. "Prisoners’ Dilemma in a Spatially Separated System Based on Spin–Photon Interactions" Photonics 9, no. 9: 617. https://doi.org/10.3390/photonics9090617
APA StyleAltintas, A. A., Ozaydin, F., Bayindir, C., & Bayrakci, V. (2022). Prisoners’ Dilemma in a Spatially Separated System Based on Spin–Photon Interactions. Photonics, 9(9), 617. https://doi.org/10.3390/photonics9090617






