Measuring of Transverse Energy Flows in a Focus of an Aluminum Lens
Abstract
:1. Introduction
2. Materials and Methods
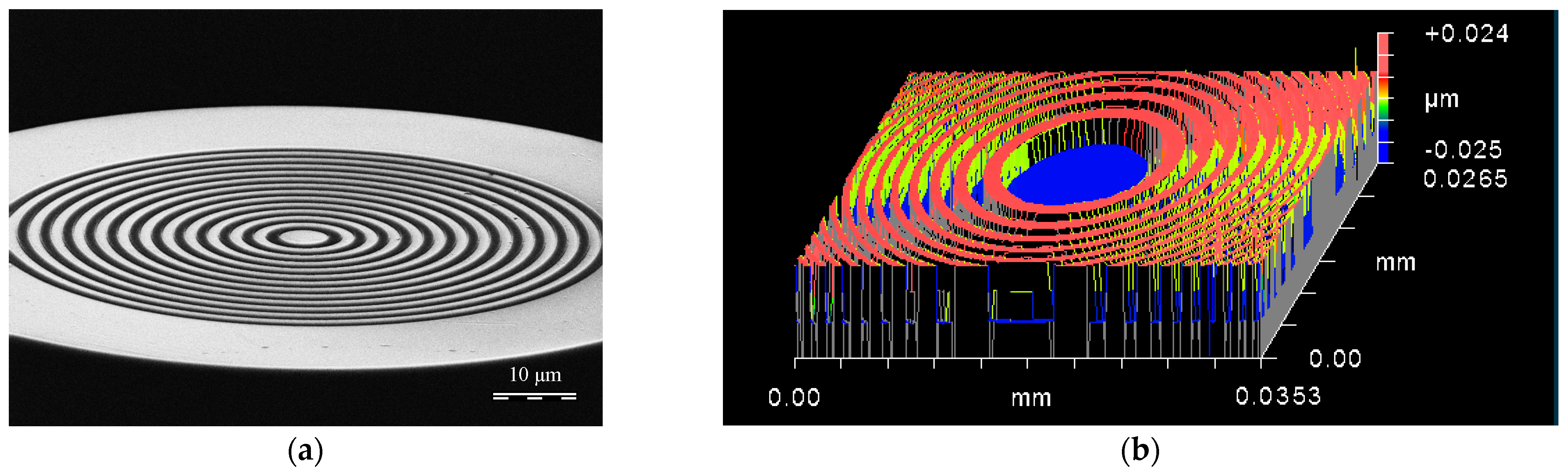
Structure and Manufacturing of the Lens
3. Results and Discussion
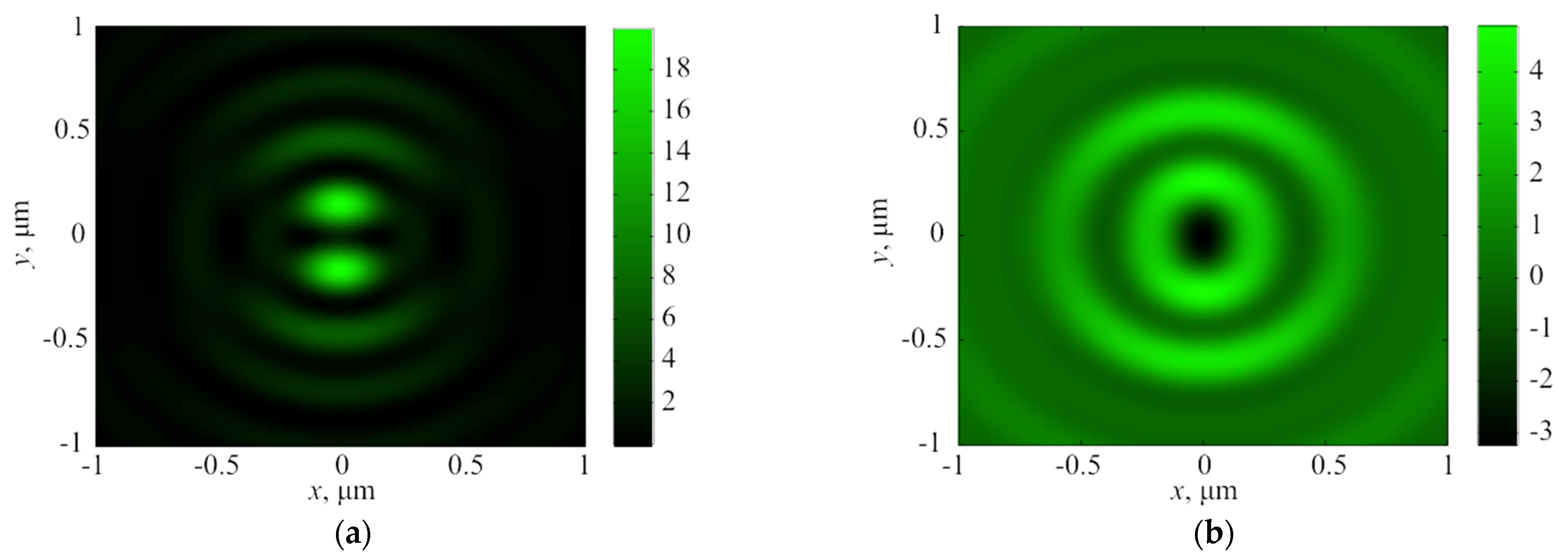
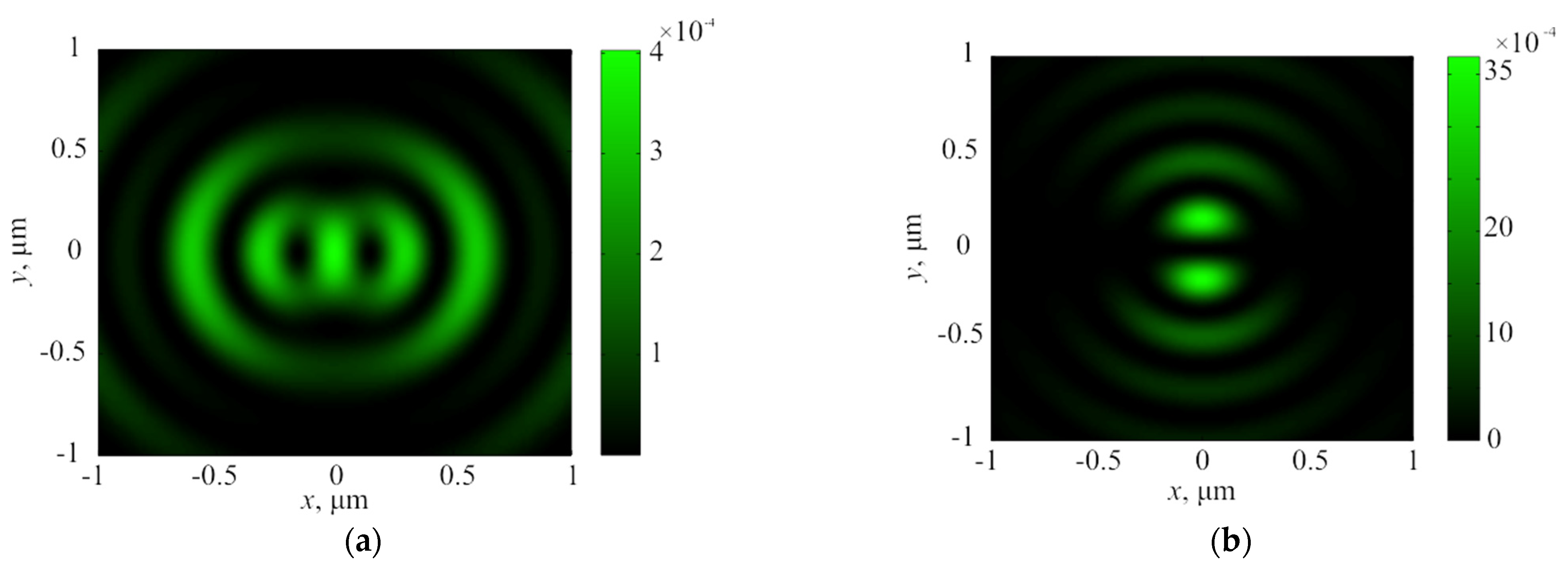
3.1. The Results of Modeling by the FDTD Method
3.2. The Numerical Simulation by the Richards–Wolf Formulae
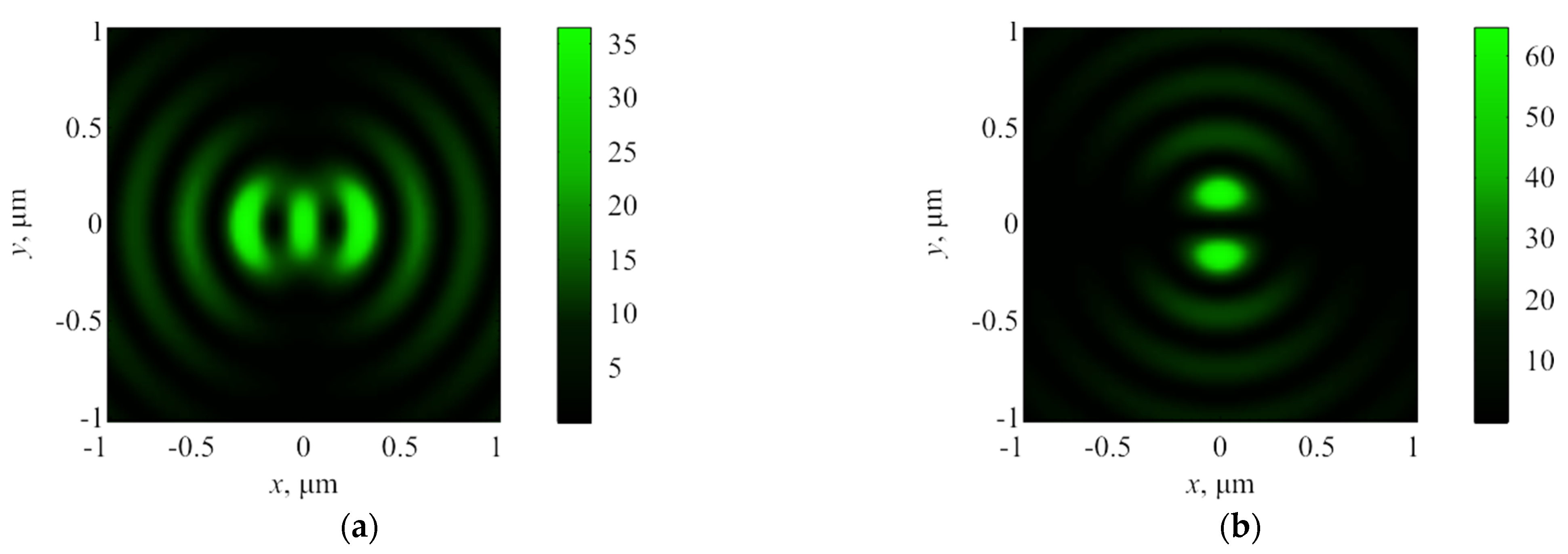
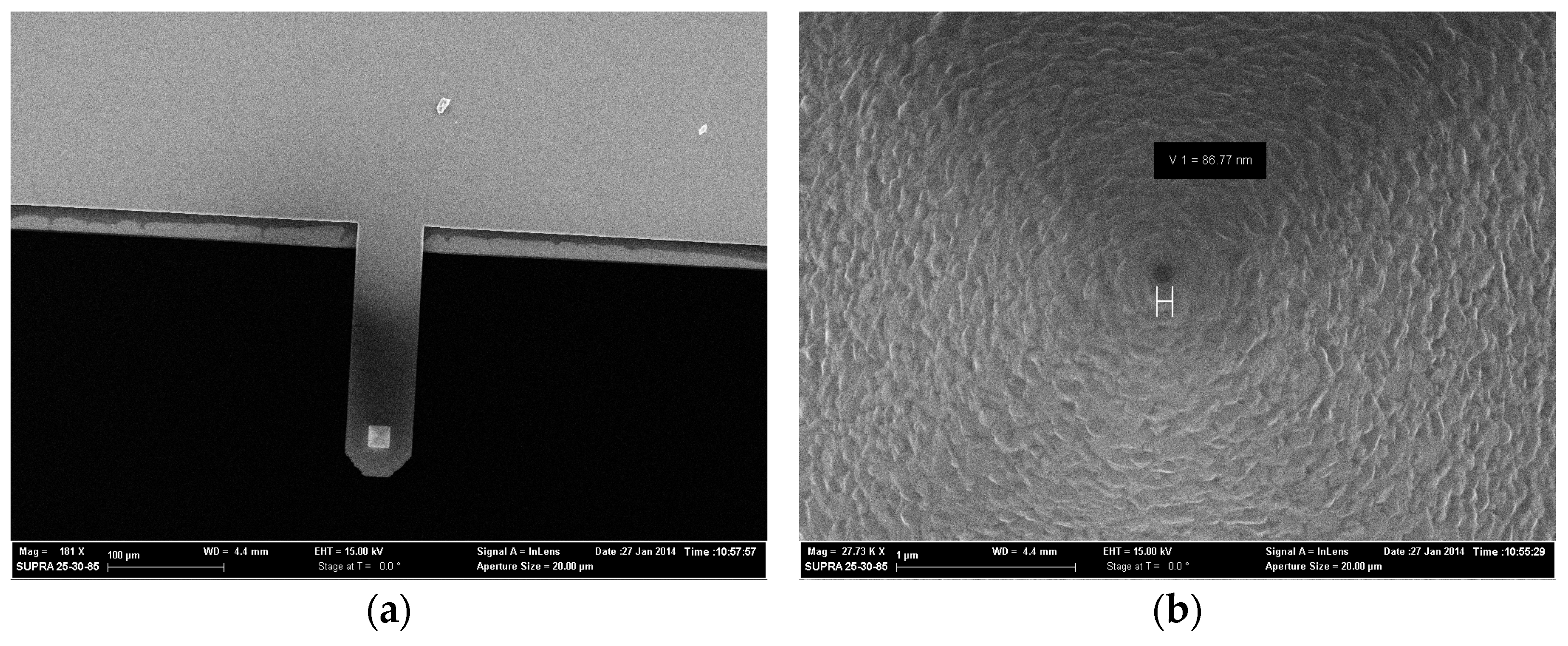
3.3. Experiment
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Abed, J.; Alexander, F.; Taha, I.; Rajput, N.; Aubry, C.; Jouiad, M. Investigation of Broadband Surface Plasmon Resonance of Dewetted Au Structures on TiO2 by Aperture-Probe SNOM and FDTD Simulations. Plasmonics 2019, 14, 205–218. [Google Scholar] [CrossRef]
- Bazylewski, P.; Ezugwu, S.; Fanchini, G. Review of Three-Dimensional Scanning Near-Field Optical Microscopy (3D-SNOM) and Its Applications in Nanoscale Light Management. Appl. Sci. 2017, 7, 973. [Google Scholar] [CrossRef] [Green Version]
- Heydarian, H.; Shahmansouri, A.; Yazdanfar, P.; Rashidian, B. Dual-color plasmonic probes for improvement of scanning near-field optical microscopy. J. Opt. Soc. Am. B 2018, 35, 627–635. [Google Scholar] [CrossRef]
- Minin, I.V.; Minin, O.V.; Glinskiy, I.A.; Khabibullin, R.A.; Malureanu, R.; Lavrinenko, A.V.; Yakubovsky, D.I.; Arsenin, A.V.; Volkov, V.S.; Ponomarev, D.S. Plasmonic nanojet: An experimental demonstration: Publisher’s note. Opt. Lett. 2020, 45, 3418. [Google Scholar] [CrossRef] [PubMed]
- Pohl, D.W. Scanning Near-field Optical Microscopy (SNOM). Adv. Opt. Electron. Microsc. 1991, 12, 243–312. [Google Scholar] [CrossRef]
- Kazantsev, D.V.; Kuznetsov, E.V.; Timofeev, S.V.; Shelaev, A.V.; Kazantseva, E.A. Apertureless near-field optical microscopy. Phys. Uspekhi 2017, 60, 259–275. [Google Scholar] [CrossRef]
- Huber, C.; Prior, Y.; Kautek, W. Laser-induced cantilever behaviour in apertureless scanning near-field optical microscopes. Meas. Sci. Technol. 2014, 25, 075604. [Google Scholar] [CrossRef]
- Sun, W.X.; Shen, Z.X.; Cheong, F.C.; Yu, G.Y.; Lim, K.Y.; Lin, J.Y. Preparation of cantilevered W tips for atomic force microscopy and apertureless near-field scanning optical microscopy. Rev. Sci. Instrum. 2002, 73, 2942. [Google Scholar] [CrossRef]
- Kassing, R.; Rangelow, I.; Oesterschulze, E.; Stuke, M. Sensors for scanning probe microscopy. Appl. Phys. A 2003, 76, 907–911. [Google Scholar] [CrossRef]
- Ingham, J.; Pilling, M.J.; Craig, T.; Siggel-King, M.R.F.; Smith, C.I.; Gardner, P.; Varro, A.; Pritchard, D.M.; Barrett, S.D.; Martin, D.S.; et al. An evaluation of the application of the aperture infrared SNOM technique to biomedical imaging. Biomed. Phys. Eng. Express 2018, 4, 025011. [Google Scholar] [CrossRef]
- Frolov, A.Y.; Van de Vondel, J.; Panov, V.I.; Van Dorpe, P.; Fedyanin, A.A.; Moshchalkov, V.V.; Verellen, N. Probing higher order optical modes in all-dielectric nanodisk, -square, and -triangle by aperture type scanning near-field optical microscopy. Nanophotonics 2022, 11, 543–557. [Google Scholar] [CrossRef]
- Vollkopf, A.; Rudow, O.; Leinhos, T.; Mihalcea, C.; Oesterschulze, E. Modified fabrication process for aperture probe cantilevers. J. Microsc. 1999, 194, 344–348. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Werner, S.; Rudow, O.; Mihalcea, C.; Oesterschulze, E. Cantilever probes with aperture tips for polarization-sensitive scanning near-field optical microscopy. Appl. Phys. A Mater. Sci. Process. 1998, 66, S367–S370. [Google Scholar] [CrossRef]
- Dvořák, P.; Édes, Z.; Kvapil, M.; Šamořil, T.; Ligmajer, F.; Hrtoň, M.; Kalousek, R.; Křápek, V.; Dub, P.; Spousta, J.; et al. Imaging of near-field interference patterns by aperture-type SNOM—influence of illumination wavelength and polarization state. Opt. Express 2017, 25, 16560–16573. [Google Scholar] [CrossRef] [PubMed]
- González Mora, C.A.; Hartelt, M.; Bayer, D.; Aeschlimann, M.; Ilin, E.A.; Oesterschulze, E. Microsphere-based cantilevers for polarization-resolved and femtosecond SNOM. Appl. Phys. B 2016, 122, 86. [Google Scholar] [CrossRef]
- Atie, E.M.; Xie, Z.; El Eter, A.; Salut, R.; Nedeljkovic, D.; Tannous, T.; Baida, F.I.; Grosjean, T. Remote optical sensing on the nanometer scale with a bowtie aperture nano-antenna on a fiber tip of scanning near-field optical microscopy. Appl. Phys. Lett. 2015, 106, 151104. [Google Scholar] [CrossRef]
- El Eter, A.; Hameed, N.M.; Baida, F.I.; Salut, R.; Filiatre, C.; Nedeljkovic, D.; Atie, E.; Bole, S.; Grosjean, T. Fiber-integrated optical nano-tweezer based on a bowtie-aperture nano-antenna at the apex of a SNOM tip. Opt. Express 2014, 22, 10072–10080. [Google Scholar] [CrossRef]
- Murphy-DuBay, N.; Wang, L.; Kinzel, E.C.; Uppuluri, S.M.V.; Xu, X. Nanopatterning using NSOM probes integrated with high transmission nanoscale bowtie aperture. Opt. Express 2008, 16, 2584–2589. [Google Scholar] [CrossRef] [Green Version]
- Biagioni, P.; Polli, D.; Labardi, M.; Pucci, A.; Ruggeri, G.; Cerullo, G.; Finazzi, M.; Duò, L. Unexpected polarization behavior at the aperture of hollow-pyramid near-field probes. Appl. Phys. Lett. 2005, 87, 223112. [Google Scholar] [CrossRef]
- Biagioni, P.; Coduri, M.; Polli, D.; Virgili, T.; Labardi, M.; Cerullo, G.; Finazzi, M.; Duò, L. Near-field vs. far-field polarization properties of hollow pyramid SNOM tips. Phys. Status Solidi 2005, 2, 4078–4082. [Google Scholar] [CrossRef]
- Shershulin, V.A.; Samoylenko, S.R.; Shenderova, O.A.; Konov, V.I.; Vlasov, I.I. Use of scanning near-field optical microscope with an aperture probe for detection of luminescent nanodiamonds. Laser Phys. 2017, 27, 025201. [Google Scholar] [CrossRef] [Green Version]
- Kotlyar, V.V.; Stafeev, S.S.; Liu, Y.; O’Faolain, L.; Kovalev, A.A. Analysis of the shape of a subwavelength focal spot for the linearly polarized light. Appl. Opt. 2013, 52, 330–339. [Google Scholar] [CrossRef] [PubMed]
- Mikhailova, T.V.; Vysokikh, Y.E.; Krasnoborodko, S.Y.; Kolomiytsev, A.S.; Fedotov, A.A. Light polarization and intensity behaviour in aperture cantilevers with carbon tip created by focused ion beam. IOP Conf. Ser. Mater. Sci. Eng. 2019, 699, 012030. [Google Scholar] [CrossRef]
- Vysokikh, Y.E.; Mikhailova, T.V.; Krasnoborodko, S.Y.; Kolomiytsev, A.S.; Ilin, O.I.; Shaposhnikov, A.N.; Berzhansky, V.N.; Bulatov, M.F.; Churikov, D.V.; Shevyakov, V.I. Carbon tip aperture cantilevers: Fabrication & features in near-field magneto-optical imaging. J. Magn. Magn. Mater. 2021, 529, 167837. [Google Scholar] [CrossRef]
- Achmari, P.; Siddiquee, A.M.; Si, G.; Lin, J.; Abbey, B.; Kou, S. Investigating the probe-tip influence on imaging using scanning near-field optical microscopy. OSA Contin. 2021, 4, 1143–1154. [Google Scholar] [CrossRef]
- Stafeev, S.S.; Kotlyar, V.V.; Nalimov, A.G.; Kozlova, E.S. The Non-Vortex Inverse Propagation of Energy in a Tightly Focused High-Order Cylindrical Vector Beam. IEEE Photonics J. 2019, 11, 4500810. [Google Scholar] [CrossRef]
- Kotlyar, V.V.; Stafeev, S.S.; Nalimov, A.G. Energy backflow in the focus of a light beam with phase or polarization singularity. Phys. Rev. A 2019, 99, 033840. [Google Scholar] [CrossRef]
- Huang, P.; Zhou, Z.; Ren, H. Fresnel zone plate fabricated using a polyvinyl chloride gel. Opt. Eng. 2018, 57, 117101. [Google Scholar] [CrossRef]
- Liu, Q.; Liu, T.; Yang, S.; Wang, T.; Wang, Y. Validation of vectorial theories for the focusing of high numerical aperture Fresnel zone plates. Opt. Commun. 2018, 429, 119–126. [Google Scholar] [CrossRef]
- Minerbi, E.; Keren-Zur, S.; Ellenbogen, T. Nonlinear Metasurface Fresnel Zone Plates for Terahertz Generation and Manipulation. Nano Lett. 2019, 19, 6072–6077. [Google Scholar] [CrossRef] [Green Version]
- Geints, Y.E.; Minin, O.V.; Panina, E.K.; Minin, I.V. Controlling near-field focusing of a mesoscale binary phase plate in an optical radiation field with circular polarization. Comput. Opt. 2021, 45, 512–519. [Google Scholar] [CrossRef]
- Yoon, G.; Jang, J.; Mun, J.; Nam, K.T.; Rho, J. Metasurface zone plate for light manipulation in vectorial regime. Commun. Phys. 2019, 2, 156. [Google Scholar] [CrossRef] [Green Version]
- Kotlyar, V.V.; Stafeev, S.S.; Nalimov, A.G.; Kotlyar, M.V.; O’Faolain, L.; Kozlova, E.S. Tight focusing of laser light using a chromium Fresnel zone plate. Opt. Express 2017, 25, 19662–19671. [Google Scholar] [CrossRef]
- Mote, R.G.; Minin, O.V.; Minin, I.V. Focusing behavior of 2-dimensional plasmonic conical zone plate. Opt. Quantum Electron. 2017, 49, 271. [Google Scholar] [CrossRef]
- Kozlova, E.S.; Kotlyar, V.V. Simulation of tight focusing of laser light by gold zone plate. In Proceedings of the 2018 20th International Conference on Transparent Optical Networks (ICTON), Bucharest, Romania, 1–5 July 2018; pp. 1–4. [Google Scholar] [CrossRef]
- Kim, J.; Kim, H.; Lee, G.-Y.; Kim, J.; Lee, B.; Jeong, Y. Numerical and Experimental Study on Multi-Focal Metallic Fresnel Zone Plates Designed by the Phase Selection Rule via Virtual Point Sources. Appl. Sci. 2018, 8, 449. [Google Scholar] [CrossRef] [Green Version]
- Yang, J.; Zhong, Y.; Zheng, C.; Ding, S.; Zang, H.; Liang, E.; Cao, L. Dual-type fractal spiral zone plate for generating sequence of square optical vortices. J. Opt. Soc. Am. A 2019, 36, 893. [Google Scholar] [CrossRef]
- Zang, H.; Ding, S.; Wei, L.; Wang, C.; Fan, Q.; Cao, L. Fractal spiral zone plate with high-order harmonics suppression. Appl. Opt. 2019, 58, 8680–8686. [Google Scholar] [CrossRef]
- Kozlova, E.S. Modeling of the optical vortex generation using a silver spiral zone plate. Comput. Opt. 2018, 42, 977–984. [Google Scholar] [CrossRef]
- Cheng, S.; Xia, T.; Liu, M.; Zheng, C.; Zang, H.; Tao, S. Composite Spiral Zone Plate. IEEE Photonics J. 2019, 11, 1–11. [Google Scholar] [CrossRef]
- Kozlova, E.S. Investigation of the influence of amplitude spiral zone plate parameters on produced energy backflow. Comput. Opt. 2019, 43, 1093–1097. [Google Scholar] [CrossRef]
- Kim, H.; Jeong, Y. Theoretical and numerical study of cylindrical-vector-mode radiation characteristics in periodic metallic annular slits and their applications. Curr. Opt. Photonics 2018, 2, 482–487. [Google Scholar]
- Luo, X.-G.; Pu, M.-B.; Li, X.; Ma, X.-L. Broadband spin Hall effect of light in single nanoapertures. Light Sci. Appl. 2017, 6, e16276. [Google Scholar] [CrossRef] [PubMed]
- Kotlyar, V.V.; Nalimov, A.G. Sharp focusing of vector optical vortices using a metalens. J. Opt. 2018, 20, 075101. [Google Scholar] [CrossRef]
- Zhang, Y.; Liu, H.; Cheng, H.; Tian, J.; Chen, S. Multidimensional manipulation of wave fields based on artificial microstructures. Opto-Electron. Adv. 2020, 3, 200002. [Google Scholar] [CrossRef]
- Luo, X.; Pu, M.; Zhang, F.; Xu, M.; Guo, Y.; Li, L.; Ma, X. Vector optical field manipulation via structural functional materials: Tutorial. J. Appl. Phys. 2022, 131, 181101. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, Y.; Zhu, Y. Probability distribution of the orbital angular momentum mode of the ultrashort Laguerre-Gaussian pulsed beam propagation in oceanic turbulence. Results Phys. 2018, 11, 698–705. [Google Scholar] [CrossRef]
- Zukersteina, M.; Hrabovsky, J.; Sladek, J.; Mirza, I.; Levy, Y.; Bulgakova, N.M. Formation of tubular structures and microneedles on silicon surface by doughnut-shaped ultrashort laser pulses. Appl. Surf. Sci. 2022, 592, 153228. [Google Scholar] [CrossRef]
- Richards, B.; Wolf, E. Electromagnetic diffraction in optical systems. II. Structure of the image field in an aplanatic system. Proc. R. Soc. A 1959, 253, 358–379. [Google Scholar] [CrossRef]
- Cui, T.; Zhang, M.; Zhao, Y.; Yang, Y.; Bai, B.; Sun, H.-B. Near-field nonlinear imaging of an anapole mode beyond diffraction limit. Opt. Lett. 2021, 46, 2095–2098. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kozlova, E.; Stafeev, S.; Fomchenkov, S.; Podlipnov, V.; Savelyeva, A.; Kotlyar, V. Measuring of Transverse Energy Flows in a Focus of an Aluminum Lens. Photonics 2022, 9, 592. https://doi.org/10.3390/photonics9080592
Kozlova E, Stafeev S, Fomchenkov S, Podlipnov V, Savelyeva A, Kotlyar V. Measuring of Transverse Energy Flows in a Focus of an Aluminum Lens. Photonics. 2022; 9(8):592. https://doi.org/10.3390/photonics9080592
Chicago/Turabian StyleKozlova, Elena, Sergey Stafeev, Sergey Fomchenkov, Vladimir Podlipnov, Alexandra Savelyeva, and Victor Kotlyar. 2022. "Measuring of Transverse Energy Flows in a Focus of an Aluminum Lens" Photonics 9, no. 8: 592. https://doi.org/10.3390/photonics9080592
APA StyleKozlova, E., Stafeev, S., Fomchenkov, S., Podlipnov, V., Savelyeva, A., & Kotlyar, V. (2022). Measuring of Transverse Energy Flows in a Focus of an Aluminum Lens. Photonics, 9(8), 592. https://doi.org/10.3390/photonics9080592





