Security of Optical Beam Splitter in Quantum Key Distribution
Abstract
:1. Introduction
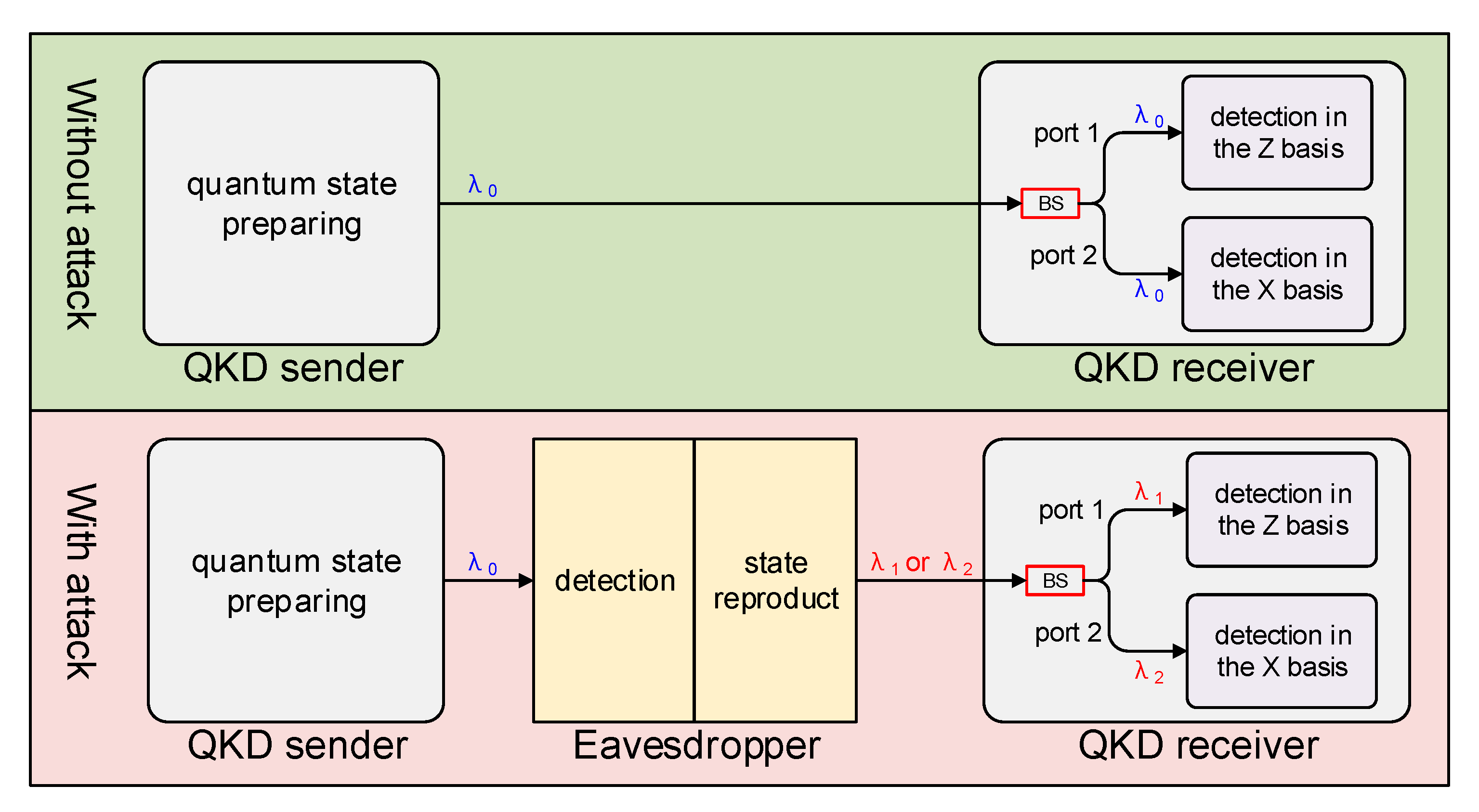
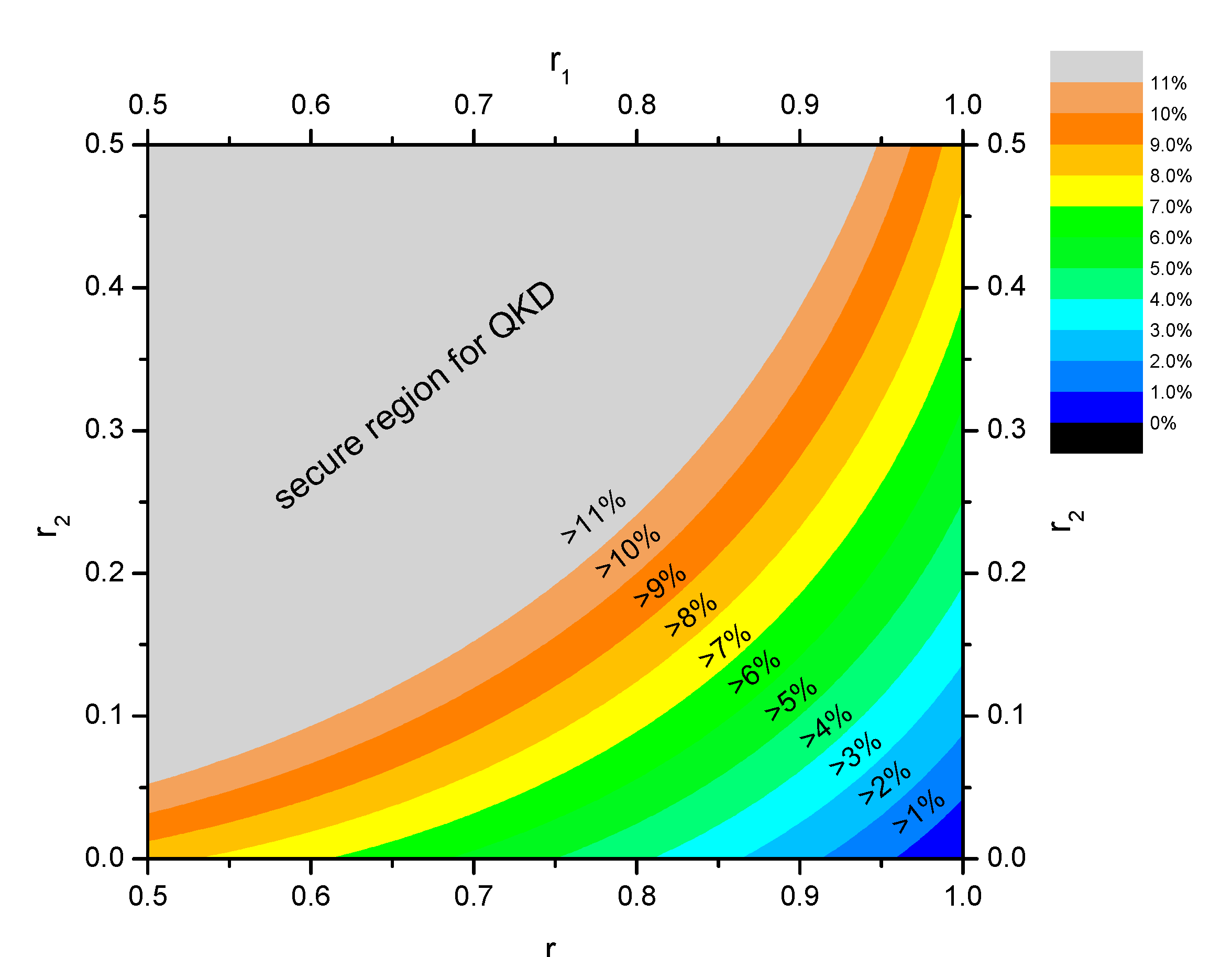
2. Wavelength-Dependent Attack of Beam Splitters
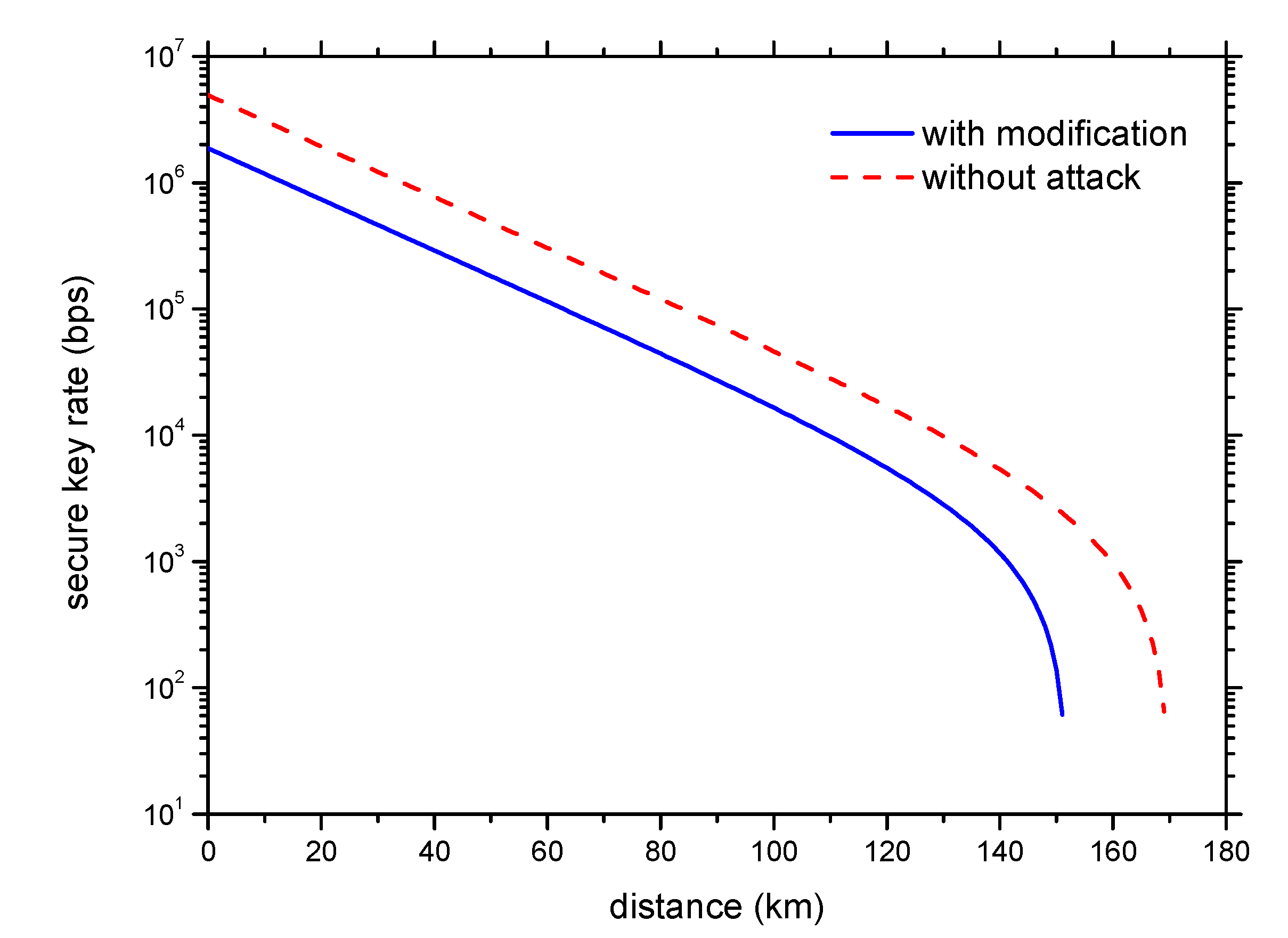
3. Countermeasure of Error Rate Alerting
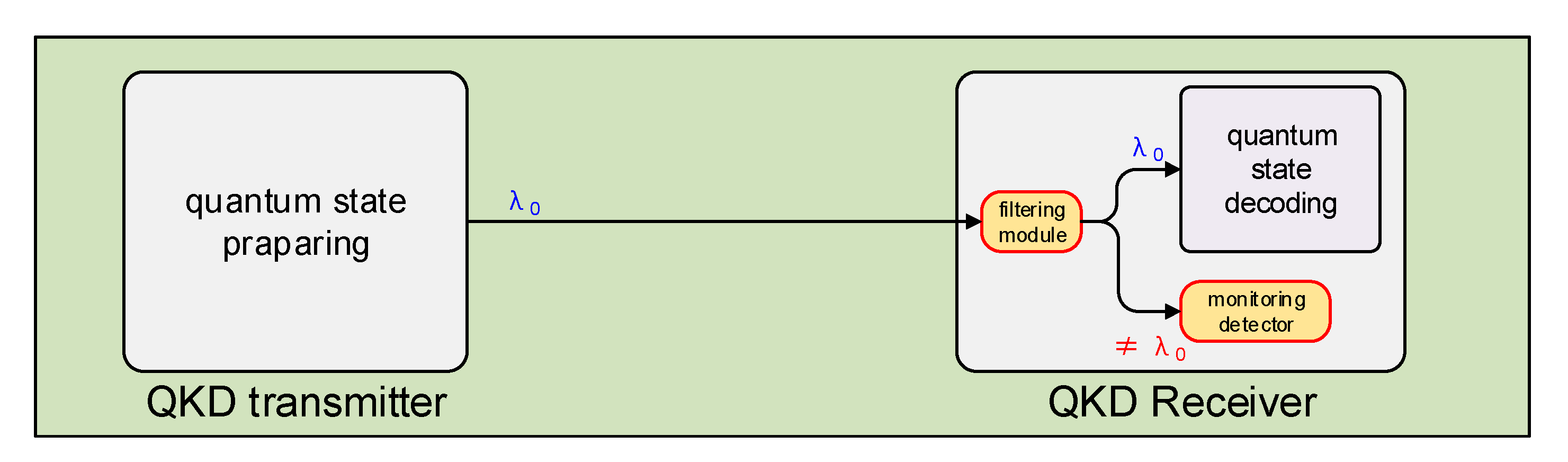
4. Countermeasure of Monitoring
5. Discussions
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Wehner, S.; Elkouss, D.; Hanson, R. Quantum internet: A vision for the road ahead. Science 2018, 362, eaam9288. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wu, Y.; Bao, W.-S.; Cao, S.; Chen, F.; Chen, M.-C.; Chen, X.; Chung, T.-H.; Deng, H.; Du, Y.; Fan, D.; et al. Strong Quantum Computational Advantage Using a Superconducting Quantum Processor. Phys. Rev. Lett. 2021, 127, 180501. [Google Scholar] [CrossRef] [PubMed]
- Gisin, N.; Ribordy, G.; Tittel, W.; Zbinden, H. Quantum cryptography. Rev. Mod. Phys. 2002, 74, 145–195. [Google Scholar] [CrossRef] [Green Version]
- Scarani, V.; Bechmann-Pasquinucci, H.; Cerf, N.; Dusek, M.; Lutkenhaus, M.; Peev, M. The security of practical quantum key distribution. Rev. Mod. Phys. 2009, 81, 1301–1350. [Google Scholar] [CrossRef] [Green Version]
- Bennett, C.H.; Brassard, G. Quantum Cryptography: Public Key Distribution and Coin Tossing. In Proceedings of the IEEE International Conference on Computers, Systems, and Signal Processing, Bangalore, India, 10–12 December 1984; pp. 175–179. [Google Scholar]
- Gottesman, D.; Lo, H.-K.; Lutkenhaus, N.; Preskill, J. Security of quantum key distribution with imperfect devices. Quant. Inf. Comput. 2004, 4, 325–360. [Google Scholar]
- Hwang, W.Y. Quantum Key Distribution with High Loss: Toward Global Secure Communication. Phys. Rev. Lett. 2003, 91, 057901. [Google Scholar] [CrossRef] [Green Version]
- Wang, X.-B. Beating the Photon-Number-Splitting Attack in Practical Quantum Cryptography. Phys. Rev. Lett. 2005, 94, 230503. [Google Scholar] [CrossRef] [Green Version]
- Lo, H.-K.; Ma, X.; Chen, K. Decoy State Quantum Key Distribution. Phys. Rev. Lett. 2005, 94, 230504. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Q.; Xu, F.; Chen, Y.-A.; Peng, C.-Z.; Pan, J.-W. Large scale quantum key distribution: Challenges and solutions. Opt. Express 2018, 26, 24260–24273. [Google Scholar] [CrossRef] [Green Version]
- Peng, C.-Z.; Zhang, J.; Yang, D.; Gao, W.-B.; Ma, H.-X.; Yin, H.; Zeng, H.-P.; Yang, T.; Wang, X.-B.; Pan, J.-W. Experimental Long-Distance Decoy-State Quantum Key Distribution Based on Polarization Encoding. Phys. Rev. Lett. 2007, 98, 010505. [Google Scholar] [CrossRef] [Green Version]
- Chen, T.-Y.; Wang, J.; Liang, H.; Liu, W.-Y.; Liu, Y.; Jiang, X.; Wang, Y.; Wan, X.; Cai, W.-Q.; Lei, J.; et al. Metropolitan all-pass and inter-city quantum communication network. Opt. Express 2010, 18, 27217–27225. [Google Scholar] [CrossRef]
- Zhou, F.; Yong, H.-L.; Li, D.-D.; Yin, J.; Ren, J.-G.; Peng, C.-Z. Study on quantum key distribution between different media. Acta Phys. Sin. 2014, 63, 140303. [Google Scholar] [CrossRef]
- Tang, Y.-L.; Yin, H.-L.; Zhao, Q.; Liu, H.; Sun, X.-X.; Huang, M.-Q.; Zhang, W.-J.; Chen, S.-J.; Zhang, L.; You, L.-X.; et al. Measurement-Device-Independent Quantum Key Distribution over Untrustful Metropolitan Network. Phys. Rev. X 2016, 6, 011024. [Google Scholar] [CrossRef] [Green Version]
- Liao, S.-K.; Yong, H.-L.; Liu, C.; Shentu, G.-L.; Li, D.-D.; Lin, J.; Dai, H.; Zhao, S.-Q.; Li, B.; Guan, J.-Y.; et al. Long-distance free-space quantum key distribution in daylight towards inter-satellite communication. Nat. Photonics 2017, 11, 509–513. [Google Scholar] [CrossRef]
- Boaron, A.; Boso, G.; Rusca, D.; Vullliez, C.; Autebert, C.; Caloz, M.; Perrenoud, M.; Gras, G.; Bussieres, F.; Li, M.-J.; et al. Secure Quantum Key Distribution over 421 km of Optical Fiber. Phys. Rev. Lett. 2018, 121, 190502. [Google Scholar] [CrossRef] [Green Version]
- Li, D.-D.; Gao, S.; Li, G.-C.; Xue, L.; Wang, L.-W.; Lu, C.-B.; Xiang, Y.; Zhao, Z.-Y.; Yan, L.-C.; Chen, Z.-Y.; et al. Field implementation of long-distance quantum key distribution over aerial fiber with fast polarization feedback. Opt. Express 2018, 26, 22793–22800. [Google Scholar] [CrossRef]
- Li, D.-D.; Shen, Q.; Chen, W.; Li, Y.; Han, X.; Yang, K.-X.; Xu, Y.; Lin, J.; Wang, C.-Z.; Yong, H.-L.; et al. Proof-of-principle demonstration of quantum key distribution with seawater channel: Towards space-to-underwater quantum communication. Opt. Commun. 2019, 452, 220–226. [Google Scholar] [CrossRef]
- Jiang, X.-L.; Deng, X.-Q.; Wang, Y.; Lu, Y.-F.; Li, J.-J.; Zhou, C.; Bao, W.-S. Weak Randomness Analysis of Measurement-Device-Independent Quantum Key Distribution with Finite Resources. Photonics 2022, 9, 356. [Google Scholar] [CrossRef]
- Zhang, Q.; Liu, Y.; Yu, X.; Zhao, Y.; Zhang, J. Topology-Abstraction-Based Protection Scheme in Quantum Key Distribution Networks with Partially Trusted Relays. Photonics 2022, 9, 239. [Google Scholar] [CrossRef]
- Lu, Y.-F.; Wang, Y.; Jiang, M.-S.; Zhang, X.-X.; Liu, F.; Li, H.-W.; Zhou, C.; Tang, S.-B.; Wang, J.-Y.; Bao, W.-S. Sending or Not-Sending Twin-Field Quantum Key Distribution with Flawed and Leaky Sources. Entropy 2021, 23, 1103. [Google Scholar] [CrossRef]
- Lu, Y.-F.; Jiang, M.-S.; Wang, Y.; Zhang, X.-X.; Liu, F.; Zhou, C.; Li, H.-W.; Tang, S.-B.; Wang, J.-Y.; Bao, W.-S. Practical Analysis of Sending or Not-Sending Twin-Field Quantum Key Distribution with Frequency Side Channels. Appl. Sci. 2021, 11, 9560. [Google Scholar] [CrossRef]
- Chen, Y.-A.; Zhang, Q.; Chen, T.-Y.; Cai, W.-Q.; Liao, S.-K.; Zhang, J.; Chen, K.; Yin, J.; Ren, J.-G.; Chen, Z.; et al. An integrated space-to-ground quantum communication network over 4600 kilometers. Nature 2021, 589, 214–219. [Google Scholar] [CrossRef]
- Chen, T.-Y.; Jiang, X.; Tang, S.-B.; Zhou, L.; Yuan, X.; Zhou, H.; Wang, J.; Liu, Y.; Chen, L.-K.; Liu, W.-Y.; et al. Implementation of a 46-node quantum metropolitan area network. NPJ Quant. Inf. 2021, 7, 134. [Google Scholar] [CrossRef]
- Politi, A.; Matthews, J.C.F.; Thompson, M.G.; O’Brien, J.L. Integrated Quantum Photonics. IEEE J. Selected. Top. Quantum Electron. 2009, 20, 1077. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Z.; Felipe, D.; Katopodis, V.; Groumsa, P.; Kouloumentas, C.; Avramopoulos, H.; Dupuy, J.-Y.; Konczykowska, A.; Dede, A.; Beretta, A.; et al. Hybrid photonic integration on a Polymer Platform. Photonics 2015, 2, 1005–1026. [Google Scholar] [CrossRef]
- Wang, J.-W.; Sciarrino, F.; Laing, A.; Thompson, M.G. Integrated photonic quantum technologies. Nat. Photonics 2020, 14, 273–284. [Google Scholar] [CrossRef]
- Pelucchi, E.; Fagas, G.; Aharonovich, I.; Englund, D.; Figueroa, E.; Gong, Q.; Hannes, H.; Liu, J.; Lu, C.-Y.; Matsuda, N.; et al. The potential and global outlook of integrated photonics for quantum technologies. Nat. Rev. Phys. 2022, 4, 194–208. [Google Scholar] [CrossRef]
- Mazeas, F.; Traetta, M.; Bentivegna, M.; Kaiser, F.; Akatas, D.; Zhang, W.; Ramos, C.A.; Ngah, L.A.; Lunchi, T.; Picholle, E.; et al. High-quality photonic entanglement for wavelength-multiplexed quantum communication based on a silicon chip. Opt. Express 2016, 24, 28731–28738. [Google Scholar] [CrossRef]
- Brunetti, G.; Sasanelli, N.; Armenise, M.N.; Ciminelli, C. High performance and tunable optical pump-rejection filter for quantum photonic systems. Opt. Laser Technol. 2021, 139, 106987. [Google Scholar] [CrossRef]
- Akca, B.I.; Povazay, B.; Alex, A.; Worhoff, K.; Ridder, R.M.; Drexler, W.; Pollnau, M. Miniature spectrometer and beam splitter for an optical coherence tomography on a silicon chip. Opt. Express 2013, 21, 16648–16656. [Google Scholar] [CrossRef] [Green Version]
- Sibson, P.; Erven, C.; Godfrey, M.; Miki, S.; Yamashita, T.; Fujiwara, M.; Sasaki, M.; Terai, H.; Tanner, M.G.; Natarajan, C.M.; et al. Chip-based quantum key distribution. Nat. Commun. 2017, 8, 13984. [Google Scholar] [CrossRef] [Green Version]
- Ma, C.; Sacher, W.D.; Tang, Z.-Y.; Mikkelsen, J.C.; Yang, Y.; Xu, F.; Thiessen, T.; Lo, H.-K.; Poon, J.K.S. Silicon photonic transmitter for polarization encoded quantum key distribution. Optica 2016, 3, 1274–1278. [Google Scholar] [CrossRef]
- Wei, K.-J.; Li, W.; Tan, H.; Li, Y.; Min, H.; Zhang, W.-J.; Li, H.; You, L.-X.; Wang, Z.; Jiang, X.; et al. High-Speed Measurement-Device-Independent Quantum Key Distribution with Integrated Silicon Photonics. Phys. Rev. X 2020, 10, 031030. [Google Scholar] [CrossRef]
- Paraiso, T.K.; Roger, T.; Marangon, D.G.; Marco, I.D.; Sanzaro, M.; Woodward, R.I.; Dynes J., F.; Yuan, Z.; Shields, A.J. A photonic integrated quantum secure communication system. Nat. Photonics 2021, 15, 850–856. [Google Scholar]
- Bunandar, D.; Lentine, A.; Lee, C.; Cai, H.; Long, C.M.; Boynton, N.; Martinez, N.; DeRose, C.; Chen, C.; Grein, M.; et al. Metropolitan Quantum Key Distribution with Silicon Photonics. Phys. Rev. X 2018, 8, 021009. [Google Scholar] [CrossRef] [Green Version]
- Zhao, Y.; Fung, C.H.F.; Qi, B.; Chen, C.; Lo, H.-K. Quantum hacking: Experimental demonstration of time-shift attack against practical quantum-key-distribution systems. Phys. Rev. A 2008, 78, 042333. [Google Scholar] [CrossRef] [Green Version]
- Qi, B.; Fung, C.H.F.; Lo, H.-K.; Ma, X. Time-shift attack in practical quantum cryptosystems. Quant. Inf. Comput. 2007, 7, 73–82. [Google Scholar] [CrossRef]
- Lydersen, L.; Wiechers, C.; Wittmann, C.; Elser, D.; Skaar, J.; Makarov, V. Hacking commercial quantum cryptography systems by tailored bright illumination. Nat. Photonics 2010, 4, 686–689. [Google Scholar] [CrossRef] [Green Version]
- Xu, F.; Ma, X.; Zhang, Q.; Lo, H.-K.; Pan, J.-W. Secure quantum key distribution with realistic devices. Rev. Mod. Phys. 2020, 92, 025002. [Google Scholar] [CrossRef]
- Tang, G.-Y.; Huang, H.-M.; Liu, Y.-Q.; Wang, H. Compact Photonic Crystal Polarization Beam Splitter Based on the Self-Collimation Effect. Photonics 2021, 8, 198. [Google Scholar]
- Chang, R.-J.; Huang, C.-C. Simulation of a High-Performance Polarization Beam Splitter Assisted by Two-Dimensional Metamaterials. Nanomaterials 2022, 12, 1852. [Google Scholar] [CrossRef]
- Li, J.; He, Y.-G.; Ye, H.; Wu, T.; Liu, Y.; He, X.; Li, J.; Cheng, J. High-Efficiency, Dual-Band Beam Splitter Based on an All-Dielectric Quasi-Continuous Metasurface. Materials 2021, 14, 3184. [Google Scholar] [CrossRef]
- He, Q.; Shen, Z. Polarization-Insensitive Beam Splitter with Variable Split Angles and Ratios Based on Phase Gradient Metasurfaces. Nanomaterials 2022, 12, 113. [Google Scholar]
- Prajzler, V.; Zazvorka, J. Polymer large core optical splitter 1 × 2 Y for high-temperature operation. Opt. Quantum Electron. 2019, 51, 216. [Google Scholar]
- Li, H.-W.; Wang, S.; Huang, J.-Z.; Chen, W.; Yin, Z.-Q.; Li, F.-Y.; Zhou, Z.; Liu, D.; Zhang, Y.; Guo, G.-C.; et al. Attacking a practical quantum-key-distribution system with wavelength-dependent beam-splitter and multiwavelength sources. Phys. Rev. A 2011, 84, 062308. [Google Scholar]
- ITU. Recommendation ITU-T G.652, Characteristics of a Single-Mode Optical Fibre and Cable. 2016. Available online: https://www.itu.int/itu-t/recommendations/rec.aspx?rec=13076&lang=en (accessed on 1 June 2022).
- Zhang, J.; Itzler, M.A.; Zbinden, H.; Pan, J.-W. Advances in InGaAs/InP single-photon detector systems for quantum communication. Light Sci. Appl. 2015, 4, e286. [Google Scholar] [CrossRef] [Green Version]
- Lin, W.; Zhang, H.; Song, B.; Miao, Y.; Liu, B.; Yan, D.; Liu, Y. Magnetically controllable wavelength-division multiplexing fiber coupler. Opt. Express 2015, 23, 11123–11134. [Google Scholar]
- Lee, Y.L.; Eom, T.J.; Shin, W.; Yu, B.-A.; Ko, D.-K.; Kim, W.-K.; Lee, H.-Y. Characteristics of a multi-mode interference device based on Ti:LiNbO3 channel waveguide. Opt. Express 2009, 17, 10718–10724. [Google Scholar] [CrossRef]
- Hua, P.-R.; Pun, E.Y.-B.; Yu, D.-Y.; Zhang, D.-L. Nonperiodic Oscillation with Wavelength of Mode Guided in a Special Ti-Diffused LiNbO3 Waveguide Structure. IEEE Photonics J. 2013, 5, 2202307. [Google Scholar]
- Liao, S.-K.; Cai, W.-Q.; Liu, W.-Y.; Zhang, L.; Li, Y.; Ren, J.-G.; Yin, J.; Shen, Q.; Cao, Y.; Li, Z.-P.; et al. Satellite-to-ground quantum key distribution. Nature 2017, 549, 43–47. [Google Scholar] [CrossRef] [Green Version]
- Fung, C.H.F.; Ma, X.; Chau, H.F. Practical issues in quantum-key-distribution postprocessing. Phys. Rev. A 2010, 81, 012318. [Google Scholar] [CrossRef] [Green Version]
- Lim, C.C.W.; Curty, M.; Walenta, N.; Xu, F.; Zbinden, H. Concise security bounds for practical decoy-state quantum key distribution. Phys. Rev. A 2014, 89, 022307. [Google Scholar] [CrossRef] [Green Version]
- Ma, X.; Qi, B.; Zhao, Y.; Lo, H.-K. Practical decoy state for quantum key distribution. Phys. Rev. A 2005, 72, 012326. [Google Scholar] [CrossRef] [Green Version]
- Lo, H.-K.; Curty, M.; Qi, B. Measurement-device-independent quantum key distribution. Phys. Rev. Lett. 2012, 108, 130503. [Google Scholar] [CrossRef] [Green Version]
- Cao, Y.; Li, Y.-H.; Yang, K.-X.; Jiang, Y.-F.; Li, S.-L.; Hu, X.-L.; Abulizi, M.; Li, C.-L.; Zhang, W.-J.; Sun, Q.-C.; et al. Long-Distance Free-Space Measurement-Device-Independent Quantum Key Distribution. Phys. Rev. Lett. 2020, 125, 260503. [Google Scholar] [CrossRef]
- Acin, A.; Brunner, N.; Gisin, N.; Massar, S.; Pironio, S.; Scarani, V. Device-Independent Security of Quantum Cryptography against Collective Attacks. Phys. Rev. Lett. 2007, 98, 230501. [Google Scholar] [CrossRef] [Green Version]
- Xu, F.-H.; Zhang, Y.-Z.; Zhang, Q.; Pan, J.-W. Device-Independent Quantum Key Distribution with Random Postselection. Phys. Rev. Lett. 2022, 128, 110506. [Google Scholar] [CrossRef]
- Lucamarini, M.; Yuan, Z.; Dynes, J.; Shields, A. Overcoming the rate–distance limit of quantum key distribution without quantum repeaters. Nature 2018, 557, 400–403. [Google Scholar] [CrossRef]
- Fang, X.-T.; Zeng, P.; Liu, H.; Zou, M.; Wu, W.; Tang, Y.-L.; Sheng, Y.-J.; Xiang, Y.; Zhang, W.-J.; Li, H.; et al. Implementation of quantum key distribution surpassing the linear rate-transmittance bound. Nat. Photonics 2020, 14, 422–425. [Google Scholar] [CrossRef]
- Lo, H.-K.; Chau, H.F.; Ardehali, M. Efficient Quantum Key Distribution Scheme and Proof of its Security. J. Cryptol. 2005, 18, 133–165. [Google Scholar] [CrossRef] [Green Version]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, D.-D.; Tang, Y.-L.; Zhao, Y.-K.; Zhou, L.; Zhao, Y.; Tang, S.-B. Security of Optical Beam Splitter in Quantum Key Distribution. Photonics 2022, 9, 527. https://doi.org/10.3390/photonics9080527
Li D-D, Tang Y-L, Zhao Y-K, Zhou L, Zhao Y, Tang S-B. Security of Optical Beam Splitter in Quantum Key Distribution. Photonics. 2022; 9(8):527. https://doi.org/10.3390/photonics9080527
Chicago/Turabian StyleLi, Dong-Dong, Yan-Lin Tang, Yu-Kang Zhao, Lei Zhou, Yong Zhao, and Shi-Biao Tang. 2022. "Security of Optical Beam Splitter in Quantum Key Distribution" Photonics 9, no. 8: 527. https://doi.org/10.3390/photonics9080527
APA StyleLi, D.-D., Tang, Y.-L., Zhao, Y.-K., Zhou, L., Zhao, Y., & Tang, S.-B. (2022). Security of Optical Beam Splitter in Quantum Key Distribution. Photonics, 9(8), 527. https://doi.org/10.3390/photonics9080527




