Improved Carrier-Envelope Phase Determination Method for Few-Cycle Laser Pulses Using High-Order Above-Threshold Ionization
Abstract
1. Introduction
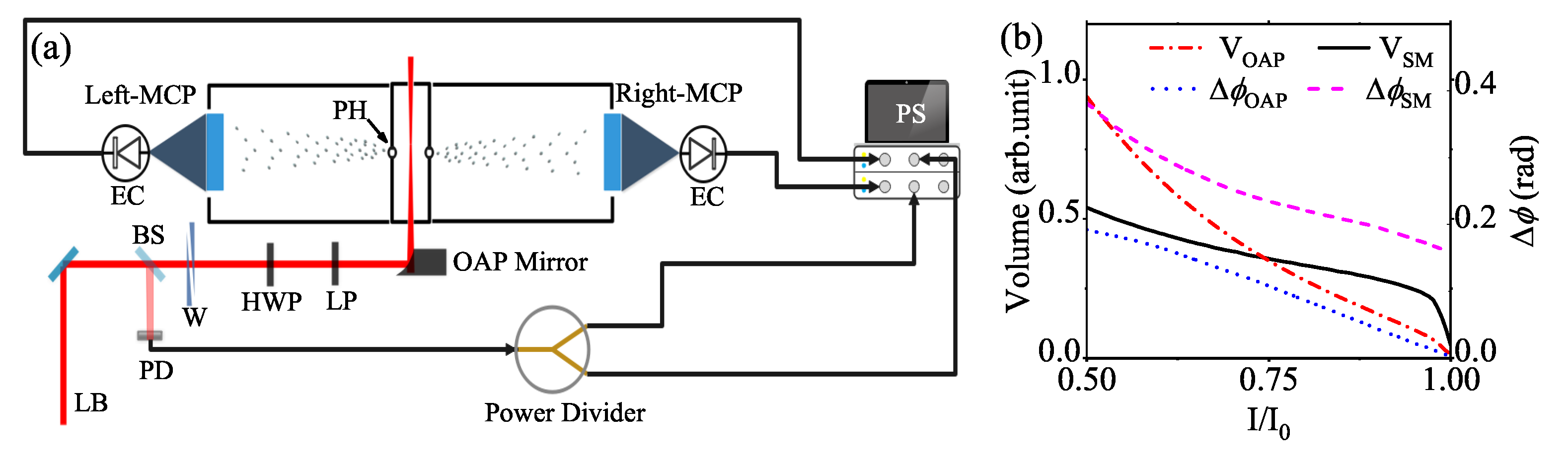
2. Experimental Setup
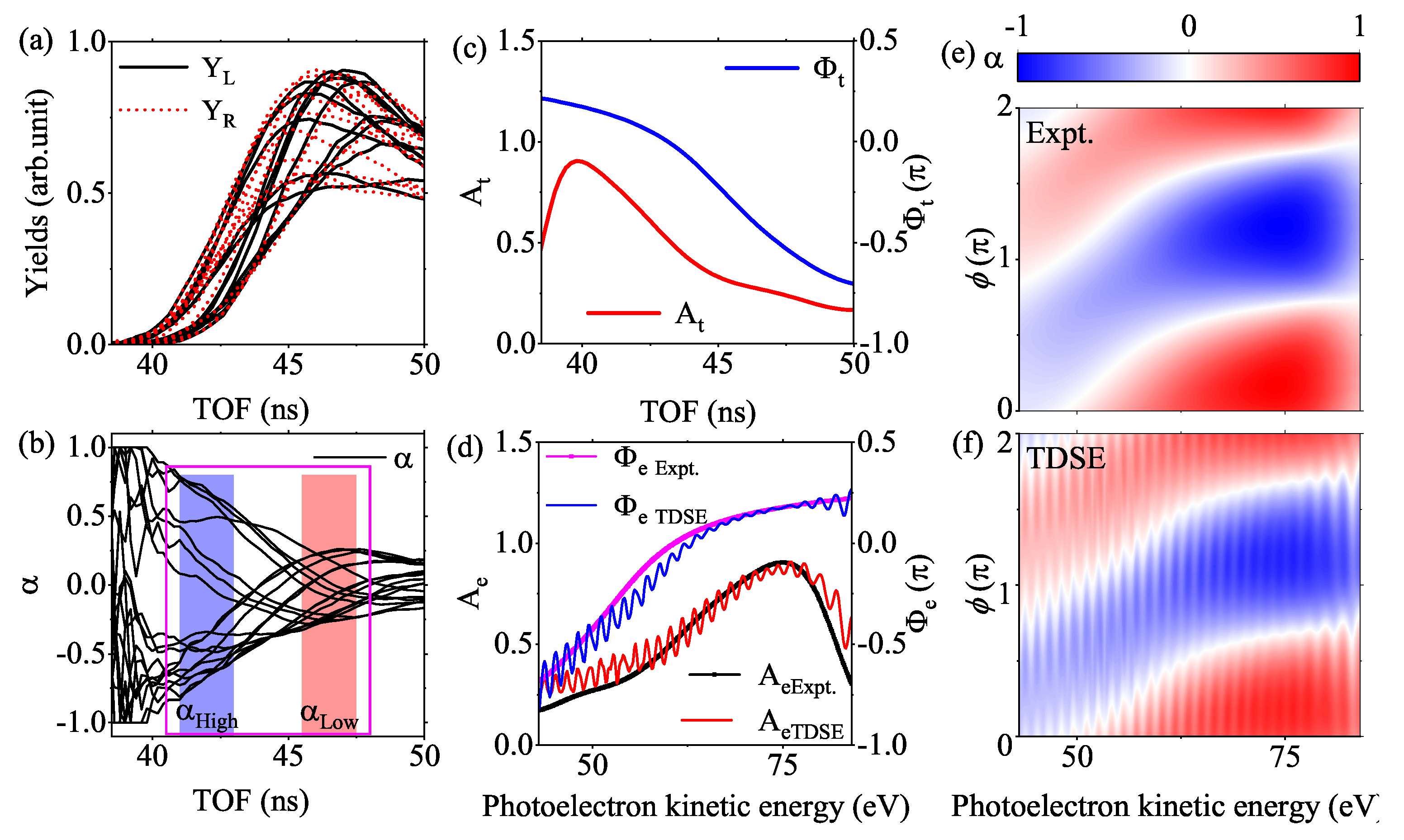
3. The Procedure to Extract the Absolute Carrier-Envelope Phase
4. Numerical Solution of the Time-Dependent Schrödinger Equation (TDSE)
5. Results and Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Paulus, G.G.; Grasbon, F.; Walther, H.; Villoresi, P.; Nisoli, M.; Stagira, S.; Priori, E.; De Silvestri, S. Absolute-phase phenomena in photoionization with few-cycle laser pulses. Nature 2001, 414, 182–184. [Google Scholar] [CrossRef] [PubMed]
- Baltuška, A.; Udem, T.; Uiberacker, M.; Hentschel, M.; Goulielmakis, E.; Gohle, C.; Holzwarth, R.; Yakovlev, V.S.; Scrinzi, A.; Hänsch, T.W.; et al. Attosecond control of electronic processes by intense light fields. Nature 2003, 421, 611–615. [Google Scholar] [CrossRef] [PubMed]
- Kling, M.F.; Siedschlag, C.; Verhoef, A.J.; Khan, J.I.; Schultze, M.; Uphues, T.; Ni, Y.; Uiberacker, M.; Drescher, M.; Krausz, F.; et al. Control of electron localization in molecular dissociation. Science 2006, 312, 246–248. [Google Scholar] [CrossRef] [PubMed]
- Milošević, D.B.; Paulus, G.G.; Bauer, D.; Becker, W. Above-threshold ionization by few-cycle pulses. J. Phys. B At. Mol. Opt. Phys. 2006, 39, R203–R262. [Google Scholar] [CrossRef]
- Bergues, B.; Kübel, M.; Johnson, N.G.; Fischer, B.; Camus, N.; Betsch, K.J.; Herrwerth, O.; Senftleben, A.; Sayler, A.M.; Rathje, T.; et al. Attosecond tracing of correlated electron-emission in non-sequential double ionization. Nat. Commun. 2012, 3, 813. [Google Scholar] [CrossRef]
- Liu, X.; Figueira de Morisson Faria, C. Nonsequential Double Ionization with Few-Cycle Laser Pulses. Phys. Rev. Lett. 2004, 92, 133006. [Google Scholar] [CrossRef]
- Li, H.; Gong, X.; Lin, K.; de Vivie-Riedle, R.; Tong, X.; Wu, J.; Kling, M.F. Sub-cycle directional control of the dissociative ionization of H2 in tailored femtosecond laser fields. J. Phys. B At. Mol. Opt. Phys. 2017, 50, 172001. [Google Scholar] [CrossRef]
- Alnaser, A.S.; Litvinyuk, I.V. Subfemtosecond directional control of chemical processes in molecules. J. Phys. B At. Mol. Opt. Phys. 2017, 50, 032002. [Google Scholar] [CrossRef]
- Sansone, G.; Benedetti, E.; Calegari, F.; Vozzi, C.; Avaldi, L.; Flammini, R.; Poletto, L.; Villoresi, P.; Altucci, C.; Velotta, R.; et al. Isolated single-cycle attosecond pulses. Science 2006, 314, 443–446. [Google Scholar] [CrossRef]
- Liu, X.; Rottke, H.; Eremina, E.; Sandner, W.; Goulielmakis, E.; Keeffe, K.O.; Lezius, M.; Krausz, F.; Lindner, F.; Schätzel, M.G.; et al. Nonsequential Double Ionization at the Single-Optical-Cycle Limit. Phys. Rev. Lett. 2004, 93, 263001. [Google Scholar] [CrossRef]
- Quan, W.; Xiao, Z.; Chen, Y.; Xu, S.; Lai, X.; Hua, L.; Gong, C.; Chen, J.; Liu, X. Nonsequential double ionization of Ne subject to few-cycle femtosecond laser pulses. Sci. Sin. Phys. Mech. Astron. 2017, 47, 033007. [Google Scholar] [CrossRef]
- Chen, Y.J.; Yu, S.G.; Lai, X.Y.; Quan, W.; Liu, X.J. Coulomb effect on the left–right asymmetry in photoelectron emission with few-cycle laser pulses. Phys. Lett. A 2016, 380, 2085–2089. [Google Scholar] [CrossRef]
- Rathje, T.; Sayler, A.M.; Zeng, S.; Wustelt, P.; Figger, H.; Esry, B.D.; Paulus, G.G. Coherent control at its most fundamental: Carrier-envelope-phase-dependent electron localization in photodissociation of a H2+ molecular ion beam target. Phys. Rev. Lett. 2013, 111, 093002. [Google Scholar] [CrossRef] [PubMed]
- Zherebtsov, S.; Fennel, T.; Plenge, J.; Antonsson, E.; Znakovskaya, I.; Wirth, A.; Herrwerth, O.; Süßmann, F.; Peltz, C.; Ahmad, I.; et al. Controlled near-field enhanced electron acceleration from dielectric nanospheres with intense few-cycle laser fields. Nat. Phys. 2011, 7, 656–662. [Google Scholar] [CrossRef]
- Krüger, M.; Schenk, M.; Hommelhoff, P. Attosecond control of electrons emitted from a nanoscale metal tip. Nature 2011, 475, 78–81. [Google Scholar] [CrossRef]
- Ciappina, M.F.; Pérez-Hernández, J.A.; Landsman, A.S.; Okell, W.A.; Zherebtsov, S.; Förg, B.; Schötz, J.; Seiffert, L.; Fennel, T.; Shaaran, T.; et al. Attosecond physics at the nanoscale. Rep. Prog. Phys. 2017, 80, 054401. [Google Scholar] [CrossRef]
- Jones, D.J.; Diddams, S.A.; Ranka, J.K.; Stentz, A.; Windeler, R.S.; Hall, J.L.; Cundiff, S.T. Carrier-envelope phase control of femtosecond mode-locked lasers and direct optical frequency synthesis. Science 2000, 288, 635–639. [Google Scholar] [CrossRef]
- Kurucz, M.; Flender, R.; Haizer, L.; Nagymihaly, R.S.; Cho, W.; Kim, K.T.; Toth, S.; Cormier, E.; Kiss, B. 2.3-cycle mid-infrared pulses from hybrid thin-plate post-compression at 7 W average power. Opt. Commun. 2020, 472, 126035. [Google Scholar] [CrossRef]
- Peters, W.K.; Jones, T.; Efimov, A.; Pedersoli, E.; Foglia, L.; Mincigrucci, R.; Nikolov, I.; Trebino, R.; Danailov, M.B.; Capotondi, F.; et al. All-optical single-shot complete electric field measurement of extreme ultraviolet free electron laser pulses. Optica 2021, 8, 545–550. [Google Scholar] [CrossRef]
- Kurucz, M.; Tóth, S.; Flender, R.; Haizer, L.; Kiss, B.; Persielle, B.; Cormier, E. Single-shot CEP drift measurement at arbitrary repetition rate based on dispersive Fourier transform. Opt. Express 2019, 27, 13387–13399. [Google Scholar] [CrossRef]
- Fukahori, S.; Ando, T.; Miura, S.; Kanya, R.; Yamanouchi, K.; Rathje, T.; Paulus, G.G. Determination of the absolute carrier-envelope phase by angle-resolved photoelectron spectra of Ar by intense circularly polarized few-cycle pulses. Phys. Rev. A 2017, 95, 053410. [Google Scholar] [CrossRef]
- Kübel, M.; Wustelt, P.; Zhang, Y.; Skruszewicz, S.; Hoff, D.; Würzler, D.; Kang, H.; Zille, D.; Adolph, D.; Paulus, G.G.; et al. High-Order Phase-Dependent Asymmetry in the Above-Threshold Ionization Plateau. Phys. Rev. Lett. 2021, 126, 113201. [Google Scholar] [CrossRef] [PubMed]
- Wittmann, T.; Horvath, B.; Helml, W.; Schätzel, M.G.; Gu, X.; Cavalieri, A.L.; Paulus, G.G.; Kienberger, R. Single-shot carrier–envelope phase measurement of few-cycle laser pulses. Nat. Phys. 2009, 5, 357–362. [Google Scholar] [CrossRef]
- Sayler, A.M.; Rathje, T.; Müller, W.; Rühle, K.; Kienberger, R.; Paulus, G.G. Precise, real-time, every-single-shot, carrier-envelope phase measurement of ultrashort laser pulses. Opt. Lett. 2011, 36, 1–3. [Google Scholar] [CrossRef] [PubMed]
- Hoff, D.; Furch, F.J.; Witting, T.; Rühle, K.; Adolph, D.; Sayler, A.M.; Vrakking, M.J.J.; Paulus, G.G.; Schulz, C.P. Continuous every-single-shot carrier-envelope phase measurement and control at 100 kHz. Opt. Lett. 2018, 43, 3850–3853. [Google Scholar] [CrossRef] [PubMed]
- Adolph, D.; Möller, M.; Bierbach, J.; Schwab, M.; Sävert, A.; Yeung, M.; Sayler, A.M.; Zepf, M.; Kaluza, M.C.; Paulus, G.G. Real-time, single-shot, carrier-envelope-phase measurement of a multi-terawatt laser. Appl. Phys. Lett. 2017, 110, 081105. [Google Scholar] [CrossRef]
- Johnson, N.G.; Herrwerth, O.; Wirth, A.; De, S.; Ben-Itzhak, I.; Lezius, M.; Bergues, B.; Kling, M.F.; Senftleben, A.; Schröter, C.D.; et al. Single-shot carrier-envelope-phase-tagged ion-momentum imaging of nonsequential double ionization of argon in intense 4-fs laser fields. Phys. Rev. A 2011, 83, 013412. [Google Scholar] [CrossRef]
- Schöffler, M.S.; Xie, X.; Wustelt, P.; Möller, M.; Roither, S.; Kartashov, D.; Sayler, A.M.; Baltuska, A.; Paulus, G.G.; Kitzler, M. Laser-subcycle control of sequential double-ionization dynamics of helium. Phys. Rev. A 2016, 93, 063421. [Google Scholar] [CrossRef]
- Kangaparambil, S.; Hanus, V.; Dorner-Kirchner, M.; He, P.; Larimian, S.; Paulus, G.; Baltuška, A.; Xie, X.; Yamanouchi, K.; He, F.; et al. Generalized Phase Sensitivity of Directional Bond Breaking in the Laser-Molecule Interaction. Phys. Rev. Lett. 2020, 125, 023202. [Google Scholar] [CrossRef]
- Hanus, V.; Kangaparambil, S.; Larimian, S.; Dorner-Kirchner, M.; Xie, X.; Schöffler, M.S.; Paulus, G.G.; Baltuška, A.; Staudte, A.; Kitzler-Zeiler, M. Experimental Separation of Subcycle Ionization Bursts in Strong-Field Double Ionization of H2. Phys. Rev. Lett. 2020, 124, 103201. [Google Scholar] [CrossRef]
- Kim, Y.H.; Ivanov, I.A.; Hwang, S.I.; Kim, K.; Nam, C.H.; Kim, K.T. Attosecond streaking using a rescattered electron in an intense laser field. Sci. Rep. 2020, 10, 22075. [Google Scholar] [CrossRef] [PubMed]
- Hansinger, P.; Töpfer, P.; Dimitrov, N.; Adolph, D.; Hoff, D.; Rathje, T.; Sayler, A.M.; Dreischuh, A.; Paulus, G.G. Refractive index dispersion measurement using carrier-envelope phasemeters. New J. Phys. 2017, 19, 023040. [Google Scholar] [CrossRef][Green Version]
- Paulus, G.G.; Lindner, F.; Walther, H.; Baltuška, A.; Goulielmakis, E.; Lezius, M.; Krausz, F. Measurement of the Phase of Few-Cycle Laser Pulses. Phys. Rev. Lett. 2003, 91, 253004. [Google Scholar] [CrossRef] [PubMed]
- Milošević, D.B.; Paulus, G.G.; Becker, W. High-order above-threshold ionization with few-cycle pulse: A meter of the absolute phase. Opt. Express 2003, 11, 1418–1429. [Google Scholar] [CrossRef] [PubMed]
- Chelkowski, S.; Bandrauk, A.D. Asymmetries in strong-field photoionization by few-cycle laser pulses: Kinetic-energy spectra and semiclassical explanation of the asymmetries of fast and slow electrons. Phys. Rev. A 2005, 71, 053815. [Google Scholar] [CrossRef]
- Möller, M.; Sayler, A.M.; Rathje, T.; Chini, M.; Chang, Z.; Paulus, G.G. Precise, real-time, single-shot carrier-envelope phase measurement in the multi-cycle regime. Appl. Phys. Lett. 2011, 99, 121108. [Google Scholar] [CrossRef]
- Kübel, M.; Betsch, K.J.; Johnson, N.G.; Kleineberg, U.; Moshammer, R.; Ullrich, J.; Paulus, G.G.; Kling, M.F.; Bergues, B. Carrier-envelope-phase tagging in measurements with long acquisition times. New J. Phys. 2012, 14, 093027. [Google Scholar] [CrossRef]
- Chew, S.; Gliserin, A.; Schmidt, J.; Kleineberg, U. Increasing the Sensitivity of Carrier-Envelope-Phase Tagging in Photoemission From Solids by Single-Shot Intensity Correction. Phys. Rev. Appl. 2019, 11, 054080. [Google Scholar] [CrossRef]
- Chew, S.H.; Gliserin, A.; Schmidt, J.; Bian, H.; Nobis, S.; Schertz, F.; Kübel, M.; Yang, Y.Y.; Loitsch, B.; Stettner, T.; et al. Laser intensity effects in carrier-envelope phase-tagged time of flight-photoemission electron microscopy. Appl. Phys. B 2016, 122, 102. [Google Scholar] [CrossRef]
- Zhou, Y.; Guo, L.; Quan, W.; Wei, M.; Zhao, M.; Xu, S.; Xiao, Z.; Sun, R.; Wang, Y.; Lai, X.; et al. Carrier-envelope phase dependence of high-order above-threshold ionization by few-cycle laser pulses. J. Phys. B At. Mol. Opt. Phys. 2021, 54, 144008. [Google Scholar] [CrossRef]
- Rathje, T.; Johnson, N.G.; Möller, M.; Süßmann, F.; Adolph, D.; Kübel, M.; Kienberger, R.; Kling, M.F.; Paulus, G.G.; Sayler, A.M. Review of attosecond resolved measurement and control via carrier–envelope phase tagging with above-threshold ionization. J. Phys. B At. Mol. Opt. Phys. 2012, 45, 074003. [Google Scholar] [CrossRef]
- Porras, M.A. Characterization of the electric field of focused pulsed Gaussian beams for phase-sensitive interactions with matter. Opt. Lett. 2009, 34, 1546–1548. [Google Scholar] [CrossRef] [PubMed]
- Hoff, D.; Krüger, M.; Maisenbacher, L.; Sayler, A.M.; Paulus, G.G.; Hommelhoff, P. Tracing the phase of focused broadband laser pulses. Nat. Phys. 2017, 13, 947–951. [Google Scholar] [CrossRef]
- Hoff, D.; Krüger, M.; Maisenbacher, L.; Paulus, G.G.; Hommelhoff, P.; Sayler, A.M. Using the focal phase to control attosecond processes. J. Opt. 2017, 19, 124007. [Google Scholar] [CrossRef]
- Zhang, Y.; Zille, D.; Hoff, D.; Wustelt, P.; Würzler, D.; Möller, M.; Sayler, A.M.; Paulus, G.G. Observing the Importance of the Phase-Volume Effect for Few-Cycle Light-Matter Interactions. Phys. Rev. Lett. 2020, 124, 133202. [Google Scholar] [CrossRef] [PubMed]
- Sayler, A.M.; Rathje, T.; Müller, W.; Kürbis, C.; Rühle, K.; Stibenz, G.; Paulus, G.G. Real-time pulse length measurement of few-cycle laser pulses using above-threshold ionization. Opt. Express 2011, 19, 4464–4471. [Google Scholar] [CrossRef]
- Tong, X.M.; Lin, C.D. Empirical formula for static field ionization rates of atoms and molecules by lasers in the barrier-suppression regime. J. Phys. B At. Mol. Opt. Phys. 2005, 38, 2593–2600. [Google Scholar] [CrossRef]
- Milošević, D.B.; Becker, W.; Okunishi, M.; Prümper, G.; Shimada, K.; Ueda, K. Strong-field electron spectra of rare-gas atoms in the rescattering regime: Enhanced spectral regions and a simulation of the experiment. J. Phys. B At. Mol. Opt. Phys. 2010, 43, 015401. [Google Scholar] [CrossRef]
- Bauer, D.; Koval, P. Qprop: A Schrödinger-solver for intense laser–atom interaction. Comput. Phys. Commun. 2006, 174, 396–421. [Google Scholar] [CrossRef]
- Kopold, R.; Becker, W.; Kleber, M.; Paulus, G.G. Channel-closing effects in high-order above-threshold ionization and high-order harmonic generation. J. Phys. B At. Mol. Opt. Phys. 2002, 35, 217–232. [Google Scholar] [CrossRef]
- Xu, S.; Liu, M.; Hu, S.; Shu, Z.; Quan, W.; Xiao, Z.; Zhou, Y.; Wei, M.; Zhao, M.; Sun, R.; et al. Observation of a transition in the dynamics of strong-field atomic excitation. Phys. Rev. A 2020, 102, 043104. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, Y.; Quan, W.; Zhao, M.; Wang, Z.; Wang, M.; Cheng, S.; Chen, J.; Liu, X. Improved Carrier-Envelope Phase Determination Method for Few-Cycle Laser Pulses Using High-Order Above-Threshold Ionization. Photonics 2022, 9, 528. https://doi.org/10.3390/photonics9080528
Zhou Y, Quan W, Zhao M, Wang Z, Wang M, Cheng S, Chen J, Liu X. Improved Carrier-Envelope Phase Determination Method for Few-Cycle Laser Pulses Using High-Order Above-Threshold Ionization. Photonics. 2022; 9(8):528. https://doi.org/10.3390/photonics9080528
Chicago/Turabian StyleZhou, Yu, Wei Quan, Meng Zhao, Zhiqiang Wang, Minghui Wang, Sijin Cheng, Jing Chen, and Xiaojun Liu. 2022. "Improved Carrier-Envelope Phase Determination Method for Few-Cycle Laser Pulses Using High-Order Above-Threshold Ionization" Photonics 9, no. 8: 528. https://doi.org/10.3390/photonics9080528
APA StyleZhou, Y., Quan, W., Zhao, M., Wang, Z., Wang, M., Cheng, S., Chen, J., & Liu, X. (2022). Improved Carrier-Envelope Phase Determination Method for Few-Cycle Laser Pulses Using High-Order Above-Threshold Ionization. Photonics, 9(8), 528. https://doi.org/10.3390/photonics9080528






