High-Performance Electro-Optical Mach–Zehnder Modulators in a Silicon Nitride–Lithium Niobate Thin-Film Hybrid Platform
Abstract
:1. Introduction
2. Device Description and Methods
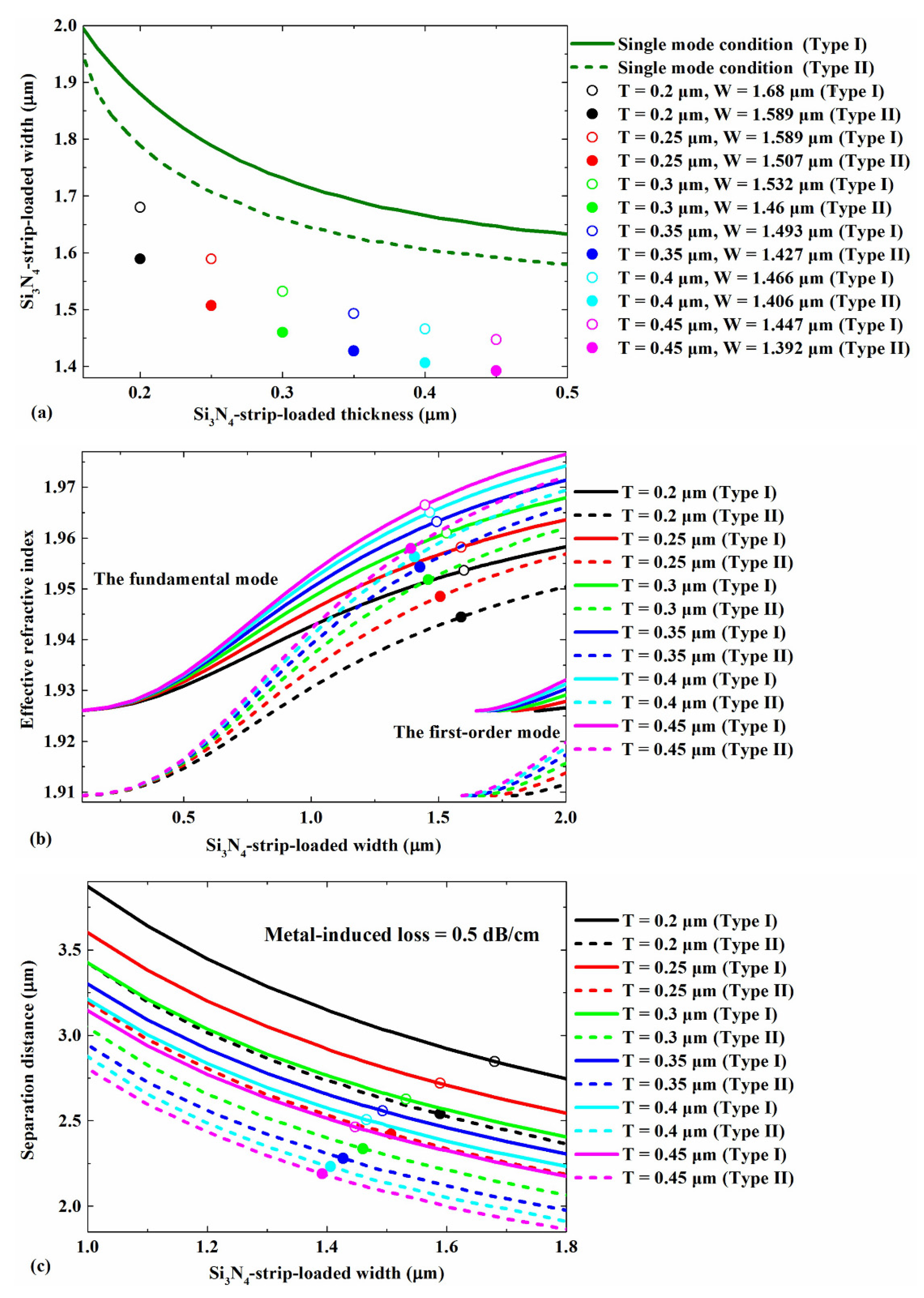
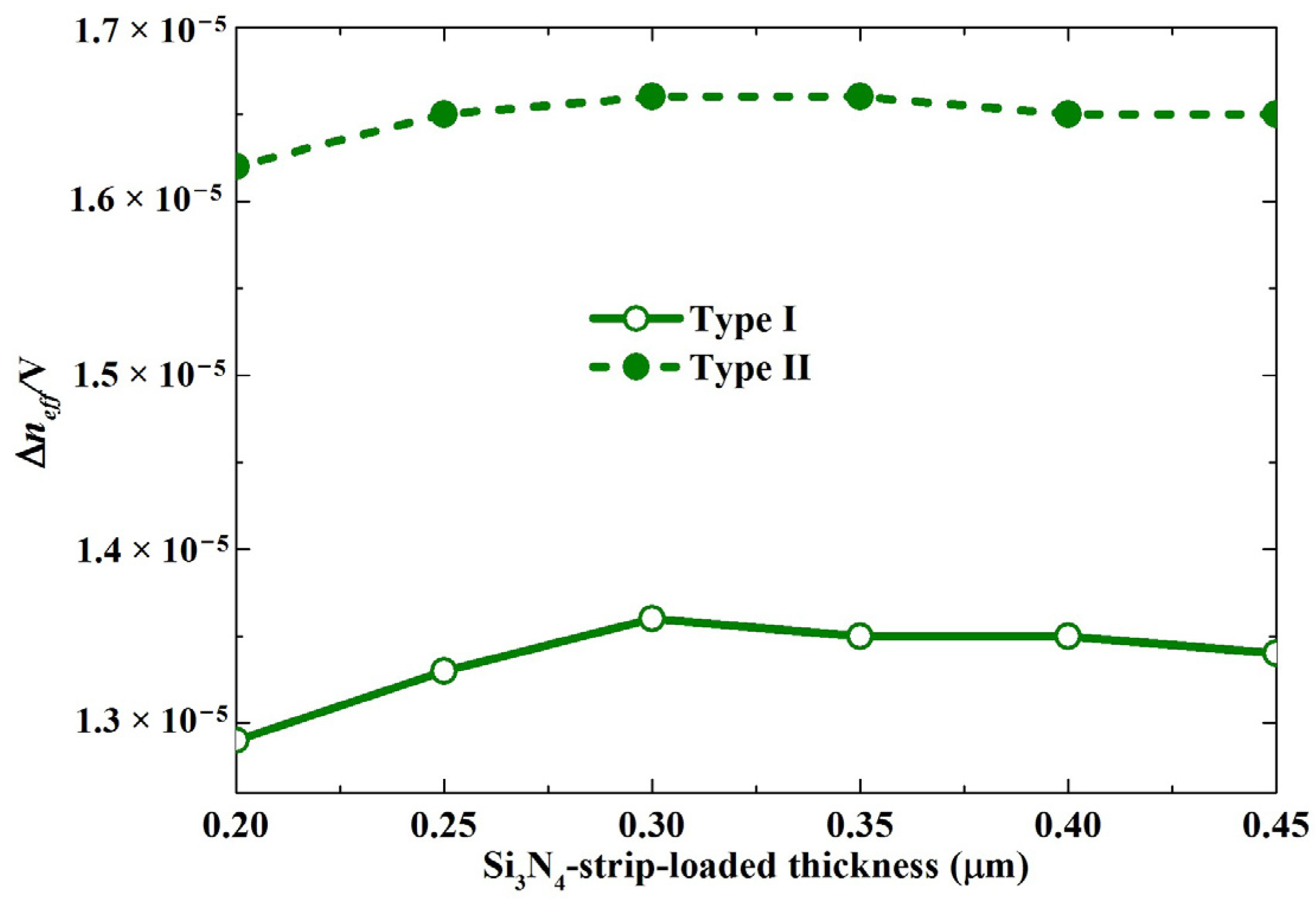
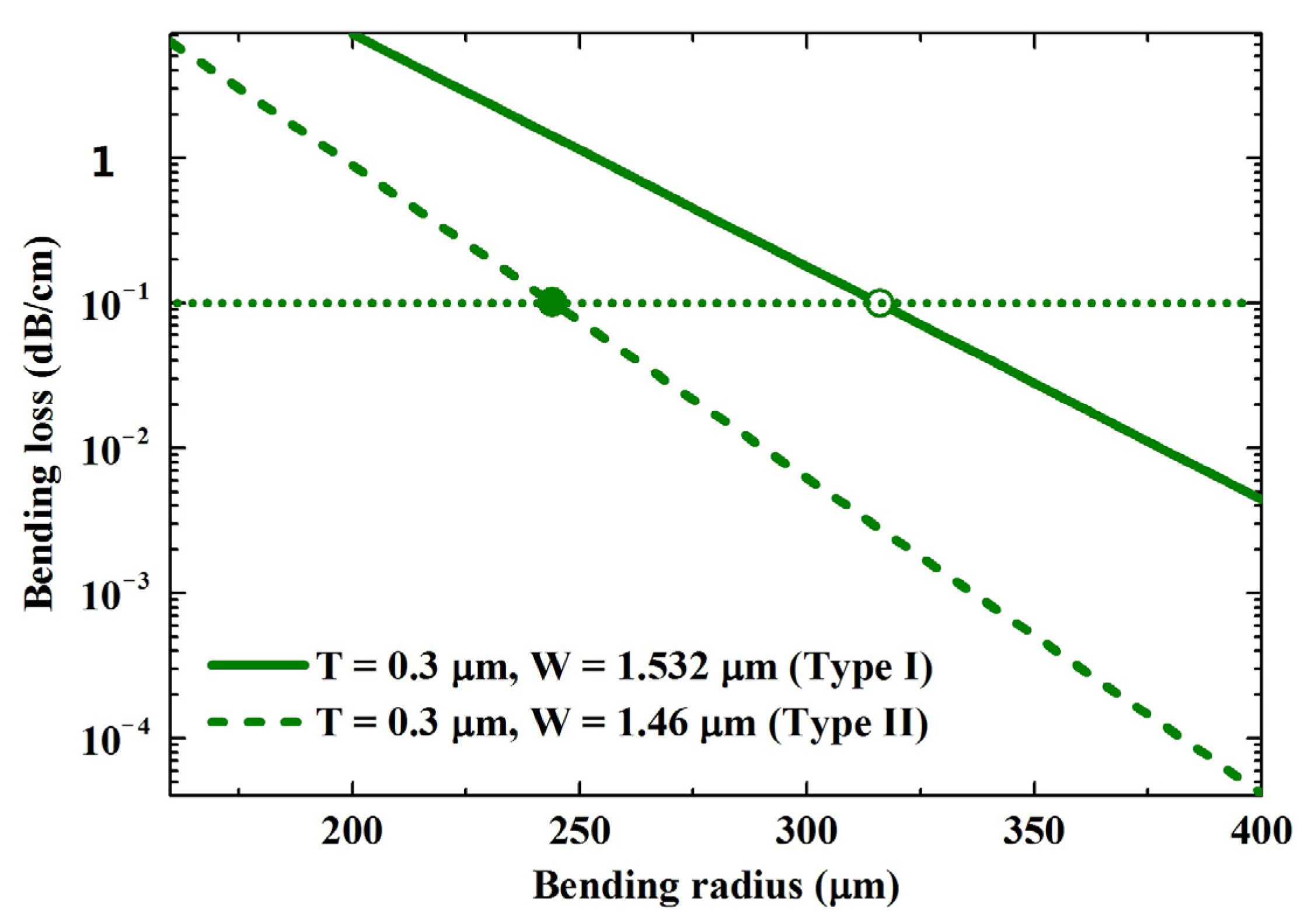
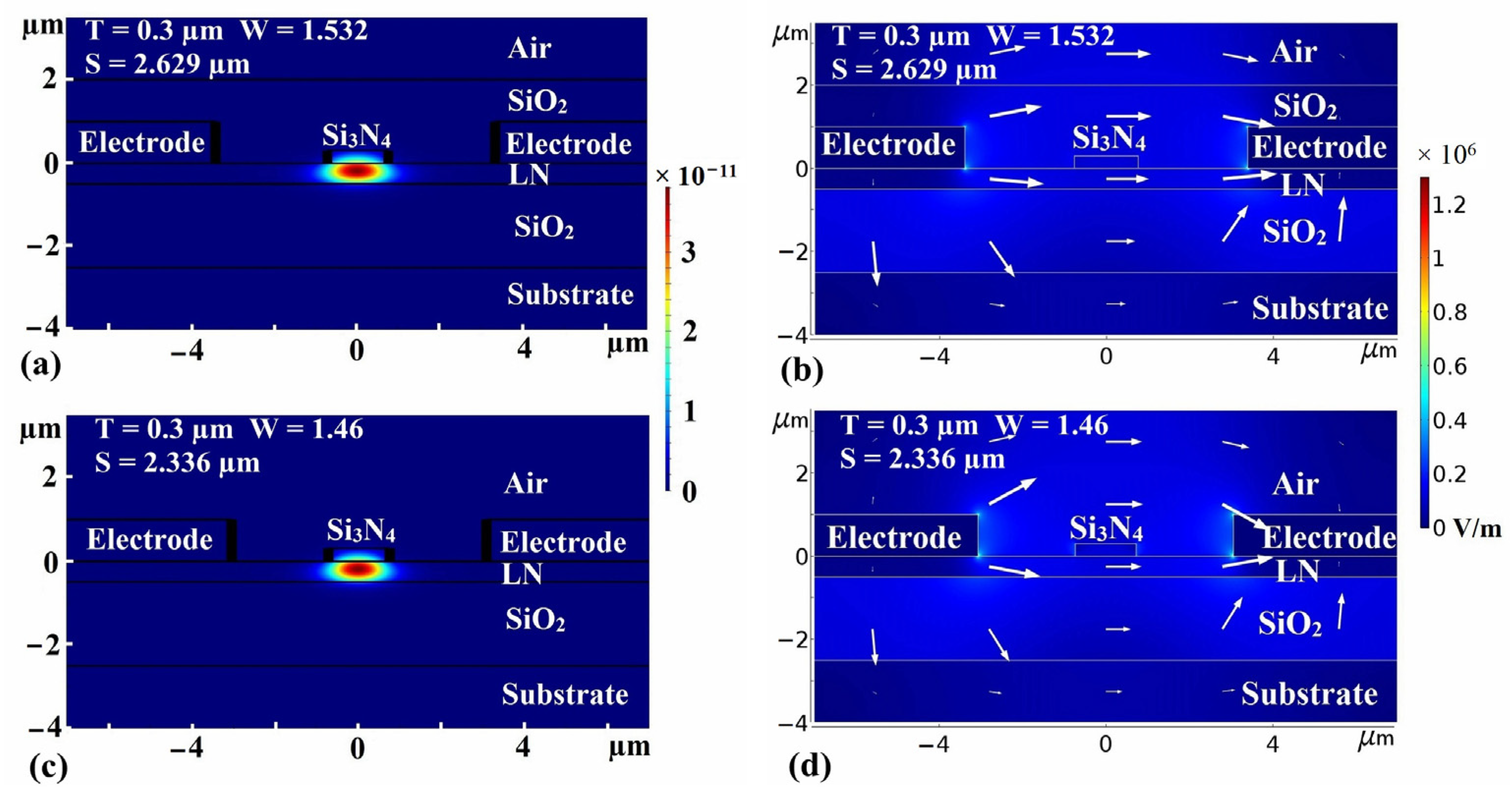
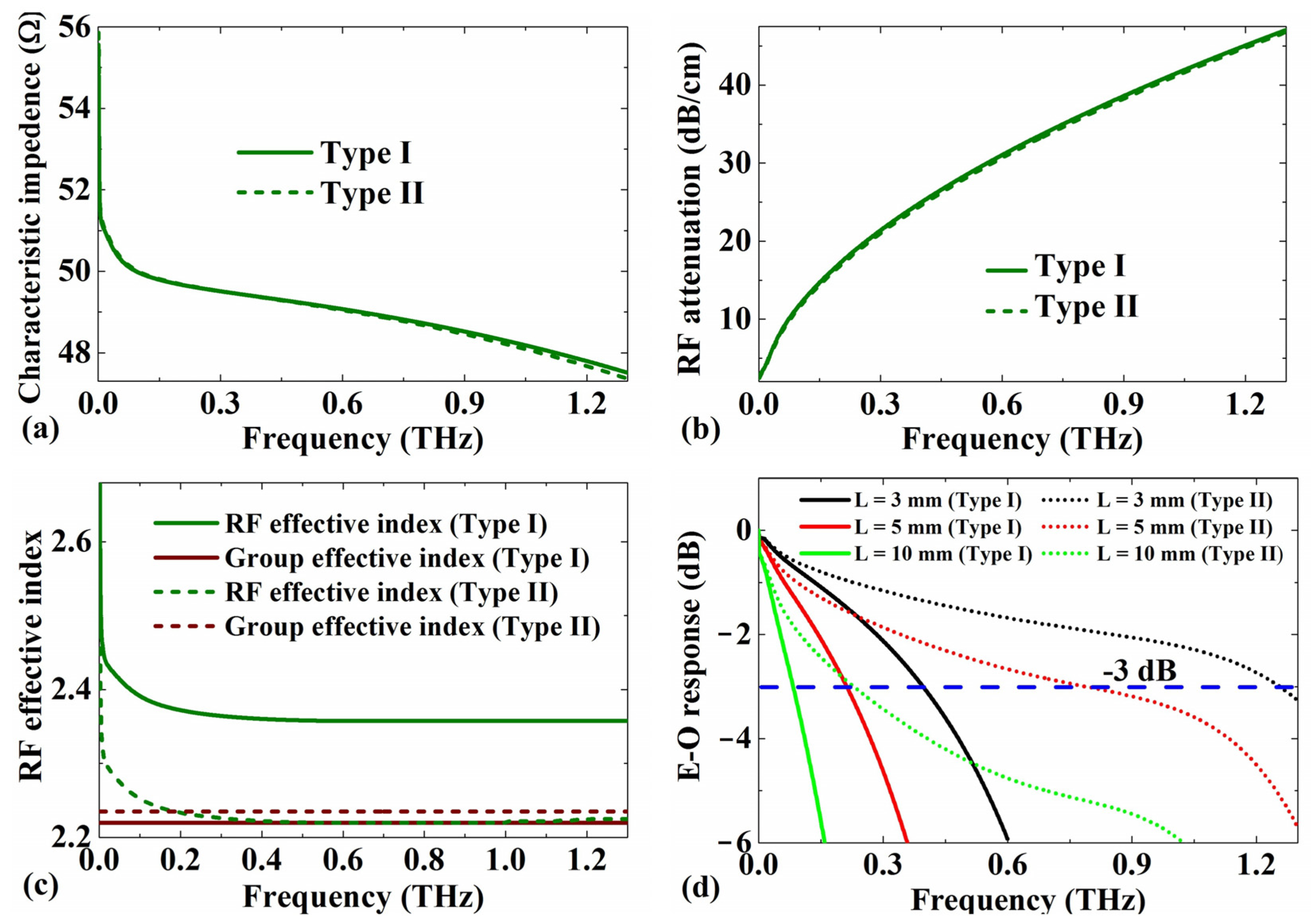
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Poberaj, G.; Hu, H.; Sohler, W.; Günter, P. Lithium niobate on insulator (LNOI) for micro-photonic devices. Laser Photonics Rev. 2012, 6, 488–503. [Google Scholar] [CrossRef]
- Han, H.; Cai, L.; Hu, H. Optical and structural properties of Single-crystal lithium niobate thin film. Opt. Mater. 2015, 42, 47–51. [Google Scholar] [CrossRef]
- Wang, C.; Zhang, M.; Chen, X.; Bertrand, M.; Shams-Ansari, A.; Chandrasekhar, S.; Winzer, P.; Lončar, M. Integrated lithium niobate electro-optic modulators operating at CMOS-compatible voltages. Nature 2018, 562, 101–104. [Google Scholar] [CrossRef] [PubMed]
- Xu, M.; He, M.; Zhang, H.; Jian, J.; Pan, Y.; Liu, X.; Chen, L.; Meng, X.; Chen, H.; Li, Z.; et al. High-performance coherent optical modulators based on thin-film lithium niobate platform. Nat. Commun. 2020, 11, 3911. [Google Scholar] [CrossRef] [PubMed]
- Hu, H.; Ricken, R.; Sohler, W. Lithium niobate photonic wires. Opt. Express 2009, 17, 24261–24268. [Google Scholar] [CrossRef]
- Krasnokutska, I.; Tambasco, J.-L.J.; Li, X.; Peruzzo, A. Ultra-low loss photonic circuits in Lithium Niobate on Insulator. Opt. Express 2017, 26, 897–904. [Google Scholar] [CrossRef] [Green Version]
- Guarino, A.; Poberaj, G.; Rezzonico, D.; Degl’innocenti, R.; Günter, P. Electro-optically tunable microring resonators in lithium niobate. Nat. Photon. 2007, 1, 407–410. [Google Scholar] [CrossRef]
- Ahmed, A.N.R.; Shi, S.; Zablocki, M.; Yao, P.; Prather, D.W. Tunable hybrid silicon nitride and thin-film lithium niobate electro-optic microresonator. Opt. Lett. 2019, 44, 618–621. [Google Scholar] [CrossRef] [Green Version]
- Ahmed, A.N.R.; Nelan, S.; Shi, S.; Yao, P.; Mercante, A.; Prather, D.W. Subvolt electro-optical modulator on thin-film lithium niobate and silicon nitride hybrid platform. Opt. Lett. 2020, 45, 1112–1115. [Google Scholar] [CrossRef]
- Jin, S.; Xu, L.; Zhang, H.; Li, Y. LiNbO3 thin-film modulators using silicon nitride surface ridge waveguides. IEEE Photonics Technol. Lett. 2016, 28, 736–739. [Google Scholar] [CrossRef]
- Liu, D.; Feng, L.; Jia, Y.; Ma, H. Heterogeneous integration of LN and Si3N4 waveguides using an optical interlayer coupler. Opt. Commun. 2018, 436, 1–6. [Google Scholar] [CrossRef]
- Ahmed, A.N.R.; Mercante, A.; Shi, S.; Yao, P.; Prather, D.W. Vertical mode transition in hybrid lithium niobate and silicon nitride-based photonic integrated circuit structures. Opt. Lett. 2018, 43, 4140–4143. [Google Scholar] [CrossRef] [Green Version]
- Qiu, W.; Bernal, M.P.; Ndao, A.; Guyot, C.; Hameed, N.M.; Courjal, N.; Maillotte, H.; Baida, F.I. Analysis of ultra-compact waveguide modes in thin film lithium niobate. Appl. Phys. B 2015, 118, 261–267. [Google Scholar] [CrossRef]
- Wang, Y.; Chen, Z.; Hu, H. Analysis of waveguides on lithium niobate thin films. Crystals 2018, 8, 191. [Google Scholar] [CrossRef] [Green Version]
- Sarma, A.K.; Saha, M.; Biswas, A. Effect of two-photon absorption on soliton propagation and soliton-soliton interaction in a silicon waveguide. Opt. Eng. 2010, 49, 211–223. [Google Scholar] [CrossRef]
- Cao, L.; Aboketaf, A.; Wang, Z.; Preble, S. Hybrid amorphous silicon (a-Si:H)–LiNbO3 electro-optic modulator. Opt. Commun. 2014, 330, 40–44. [Google Scholar] [CrossRef]
- Chiles, J.; Fathpour, S. Mid-infrared integrated waveguide modulators based on silicon-on-lithium-niobate photonics. Optica 2014, 1, 350–355. [Google Scholar] [CrossRef]
- Rao, A.; Malinowski, M.; Honardoost, A.; Talukder, J.R.; Rabiei, P.; Delfyett, P.; Fathpour, S. Secondharmonic generation in periodically-poled thin film lithium niobate wafer-bonded on silicon. Opt. Express 2016, 24, 29941–29947. [Google Scholar] [CrossRef] [Green Version]
- Chang, L.; Pfeiffer, M.H.P.; Volet, N.; Zervas, M.; Peters, J.D.; Manganelli, C.L.; Stanton, E.J.; Li, Y.; Kippenberg, T.J.; Bowers, J.E. Heterogeneous integration of lithium niobate and silicon nitride waveguides for wafer-scale photonic integrated circuits on silicon. Opt. Lett. 2017, 42, 803–806. [Google Scholar] [CrossRef]
- Boes, A.; Corcoran, B.; Chang, L.; Bowers, J.; Mitchell, A. Status and potential of lithium niobate on insulator (LNOI) for photonic integrated circuits. Laser Photonics Rev. 2018, 12, 1700256. [Google Scholar] [CrossRef]
- Huffman, T.; Brodnik, G.M.; Pinho, C.; Gundavarapu, S.; Baney, D.; Blumenthal, D.J. Integrated resonators in ultra-Low loss Si3N4/SiO2 for multifunction applications. IEEE J. Sel. Top. Quant. 2018, 24, 1. [Google Scholar] [CrossRef]
- Wong, K.K. Properties of Lithium Niobate, 1st ed.; INSPEC: London, UK, 2002; pp. 115–128. [Google Scholar]
- Dai, D.; Zhen, S. Numerical analysis of silicon-on-insulator ridge nanowires by using a full-vectorial finite-difference method mode solver. J. Opt. Soc. Am. B 2007, 24, 2853–2859. [Google Scholar] [CrossRef]
- Zhu, Z.; Brown, T.G. Full-vectorial finite-difference analysis of microstructure optical fibers. Opt. Express 2002, 10, 853–864. [Google Scholar] [CrossRef] [PubMed]
- Afrouzi, H.N.; Abdul-Malek, Z.; Mashak, S.V.; Naderipour, A. Three-dimensional potential and electric field distributions in hv cable insulation containing multiple cavities. Adv. Mat. Res. 2014, 845, 372–377. [Google Scholar]
- Rahman, B.; Fernandez, F.A. Review of finite element methods for microwave and optical waveguides. Proc. IEEE 1991, 79, 1442–1448. [Google Scholar] [CrossRef]
- Lvescan, S.; Vonsovici, A. The single-mode condition for semiconductor rib waveguides with large cross section. J. Lightwave Technol. 1998, 16, 1851–1853. [Google Scholar]
- Uchida, N. Optical waveguide loaded with high refractive-index strip film. Appl. Opt. 1976, 15, 179–182. [Google Scholar] [CrossRef]
- Sieger, M.; Balluff, F.; Wang, X.; Kim, S.S.; Leidner, L.; Gauglitz, G.; Mizaikoff, B. On-chip integrated mid-infrared GaAs/AlGaAs Mach-Zehnder interferometer. Anal. Chem. 2013, 85, 3050–3052. [Google Scholar] [CrossRef]
- Wang, J.; Xu, S.; Chen, J.; Zou, W. A heterogeneous silicon on lithium niobate modulator for ultra-compact and high-performance photonic integrated circuits. IEEE Photonics J. 2021, 1, 99. [Google Scholar] [CrossRef]
- García-Granda, M.; Hu, H.; Rodríguez-García, J.; Sohler, W. Design and fabrication of novel ridge guide modulators in lithium niobate. J. Lightwave Technol. 2010, 27, 5690–5697. [Google Scholar] [CrossRef]
- Ghion, G. Semiconductor Devices for High-Speed Optoelectronics, 1st ed.; Cambridge University: New York, NY, USA, 2009; pp. 375–391. [Google Scholar]
- He, M.; Xu, M.; Ren, Y.; Jian, J.; Ruan, Z.; Xu, Y.; Gao, S.; Sun, S.; Wen, X.; Zhou, L.; et al. High-performance hybrid silicon and lithium niobate Mach–Zehnder modulators for 100 Gbit s−1 and beyond. Nat. Photonics 2019, 13, 359–364. [Google Scholar] [CrossRef]
- Rao, A.; Patil, A.; Rabiei, P.; Honardoost, A.; Desalvo, R.; Paolella, A.; Fathpour, S. High-performance and linear thin-film lithium niobate Mach–Zehnder modulators on silicon up to 50 GHz. Opt. Lett. 2016, 41, 5700–5703. [Google Scholar] [CrossRef] [PubMed]
- Zhang, P.; Huang, H.; Jiang, Y.; Han, X.; Xiao, H.; Frigg, A.; Nguyen, T.G.; Boes, A.; Ren, G.; Su, Y.; et al. High-speed electro-optic modulator based on silicon nitride loaded lithium niobate on an insulator platform. Opt. Lett. 2021, 46, 5986–5989. [Google Scholar] [CrossRef] [PubMed]
- Honardoost, A.; Juneghani, F.A.; Safian, R.; Fathpour, S. Towards subterahertz bandwidth ultracompact lithium niobate electrooptic modulators. Opt. Express 2019, 27, 6495–6501. [Google Scholar] [CrossRef]
- Boynton, N.; Cai, H.; Gehl, M.; Arterburn, S.; Dallo, C.; Pomerene, A.; Starbuck, A.; Hood, D.; Trotter, D.C.; Friedmann, T.; et al. A heterogeneously integrated silicon photonic/lithium niobate travelling wave electro-optic modulator. Opt. Express 2020, 28, 1868–1884. [Google Scholar] [CrossRef] [PubMed]
- Weigel, P.O.; Zhao, J.; Fang, K.; Al-Rubaye, H.; Trotter, D.; Hood, D.; Mudrick, J.; Dallo, C.; Pomerene, A.T.; Starbuck, A.L.; et al. Bonded thin film lithium niobate modulator on a silicon photonics platform exceeding 100 GHz 3-dB electrical modulation bandwidth. Opt. Express 2018, 26, 23728–23739. [Google Scholar] [CrossRef] [PubMed] [Green Version]



| Material | Refractive Index | Dielectric Constant | |
|---|---|---|---|
| Ordinary Refractive Index (no) | Extraordinary Refractive Index (ne) | ||
| LN [22] | 2.211 | 2.138 | 28.4 |
| SiO2 | 1.445 | 3.9 | |
| Si3N4 | 1.989 | 7.5 | |
| Type | Thickness (µm) | Width (µm) | Separation Distance (µm) |
|---|---|---|---|
| Type I | 0.3 | 1.532 | 2.629 |
| Type II | 0.3 | 1.46 | 2.336 |
| Structure | Experimental or Theoretical | Interaction Length (mm) | Vπ·L (V·cm) | Bandwidth (GHz) | References |
|---|---|---|---|---|---|
| SiNx-LNOI | Experimental | 12 | 3 | 8 | [10] |
| SiN-LNOI | Experimental | 8 | 3.1 | 33 | [34] |
| Si3N4-LNOI | Experimental | 7.8 | 2.24 | 80 | [35] |
| SiN-LNOI | Theoretical | 3 | 3.6 | 420 | [36] |
| LNOI-SiNx | Experimental | 5 | 6.67 | 30.6 | [37] |
| LN ridge | Experimental | 10 | 2.3 | 80 | [3] |
| LN ridge | Experimental | 3 | 2.2 | >70 | [33] |
| Si-LNOI | Experimental | 5 | 6.7 | >100 | [38] |
| Si-LNOI | Theoretical | 5 | 1.76 | 350 | [30] |
| Si3N4-LNOI (Type I) | Theoretical | 3 | 2.85 | 400 | This work |
| Si3N4-LNOI (Type I) | Theoretical | 10 | 2.85 | 83 | This work |
| Si3N4-LNOI (Type II) | Theoretical | 3 | 2.33 | 1260 | This work |
| Si3N4-LNOI (Type II) | 10 | 2.33 | 230 | This work |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Han, H.; Yang, F.; Liu, C.; Wang, Z.; Jiang, Y.; Chai, G.; Ruan, S.; Xiang, B. High-Performance Electro-Optical Mach–Zehnder Modulators in a Silicon Nitride–Lithium Niobate Thin-Film Hybrid Platform. Photonics 2022, 9, 500. https://doi.org/10.3390/photonics9070500
Han H, Yang F, Liu C, Wang Z, Jiang Y, Chai G, Ruan S, Xiang B. High-Performance Electro-Optical Mach–Zehnder Modulators in a Silicon Nitride–Lithium Niobate Thin-Film Hybrid Platform. Photonics. 2022; 9(7):500. https://doi.org/10.3390/photonics9070500
Chicago/Turabian StyleHan, Huangpu, Fan Yang, Chenghao Liu, Zhengfang Wang, Yunpeng Jiang, Guangyue Chai, Shuangchen Ruan, and Bingxi Xiang. 2022. "High-Performance Electro-Optical Mach–Zehnder Modulators in a Silicon Nitride–Lithium Niobate Thin-Film Hybrid Platform" Photonics 9, no. 7: 500. https://doi.org/10.3390/photonics9070500
APA StyleHan, H., Yang, F., Liu, C., Wang, Z., Jiang, Y., Chai, G., Ruan, S., & Xiang, B. (2022). High-Performance Electro-Optical Mach–Zehnder Modulators in a Silicon Nitride–Lithium Niobate Thin-Film Hybrid Platform. Photonics, 9(7), 500. https://doi.org/10.3390/photonics9070500






