Flexible Image Reconstruction in the Orbital Angular Momentum Holography with Binarized Airy Lens
Abstract
:1. Introduction
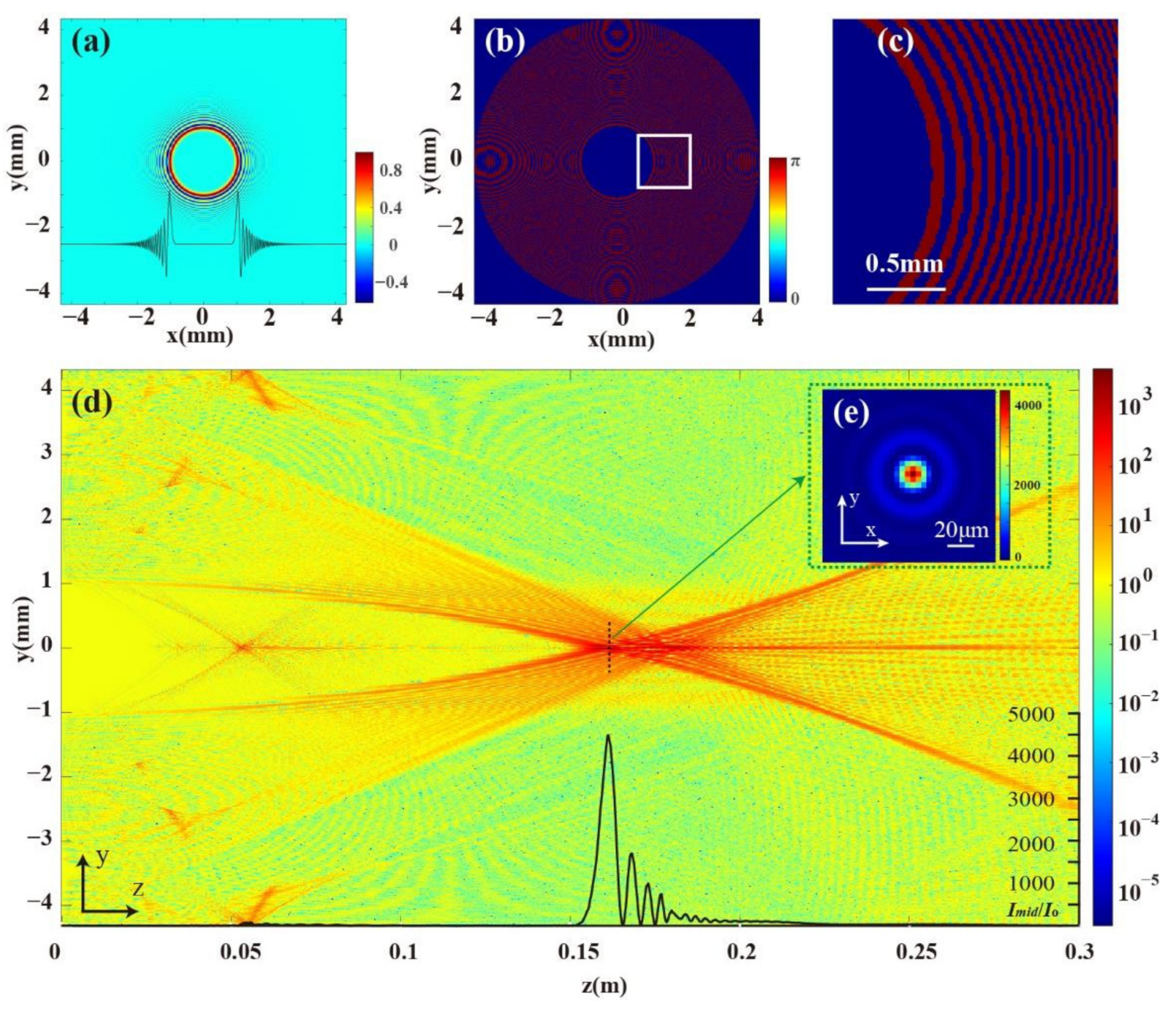
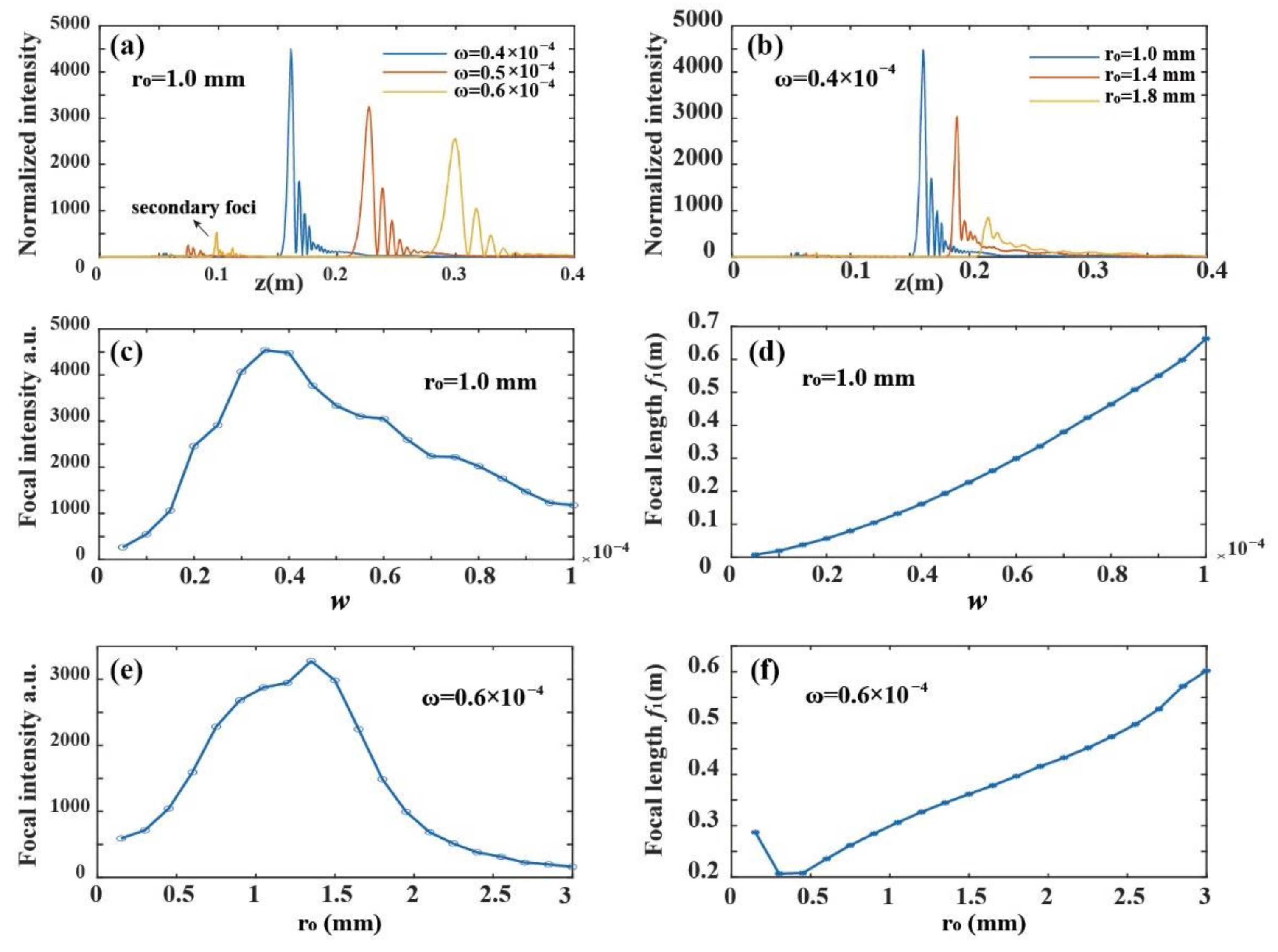
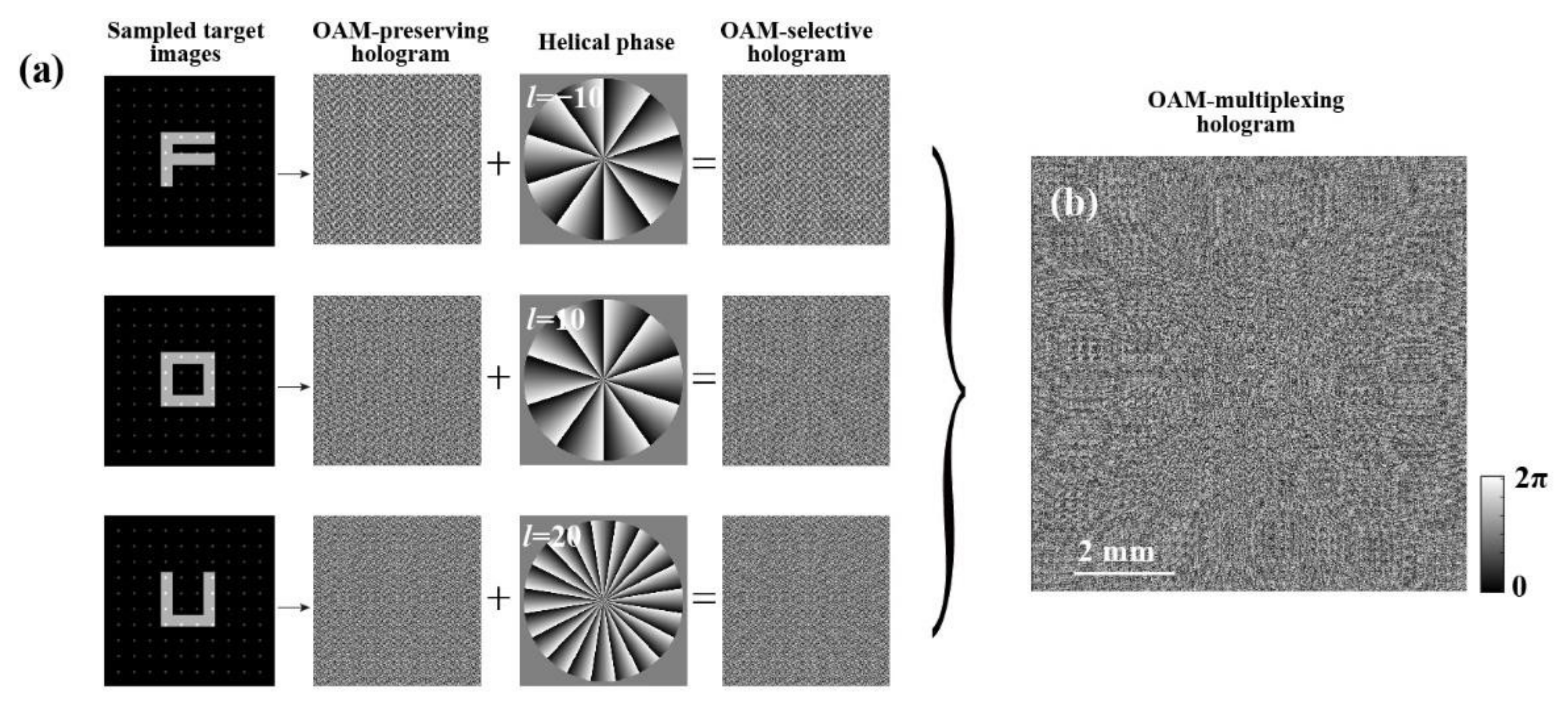
2. Principle and Implementation
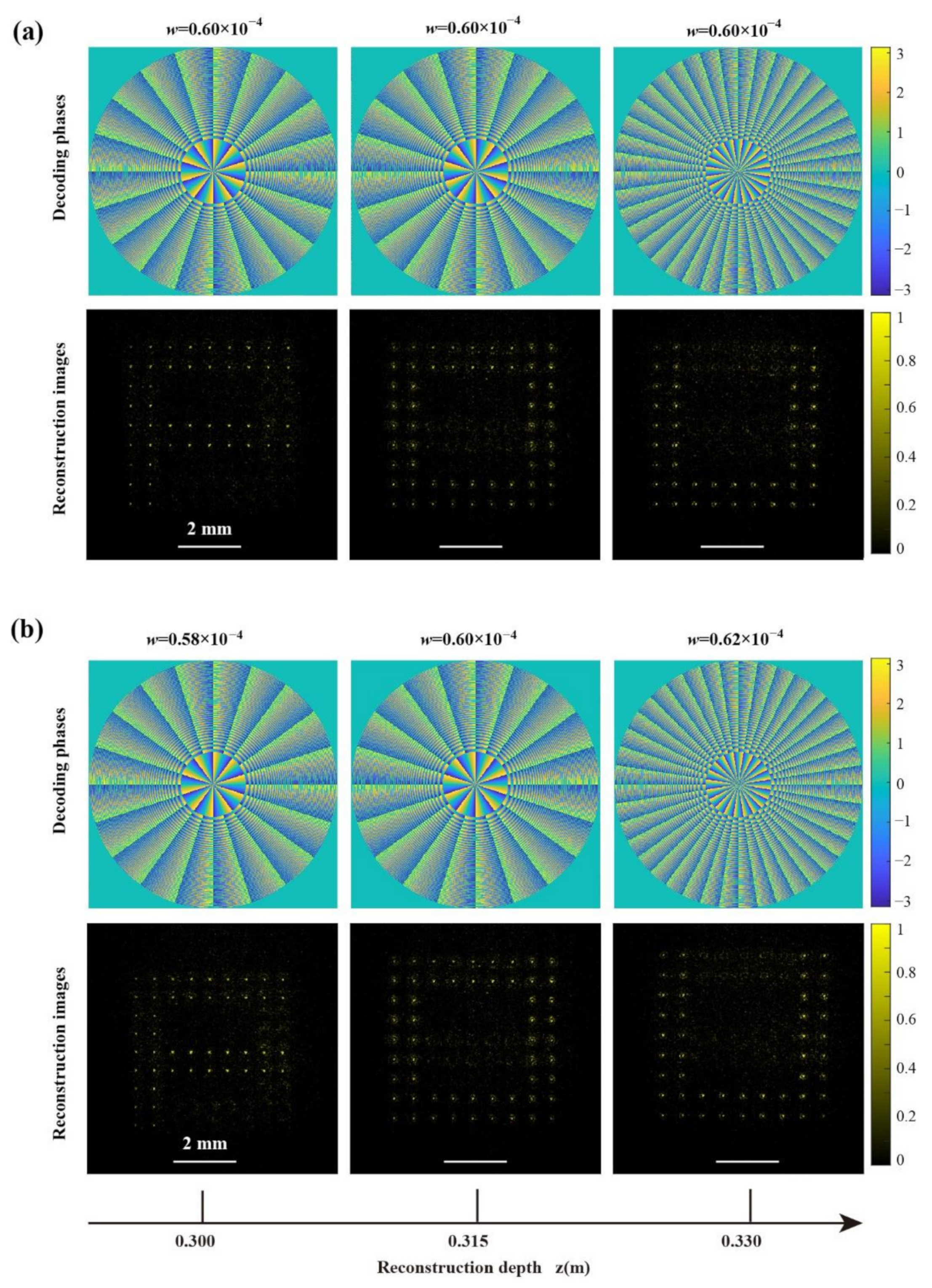
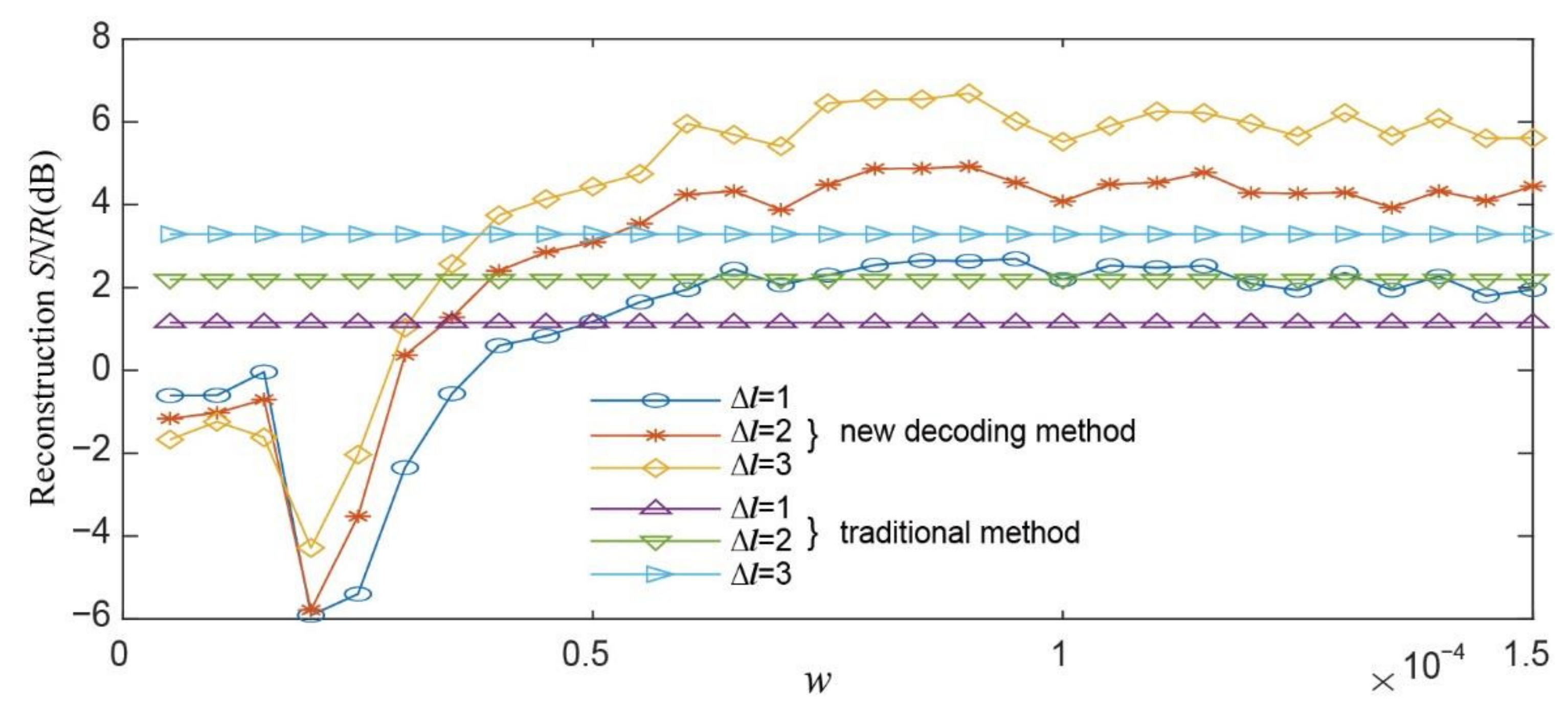
3. Experimental Demonstration
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Gabor, D. A New Microscopic Principle. Nature 1948, 161, 777–778. [Google Scholar] [CrossRef] [PubMed]
- Downing, E.; Hesselink, L.; Ralston, J.; Macfarlane, R. A Three-Color, Solid-State, Three-Dimensional Display. Science 1996, 273, 1185–1189. [Google Scholar] [CrossRef]
- Tay, S.; Blanche, P.A.; Voorakaranam, R.; Tunç, A.V.; Lin, W.; Rokutanda, S.; Gu, T.; Flores, D.; Wang, P.; Li, G.; et al. An Updatable Holographic Three-Dimensional Display. Nature 2008, 451, 694–698. [Google Scholar] [CrossRef] [PubMed]
- Grier, D.G. A Revolution in Optical Manipulation. Nature 2003, 424, 810–816. [Google Scholar] [CrossRef]
- Wang, J.; Yang, J.Y.; Fazal, I.M.; Ahmed, N.; Yan, Y.; Huang, H.; Ren, Y.; Yue, Y.; Dolinar, S.; Tur, M.; et al. Terabit Free-Space Data Transmission Employing Orbital Angular Momentum Multiplexing. Nat. Photonics 2012, 6, 488–496. [Google Scholar] [CrossRef]
- Willner, A.E.; Huang, H.; Yan, Y.; Ren, Y.; Ahmed, N.; Xie, G.; Bao, C.; Li, L.; Cao, Y.; Zhao, Z.; et al. Optical Communications Using Orbital Angular Momentum Beams. Adv. Opt. Photonics 2015, 7, 66–106. [Google Scholar] [CrossRef] [Green Version]
- Yao, A.M.; Padgett, M.J. Orbital Angular Momentum: Origins, Behavior and Applications. Adv. Opt. Photonics 2011, 3, 161–204. [Google Scholar] [CrossRef] [Green Version]
- Ren, H.; Briere, G.; Fang, X.; Ni, P.; Sawant, R.; Héron, S.; Chenot, S.; Vézian, S.; Damilano, B.; Brändli, V.; et al. Metasurface Orbital Angular Momentum Holography. Nat. Commun. 2019, 10, 102–108. [Google Scholar] [CrossRef]
- Fang, X.; Ren, H.; Gu, M. Orbital Angular Momentum Holography for High-Security Encryption. Nat. Photonics 2020, 14, 102–108. [Google Scholar] [CrossRef]
- Zhou, H.; Sain, B.; Wang, Y.; Schlickriede, C.; Zhao, R.; Zhang, X.; Wei, Q.; Li, X.; Huang, L.; Zentgraf, T. Polarization-Encrypted Orbital Angular Momentum Multiplexed Metasurface Holography. ACS Nano 2020, 14, 5553–5559. [Google Scholar] [CrossRef]
- Ren, H.; Shao, W.; Li, Y.; Salim, F.; Gu, M. Three-Dimensional Vectorial Holography Based on Machine Learning Inverse Design. Sci. Adv. 2020, 6, eaaz4261. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gu, M.; Fang, X.; Ren, H.; Goi, E. Optically Digitalized Holography: A Perspective for All-Optical Machine Learning. Engineering 2019, 5, 363–365. [Google Scholar] [CrossRef]
- Ren, H.; Fang, X.; Jang, J.; Bürger, J.; Rho, J.; Maier, S.A. Complex-Amplitude Metasurface-Based Orbital Angular Momentum Holography in Momentum Space. Nat. Nanotechnol. 2020, 15, 948–955. [Google Scholar] [CrossRef] [PubMed]
- Wang, F.; Zhang, X.; Yuan, H.; Xiong, R.; Jiang, X. Enhancing the Information Capacity with Modulated Orbital Angular Momentum Holography. IEEE Photonics J. 2022, 14, 3146189. [Google Scholar] [CrossRef]
- Fang, X.; Wang, H.; Yang, H.; Ye, Z.; Wang, Y.; Zhang, Y.; Hu, X.; Zhu, S.; Xiao, M. Multichannel Nonlinear Holography in a Two-Dimensional Nonlinear Photonic Crystal. Phys. Rev. A 2020, 102, 43506. [Google Scholar] [CrossRef]
- Fang, X.; Yang, H.; Yao, W.; Wang, T.; Zhang, Y.; Gu, M.; Xiao, M. High-Dimensional Orbital Angular Momentum Multiplexing Nonlinear Holography. Adv. Photonics 2021, 3, 015001. [Google Scholar] [CrossRef]
- Efremidis, N.K.; Christodoulides, D.N. Abruptly Autofocusing Waves. Opt. Lett. 2010, 35, 4045–4047. [Google Scholar] [CrossRef]
- Efremidis, N.K.; Chen, Z.; Segev, M.; Christodoulides, D.N. Airy Beams and Accelerating Waves: An Overview of Recent Advances. Optica 2019, 6, 686–701. [Google Scholar] [CrossRef] [Green Version]
- Jiang, Y.; Huang, K.; Lu, X. Propagation Dynamics of Abruptly Autofocusing Airy Beams with Optical Vortices. Opt. Express 2012, 20, 18579–18584. [Google Scholar] [CrossRef]
- Chremmos, I.; Zhang, P.; Prakash, J.; Efremidis, N.K.; Christodoulides, D.N.; Chen, Z. Fourier-Space Generation of Abruptly Autofocusing Beams and Optical Bottle Beams. Opt. Lett. 2011, 36, 3675–3677. [Google Scholar] [CrossRef]
- Zhang, P.; Prakash, J.; Zhang, Z.; Mills, M.S.; Efremidis, N.K.; Christodoulides, D.N.; Chen, Z. Trapping and Guiding Microparticles with Morphing Autofocusing Airy Beams. Opt. Lett. 2011, 36, 2883–2885. [Google Scholar] [CrossRef]
- Jiang, Y.; Huang, K.; Lu, X. Radiation Force of Abruptly Autofocusing Airy Beams on a Rayleigh Particle. Opt. Express 2013, 21, 24413–24421. [Google Scholar] [CrossRef]
- Panagiotopoulos, P.; Papazoglou, D.G.; Couairon, A.; Tzortzakis, S. Sharply Autofocused Ring-Airy Beams Transforming into Non-Linear Intense Light Bullets. Nat. Commun. 2013, 4, 2622. [Google Scholar] [CrossRef] [Green Version]
- Jiang, Y.; Zhu, X.; Yu, W.; Shao, H.; Zheng, W.; Lu, X. Propagation Characteristics of the Modified Circular Airy Beam. Opt. Express 2015, 23, 29834–29841. [Google Scholar] [CrossRef]
- Shen, D.; Zhao, D. Measuring the Topological Charge of Optical Vortices with a Twisting Phase. Opt. Lett. 2019, 44, 2334–2337. [Google Scholar] [CrossRef]
- Gerchberg, R.W.; Saxton, W. A Practical Algorithm for the Determination of Phase from Image and Diffraction Plane Pictures. Optik 1971, 35, 237–250. [Google Scholar]
- Dan, D.; Wang, Z.; Zhou, X.; Lei, M.; Zhao, T.; Qian, J.; Yu, X.; Yan, S.; Min, J.; Bianco, P.R.; et al. Rapid Image Reconstruction of Structured Illumination Microscopy Directly in the Spatial Domain. IEEE Photonics J. 2021, 13, 3900411. [Google Scholar] [CrossRef]
- Lei, X.; Ma, X.; Yang, Z.; Peng, X.; Yun, L.; Zhao, M.; Fan, M. Improving Compressed Sensing Image Reconstruction Based on Atmospheric Modulation Using the Distributed Cumulative Synthesis Method. IEEE Photonics J. 2021, 13, 3108194. [Google Scholar] [CrossRef]
- Liu, Z.; Shen, C.; Tan, J.; Liu, S. A Recovery Method of Double Random Phase Encoding System with a Parallel Phase Retrieval. IEEE Photonics J. 2016, 8, 2527699. [Google Scholar] [CrossRef]
- Gahagan, K.T.; Swartzlander, G.A. Optical Vortex Trapping of Particles. Opt. Lett. 1996, 21, 827–829. [Google Scholar] [CrossRef]
- Ng, J.; Lin, Z.; Chan, C.T. Theory of Optical Trapping by an Optical Vortex Beam. Phys. Rev. Lett. 2010, 104, 103601. [Google Scholar] [CrossRef] [Green Version]
- Jia, S.; Vaughan, J.C.; Zhuang, X. Isotropic Three-Dimensional Super-Resolution Imaging with a Self-Bending Point Spread Function. Nat. Photonics 2014, 8, 302–306. [Google Scholar] [CrossRef] [Green Version]


| Method | SNR/dB (Δl = 5) | SNR/dB (Δl = 10) | |
|---|---|---|---|
| Traditional | 4.39 | 8.36 | |
| BAL method | w = 0.6 × 10−4 | 8.19 | 10.21 |
| w = 0.8 × 10−4 | 8.96 | 11.06 | |
| w = 1.0 × 10−4 | 7.10 | 9.38 | |
| w = 1.2 × 10−4 | 7.98 | 11.43 | |
| w = 1.4 × 10−4 | 8.54 | 12.12 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, F.; Zhang, X.; Xiong, R.; Ma, X.; Yuan, H.; Li, L.; Jiang, X. Flexible Image Reconstruction in the Orbital Angular Momentum Holography with Binarized Airy Lens. Photonics 2022, 9, 460. https://doi.org/10.3390/photonics9070460
Wang F, Zhang X, Xiong R, Ma X, Yuan H, Li L, Jiang X. Flexible Image Reconstruction in the Orbital Angular Momentum Holography with Binarized Airy Lens. Photonics. 2022; 9(7):460. https://doi.org/10.3390/photonics9070460
Chicago/Turabian StyleWang, Feili, Xiangchao Zhang, Rui Xiong, Xinyang Ma, He Yuan, Leheng Li, and Xiangqian Jiang. 2022. "Flexible Image Reconstruction in the Orbital Angular Momentum Holography with Binarized Airy Lens" Photonics 9, no. 7: 460. https://doi.org/10.3390/photonics9070460
APA StyleWang, F., Zhang, X., Xiong, R., Ma, X., Yuan, H., Li, L., & Jiang, X. (2022). Flexible Image Reconstruction in the Orbital Angular Momentum Holography with Binarized Airy Lens. Photonics, 9(7), 460. https://doi.org/10.3390/photonics9070460






