Design Study of Broadband and Ultrahigh-Resolution Imaging Spectrometer Using Snapshot Multimode Interference in Fiber Bundles
Abstract
:1. Introduction
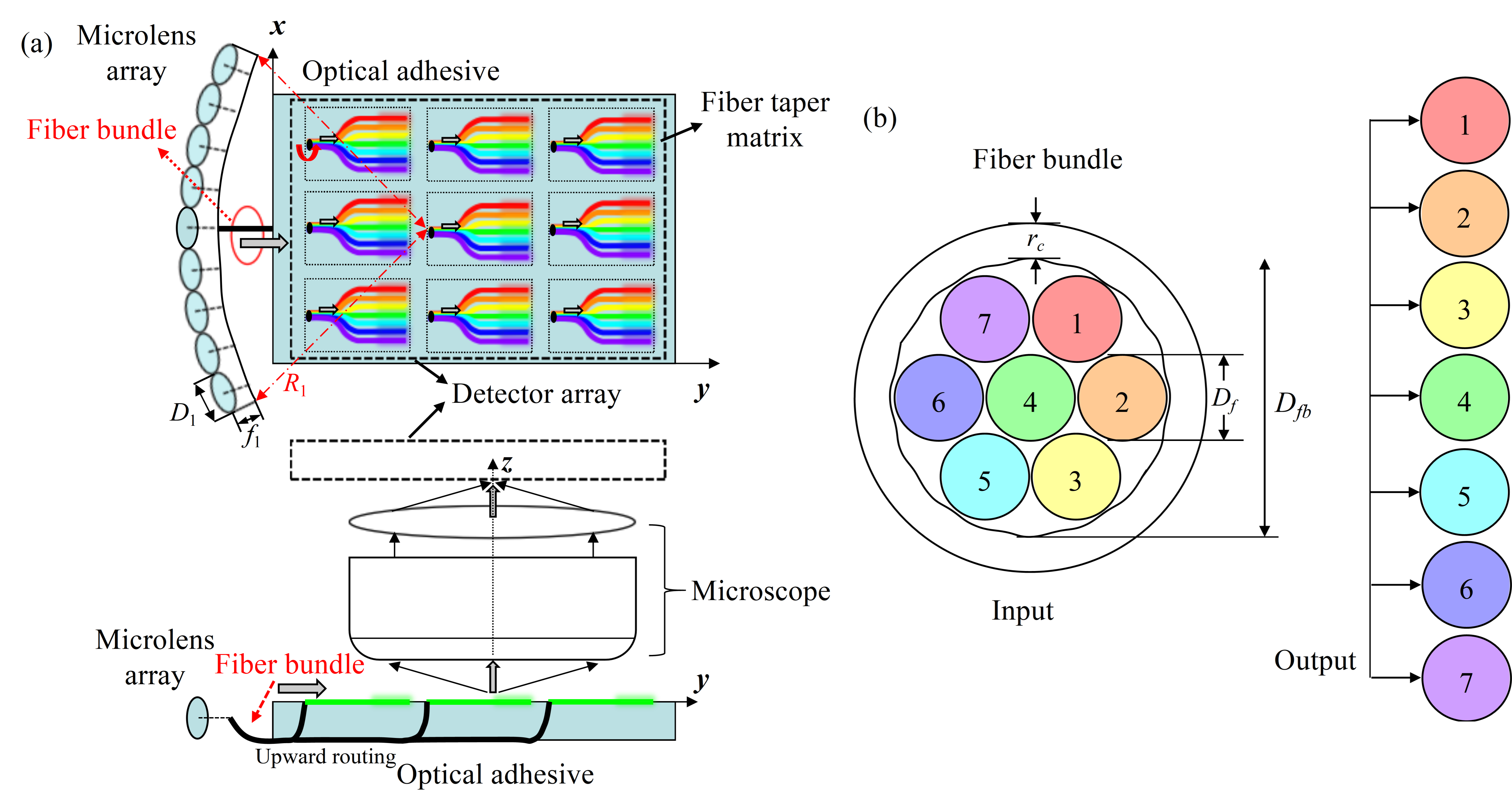
2. Optical Layout for the CBURIS
3. Operating Principles of the CBURIS
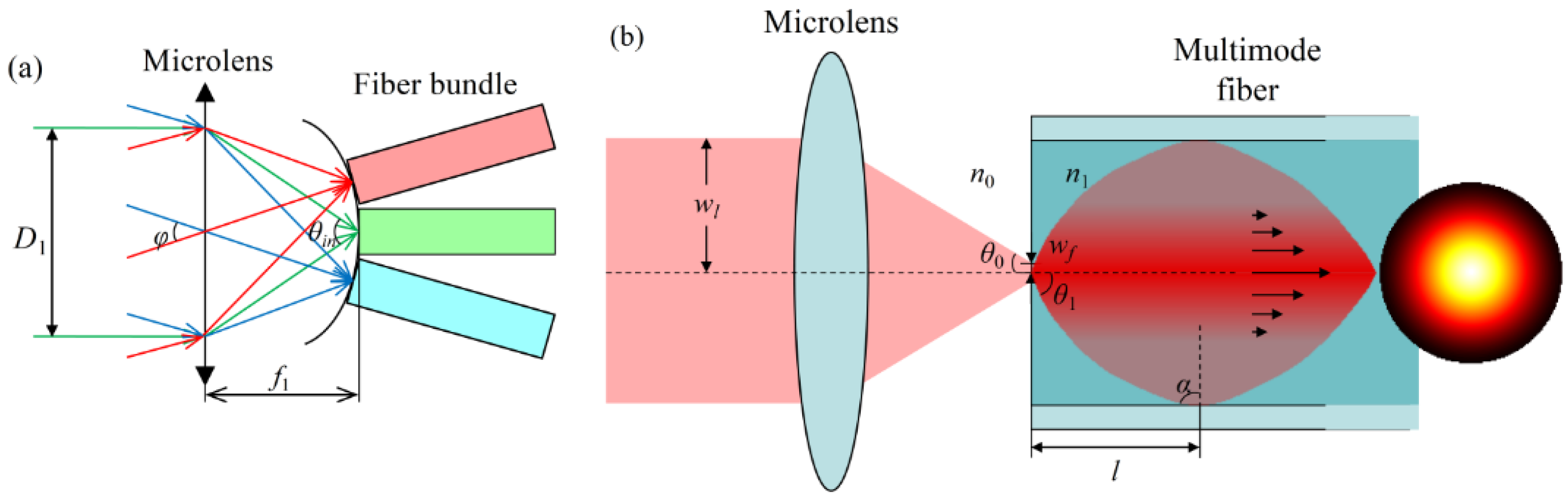
3.1. Coupled-Mode Theory from MLA to MMF
3.2. MMI Theory within the MMF
4. Analysis of the Spectral Performance
4.1. Simulation of MMI Model in Fiber
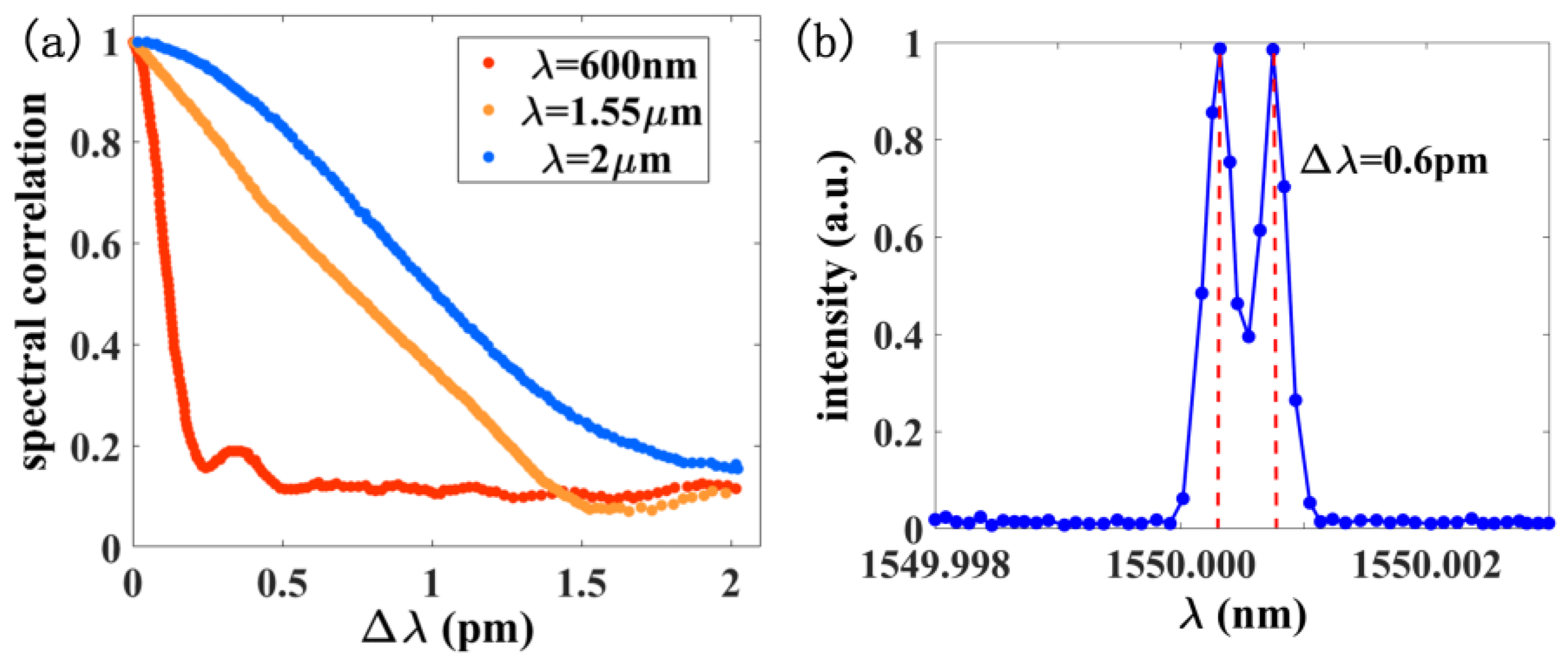
4.2. Ultra-Wide Bandwidth Calibration
4.3. Optimization for Ultrahigh Resolving Power
4.4. Operating Mode and Thermal Stability
5. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Goetz, A.F.; Vane, G.; Solomon, J.E.; Rock, B.N. Imaging Spectrometry for Earth Remote Sensing. Science 1985, 228, 1147–1153. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Pawlowski, M.E.; Cheng, S.; Dwight, J.G.; Stoian, R.I.; Lu, J.; Alexander, D.; Tkaczyk, T.S. Light-guide snapshot imaging spectrometer for remote sensing applications. Opt. Express 2019, 27, 15701–15725. [Google Scholar] [CrossRef] [PubMed]
- Peng, W.; Rajesh, M. Computational multispectral video imaging. J. Opt. Soc. Am. A 2018, 35, 189–199. [Google Scholar]
- Kato, T.; Uchida, M.; Tanaka, Y.; Minoshima, K. One-shot three-dimensional imaging using a two-dimensional spectrometer with a fiber bundle. Opt. Express 2021, 29, 43778–43792. [Google Scholar] [CrossRef]
- Flynn, C.; Stoian, R.I.; Weers, B.D.; Mullet, J.E.; Thomasson, J.A.; Alexander, D.; Tkaczyk, T.S. Ruggedized, field-ready snapshot light-guide-based imaging spectrometer for environmental and remote sensing applications. Opt. Express 2022, 30, 10614–10632. [Google Scholar] [CrossRef]
- Palombi, L.; Raimondi, V. A compact LIF spectrometer for in-field operation in polar environments. Sensors 2021, 21, 1729. [Google Scholar] [CrossRef]
- Uddin, N.; Sheng, Q.; Lee, S.; Reinke, M.L.; Donovan, D.; Shafer, M.; Han, M. Multichannel fiber-optic silicon Fabry-Perot interferometric bolometer system for plasma radiation measurements. Photonics 2021, 8, 344. [Google Scholar] [CrossRef]
- Malara, P.; Giorgini, A.; Avino, S.; Sarno, V.D.; Aiello, R.; Maddaloni, P.; Natale, P.D.; Gagliardi, G. A self-operating broadband spectrometer on a droplet. Nat. Commun. 2020, 11, 2263. [Google Scholar] [CrossRef]
- Korablev, O.I.; Belyaev, D.A.; Dobrolenskiy, Y.S.; Trokhimovskiy, A.Y.; Kalinnikov, Y.K. Acousto-optic tunable filter spectrometers in space missions. Appl. Opt. 2018, 57, C103–C119. [Google Scholar] [CrossRef]
- Sahin, F.E.; Tanguay, A.R. Distortion optimization for wide-angle computational cameras. Opt. Express 2018, 26, 5478–5487. [Google Scholar] [CrossRef]
- Jullien, A.; Pascal, R.; Bortolozzo, U.; Forget, N.; Residori, S. High-resolution hyperspectral imaging with cascaded liquid crystal cells. Optica 2017, 4, 400–405. [Google Scholar] [CrossRef]
- August, I.; Oiknine, Y.; AbuLeil, M.; Stern, A. Miniature compressive ultra-spectral imaging system utilizing a single liquid crystal phase retarder. Sci. Rep. 2016, 6, 23524. [Google Scholar] [CrossRef] [PubMed]
- Yang, Q. Moving corner-cube-mirror interferometer and reflection characteristic of corner-cube mirror. Appl. Opt. 2010, 49, 4088–4095. [Google Scholar] [CrossRef]
- Zucco, M.; Pisani, M.; Caricato, V.; Egidi, A. A hyperspectral imager based on a Fabry-Perot interferometer with dielectric mirrors. Opt. Express 2014, 22, 1824–1834. [Google Scholar] [CrossRef] [PubMed]
- Pisani, M.; Zucco, M. Compact imaging spectrometer combining Fourier transform spectroscopy with a Fabry-Perot interferometer. Opt. Express 2009, 17, 8319–8331. [Google Scholar] [CrossRef] [PubMed]
- Yang, Q. Design study of a compact ultra-wide-angle high-spatial-resolution high-spectral-resolution snapshot imaging spectrometer. Opt. Express 2021, 29, 2893–2908. [Google Scholar] [CrossRef] [PubMed]
- Liu, J.; Chen, J.; Liu, J.; Feng, S.; Li, X.; Cui, J. Optical design of a prism-grating-based lenslet array integral field spectrometer. Opt. Express 2018, 26, 19456–19469. [Google Scholar] [CrossRef]
- Redding, B.; Alam, M.; Seifert, M.; Cao, H. High-resolution and broadband all-fiber spectrometers. Optica 2014, 1, 175–180. [Google Scholar] [CrossRef]
- Redding, B.; Cao, H. Using a multimode fiber as high-resolution, low-loss spectrometer. Opt. Lett. 2012, 37, 3384–3386. [Google Scholar] [CrossRef]
- Wang, J.; Yan, S.; Ruan, Y.; Xu, F.; Lu, Y. Fiber-optic point-based sensor using specklegram measurement. Sensors 2017, 17, 2429. [Google Scholar] [CrossRef] [Green Version]
- Chanclou, P.; Kaczmarek, C.; Mouzer, G.; Gravey, P.; Thual, M.; Lecollinet, M.A.; Rochard, P. Expanded single-mode fiber using graded index multimode fiber. Opt. Eng. 2004, 43, 1634–1642. [Google Scholar]
- Redding, B.; Popoff, S.M.; Cao, H. All-fiber spectrometer based on speckle pattern reconstruction. Opt. Express 2013, 21, 6584–6600. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, Z.; Yu, Z. Spectral analysis based on compressive sensing in nanophotonic structures. Opt. Express 2014, 22, 25608–25614. [Google Scholar] [CrossRef] [PubMed]
- Redding, B.; Liew, S.F.; Bromberg, Y.; Sarma, B.; Cao, H. Evanescently coupled multimode spiral spectrometer. Optica 2016, 3, 956–962. [Google Scholar] [CrossRef] [Green Version]
- Meng, F.; Zhao, Y.; Wang, H.; Zhang, Y. High-performance compact spectrometer based on multimode interference in a tapered spiral-shaped waveguide. Opt. Express 2019, 27, 38349–38358. [Google Scholar] [CrossRef]
- Xu, Z.; Wang, Z.; Sullivan, M.; Brady, D.; Adibi, A. Multimodal multiplex spectroscopy using photonic crystals. Opt. Express 2003, 11, 2126–2133. [Google Scholar] [CrossRef]




| Operating Bandwidth (nm) | Spectral Resolution (nm) | Spatial Resolution (⁰) | Ref. |
|---|---|---|---|
| 450–750 | 10 | 0.06 | [2] |
| 1520–1620 | 0.2 | 0.97 | [4] |
| 470–700 | 20 | 0.12 | [5] |
| 498.5–501.5 | 0.005 | 0.10 | [16] |
| 400–2200 | 0.0006 | 0.17 | CBURIS |
| Symbol | Description | Value |
|---|---|---|
| λ1–λ2 | wavelength range | 0.4–2.4 μm |
| DFB | diameter of MMFB | 0.67 mm |
| Df | diameter of MMF | 200 μm |
| NA | numerical aperture of MMF | 0.39 |
| n1 | refractive index of SiO2 | 1.45 |
| n0 | refractive index of air | 1.00 |
| Δ | relative variation of refractive index | 0.02 |
| L | length of MMF | 0.1 m |
| Symbol | Description | Value |
| R1 | radius of the MLA | 35 mm |
| D1 | size of the micorlens | 300 μm |
| L | length of the fiber bundle | 0.1 m |
| FOV | field of view | 120° |
| φ′ | spatial resolution | 0.17° |
| operation bandwidth | 1.8 μm | |
| RP (Δλ) | resolving power (spectral resolution) | 2.58 × 106(0.6 pm)@1.55 μm |
| V | footprint | 600 cm3 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meng, F.; Liu, Z.; Wu, P.; Feng, W.; Cui, J. Design Study of Broadband and Ultrahigh-Resolution Imaging Spectrometer Using Snapshot Multimode Interference in Fiber Bundles. Photonics 2022, 9, 334. https://doi.org/10.3390/photonics9050334
Meng F, Liu Z, Wu P, Feng W, Cui J. Design Study of Broadband and Ultrahigh-Resolution Imaging Spectrometer Using Snapshot Multimode Interference in Fiber Bundles. Photonics. 2022; 9(5):334. https://doi.org/10.3390/photonics9050334
Chicago/Turabian StyleMeng, Fan, Zefang Liu, Pengfei Wu, Weiwei Feng, and Jiangong Cui. 2022. "Design Study of Broadband and Ultrahigh-Resolution Imaging Spectrometer Using Snapshot Multimode Interference in Fiber Bundles" Photonics 9, no. 5: 334. https://doi.org/10.3390/photonics9050334
APA StyleMeng, F., Liu, Z., Wu, P., Feng, W., & Cui, J. (2022). Design Study of Broadband and Ultrahigh-Resolution Imaging Spectrometer Using Snapshot Multimode Interference in Fiber Bundles. Photonics, 9(5), 334. https://doi.org/10.3390/photonics9050334





