AC/DC: The FERMI FEL Split and Delay Optical Device for Ultrafast X-ray Science
Abstract
:1. Introduction
2. Materials and Methods
3. Results
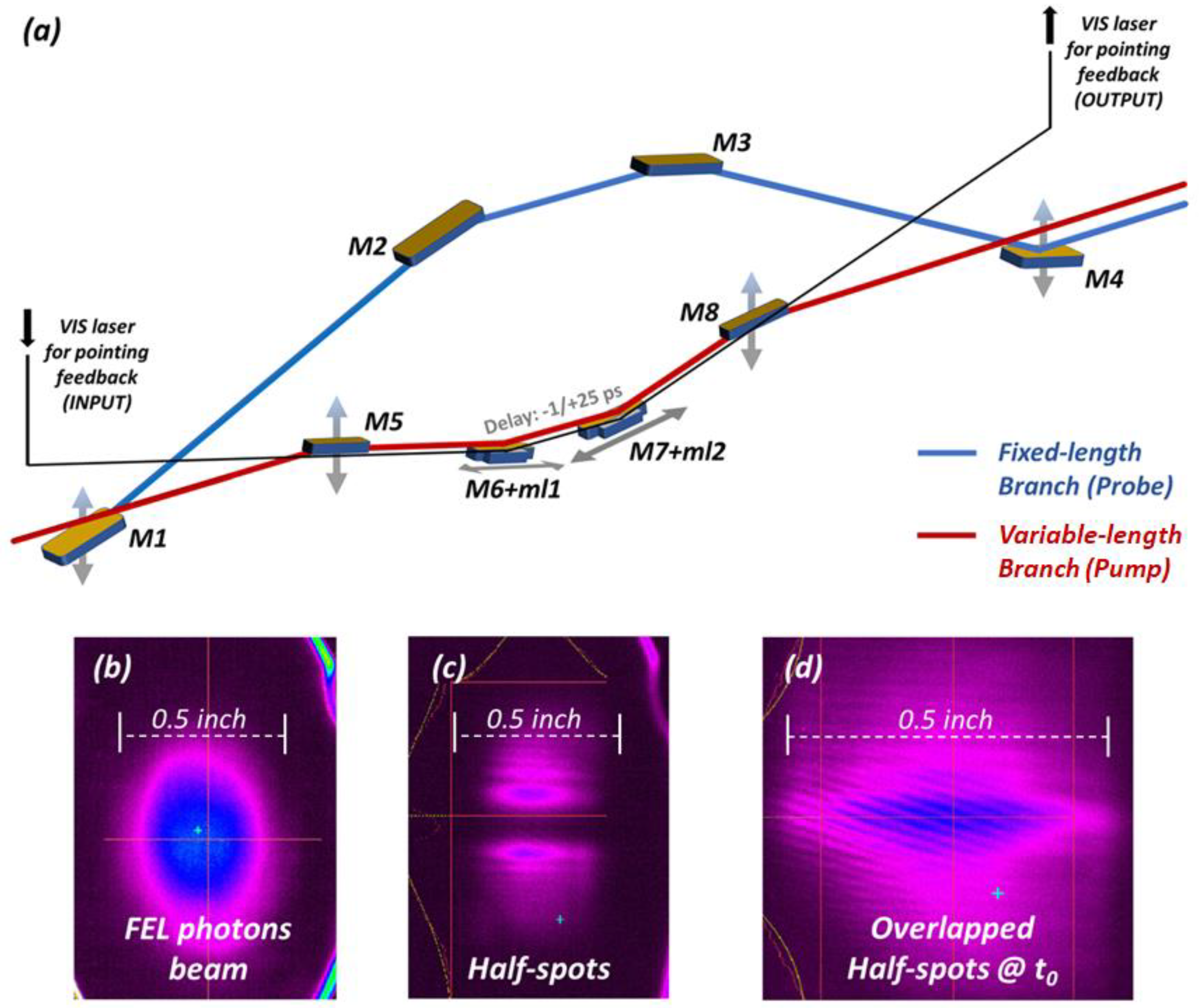
3.1. Alignment
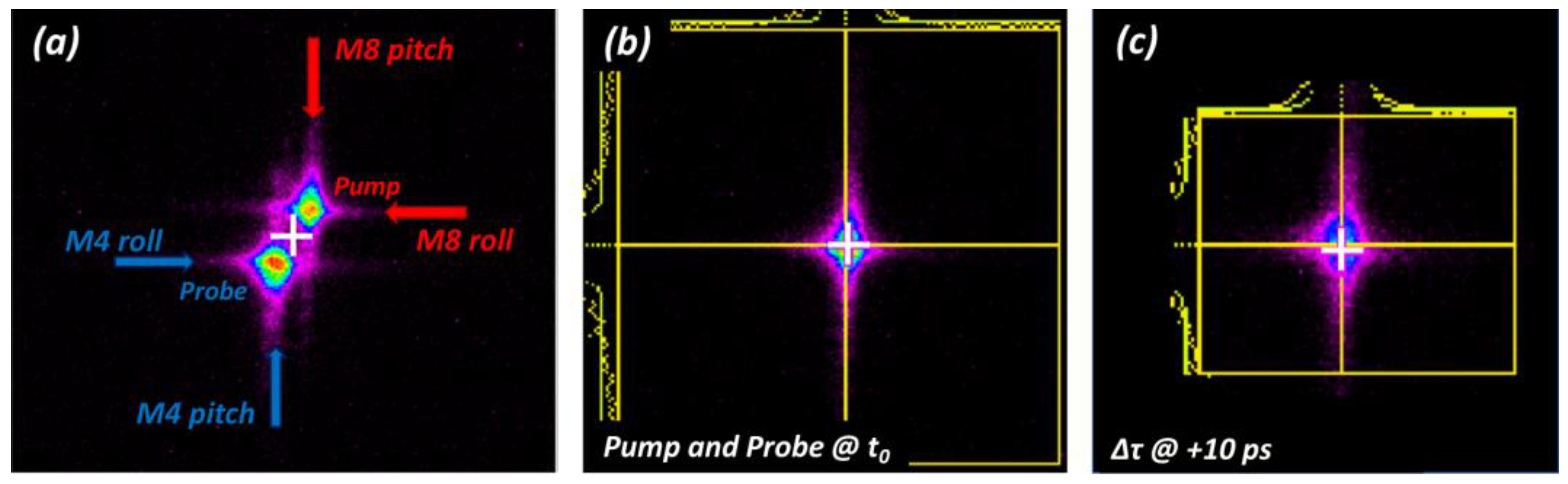
3.2. Pointing Instability and Feedback System
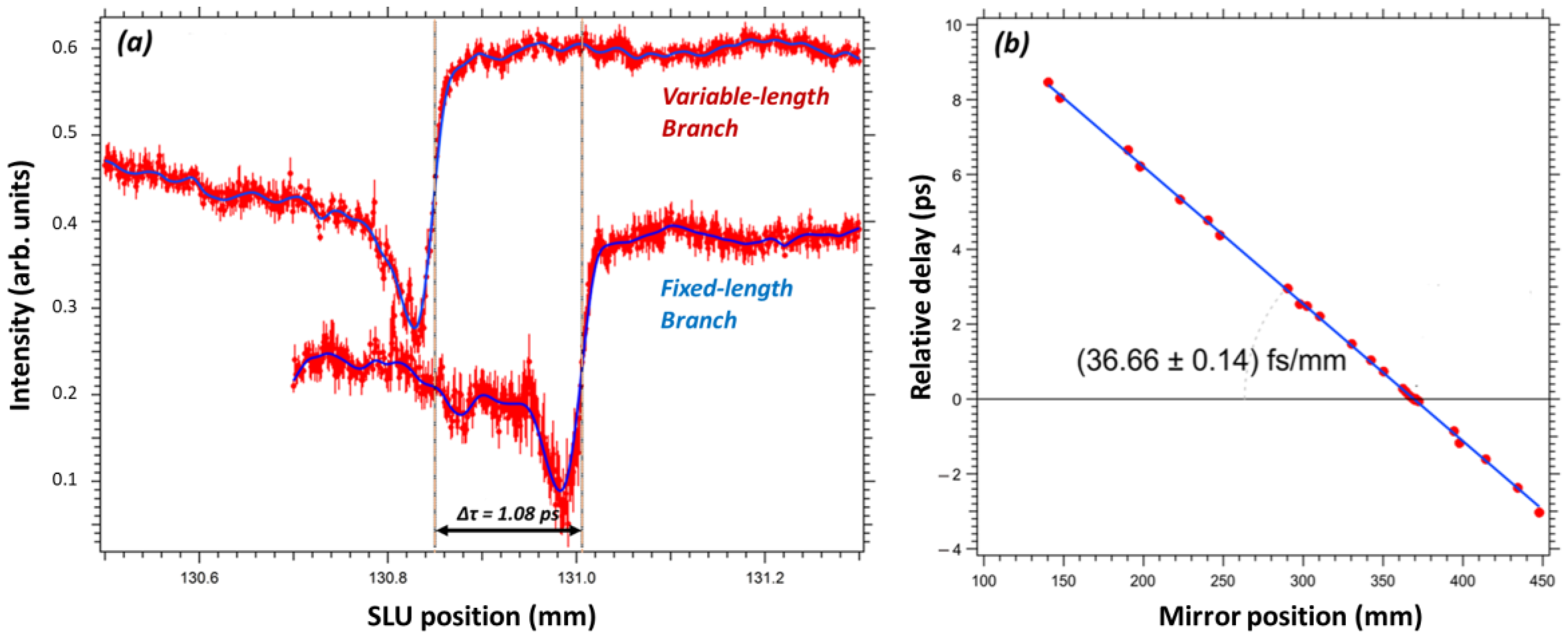
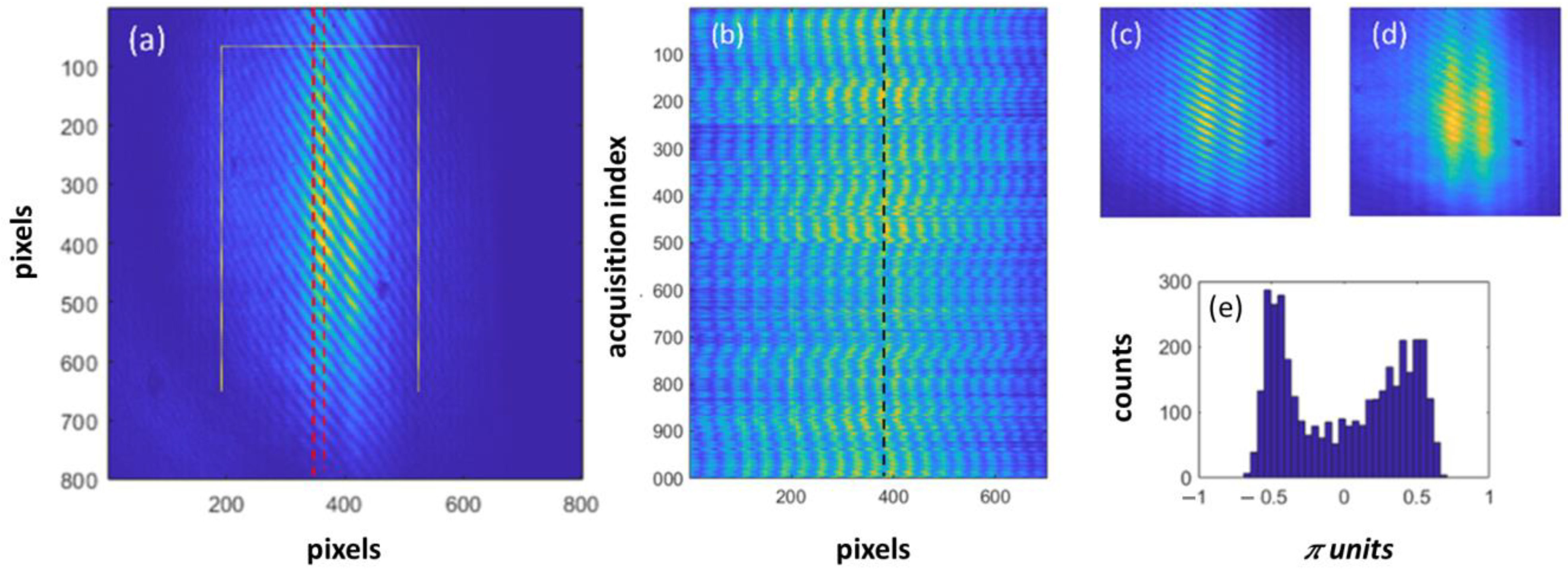
3.3. Fringe Stability
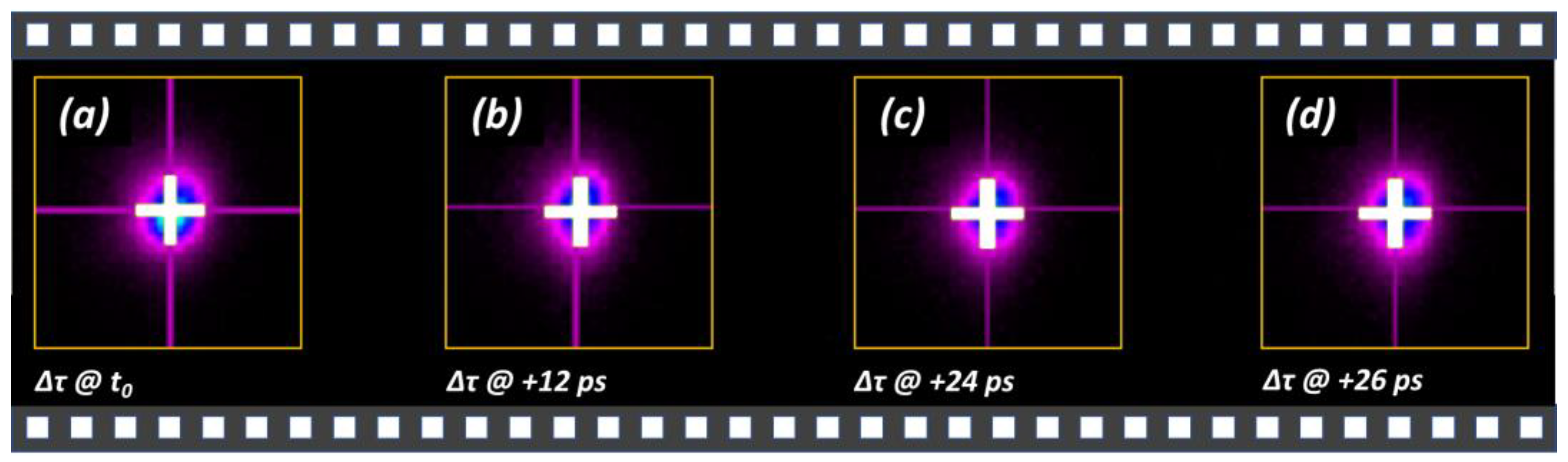
3.4. The Time-Dependent Amplitude-Autocorrelation
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Pellegrini, C.; Marinelli, A.; Reiche, S. The physics of x-ray free electron lasers. Rev. Mod. Phys. 2016, 88, 015006. [Google Scholar] [CrossRef]
- Bostedt, C.; Boutet, S.; Fritz, D.M.; Huang, Z.; Lee, H.J.; Lemke, H.T.; Robert, A.; Schlotter, W.F.; Turner, J.J.; Williams, G.J. Linac Coherent Light Source: The first five years. Rev. Mod. Phys. 2016, 88, 015007. [Google Scholar] [CrossRef] [Green Version]
- Schreiber, S. First Lasing at 32 nm of the VUV-FEL at DESY. In Proceedings of the 27th International Free Electron Laser Conference (FEL 2005), Stanford, CA, USA, 21–26 August 2005; pp. 12–18. [Google Scholar]
- Chalupský, J.; Krzywinski, J.; Juha, L.; Hájková, V.; Cihelka, J.; Burian, T.; Vyšín, L.; Gaudin, J.; Gleeson, A.; Jurek, M.; et al. Spot size characterization of focused non-Gaussian X-ray laser beams. Opt. Express 2010, 18, 27836. [Google Scholar] [CrossRef] [PubMed]
- Grguraš, I.; Maier, A.R.; Behrens, C.; Mazza, T.; Kelly, T.J.; Radcliffe, P.; Düsterer, S.; Kazansky, A.K.; Kabachnik, N.M.; Tschentscher, T.; et al. Ultrafast X-ray pulse characterization at free-electron lasers. Nat. Photonics 2012, 6, 852. [Google Scholar] [CrossRef]
- Schulz, S.; Grguras, I.; Behrens, C.; Bromberger, H.; Costello, J.T.; Czwalinna, M.K.; Felber, M.; Hoffmann, M.C.; Ilchen, M.; Liu, H.Y.; et al. Femtosecond all-optical synchronization of an X-ray free-electron laser. Nat. Commun. 2015, 6, 5938. [Google Scholar] [CrossRef] [Green Version]
- Allaria, E.; Appio, R.; Badano, L.; Barletta, W.A.; Bassanese, S.; Biedron, S.G.; Borga, A.; Busetto, E.; Castronovo, D.; Cinquegrana, P.; et al. Highly coherent and stable pulses from the FERMI seeded free-electron laser in the extreme ultraviolet. Nat. Photonics 2012, 6, 699–704. [Google Scholar] [CrossRef]
- Allaria, E.M.; Diviacco, B.; Callegari, C.; Finetti, P.; Mahieu, B.; Viefhaus, J.; Zangrando, M.; De Ninno, G.; Lambert, G.; Ferrari, E.; et al. Control of the Polarization of a Vacuum-Ultraviolet, High-Gain, Free-Electron Laser. Phys. Rev. X 2014, 4, 41040. [Google Scholar] [CrossRef] [Green Version]
- Allaria, E.M.; Battistoni, A.; Bencivenga, F.; Borghes, R.; Callegari, C.; Capotondi, F.; Castronovo, D.; Cinquegrana, P.; Cocco, D.; Coreno, M.; et al. Tunability experiments at the FERMI@Elettra free-electron laser. New J. Phys. 2012, 14, 113009. [Google Scholar] [CrossRef]
- Dell’Angela, M.; Hieke, F.; Malvestuto, M.; Sturari, L.; Bajt, S.; Kozhevnikov, I.V.; Ratanapreechachai, J.; Caretta, A.; Casarin, B.; Glerean, F.; et al. Extreme ultraviolet resonant inelastic X-ray scattering (RIXS) at a seeded free-electron laser. Sci. Rep. 2016, 6, 38796. [Google Scholar] [CrossRef] [Green Version]
- Prince, K.C.; Allaria, E.; Callegari, C.; Cucini, R.; de Ninno, G.; di Mitri, S.; Diviacco, B.; Ferrari, E.; Finetti, P.; Gauthier, D.; et al. Coherent control with a short-wavelength free-electron laser. Nat. Photonics 2016, 10, 176–179. [Google Scholar] [CrossRef]
- Principi, E.; Krylow, S.; Garcia, M.E.; Simoncig, A.; Foglia, L.; Mincigrucci, R.; Kurdi, G.; Gessini, A.; Bencivenga, F.; Giglia, A.; et al. Atomic and electronic structure of solid-density liquid carbon. Phys. Rev. Lett. 2020, 125, 155703. [Google Scholar] [CrossRef] [PubMed]
- Lu, W.; Friedrich, B.; Noll, T.; Zhou, K.; Hallmann, J.; Ansaldi, G.; Roth, T.; Serkez, S.; Geloni, G.; Madsen, A.; et al. Development of a hard X-ray split-and-delay line and performance simulations for two-color pump-probe experiments at the European XFEL. Rev. Sci. Instrum. 2018, 89, 063121. [Google Scholar] [CrossRef] [PubMed]
- Roseker, W.; Hruszkewycz, S.O.; Lehmkühler, F.; Walther, M.; Schulte-Schrepping, H.; Lee, S.; Osaka, T.; Strüder, L.; Hartmann, R.; Sikorski, M.; et al. Towards ultrafast dynamics with split-pulse X-ray photon correlation spectroscopy at free electron laser sources. Nat. Commun. 2018, 9, 1704. [Google Scholar] [CrossRef] [PubMed]
- Mandal, A.; Sidhu, M.S.; Rost, J.M.; Pfeifer, T.; Singh, K.P. Attosecond delay lines: Design, characterization and applications. Eur. Phys. J. Spec. Top. 2021, 230, 4195. [Google Scholar] [CrossRef]
- Zangrando, M.; Cudin, I.; Fava, C.; Gerusina, S.; Gobessi, R.; Godnig, R.; Rumiz, L.; Svetina, C.; Parmigiani, F.; Cocco, D. First Results from the Commissioning of the FERMI@Elettra Free Electron Laser by Means of the Photon Analysis Delivery and Reduction System (PADReS). Proc. SPIE 2011, 8078, 80780I. [Google Scholar]
- Allaria, E.; Bencivenga, F.; Borghes, R.; Capotondi, F.; Castronovo, D.; Charalambous, P.; Cinquegrana, P.; Danailov, M.B.; de Ninno, G.; Demidovich, A.; et al. Two-colour pump–probe experiments with a twin-pulse-seed extreme ultraviolet free-electron laser. Nat. Commun. 2013, 4, 2476. [Google Scholar] [CrossRef]
- Danailov, M.B.; Bencivenga, F.; Capotondi, F.; Casolari, F.; Cinquegrana, P.; Demidovich, A.; Giangrisostomi, E.; Kiskinova, M.P.; Kurdi, G.; Manfredda, M.; et al. Towards jitter-free pump-probe measurements at seeded free electron laser facilities. Opt. Express 2014, 22, 12869–12879. [Google Scholar] [CrossRef]
- Weaver, J.H.; Krafka, C.; Lynch, D.W.; Koch, E.E. Physics Data: Optical Properties of Metals, 18; Fach-Iformation Zentrum: Karlsruhe, Germany, 1981. [Google Scholar]
- Center for X-Rays Optics Database. Available online: https://henke.lbl.gov/optical_constants/ (accessed on 31 December 2021).
- Raimondi, L.; Svetina, C.; Mahne, N.; Cocco, D.; Abrami, A.; de Marco, M.; Fava, C.; Gerusina, S.; Gobessi, R.; Capotondi, F.; et al. Microfocusing of the FERMI@Elettra FEL beam with a K-B active optics system: Spot size predictions by application of the WISE code. Nucl. Instrum. Meth. A 2013, 710, 131–138. [Google Scholar] [CrossRef]
- Raimondi, L.; Svetina, C.; Mahne, N.; Cocco, D.; Capotondi, F.; Pedersoli, E.; Kiskinova, M.; Keitel, B.; Brenner, G.; Ploenjes, E.; et al. K-B bendable system optimization at FERMI@Elettra FEL: Impact of different spatial wavelengths on the spot size. Proc. SPIE 2013, 8848, 63–70. [Google Scholar]
- Manfredda, M.; Fava, C.; Gobessi, S.G.R.; Mahne, N.; Raimondi, L.; Simoncig, A.; Zangrando, M. The evolution of KAOS, a multipurpose active optics system for EUV/Soft X-rays. Syn. Rad. News 2022. accepted. [Google Scholar]
- Menk, R.H.; Arfelli, F.; Cautero, M.; Cautero, G.; di Fraia, M.; Coreno, M.; Galdenzi, F.; Tutsch, W. On the possibility to utilize a PCO Edge 4.2 bi scientific CMOS imager for extended ultra violet and soft X-ray photon detection. JINST 2022, 17, C01058. [Google Scholar] [CrossRef]
- Capotondi, F.; Foglia, L.; Kiskinova, M.P.; Masciovecchio, C.; Mincigrucci, R.; Naumenko, D.; Pedersoli, E.; Simoncig, A.; Bencivenga, F. Characterization of ultrafast free-electron laser pulses using extreme-ultraviolet transient gratings. J. Synchrotron Rad. 2018, 25, 32. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Hartmann, N.; Hartmann, G.; Heider, R.; Wagner, M.S.; Ilchen, M.; Buck, J.; Lindahl, A.O.; Benko, C.; Grünert, J.; Krzywinski, J.; et al. Attosecond time–energy structure of X-ray free-electron laser pulses. Nat. Photonics 2018, 12, 215. [Google Scholar] [CrossRef]
- Ackermann, W.A.; Asova, G.; Ayvazyan, V.; Azima, A.; Baboi, N.; Bähr, J.; Balandin, V.; Beutner, B.; Brandt, A.; Bolzmann, A.; et al. Operation of a free-electron laser from the extreme ultraviolet to the water window. Nat. Photonics 2007, 1, 336–342. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Simoncig, A.; Manfredda, M.; Gaio, G.; Mahne, N.; Raimondi, L.; Fava, C.; Gerusina, S.; Gobessi, R.; Abrami, A.; Capotondi, F.; et al. AC/DC: The FERMI FEL Split and Delay Optical Device for Ultrafast X-ray Science. Photonics 2022, 9, 314. https://doi.org/10.3390/photonics9050314
Simoncig A, Manfredda M, Gaio G, Mahne N, Raimondi L, Fava C, Gerusina S, Gobessi R, Abrami A, Capotondi F, et al. AC/DC: The FERMI FEL Split and Delay Optical Device for Ultrafast X-ray Science. Photonics. 2022; 9(5):314. https://doi.org/10.3390/photonics9050314
Chicago/Turabian StyleSimoncig, Alberto, Michele Manfredda, Giulio Gaio, Nicola Mahne, Lorenzo Raimondi, Claudio Fava, Simone Gerusina, Riccardo Gobessi, Alessandro Abrami, Flavio Capotondi, and et al. 2022. "AC/DC: The FERMI FEL Split and Delay Optical Device for Ultrafast X-ray Science" Photonics 9, no. 5: 314. https://doi.org/10.3390/photonics9050314
APA StyleSimoncig, A., Manfredda, M., Gaio, G., Mahne, N., Raimondi, L., Fava, C., Gerusina, S., Gobessi, R., Abrami, A., Capotondi, F., De Angelis, D., Menk, R. H., Pancaldi, M., Pedersoli, E., & Zangrando, M. (2022). AC/DC: The FERMI FEL Split and Delay Optical Device for Ultrafast X-ray Science. Photonics, 9(5), 314. https://doi.org/10.3390/photonics9050314






