Accelerated Generation of a Pinhole-Type Holographic Stereogram Based on Human Eye Characteristics in Near-Eye Displays
Abstract
:1. Introduction
2. Materials and Methods
2.1. Pinhole Type HS
2.2. Proposed Methods
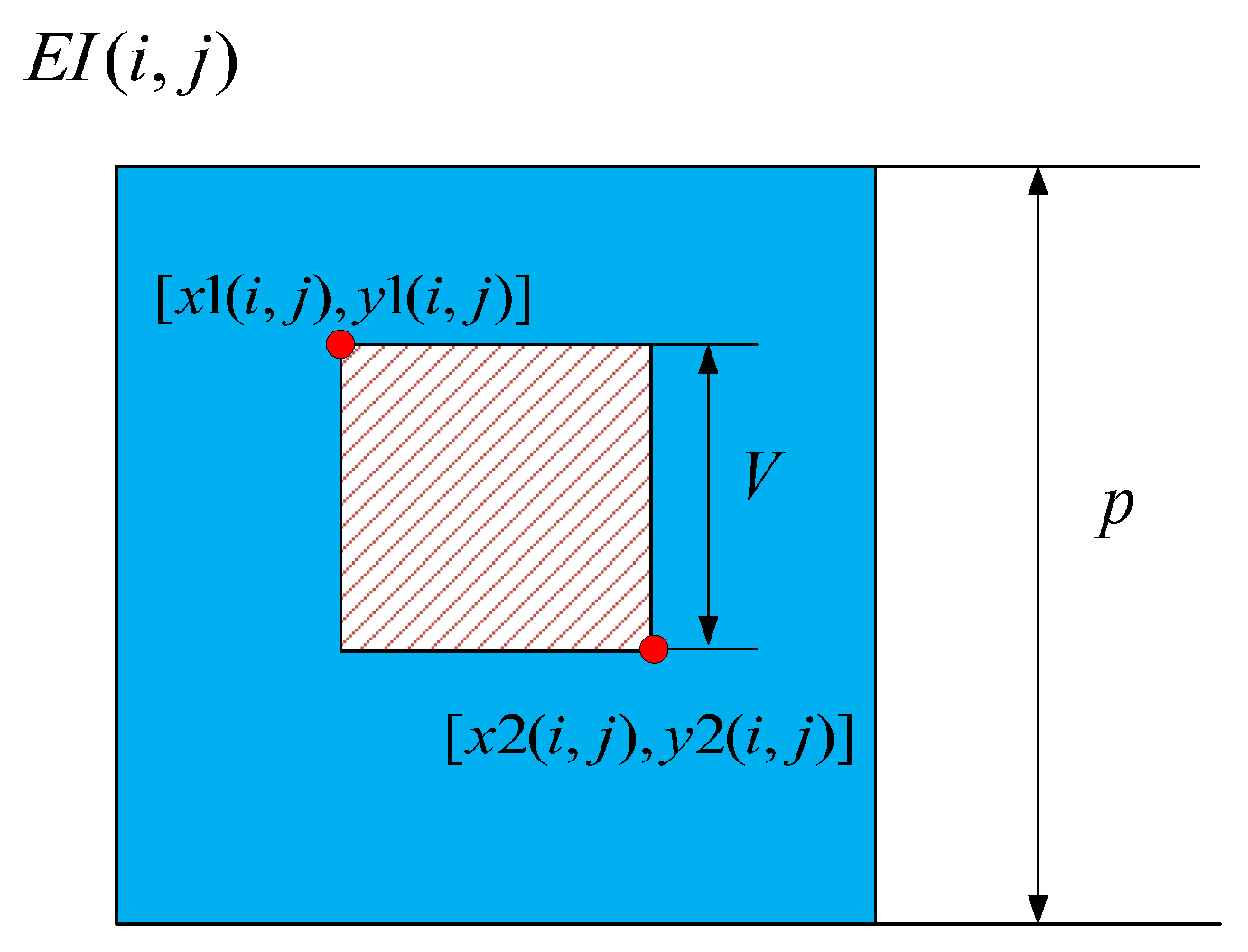
2.2.1. Calculation Acceleration Based on Visible Pixels
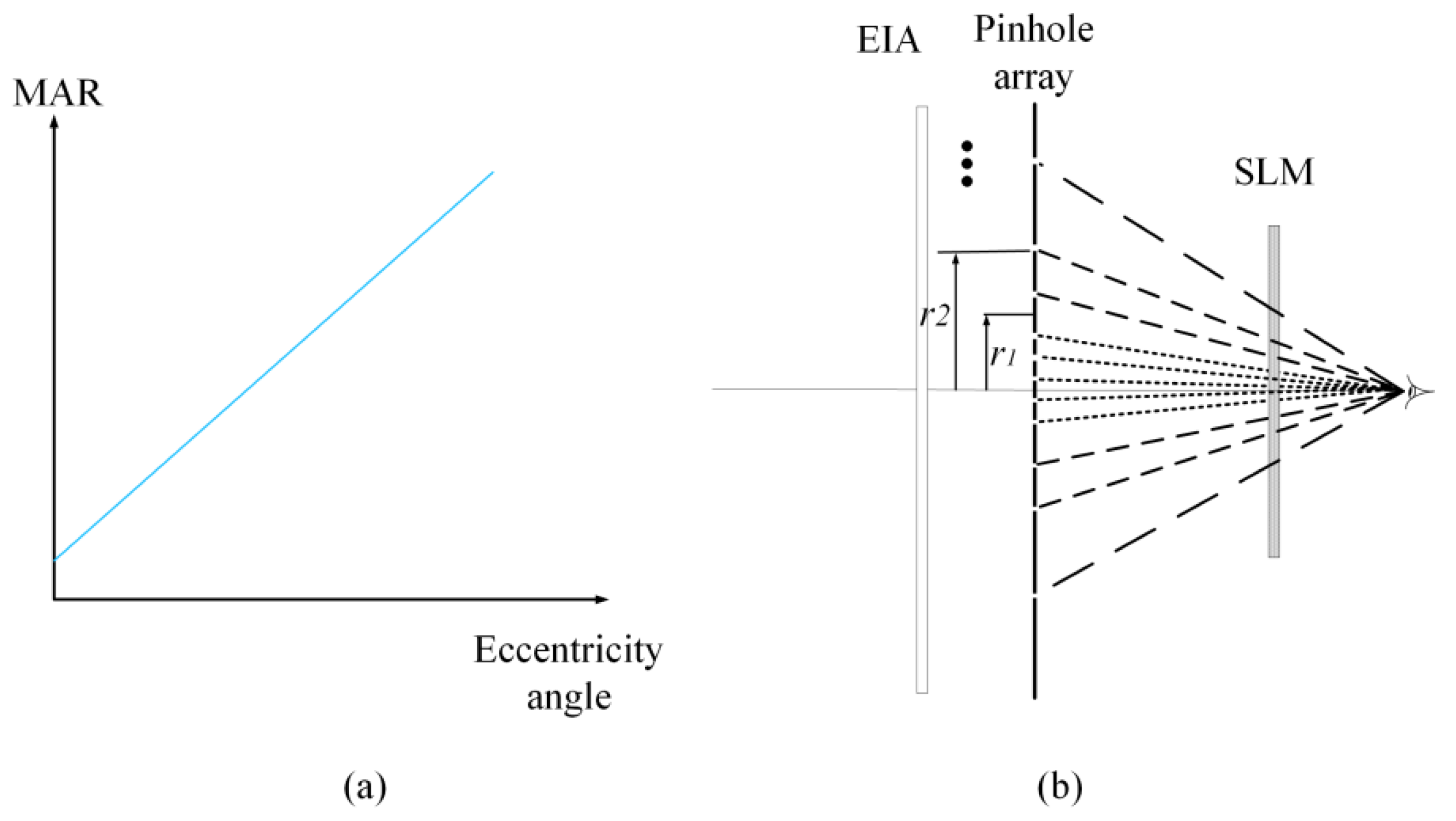
2.2.2. Calculation Acceleration Based on Foveated Region Rendering
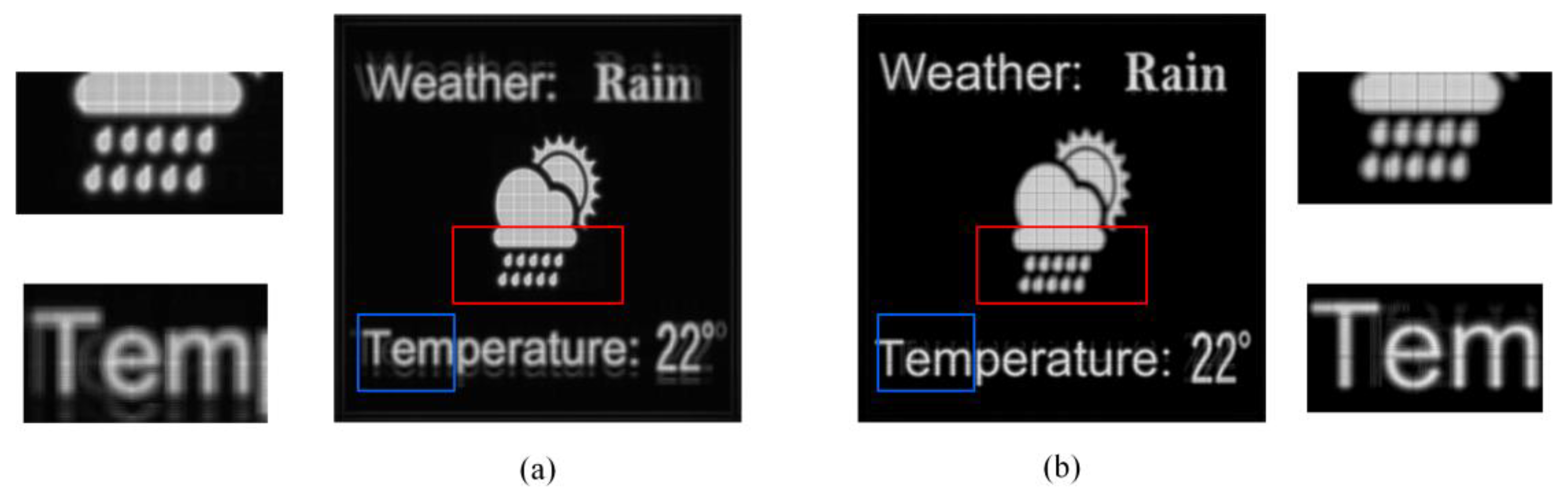
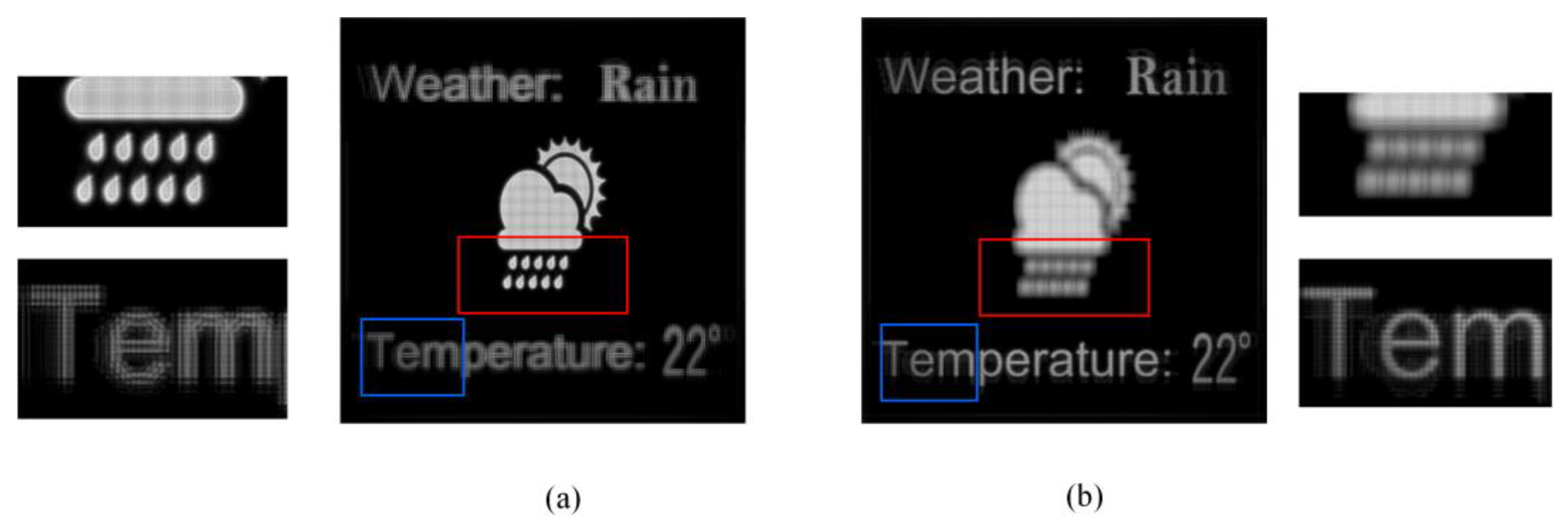
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- He, Z.; Sui, X.; Jin, G.; Cao, L. Progress in virtual reality and augmented reality based on holographic display. Appl. Opt. 2018, 58, A74–A81. [Google Scholar] [CrossRef] [PubMed]
- Chen, C.P.; Cui, Y.; Ye, Y.; Yin, F.; Shao, H.; Lu, Y.; Li, G. Wide-Field-of-View Near-Eye Display with Dual-Channel Waveguide. Photonics 2021, 8, 557. [Google Scholar] [CrossRef]
- Eunkyong, O.; Myeongjae, K.; Jinyoung, R.; Hwi, K.; Joonku, H. Holographic Head-Mounted Display with RGB Light Emitting Diode Light Source. Opt. Express 2014, 22, 6526–6534. [Google Scholar]
- Yasuhiro, T.; Nago, N. Multi-Projection of Lenticular Displays to Construct a 256-View Super Multi-View Display. Opt. Express 2010, 18, 8824–8835. [Google Scholar]
- Lanman, D.; Luebke, D. Near-eye light field displays. ACM Trans. Graph. 2013, 32, 1–10. [Google Scholar] [CrossRef]
- Wang, Q.; Ji, C.; Li, L.; Deng, H. Dual-view integral imaging 3D display by using orthogonal polarizer array and polarization switcher. Opt. Express 2016, 24, 9–16. [Google Scholar] [CrossRef] [PubMed]
- Kaan, A.; Ward, L.; Jonghyun, K.; Peter, S.; David, L. Near-Eye Varifocal Augmented Reality Display Using See-through Screens. ACM Trans. Graph. 2017, 36, 189. [Google Scholar]
- Mali, L.; Chihao, L.; Haifeng, L.; Xu, L. Near Eye Light Field Display Based on Human Visual Features. Opt. Express 2017, 25, 9886–9900. [Google Scholar]
- Tomoyoshi, S.; Hirotaka, N.; Nobuyuki, M.; Tomoyoshi, I. Rapid Calculation Algorithm of Fresnel Computer-Generated-Hologram Using Look-up Table and Wavefront-Recording Plane Methods for Three-Dimensional Display. Opt. Express 2010, 18, 19504–19509. [Google Scholar]
- Kyoji, M.; Nakahara, S. Extremely High-Definition Full-Parallax Computer-Generated Hologram Created by the Polygon-Based Method. Appl. Opt. 2009, 48, H54–H63. [Google Scholar]
- Yan, Z.; Cao, L.; Zhang, H.; Kong, D.; Jin, G. Accurate Calculation of Computer-Generated Holograms Using Angular-Spectrum Layer-Oriented Method. Opt. Express 2015, 23, 25440–25449. [Google Scholar]
- Wang, Z.; Zhu, L.; Zhang, X.; Dai, P.; Lv, G.; Feng, Q.; Wang, A.; Ming, H. Computer-Generated Photorealistic Hologram Using Ray-Wavefront Conversion Based on the Additive Compressive Light Field Approach. Opt. Lett. 2020, 45, 615–618. [Google Scholar] [CrossRef] [PubMed]
- Nishi, H.; Matsushima, K.; Nakahara, S. Smooth shading of specular surfaces in polygon-based high-definition CGH. In Proceedings of the 2011 3DTV Conference: The True Vision—Capture, Transmission and Display of 3D Video (3DTV-CON), Antalya, Turkey, 16–18 May 2011; pp. 1–4. [Google Scholar]
- Blinder, D.; Chlipala, M.; Kozacki, T.; Schelkens, P. Photorealistic computer generated holography with global illumination and path tracing. Opt. Lett. 2021, 46, 2188–2191. [Google Scholar] [CrossRef] [PubMed]
- Tomoyuki, M.; Makoto, O.; Fumio, O. Calculation of Holograms from Elemental Images Captured by Integral Photography. Appl. Opt. 2006, 45, 4026–4036. [Google Scholar]
- Hyeung, P.J.; Su, K.M.; Ganbat, B.; Nam, K. Fresnel and Fourier Hologram Generation Using Orthographic Projection Images. Opt. Express 2009, 17, 6320–6334. [Google Scholar]
- Yasuyuki, I.; Ryutaro, O.; Takanori, S.; Kenji, Y.; Taiichiro, K. Real-Time Capture and Reconstruction System with Multiple GPUs for a 3D Live Scene by a Generation from 4K IP Images to 8K Holograms. Opt. Express 2012, 20, 21645–21655. [Google Scholar]
- Koki, W.; Masahiro, Y.; Bahram, J. High-Resolution Three-Dimensional Holographic Display Using Dense Ray Sampling from Integral Imaging. Opt. Lett. 2012, 37, 5103–5105. [Google Scholar]
- Ichikawa, T.; Yoneyama, T.; Sakamoto, Y. CGH calculation with the ray tracing method for the Fourier transform optical system. Opt. Express 2013, 21, 32019–32031. [Google Scholar] [CrossRef] [Green Version]
- Wang, Z.; Lv, G.; Feng, Q.; Wang, A.; Ming, H. Resolution priority holographic stereogram based on integral imaging with enhanced depth range. Opt. Express 2019, 27, 2689–2702. [Google Scholar] [CrossRef]
- Wang, Z.; Lv, G.; Feng, Q.; Wang, A.; Ming, H. Simple and Fast Calculation Algorithm for Computer-Generated Hologram Based on Integral Imaging Using Look-up Table. Opt. Express 2018, 26, 13322–13330. [Google Scholar] [CrossRef]
- Wang, Z.; Lv, G.; Feng, Q.; Wang, A.; Ming, H. Enhanced resolution of holographic stereograms by moving or diffusing a virtual pinhole array. Opt. Express 2020, 28, 22755–22766. [Google Scholar] [CrossRef] [PubMed]
- Kim, Y.; Kim, J.; Kang, J.M.; Jung, J.H.; Choi, H.; Lee, H. Point light source integral imaging with improved resolution and viewing angle by the use of electrically movable pinhole array. Opt. Express 2007, 15, 18253–18267. [Google Scholar] [CrossRef]
- Deng, H.; Wang, Q.; Wu, F.; Luo, C.; Liu, Y. Cross-talk-free integral imaging three-dimensional display based on a pyramid pinhole array. Photonics Res. 2015, 3, 173–176. [Google Scholar] [CrossRef]
- Chen, Z.; Sang, X.; Lin, Q.; Li, J.; Yu, X.; Gao, X.; Yan, B.; Yu, C.; Dou, W.; Xiao, L. Acceleration for Computer-Generated Hologram in Head-Mounted Display with Effective Diffraction Area Recording Method for Eyes. Chin. Opt. Lett. 2016, 14, 80901–80905. [Google Scholar] [CrossRef]
- Jisoo, H.; Youngmin, K.; Sunghee, H.; Choonsung, S.; Hoonjong, K. Gaze Contingent Hologram Synthesis for Holographic Head-Mounted Display. In Practical Holography XXX: Materials and Applications; SPIE: Bellingham, WA, USA, 2016; Volume 9771, p. 97710K. [Google Scholar]
- Lingjie, W.; Sakamoto, Y. Fast Calculation Method with Foveated Rendering for Computer-Generated Holograms Using an Angle-Changeable Ray-Tracing Method. Appl. Opt. 2019, 58, A258–A266. [Google Scholar]
- Yeon-Gyeong, J.; Park, J. Foveated Computer-Generated Hologram and Its Progressive Update Using Triangular Mesh Scene Model for near-Eye Displays. Opt. Express 2019, 27, 23725–23738. [Google Scholar]
- Liang, C.C.; Wei, C.; Liang, G. Foveated Holographic near-Eye 3D Display. Opt. Express 2020, 28, 1345–1356. [Google Scholar]
- Wang, Z.; Zhang, X.; Lv, G.; Feng, Q.; Ming, H.; Wang, A. Hybrid holographic Maxwellian near-eye display based on spherical wave and plane wave reconstruction for augmented reality display. Opt. Express 2021, 29, 4927–4935. [Google Scholar] [CrossRef]
- Maimone, A.; Georgiou, A.; Kollin, J.S. Holographic near-eye displays for virtual and augmented reality. ACM Trans. Graph. (Tog) 2017, 36, 1–16. [Google Scholar] [CrossRef]








| The Method | FFT-Based Method | Pinhole-Type HS | Visible Pixels Method | Foveated Region Rendering Method | Visible Pixels Method and Foveated Region Rendering Method |
| Time Cost (ms) | 2590 | 2170 | 337 | 638 | 102 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, X.; Chen, T.; Pang, Y.; Tu, K.; Dai, P.; Lv, G.; Wang, Z.; Feng, Q. Accelerated Generation of a Pinhole-Type Holographic Stereogram Based on Human Eye Characteristics in Near-Eye Displays. Photonics 2022, 9, 95. https://doi.org/10.3390/photonics9020095
Zhang X, Chen T, Pang Y, Tu K, Dai P, Lv G, Wang Z, Feng Q. Accelerated Generation of a Pinhole-Type Holographic Stereogram Based on Human Eye Characteristics in Near-Eye Displays. Photonics. 2022; 9(2):95. https://doi.org/10.3390/photonics9020095
Chicago/Turabian StyleZhang, Xu, Tao Chen, Yujian Pang, Kefeng Tu, Piao Dai, Guoqiang Lv, Zi Wang, and Qibin Feng. 2022. "Accelerated Generation of a Pinhole-Type Holographic Stereogram Based on Human Eye Characteristics in Near-Eye Displays" Photonics 9, no. 2: 95. https://doi.org/10.3390/photonics9020095
APA StyleZhang, X., Chen, T., Pang, Y., Tu, K., Dai, P., Lv, G., Wang, Z., & Feng, Q. (2022). Accelerated Generation of a Pinhole-Type Holographic Stereogram Based on Human Eye Characteristics in Near-Eye Displays. Photonics, 9(2), 95. https://doi.org/10.3390/photonics9020095




