Squeezing Light via Levitated Cavity Optomechanics
Abstract
1. Introduction
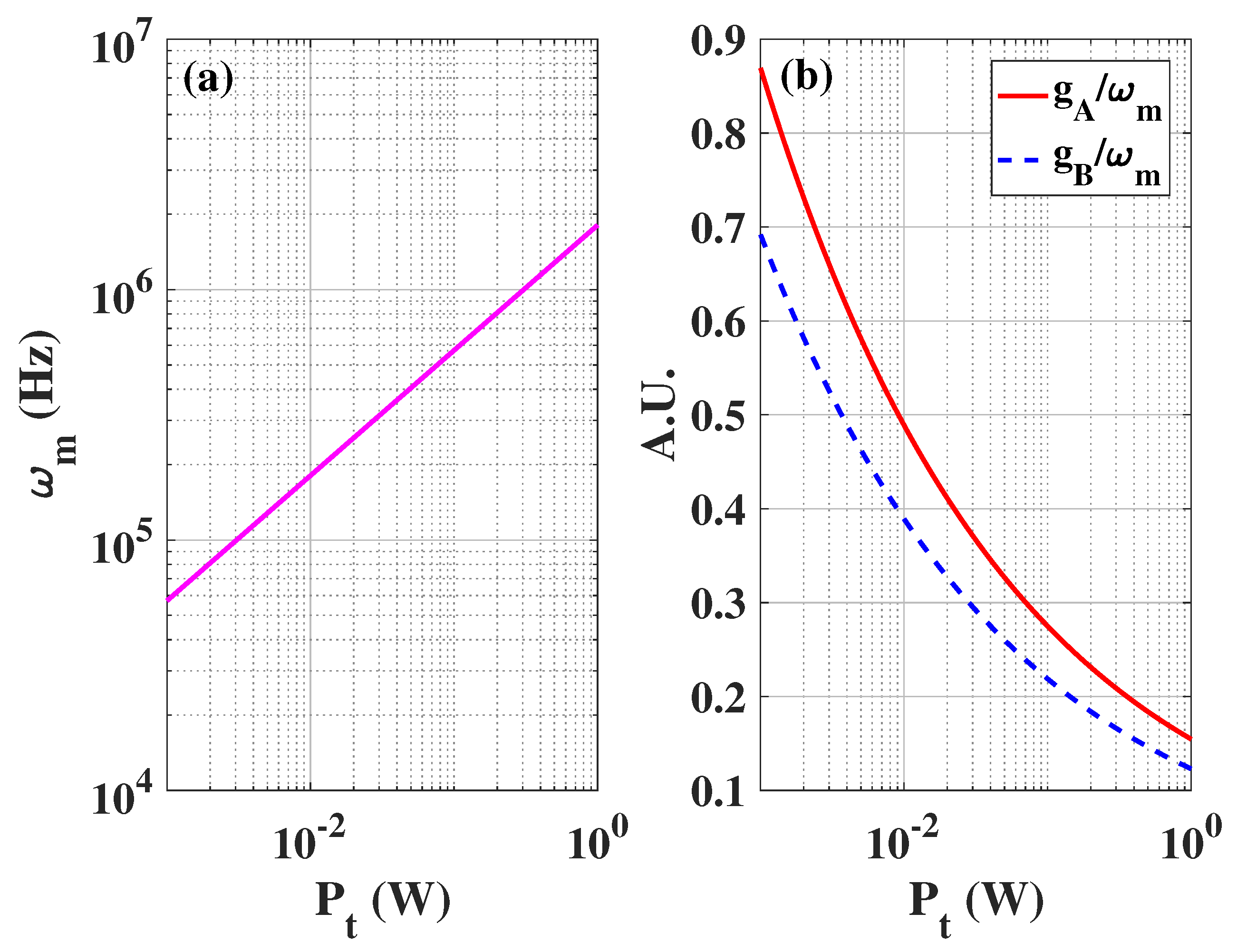
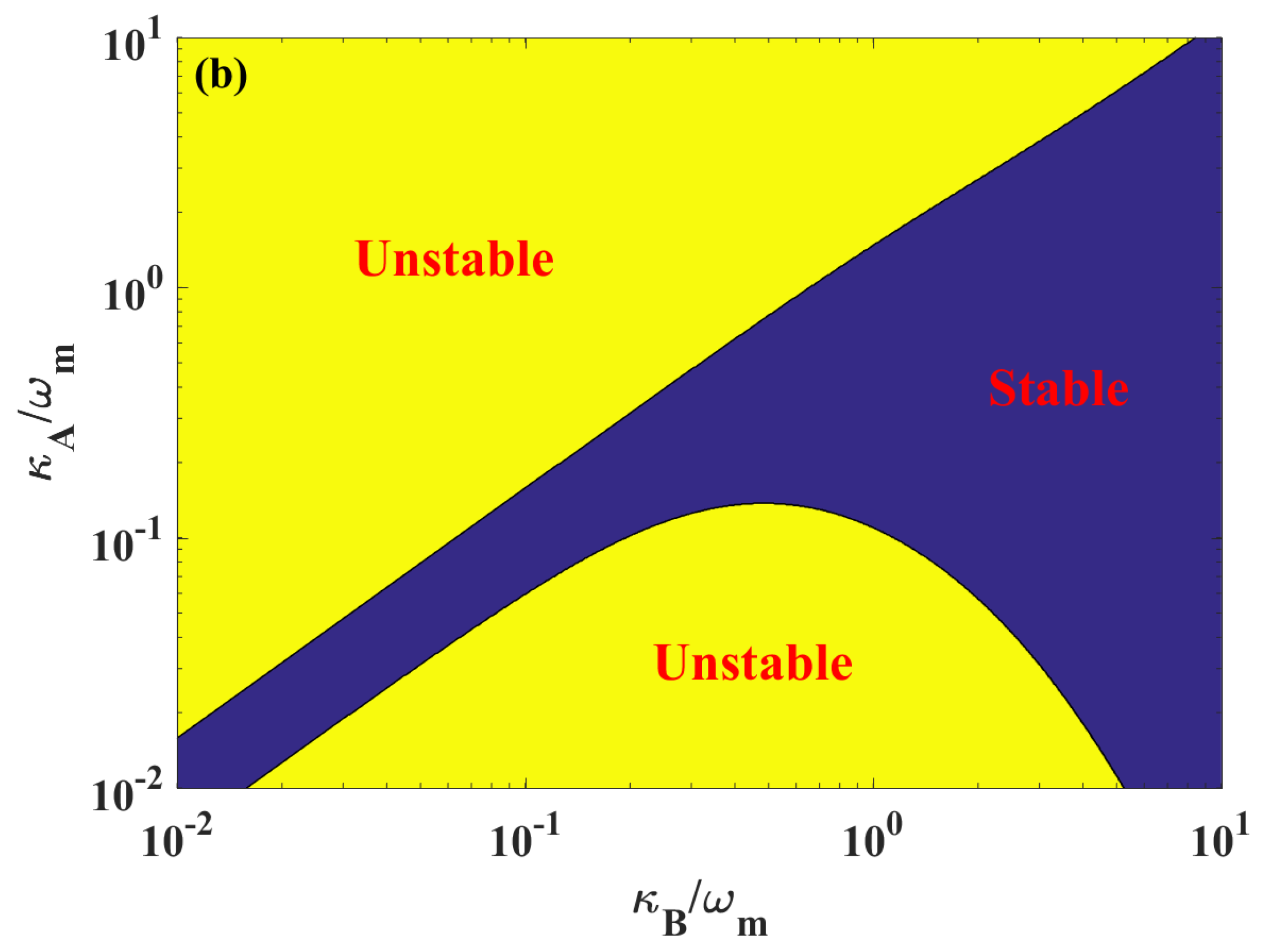
2. Model and Dynamics
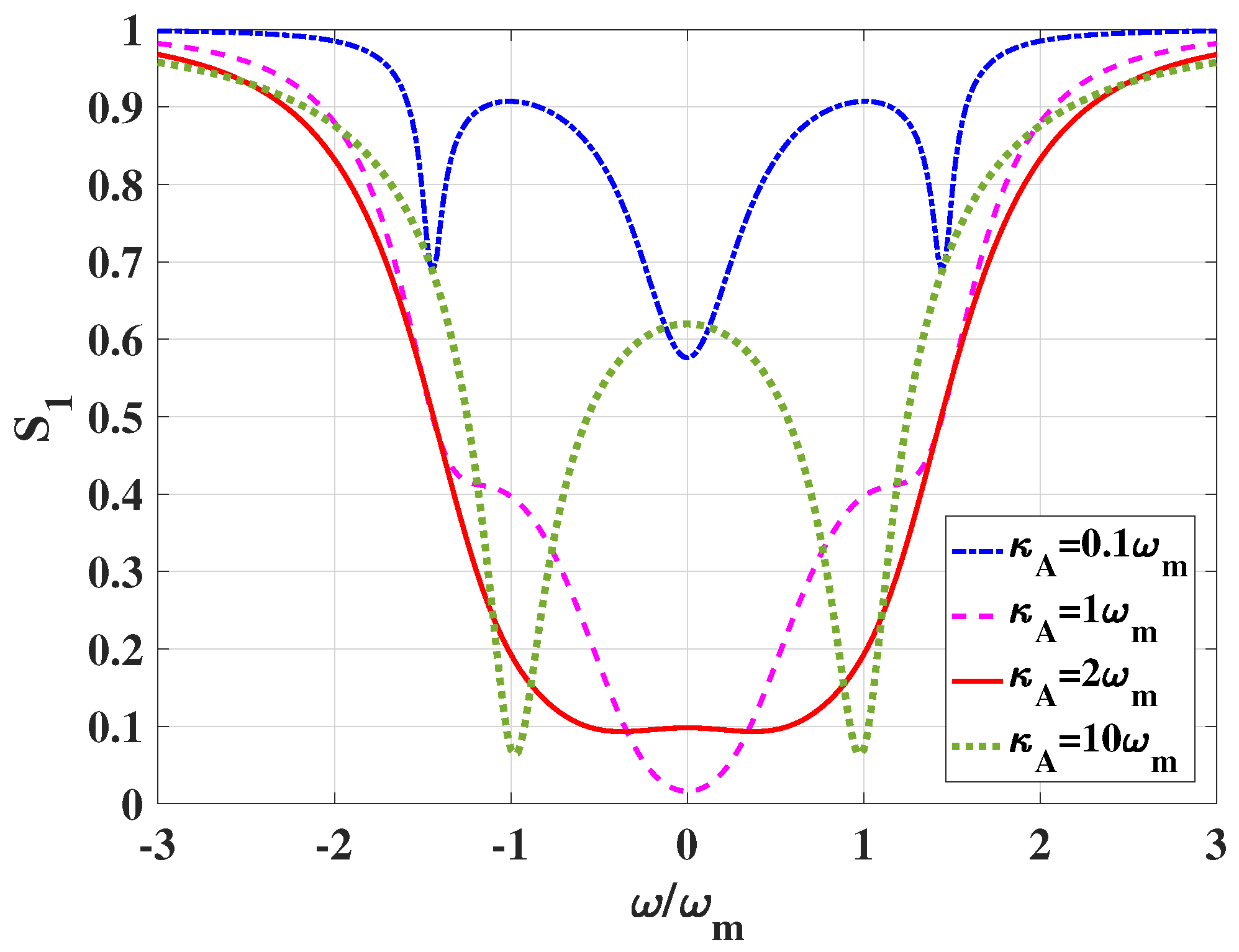
3. Single-Mode Squeezing
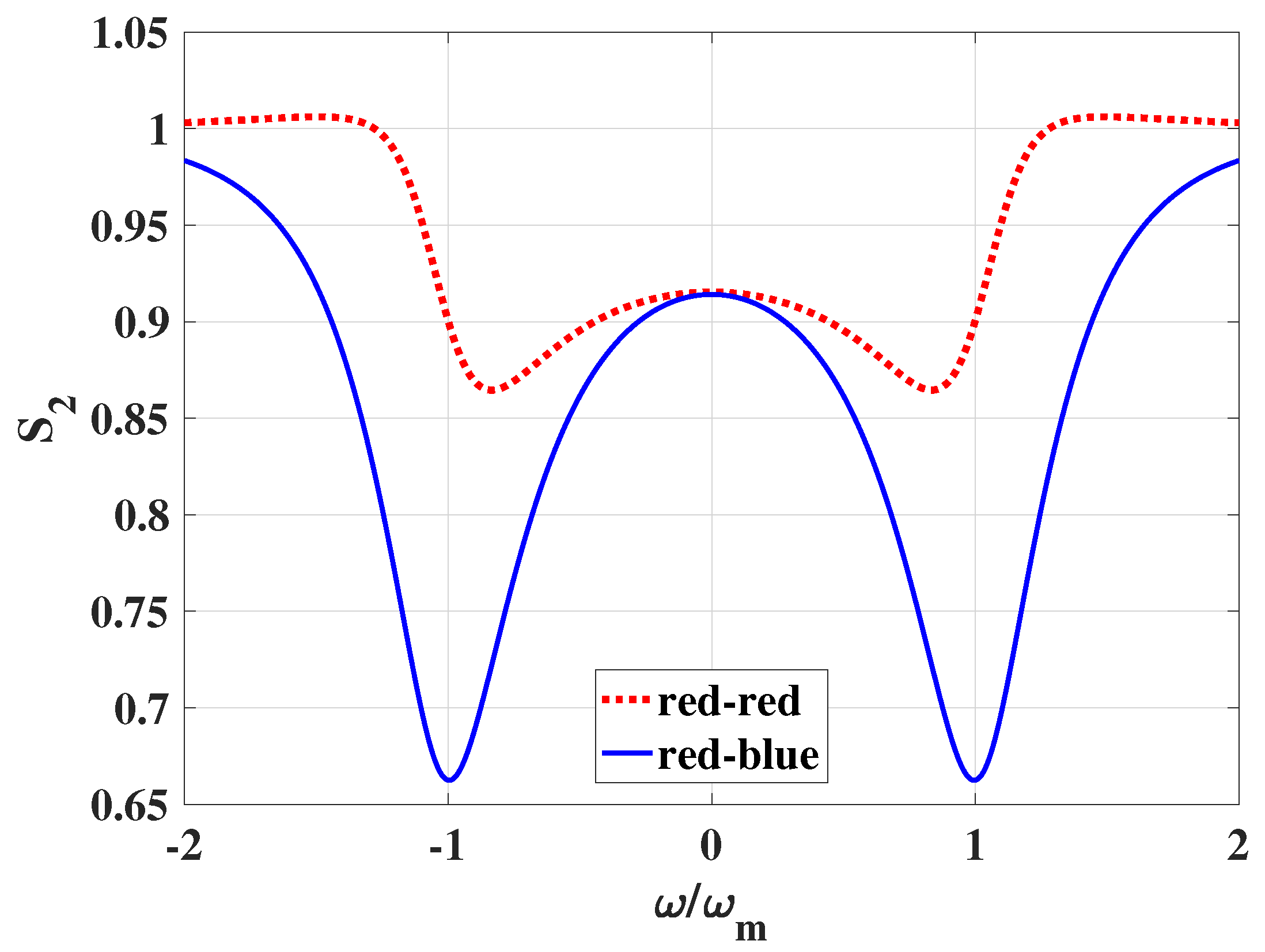
4. Two-Mode Squeezing
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Polarization Tensor
Appendix B. Hamiltonian for Coherent Scattering
Appendix C. Damping for Torsional Motion
References
- Aasi, J.; Abadie, J.; Abbott, B.P.; Abbott, R.; Abbott, T.D.; Abernathy, M.R.; Adams, C.; Adams, T.; Addesso, P.; Adhikari, R.X.; et al. Enhanced sensitivity of the LIGO gravitational wave detector by using squeezed states of light. Nat. Photonics 2013, 7, 613–619. [Google Scholar] [CrossRef]
- Clark, J.B.; Lecocq, F.; Simmonds, R.W.; Aumentado, J.; Teufel, J.D. Sideband cooling beyond the quantum backaction limit with squeezed light. Nature 2017, 541, 191–195. [Google Scholar] [CrossRef] [PubMed]
- Zeytinoğlu, S.; İmamoğlu, A.; Huber, S. Engineering matter interactions using squeezed vacuum. Phys. Rev. X 2017, 7, 021041. [Google Scholar] [CrossRef]
- Peano, V.; Houde, M.; Brendel, C.; Marquardt, F.; Clerk, A.A. Topological phase transitions and chiral inelastic transport induced by the squeezing of light. Nat. Commun. 2016, 7, 10779. [Google Scholar] [CrossRef] [PubMed]
- Fabre, C.; Pinard, M.; Bourzeix, S.; Heidmann, A.; Giacobino, E.; Reynaud, S. Quantum-noise reduction using a cavity with a movable mirror. Phys. Rev. A 1994, 49, 1337–1343. [Google Scholar] [CrossRef]
- Mancini, S.; Tombesi, P. Quantum noise reduction by radiation pressure. Phys. Rev. A 1994, 49, 4055–4065. [Google Scholar] [CrossRef]
- Wu, L.A.; Kimble, H.; Hall, J.; Wu, H. Generation of squeezed states by parametric down conversion. Phys. Rev. Lett. 1986, 57, 2520. [Google Scholar] [CrossRef]
- Aspelmeyer, M.; Kippenberg, T.J.; Marquardt, F. Cavity optomechanics. Rev. Mod. Phys. 2014, 86, 1391. [Google Scholar] [CrossRef]
- Purdy, T.P.; Yu, P.L.; Peterson, R.W.; Kampel, N.S.; Regal, C.A. Strong Optomechanical Squeezing of Light. Phys. Rev. X 2013, 3, 031012. [Google Scholar] [CrossRef]
- Ockeloen-Korppi, C.; Damskägg, E.; Pirkkalainen, J.M.; Heikkilä, T.; Massel, F.; Sillanpää, M. Noiseless quantum measurement and squeezing of microwave fields utilizing mechanical vibrations. Phys. Rev. Lett. 2017, 118, 103601. [Google Scholar] [CrossRef]
- Pontin, A.; Biancofiore, C.; Serra, E.; Borrielli, A.; Cataliotti, F.; Marino, F.; Prodi, G.; Bonaldi, M.; Marin, F.; Vitali, D. Frequency-noise cancellation in optomechanical systems for ponderomotive squeezing. Phys. Rev. A 2014, 89, 033810. [Google Scholar] [CrossRef]
- Yin, Z.Q.; Han, Y.J. Generating EPR beams in a cavity optomechanical system. Phys. Rev. A 2009, 79, 024301. [Google Scholar] [CrossRef]
- Sainadh, S.; Kumar, M.A. Effects of linear and quadratic dispersive couplings on optical squeezing in an optomechanical system. Phys. Rev. A 2015, 92, 033824. [Google Scholar] [CrossRef]
- Zippilli, S.; Di Giuseppe, G.; Vitali, D. Entanglement and squeezing of continuous-wave stationary light. New J. Phys. 2015, 17, 043025. [Google Scholar] [CrossRef][Green Version]
- Kronwald, A.; Marquardt, F.; Clerk, A.A. Dissipative optomechanical squeezing of light. New J. Phys. 2014, 16, 063058. [Google Scholar] [CrossRef]
- Yin, Z.q.; Li, T.; Zhang, X.; Duan, L. Large quantum superpositions of a levitated nanodiamond through spin-optomechanical coupling. Phys. Rev. A 2013, 88, 033614. [Google Scholar] [CrossRef]
- Romero-Isart, O.; Pflanzer, A.C.; Blaser, F.; Kaltenbaek, R.; Kiesel, N.; Aspelmeyer, M.; Cirac, J.I. Large quantum superpositions and interference of massive nanometer-sized objects. Phys. Rev. Lett. 2011, 107, 020405. [Google Scholar] [CrossRef]
- Chen, X.Y.; Yin, Z.Q. High-precision gravimeter based on a nano-mechanical resonator hybrid with an electron spin. Opt. Express 2018, 26, 31577. [Google Scholar] [CrossRef]
- Huang, Y.; Guo, Q.; Xiong, A.; Li, T.; Yin, Z.Q. Classical and quantum time crystals in a levitated nanoparticle without drive. Phys. Rev. A 2020, 102, 023113. [Google Scholar] [CrossRef]
- Huang, Y.; Li, T.; Yin, Z.Q. Symmetry-breaking dynamics of the finite-size Lipkin-Meshkov-Glick model near ground state. Phys. Rev. A 2018, 97, 012115. [Google Scholar] [CrossRef]
- Zhang, H.; Chen, X.; Yin, Z.q. Quantum Information Processing and Precision Measurement Using a Levitated Nanodiamond. Adv. Quantum Technol. 2021, 4, 2000154. [Google Scholar] [CrossRef]
- Chang, D.E.; Regal, C.; Papp, S.; Wilson, D.; Ye, J.; Painter, O.; Kimble, H.J.; Zoller, P. Cavity opto-mechanics using an optically levitated nanosphere. Proc. Natl. Acad. Sci. USA 2010, 107, 1005–1010. [Google Scholar] [CrossRef] [PubMed]
- Rashid, M.; Tufarelli, T.; Bateman, J.; Vovrosh, J.; Hempston, D.; Kim, M.; Ulbricht, H. Experimental realization of a thermal squeezed state of levitated optomechanics. Phys. Rev. Lett. 2016, 117, 273601. [Google Scholar] [CrossRef]
- Gonzalez-Ballestero, C.; Maurer, P.; Windey, D.; Novotny, L.; Reimann, R.; Romero-Isart, O. Theory for cavity cooling of levitated nanoparticles via coherent scattering: Master equation approach. Phys. Rev. A 2019, 100, 013805. [Google Scholar] [CrossRef]
- Toroš, M.; Delić, U.; Hales, F.; Monteiro, T.S. Coherent-scattering two-dimensional cooling in levitated cavity optomechanics. Phys. Rev. Res. 2021, 3, 023071. [Google Scholar] [CrossRef]
- Delić, U.; Reisenbauer, M.; Dare, K.; Grass, D.; Vuletić, V.; Kiesel, N.; Aspelmeyer, M. Cooling of a levitated nanoparticle to the motional quantum ground state. Science 2020, 367, 892–895. [Google Scholar] [CrossRef] [PubMed]
- Schäfer, J.; Rudolph, H.; Hornberger, K.; Stickler, B.A. Cooling nanorotors by elliptic coherent scattering. Phys. Rev. Lett. 2021, 126, 163603. [Google Scholar] [CrossRef] [PubMed]
- Magrini, L.; Rosenzweig, P.; Bach, C.; Deutschmann-Olek, A.; Hofer, S.G.; Hong, S.; Kiesel, N.; Kugi, A.; Aspelmeyer, M. Real-time optimal quantum control of mechanical motion at room temperature. Nature 2021, 595, 373–377. [Google Scholar] [CrossRef]
- Tebbenjohanns, F.; Mattana, M.L.; Rossi, M.; Frimmer, M.; Novotny, L. Quantum control of a nanoparticle optically levitated in cryogenic free space. Nature 2021, 595, 378–382. [Google Scholar] [CrossRef]
- De los Ríos Sommer, A.; Meyer, N.; Quidant, R. Strong optomechanical coupling at room temperature by coherent scattering. Nat. Commun. 2021, 12, 276–281. [Google Scholar] [CrossRef]
- Asenbaum, P.; Kuhn, S.; Nimmrichter, S.; Sezer, U.; Arndt, M. Cavity cooling of free silicon nanoparticles in high vacuum. Nat. Commun. 2013, 4, 1–7. [Google Scholar] [CrossRef] [PubMed]
- Millen, J.; Monteiro, T.S.; Pettit, R.; Vamivakas, A.N. Optomechanics with levitated particles. Rep. Prog. Phys. 2020, 83, 026401. [Google Scholar] [CrossRef] [PubMed]
- Jain, V.; Gieseler, J.; Moritz, C.; Dellago, C.; Quidant, R.; Novotny, L. Direct measurement of photon recoil from a levitated nanoparticle. Phys. Rev. Lett. 2016, 116, 243601. [Google Scholar] [CrossRef] [PubMed]
- Černotík, O.; Filip, R. Strong mechanical squeezing for a levitated particle by coherent scattering. Phys. Rev. Res. 2020, 2, 013052. [Google Scholar] [CrossRef]
- Yin, Z.Q.; Geraci, A.A.; Li, T. Optomechanics of levitated dielectric particles. Int. J. Mod. Phys. B 2013, 27, 1330018. [Google Scholar] [CrossRef]
- Kockum, A.F.; Miranowicz, A.; De Liberato, S.; Savasta, S.; Nori, F. Ultrastrong coupling between light and matter. Nat. Rev. Phys. 2019, 1, 19–40. [Google Scholar] [CrossRef]
- Li, G.; Yin, Z.Q. Steady motional entanglement between two distant levitated nanoparticles. arXiv 2021, arXiv:2111.11620. [Google Scholar]
- Hoang, T.M.; Ma, Y.; Ahn, J.; Bang, J.; Robicheaux, F.; Yin, Z.Q.; Li, T. Torsional optomechanics of a levitated nonspherical nanoparticle. Phys. Rev. Lett. 2016, 117, 123604. [Google Scholar] [CrossRef]
- Ahn, J.; Xu, Z.; Bang, J.; Deng, Y.H.; Hoang, T.M.; Han, Q.; Ma, R.M.; Li, T. Optically levitated nanodumbbell torsion balance and GHz nanomechanical rotor. Phys. Rev. Lett. 2018, 121, 033603. [Google Scholar] [CrossRef]
- Genes, C.; Mari, A.; Tombesi, P.; Vitali, D. Robust entanglement of a micromechanical resonator with output optical fields. Phys. Rev. A 2008, 78, 032316. [Google Scholar] [CrossRef]
- Vitali, D.; Gigan, S.; Ferreira, A.; Böhm, H.; Tombesi, P.; Guerreiro, A.; Vedral, V.; Zeilinger, A.; Aspelmeyer, M. Optomechanical entanglement between a movable mirror and a cavity field. Phys. Rev. Lett. 2007, 98, 030405. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.C.; Wang, Y.P.; Yu, Y.F.; Zhang, Z.M. Quantum squeezing in a modulated optomechanical system. Opt. Express 2018, 26, 11915–11927. [Google Scholar] [CrossRef] [PubMed]
- Li, Z.; Ma, S.L.; Li, F.L. Generation of broadband two-mode squeezed light in cascaded double-cavity optomechanical systems. Phys. Rev. A 2015, 92, 023856. [Google Scholar] [CrossRef]
- Vinante, A.; Falferi, P. Feedback-enhanced parametric squeezing of mechanical motion. Phys. Rev. Lett. 2013, 111, 207203. [Google Scholar] [CrossRef]
- Dassonneville, R.; Assouly, R.; Peronnin, T.; Clerk, A.; Bienfait, A.; Huard, B. Dissipative stabilization of squeezing beyond 3 dB in a microwave mode. PRX Quantum 2021, 2, 020323. [Google Scholar] [CrossRef]
- Bohren, C.F.; Huffman, D.R. Absorption and Scattering of Light by Small Particles; John Wiley & Sons: Hoboken, NJ, USA, 1983. [Google Scholar]
- Gonzalez-Ballestero, C.; Aspelmeyer, M.; Novotny, L.; Quidant, R.; Romero-Isart, O. Levitodynamics: Levitation and control of microscopic objects in vacuum. Science 2021, 374, eabg3027. [Google Scholar] [CrossRef]
- Halbritter, J. Torque on a rotating ellipsoid in a rarefied gas. Z. Für Naturforsch. A 1974, 29, 1717–1722. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, G.; Yin, Z.-Q. Squeezing Light via Levitated Cavity Optomechanics. Photonics 2022, 9, 57. https://doi.org/10.3390/photonics9020057
Li G, Yin Z-Q. Squeezing Light via Levitated Cavity Optomechanics. Photonics. 2022; 9(2):57. https://doi.org/10.3390/photonics9020057
Chicago/Turabian StyleLi, Guoyao, and Zhang-Qi Yin. 2022. "Squeezing Light via Levitated Cavity Optomechanics" Photonics 9, no. 2: 57. https://doi.org/10.3390/photonics9020057
APA StyleLi, G., & Yin, Z.-Q. (2022). Squeezing Light via Levitated Cavity Optomechanics. Photonics, 9(2), 57. https://doi.org/10.3390/photonics9020057




