Photoelectron Yield Spectroscopy and Transient Photocurrent Analysis for Triphenylamine-Based Photorefractive Polymer Composites
Abstract
1. Introduction
2. Materials and Methods
3. Results and Discussion
3.1. Photoelectron Yield Spectroscopy and Energy Diagram
3.2. Evaluation of the Transient Photocurrent Determined Using Two-Trap Model
3.3. Analysis of the Trapping Behavior and Transient Density for Filled Traps
3.4. Relationship between Trapping Behavior and Photorefractive Response
3.5. Estimation of Value for Trap State
3.6. DOS Width and Hole Mobility
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
| PDAA/TPAOH/7-DCST/PCBM (35/35/30/0.6) | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| Type | QE/α (cm−1) | μ (cm2 V−1 s−1) | γT (cm3 s−1) | T (cm−3) | βT (s−1) | γM (cm3 s−1) | M (cm−3) | βM (s−1) | γR (cm3 s−1) |
| No. 1 | 1.6 × 10−2/59 | 9.0 × 10−7 | 1.6 × 10−13 | 1.2 × 1016 | 120 | 1.5 × 10−16 | 2 × 1016 | 0.01 | 1.0 × 10−13 |
| No. 2 | 6.0 × 10−3/59 | 2.3 × 10−6 | 1.6 × 10−13 | 1.2 × 1016 | 200 | 2.0 × 10−16 | 2 × 1016 | 0.01 | 2.0 × 10−13 |
| No. 3 | 4.3 × 10−3/59 | 3.3 × 10−6 | 1.6 × 10−13 | 1.2 × 1016 | 200 | 2.0 × 10−16 | 2 × 1016 | 0.01 | 2.7 × 10−13 |
| No. 4 | 3.8 × 10−3/59 | 3.7 × 10−6 | 1.6 × 10−13 | 1.2 × 1016 | 200 | 2.2 × 10−16 | 2 × 1016 | 0.01 | 2.9 × 10−13 |
| PDAA/TAA/7-DCST/PCBM (35/35/30/0.6) | |||||||||
| Type | QE/α (cm−1) | μ (cm2 V−1 s−1) | γT (cm3 s−1) | T (cm−3) | βT (s−1) | γM (cm3 s−1) | M (cm−3) | βM (s−1) | γR (cm3 s−1) |
| No. 1 | 7.0 × 10−4/45 | 3.9 × 10−7 | 1.7 × 10−13 | 1.1 × 1016 | 0.2 | 1.6 × 10−19 | 1 × 1016 | 0.001 | 1.4 × 10−13 |
| No. 2 | 6.4 × 10−4/45 | 3.7 × 10−7 | 1.5 × 10−13 | 1.1 × 1016 | 0.2 | 1.0 × 10−19 | 1 × 1016 | 0.001 | 1.2 × 10−13 |
| No. 3 | 4.0 × 10−4/45 | 6.2 × 10−7 | 1.5 × 10−13 | 1.1 × 1016 | 0.2 | 1.0 × 10−19 | 1 × 1016 | 0.001 | 1.3 × 10−13 |
References
- Ducharme, S.; Scott, J.C.; Twieg, R.J.; Moerner, W.E. Observation of the Photorefractive Effect in a Polymer. Phys. Rev. Lett. 1991, 66, 1846. [Google Scholar] [CrossRef] [PubMed]
- Blanche, P.-A.; Ka, J.W.; Peyghambarian, N. Review of Organic Photorefractive Materials and Their Use for Updateable 3D Display. Materials 2021, 14, 5799. [Google Scholar] [CrossRef] [PubMed]
- Moerner, W.E.; Silence, S.M. Polymeric Photorefractive Materials. Chem. Rev. 1994, 94, 127–155. [Google Scholar] [CrossRef]
- Tsutsumi, N. Molecular Design of Photorefractive Polymers. Polym. J. 2016, 48, 571–588. [Google Scholar] [CrossRef]
- Däubler, T.K.; Bittner, R.; Meerholz, K.; Cimrová, V.; Neher, D. Charge Carrier Photogeneration, Trapping, and Space-Charge Field Formation in PVK-Based Photorefractive Materials. Phys. Rev. B 2000, 61, 13515–13527. [Google Scholar] [CrossRef]
- Däubler, T.K.; Kulikovsky, L.; Neher, D.; Cimrová, V.; Hummelen, J.C.; Mecher, E.; Bittner, R.; Meerholz, K. Photoconductivity and Charge-Carrier Photogeneration in Photorefractive Polymers. Proc. SPIE 2002, 4462, 206–216. [Google Scholar] [CrossRef]
- Tsutsumi, N.; Kinashi, K.; Masumura, K.; Kono, K. Photorefractive Performance of Poly(triarylamine)-Based Polymer Composites: An Approach from the Photoconductive Properties. J. Polym. Sci. Part B Polym. Phys. 2015, 53, 502–508. [Google Scholar] [CrossRef]
- Tsutsumi, N.; Kinashi, K.; Masumura, K.; Kono, K. Photorefractive Dynamics in Poly (triarylamine)-Based Polymer Composites. Opt. Express 2015, 23, 25158–25170. [Google Scholar] [CrossRef] [PubMed]
- Masumura, K.; Oka, T.; Kinashi, K.; Sakai, W.; Tsutsumi, N. Photorefractive Dynamics in Poly(triarylamine)-Based Polymer Composite: An Approach Utilizing a Second Electron Trap to Reduce the Photo-Conductivity. Opt. Mater. Express 2018, 8, 401–412. [Google Scholar] [CrossRef]
- Masumura, K.; Nakanishi, I.; Khuat, K.V.T.; Kinashi, K.; Sakaki, W.; Tsutsumi, N. Optimal Composition of the Poly(triarylamine)-Based Polymer Composite to Maximize Photorefractive Performance. Sci. Reports 2019, 9, 739. [Google Scholar] [CrossRef]
- Tsutsumi, N.; Sakamoto, S.; Kinashi, K.; Jackin, B.J.; Sakai, W. Photorefractive Response Enhancement in Poly(triarylamine)-Based Polymer Composites by a Second Electron Trap Chromophore. ACS Omega 2022, 7, 12120–12126. [Google Scholar] [CrossRef] [PubMed]
- Schildkraut, J.S.; Buettner, A.V. Theory and Simulation of the Formation and Erasure of Space Charge Gratings in Photoconductive Polymers. J. Appl. Phys. 1992, 72, 1888–1893. [Google Scholar] [CrossRef]
- Ostroverkhova, O.; Singer, K.D. Space-Charge Dynamics in Photorefractive Polymers. J. Appl. Phys. 2002, 92, 1727–1743. [Google Scholar] [CrossRef]
- Kulikovsky, L.; Neher, D.; Mecher, E.; Meerholz, K.; Hörhold, H.-H.; Ostroverkhova, O. Photocurrent Dynamics in a Poly(phenylene vinylene)-Based Photorefractive Composite. Phys. Rev. B 2004, 69, 125216. [Google Scholar] [CrossRef]
- Tsujimura, S.; Fujihara, T.; Sassa, T.; Kinashi, K.; Sakai, W.; Ishibashi, K.; Tsutsumi, N. Enhanced Photoconductivity and Trapping Rate through Control of Bulk State in Organic Triphenylamine-Based Photorefractive Materials. Org. Electron. 2014, 15, 3471–3475. [Google Scholar] [CrossRef]
- Giang, H.N.; Kinashi, K.; Sakai, W.; Tsutsumi, N. Triphenylamine Photoconductive Polymers for High Performance Photorefractive Devices. J. Photochem. Photobiol. A Chem. 2014, 291, 26–33. [Google Scholar] [CrossRef][Green Version]
- Giang, H.N.; Sassa, T.; Fujihara, T.; Tsujimura, S.; Kinashi, K.; Sakai, W.; Wada, S.; Tsutsumi, N. Triphenylamine-Based Plasticizer in Controlling Traps and Photorefractivity Enhancement . ACS Appl. Electron. Mater. 2021, 3, 2170–2177. [Google Scholar] [CrossRef]
- Kukhtarev, N.V.; Markov, V.B.; Odulov, S.G.; Soskin, M.S.; Vinetskii, V.L. Holographic Storage in Electrooptic Crystals. I. Steady State. Ferroelectrics 1979, 22, 949–960. [Google Scholar] [CrossRef]
- Li, C.; Duan, L.; Sun, Y.; Li, H.; Qiu, Y. Charge Transport in Mixed Organic Disorder Semiconductors: Trapping, Scattering, and Effective Energetic Disorder. J. Phys. Chem. C 2012, 116, 19748–19754. [Google Scholar] [CrossRef]
- Borsenberger, P.M.; Pautmeier, L.; Bässler, H. Charge Transport in Disordered Molecular Solids. J. Chem. Phys. 1991, 94, 5447. [Google Scholar] [CrossRef]
- Bässler, H.; Borsenberger, P.M. The Transition from Nondispersive to Dispersive Charge Transport in Vapor Deposited Films of 1-Phenyl-3-p-diethylamino-styryl-5-p-diethylphenylpyrazoline (DEASP). Chem. Phys. 1993, 177, 763–771. [Google Scholar] [CrossRef]
- Tanaka, Y.; Kinashi, K.; Kono, K.; Sakai, W.; Tsutsumi, N. Electron Spin Resonance and Photoelectron Yield Spectroscopic Studies for Photocarrier Behavior in Photorefractive Polymeric Composites. Org. Electron. 2019, 68, 248–255. [Google Scholar] [CrossRef]
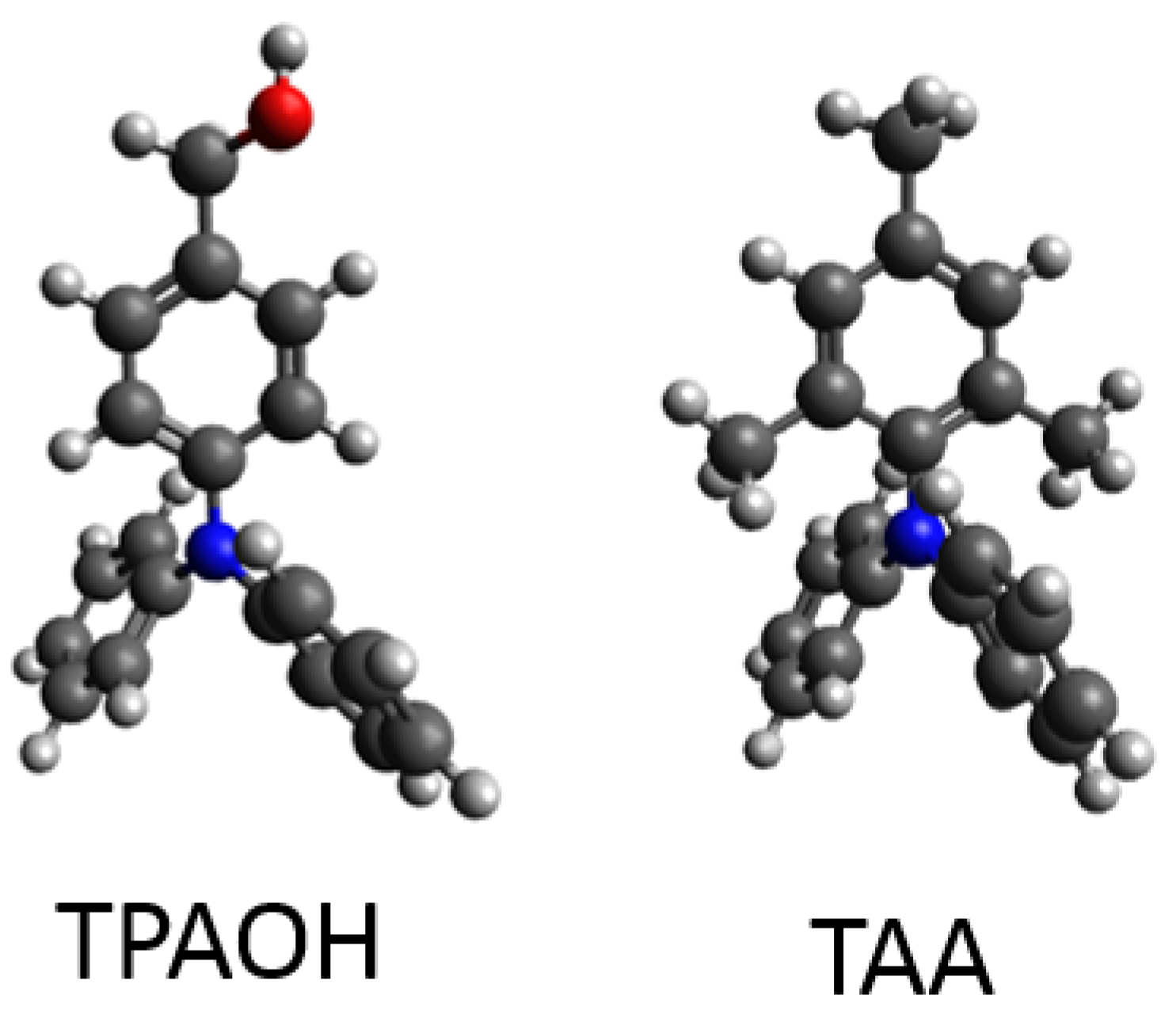

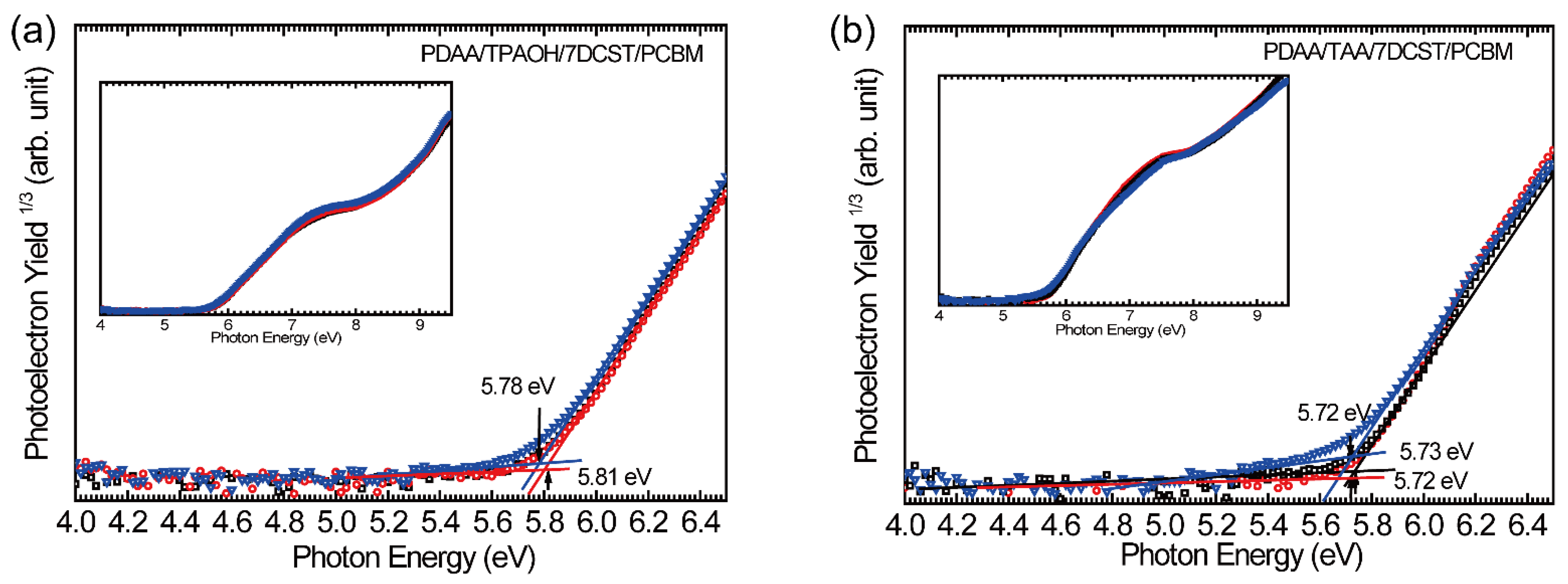

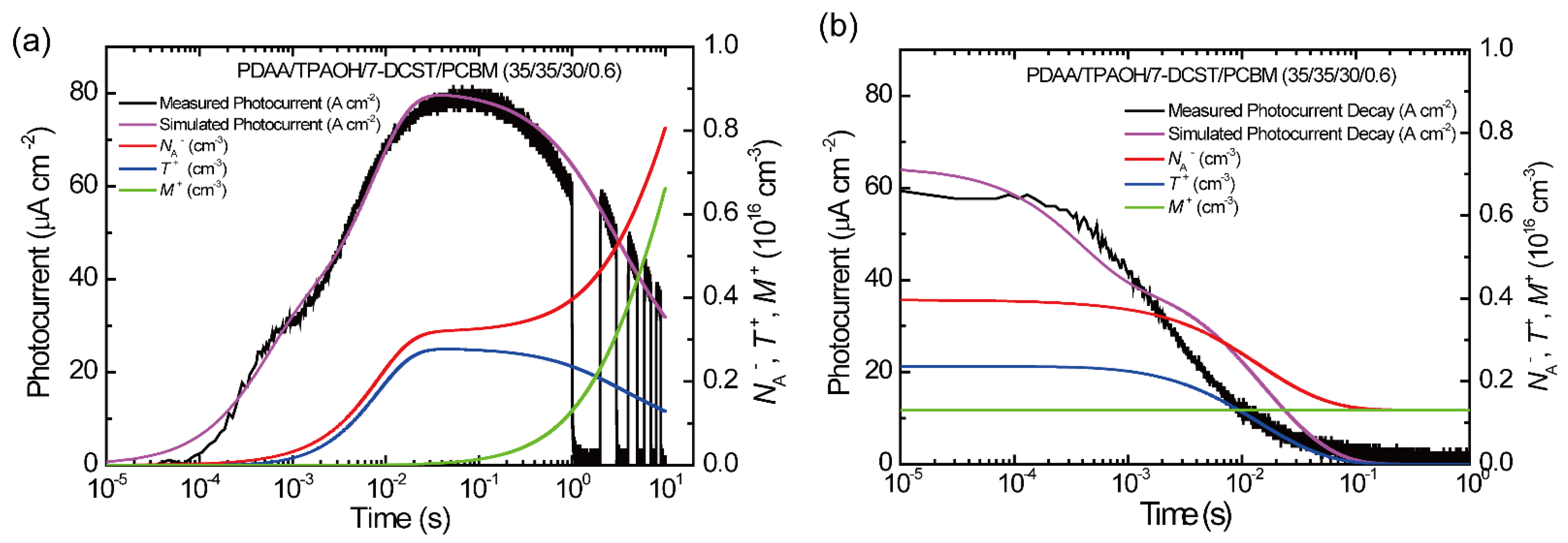


| Sample | η (%) | τ (ms) | α532/α640 | QE |
|---|---|---|---|---|
| PDAA/TPAOH/7-DCST/PCBM (35/35/30/0.6) | 39 ± 1 | 8 ± 0.8 | 200/59 | 4.3 × 10−3 |
| PDAA/TAA/7-DCST/PCBM (35/35/30/0.6) | 75 ± 0.8 | 67 ± 0.6 | 134/45 | 7.0 × 10−4 |
| PDAA/TPAOH/7-DCST/PCBM (35/35/30/0.6) | ||||||||
|---|---|---|---|---|---|---|---|---|
| QE/α (cm−1) | μ (cm2 V−1 s−1) | γT (cm3 s−1) | T (cm−3) | βT (s−1) | γM (cm3 s−1) | M (cm−3) | βM (s−1) | γR (cm3 s−1) |
| 4.3 × 10−3/59 | 3.3 × 10−6 | 1.6 × 10−13 | 1.2 × 1016 | 200 | 2.0 × 10−16 | 2.0 × 1016 | 0.01 | 2.7 × 10−13 |
| PDAA/TAA/7-DCST/PCBM (35/35/30/0.6) | ||||||||
| QE/α (cm−1) | μ (cm2 V−1 s−1) | γT (cm3 s−1) | T (cm−3) | βT (s−1) | γM (cm3 s−1) | M (cm−3) | βM (s−1) | γR (cm3 s−1) |
| 7.0 × 10−4/45 | 3.9 × 10−7 | 1.7 × 10−13 | 1.1 × 1016 | 0.2 | - | - | - | 1.4 × 10−13 |
| DOS Width (eV) | μ1 (cm2 V−1 s−1) | μ2 (cm2 V−1 s−1) | |
|---|---|---|---|
| PDAA/TPAOH/7DCST/PCBM (35/35/30/0.6) | 0.138 | 3.4 × 10−6 | 3.3 × 10−6 |
| PDAA/TAA/7DCST/PCBM (35/35/30/0.6) | 0.153 | 4.0 × 10−7 | 3.9 × 10−7 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tsutsumi, N.; Mizuno, Y.; Jackin, B.J.; Kinashi, K.; Sassa, T.; Giang, H.N.; Sakai, W. Photoelectron Yield Spectroscopy and Transient Photocurrent Analysis for Triphenylamine-Based Photorefractive Polymer Composites. Photonics 2022, 9, 996. https://doi.org/10.3390/photonics9120996
Tsutsumi N, Mizuno Y, Jackin BJ, Kinashi K, Sassa T, Giang HN, Sakai W. Photoelectron Yield Spectroscopy and Transient Photocurrent Analysis for Triphenylamine-Based Photorefractive Polymer Composites. Photonics. 2022; 9(12):996. https://doi.org/10.3390/photonics9120996
Chicago/Turabian StyleTsutsumi, Naoto, Yusuke Mizuno, Boaz Jessie Jackin, Kenji Kinashi, Takafumi Sassa, Ha Ngoc Giang, and Wataru Sakai. 2022. "Photoelectron Yield Spectroscopy and Transient Photocurrent Analysis for Triphenylamine-Based Photorefractive Polymer Composites" Photonics 9, no. 12: 996. https://doi.org/10.3390/photonics9120996
APA StyleTsutsumi, N., Mizuno, Y., Jackin, B. J., Kinashi, K., Sassa, T., Giang, H. N., & Sakai, W. (2022). Photoelectron Yield Spectroscopy and Transient Photocurrent Analysis for Triphenylamine-Based Photorefractive Polymer Composites. Photonics, 9(12), 996. https://doi.org/10.3390/photonics9120996









