Multi-Channel Polarization Manipulation Based on All-Dielectric Metasurface

Abstract

A metasurface is a planar structure that can be utilized to manipulate the amplitude, phase, and polarization of light on a subwavelength scale. Although a variety of functional optical devices based on metasurface have been proposed, the simultaneous transmission of different types of polarized waves has rarely been reported. The transmission of polarized waves with different types plays an important role in the fields of wavelength division multiplexing, quantum cryptography, and quantum computing. The simulated results in this work demonstrate that the independent manipulation of transmitted waves can be realized by the designed all-dielectric metasurface, and different types of polarized waves can be simultaneously generated. In addition, different optical responses under orthogonal circularly polarized wave incidences can be generated by this metasurface. The proposed method provides potential applications for the development of integrated optical quantum information transmission and the design of integrated optical systems on a chip.

1. Introduction

As one of the basic characteristics of an electromagnetic (EM) wave, polarization refers to the vibrational state of light in a two-dimensional space perpendicular to its propagation direction. Much research on polarization has been conducted, which has greatly promoted the application of polarization in photonics and information transmission [1]. The generation of polarization states and the conversion between different polarization states are usually fulfilled by polarizers, wave plates, or even optical systems [2]. A meticulously designed device can be applied to efficiently change the polarization state of light. Traditionally, phase delay can gradually be accumulated using birefringence in the crystal during the propagation of light. However, the optical components produced in this way are often limited by specific thickness and bulky structure, which is inconsistent with the trend of photonics integration and miniaturization [3,4].

Metasurfaces, known as artificial electromagnetic media, are typically constructed by periodically or aperiodically arranging artificial “atoms” with subwavelength dimensions. For this new type of artificial electromagnetic medium, by judiciously designing the structure, arrangement, and anisotropy of the unit cell, it is possible to achieve physical properties that do not exist in nature or are difficult to achieve, such as negative refractive index, abnormal transmission, etc. Manipulating the polarization of light is key to integrating photonics and quantum optics and the flexible and efficient control of the polarization state of light required in many fields. Currently, it has been validated that the polarization, phase, amplitude, and other characteristics of electromagnetic waves can be effectively controlled by designing subwavelength metasurface microstructures [5].

To the best of our knowledge, geometric metasurface is an effective method for phase wavefront manipulation. For the circularly polarized (CP) incidence, the geometric phase induced by rotation, called the Pancharatnam–Berry (P–B) phase, is generated by rotating the constructing units. Due to the spatial rotation operation of building units, the phase modulation of the left-handed circularly polarized (LHCP) and right-handed circularly polarized (RHCP) waves are closely interrelated [6,7], which leads to the fact that the different types of polarization states cannot be simultaneously generated by the PB phase method alone [8,9,10]. Spin decoupling realizes the independent manipulation of different circularly polarized waves by combining the propagation phase with the geometric phase. It has been demonstrated that the spin-decoupling phase control method is promising for more exotic functions such as vector holograms, achromatic lenses, etc. [11,12,13,14,15].

However, currently, there are few reports of multi-channel diffraction work based on this method. By introducing the geometrical-scaling-induced (GSI) phase modulation, Ya-Jun Gao’s group at Nanjing University demonstrated that a single metasurface composed of L-shaped resonators can simultaneously diffract both the CP wave and the LP wave [16]. However, the metasurface they designed is only suitable for the incidence of the LP wave, and the metasurface formed by the resonator will inevitably bring a certain ohmic loss relative to the all-dielectric metasurface, which will result in the loss of conversion efficiency. Notably, many other related studies are reported, but they mainly focus on the optical and microwave range, and there are few studies in the terahertz range [17,18].

The simultaneous generation of the different types of polarized waves is crucial to the fields of wavelength division multiplexing, quantum cryptography, and quantum computing [19]. However, it is still challenging to generate the different types of polarization states simultaneously, especially for all-dielectric metasurfaces [20,21,22]. In this paper, a transmissive, all-dielectric metasurface for multi-channel polarization manipulation was proposed. Specifically, when different types of circularly polarized light act perpendicularly on the designed all-dielectric metasurface, each meta-atom transmits RHCP- or LHCP-type polarized light, and additional phase modulation was determined by the geometry of each meta-atom. Through theoretical calculations, the metasurface designed in this paper can simultaneously generate four polarized beams of different types. In addition, the simulation results are consistent with the theoretical calculations, validating that the all-dielectric metasurface designed in this paper can generate multi-channel polarized waves with different polarization states when a specific polarized wave is working perpendicularly.

2. Theoretical Analysis

2.1. General Scheme for Spin Decoupling

First, we expound on the mechanism of spin decoupling for circularly polarized light, in which the output light and the input light are connected through the Jones matrix. The Jones matrix can be expressed as $J=M{\left(\alpha \right)}^{-1}TM\left(\alpha \right)$ with $M\left(\alpha \right)=\left[{}_{\sin \alpha }^{\cos \alpha }\left.{}_{\cos \alpha }{}^{-\sin \alpha }\right]\right.$, which is a rotation matrix, and the α is the rotation angle of the units. The T is the transmission matrix, which can be described as: $T=\left[{}_0^{T_{\mathrm{x}}{e}^{i{\phi }_x}}\left.{}_{T_y{e}^{i{\phi }_y}}{}^0\right]\right.$, where t_x = T_xe^iφx and t_y = T_ye^iφy; then, J can be rewritten as:

$$J=\left[\begin{array}{cc}{t}_x{\cos }^2\alpha +t_y{\sin }^2\alpha & t_x\sin \alpha \cos \alpha -t_y\sin \alpha \cos \alpha \\ t_x\sin \alpha \cos \alpha -t_y\sin \alpha \cos \alpha & t_x{\sin }^2\alpha +t_y{\cos }^2\alpha \end{array}\right]$$

(1)

Under the linear polarization base, $\left|R\right.〉=\left[\begin{array}{c}1\\ i\end{array}\right]$, $\left|L\right.〉=\left[\begin{array}{c}1\\ -i\end{array}\right]$, we assume that T_x =T_y= 1, then

$$\begin{array}{l}J\cdot \left|R\right.〉=\frac{e^{i{\phi }_x}+e^{i{\phi }_y}}{2}\cdot \left|R\right.〉+\frac{e^{i{\phi }_x}-e^{i{\phi }_y}}{2}\cdot e^{i2\alpha }\cdot \left|L\right.〉\\ J\cdot \left|L\right.〉=\frac{e^{i{\phi }_x}+e^{i{\phi }_y}}{2}\cdot \left|L\right.〉+\frac{e^{i{\phi }_x}-e^{i{\phi }_y}}{2}\cdot e^{-i2\alpha }\cdot \left|R\right.〉\end{array}$$

(2)

As the selected units all have the function of a half-wave plate, the phase difference along x- and y-axes can be expressed as Δφ = φ_x − φ_y = π, and we can obtain the following equations under these conditions:

$${\phi }_x=\frac{{\phi }_L+{\phi }_R}{2}$$

(3)

$${\phi }_x=\frac{{\phi }_L+{\phi }_R}{2}-\pi$$

(4)

$$\alpha =\frac{{\phi }_L-{\phi }_R}{4}$$

(5)

Equations (2)–(5) illustrate the correlation between spin decoupling and the structural parameters of anisotropic units. Thus, the arbitrary phase combinations of φ_L and φ_R can be generated by artificially designed structure and rotation angle. In short, by combining the PB phase with the propagation phase, the arbitrary phase distribution can be achieved at the incidence of LHCP and RHCP.

2.2. Theory of Different Types of Polarization in Transmission

Assuming that the plane wave is illuminated on a metasurface composed of a cell array (N1 × N2), the diffraction field E(k_x, k_y) at a point (Q) in the far field can be represented by the superposition of the diffraction fields induced by each unit,

$$E\left(k_x,k_y\right)=e^{i\left[-\omega t+\left(2\pi /\lambda \right)r_0\right]}\times {\displaystyle {\sum }_{u=1}^{N_1}{\displaystyle {\sum }_{v=1}^{N_2}{\displaystyle \int E\left(x^{\prime },y^{\prime }\right)}}}{e}^{-i\left[k_x\left(x^{\prime }+x_u\right)+k_y\left(y^{\prime }+y_v\right)\right]}d{x}^{\prime }d{y}^{\prime }$$

(6)

where λ is the wavelength of the plane wave, and r₀ is the distance from the center of the metasurface to the point Q. The E(x, y) is the electric field of each unit, and (x_u, y_v) is the coordinate of the center of the unit cell. Here, k_x (k_y) is the x (y) component of the wave vector. The diffraction field of each meta-atom is denoted by E_pj; thus, the nth order of the diffraction field of the metasurface can be expressed as

$$E\left(k_n\right)=e^{i[-wt+(2\pi /\lambda )r0]}N1{\displaystyle \sum _{j=1}^Q{\displaystyle {\int }_{(j-\frac{Q}{2}-1)\frac{Dx}{Q}}^{(j-\frac{Q}{2})\frac{Dx}{Q}}{E}_{Pj}}}{e}^{-i(2\pi n/Dx)x}dx.$$

(7)

From the above, it can be deduced that

$$\begin{array}{l}{E}_{\left(k=-3\right)}=a_1{{\displaystyle {\sum }_{j=1}^{8}E}}_{pj}{e}^{i\left(3\pi /4\right)\left(j-4.5\right)}\\ E_{\left(k=-1\right)}=a_1{{\displaystyle {\sum }_{j=1}^{8}E}}_{pj}{e}^{i\left(\pi /4\right)\left(j-4.5\right)}\\ E_{\left(k=1\right)}=a_1{{\displaystyle {\sum }_{j=1}^{8}E}}_{pj}{e}^{-i\left(\pi /4\right)\left(j-4.5\right)}\\ E_{\left(k=3\right)}=a_1{{\displaystyle {\sum }_{j=1}^{8}E}}_{pj}{e}^{-i\left(3\pi /4\right)\left(j-4.5\right)}\end{array}$$

(8)

where a₁ is correlated to the wavelength of the incidence wave.

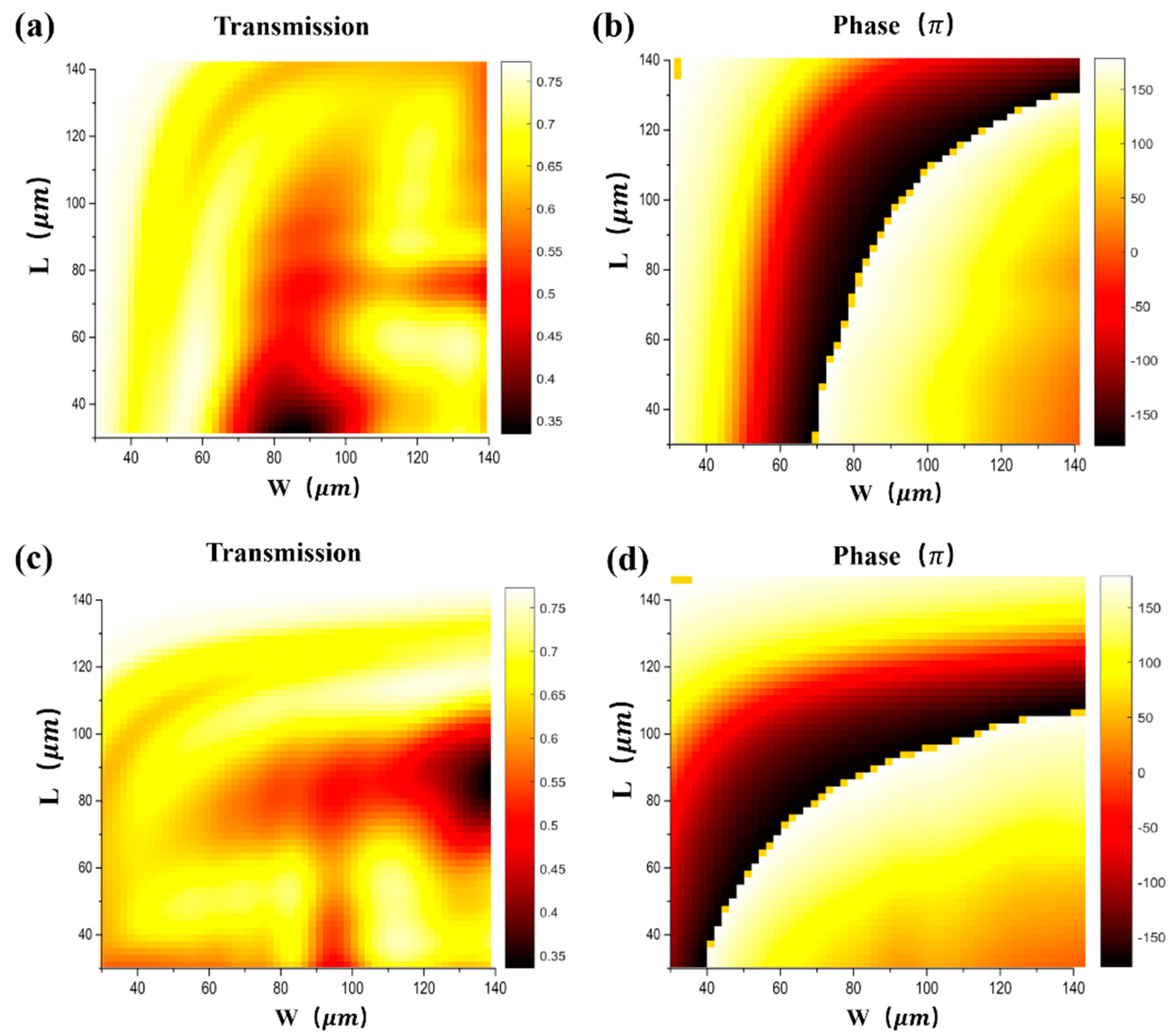
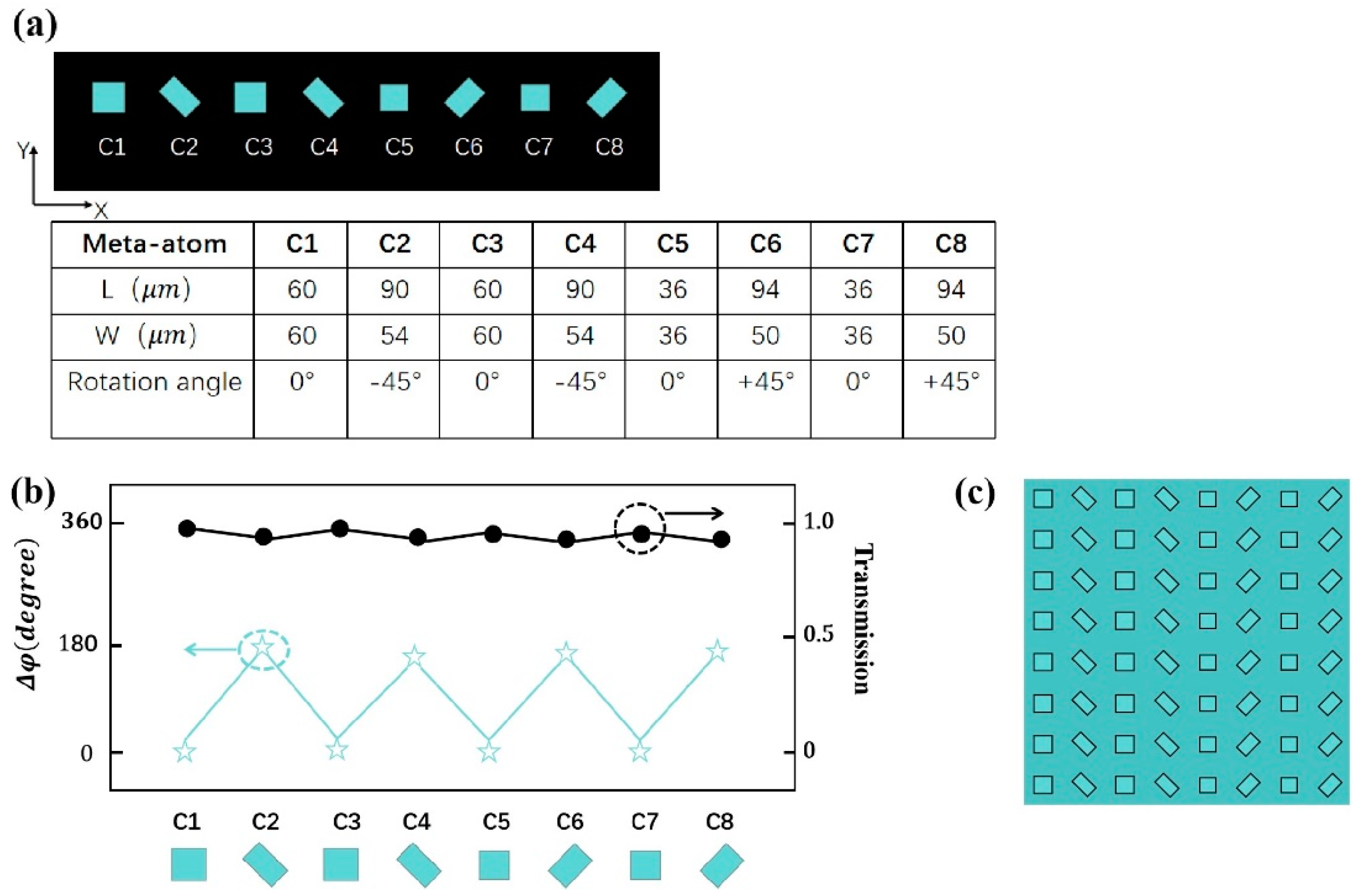
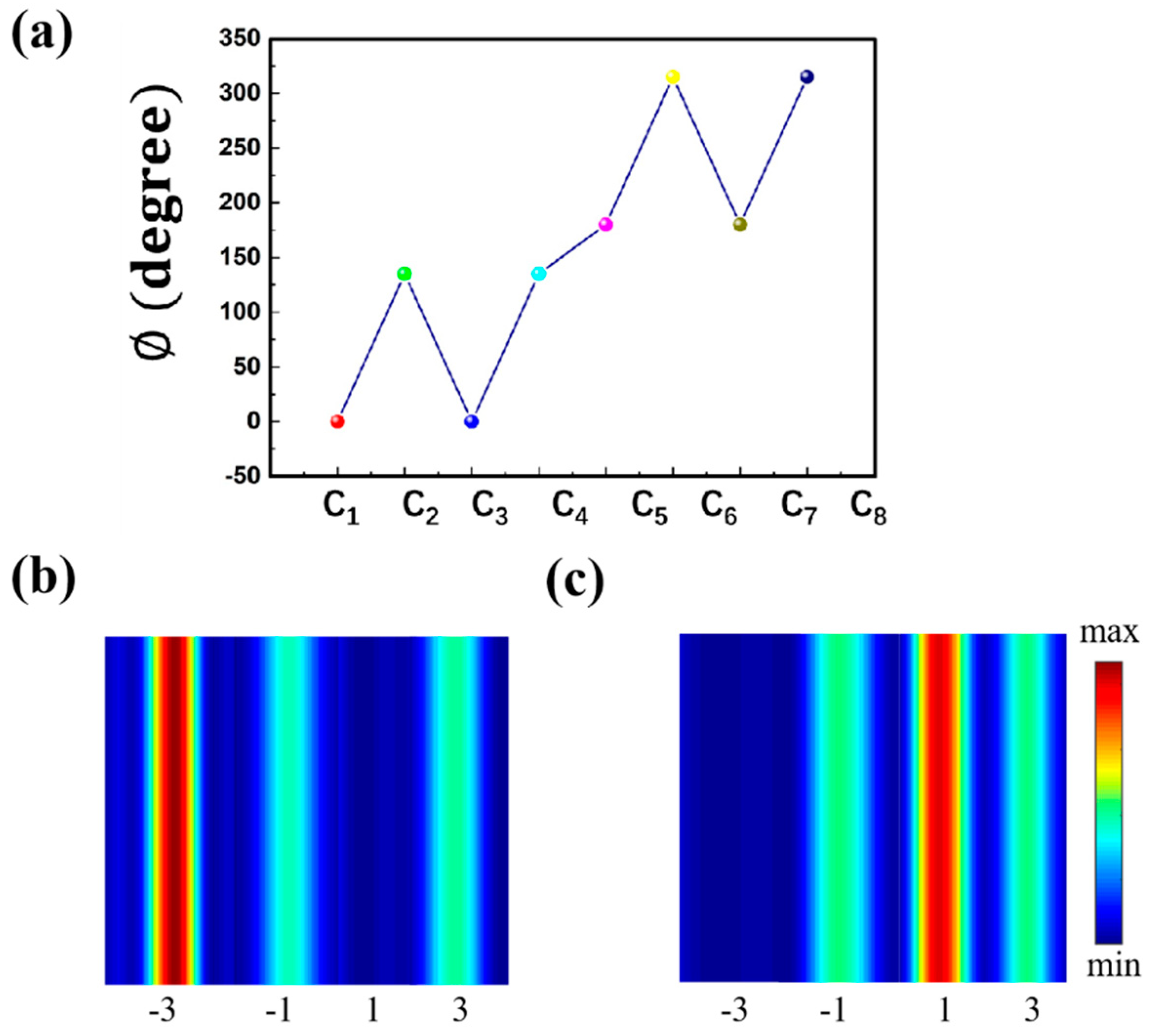
3. Metasurface Design

It is well known that the beam polarization and wave front manipulation can be realized efficiently through the rational design of metasurface.Firstly, high transmission efficiency should be provided by the design of metasurface cells. Secondly, the designed metasurface should provide 0–2 π phase control of transmission for incident waves. In addition, the π control of the phase difference between the transmission of two orthogonal polarizations should be satisfied by the designed metasurface, which is φ_x − φ_y = π.

Figure 1a schematically illustrates the polarization conversion mechanism through which the metasurface designed in this paper can simultaneously generate different polarization states. Figure 1b schematically depicts the meta-atoms of the dielectric metasurface, and the height of its substrate composed of a square (P × P) dielectric column (the material is silicon) is h1. The substrate carries a length of L, a width of W, and a dielectric pillar (the material is silicon) with a height of h2. Both the substrate and the dielectric pillar are made of all-silicon (ε = 11.9). The thicknesses of the dielectric column and the substrate dielectric column are h2 = 200 μm and h1 = 300 μm, respectively. These meta-atoms have a period of P = 150 μm. Due to the high refractive index of the silicon dielectric pillar, in addition to the electric dipole mode, the circular displacement current can also be used to obtain the magnetic dipole mode, enabling efficient light manipulation.

To investigate this ultrathin metasurface, numerical simulations were conducted utilizing CST software. The x- and y-polarized light were set to be perpendicular to the incident meta-atom, respectively, and the transmission amplitude and phase shift of the transmitted polarized light were changed by the difference in the size of the dielectric column. The simulation results are plotted in Figure 2. High transmission had a similar feature because of the particularity of the rectangular structure, and the whole 2π phase coverage could be achieved only by changing the geometric parameters of the dielectric columns under the y-polarized incidence, which can be clearly seen in Figure 2a,c. As shown in Figure 2b,d, when the x-polarized light with an operating frequency of 1.0 THz is perpendicularly incident on the meta-atom, the phase shift of the transmitted x-polarized light can be tuned in the range of 0~2π by appropriately adjusting the size of the dielectric column, and there is no obvious loss of energy, which is important for the wavefront shaping of the cross-polarized light. Therefore, the arbitrary phase difference in the transmitted x-and y-polarized light can be obtained by the careful selection of dielectric column parameters L and W.

Next, we judiciously selected eight dielectric pillars according to the simulation results shown in Figure 2. The specifications of the eight meta-atoms are shown in Figure 3a. As shown in Figure 3b, when the x-polarized light is perpendicularly incident at the frequency of 1 THz, the transmission efficiency of the eight dielectric columns is almost identical and close to 100%, which is consistent with the general job design requirements of the above-mentioned high-efficiency ultra-thin transmission components. For the selected eight dielectric columns, we also calculated the phase difference between their long and short axes, plotted in Figure 3b (indicated by the five-pointed stars). It is noteworthy that the phase difference is close to 0 for polarization-insensitive cells (dielectric columns with the same length and width dimensions). Meanwhile, for the rectangular elements, the phase difference in each element is close to π, which can be regarded as a nearly perfect half-wave plate (HWP). The rectangular element with a half-wave plate function was selected to control the orthogonal polarization components under different circular polarization incidences. Then, for the selected rectangular element, C2 and C4 were rotated 45° counterclockwise along the z-axis, and C6 and C8 were rotated 45° clockwise along the z-axis. The specification of the eight meta-atoms is shown in Figure 3a. Finally, the designed metasurface in this paper extended the unit composed of the eight selected meta-atoms (C1–C8) by eight periods in the y direction. The top view of the all-dielectric metasurface is shown in Figure 3c.

4. Results and Discussion

Next, the specific situation of the transmission field when the circularly polarized light (CP) of the two polarization states is perpendicularly incident on the all-dielectric metasurface is investigated.

When the incident light is the RHCP wave, the transmission electric field is calculated according to Formula (8) as:

$$\begin{array}{l}{E}_{\left(k=-3\right)}=-4a_1{e}^{i\left(3\pi /8\right)}(\left|H\right.〉+\left|V\right.〉)\\ E_{\left(k=-1\right)}=-(i+1)4a_1{e}^{i\left(\pi /8\right)}\left|H\right.〉\\ E_{\left(k=1\right)}=4a_1{e}^{i\left(3\pi /8\right)}(\left|H\right.〉-\left|V\right.〉)\\ E_{\left(k=3\right)}=-(i+1)4a_1{e}^{i\left(\pi /8\right)}\left|V\right.〉\end{array}$$

(9)

The corresponding simulation results are shown in Figure 4. Figure 4a shows the phase distribution of the eight well-arranged units under the RHCP wave. It can be clearly seen from Figure 4a that the phases of C1 to C8 are 0, 7π/4, 0, 7π/4, π, 3π/4, π, and 3π/4, respectively, while Figure 4b,c is the two-dimensional diagram of the intensity distribution of the $\left|H\right.〉+\left|V\right.〉$ ($\left|H\right.〉-\left|V\right.〉$) component obtained by simulation. Obviously, from the distribution of electric field intensity (Figure 4b), it can be clearly seen that the electric field intensity of the −3 diffraction order is the strongest, while the electric field intensity of −1 and +3 diffraction orders is almost half that of the −3 diffraction order, respectively, and the electric field intensity of the +1 diffraction order is almost zero. It can be seen from Figure 4c that the electric field intensity of the +1 diffraction order is the strongest, while the electric field intensity of −1 and +3 diffraction orders are almost half that of the +1 diffraction order, respectively, and the electric field intensity of the −3 diffraction order is almost zero. Combining Figure 4b,c, four beams of different polarization states are simultaneously generated by the designed metasurface under the RHCP incidence. Additionally, the four different polarization states are $\left|H\right.〉+\left|V\right.〉$ (−3 diffraction order), $\left|H\right.〉$ (−1 diffraction order), $\left|H\right.〉-\left|V\right.〉$ (+1 diffraction order), and $\left|V\right.〉$ (+3 diffraction order), respectively, which is consistent with the theoretical calculation result of Equation (9).

For the LHCP beam, the transmission electric field can be expressed according to Formula (8) as:

$$\begin{array}{l}{E}_{\left(k=-3\right)}=4a_1{e}^{i\left(3\pi /8\right)}(\left|H\right.〉+\left|V\right.〉)\\ E_{\left(k=-1\right)}=(i-1)4a_1{e}^{i\left(\pi /8\right)}\left|V\right.〉\\ E_{\left(k=1\right)}=4a_1{e}^{i\left(3\pi /8\right)}(\left|H\right.〉-\left|V\right.〉)\\ E_{\left(k=3\right)}=(i-1)4a_1{e}^{i\left(\pi /8\right)}\left|H\right.〉\end{array}$$

(10)

The corresponding simulation results are shown in Figure 5. Figure 5a shows the phase distribution of the eight well-arranged units under the LHCP wave. It can be clearly seen from Figure 5a that the phases of C1 to C8 are 0, 3π/4, 0, 3π/4, π, 7π/4, π, and 7π/4, respectively. Figure 5b,c is the two-dimensional diagram of the intensity distribution of the $\left|H\right.〉+\left|V\right.〉$ ($\left|H\right.〉-\left|V\right.〉$) component obtained under the LHCP wave. From Figure 5b, it can be seen that the electric field intensity of the −3 diffraction order is the strongest, while the electric field intensity of −1 and +3 diffraction orders is almost half of that of the −3 diffraction order, respectively, and the electric field intensity of the +1 diffraction order is almost zero. It can be seen from Figure 5c that the electric field intensity of the +1 diffraction order is the strongest, while the electric field intensity of −1 and +3 diffraction orders are almost half of that of the +1 diffraction order, respectively, and the electric field intensity of the −3 diffraction order is almost zero. Combining Figure 5b,c, four beams of different polarization states are simultaneously generated by the designed metasurface under the LHCP incidence. Additionally, the four different polarization states are $\left|H\right.〉+\left|V\right.〉$ (−3 diffraction order), $\left|V\right.〉$ (−1 diffraction order), $\left|H\right.〉-\left|V\right.〉$ (+1 diffraction order), and $\left|H\right.〉$ (+3 diffraction order), respectively, which is consistent with the theoretical calculation result of Equation (10).

In summary, the designed metasurface in this paper can realize the independent manipulation of the right-handed circularly polarized wave or the left-handed circularly polarized wave. Moreover, four beams of different polarization states are simultaneously generated under both the RHCP wave and the LHCP wave.

5. Conclusions

In conclusion, we proposed an all-dielectric metasurface that can simultaneously generate four different polarization states. By rationally choosing the geometric size and symmetry of each meta-atom in the unit of the metasurface, the premeditated design period parameters, etc., the output polarization state, the number of output waves, and the propagation direction of each wave can be precisely controlled. This design method is expected to be very advantageous for quantum information science problems, such as information encoding, transmission, and processing. We believe it also provides a new avenue for developing principle optical devices in future integrated photonics and integrated technologies.