Numerical and Experimental Study on Thermal Damage Induced by Medium—Infrared Laser
Abstract
1. Introduction
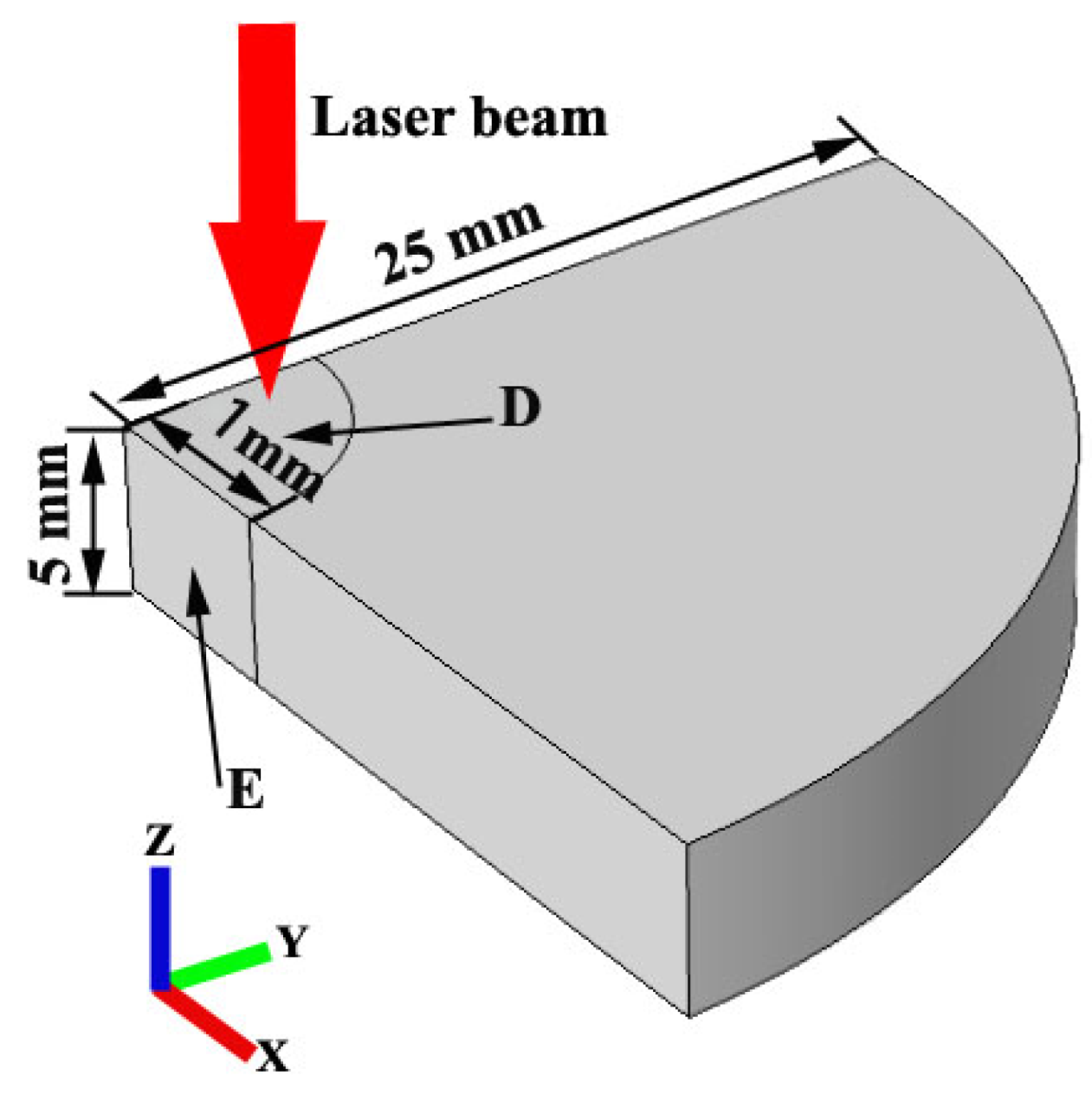
2. Geometric Model and Method
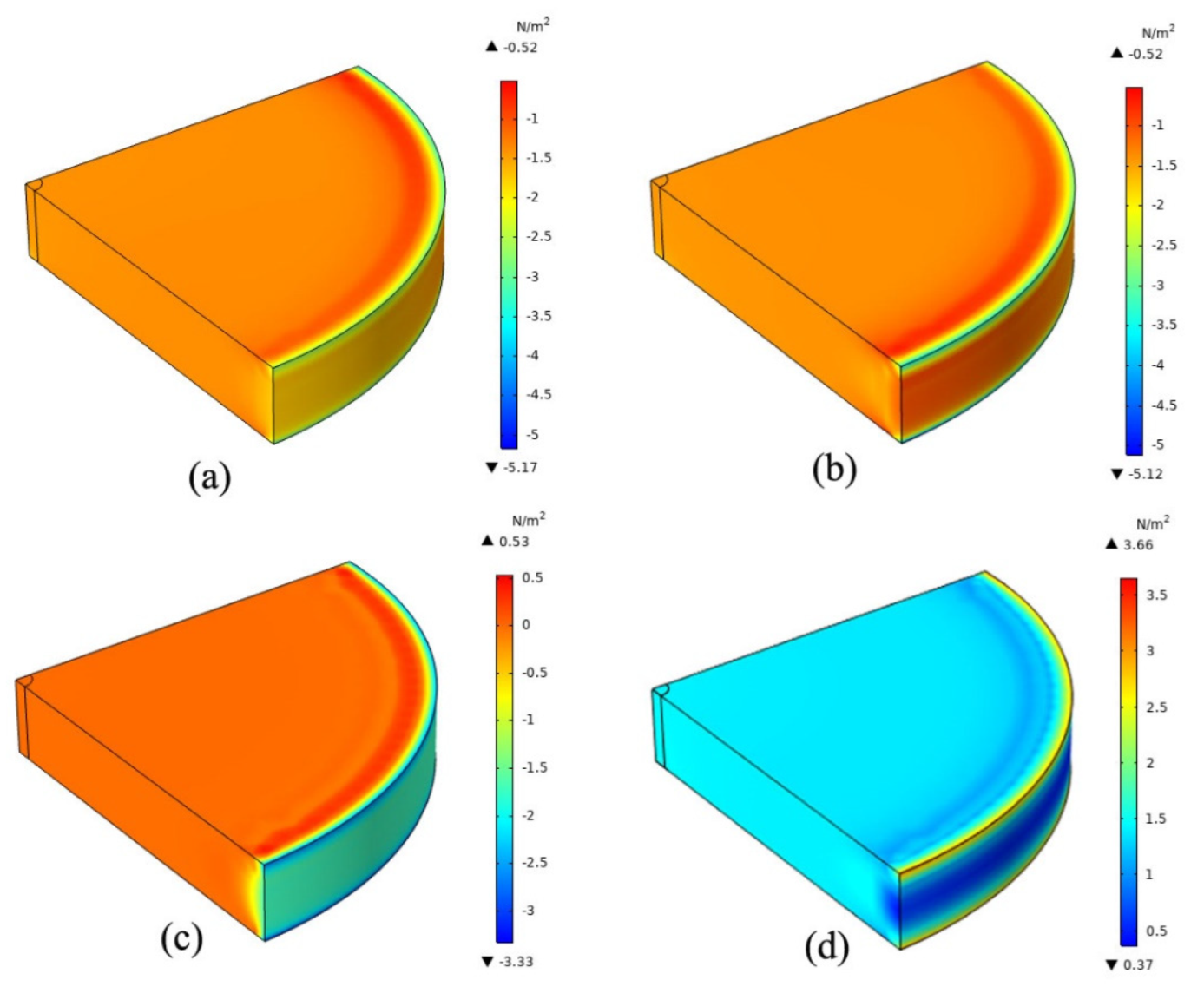
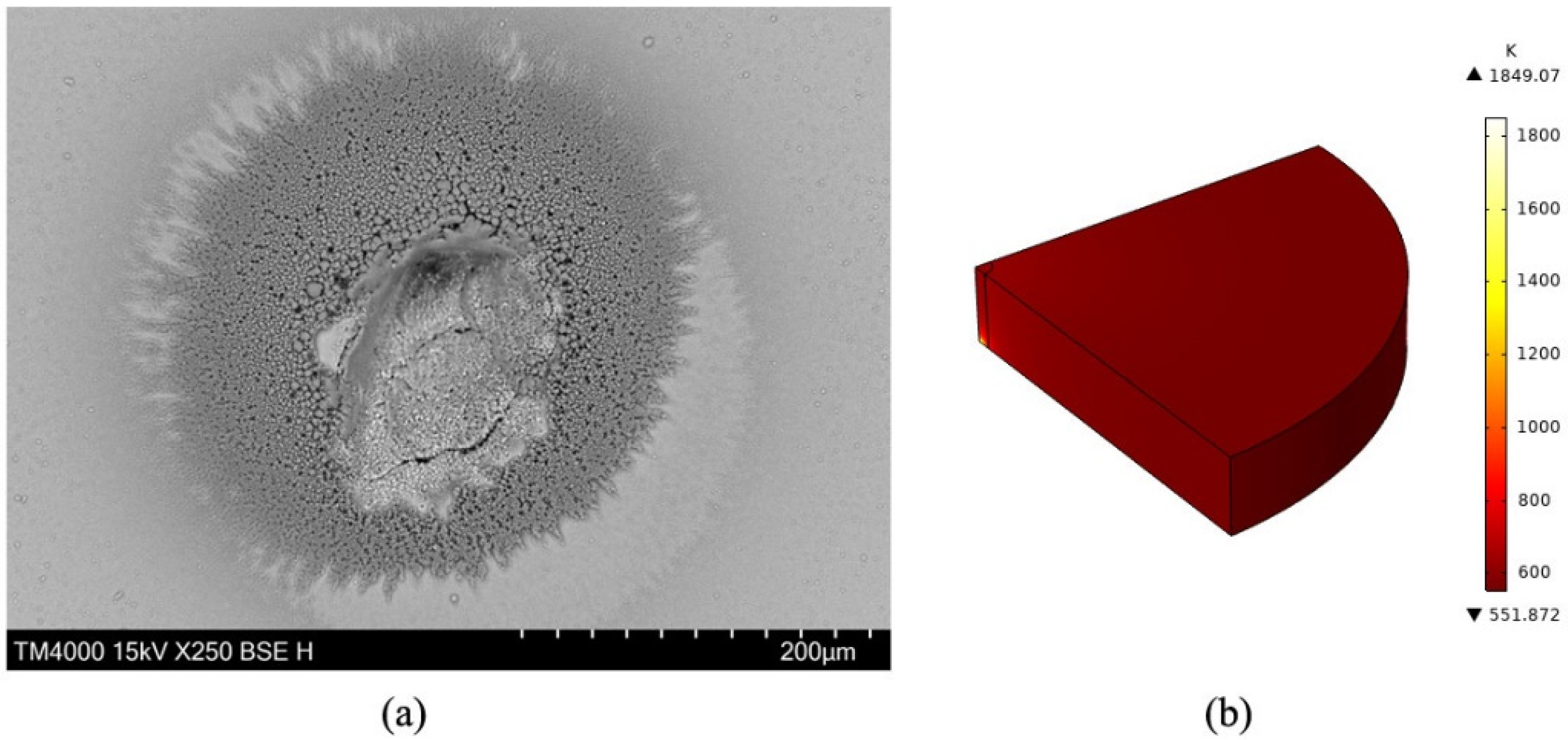
3. Analysis and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Jauregui, C.; Limpert, J.; Tünnermann, A. High-power fibre lasers. Nat. Photonics 2013, 11, 861–867. [Google Scholar] [CrossRef]
- Wang, D.Y.; Yi, Z.; Ma, G.L.; Dai, B.; Yang, J.B.; Zhang, J.F.; Yu, Y.; Liu, C.; Wu, X.W.; Bian, Q. Two channels photonic crystal fiber based on surface plasmon resonance for magnetic field and temperature dual-parameter sensing. Phys. Chem. Chem. Phys. 2022, 24, 21233. [Google Scholar] [CrossRef] [PubMed]
- Li, L.; Gao, H.; Liu, G.; Wang, S.; Yi, Z.; Wu, X.; Yang, H. Synthesis of carnation flower-like Bi2O2CO3 photocatalyst and its promising application for photoreduction of Cr(VI). Adv. Powder Technol. 2022, 33, 103481. [Google Scholar] [CrossRef]
- De Araújo, C.B.; Gomes, A.S.L.; Boudebs, G. Techniques for nonlinear optical characterization of materials: A review. Rep. Prog. Phys. 2016, 79, 036401. [Google Scholar] [CrossRef] [PubMed]
- Chen, H.; Chen, Z.; Yang, H.; Wen, L.; Yi, Z.; Zhou, Z.; Dai, B.; Zhang, J.; Wu, X.; Wu, P. Multi-mode surface plasmon resonance absorber based on dart-type single-layer graphene. RSC Adv. 2022, 12, 7821–7829. [Google Scholar] [CrossRef]
- Lin, X.; Li, M.; Li, Y.J.; Chen, W. Enhancement of the catalytic activity of ordered mesoporous TiO2 by using carbon fiber support and appropriate evaluation of synergy between surface adsorption and photocatalysis by Langmuir-Hinshelwood (L-H) integration equation. RSC Adv. 2015, 5, 105227–105238. [Google Scholar] [CrossRef]
- Cao, W.X.; Lin, L.; Qi, H.F.; He, Q.; Wu, Z.J.; Wang, A.Q.; Luo, W.H.; Zhang, T. In-situ synthesis of single-atom Ir by utilizing metal-organic frameworks: An acid-resistant catalyst for hydrogenation of levulinic acid to gamma-valerolactone. J. Catal. 2019, 373, 161–172. [Google Scholar] [CrossRef]
- Li, H.; Li, G.; Wu, K.; Zhang, B.; Yang, Z.; Pan, S. BaB2S4: An Efficient and Air-stable Thioborate as Infrared Nonlinear Optical Material with High Laser Damage Threshold. Chem. Mater. 2018, 30, 7428–7432. [Google Scholar] [CrossRef]
- Shangguan, Q.Y.; Chen, Z.H.; Yang, H.; Cheng, S.B.; Yang, W.X.; Yi, Z.; Wu, X.W.; Wang, S.F.; Yi, Y.G.; Wu, P.H. Design of Ultra-Narrow Band Graphene Refractive Index Sensor. Sensors 2022, 22, 6483. [Google Scholar] [CrossRef]
- Emmony, D. Laser mirror damage in germanium at 10.6 μm. Appl. Phys. Lett. 1973, 23, 598. [Google Scholar] [CrossRef]
- Laurence, T.; Bude, J.; Ly, S.; Shen, N.; Feit, M. Extracting the distribution of laser damage precursors on fused silica surfaces for 351 nm, 3 ns laser pulses at high fluences (20–150 J/cm2). Opt. Express 2012, 20, 11561. [Google Scholar] [CrossRef]
- Luo, W.H.; Cao, W.X.; Bruijnincx, P.C.A.; Lin, L.; Wang, A.Q.; Zhang, T. Zeolite-supported metal catalysts for selective hydrodeoxygenation of biomass-derived platform molecules. Green Chem. 2019, 21, 3744–3768. [Google Scholar] [CrossRef]
- Couairon, A.; Sudrie, L.; Franco, M.; Prade, B.; Mysyrowicz, A. Filamentation and damage in fused silica induced by tightly focused femtosecond laser pulses. Phys. Rev. B 2005, 71, 125435. [Google Scholar] [CrossRef]
- Michael, A.S.; Kirchner, G.; Koidl, F.; Wang, P.; Jilete, B.; Flohrer, T. Daylight space debris laser ranging. Nat. Commun. 2020, 11, 3735. [Google Scholar] [CrossRef]
- Chandler-Horowitz, D.; Amirtharaj, P.M. High-accuracy, midinfrared (450 cm−1 ≤ ω ≤ 4000 cm-1) refractive index values of silicon. J. Appl. Phys. 2005, 97, 123526. [Google Scholar] [CrossRef]
- Fu, C.; Zhang, Z. Nanoscaleradiation heat transferfor silicon at different doping levels. Int. J. Heat Mass Transf. 2006, 49, 1703–1718. [Google Scholar] [CrossRef]
- Wang, X.; Qin, Y.; Wang, B.; Zhang, L.; Shen, Z.H.; Lu, J.; Ni, X.W. Numerical and experimental study of the thermal stress of silicon induced by a millisecond laser. Appl. Opt. 2011, 50, 3725–3732. [Google Scholar] [CrossRef] [PubMed]
- Wang, B.L.; Li, J.E. Thermal shock resistance of solids associated with hyperbolic heat conduction theory. Proc. R. Soc. A Math. Phys. Eng. Sci. 2013, 469, 20120754. [Google Scholar] [CrossRef]
- Choi, S.; Jhang, K. Thermal damages on the surface of a silicon wafer induced by a near-infrared laser. Opt. Eng. 2014, 53, 017103. [Google Scholar] [CrossRef][Green Version]
- Rämer, A.; Osmani, O.; Rethfeld, B. Laser damage in silicon: Energy absorption, relaxation, and transport. J. Appl. Phys. 2014, 116, 053508. [Google Scholar] [CrossRef]
- Exarhos, G.; Gruzdev, V.; Menapace, J.; Ristau, D.; Soileau, M.; Bude, J.; Miller, P.; Shen, N.; Suratwala, T.; Laurence, T.; et al. Silica laser damage mechanisms, precursors and their mitigation. In Laser-Induced Damage in Optical Materials: 2014; SPIE: Boulder, CO, USA, 2014; Volume 9237, p. 92370S. [Google Scholar] [CrossRef]
- Matthews, M.; Yang, S.; Shen, N.; Elhadj, S.; Raman, R.; Guss, G.; Bass, I.; Nostrand, M.; Wegner, P. Micro-Shaping, Polishing, and Damage Repair of Fused Silica Surfaces Using Focused Infrared Laser Beams. Adv. Eng. Mater. 2015, 17, 247–252. [Google Scholar] [CrossRef]
- Doualle, T.; Gallais, L.; Cormont, P.; Hébert, D.; Combis, P.; Rullier, J. Thermo-mechanical simulations of CO2 laser–fused silica interactions. J. Appl. Phys. 2016, 119, 113106. [Google Scholar] [CrossRef]
- Li, M.; Jin, G.; Tan, Y. Simulation of the Si-CCD irradiated by millisecond pulse laser. Optik Int. J. Light Electron. Opt. 2017, 131, 67–71. [Google Scholar] [CrossRef]
- Zheng, C.; Shao, J.; Li, X.; Wang, H.; Wang, C.; Chen, F.; Wang, T.; Guo, J. Femtosecond pulsed laser induced damage characteristics on Si-based multi-layer film. Chin. Opt. Lett. 2019, 12, 371–381. [Google Scholar] [CrossRef]
- Kou, Z.; Li, Y.; Wang, G.; Li, K.; Hou, Y.; Xie, X.; Yu, Y.; Wang, Y.; Lu, Z. Temporal evolution characteristics and mechanism analysis of CCD breakdown induced by nanosecond and picosecond pulse lasers. Optik 2022, 162, 169313. [Google Scholar] [CrossRef]
- Sun, L.; Huang, J.; Liu, H.; Ye, X.; Wu, J.; Jiang, X.; Yang, L.; Zheng, W.; Wu, W. Combination of reaction ion etching and dynamic chemical etching for improving laser damage resistance of fused silica optical surfaces. Opt. Lett. 2016, 41, 4464–4467. [Google Scholar] [CrossRef]
- Liu, X.; Lu, X.; Wen, P.; Shu, X.; Chi, F. Synthesis of ultrasmall silica nanoparticles for application as deep-ultraviolet antireflection coatings. Appl. Surf. Sci. 2017, 420, 180–185. [Google Scholar] [CrossRef]
- Cheng, Z.; Liao, J.; He, B.; Zhang, F.; Zhang, F.; Huang, X.; Zhou, L. One-Step Fabrication of Graphene Oxide Enhanced Magnetic Composite Gel for Highly Efficient Dye Adsorption and Catalysis. ACS Sustain. Chem. Eng. 2015, 3, 1677–1685. [Google Scholar] [CrossRef]
- Li, Y.; Li, M.; Xu, P.; Tang, S.; Liu, C. Efficient photocatalytic degradation of acid orange 7 over N-doped ordered mesoporous titania on carbon fibers under visible-light irradiation based on three synergistic effects. Appl. Catal. A Gen. 2016, 524, 163–172. [Google Scholar] [CrossRef]
- Cai, L.; Zhang, Z.; Xiao, H.; Chen, S.; Fu, J. An eco-friendly imprinted polymer based on graphene quantum dots for fluorescent detection of p-nitroaniline. RSC Adv. 2019, 9, 41383–41391. [Google Scholar] [CrossRef]
- Lv, P.; Xie, D.; Zhang, Z. Magnetic carbon dots based molecularly imprinted polymers for fluorescent detection of bovine hemoglobin. Talanta 2018, 188, 145–151. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Bo, M.; Yang, X.; Zhang, P.; Sun, C.Q.; Huang, Y. Size modulation electronic and optical properties of phosphorene nanoribbons: DFT–BOLS approximation. Phys. Chem. Chem. Phys. 2017, 19, 5304–5309. [Google Scholar] [CrossRef] [PubMed]
- Long, F.; Zhang, Z.; Wang, J.; Yan, L.; Zhou, B. Cobalt-nickel bimetallic nanoparticles decorated graphene sensitized imprinted electrochemical sensor for determination of octylphenol. Electrochim. Acta 2015, 168, 337–345. [Google Scholar] [CrossRef]
- Zheng, Z.; Luo, Y.; Yang, H.; Yi, Z.; Zhang, J.; Song, Q.; Yang, W.; Liu, C.; Wu, X.; Wu, P. Thermal tuning of terahertz metamaterial properties based on phase change material vanadium dioxide. Phys. Chem. Chem. Phys. 2022, 24, 8846–8853. [Google Scholar] [CrossRef] [PubMed]
- Zhou, F.; Qin, F.; Yi, Z.; Yao, W.-T.; Liu, Z.; Wu, X.; Wu, P. Ultra-wideband and wide-angle perfect solar energy absorber based on Ti nanorings surface plasmon resonance. Phys. Chem. Chem. Phys. 2021, 23, 17041–17048. [Google Scholar] [CrossRef] [PubMed]
- Deng, Y.; Cao, G.; Wu, Y.; Zhou, X.; Liao, W. Theoretical Description of Dynamic Transmission Characteristics in MDM Waveguide Aperture-Side-Coupled with Ring Cavity. Plasmonics 2015, 10, 1537–1543. [Google Scholar] [CrossRef]
- Zhang, Z.; Cai, R.; Long, F.; Wang, J. Development and application of tetrabromobisphenol A imprinted electrochemical sensor based on graphene/carbon nanotubes three-dimensional nanocomposites modified carbon electrode. Talanta 2015, 134, 435–442. [Google Scholar] [CrossRef]
- Lin, X.; Li, Y.J.; Chen, F.T.; Xu, P.; Li, M. Facile synthesis of mesoporous titanium dioxide doped by Ag-coated graphene with enhanced visible-light photocatalytic performance for methylene blue degradation. RSC Adv. 2017, 7, 25314–25324. [Google Scholar] [CrossRef]
- Li, J.; Jiang, J.; Xu, Z.; Liu, M.; Tang, S.; Yang, C.; Qian, D. Facile synthesis of Ag@Cu2O heterogeneous nanocrystals decorated N-doped reduced graphene oxide with enhanced electrocatalytic activity for ultrasensitive detection of H2O2. Sens. Actuators B Chem. 2018, 260, 529–540. [Google Scholar] [CrossRef]
- Zhao, F.; Lin, J.; Lei, Z.; Yi, Z.; Qin, F.; Zhang, J.; Liu, L.; Wu, X.; Yang, W.; Wu, P. Realization of 18.97% theoretical efficiency of 0.9 μm thick c-Si/ZnO heterojunction ultrathin-film solar cells via surface plasmon resonance enhancement. Phys. Chem. Chem. Phys. 2022, 24, 4871–4880. [Google Scholar] [CrossRef]
- Deng, Y.; Cao, G.; Yang, H.; Zhou, X.; Wu, Y. Dynamic Control of Double Plasmon-Induced Transparencies in Aperture-Coupled Waveguide-Cavity System. Plasmonics 2018, 13, 345–352. [Google Scholar] [CrossRef]
- Tang, N.; Li, Y.; Chen, F.; Han, Z. In situ fabrication of a direct Z-scheme photocatalyst by immobilizing CdS quantum dots in the channels of graphene-hybridized and supported mesoporous titanium nanocrystals for high photocatalytic performance under visible light. RSC Adv. 2018, 8, 42233–42245. [Google Scholar] [CrossRef] [PubMed]
- Li, J.; Jiang, J.; Zhao, D.; Xu, Z.; Liu, M.; Liu, X.; Tong, H.; Qian, D. Novel hierarchical sea urchin-like Prussian blue@palladium core–shell heterostructures supported on nitrogen-doped reduced graphene oxide: Facile synthesis and excellent guanine sensing performance. Electrochim. Acta 2020, 330, 135196. [Google Scholar] [CrossRef]
- He, J.; Qian, T.; Cai, C.; Xiang, X.; Li, S.; Zu, X. Nickel-Based Selenides with a Fractal Structure as an Excellent Bifunctional Electrocatalyst for Water Splitting. Nanomaterials 2022, 12, 281. [Google Scholar] [CrossRef] [PubMed]
- Xiao, L.; Zhang, Q.I.; Chen, P.; Chen, L.; Ding, F.; Tang, J.; Li, Y.J.; Au, C.T.; Yin, S.F. Copper-mediated metal-organic framework as efficient photocatalyst for the partial oxidation of aromatic alcohols under visible-light irradiation: Synergism of plasmonic effect and schottky junction. Appl. Catal. B Environ. 2019, 248, 380–387. [Google Scholar] [CrossRef]
- Zheng, Z.; Zheng, Y.; Luo, Y.; Yi, Z.; Zhang, J.; Liu, Z.; Yang, W.; Yu, Y.; Wu, X.; Wu, P. A switchable terahertz device combining ultra-wideband absorption and ultra-wideband complete reflection. Phys. Chem. Chem. Phys. 2022, 24, 2527–2533. [Google Scholar] [CrossRef]
- Lin, X.; Du, S.W.; Li, C.H.; Li, G.J.; Li, Y.J.; Chen, F.T.; Fang, P.F. Consciously constructing the robust NiS/g-C3N4 hybrids for enhanced photocatalytic hydrogen evolution. Catal. Lett. 2020, 150, 1898–1908. [Google Scholar] [CrossRef]
- Chen, P.; Liu, F.; Ding, H.; Chen, S.; Chen, L.; Li, Y.-J.; Au, C.-T.; Yin, S.-F. Porous double-shell CdS@C3N4 octahedron derived by in situ supramolecular self-assembly for enhanced photocatalytic activity. Appl. Catal. B Environ. 2019, 252, 33–40. [Google Scholar] [CrossRef]
- Wu, X.; Zheng, Y.; Luo, Y.; Zhang, J.; Yi, Z.; Wu, X.; Cheng, S.; Yang, W.; Yu, Y.; Wu, P. A four-band and polarization-independent BDS-based tunable absorber with high refractive index sensitivity. Phys. Chem. Chem. Phys. 2021, 23, 26864–26873. [Google Scholar] [CrossRef]
- Liu, H.; Wang, Q.; Zhang, F. Preparation of Fe3O4@SiO2@ P(AANa-co-AM) Composites and Their Adsorption for Pb(II). ACS Omega 2020, 5, 8816–8824. [Google Scholar] [CrossRef]
- Cao, G.; Li, H.; Deng, Y.; Zhan, S.; He, Z.; Li, B. Systematic Theoretical Analysis of Selective-Mode Plasmonic Filter Based on Aperture-Side-Coupled Slot Cavity. Plasmonics 2014, 9, 1163–1169. [Google Scholar] [CrossRef]
- Cheng, T.; Gao, H.; Liu, G.; Pu, Z.; Wang, S.; Yi, Z.; Wu, X.; Yang, H. Preparation of core-shell heterojunction photocatalysts by coating CdS nanoparticles onto Bi4Ti3O12 hierarchical microspheres and their photocatalytic removal of organic pollutants and Cr(VI) ions. Colloids Surf. A Physicochem. Eng. Asp. 2021, 633, 127918. [Google Scholar] [CrossRef]
- Cai, C.; Han, S.B.; Zhang, X.T.; Yu, J.X.; Xiang, X.; Yang, J.; Qiao, L.; Zu, X.T.; Chen, Y.Z.; Li, S.A. Ultrahigh oxygen evolution reaction activity in Au doped co-based nanosheets. RSC Adv. 2022, 12, 6205–6213. [Google Scholar] [CrossRef] [PubMed]
- Long, F.; Wang, J.; Zhang, Z.; Yan, L. Magnetic imprinted electrochemical sensor combined with magnetic imprinted solid-phase extraction for rapid and sensitive detection of tetrabromobisphenol S. J. Electroanal. Chem. 2016, 777, 58–66. [Google Scholar] [CrossRef]
- Gu, Y.F.; Guo, B.B.; Yi, Z.; Wu, X.W.; Zhang, J.; Yang, H. Synthesis of a Self-assembled Dual Morphologies Ag-NPs/SrMoO4 Photocatalyst with LSPR Effect for the Degradation of Methylene Blue Dye. ChemistrySelect 2022, 7, e202201274. [Google Scholar] [CrossRef]
- Li, L.; Sun, X.; Xian, T.; Gao, H.; Wang, S.; Yi, Z.; Wu, X.; Yang, H. Template-free synthesis of Bi2O2CO3 hierarchical nanotubes self-assembled from ordered nanoplates for promising photocatalytic applications. Phys. Chem. Chem. Phys. 2022, 24, 8279–8295. [Google Scholar] [CrossRef]
- Bacar, Z. Modeling of CW laser diode irradiation of amorphous silicon films. Appl. Surf. Sci. 2011, 257, 5127–5131. [Google Scholar] [CrossRef]
- Paul, I. Statistical fracture modelling of silicon with varying thickness. Acta Mater. 2006, 54, 3991–4000. [Google Scholar] [CrossRef]
- Li, L.; Gao, H.; Yi, Z.; Wang, S.; Wu, X.; Li, R.; Yang, H. Comparative investigation on synthesis, morphological tailoring and photocatalytic activities of Bi2O2CO3 nanostructures. Colloids Surf. A Physicochem. Eng. Asp. 2022, 644, 128758. [Google Scholar] [CrossRef]
- Gu, Y.F.; Guo, B.B.; Yi, Z.; Wu, X.W.; Zhang, J.; Yang, H. Morphology modulation of hollow-shell ZnSn(OH)6 for enhanced photodegradation of methylene blue. Colloids Surf. A Physicochem. Eng. Asp. 2022, 653, 129908. [Google Scholar] [CrossRef]
- Tang, B.; Ren, Y. Tunable and switchable multi-functional terahertz metamaterials based on a hybrid vanadium dioxide–graphene integrated configuration. Phys. Chem. Chem. Phys. 2022, 24, 8408–8414. [Google Scholar] [CrossRef] [PubMed]
- Drake, R.A.; Kyle, R.P.K.; Lai, Y.; Wang, Z.; Cosmin, I.B.; Enam, A.C. Femtosecond laser damage of germanium from near- to mid-infrared wavelengths. Opt. Lett. 2018, 43, 3702. [Google Scholar] [CrossRef]
- Veres, I.; Berer, T.; Burgholzer, P. Numerical modeling of thermoelastic generation of ultrasound by laser irradiation in the coupled thermoelasticity. Ultrasonics 2013, 53, 141–149. [Google Scholar] [CrossRef] [PubMed]






| Thermodynamic Parameters of Silicon Materials | ||
|---|---|---|
| Si | Solid | Liquid |
| Density (kg/cm3) | 2330 − 2.19 × 10−2 × T | 2330 − 2.19 × 10−2 × T − 1.21 × 10−5 × T2 |
| Thermal conductivity (W/(m*K)) | 22.23 + 422.52 × exp(−T/255.45) | 62 |
| Heat capacity (J/kg*K) | 352.43 + 1.78 × T − 2.21 × 10−3 × T2 + 1.3 × 10−6 × T3 − 2.83 × 10−10 × T4 | 1021.84 |
| Absorption coefficient (1/m) | 1023 × (T/273)4 | 8.6 × 107 |
| Reflectivity | 0.08 | 0.72 |
| Young’s modulus (Gpa) | 107 | |
| Thermal expansion coefficient (1/K) | 2 × 10−5 | |
| Poisson’s ratio | 0.28 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lei, Z.; Zhang, Y.; Li, Q.; Shao, T.; Sun, L.; Wang, K.; Yi, Z.; Ye, X.; Zheng, W.; Wu, P. Numerical and Experimental Study on Thermal Damage Induced by Medium—Infrared Laser. Photonics 2022, 9, 838. https://doi.org/10.3390/photonics9110838
Lei Z, Zhang Y, Li Q, Shao T, Sun L, Wang K, Yi Z, Ye X, Zheng W, Wu P. Numerical and Experimental Study on Thermal Damage Induced by Medium—Infrared Laser. Photonics. 2022; 9(11):838. https://doi.org/10.3390/photonics9110838
Chicago/Turabian StyleLei, Zhenhua, Yubin Zhang, Qingzhi Li, Ting Shao, Laixi Sun, Kaixuan Wang, Zao Yi, Xin Ye, Wanguo Zheng, and Pinghui Wu. 2022. "Numerical and Experimental Study on Thermal Damage Induced by Medium—Infrared Laser" Photonics 9, no. 11: 838. https://doi.org/10.3390/photonics9110838
APA StyleLei, Z., Zhang, Y., Li, Q., Shao, T., Sun, L., Wang, K., Yi, Z., Ye, X., Zheng, W., & Wu, P. (2022). Numerical and Experimental Study on Thermal Damage Induced by Medium—Infrared Laser. Photonics, 9(11), 838. https://doi.org/10.3390/photonics9110838









