Mode-Conversion-Based Chirped Bragg Gratings on Thin-Film Lithium Niobate
Abstract
1. Introduction
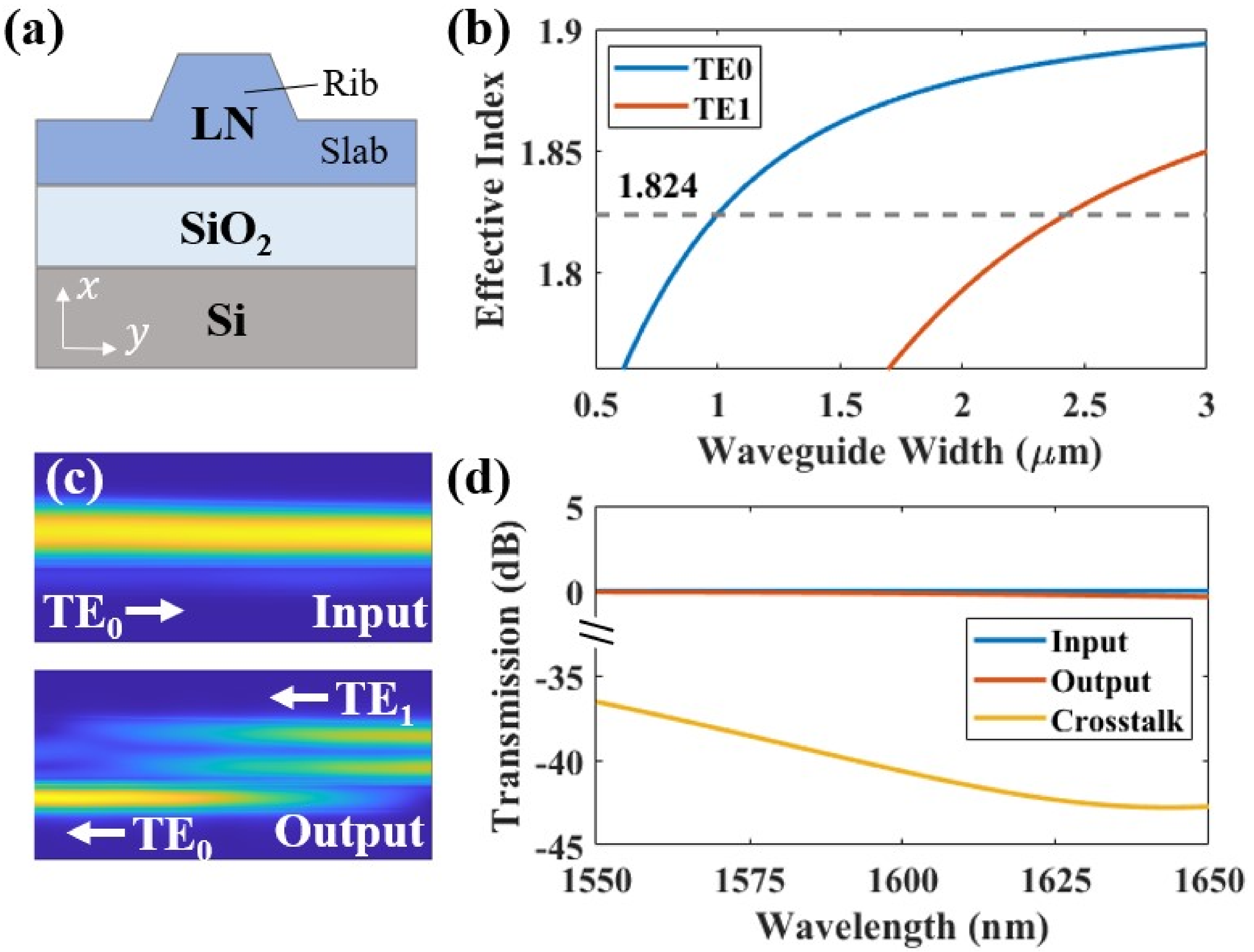
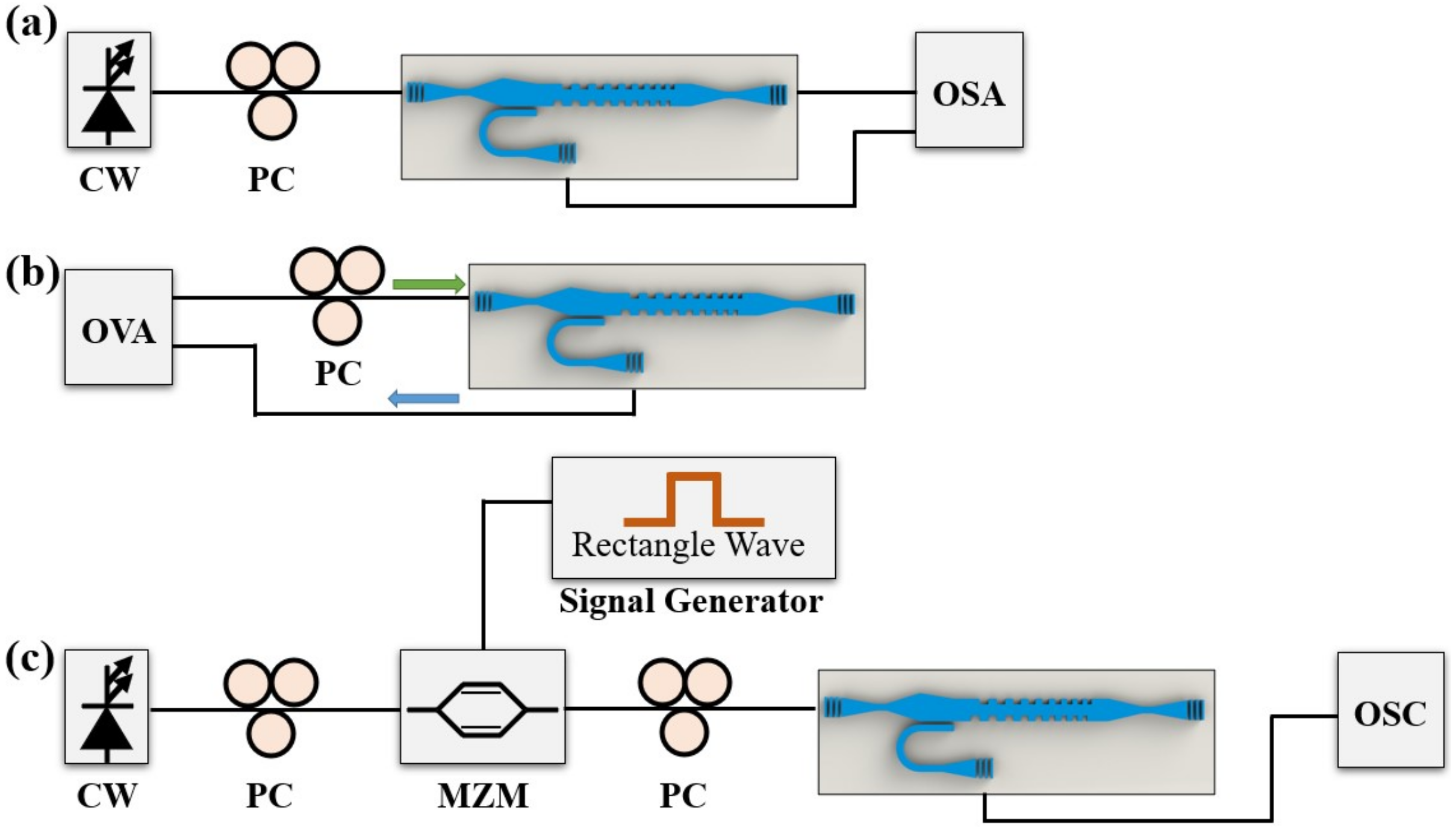
2. Methods
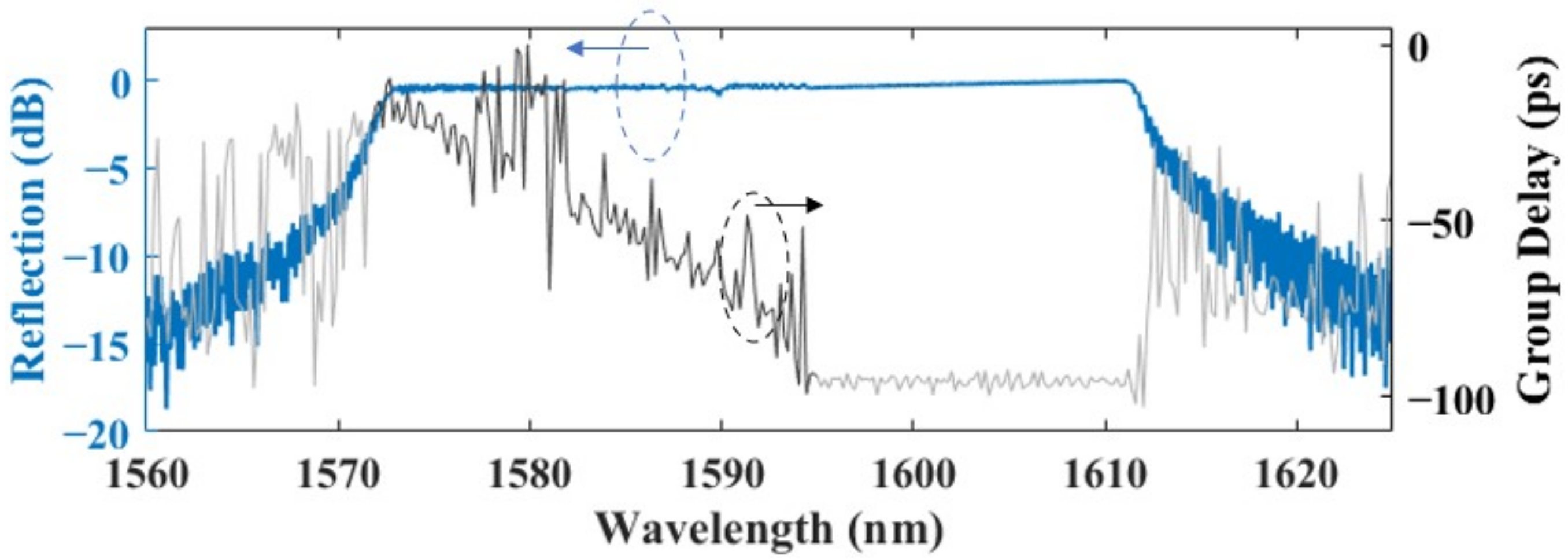
3. Results
4. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sumetsky, M.; Eggleton, B. Fiber Bragg gratings for dispersion compensation in optical communication systems. J. Opt. Fiber Commun. Rep. 2005, 2, 256–278. [Google Scholar] [CrossRef]
- Mustafa, F.M.; Zaky, S.A.; Khalaf, A.A.M.; Aly, M.H. A cascaded FBG scheme based OQPSK/DPSK modulation for chromatic dispersion compensation. Opt. Quantum Electron. 2022, 54, 7. [Google Scholar] [CrossRef]
- Min, R.; Korganbayev, S.; Molardi, C.; Broadway, C.; Hu, X.; Caucheteur, C.; Bang, O.; Antunes, P.; Tosi, D.; Marques, C.; et al. Largely tunable dispersion chirped polymer FBG. Opt. Lett. 2018, 43, 5106–5109. [Google Scholar] [CrossRef]
- Min, R.; Ortega, B.; Marques, C. Fabrication of tunable chirped mPOF Bragg gratings using a uniform phase mask. Opt. Express 2018, 26, 4411–4420. [Google Scholar] [CrossRef] [PubMed]
- Chang, H.Y.; Chang, Y.C.; Sheng, H.J.; Fu, M.Y.; Liu, W.F.; Kashyap, R. An Ultra-Sensitive Liquid-Level Indicator Based on an Etched Chirped-Fiber Bragg Grating. IEEE Photonics Technol. Lett. 2016, 28, 268–271. [Google Scholar] [CrossRef]
- Tosi, D.; Macchi, E.G.; Gallati, M.; Braschi, G.; Cigada, A.; Rossi, S.; Leen, G.; Lewis, E. Fiber-optic chirped FBG for distributed thermal monitoring of ex-vivo radiofrequency ablation of liver. Biomed. Opt. Express 2014, 5, 1799–1811. [Google Scholar] [CrossRef]
- Zhou, W.; Dong, X.; Ni, K.; Chan, C.; Shum, P. Temperature-insensitive accelerometer based on a strain-chirped FBG. Sens. Actuators A Phys. 2010, 157, 15–18. [Google Scholar] [CrossRef]
- He, X.; bo Liu, Z.; Wang, D.N. Wavelength-tunable, passively mode-locked fiber laser based on graphene and chirped fiber Bragg grating. Opt. Lett. 2012, 37, 2394–2396. [Google Scholar] [CrossRef]
- Li, S.; Chan, T. Electrical wavelength-tunable actively mode-locked fiber ring laser with a linearly chirped fiber Bragg grating. IEEE Photonics Technol. Lett. 1998, 10, 799–801. [Google Scholar] [CrossRef]
- Tang, Z.; Pan, S.; Zhu, D.; Guo, R.; Zhao, Y.; Pan, M.; Ben, D.; Yao, J. Tunable Optoelectronic Oscillator Based on a Polarization Modulator and a Chirped FBG. IEEE Photonics Technol. Lett. 2012, 24, 1487–1489. [Google Scholar] [CrossRef]
- Wang, C.; Yao, J. A Nonuniformly Spaced Microwave Photonic Filter Using a Spatially Discrete Chirped FBG. IEEE Photonics Technol. Lett. 2013, 25, 1889–1892. [Google Scholar] [CrossRef]
- Burla, M.; Cortés, L.R.; Li, M.; Wang, X.; Chrostowski, L.; Azaña, J. Integrated waveguide Bragg gratings for microwave photonics signal processing. Opt. Express 2013, 21, 25120–25147. [Google Scholar] [CrossRef] [PubMed]
- Kaushal, S.; Cheng, R.; Ma, M.; Mistry, A.; Burla, M.; Chrostowski, L.; Azaña, J. Optical signal processing based on silicon photonics waveguide Bragg gratings. Front. Optoelectron. 2018, 11, 163–188. [Google Scholar] [CrossRef]
- Cheng, R.; Chrostowski, L. Spectral design of silicon integrated Bragg gratings: A tutorial. J. Light. Technol. 2020, 39, 712–729. [Google Scholar] [CrossRef]
- Liu, Y.; Huang, X.; Guan, H.; Yu, Z.; Wei, Q.; Fan, Z.; Han, W.; Li, Z. C-band four-channel CWDM (de-) multiplexers on a thin film lithium niobate–silicon rich nitride hybrid platform. Opt. Lett. 2021, 46, 4726–4729. [Google Scholar] [CrossRef] [PubMed]
- Strain, M.J.; Sorel, M. Design and fabrication of integrated chirped Bragg gratings for on-chip dispersion control. IEEE J. Quantum Electron. 2010, 46, 774–782. [Google Scholar] [CrossRef]
- Giuntoni, I.; Stolarek, D.; Bruns, J.; Zimmermann, L.; Tillack, B.; Petermann, K. Integrated dispersion compensator based on apodized SOI Bragg gratings. IEEE Photonics Technol. Lett. 2013, 25, 1313–1316. [Google Scholar] [CrossRef]
- Sun, H.; Wang, Y.; Chen, L.R. Integrated discretely tunable optical delay line based on step-chirped subwavelength grating waveguide Bragg gratings. J. Light. Technol. 2020, 38, 5551–5560. [Google Scholar] [CrossRef]
- Chen, L.R. Photonic generation of chirped microwave and millimeter wave pulses based on optical spectral shaping and wavelength-to-time mapping in silicon photonics. Opt. Commun. 2016, 373, 70–81. [Google Scholar] [CrossRef]
- Yu, M.; Reimer, C.; Barton, D.; Kharel, P.; Cheng, R.; He, L.; Shao, L.; Zhu, D.; Hu, Y.; Grant, H.R.; et al. Femtosecond pulse generation via an integrated electro-optic time lens. arXiv 2021. [Google Scholar] [CrossRef]
- Du, Z.; Xiang, C.; Fu, T.; Chen, M.; Yang, S.; Bowers, J.E.; Chen, H. Silicon nitride chirped spiral Bragg grating with large group delay. APL Photonics 2020, 5, 101302. [Google Scholar] [CrossRef]
- Chen, Z.; Flueckiger, J.; Wang, X.; Zhang, F.; Yun, H.; Lu, Z.; Caverley, M.; Wang, Y.; Jaeger, N.A.; Chrostowski, L. Spiral Bragg grating waveguides for TM mode silicon photonics. Opt. Express 2015, 23, 25295–25307. [Google Scholar] [CrossRef] [PubMed]
- Sun, Y.; Wang, D.; Deng, C.; Lu, M.; Huang, L.; Hu, G.; Yun, B.; Zhang, R.; Li, M.; Dong, J.; et al. Large Group Delay in Silicon-on-Insulator Chirped Spiral Bragg Grating Waveguide. IEEE Photonics J. 2021, 13, 5500205. [Google Scholar] [CrossRef]
- Ma, M.; Chen, Z.; Yun, H.; Wang, Y.; Wang, X.; Jaeger, N.A.; Chrostowski, L. Apodized spiral Bragg grating waveguides in silicon-on-insulator. IEEE Photonics Technol. Lett. 2017, 30, 111–114. [Google Scholar] [CrossRef]
- Giuntoni, I.; Stolarek, D.; Kroushkov, D.I.; Bruns, J.; Zimmermann, L.; Tillack, B.; Petermann, K. Continuously tunable delay line based on SOI tapered Bragg gratings. Opt. Express 2012, 20, 11241–11246. [Google Scholar] [CrossRef]
- Giuntoni, I.; Stolarek, D.; Gajda, A.; Winzer, G.; Bruns, J.; Tillack, B.; Petermann, K.; Zimmermann, L. Integrated drop-filter for dispersion compensation based on SOI rib waveguides. In Proceedings of the Optical Fiber Communication Conference 2010, San Diego, CA, USA, 21–25 March 2010; p. OThJ5. [Google Scholar] [CrossRef]
- Shi, W.; Wang, X.; Lin, C.; Yun, H.; Liu, Y.; Baehr-Jones, T.; Hochberg, M.; Jaeger, N.A.; Chrostowski, L. Silicon photonic grating-assisted, contra-directional couplers. Opt. Express 2013, 21, 3633–3650. [Google Scholar] [CrossRef]
- Simard, A.D.; LaRochelle, S. Complex apodized Bragg grating filters without circulators in silicon-on-insulator. Opt. Express 2015, 23, 16662–16675. [Google Scholar] [CrossRef]
- Qiu, H.; Jiang, J.; Yu, P.; Dai, T.; Yang, J.; Yu, H.; Jiang, X. Silicon band-rejection and band-pass filter based on asymmetric Bragg sidewall gratings in a multimode waveguide. Opt. Lett. 2016, 41, 2450–2453. [Google Scholar] [CrossRef]
- Xiao, R.; Shi, Y.; Li, J.; Dai, P.; Ma, C.; Chen, M.; Zhao, Y.; Chen, X. Integrated Bragg grating filter with reflection light dropped via two mode conversions. J. Light. Technol. 2019, 37, 1946–1953. [Google Scholar] [CrossRef]
- Zhu, D.; Shao, L.; Yu, M.; Cheng, R.; Desiatov, B.; Xin, C.; Hu, Y.; Holzgrafe, J.; Ghosh, S.; Shams-Ansari, A.; et al. Integrated photonics on thin-film lithium niobate. Adv. Opt. Photonics 2021, 13, 242–352. [Google Scholar] [CrossRef]
- Wang, C.; Zhang, M.; Chen, X.; Bertrand, M.; Shams-Ansari, A.; Chandrasekhar, S.; Winzer, P.; Lončar, M. Integrated lithium niobate electro-optic modulators operating at CMOS-compatible voltages. Nature 2018, 562, 101–104. [Google Scholar] [CrossRef] [PubMed]
- Huang, X.; Liu, Y.; Tu, D.; Yu, Z.; Wei, Q.; Li, Z. Linearity-Enhanced Dual-Parallel Mach–Zehnder Modulators Based on a Thin-Film Lithium Niobate Platform. Photonics 2022, 9, 197. [Google Scholar] [CrossRef]
- Escalé, M.R.; Pohl, D.; Sergeyev, A.; Grange, R. Extreme electro-optic tuning of Bragg mirrors integrated in lithium niobate nanowaveguides. Opt. Lett. 2018, 43, 1515–1518. [Google Scholar] [CrossRef] [PubMed]




| Drop Function Method | Grating Type | Operation Bandwidth (nm) | Delay Range (ps) | Grating Length (mm) | Platform | Ref. |
|---|---|---|---|---|---|---|
| Y-branch | Spiral chirped | 11.7 | 128.7 | 4 | SOI | [22] |
| Y-branch | Spiral chirped | 8.8 | 31.2 | 3 | SOI | [24] |
| Circulator | Step-chirped | 41.7 | 60 | 4.98 | SOI | [18] |
| Circulator | Spiral chirped | 9.2 | 1440 | 138 | SiN | [21] |
| Circulator | Chirped | 20 | 32 | 2.5 | LNOI | [20] |
| ADC | Chirped | 15 | 73.4 | 4.7 | LNOI | This work |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tu, D.; Huang, X.; Yin, Y.; Yu, H.; Yu, Z.; Guan, H.; Li, Z. Mode-Conversion-Based Chirped Bragg Gratings on Thin-Film Lithium Niobate. Photonics 2022, 9, 828. https://doi.org/10.3390/photonics9110828
Tu D, Huang X, Yin Y, Yu H, Yu Z, Guan H, Li Z. Mode-Conversion-Based Chirped Bragg Gratings on Thin-Film Lithium Niobate. Photonics. 2022; 9(11):828. https://doi.org/10.3390/photonics9110828
Chicago/Turabian StyleTu, Donghe, Xingrui Huang, Yuxiang Yin, Hang Yu, Zhiguo Yu, Huan Guan, and Zhiyong Li. 2022. "Mode-Conversion-Based Chirped Bragg Gratings on Thin-Film Lithium Niobate" Photonics 9, no. 11: 828. https://doi.org/10.3390/photonics9110828
APA StyleTu, D., Huang, X., Yin, Y., Yu, H., Yu, Z., Guan, H., & Li, Z. (2022). Mode-Conversion-Based Chirped Bragg Gratings on Thin-Film Lithium Niobate. Photonics, 9(11), 828. https://doi.org/10.3390/photonics9110828





