Tunable Microwave Pulse Generation Based on an Actively Mode-Locked Optoelectronic Oscillator
Abstract
1. Introduction
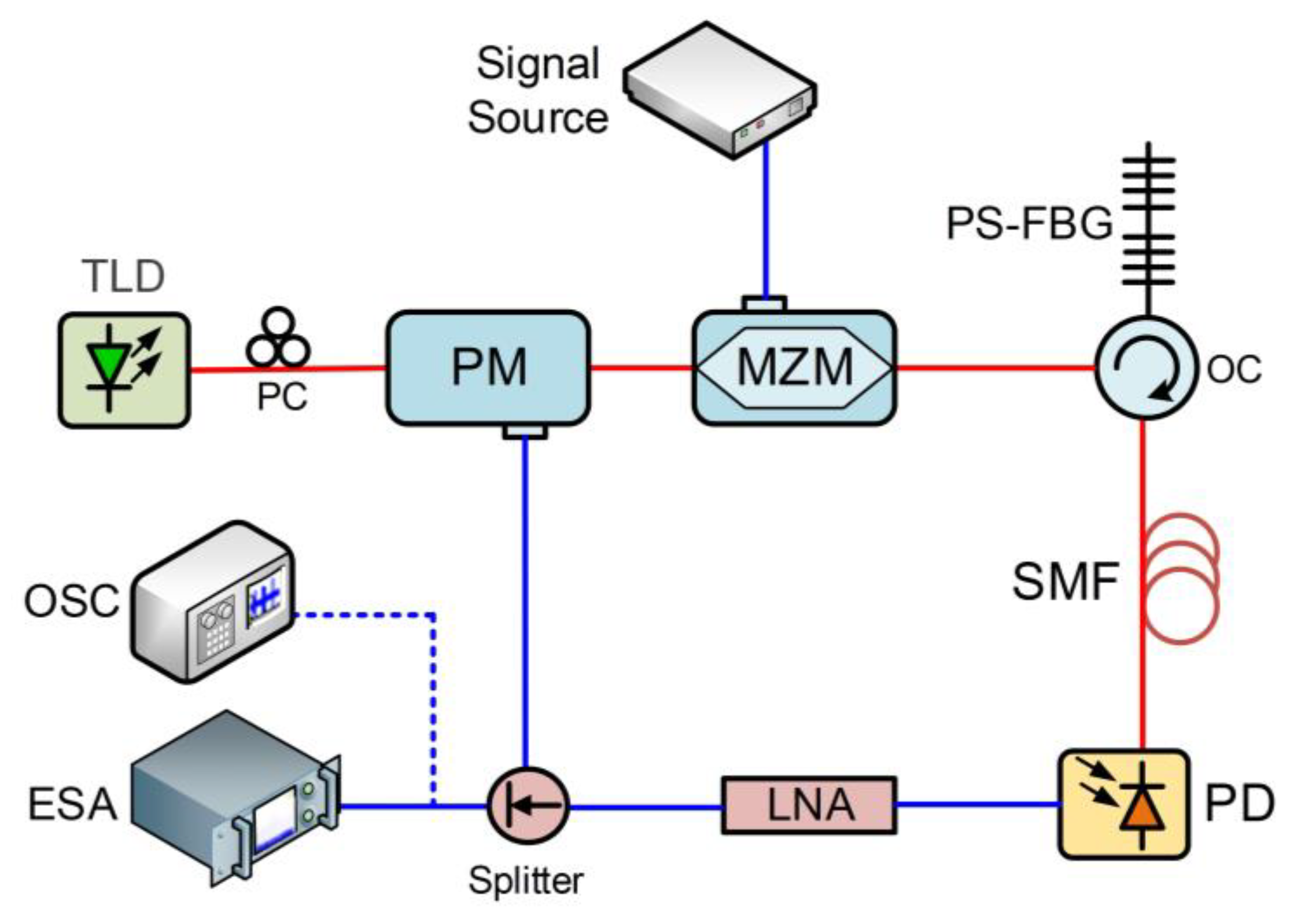
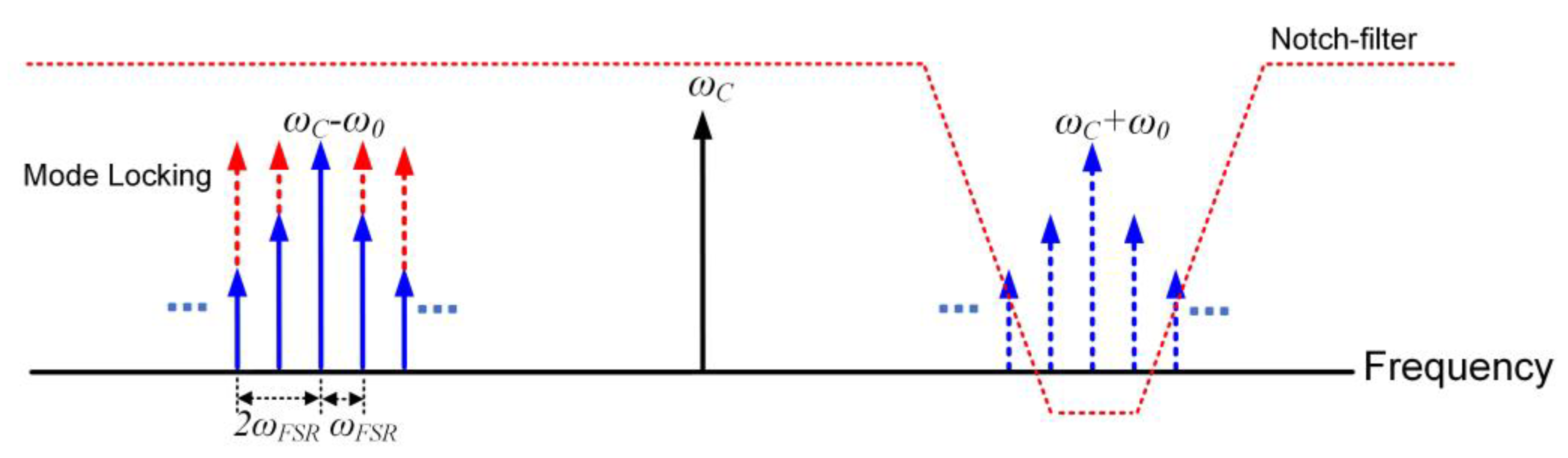
2. Theoretical Analysis
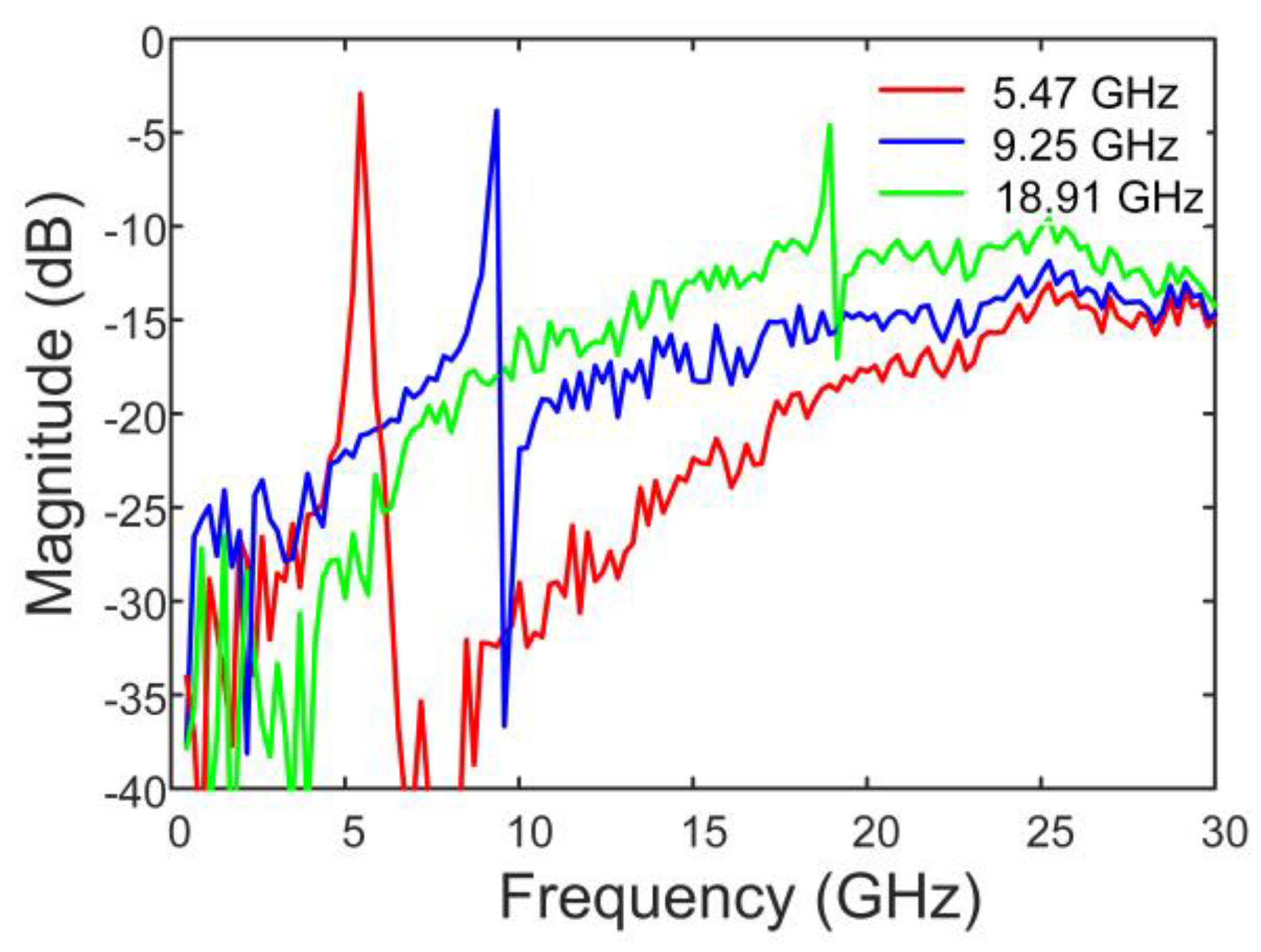
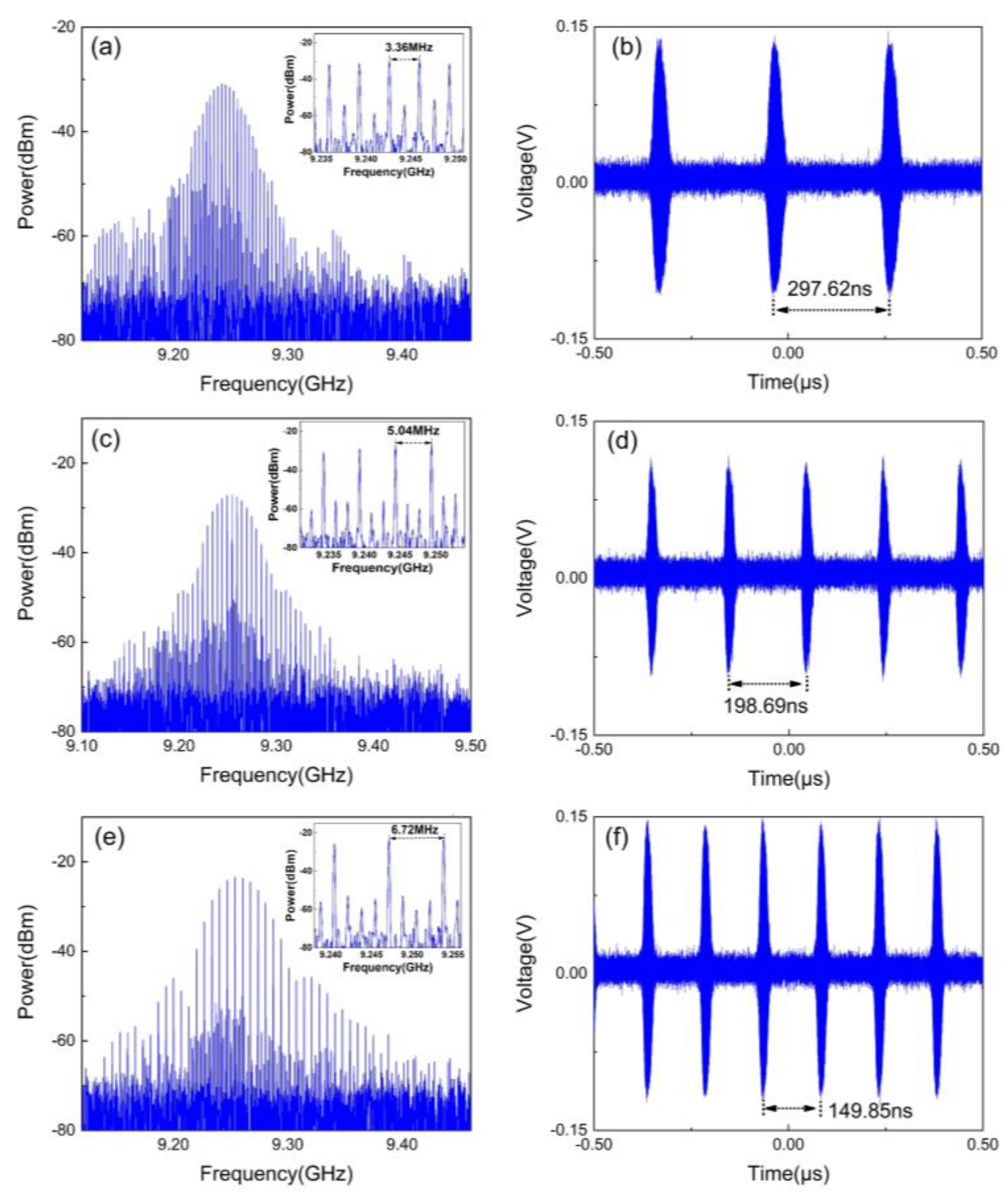
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Capmany, J.; Novak, D. Microwave photonics combines two worlds. Nat. Photon. 2007, 1, 319–330. [Google Scholar] [CrossRef]
- Yao, J. Microwave photonics. J. Lightwave Technol. 2009, 27, 314–335. [Google Scholar] [CrossRef]
- Prasad, R. Overview of wireless personal communications: Microwave perspectives. IEEE Commun. Mag. 1997, 35, 104–108. [Google Scholar] [CrossRef]
- Peng, Z.Y.; Li, C.Z. Portable Microwave Radar Systems for Short-Range Localization and Life Tracking: A Review. Sensors 2019, 19, 1136. [Google Scholar] [CrossRef] [PubMed]
- Cibiel, G.; Regis, M.; Llopis, O.; Rennane, A.; Bary, L.; Plana, R.; Kersale, Y.; Giordano, V. Optimization of an Ultra Low Phase Noise Sapphire-SiGe HBT Oscillator Using Nonlinear CAD. IEEE Trans. Ultrason. Eng. 2004, 51, 33–40. [Google Scholar]
- Gravel, J.F.; Wight, J.S. On the Conception and Analysis of a 12-GHz Push-Push Phase-Locked DRO. IEEE Trans. Microwave Theory Tech. 2006, 54, 153–159. [Google Scholar] [CrossRef]
- Madani, M.H.; Abdipour, A.; Mohammadi, A. Analysis of performance degradation due to non-linearity and phase noise in orthogonal frequency division multiplexing systems. IET Commun. 2010, 4, 1226–1237. [Google Scholar] [CrossRef]
- Porzi, C.; Falconi, F.; Sorel, M.; Ghelfi, P.; Bogoni, A. Flexible millimeter-wave carrier generation up to the Sub-THz with silicon photonics filters. J. Lightwave Technol. 2021, 39, 7689–7697. [Google Scholar] [CrossRef]
- Singh, M.; Raghuwanshi, S.K. Effect of higher order dispersion parameters on optical millimeter-wave generation using three parallel external optical modulators. J. Appl. Phys. 2015, 117, 023116. [Google Scholar] [CrossRef]
- Yao, X.S.; Maleki, L. Optoelectronic microwave oscillator. J. Opt. Soc. Amer. B 1996, 13, 1725–1735. [Google Scholar] [CrossRef]
- Bouchier, A.; Saleh, K.; Merrer, P.H.; Llopis, O.; Cibiel, G. Theoretical and experimental study of the phase noise of opto-electronic oscillators based on high quality factor optical resonators. In Proceedings of the 2010 IEEE International Frequency Control Symposium, Newport Beach, CA, USA, 1–4 June 2010; pp. 544–548. [Google Scholar]
- Eliyahu, D.; Seidel, D.; Maleki, L. Phase Noise of a High Performance OEO and an Ultra Low Noise Floor Cross-Correlation Microwave Photonic Homodyne System. In Proceedings of the 2008 IEEE International Frequency Control Symposium, Honolulu, HI, USA, 19–21 May 2008; pp. 811–814. [Google Scholar]
- Saleh, K.; Henriet, R.; Diallo, S.; Lin, G.; Martinenghi, P.; Balakireva, I.V.; Chembo, Y.K. Phase noise performance comparison between optoelectronic oscillators based on optical delay lines and whispering gallery mode resonators. Opt. Express 2014, 22, 32158–32173. [Google Scholar] [CrossRef] [PubMed]
- Li, W.; Yao, J. A wideband frequency tunable optoelectronic oscillator incorporating a tunable microwave photonic filter based on phase-modulation to intensity-modulation conversion using a phase-shifted fiber bragg grating. IEEE Trans. Microwave Theory Tech. 2012, 60, 1735–1742. [Google Scholar] [CrossRef]
- Jiang, Y.; Yu, J.-L.; Wang, Y.-T.; Zhang, L.-T.; Yang, E.-Z. An optical domain combined dual-loop optoelectronic oscillator. IEEE Photonics Technol. Lett. 2007, 19, 807–809. [Google Scholar]
- Zhou, W.; Blasche, G. Injection-locked dual opto-electronic oscillator with ultra-low phase noise and ultra-low spurious level. IEEE Trans. Microwave Theory Tech. 2005, 53, 929–933. [Google Scholar] [CrossRef]
- Banerjee, A.; de Britto, L.A.D.; Pacheco, G.M. Analysis of injection locking and pulling in single-loop optoelectronic oscillator. IEEE Trans. Microw. Theory Tech. 2019, 67, 2087–2094. [Google Scholar] [CrossRef]
- Xu, X.; Dai, J.; Dai, Y.; Yin, F.; Zhou, Y.; Li, J.; Yin, J.; Wang, Q.; Xu, K. Broadband and wide-range feedback tuning scheme for phase-locked loop stabilization of tunable optoelectronic oscillators. Opt. Lett. 2015, 40, 5858–5861. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Hou, D.; Zhao, J. Long-term frequency stabilization of an optoelectronic oscillator using phase-locked loop. J. Lightwave Technol. 2014, 32, 2408–2414. [Google Scholar] [CrossRef]
- A Saleh, K.; Lin, G.; Chembo, Y.K. Effect of Laser Coupling and Active Stabilization on the Phase Noise Performance of Optoelectronic Microwave Oscillators Based on Whispering-Gallery-Mode Resonators. IEEE Photon. J. 2014, 7, 5500111. [Google Scholar] [CrossRef]
- Brunetti, G.; Armenise, M.N.; Ciminelli, C. Chip-Scaled Ka-Band Photonic Linearly Chirped Microwave Waveform Generator. Front. Phys. 2022, 10, 785650. [Google Scholar] [CrossRef]
- Hao, T.; Cen, Q.; Dai, Y.; Tang, J.; Li, W.; Yao, J.; Zhu, N.; Li, M. Breaking the limitation of mode building time in an optoelectronic oscillator. Nat. Commun. 2018, 9, 1839. [Google Scholar] [CrossRef]
- Wang, Y.; Li, X.; Zhang, J.; Wo, J. Spurious level and phase noise improved fourier domain mode-locked optoelectronic oscillator based on a self-injection-locking technique. Opt. Express 2021, 29, 7535–7543. [Google Scholar] [CrossRef]
- Zeng, Z.; Zhang, L.; Zhang, Y.; Tian, H.; Liu, Y. Microwave pulse generation via employing an electric signal modulator to achieve time-domain mode locking in an optoelectronic oscillator. Opt. Lett. 2021, 46, 2107–2110. [Google Scholar] [CrossRef]
- Wu, Y.; Zeng, Z.; Zhang, L.; Zhang, Z.; Liu, Y. Modeling an actively mode-locked optoelectronic oscillator based on electric amplitude modulation. Opt. Express 2021, 29, 23835–23846. [Google Scholar] [CrossRef]
- Zhao, H.; Cao, Z.; Yang, S.; Zhai, Y.; Ou, J.; Chi, H. Active mode-locking optoelectronic oscillator. Opt. Express 2020, 28, 33220–33227. [Google Scholar]
- Wo, J.; Zhang, J.; Wang, Y. Actively mode-locked optoelectronic oscillator for microwave pulse generation. Opt. Laser Technol. 2022, 146, 107563. [Google Scholar] [CrossRef]
- Zeng, Z.; Zhang, Z.; Zhang, L.; Wu, Y.; Zhang, S.; Li, H.; Liu, Y. Harmonically mode-locked optoelectronic oscillator with ultra-low supermode noise. Opt. Laser Technol. 2022, 151, 413–416. [Google Scholar] [CrossRef]
- Li, Y.; Wang, M.; Zhang, J.; Mu, H.; Wang, C.; Yan, F. Supermode noise suppression with polarization-multiplexed dual-loop for active mode-locking optoelectronic oscillator. Opt. Lett. 2022, 47, 413–416. [Google Scholar] [CrossRef] [PubMed]
- Jahanbakht, S. Frequency domain analysis of optoelectronic oscillators utilizing optical and RF resonators with arbitrary transfer functions. J. Opt. Soc. Am. B 2021, 38, 2813–2822. [Google Scholar] [CrossRef]
- Zhen, Z.; Zhang, L.; Wu, Y.; Zhang, Z.; Zhang, S.; Zhang, Y.; Sun, B.; Liu, Y. Multi-format microwave signal generation based on an optoelectronic oscillator. Opt. Express 2021, 29, 30834. [Google Scholar]
- Mahafza, B.R. Radar systems analysis and design using MATLAB. Sci. Rep. 2000, 21, 18–19. [Google Scholar]



| Equipment | Parameters | Values and Units |
|---|---|---|
| TLD | Wavelength | 1549.966 nm |
| RF source | Frequency | 1.68 MHz |
| PS-FBG | Notch wavelength | 1549.892 nm |
| PM | Half-wave voltage | 4.5 V |
| MZM | Half-wave voltage | 4.5 V |
| PD | Responsivity | 0.7 A/W |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, J.; Zhang, D.; Zhang, M.; Zheng, D.; Wang, A.; Liu, X.; Hu, L.; Peng, X.; Wang, Y. Tunable Microwave Pulse Generation Based on an Actively Mode-Locked Optoelectronic Oscillator. Photonics 2022, 9, 772. https://doi.org/10.3390/photonics9100772
Zhang J, Zhang D, Zhang M, Zheng D, Wang A, Liu X, Hu L, Peng X, Wang Y. Tunable Microwave Pulse Generation Based on an Actively Mode-Locked Optoelectronic Oscillator. Photonics. 2022; 9(10):772. https://doi.org/10.3390/photonics9100772
Chicago/Turabian StyleZhang, Jin, Depei Zhang, Maolong Zhang, Daikun Zheng, Anle Wang, Xiaotong Liu, Lei Hu, Xiaoniu Peng, and Yalan Wang. 2022. "Tunable Microwave Pulse Generation Based on an Actively Mode-Locked Optoelectronic Oscillator" Photonics 9, no. 10: 772. https://doi.org/10.3390/photonics9100772
APA StyleZhang, J., Zhang, D., Zhang, M., Zheng, D., Wang, A., Liu, X., Hu, L., Peng, X., & Wang, Y. (2022). Tunable Microwave Pulse Generation Based on an Actively Mode-Locked Optoelectronic Oscillator. Photonics, 9(10), 772. https://doi.org/10.3390/photonics9100772




