Raman Scattering in a Double-Doped Single Crystal LiTaO3:Cr(0.2):Nd(0.45 wt%)
Abstract
:1. Introduction
2. Materials and Methods
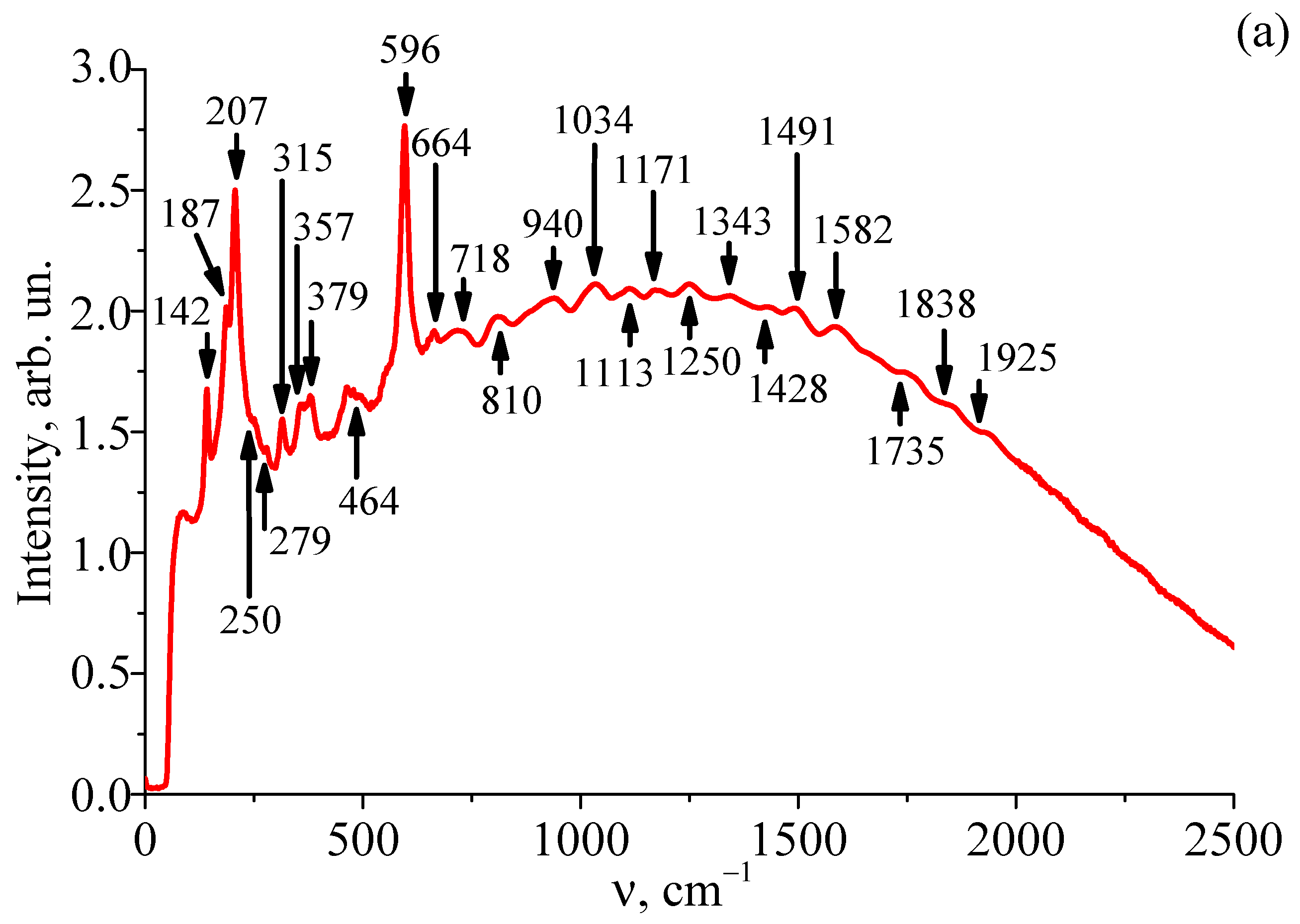
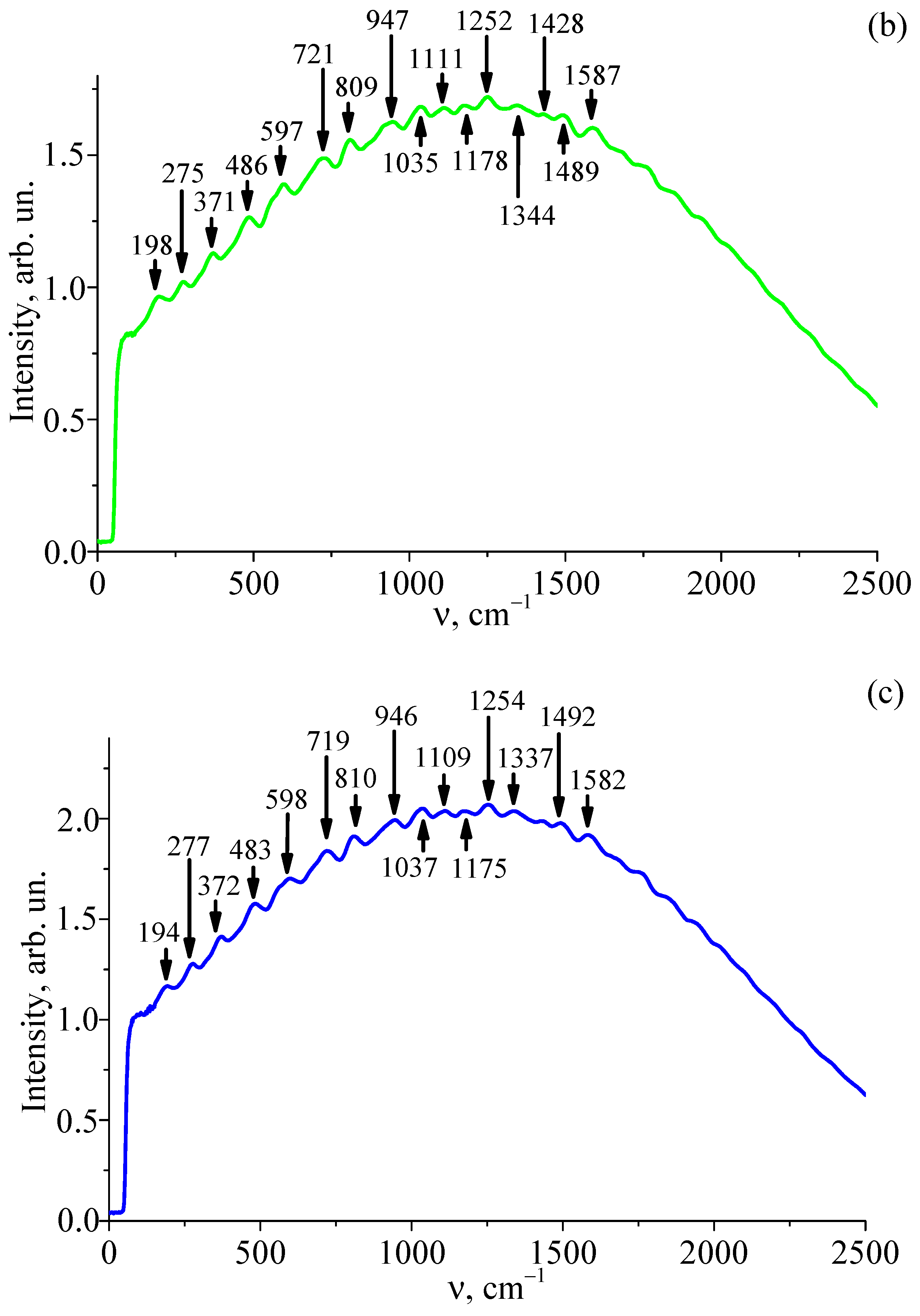
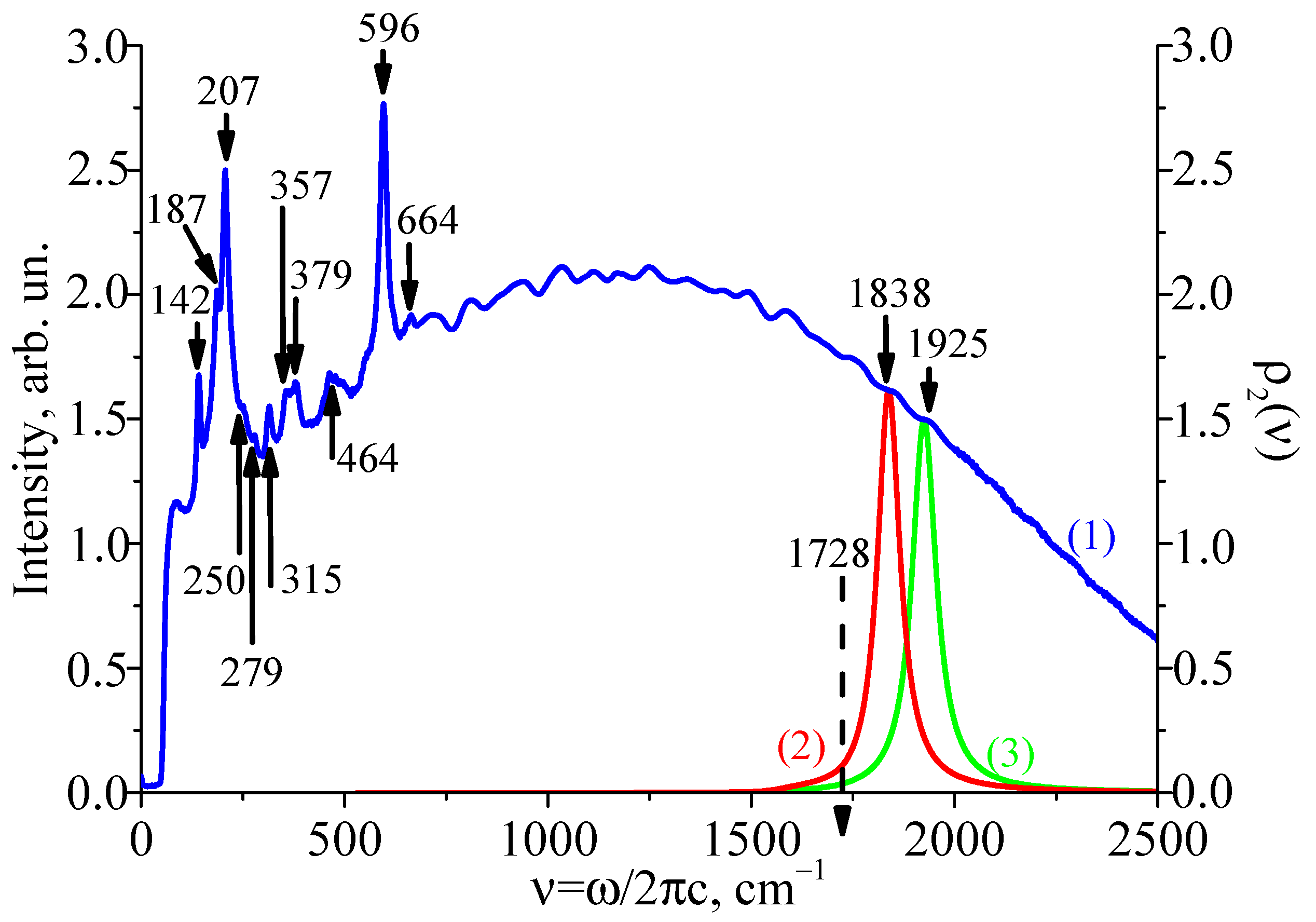
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gunter, P.; Huignard, J.P. Photorefractive Materials and Their Applications, Part 1; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Prokhorov, A.M.; Kuz’minov, Y.S. Physics and Chemistry of Crystalline Lithium Niobate; Adam Hilger: New York, NY, USA, 1990. [Google Scholar]
- Lines, M.E.; Glass, A.M. Principles and Application of Ferroelectrics and Related Materials; Clarendon Press: Oxford, UK, 1977. [Google Scholar]
- Bazzan, M.; Sada, C. Optical waveguides in lithium niobate: Recent developments and applications. Appl. Phys. Rev. 2015, 2, 040603. [Google Scholar] [CrossRef]
- Uchino, K. Advanced Piezoelectric Materials: Science and Technology, 2nd ed.; Woodhead Publishing: Oxford, UK, 2017. [Google Scholar]
- Arizmendi, L. Photonic applications of lithium niobate crystals. Phys. Status Solidi A 2004, 201, 253–283. [Google Scholar] [CrossRef]
- Abrahams, S.C.; Levinstein, H.J.; Reddy, J.M. Ferroelectric lithium niobate. 5. Polycrystal X-ray diffraction study between 24 and 1200 C. J. Phys. Chem. Solids 1966, 27, 1019–1026. [Google Scholar] [CrossRef]
- Huband, S.; Keeble, D.S.; Zhang, N.; Glazer, A.M.; Bartasyte, A.; Thomas, P.A. Relationship between the structure and optical properties of lithium tantalate at the zero-birefringence point. J. Appl. Phys. 2017, 121, 024102. [Google Scholar] [CrossRef]
- Weis, R.S.; Gaylord, T.K. Lithium niobate: Summary of physical properties and crystal structure. Appl. Phys. A 1985, 37, 191–203. [Google Scholar] [CrossRef]
- Wu, X.-L.; Yany, F.; Zhangy, M.-S.; Jiangy, S.-S.; Fengy, D. The microstructural difference between proton-exchanged LiNbO3 and LiTaO3 crystals by Raman spectroscopy. J. Phys. Condens. Matter 1996, 8, 2073–2080. [Google Scholar] [CrossRef]
- Stone, G.; Dierolf, V. Influence of ferroelectric domain walls on the Raman scattering process in lithium tantalate and niobate. Opt. Lett. 2012, 37, 1032–1034. [Google Scholar] [CrossRef]
- Kostritskii, S.M.; Aillerie, M.; Bourson, P.; Kip, D. Raman spectroscopy study of compositional inhomogeneity in lithium tantalate crystals. Appl. Phys. A 2009, 95, 125–130. [Google Scholar] [CrossRef]
- Golab, S.; Sokolska, I.; Dominiak-Dzik, G.; Palatnikov, M.N.; Sidorov, N.V.; Biryukova, I.V.; Kalinnikov, V.T.; Ryba-Romanowski, W. Optical absorption and luminescence of LiTaO3:Cr and LiTaO3:Cr,Nd crystals. Proc. SPIE 1999, 3724, 270–274. [Google Scholar] [CrossRef]
- Zhang, P.; Zhang, L.; Li, T.; Hang, Y. Passively Q-switched Tm/Mg:LiTaO3 laser using single-layer graphene as a saturable absorber. Opt. Commun. 2015, 349, 89–93. [Google Scholar] [CrossRef]
- Zhang, P.; Yin, J.; Zhang, S.; Zhang, R.; Zhang, L.; Liu, Y.; Xu, J.; Hang, Y. Crystal growth, spectroscopic characterization, and laser operation of Tm/Mg: LiTaO3 crystal. Opt. Mater. Express 2014, 4, 255–265. [Google Scholar] [CrossRef]
- Feng, T.; Li, T.; Zhao, S.; Yang, K.; Zhao, J.; Qiao, W.; Hang, Y.; Zhang, P.; Xu, J. The investigation on wavelength tunable and actively Q-switched Tm,Mg:LiTaO3 lasers. IEEE Photon Technol. Lett. 2014, 26, 1546–1548. [Google Scholar] [CrossRef]
- Feng, T.; Li, T.; Zhao, S.; Li, Q.; Yang, K.; Zhao, J.; Qiao, W.; Hang, Y.; Zhang, P.; Wang, Y.; et al. Diode-pumped continuous wave and passively Q-switched Tm, Mg: LiTaO3 lasers. Opt. Express 2014, 22, 3818–3823. [Google Scholar] [CrossRef] [PubMed]
- Gong, J.; Zhao, C.C.; Yin, J.G.; Hu, P.C.; He, X.M.; Hang, Y. Optical properties of Tm, Mg:LiTaO3 laser crystal. Laser Phys. 2011, 22, 455–460. [Google Scholar] [CrossRef]
- Chu, H.; Zhao, S.; Li, T.; Yang, K.; Li, G.; Li, D.; Zhao, J.; Qiao, W.; Xu, J.; Hang, Y. Dual-wavelength passively Q-switched Nd,Mg:LiTaO3 laser with a monolayer graphene as saturable absorber. IEEE J. Sel. Top. Quantum Electron. 2014, 21, 343–347. [Google Scholar] [CrossRef]
- Feng, T.; Li, T.; Zhao, S.; Li, Q.; Yang, K.; Zhao, J.; Qiao, W.; Hang, Y.; Zhang, P.; Wang, Y.; et al. Performance of diode-pumped continuous wave tunable and passively Q-switched Nd,Mg:LiTaO3 laser. Opt. Commun. 2014, 325, 92–94. [Google Scholar] [CrossRef]
- Wang, D.Z.; Yan, B.X.; Bi, Y.; Sun, D.H.; Sang, Y.H.; Liu, H.; Kumar, A.; Boughton, R.I. Three-wavelength green laser using intracavity frequency conversion of Nd:Mg:LiTaO3 with a MgO:PPLN crystal. Appl. Phys. A 2014, 117, 1117–1121. [Google Scholar] [CrossRef]
- Li, H.Q.; Tang, Y.L.; Zhang, R.; Yang, N.; Xu, J.Q.; Gong, J.; Hang, Y. Efficient acousto-optic Q-switched Nd,Mg:LiTaO3 lasers. Laser Phys. Lett. 2013, 10, 045809. [Google Scholar] [CrossRef]
- Sun, D.; Leng, Y.; Sang, Y.; Kang, X.; Liu, S.; Qin, X.; Cui, K.; Wan Hairul Anuar, B.K.; Liu, H.; Bi, Y. Nd:MgO:LiTaO3 crystal for self-doubling laser applications: Growth, structure, thermal and laser properties. CrystEngComm 2013, 15, 7468–7474. [Google Scholar] [CrossRef]
- Sun, D.; Liu, S.; Wang, D.; Sang, Y.; Kang, X.; Liu, H.; Bi, Y.; Yan, B.; He, J.; Wang, J. High output power of differently cut Nd:MgO:LiTaO3 CW lasers. Laser Phys. 2013, 23, 045802. [Google Scholar] [CrossRef]
- Zhang, P.; Gong, J.; Hang, Y.; Li, H.; Zhang, R.; Xu, J. A Nd:MgO:LiTaO3 crystal as a gain medium for 1083 nm lasers. Laser Phys. 2013, 23, 015803. [Google Scholar] [CrossRef]
- Zhang, P.X.; Hang, Y.; Gong, J.; Zhao, C.C.; Yin, J.G.; Zhang, L.H.; Zhu, Y.Y. Optical spectroscopy of Nd3+/Mg2+ co-doped LiTaO3 laser crystal. Laser Phys. 2012, 23, 015803. [Google Scholar] [CrossRef]
- Hu, P.; Hang, Y.; Li, R.; Gong, J.; Yin, J.; Zhao, C.; He, X.; Yu, T.; Zhang, L.; Chen, W.; et al. 1.083 μm laser operation in Nd,Mg:LiTaO3 crystal. Laser Phys. Lett. 2011, 8, 710–714. [Google Scholar] [CrossRef]
- Hsu, W.T.; Chen, Z.B.; Wu, R.C.C.; Choubey, K.; Lan, C.W. Optical properties of Mg, Fe, co-doped near-stoichiometric LiTaO3 single crystals. Materials 2012, 5, 227–238. [Google Scholar] [CrossRef]
- Hsu, W.T.; Chen, Z.B.; You, C.A.; Huang, S.W.; Liu, J.P.; Lan, C.W. Growth and photorefractive properties of Mg, Fe co-doped near-stoichiometric lithium tantalate single crystals. Opt. Mater. 2010, 32, 1071–1076. [Google Scholar] [CrossRef]
- Fang, S.; Ma, D.; Zhang, T.; Ling, F.; Wang, B. Growth and optical properties of Mg, Fe Co-doped LiTaO3 crystal. Optik 2006, 117, 72–76. [Google Scholar] [CrossRef]
- Chongjun, H.; Weili, L.; Jiming, W.; Xiaorong, G.; Tong, W.; Youwen, L. Growth and optical properties of lithium tantalate single crystals doped with indium and neodymium. Acta Opt. Sin. 2018, 38, 0116003. [Google Scholar] [CrossRef]
- Zhang, P.; Hang, Y.; Gong, J.; Zhao, C.; Yin, J.; Zhang, L. Growth, optical characterization and evaluation of laser properties of Yb3+, Mg2+:LiTaO3 crystal. J. Cryst. Growth 2013, 364, 57–61. [Google Scholar] [CrossRef]
- Liu, X. Influence of Sc ions on optical properties of Ce: LiTaO3 crystals. Optik 2013, 124, 3646–3648. [Google Scholar] [CrossRef]
- Fang, S.; Wang, B.; Zhang, T.; Ling, F.; Wang, R. Growth and photorefractive properties of Zn, Fe double-doped LiTaO3 crystal. Opt. Mater. 2006, 28, 207–211. [Google Scholar] [CrossRef]
- Ma, D.; Wang, B.; Fang, S.; Zhang, T.; Wang, R.; Wei, Y. Growth and studies of optical properties of double-doped In:Fe:LiTaO3 crystal. Microw. Opt. Technol. Lett. 2006, 48, 2227–2230. [Google Scholar] [CrossRef]
- Gang, B.H.; Rhee, B.K.; Lim, K.S.; Bae, S.H.; Joo, G.T. Growth and properties of co-doped Ce,Mn:LiTaO3 single crystals. J. Korean Ceram. Soc. 2002, 39, 711–714. [Google Scholar] [CrossRef]
- Kim, B.K.; Kang, G.Y.; Yoon, J.K.; Ro, J.H. The photorefractive effects of Fe and Fe+Ce doped LiTaO3 single crystal. J. Phys. Chem. Solids 2000, 61, 637–646. [Google Scholar] [CrossRef]
- Sokolska, I.; Ryba-Romanowski, W.; Golab, S.; Lukasiewicz, T. The optical properties of Yb3+ ions in LiTaO3:Nd, Yb crystals. Appl. Phys. A 1997, 65, 495–498. [Google Scholar] [CrossRef]
- Raptis, C. Assignment and temperature dependence of the Raman modes of LiTaO3 studied over the ferroelectric and paraelectric phases. Phys. Rev. B 1988, 38, 10007–10019. [Google Scholar] [CrossRef] [PubMed]
- Penna, A.F.; Chaves, A.; Andrade, P.D.R.; Porto, S.P. Light scattering by lithium tantalate at room temperature. Phys. Rev. B 1976, 13, 4907–4917. [Google Scholar] [CrossRef]
- Sanna, S.; Neufeld, S.; Rüsing, M.; Berth, G.; Zrenner, A.; Schmidt, W.G. Raman scattering efficiency in LiTaO3 and LiNbO3 crystals. Phys. Rev. B 2015, 91, 224302. [Google Scholar] [CrossRef]
- Rousseau, D.L.; Bauman, R.P.; Porto, S.P.S. Normal mode determination in crystals. J. Raman Spectrosc. 1981, 10, 253–290. [Google Scholar] [CrossRef]
- Margueron, S.; Bartasyte, A.; Glazer, A.M.; Simon, E.; Hlinka, J.; Gregora, I.; Gleize, J. Resolved E-symmetry zone-centre phonons in LiTaO3 and LiNbO3. J. Appl. Phys. 2012, 111, 104105. [Google Scholar] [CrossRef]
- Shi, L.; Kong, Y.; Yan, W.; Liu, H.; Li, X.; Xie, X.; Zhao, D.; Sun, L.; Xu, J.; Sun, J.; et al. The composition dependence and new assignment of the Raman spectrum in lithium tantalate. Solid State Commun. 2005, 135, 251–256. [Google Scholar] [CrossRef]
- Ruvalds, J.; Zawadowski, A. Two-phonon resonances and hybridization of the resonance with single-phonon states. Phys. Rev. B 1970, 2, 1172–1175. [Google Scholar] [CrossRef]
- Ruvalds, J.; Zawadowski, A. Indirect coupling and antiresonance of two optic phonons. Phys. Rev. Lett. 1969, 23, 1378–1381. [Google Scholar] [CrossRef]
- Ruvalds, J.; Zawadowski, A. Resonances of two phonons from different dispersion branches. Solid State Commun. 1971, 9, 129–132. [Google Scholar] [CrossRef]
- Friedrich, M.; Schindlmayr, A.; Schmidt, W.G.; Sanna, S. LiTaO3 phonon dispersion and ferroelectric transition calculated from first principles. Phys. Status Solidi B 2015, 253, 683–689. [Google Scholar] [CrossRef]

| Impurity | Concentration, wt% | |
|---|---|---|
| In Charge | In the Crystal | |
| Mn, V, Mg, Sn | <4 × 10−4 | <1 × 10−4 |
| Pb, Ni | <5 × 10−4 | <4 × 10−4 |
| Co, Mo | <3 × 10−4 | <4 × 10−4 |
| Si, Fe | <3 × 10−4 | <5 × 10−4 |
| Ti | <3 × 10−4 | <4 × 10−4 |
| Al | <8 × 10−4 | <7 × 10−4 |
| Zr | <5 × 10−4 | <1 × 10−3 |
| Ca | <4 × 10−4 | <6 × 10−4 |
| Te, Sb | <5 × 10−4 | <3 × 10−4 |
| Bi | <4 × 10−4 | <3 × 10−4 |
| Rh | <1 × 10−4 | <1 × 10−2 |
| ν, cm−1 | Attribution 1 | Attribution 2 |
|---|---|---|
| 142 | 1E(x,y)TO | 1E(x,y) |
| 187 | 1E(x,y)LO | 2E(x,y) |
| 207 | 2E(x,y)LO (1A1(z)TO) | 3E(x,y)TO |
| 250 | 3E(x,y)TO | 4E(x,y)TO |
| 279 | 3E(x,y)LO | |
| 315 | 4E(x,y)TO | 5E(x,y)TO |
| 357 | 3A1(z)TO | |
| 379 | 5E(x,y)LO | 6E(x,y)TO |
| 464 | 7E(x,y)TO | 7E(x,y)TO |
| 596 | 4A1(z)TO (8E(x,y)TO) | 8E(x,y)TO |
| 664 | 8E(x,y)LO (9E(x,y)TO) | 9E(x,y)TO |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sidorov, N.; Palatnikov, M.; Pyatyshev, A. Raman Scattering in a Double-Doped Single Crystal LiTaO3:Cr(0.2):Nd(0.45 wt%). Photonics 2022, 9, 712. https://doi.org/10.3390/photonics9100712
Sidorov N, Palatnikov M, Pyatyshev A. Raman Scattering in a Double-Doped Single Crystal LiTaO3:Cr(0.2):Nd(0.45 wt%). Photonics. 2022; 9(10):712. https://doi.org/10.3390/photonics9100712
Chicago/Turabian StyleSidorov, Nikolay, Mikhail Palatnikov, and Alexander Pyatyshev. 2022. "Raman Scattering in a Double-Doped Single Crystal LiTaO3:Cr(0.2):Nd(0.45 wt%)" Photonics 9, no. 10: 712. https://doi.org/10.3390/photonics9100712
APA StyleSidorov, N., Palatnikov, M., & Pyatyshev, A. (2022). Raman Scattering in a Double-Doped Single Crystal LiTaO3:Cr(0.2):Nd(0.45 wt%). Photonics, 9(10), 712. https://doi.org/10.3390/photonics9100712







